Open Access Library Journal
Vol.02 No.12(2015), Article ID:69022,9 pages
10.4236/oalib.1102135
Constructing a Subsequence of 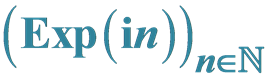 Converging towards
Converging towards 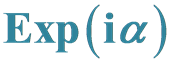 for a Given
for a Given 
Vito Lampret
University of Ljubljana, Ljubljana, Slovenia

Copyright © 2015 by author and OALib.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/


Received 13 December 2015; accepted 27 December 2015; published 31 December 2015

ABSTRACT
For a given positive irrational 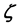 and a real
and a real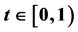 , the explicit construction of a sequence
, the explicit construction of a sequence 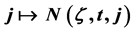 of positive integers, such that the sequence of fractional parts of products
of positive integers, such that the sequence of fractional parts of products 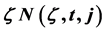 converges towards t, is given. Moreover, a constructive and quantitative demonstration of the well known fact, that the ranges of the functions cos and sin are dense in the interval
converges towards t, is given. Moreover, a constructive and quantitative demonstration of the well known fact, that the ranges of the functions cos and sin are dense in the interval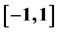 , is presented. More precisely, for any
, is presented. More precisely, for any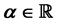 , a sequence
, a sequence 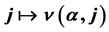 of positive integers is constructed explicitly in such a way that the estimate
of positive integers is constructed explicitly in such a way that the estimate

holds true for any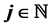 . The technique used in the paper can give more general results, e.g. by replacing sine or cosine with continuous function
. The technique used in the paper can give more general results, e.g. by replacing sine or cosine with continuous function 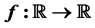 having an irrational period.
having an irrational period.
Keywords:
Convergence, Dense, Estimate, Exponential, Fractional Part, Integer Part, Irrational, Limit Point, Sequence
Subject Areas: Mathematical Analysis, Number Theory, Numerical Mathematics

1. Introduction
There are several arguments known showing that the ranges of the sequences 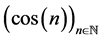 and
and 


theory, that the set 

directions in [6] , considering instead of cosine and sine, a continuous function 
period. As a corollary the authors obtained that the sets 







We offer a concrete―direct, constructive and also quantitative (computational) approach to the limit points of
the sequences 


The idea of continued fraction representation of a number suggests how to construct an algorithm producing a sequence of positive integers such that by applying the functions sin and cos we obtain two convergent sequences with prescribed limits in the interval










holds true for any
2. Preliminaries
We begin with formal definition making possible to construct the desired sequence.
1The literature usually uses for fractional part of x different notations such as for example 


Definition 2.1 For any 


As an immediate consequence of this definition we have, for any
1)
2)
Moreover, for any positive irrational number 


Indeed, considering Definition above, the numbers 





The crucial role is played by the following lemma.
Lemma 2.2. Let




there exist 





the numbers
verify the statement.
Proof. Let us suppose that

Then



Moreover,

where 

Consequently,

Now, we distinguish two cases: (A) 

(A) In this case we can set in Lemma 2.2 the integers 


(B) In this case we have the difference

Therefore, there exists an integer 

holds. Now, referring to (4), (6) and (8), we have
Hence,

where

and, according to (8),

Since



We also note that the integer 
i.e., referring to (9), we have
Thus, 





in case (B), we estimate 

Corollary 2.3. Let 


1)
2)
3)
4)
5)
6)
In this way we obtain the sequences 



i)
ii) 
iii)
iv)
Proof. For the sequences, which are given inductively, we can apply the preceding Lemma 2.2 to verify the assertions i)-iii) of the Corollary 2.3. Concerning the estimate iv), it is certainly true for 



Remark 2.4. The estimate iii) in Corollary 2.3 is rather sharp as is illustrated2 in Figure 1 where the graph of the sequence 

Remark 2.5. The estimate iv) in Corollary 2.3 seems to be rather rough as it is evident from Figure 2 showing the graph of the sequence
Remark 2.6. Given positive irrational




integer m should be chosen in such a way that the number 
ble. The Table 1 illustrates the dynamics of the sequence
Remark 2.7. The Table 2 shows, for



for 

3. Denseness
Theorem 3.1. For 



1)
2)
Figure 1. The graph of the sequence
Figure 2. The graph of the sequence
Table 1. Dynamics of the sequence
Table 2. Dynamics of the sequences 

Then the sequence 




For several


Proof. Let us take 

Therefore, according to the definition i) of 

Consequently, again thanks to Corollary 2.3 iv),
or

Now, for

or

Also, using (14),
holds for
Thus, according to (15), the fractional part of 



Theorem 3.2. The closures of the sets 







1)
2)
Then

The estimate (16) is illustrated on the Figure 5 where is plotted the graph of the sequence 

Proof. Assume that all the suppositions of Theorem 3.2 are fulfilled. Then, since



Moreover, referring to the definition of
Figure 3. The graphs of the sequences 

Figure 4. The graphs of the sequences 

Figure 5. The graph of the sequence 

That is, considering (17), we estimate

Now, according to Corollary 2.3, we have
Hence,

To conclude the proof we estimate

for

The relations (18)-(21) imply the inequalities
verifying (16).
4. Conclusions
Using only elementary tools, no use of convergents of continued fraction theory, we derived two main results about the denseness:
1) For any positive irrational 



2) We demonstrated constructively and quantitatively the well known fact that the ranges of cosine and sine are dense in the interval




In [7] is presented very nice approach to the denseness problem which is also constructive. Essential for this paper are two lemmas.
Lemma A. [Lemma 1, p. 402] Let L be any irrational number greater than 1, and suppose that




for all
Lemma B. [Lemma 2, p. 403] For each xk defined in Lemma A we can find integers mk and nk such that



Theorem. [Theorem 3, p. 404] Let 
point 


This theorem could be proved and expanded also using our technique.
Cite this paper
Vito Lampret, (2015) Constructing a Subsequence of (Exp(in))n∈N Converging towards Exp(iα) for a Given α∈R. Open Access Library Journal,02,1-9. doi: 10.4236/oalib.1102135
References
- 1. Aliprantis, C.D. and Burkinshaw, O. (1999) Problems in Real Analysis—A Workbook with Solutions. Academic Press, Inc., San Diego.
- 2. Rădulescu, T.-L.T., Rădulescu, V.D. and Andreescu, T. (2009) Problems in Real Analysis—Advanced Calculus on the Real Axis. Springer, Dodrecht, Heidelberg, New York.
http://dx.doi.org/10.1007/978-0-387-77379-7 - 3. Staib, J.H. and Demos, M.S. (1967) On the Limit Points of the Sequence {sin n }. Mathematics Magazine, 40, 210-213.
http://dx.doi.org/10.2307/2688681 - 4. Ogilvy, S.C. (1969) The Sequence {sin n } . Mathematics Magazine, 42, 94.
- 5. Luca, F. (1999) {(cos(n))n} n≥1 is dense in[-1,1] . Bulletin Mathématique de la Société des Sciences Mathématiques de Roumanie—Nouvelle Série, 42, 369-376.
- 6. Ahmadi, M.F. and Hedayatian, K. (2006) Limit Points of Trigonometric Sequences. Journal of Mathematical Extension, 1, 21-26.
- 7. Zheng, S. and Cheng, J.C. (1999) Density of the Images of Integers under Continuous Functions with Irrational Periods. Mathematics Magazine, 72, 402-404.
http://dx.doi.org/10.2307/2690800 - 8. Wolfram, S. (1988-2008) Mathematica—Version 8.0. Wolfram Research, Inc., Champaign, IL.
NOTES
2In this article all figures are produced using Mathematica [8] .





































