Open Access Library Journal
Vol.02 No.12(2015), Article ID:69008,9 pages
10.4236/oalib.1102272
Cumulative Perturbations Affecting a Spacecraft on a Mars Equatorial Orbit from the Waxing and Waning of the Polar Caps of the Planet
Jean-Pierre Barriot
Geodesy Observatory of Tahiti, University of French Polynesia, Faa’a, Tahiti

Copyright © 2015 by author and OALib.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 5 December 2015; accepted 19 December 2015; published 23 December 2015
ABSTRACT
We demonstrate in this paper that periodic variations of the J2 gravity coefficient of a planet induce small cumulative perturbations on a given family of circular equatorial orbits, and that these perturbations could be measurable with current radiosciences technology. For this purpose, we first consider a Poincaré expansion of the Newtonian equations of motion. Then, by using Floquet’s theory, we demonstrate that, unlike the excitation mechanism, the perturbations are non- periodic, and that the orbit is not “stable” in the long-term, with perturbations growing exponentially. We give the full theory and an application to the case of planet Mars.
Keywords:
Mars’ Length-of-Day, Orbit Perturbations, Floquet’s Theorem
Subject Areas: Geodesy, Geophysics

1. Rationale
Chao and Rubincam [1] demonstrated that the J2 harmonic moment of Mars is subject to large annual variation as about one quarter of the CO2 atmosphere condenses during winters at the poles, and sublimes during summers (see Figure 1), with
Figure 1. The hourglass model of the sublimation/condensation mechanism on Mars. No terrestrial phenomenon is known to produce a mass redistribution of such a magnitude over a year.
where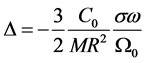 ;
; 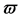 is an arbitrary phase; s is the annual length-of-day variation; C0 is the Mars
is an arbitrary phase; s is the annual length-of-day variation; C0 is the Mars
mean polar inertial moment; Ω0 is the mean Mars angular rotation velocity; ω is the mean Mars angular orbital velocity; R is the Mars radius; and M is the planet’s mass. Similar variations, but of a lesser amplitude, are also observed on the Earth. In this paper, we show that these small variations can end up in cumulative perturbations on selected families of orbits. This work can be easily extended to semi-annual perturbations and larger degree and order gravity coefficients [2] . The only restriction is that these perturbations must have commensurate periods.
2. Orbital Mechanics
With respect to a given inertial cartesian coordinate frame, the Newtonian equations of motion of a space probe orbiting a planet are
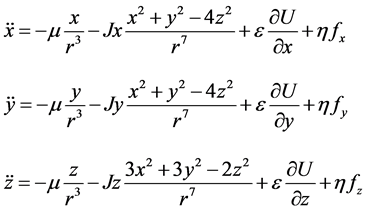
where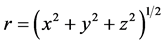 ,
, 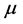 is the gravitational constant of Mars,
is the gravitational constant of Mars, 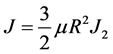 ,
,  represents the re-
represents the re-
maining part of the gravity field (without the J2 term), and the 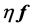 term summarizes all the other forces like atmospheric drag (the main contributor for low orbits), sun tidal acceleration, solar pressure, relativistic corrections, etc. We use the notation
term summarizes all the other forces like atmospheric drag (the main contributor for low orbits), sun tidal acceleration, solar pressure, relativistic corrections, etc. We use the notation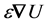 , where
, where 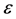 is a global scaling factor [3] , to emphasize the fact that these forces are of small amplitudes with respect to the central and J2 terms. The aims of the notation
is a global scaling factor [3] , to emphasize the fact that these forces are of small amplitudes with respect to the central and J2 terms. The aims of the notation 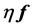 is similar.
is similar.
We now consider a probe orbiting the planet on a high altitude (i.e. with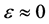 ) near equatorial orbit (i.e. with
) near equatorial orbit (i.e. with


where

It is clear that the last equation is decoupled from the first two ones. The solution of the third one corresponds to an oscillation with respect to the mean orbital plane, of no interest for the following discussion.
If we switch to cylindrical coordinates

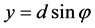

The constant h0 can be identified as an angular momentum.
Jezewski [4] [5] demonstrated that if

where










ent periods, and define an “ellipsis” with an apse line slowly rotating in the equatorial plane, with a period 








The Hamiltonian of the unperturbed motion is given by

If


where 

For the first order, after some uninteresting algebra, we arrive at





This system can be rewritten as a first order system by using the usual trick




Similar equations can be obtained from the formalisms of Hill or Lagrange. The approach that we retained is the simplest one. Considering an equatorial circular orbit is a fundamental assumption, as it allows us to write a very simple analytical solution.
3. Floquet’s Theory
System (5) is of Floquet’s type [7] , i.e. the coefficient matrix N is periodic, here with a period of half an orbit. More precisely, system (5) is a two-dimensional generalization of the Mathieu’s equation [8] .
The solution of the system with the second member w is given, as

where 

with 












4. Long-Term Behaviour of the Orbit
If 

with





This relation shows that the behavior of this system in the “long” term is governed by







5. Numerical Results and Conclusions
Let us consider the solution for the particular case of the planet Mars and for a circular equatorial orbit. The period T of a circular equatorial orbit of radius d is given from (1’) by
We take the numerical values from [15] [16] .







From these values, we derive

We now consider a circular orbit well beyond the atmosphere, at an altitude of 1000.629961 km (semi-major axis 4394.829961 km), in order to have exactly 6716 orbits/Martian year, corresponding to an orbital period of 147.266 min.
This leads to 

For the first year, the perturbations (norm of the differences between the perturbed and unperturbed motion) range up to 172.58 m in position, most of it in the along-track direction, and 122.69 mm/s in velocity. They are measurable with state-of-the-art technology, both for laser and Doppler tracking [17] , and they are slowly building up with time (see Figure 2 and Figure 3), as the spectral radius 



Figure 2. Building up of the position perturbations δr over the Martian years in meters. The building up of the perturbations is not strictly periodic (with a shift of about 14 minutes/year), albeit the excitation mechanism is by itself periodic.
Figure 3. Building up of the velocity perturbations δv over the years in mm/second.
a sufficient amount of time, from other Mars satellites, or even from the Earth, if it is equipped with “active” laser receptors [19] instead of passive retroreflectors.
We believe that this phenomenon is general, and that the theory described in this paper deserves to be generalized to any type of orbit, including polar orbits dedicated to mapping. The analysis will be then complicated by the presence of the secular perturbations caused by the even zonal coefficients of the gravity field, and other long period perturbations. The effect of the perturbations that originate from the triaxiality of Mars is investigated in Appendix B. We plan also to study semiannual variations of the J2 gravity coefficient, and to understand how the excitation mechanism described in this paper acts on the orbits of Phobos and Deimos that are near circular equatorial orbits.
Acknowledgements
This research was funded by the Centre National d’Etudes Spatiales (CNES).
Cite this paper
Jean-Pierre Barriot, (2015) Cumulative Perturbations Affecting a Spacecraft on a Mars Equatorial Orbit from the Waxing and Waning of the Polar Caps of the Planet. Open Access Library Journal,02,1-9. doi: 10.4236/oalib.1102272
References
- 1. Chao, B.F. and Rubincam, D.P. (1990) Variations of Mars Gravitational Field and Rotation Due to Seasonal CO2 Exchanges. Journal of Geophysical Research, 95, 14755-14760.
http://dx.doi.org/10.1029/JB095iB09p14755 - 2. Karatekin, O., Duron, J., Rosenblatt, P., Van Hoolst, T., Dehant, V. and Barriot, J.P. (2005) Mar’s Time-Variable Gravity and Its Determination: Simulated Geodesy Experiments. Journal of Geophysical Research—Planets, 110, E06001.
http://dx.doi.org/10.1029/2004JE002378 - 3. Mioc, V. and Stavinschi, M. (2004) Stability of Satellite Orbits around Nonspherical Planets. Artificial Satellites, 39, 129-133.
- 4. Jezewski, D.J. (1983) A Noncanonical Analytic Solution to the J2 Perturbed Two-Body Problem. Celestial Mechanics, 30, 343-361.
http://dx.doi.org/10.1007/BF01375505 - 5. Jezewski, D.J. (1983) An Analytical Solution for the J2 Perturbed Equatorial Orbit. Celestial Mechanics, 30, 363-371.
http://dx.doi.org/10.1007/BF01375506 - 6. Chazy, J. (1953) Mécanique Céleste. Presses Universitaires de France, Paris.
- 7. Dieudonné, J. (1980) Calcul Infinitésimal. Hermann Ed., Paris.
- 8. Angot, A. (1972) Compléments de Mathématiques. Masson et Cie Ed., Paris.
- 9. Walter, W. (1998) Ordinary Differential Equations. Springer, New-York.
- 10. Roseau, M. (1976) Equations différentielles. Masson, Paris.
- 11. Vijayaraghavan, A. (1984) An Analytic Solution for the Orbital Perturbations of the Venus Radar Mapper Due to Gravitational Harmonics. AIAA/AAS Astrodynamics Conference, Paper AIAA-84-1995.
- 12. Mioc, V. and Stavinschi, M. (1998) Stability of Satellite Motion in the Equatorial Plane of the Rotating Earth. Proceedings of the Journées des Systèmes de Référence Spatio-Temporels, N. Capitaine Ed., 257-261.
- 13. Mioc, V. and Stavinschi, M. (2001) Effects of Mars’ Rotation on Orbiter Dynamics. Proceedings of the Journées des Systèmes de Référence Spatio-Temporels, N. Capitaine Ed, 120-125.
- 14. Mioc, V. and Stavinschi, M. (2003) Stability of Equatorial Satellite Orbits. Proceedings of the Journées des Systèmes de Référence Spatio-Temporels, N. Capitaine Ed., 255-258.
- 15. Folkner, W.M., Yoder, C.F., Yuan, D.N., Standish, E.M. and Preston, R.A. (1997) Interior Structure and Seasonal Mass Redistribution of Mars from Radio Tracking of Mars Pathfinder. Science, 278, 1749-1752.
http://dx.doi.org/10.1126/science.278.5344.1749 - 16. Lemoine, F.G., Smith, D.E., Rowlands, D.D., Zuber, M.T., Neumann, G.A., Chinn, D.S. and Pavlis, D.E. (2001) An Improved Solution of the Gravity Field of Mars (GMM-2B) from Mars Global Surveyor. Journal of Geophysical Research, 106, 23359-23376.
http://dx.doi.org/10.1029/2000JE001426 - 17. Moyer, T.D. (2000) Formulation for Observed and Computed Values of Deep Space Network Data Types for Navigation. Monograph 2, Deep Space Communications and Navigation Series, JPL Publication 00-7.
- 18. Yoder, C.F., Williams, J.G., Dickey, J.O., Schutz, B.E., Eanes, R.J. and Tapley, B.D. (1983) Secular Variation of Earth’s Gravitational Harmonic J2 Coefficient from LAGEOS and Non-Tidal Acceleration of Earth’s Rotation. Nature, 303, 757-762.
http://dx.doi.org/10.1038/303757a0 - 19. Samain, E. (2002) One Way Laser Ranging on the Solar System: TIPO. Geophysical Research Abstracts, 4, Article ID: 05808.
- 20. Hu, W. and Scheeres, D.J. (2004) Numerical Determination of Stability Regions for Orbital Motion in Uniformly Rotating Second Degree and Order Gravity Fields. Planetary and Space Science, 52, 685-692.
http://dx.doi.org/10.1016/j.pss.2004.01.003
Appendix A
We have, with
with
Therefore, 







Let us verify that this formula defines a continuous mapping of
For 
For 
thus proving the continuity.
Appendix B
The above analysis supposes that the equatorial moments of Mars are equal. Unfortunately, because of the Tharsis uplift, Mars is the terrestrial planet for which this assumption is the least accurate. If we take into account this triaxiality, the equations of motion (1) become, in an ad’hoc reference frame and up to degree and order two [20]

where 

uation (3)), with respect to both 






















