Vol.3, No.8, 702-722 (2011) Natural Science
http://dx.doi.org/10.4236/ns.2011.38094
Growth dynamics of individual clones of normal
human keratinocytes: observations and theoretical
considerations
John Jacob Wille
Department of Cell Biology, Mayo Medical School, Rochester, USA;
Received 22 March 2011; revised 28 April 2011; accepted 3 May 2011.
ABSTRACT
The life histories of 429 individual epidermal
keratinocyte clones picked at random were
studied. Individual basal keratinocytes were
derived from asynchronous rapidly proliferating
subconfluent cultures propagated in either a
low calcium (0.1 mM) or a high calcium (2 mM)
serum-free medium. Single-celled clones were
isolated by seeding trypsin-EDTA dissociated
cells into a Petri dish containing cloning chips.
Chips with only one cell per chip were trans-
ferred into dishes containing either low calcium
or high calcium growth factor replete serum-
free medium. Clone formation was monitored
microscopically and the number of cells in each
colony tallied at least twice daily for further
analysis. A total of 369 clones were established
from seven different neonatal foreskin cell
strains (A-F), and 60 clones were derived from
one adult human skin cell strain (G). During a
five-day culture interval, among 32 clones of
strain A, 83% divided at least once, 50% divided
once in 24 hours, 86% divided at least three
times within three days, and more than 50% di-
vided at least four to five times in five days. Of
231 clones amongst the other five cell strains
(B-F), an average of 63% (±12 S,E) divided more
than three times in an eight day period, the re-
mainder divided either once, twice or not at all.
Of the 106 clones of strain G, reared in high cal-
cium serum-free medium, 67% divided more
than three times in a six-day period, and 55%
divided five or more times in 6 days. Clones
derived from adult skin strain H had a lower
clone forming potential with 70% dividing at
least once in seven days, and only 30% dividing
three or more times. By contrast, the average
generation time (AvGT) for second and third
passage keratinocytes derived from neonatal
foreskin cultures was 24 hrs. Detailed dendro-
grams were constructed for many of the prolif-
erating clones. The majority of clones ex-
pressed a synblastic division pattern with every
cell dividing at least once per day. A fraction of
clones either exceeded this circadian division
rate or displayed a biphasic division pattern
with all cells initially dividing once a day and
then abruptly slowing to once every other day
or to an intermediate rate. A minority of clones
was committed to a few terminal divisions. The
division patterns of the non-synblastic clones fit
an alternating bifurcated branching mode of
clonal expansion expressed by the Fibonacci
sequence for numbers of accumulated cells per
clone per day. These results were analyzed in
terms of deterministic, probabilistic and a limit
cycle oscillator models of cell division timing.
Keywords: Biochemical Oscillators; Clonal Growth
Dynamics; Human Keratinocytes; Interdivision
Times; Temporal Control of Mitosis Models
1. INTRODUCTION
Previously the life histories of individual keratino-
cytes were examined by the technique of time-lapse cin-
emacrography [1]. These authors reported on pedigree
analyses of only eight guinea pig keratinocyte (GPK)
cells using time-lapse filming. They recorded the gen-
eration times and position in the field of observations up
to the sixth generation in subconfluent cultures formed
from cells that were serially passaged by trypsin disso-
ciation using a split ratio (1:500) through twenty-eight
serial subcultures in a serum-containing medium. The
results indicated inter-clonal variation in growth rates.
More than half the clone displayed a broad unimodal
distributioin of GT clustered between 1200 to 1400 min-
utes; the remaining clones displayed bimodal distribu-

J. J. Wille / Natural Science 3 (2011) 702-722
703703
tions with the larger fraction clustered around 1800 to
2200 minutes. In fact, the most proliferating of the eight
cell clones only produced 22 cells after five days of cul-
ture, while the least proliferating clones produced a total
of only 7 cells. A statistically significant positive corre-
lation was demonstrated between parental generation
time and filial generation time. No evidence was pre-
sented that these were normal diploid cells. No mention
was made about the propensity of these cultures to un-
dergo commitment and terminal differentiation under the
serum culture growth conditions. Kitano et al. [2] stud-
ied cell proliferation patterns and interdivision times of
primary cultures of normal human keratinocytes in Ea-
gle’s minimal essential medium (2 mM calcium) con-
taining 20% fetal bovine serum. Cultures were filmed
between 8 - 20 days after seeding and small polygonal
groups of cells were followed up to six days. Six differ-
ent cell strains were established from donors of both
genders, ranging in age from 5 to 55 years. The average
inter-division time for the six strains was about 26 hours
and ranged from 15.1 (±4.1) for cells from a 10-year old
female to 27.6 (±13.5) for a 5-year old male. Six den-
drograms were made from the six cultures of keratino-
cytes filmed. Detailed genealogies of the clones within
each culture were presented. Most of the cells in the
field divided two to three times. Sister-sister pairs of the
second and third generations divided after approximately
the same inter-division times. Some cells never divided,
some sister-sister pairs differed substantially in their
inter-division time, and in some clones synchronous
divisions were observed. The results were interpreted as
showing that patterns of cell proliferation do not support
a statistical scheme for maintenance of a steady state, but
rather a typical pattern of logarithmic growth. In other
studies [3,4], the clone-forming ability of normal human
keratinocyte was examined by isolating individual cells
either directly from the epidermis or from cell culture.
Keratinocytes isolated directly from the epidermis and
subsequently cultured from clones only if they were <11
μm or less in diameter. The colony-forming ability of
clone-founding cells derived from cell culture was also
shown to be a function of cell size. The colony-forming
efficiency was >80% for founding cells with a cell size
below 12 μm in diameter. Interestingly, they reported
that there was no significant difference in the distribu-
tion of cell size in progeny founding cells derived from
large or small founding cells, and large clone-forming
cells were shown to give rise to colonies with cell size
distributions that contained small progeny cells. These
results were interpreted to mean that cells in various
stage of cell cycle can regenerate the average cell size
distribution if they have not left the cell cycle, while
cells that attain a cell size larger than the cell volume
predicted for G2/M cells in the cell cycle are irreversibly
committed to terminal differentiation and exit the cell
cycle. These authors also reported that the colony form-
ing capacity of individual clones is heterogenous and can
be assigned to three different classes, holoclones, para-
clones and meroclones. Holoclones are defined as hav-
ing the highest reproductive potential, paraclones have
the shortest lifespans of less than 15 cell generations,
and meroclones that are a mixture of the former two.
The incidence of these different types is affected by age
of donors such that older donors yield a lower proportion
of holoclones than younger skin donors.
In this study, observations were made of the clone
forming potential of individual keratinocyte clones iso-
lated during the rapid logarithmic growth mode of sub-
confluent cutures propagated in serum-free medium. The
growth kinetics of over four hundred different keratino-
cyte clones derived from either neonatal foreskin or
adult skin cell strains were followed daily for up to eight
days of culture. In this manner, the individual life histo-
ries of each clone initiated from among numerous ran-
domly selected cells could be compared for their clone
forming potential and for possible differences in their
patterns of cell proliferation. Preliminary results were
reported earlier [5]
2. MATERIALS & METHODS
2.1. Cell Culture
Human epidermal keratinocytes were derived either
from neonatal foreskin and adult skin as previously de-
scribed [6], using a modified MCDB 153 serum-free
medium, containing 110 mM NaCl and 20 mM HEPES,
pH 7.2. The basal medium was supplemented with
ethanolamine (0.1 mM), phophoethanolamine (0.1 mM),
hydrocortisone (0.1 μM), human recombinant EGF (5
ng/ml, and human recombinant IGF-1, both obtained
from Sigma Chemical Co., St. Louis, MO.
2.2. Isolation of Single-Celled Clones
Parent secondary cultures of keratinocytes were estab-
lished in complete serum-free growth medium and the
cultures trypsinized in mid-logarithmic mode of growth
as established by daily cell counts of parallel cultures.
The steps of enzymatic cell dissociation and harvesting
procedures were those previously described [6]. Ap-
proximately 1000 cells were seeded into sterile100mm
diameter Petri dishes containing 5 ml of pre-warmed
complete modified serum-free medium. Petri dishes
were pre-seeded with Belco (New Brunswick, NJ) clon-
ing chips. The cells were allowed to attach for 30 min-
utes and individual cloning chips with only one cell per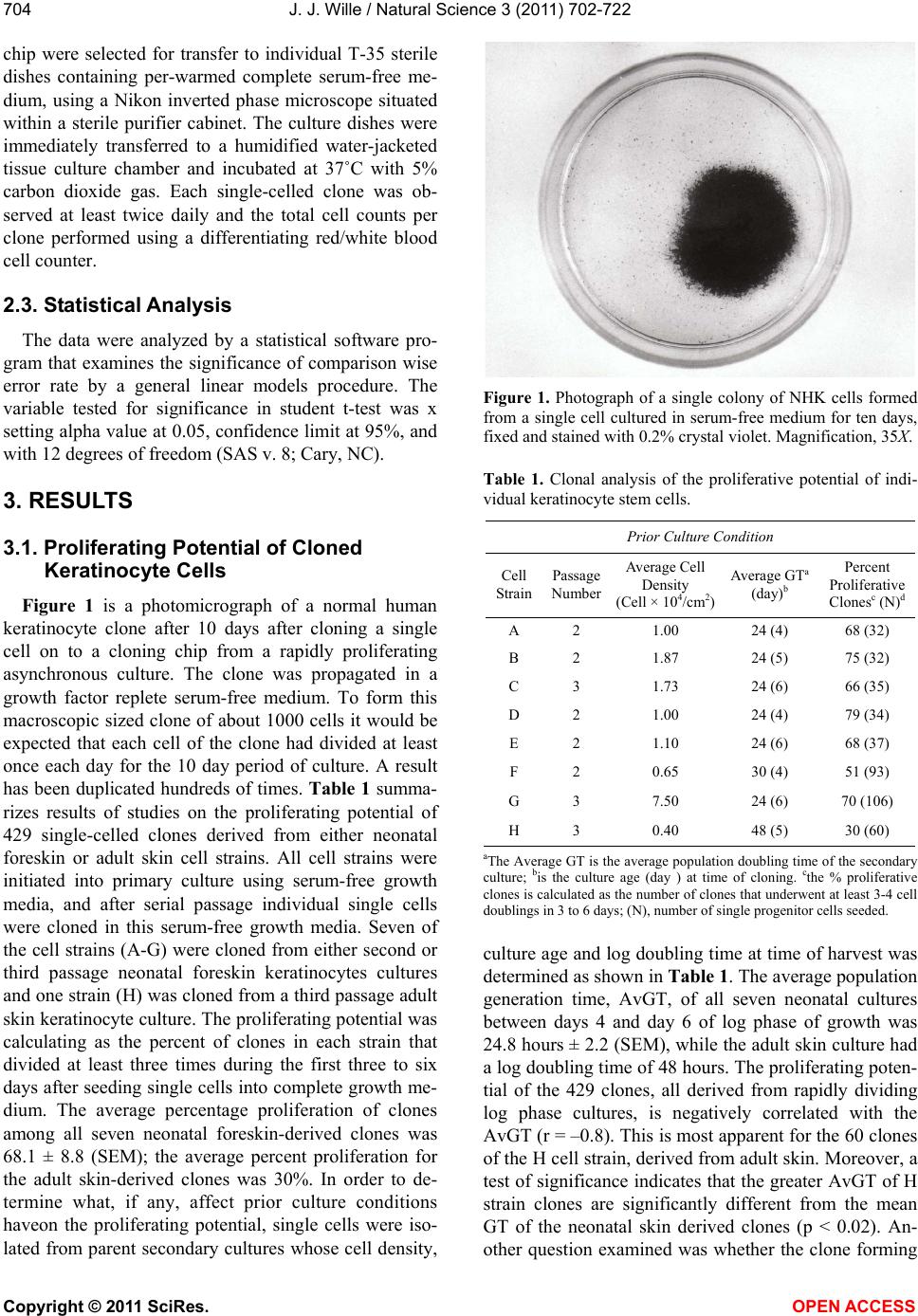

J. J. Wille / Natural Science 3 (2011) 702-722 704
chip were selected for transfer to individual T-35 sterile
dishes containing per-warmed complete serum-free me-
dium, using a Nikon inverted phase microscope situated
within a sterile purifier cabinet. The culture dishes were
immediately transferred to a humidified water-jacketed
tissue culture chamber and incubated at 37˚C with 5%
carbon dioxide gas. Each single-celled clone was ob-
served at least twice daily and the total cell counts per
clone performed using a differentiating red/white blood
cell counter.
2.3. Statistical Analysis
The data were analyzed by a statistical software pro-
gram that examines the significance of comparison wise
error rate by a general linear models procedure. The
variable tested for significance in student t-test was x
setting alpha value at 0.05, confidence limit at 95%, and
with 12 degrees of freedom (SAS v. 8; Cary, NC).
3. RESULTS
3.1. Proliferating Potential of Cloned
Keratinocyte Cells
Figure 1 is a photomicrograph of a normal human
keratinocyte clone after 10 days after cloning a single
cell on to a cloning chip from a rapidly proliferating
asynchronous culture. The clone was propagated in a
growth factor replete serum-free medium. To form this
macroscopic sized clone of about 1000 cells it would be
expected that each cell of the clone had divided at least
once each day for the 10 day period of culture. A result
has been duplicated hundreds of times. Table 1 summa-
rizes results of studies on the proliferating potential of
429 single-celled clones derived from either neonatal
foreskin or adult skin cell strains. All cell strains were
initiated into primary culture using serum-free growth
media, and after serial passage individual single cells
were cloned in this serum-free growth media. Seven of
the cell strains (A-G) were cloned from either second or
third passage neonatal foreskin keratinocytes cultures
and one strain (H) was cloned from a third passage adult
skin keratinocyte culture. The proliferating potential was
calculating as the percent of clones in each strain that
divided at least three times during the first three to six
days after seeding single cells into complete growth me-
dium. The average percentage proliferation of clones
among all seven neonatal foreskin-derived clones was
68.1 ± 8.8 (SEM); the average percent proliferation for
the adult skin-derived clones was 30%. In order to de-
termine what, if any, affect prior culture conditions
haveon the proliferating potential, single cells were iso-
lated from parent secondary cultures whose cell density,
Figure 1. Photograph of a single colony of NHK cells formed
from a single cell cultured in serum-free medium for ten days,
fixed and stained with 0.2% crystal violet. Magnification, 35X.
Table 1. Clonal analysis of the proliferative potential of indi-
vidual keratinocyte stem cells.
Prior Culture Condition
Cell
Strain
Passage
Number
Average Cell
Density
(Cell × 104/cm2)
Average GTa
(day)b
Percent
Proliferative
Clonesc (N)d
A 2 1.00 24 (4) 68 (32)
B 2 1.87 24 (5) 75 (32)
C 3 1.73 24 (6) 66 (35)
D 2 1.00 24 (4) 79 (34)
E 2 1.10 24 (6) 68 (37)
F 2 0.65 30 (4) 51 (93)
G 3 7.50 24 (6) 70 (106)
H 3 0.40 48 (5) 30 (60)
aThe Average GT is the average population doubling time of the secondary
culture; bis the culture age (day ) at time of cloning. cthe % proliferative
clones is calculated as the number of clones that underwent at least 3-4 cell
doublings in 3 to 6 days; (N), number of single progenitor cells seeded.
culture age and log doubling time at time of harvest was
determined as shown in Table 1. The average population
generation time, AvGT, of all seven neonatal cultures
between days 4 and day 6 of log phase of growth was
24.8 hours ± 2.2 (SEM), while the adult skin culture had
a log doubling time of 48 hours. The proliferating poten-
tial of the 429 clones, all derived from rapidly dividing
log phase cultures, is negatively correlated with the
AvGT (r = –0.8). This is most apparent for the 60 clones
of the H cell strain, derived from adult skin. Moreover, a
test of significance indicates that the greater AvGT of H
strain clones are significantly different from the mean
GT of the neonatal skin derived clones (p < 0.02). An-
other question examined was whether the clone forming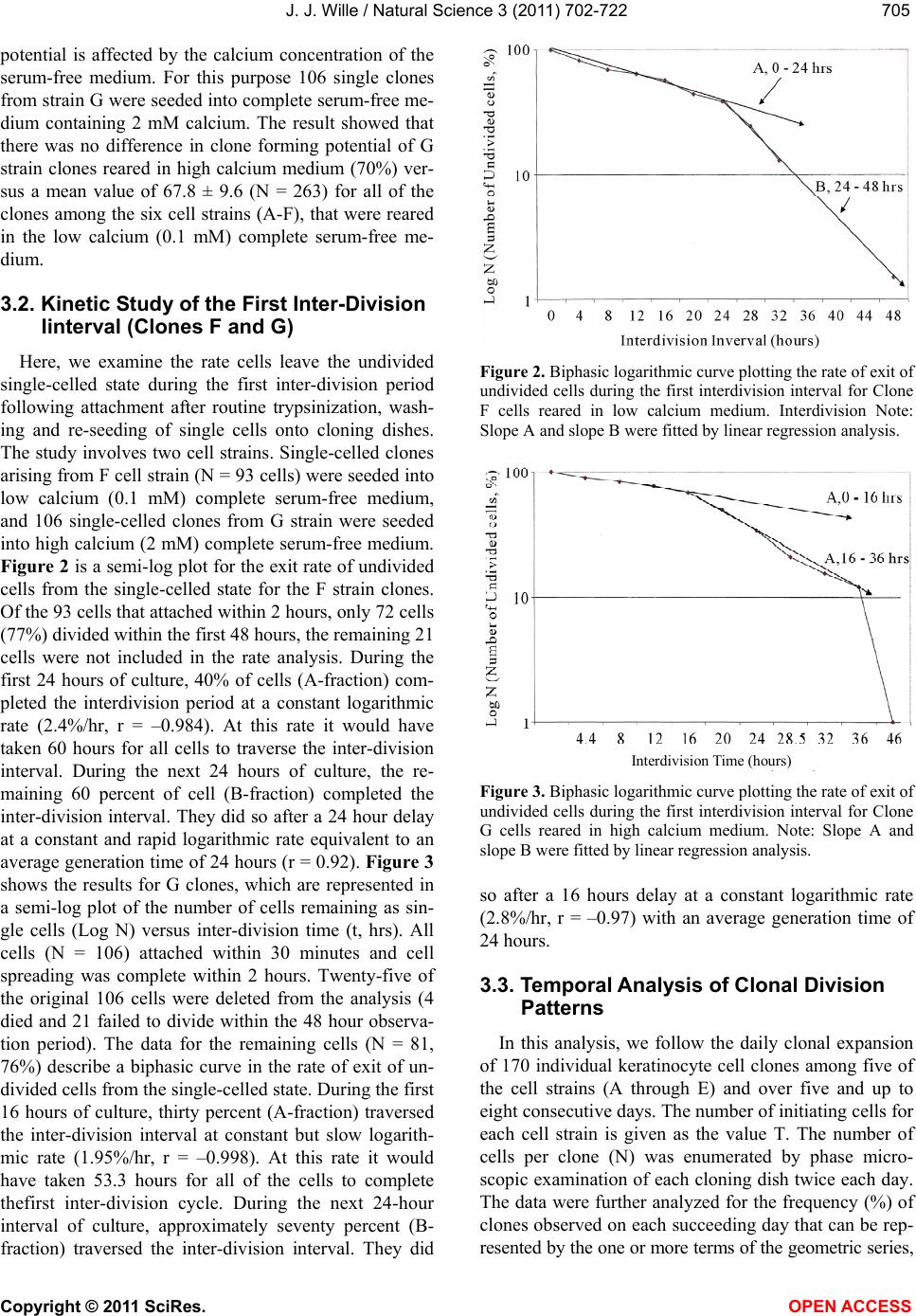

J. J. Wille / Natural Science 3 (2011) 702-722
705705
potential is affected by the calcium concentration of the
serum-free medium. For this purpose 106 single clones
from strain G were seeded into complete serum-free me-
dium containing 2 mM calcium. The result showed that
there was no difference in clone forming potential of G
strain clones reared in high calcium medium (70%) ver-
sus a mean value of 67.8 ± 9.6 (N = 263) for all of the
clones among the six cell strains (A-F), that were reared
in the low calcium (0.1 mM) complete serum-free me-
dium.
3.2. Kinetic Study of the First Inter-Division
Iinterval (Clones F and G)
Here, we examine the rate cells leave the undivided
single-celled state during the first inter-division period
following attachment after routine trypsinization, wash-
ing and re-seeding of single cells onto cloning dishes.
The study involves two cell strains. Single-celled clones
arising from F cell strain (N = 93 cells) were seeded into
low calcium (0.1 mM) complete serum-free medium,
and 106 single-celled clones from G strain were seeded
into high calcium (2 mM) complete serum-free medium.
Figure 2 is a semi-log plot for the exit rate of undivided
cells from the single-celled state for the F strain clones.
Of the 93 cells that attached within 2 hours, only 72 cells
(77%) divided within the first 48 hours, the remaining 21
cells were not included in the rate analysis. During the
first 24 hours of culture, 40% of cells (A-fraction) com-
pleted the interdivision period at a constant logarithmic
rate (2.4%/hr, r = –0.984). At this rate it would have
taken 60 hours for all cells to traverse the inter-division
interval. During the next 24 hours of culture, the re-
maining 60 percent of cell (B-fraction) completed the
inter-division interval. They did so after a 24 hour delay
at a constant and rapid logarithmic rate equivalent to an
average generation time of 24 hours (r = 0.92). Figure 3
shows the results for G clones, which are represented in
a semi-log plot of the number of cells remaining as sin-
gle cells (Log N) versus inter-division time (t, hrs). All
cells (N = 106) attached within 30 minutes and cell
spreading was complete within 2 hours. Twenty-five of
the original 106 cells were deleted from the analysis (4
died and 21 failed to divide within the 48 hour observa-
tion period). The data for the remaining cells (N = 81,
76%) describe a biphasic curve in the rate of exit of un-
divided cells from the single-celled state. During the first
16 hours of culture, thirty percent (A-fraction) traversed
the inter-division interval at constant but slow logarith-
mic rate (1.95%/hr, r = –0.998). At this rate it would
have taken 53.3 hours for all of the cells to complete
thefirst inter-division cycle. During the next 24-hour
interval of culture, approximately seventy percent (B-
fraction) traversed the inter-division interval. They did
Figure 2. Biphasic logarithmic curve plotting the rate of exit of
undivided cells during the first interdivision interval for Clone
F cells reared in low calcium medium. Interdivision Note:
Slope A and slope B were fitted by linear regression analysis.
Interdivision Time (hours)
Figure 3. Biphasic logarithmic curve plotting the rate of exit of
undivided cells during the first interdivision interval for Clone
G cells reared in high calcium medium. Note: Slope A and
slope B were fitted by linear regression analysis.
so after a 16 hours delay at a constant logarithmic rate
(2.8%/hr, r = –0.97) with an average generation time of
24 hours.
3.3. Temporal Analysis of Clonal Division
Patterns
In this analysis, we follow the daily clonal expansion
of 170 individual keratinocyte cell clones among five of
the cell strains (A through E) and over five and up to
eight consecutive days. The number of initiating cells for
each cell strain is given as the value T. The number of
cells per clone (N) was enumerated by phase micro-
scopic examination of each cloning dish twice each day.
The data were further analyzed for the frequency (%) of
clones observed on each succeeding day that can be rep-
resented by the one or more terms of the geometric series,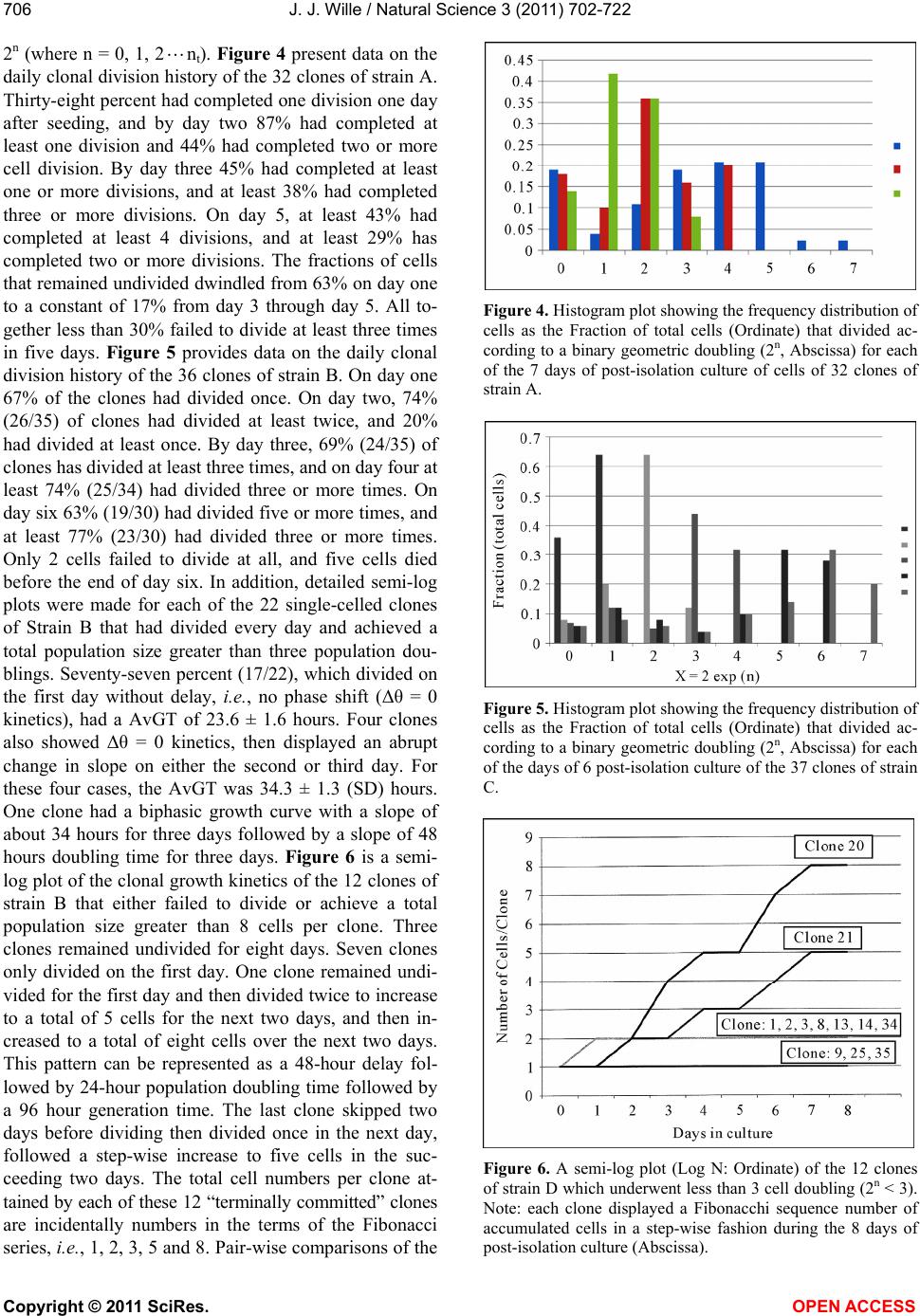

J. J. Wille / Natural Science 3 (2011) 702-722 706
2n (where n = 0, 1, 2nt). Figure 4 present data on the
daily clonal division history of the 32 clones of strain A.
Thirty-eight percent had completed one division one day
after seeding, and by day two 87% had completed at
least one division and 44% had completed two or more
cell division. By day three 45% had completed at least
one or more divisions, and at least 38% had completed
three or more divisions. On day 5, at least 43% had
completed at least 4 divisions, and at least 29% has
completed two or more divisions. The fractions of cells
that remained undivided dwindled from 63% on day one
to a constant of 17% from day 3 through day 5. All to-
gether less than 30% failed to divide at least three times
in five days. Figure 5 provides data on the daily clonal
division history of the 36 clones of strain B. On day one
67% of the clones had divided once. On day two, 74%
(26/35) of clones had divided at least twice, and 20%
had divided at least once. By day three, 69% (24/35) of
clones has divided at least three times, and on day four at
least 74% (25/34) had divided three or more times. On
day six 63% (19/30) had divided five or more times, and
at least 77% (23/30) had divided three or more times.
Only 2 cells failed to divide at all, and five cells died
before the end of day six. In addition, detailed semi-log
plots were made for each of the 22 single-celled clones
of Strain B that had divided every day and achieved a
total population size greater than three population dou-
blings. Seventy-seven percent (17/22), which divided on
the first day without delay, i.e., no phase shift (Δθ = 0
kinetics), had a AvGT of 23.6 ± 1.6 hours. Four clones
also showed Δθ = 0 kinetics, then displayed an abrupt
change in slope on either the second or third day. For
these four cases, the AvGT was 34.3 ± 1.3 (SD) hours.
One clone had a biphasic growth curve with a slope of
about 34 hours for three days followed by a slope of 48
hours doubling time for three days. Figure 6 is a semi-
log plot of the clonal growth kinetics of the 12 clones of
strain B that either failed to divide or achieve a total
population size greater than 8 cells per clone. Three
clones remained undivided for eight days. Seven clones
only divided on the first day. One clone remained undi-
vided for the first day and then divided twice to increase
to a total of 5 cells for the next two days, and then in-
creased to a total of eight cells over the next two days.
This pattern can be represented as a 48-hour delay fol-
lowed by 24-hour population doubling time followed by
a 96 hour generation time. The last clone skipped two
days before dividing then divided once in the next day,
followed a step-wise increase to five cells in the suc-
ceeding two days. The total cell numbers per clone at-
tained by each of these 12 “terminally committed” clones
are incidentally numbers in the terms of the Fibonacci
series, i. e. , 1, 2, 3, 5 and 8. Pair-wise comparisons of the
Figure 4. Histogram plot showing the frequency distribution of
cells as the Fraction of total cells (Ordinate) that divided ac-
cording to a binary geometric doubling (2n, Abscissa) for each
of the 7 days of post-isolation culture of cells of 32 clones of
strain A.
Figure 5. Histogram plot showing the frequency distribution of
cells as the Fraction of total cells (Ordinate) that divided ac-
cording to a binary geometric doubling (2n, Abscissa) for each
of the days of 6 post-isolation culture of the 37 clones of strain
C.
Figure 6. A semi-log plot (Log N: Ordinate) of the 12 clones
of strain D which underwent less than 3 cell doubling (2n < 3).
Note: each clone displayed a Fibonacchi sequence number of
accumulated cells in a step-wise fashion during the 8 days of
post-isolation culture (Abscissa).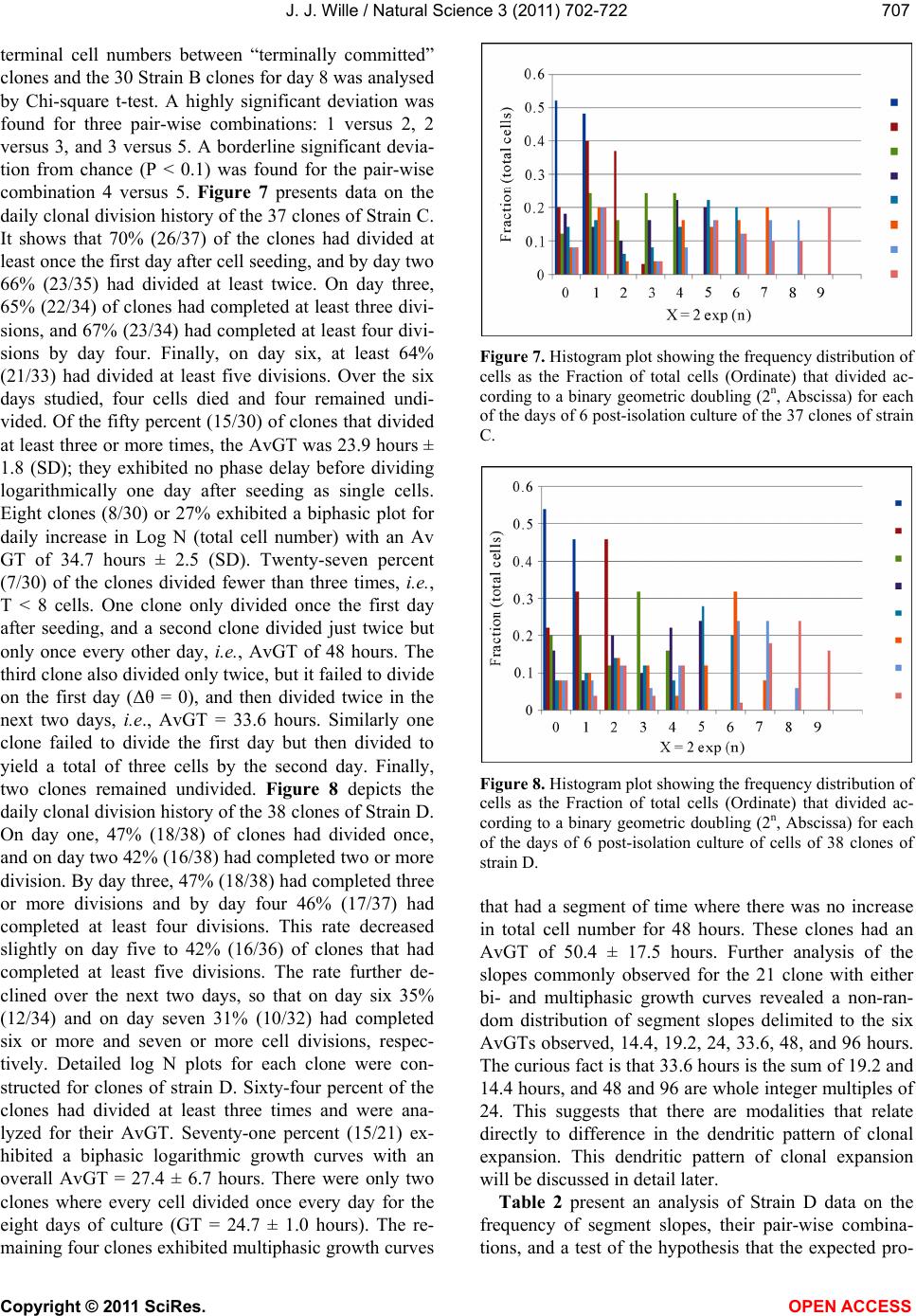

J. J. Wille / Natural Science 3 (2011) 702-722
707707
terminal cell numbers between “terminally committed”
clones and the 30 Strain B clones for day 8 was analysed
by Chi-square t-test. A highly significant deviation was
found for three pair-wise combinations: 1 versus 2, 2
versus 3, and 3 versus 5. A borderline significant devia-
tion from chance (P < 0.1) was found for the pair-wise
combination 4 versus 5. Figure 7 presents data on the
daily clonal division history of the 37 clones of Strain C.
It shows that 70% (26/37) of the clones had divided at
least once the first day after cell seeding, and by day two
66% (23/35) had divided at least twice. On day three,
65% (22/34) of clones had completed at least three divi-
sions, and 67% (23/34) had completed at least four divi-
sions by day four. Finally, on day six, at least 64%
(21/33) had divided at least five divisions. Over the six
days studied, four cells died and four remained undi-
vided. Of the fifty percent (15/30) of clones that divided
at least three or more times, the AvGT was 23.9 hours ±
1.8 (SD); they exhibited no phase delay before dividing
logarithmically one day after seeding as single cells.
Eight clones (8/30) or 27% exhibited a biphasic plot for
daily increase in Log N (total cell number) with an Av
GT of 34.7 hours ± 2.5 (SD). Twenty-seven percent
(7/30) of the clones divided fewer than three times, i.e.,
T < 8 cells. One clone only divided once the first day
after seeding, and a second clone divided just twice but
only once every other day, i.e., AvGT of 48 hours. The
third clone also divided only twice, but it failed to divide
on the first day (Δθ = 0), and then divided twice in the
next two days, i.e., AvGT = 33.6 hours. Similarly one
clone failed to divide the first day but then divided to
yield a total of three cells by the second day. Finally,
two clones remained undivided. Figure 8 depicts the
daily clonal division history of the 38 clones of Strain D.
On day one, 47% (18/38) of clones had divided once,
and on day two 42% (16/38) had completed two or more
division. By day three, 47% (18/38) had completed three
or more divisions and by day four 46% (17/37) had
completed at least four divisions. This rate decreased
slightly on day five to 42% (16/36) of clones that had
completed at least five divisions. The rate further de-
clined over the next two days, so that on day six 35%
(12/34) and on day seven 31% (10/32) had completed
six or more and seven or more cell divisions, respec-
tively. Detailed log N plots for each clone were con-
structed for clones of strain D. Sixty-four percent of the
clones had divided at least three times and were ana-
lyzed for their AvGT. Seventy-one percent (15/21) ex-
hibited a biphasic logarithmic growth curves with an
overall AvGT = 27.4 ± 6.7 hours. There were only two
clones where every cell divided once every day for the
eight days of culture (GT = 24.7 ± 1.0 hours). The re-
maining four clones exhibited multiphasic growth curves
Figure 7. Histogram plot showing the frequency distribution of
cells as the Fraction of total cells (Ordinate) that divided ac-
cording to a binary geometric doubling (2n, Abscissa) for each
of the days of 6 post-isolation culture of the 37 clones of strain
C.
Figure 8. Histogram plot showing the frequency distribution of
cells as the Fraction of total cells (Ordinate) that divided ac-
cording to a binary geometric doubling (2n, Abscissa) for each
of the days of 6 post-isolation culture of cells of 38 clones of
strain D.
that had a segment of time where there was no increase
in total cell number for 48 hours. These clones had an
AvGT of 50.4 ± 17.5 hours. Further analysis of the
slopes commonly observed for the 21 clone with either
bi- and multiphasic growth curves revealed a non-ran-
dom distribution of segment slopes delimited to the six
AvGTs observed, 14.4, 19.2, 24, 33.6, 48, and 96 hours.
The curious fact is that 33.6 hours is the sum of 19.2 and
14.4 hours, and 48 and 96 are whole integer multiples of
24. This suggests that there are modalities that relate
directly to difference in the dendritic pattern of clonal
expansion. This dendritic pattern of clonal expansion
will be discussed in detail later.
Table 2 present an analysis of Strain D data on the
frequency of segment slopes, their pair-wise combina-
tions, and a test of the hypothesis that the expected pro-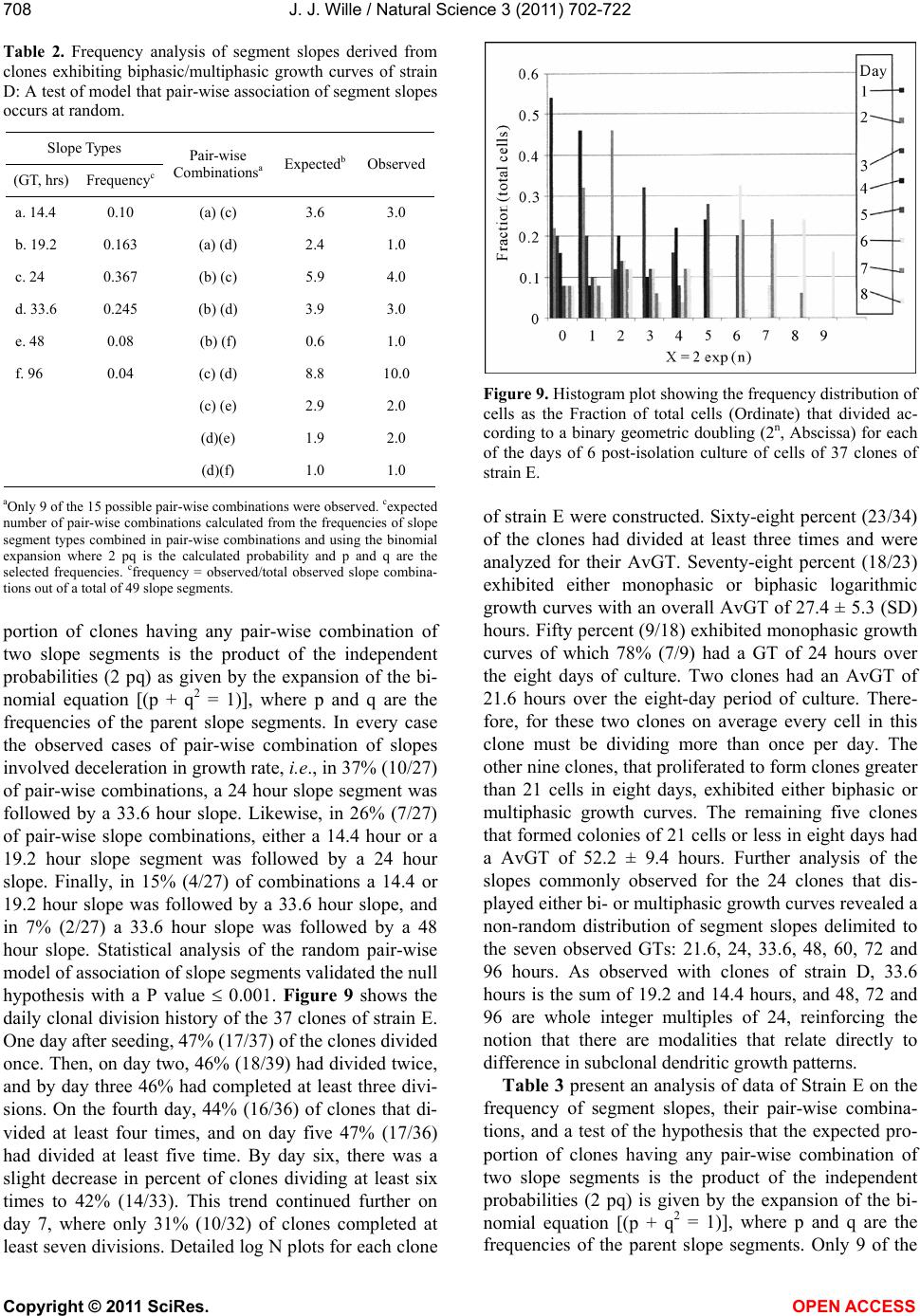

J. J. Wille / Natural Science 3 (2011) 702-722 708
Table 2. Frequency analysis of segment slopes derived from
clones exhibiting biphasic/multiphasic growth curves of strain
D: A test of model that pair-wise association of segment slopes
occurs at random.
Slope Types
(GT, hrs) Frequencyc
Pair-wise
Combinationsa Expectedb Observed
a. 14.4 0.10 (a) (c) 3.6 3.0
b. 19.2 0.163 (a) (d) 2.4 1.0
c. 24 0.367 (b) (c) 5.9 4.0
d. 33.6 0.245 (b) (d) 3.9 3.0
e. 48 0.08 (b) (f) 0.6 1.0
f. 96 0.04 (c) (d) 8.8 10.0
(c) (e) 2.9 2.0
(d)(e) 1.9 2.0
(d)(f) 1.0 1.0
aOnly 9 of the 15 possible pair-wise combinations were observed. cexpected
number of pair-wise combinations calculated from the frequencies of slope
segment types combined in pair-wise combinations and using the binomial
expansion where 2 pq is the calculated probability and p and q are the
selected frequencies. cfrequency = observed/total observed slope combina-
tions out of a total of 49 slope segments.
portion of clones having any pair-wise combination of
two slope segments is the product of the independent
probabilities (2 pq) as given by the expansion of the bi-
nomial equation [(p + q2 = 1)], where p and q are the
frequencies of the parent slope segments. In every case
the observed cases of pair-wise combination of slopes
involved deceleration in growth rate, i.e., in 37% (10/27)
of pair-wise combinations, a 24 hour slope segment was
followed by a 33.6 hour slope. Likewise, in 26% (7/27)
of pair-wise slope combinations, either a 14.4 hour or a
19.2 hour slope segment was followed by a 24 hour
slope. Finally, in 15% (4/27) of combinations a 14.4 or
19.2 hour slope was followed by a 33.6 hour slope, and
in 7% (2/27) a 33.6 hour slope was followed by a 48
hour slope. Statistical analysis of the random pair-wise
model of association of slope segments validated the null
hypothesis with a P value  0.001. Figure 9 shows the
daily clonal division history of the 37 clones of strain E.
One day after seeding, 47% (17/37) of the clones divided
once. Then, on day two, 46% (18/39) had divided twice,
and by day three 46% had completed at least three divi-
sions. On the fourth day, 44% (16/36) of clones that di-
vided at least four times, and on day five 47% (17/36)
had divided at least five time. By day six, there was a
slight decrease in percent of clones dividing at least six
times to 42% (14/33). This trend continued further on
day 7, where only 31% (10/32) of clones completed at
least seven divisions. Detailed log N plots for each clone
Figure 9. Histogram plot showing the frequency distribution of
cells as the Fraction of total cells (Ordinate) that divided ac-
cording to a binary geometric doubling (2n, Abscissa) for each
of the days of 6 post-isolation culture of cells of 37 clones of
strain E.
of strain E were constructed. Sixty-eight percent (23/34)
of the clones had divided at least three times and were
analyzed for their AvGT. Seventy-eight percent (18/23)
exhibited either monophasic or biphasic logarithmic
growth curves with an overall AvGT of 27.4 ± 5.3 (SD)
hours. Fifty percent (9/18) exhibited monophasic growth
curves of which 78% (7/9) had a GT of 24 hours over
the eight days of culture. Two clones had an AvGT of
21.6 hours over the eight-day period of culture. There-
fore, for these two clones on average every cell in this
clone must be dividing more than once per day. The
other nine clones, that proliferated to form clones greater
than 21 cells in eight days, exhibited either biphasic or
multiphasic growth curves. The remaining five clones
that formed colonies of 21 cells or less in eight days had
a AvGT of 52.2 ± 9.4 hours. Further analysis of the
slopes commonly observed for the 24 clones that dis-
played either bi- or multiphasic growth curves revealed a
non-random distribution of segment slopes delimited to
the seven observed GTs: 21.6, 24, 33.6, 48, 60, 72 and
96 hours. As observed with clones of strain D, 33.6
hours is the sum of 19.2 and 14.4 hours, and 48, 72 and
96 are whole integer multiples of 24, reinforcing the
notion that there are modalities that relate directly to
difference in subclonal dendritic growth patterns.
Table 3 present an analysis of data of Strain E on the
frequency of segment slopes, their pair-wise combina-
tions, and a test of the hypothesis that the expected pro-
portion of clones having any pair-wise combination of
two slope segments is the product of the independent
probabilities (2 pq) is given by the expansion of the bi-
nomial equation [(p + q2 = 1)], where p and q are the
frequencies of the parent slope segments. Only 9 of the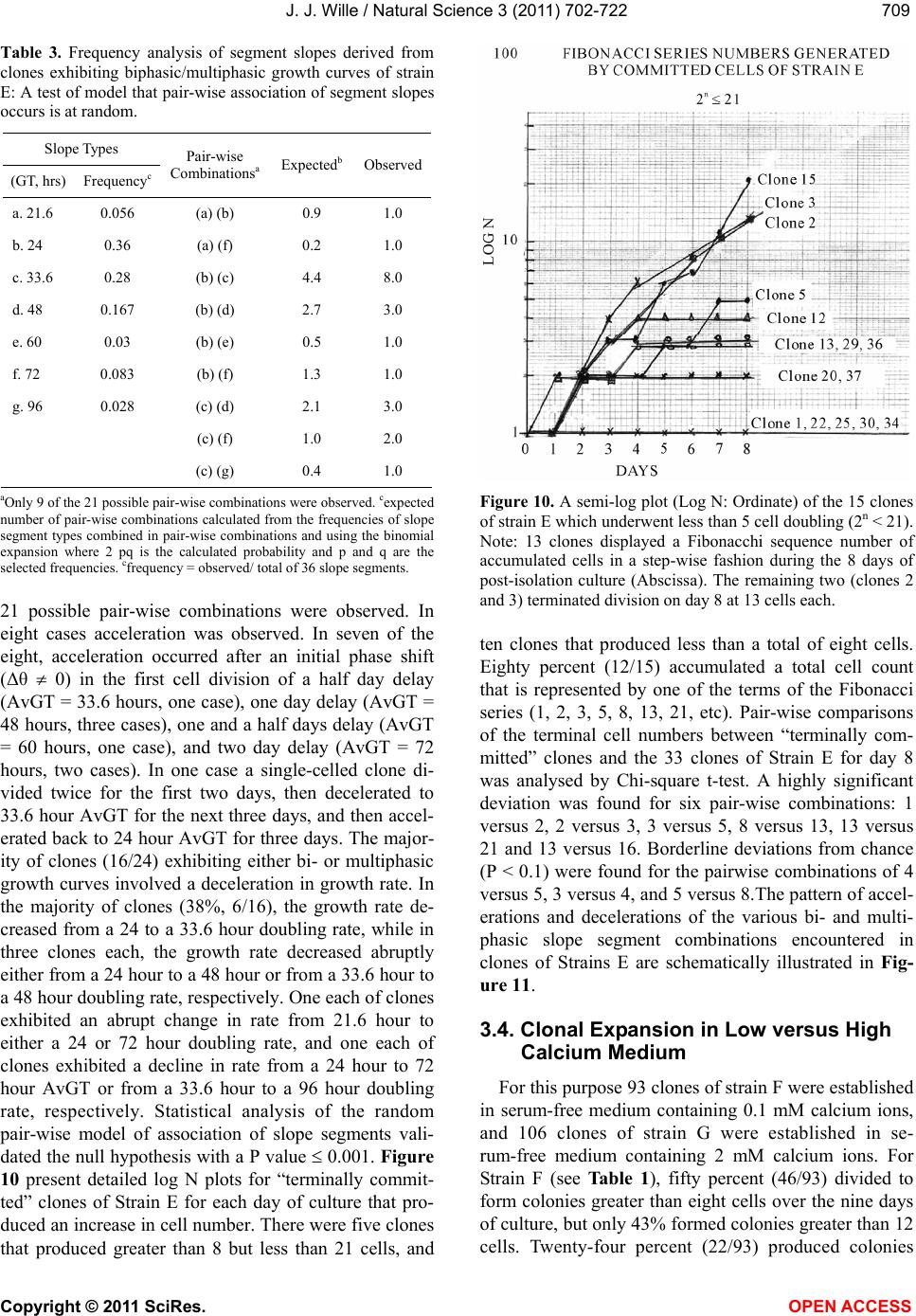

J. J. Wille / Natural Science 3 (2011) 702-722
709709
Table 3. Frequency analysis of segment slopes derived from
clones exhibiting biphasic/multiphasic growth curves of strain
E: A test of model that pair-wise association of segment slopes
occurs is at random.
Slope Types
(GT, hrs) Frequencyc
Pair-wise
Combinationsa Expectedb Observed
a. 21.6 0.056 (a) (b) 0.9 1.0
b. 24 0.36 (a) (f) 0.2 1.0
c. 33.6 0.28 (b) (c) 4.4 8.0
d. 48 0.167 (b) (d) 2.7 3.0
e. 60 0.03 (b) (e) 0.5 1.0
f. 72 0.083 (b) (f) 1.3 1.0
g. 96 0.028 (c) (d) 2.1 3.0
(c) (f) 1.0 2.0
(c) (g) 0.4 1.0
aOnly 9 of the 21 possible pair-wise combinations were observed. cexpected
number of pair-wise combinations calculated from the frequencies of slope
segment types combined in pair-wise combinations and using the binomial
expansion where 2 pq is the calculated probability and p and q are the
selected frequencies. cfrequency = observed/ total of 36 slope segments.
21 possible pair-wise combinations were observed. In
eight cases acceleration was observed. In seven of the
eight, acceleration occurred after an initial phase shift
(Δθ  0) in the first cell division of a half day delay
(AvGT = 33.6 hours, one case), one day delay (AvGT =
48 hours, three cases), one and a half days delay (AvGT
= 60 hours, one case), and two day delay (AvGT = 72
hours, two cases). In one case a single-celled clone di-
vided twice for the first two days, then decelerated to
33.6 hour AvGT for the next three days, and then accel-
erated back to 24 hour AvGT for three days. The major-
ity of clones (16/24) exhibiting either bi- or multiphasic
growth curves involved a deceleration in growth rate. In
the majority of clones (38%, 6/16), the growth rate de-
creased from a 24 to a 33.6 hour doubling rate, while in
three clones each, the growth rate decreased abruptly
either from a 24 hour to a 48 hour or from a 33.6 hour to
a 48 hour doubling rate, respectively. One each of clones
exhibited an abrupt change in rate from 21.6 hour to
either a 24 or 72 hour doubling rate, and one each of
clones exhibited a decline in rate from a 24 hour to 72
hour AvGT or from a 33.6 hour to a 96 hour doubling
rate, respectively. Statistical analysis of the random
pair-wise model of association of slope segments vali-
dated the null hypothesis with a P value  0.001. Figure
10 present detailed log N plots for “terminally commit-
ted” clones of Strain E for each day of culture that pro-
duced an increase in cell number. There were five clones
that produced greater than 8 but less than 21 cells, and
Figure 10. A semi-log plot (Log N: Ordinate) of the 15 clones
of strain E which underwent less than 5 cell doubling (2n < 21).
Note: 13 clones displayed a Fibonacchi sequence number of
accumulated cells in a step-wise fashion during the 8 days of
post-isolation culture (Abscissa). The remaining two (clones 2
and 3) terminated division on day 8 at 13 cells each.
ten clones that produced less than a total of eight cells.
Eighty percent (12/15) accumulated a total cell count
that is represented by one of the terms of the Fibonacci
series (1, 2, 3, 5, 8, 13, 21, etc). Pair-wise comparisons
of the terminal cell numbers between “terminally com-
mitted” clones and the 33 clones of Strain E for day 8
was analysed by Chi-square t-test. A highly significant
deviation was found for six pair-wise combinations: 1
versus 2, 2 versus 3, 3 versus 5, 8 versus 13, 13 versus
21 and 13 versus 16. Borderline deviations from chance
(P < 0.1) were found for the pairwise combinations of 4
versus 5, 3 versus 4, and 5 versus 8.The pattern of accel-
erations and decelerations of the various bi- and multi-
phasic slope segment combinations encountered in
clones of Strains E are schematically illustrated in Fig-
ure 11.
3.4. Clonal Expansion in Low versus High
Calcium Medium
For this purpose 93 clones of strain F were established
in serum-free medium containing 0.1 mM calcium ions,
and 106 clones of strain G were established in se-
rum-free medium containing 2 mM calcium ions. For
Strain F (see Table 1), fifty percent (46/93) divided to
form colonies greater than eight cells over the nine days
of culture, but only 43% formed colonies greater than 12
cells. Twenty-four percent (22/93) produced colonies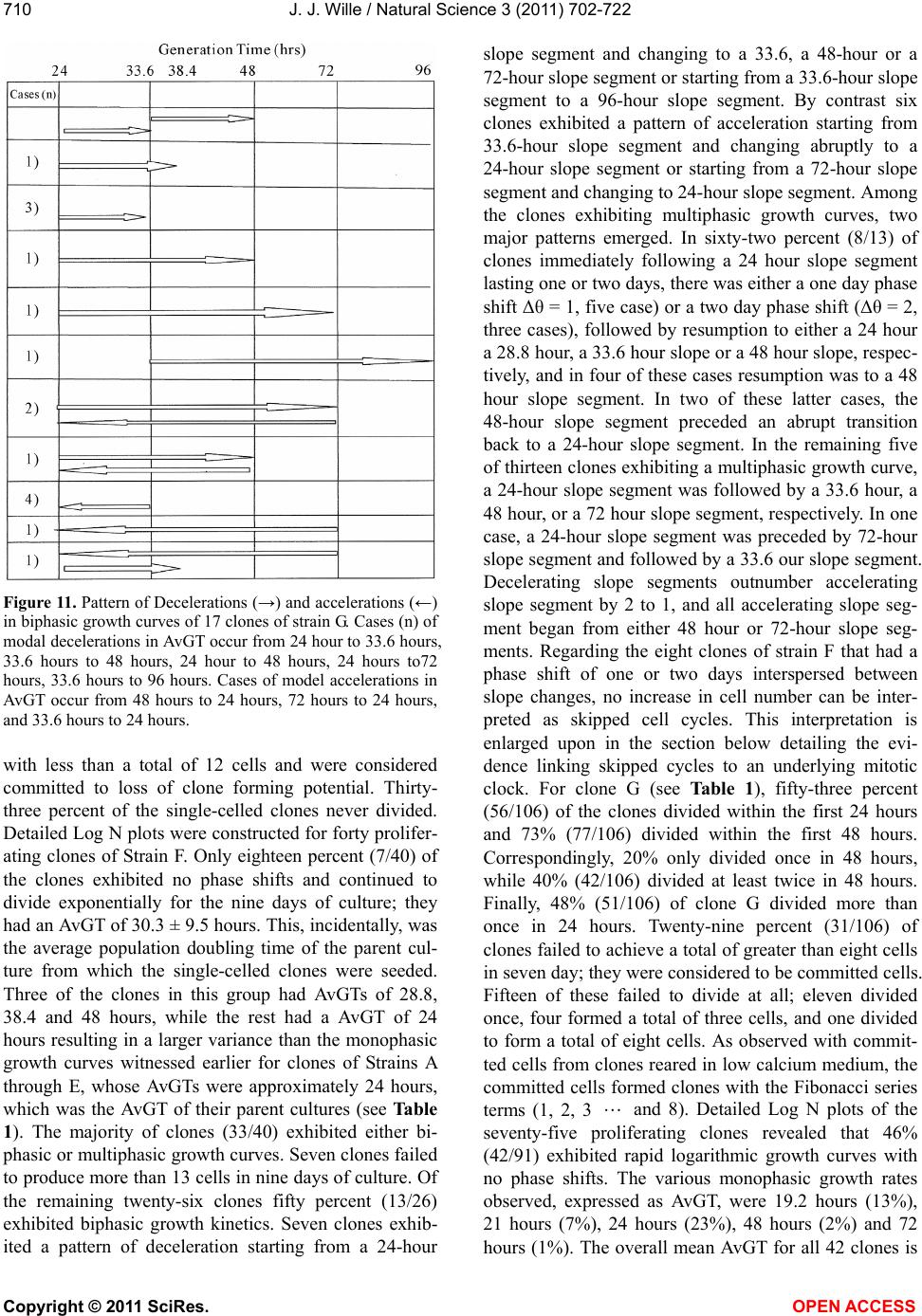

J. J. Wille / Natural Science 3 (2011) 702-722 710
Figure 11. Pattern of Decelerations (→) and accelerations (←)
in biphasic growth curves of 17 clones of strain G. Cases (n) of
modal decelerations in AvGT occur from 24 hour to 33.6 hours,
33.6 hours to 48 hours, 24 hour to 48 hours, 24 hours to72
hours, 33.6 hours to 96 hours. Cases of model accelerations in
AvGT occur from 48 hours to 24 hours, 72 hours to 24 hours,
and 33.6 hours to 24 hours.
with less than a total of 12 cells and were considered
committed to loss of clone forming potential. Thirty-
three percent of the single-celled clones never divided.
Detailed Log N plots were constructed for forty prolifer-
ating clones of Strain F. Only eighteen percent (7/40) of
the clones exhibited no phase shifts and continued to
divide exponentially for the nine days of culture; they
had an AvGT of 30.3 ± 9.5 hours. This, incidentally, was
the average population doubling time of the parent cul-
ture from which the single-celled clones were seeded.
Three of the clones in this group had AvGTs of 28.8,
38.4 and 48 hours, while the rest had a AvGT of 24
hours resulting in a larger variance than the monophasic
growth curves witnessed earlier for clones of Strains A
through E, whose AvGTs were approximately 24 hours,
which was the AvGT of their parent cultures (see Table
1). The majority of clones (33/40) exhibited either bi-
phasic or multiphasic growth curves. Seven clones failed
to produce more than 13 cells in nine days of culture. Of
the remaining twenty-six clones fifty percent (13/26)
exhibited biphasic growth kinetics. Seven clones exhib-
ited a pattern of deceleration starting from a 24-hour
slope segment and changing to a 33.6, a 48-hour or a
72-hour slope segment or starting from a 33.6-hour slope
segment to a 96-hour slope segment. By contrast six
clones exhibited a pattern of acceleration starting from
33.6-hour slope segment and changing abruptly to a
24-hour slope segment or starting from a 72-hour slope
segment and changing to 24-hour slope segment. Among
the clones exhibiting multiphasic growth curves, two
major patterns emerged. In sixty-two percent (8/13) of
clones immediately following a 24 hour slope segment
lasting one or two days, there was either a one day phase
shift Δθ = 1, five case) or a two day phase shift (Δθ = 2,
three cases), followed by resumption to either a 24 hour
a 28.8 hour, a 33.6 hour slope or a 48 hour slope, respec-
tively, and in four of these cases resumption was to a 48
hour slope segment. In two of these latter cases, the
48-hour slope segment preceded an abrupt transition
back to a 24-hour slope segment. In the remaining five
of thirteen clones exhibiting a multiphasic growth curve,
a 24-hour slope segment was followed by a 33.6 hour, a
48 hour, or a 72 hour slope segment, respectively. In one
case, a 24-hour slope segment was preceded by 72-hour
slope segment and followed by a 33.6 our slope segment.
Decelerating slope segments outnumber accelerating
slope segment by 2 to 1, and all accelerating slope seg-
ment began from either 48 hour or 72-hour slope seg-
ments. Regarding the eight clones of strain F that had a
phase shift of one or two days interspersed between
slope changes, no increase in cell number can be inter-
preted as skipped cell cycles. This interpretation is
enlarged upon in the section below detailing the evi-
dence linking skipped cycles to an underlying mitotic
clock. For clone G (see Table 1), fifty-three percent
(56/106) of the clones divided within the first 24 hours
and 73% (77/106) divided within the first 48 hours.
Correspondingly, 20% only divided once in 48 hours,
while 40% (42/106) divided at least twice in 48 hours.
Finally, 48% (51/106) of clone G divided more than
once in 24 hours. Twenty-nine percent (31/106) of
clones failed to achieve a total of greater than eight cells
in seven day; they were considered to be committed cells.
Fifteen of these failed to divide at all; eleven divided
once, four formed a total of three cells, and one divided
to form a total of eight cells. As observed with commit-
ted cells from clones reared in low calcium medium, the
committed cells formed clones with the Fibonacci series
terms (1, 2, 3  and 8). Detailed Log N plots of the
seventy-five proliferating clones revealed that 46%
(42/91) exhibited rapid logarithmic growth curves with
no phase shifts. The various monophasic growth rates
observed, expressed as AvGT, were 19.2 hours (13%),
21 hours (7%), 24 hours (23%), 48 hours (2%) and 72
hours (1%). The overall mean AvGT for all 42 clones is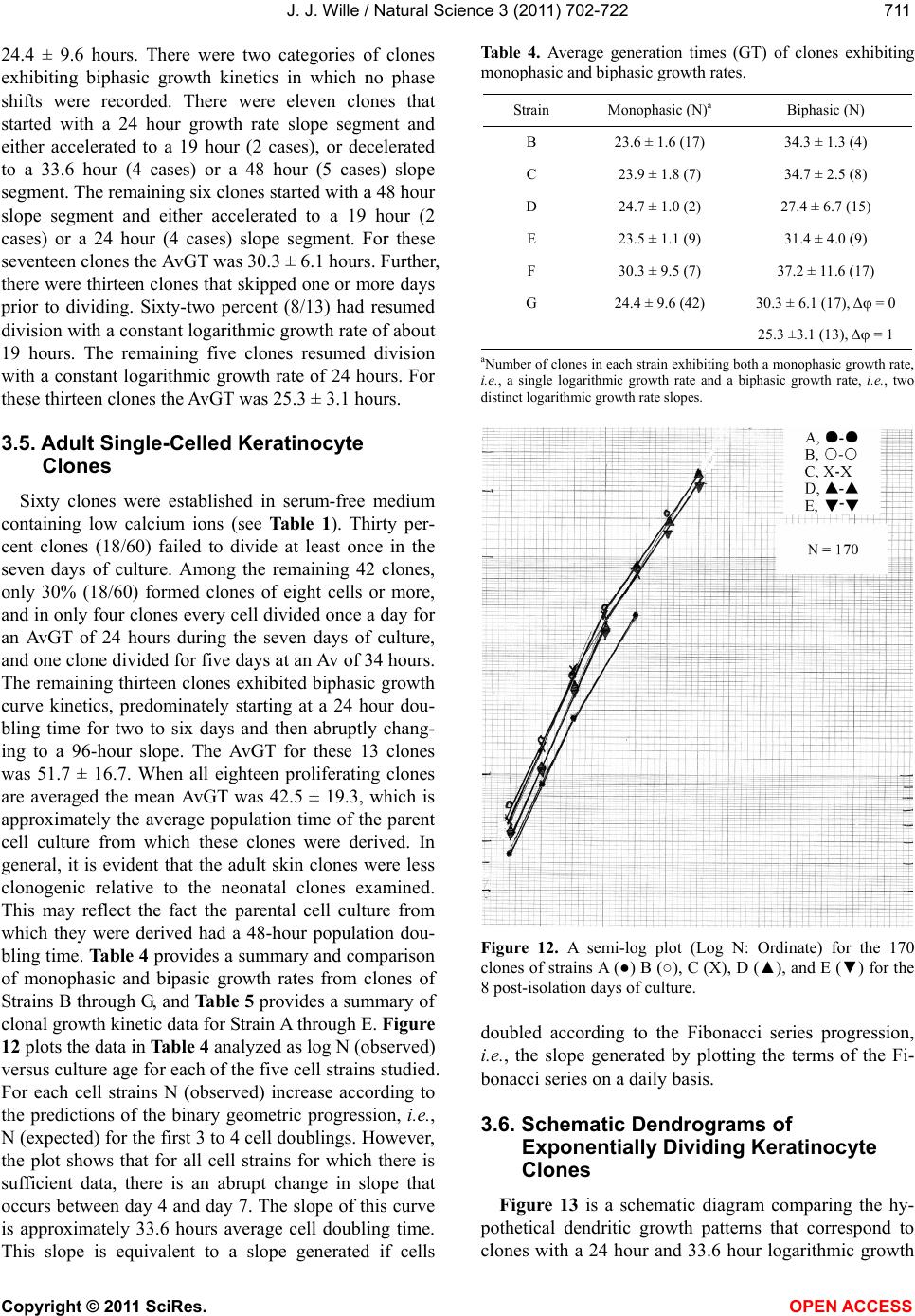

J. J. Wille / Natural Science 3 (2011) 702-722
711711
24.4 ± 9.6 hours. There were two categories of clones
exhibiting biphasic growth kinetics in which no phase
shifts were recorded. There were eleven clones that
started with a 24 hour growth rate slope segment and
either accelerated to a 19 hour (2 cases), or decelerated
to a 33.6 hour (4 cases) or a 48 hour (5 cases) slope
segment. The remaining six clones started with a 48 hour
slope segment and either accelerated to a 19 hour (2
cases) or a 24 hour (4 cases) slope segment. For these
seventeen clones the AvGT was 30.3 ± 6.1 hours. Further,
there were thirteen clones that skipped one or more days
prior to dividing. Sixty-two percent (8/13) had resumed
division with a constant logarithmic growth rate of about
19 hours. The remaining five clones resumed division
with a constant logarithmic growth rate of 24 hours. For
these thirteen clones the AvGT was 25.3 ± 3.1 hours.
3.5. Adult Single-Celled Keratinocyte
Clones
Sixty clones were established in serum-free medium
containing low calcium ions (see Table 1). Thirty per-
cent clones (18/60) failed to divide at least once in the
seven days of culture. Among the remaining 42 clones,
only 30% (18/60) formed clones of eight cells or more,
and in only four clones every cell divided once a day for
an AvGT of 24 hours during the seven days of culture,
and one clone divided for five days at an Av of 34 hours.
The remaining thirteen clones exhibited biphasic growth
curve kinetics, predominately starting at a 24 hour dou-
bling time for two to six days and then abruptly chang-
ing to a 96-hour slope. The AvGT for these 13 clones
was 51.7 ± 16.7. When all eighteen proliferating clones
are averaged the mean AvGT was 42.5 ± 19.3, which is
approximately the average population time of the parent
cell culture from which these clones were derived. In
general, it is evident that the adult skin clones were less
clonogenic relative to the neonatal clones examined.
This may reflect the fact the parental cell culture from
which they were derived had a 48-hour population dou-
bling time. Table 4 provides a summary and comparison
of monophasic and bipasic growth rates from clones of
Strains B through G, and Table 5 provides a summary of
clonal growth kinetic data for Strain A through E. Figure
12 plots the data in Table 4 analyzed as log N (observed)
versus culture age for each of the five cell strains studied.
For each cell strains N (observed) increase according to
the predictions of the binary geometric progression, i.e.,
N (expected) for the first 3 to 4 cell doublings. However,
the plot shows that for all cell strains for which there is
sufficient data, there is an abrupt change in slope that
occurs between day 4 and day 7. The slope of this curve
is approximately 33.6 hours average cell doubling time.
This slope is equivalent to a slope generated if cells
Table 4. Average generation times (GT) of clones exhibiting
monophasic and biphasic growth rates.
Strain Monophasic (N)a Biphasic (N)
B 23.6 ± 1.6 (17) 34.3 ± 1.3 (4)
C 23.9 ± 1.8 (7) 34.7 ± 2.5 (8)
D 24.7 ± 1.0 (2) 27.4 ± 6.7 (15)
E 23.5 ± 1.1 (9) 31.4 ± 4.0 (9)
F 30.3 ± 9.5 (7) 37.2 ± 11.6 (17)
G 24.4 ± 9.6 (42) 30.3 ± 6.1 (17), Δ = 0
25.3 ±3.1 (13), Δ = 1
aNumber of clones in each strain exhibiting both a monophasic growth rate,
i.e., a single logarithmic growth rate and a biphasic growth rate, i.e., two
distinct logarithmic growth rate slopes.
Figure 12. A semi-log plot (Log N: Ordinate) for the 170
clones of strains A (●) B (○), C (X), D (▲), and E (▼) for the
8 post-isolation days of culture.
doubled according to the Fibonacci series progression,
i.e., the slope generated by plotting the terms of the Fi-
bonacci series on a daily basis.
3.6. Schematic Dendrograms of
Exponentially Dividing Keratinocyte
Clones
Figure 13 is a schematic diagram comparing the hy-
pothetical dendritic growth patterns that correspond to
clones with a 24 hour and 33.6 hour logarithmic growth

J. J. Wille / Natural Science 3 (2011) 702-722 712
Table 5. Comparison of the observed to expected total cell
number per clone during daily clonal expansion.
Cell Strain (T) Observed
(Total Cell/Clone)
Expecteda
(Total No. of
Cells/Clone)
Percent
[observed/
Expected]
A
day 1(32) 44 64 38
day 2 (30) 90 120 75
day 3 (29) 187 232 80
day 5 (28) 543 896 61
B
day 1 (36) 60 72 83
day 2 (35) 136 140 97
day 3 (35) 294 280 105
day 4 (34) 518 544 95
day 6 (30) 1544 1920 80
C
day 1 (37) 71 74 96
day 2 (35) 143 140 100
day 3 (34) 282 272 104
day 4 (34) 586 544 108
day 6 (33) 1518 2112 72
D
day 1 (38) 56 76 74
day 2 (38) 108 152 71
day 3 (38) 263 304 87
day 4 (37) 483 592 82
day 5 (36) 913 1152 79
day 6 (34) 1458 2173 67
day 7 (32) 2390 4096 58
E
day 1 (37) 54 74 73
day 2 (39) 105 156 67
day 3 (38) 243 304 80
day 4 (36) 456 576 79
day 5 (36) 877 1152 76
day 6 (33 ) 1292 2112 61
day 7 (32) 2143 4096 52
aEvaluated by a method of approximation using the binary expansion of
formula, N = 2n, as the theoretical model to predict the observed clonal
expansion.
Figure 13. Hypothetical dendrograms for a synblastic clone (○)
displaying a daily division pattern with an AvGT of 24 hours
(bottom), and for an asynblastic clone (●) displaying an alter-
nating daily division pattern for each of the two daughter cells
with an AvGT of 33.6 hours (top), which results in a phase
delay of 1 cycle every 4 days. Abscissa: expected number of
cells (N) on the basis of a daily binary clonal division pattern.
rate. These two are the predominant dendritic patterns
underlying progressive clonal expansion and the two
modalities that are most commonly repeated in mono-
phasic and biphasic growth curves of individual clones.
For the case of a 24 hours GT it is assumed that each
descendant sister-sister pair divides synchronously each
day to attain the observed exponential rate of 2n, where
n = 2. For the 33.6 hour GT, which increases arithmeti-
cally according to the Fibonacci series, an alternating
pattern of sister-sister cell bifurcation is assumed in
which one of the two descendant of each sister cell un-
dergoes a bifurcating division each day with the other
sister cell dividing the following day. This results in a
one full cycle delay every four days relative to clones
with the 24-hour growth rate modality, i.e., modulo +1/4.
Alternatively, clones exhibiting the 33.6-hour doubling
time may be represented as sister-sister cell pair that
divide synchronously each division but with a 40% delay
each division relative to the 24-hour division rate modal-
ity. This would result in two full cycle delay every five
days relative to the 24-hour growth rate modality, i.e.,
modulo +2/5. The former dendritic pattern is favored
based on the step-like growth patterns observed for the
committed cell clones with overt expression of Fibo-
nacci term increments. Clones exhibiting full cycle de-
lays with an average generation time of 48, 72 and 96

J. J. Wille / Natural Science 3 (2011) 702-722
713713
hours can be represented in dendrograms as sister-sister
cell pairs that divide synchronous but only after the lapse
of one, two or three full daily cycles of logarithmic
growth relative to the 24 hour growth rate modality.
They are exactly modulo 1, 2 and 3 cycles out of phase
with single-celled clones that divide once a day. Clones
were observed that displayed a portion of their biphasic
growth with a 28.8-hour logarithmic rate of growth. This
can be diagramed as a dendrogram with bifurcating sis-
ter-sister cell pairs that are delayed fractionally 20%
longer than logarithmically dividing clones that exhibit
an average GT of 24 hours. This results in a one full cy-
cle delay every 6 days of logarithmic growth relative to
the 24-hour modality, i.e., modulo +1/6, this is depicted
with reference to the dendrogram for a synblastic clone
in Figure 14. Likewise clones were observed that dis-
played a 38.4-hour logarithmic rate of growth over some
portion of their biphasic growth. This can be diagramed
as a dendrogram with bifurcating sister-sister cell pairs
that are fractionally delayed 60% longer than logarith-
mically dividing clones with an average GT of 24 hours.
This results in a three full cycle delay every eight days,
i.e., modulo +3/8, relative to the 24-hour growth rate
modality. A number of clones were observed that dis-
played either a 19.2 or a 21.6-hour logarithmic growth
rate either as monophasic or biphasic clonal expansion
growth rates. For the case of 19.2-hour logarithmic dou-
bling time, sister-sister cell pairs divide 40% faster each
day than the 24 hour modality. This results in a one full
cycle advance every four days relative to the 24-hour
cycle of division, i.e., modulo –1/4. Figure 15 is a hy-
pothetical dendrogram for the case of 21.6-hour loga-
rithmic growth rate, sister-sister cell pairs divide 20%
faster each day relative to the 24-hour cycle (shown at
the bottom of the figure). This results in a one full cycle
advance every 10 days relative to the 24-hour cycle mo-
dality, i.e., modulo –1/10. Finally, some clones were
observed which displayed a 14.4-hour growth rate over
some portion of their biphasic or multiphasic logarithmic
growth rate. These clones can be diagramed as a den-
drogram in which sister-sister cell pairs divide synchro-
nous 60% faster each day than the corresponding clones
with a 24 hour-cycle modality; this results in a phase
advance of three cycles every eight days relative to the
24-hour cycle modality, i.e., modulo –3/8. In addition,
we propose the “Rule of Deceleration,” in which sis-
ter-sister cell pairs abruptly change their bifurcation pat-
tern from a 24, 33.6 or 48 hours exponential doubling
rate to a progressively restricted subset of sister-cell
branches, that continue to divide logarithmically but at
decelerated growth rate, resulting in either a switch a
lower full cycle modality (2, 3, or 4) or to a decelerating
dendritic pattern in which one sister cell branch alter-
Figure 14. Hypothetical dendrograms comparing the synblastic
clone (○) with a daily division pattern with an AvGT of 24 hours
(bottom), to an asynblastic clone (●) displaying an decelerated
division pattern with an AvGT of 28.8 hours (top), which results
in a phase delay of 1 cycle every 6 days. Abscissa: expected
number of cells (N) on the basis of a daily binary clonal division
pattern.
Figure 15. Hypothetical dendrogram of clones displaying an
AvGT of 21.6 hours with bifurcating sister-sister cell pairs that
are fractionally 20% faster than logarithmically dividing clones
with an average GT of 24 hours.
nates every other division and assumes an intermediate
cycle modulus (+1/6, +1/4, etc). All possible combina-
tions have been observed in the bi- and multiphasic
growth curves presented above. By contrast, a number of
clones exhibited acceleration from a lower modulus to a
higher modulus, e.g., modulus 1 to modulus –1/4 or
–1/10, and from a modulus +1/4 to modulus 1 or –1/4.
These abrupt changes in logarithmic growth rate can be

J. J. Wille / Natural Science 3 (2011) 702-722 714
depicted as dendrograms in which sister-sister cell pairs
resume synchronous daily division once a day or accel-
erate to fractional cycle advances. For these, we propose
the “Rule of Fractional Phase Advance,” for intraclonal
phase advances. The above hypothetical dendrograms
can be reinterpreted in terms of a phase resetting curve.
Figure 16, plots the amount of phase shift (ΔΦ ≠ 0) as a
whole or fractional cell cycle, Modulus equal to 0 (ΔΦ =
0) of the unshifted cell cycle so that Modulus 1 is shifted
one circadian period of 24 hours. Phase delays are plot-
ted as positive whole or fractional cycle delays (+1, +2
 n), and phase advances are plotted as negative whole
or fractional cell cycle. The data are derived from bi-
phasic and multiphasic segment slope data. Inspection of
the curve shows both phase adavances and phase delays
occur. Phase delays appear to reach an asymptote at
Modulus 3, which concurs with the greatest 72 hour
segment slope observed. Phase advances extrapolate to
the ordinate to yield a cell cycle with an average GT
equal to 12 hours. No average generation time (GT) of
12 hours was observed but such a cell cycle would be
equivalent to a G1-less cell cycle which are often en-
countered in embryonic cell cycles.
4. DISCUSSION
4.1. Interdivision Interval
On first inspection, the kinetic of the first inter-divi-
sion observed for these two different cell strains grown
under two different calcium conditions are remarkably
similar. However, any analysis of these results must take
into account the effects imposed by trypsin treatment on
steady-state conditions prevailing before seeding on to
cloning chips. Dissociation of cellular aggregates into
individual cells by “trypsin treatment” surely must dis-
rupt many on-going cellular processes the consequences
of which are too numerous and indeterminate to either
enumerate or understand. Yet, they are likely to account
to a lesser or greater extent for the observed division
delays. Earlier studies [7] attempting to assess mitotic
delay following deliberate temperature shock treatments
and other so-called perturbations when administered at a
given phase of the mitotic cycle in mitotically synchro-
nized cells subtracted out the time lags due to the dura-
tion of the treatment per se and defined the remainder of
the delay as “excess mitotic delay.” This phase-resetting
map is called a type 1 phase response curve [8]. Unfor-
tunately, this stratagem only works when the delays are
referenced comparatively to specific phases of the mi-
totic cycle. Here, we have adopted the assumption that
prior to trypsin treatment the cells were randomly dis-
tributed with respect to phase according to a “log normal
age distribution.” If the perturbation preceding cell
Figure 16. A theoretical phase resetting curve of NHK cells.
The prior unshifted phase (ΔΦ = 0) is plotted on the ordinate as
AvGT (hours) versus the reset phase (ΔΦ ≠ 0) on the abscissa
(Cell Cycle). The reset phase is plotted as fractional or whole
moduli of 24 hour cell cycle, and shows phase advances (–
modulus) and phase delays (+ modulus) of modular cell cycles.
seeding synchronized all cells to a common phase, the
interdivision interval, i.e., excess mitotic delay would
have led to a single model time of delay. This was not
observed. Therefore, the dissociation treatment did not
interfere with the set of contingent phases present prior
to dissociation. The simplest outcome expected would be
a monophasic log exit from the division cycle. This did
not occur either. Under two different conditions of re-
covery the result was a biphasic log exit from the divi-
sion cycle. Possible reasons for this are discussed below
in conjunction with the other clonal division results.
4.2. Clonal Division Rates
The clonal data presented show that  60% of single-
cell derived clones isolated at random from asynchro-
nously dividing logarithmic cultures can form colonies
of greater than eight cells, and about 50% will form an
exponentially dividing clone in which each cell divides
on average once a day for up to six to eight days of cul-
ture in a serum-free medium. The study also provided
new data showing that individual cells have different
propensities to divide at different rates. The majority of
clones that retain proliferative potential have an average
generation time of approximately 24 hours (circadian
division rate). Nevertheless, a significant number of
clones display exponential rates of growth that are
greater or less than 24 hours. Of interest was the finding
that these clones are restricted to only a few of the many
possible logarithmic growth rates. Clones exhibiting
exponential growth rates greater than 24 hours (infradian

J. J. Wille / Natural Science 3 (2011) 702-722
715715
rates) are restricted to modes at 33.6, 38.4, 48, 72 and 96
hours, while clones exhibiting exponential growth rates
less than 24 hours (ultradian rates) are restricted to
modes of 21.6, 19.2 and 14.4 hours. In addition, indi-
vidual clones may switch abruptly from a circadian
growth rate to either an infradian or ultradian growth
rate. This was amply demonstrated in the pattern and
frequencies of growth rate switches seen in biphasic and
multiphasic growth curves. Another phenomenon en-
countered in single-celled clones was the pattern of
phase delays exhibited by isolated single cells prior to
their first cell division. These delays were restricted to
24 hour multiples of the circadian inter-division periods,
i.e., 48, 72, and 96 hours. Such delays were also en-
countered during exponential growth of clones indicat-
ing that cycle skips can occur after single cells establish
a colony of cells. In particular, the biphasic exponential
rate of entry into the first cell division suggested a cell
cycle-dependent process was perturbed by the serial
passage of cells from steady-state growth to the sin-
gle-celled state. We reasoned that cells in S and G2/M
were less affected by this perturbation as represented by
30% - 40% of cells (Fraction-A see Figures 2 and 3) that
advanced through to cell division in the expected 16
hour interval for cells randomly arrayed between the
start of S phase and the end of the G2 phase. Further, the
remaining undivided cells after a 16-hour delay exited
from the cell cycle at the circadian rate as if they were
randomly arrayed at all possible phase of the circadian
cell cycle (Fraction-B, see Figures 2 and 3). Again, we
reasoned that these must represent cells that were in the
G1 phase of the cell cycle at the time of perturbation. If
so, why did it take 24 hours rather than the expected 12
hours for this cohort to advance through the cell cycle?
In an attempt to reconcile these observations with pre-
vious reports, we examined their cinemicrographic data
[2]. Not unlike our findings, they reported 70% (7/10) of
clones and 67% (14/21) of clones completed cell divi-
sion the first day of observation (Cultures 2 and Culture
3, respectively of Figures 2 and 3). In addition, they
supply dendrograms showing sister cells, in which one
member divides 24 hours after the first division, while
the other sister cells is delayed approximately 72 hours
before its next division (Culture 3, Clone A, of Figure 2).
Equally, they show sister cells that divide synchronously
on the second division cell cycle 24 hours after the first
cell division (Culture 3, Clone C and J of Figure 3).
Also, they show non-synchronous patterns of sister cell
division following the first cell division that are not mul-
tiples of 24 hour cell cycle (Culture 2, Clones A, B, C
and D and Culture 3, Clones K, Q, S, and T). Most in-
terestingly, they observed cases in which the interdivi-
sion time accelerated from 28.7 hours to 21.1 hours, and
cases in which the inter-division time of sister cells ac-
celerated from 33.7 hours to 28.8 hours (Culture 2 of
Figure 2). Finally, they provided a histogram showing
the frequency distribution of inter-divisions times (Fig-
ure 6) in which the modal inter-divisions times were
14.4, 19.2, 21.6, 24, 28.8, where clusters of data points
center around these modal times in their plot of the cor-
relation between filial and parental inter-division times
(Figure 7).
4.3. Significance of Fibonacci Series in
Keratinocyte Clonal Growth
Our results reveal yet another phenomenon: the fre-
quency with which non-proliferating clones exhibit the
Fibonacci series of terms as end-points in terminal
number of cells per clone. A standard explanation for the
appearance of the Fibonacci sequence in biological phe-
nomena is “edge-effects” consequences. In this case,
those clones comprised of daughter cells with unequal
probabilities of dividing in a 24-hour period arise from
unequal or unbalanced steady state conditions. This pos-
sibility is discussed in relation to a more general descrip-
tion of Fibonacci numbers. The Fibonacci series is one
example of a periodic continued fraction that converges
on the numerical value of 1.618, a ratio often called the”
golden mean of unity.” It is deep-set in the regular pen-
tagon, and dodecahedron, where it enters (as the chord
of an angle of 36˚ into the three-isosceles triangles, and
by continued bisection generates apices that have their
locus upon an equiangular spiral. In other biological
contexts, the Fibonacci series has been encountered in
plant morphogenesis as the phenomenon of Phyllotaxis
seen in the spiraled seeds of the sunflower, and fir cones,
and in animal morphology such as the spiraled shells in
foraminiferan and in molluscan shells [9]. There does
not appear to be any references to it in animal tissue or-
ganization and growth. He believed that, it simply re-
flects continuation of constant conditions of growth
where there is a steady production of similar growth
units (i.e. , cells) that are similarly situated at similar
successive intervals of time, This is where the time-
element enters in the development of equiangular spirals
for according the theory of gnomons “one never expects
to find the logarithmic spiral manifested in a structure
whose parts are simultaneously produced [9], see p.
766).” This idea has a parallel in the con text of the gen-
eration of recursive structures by computer algorithms
[10], where the Fibonacci series can be simulated as a
recursive transition network (RTN) This was diagramed
as bifurcating tree of nodes that are recalled successively
in time (see Diagram G, Figure 30). By analogy, we pro-
pose a similar recurrent branching pattern (RTN) under-
lies the clonal expansion of non-synblastic proliferating

J. J. Wille / Natural Science 3 (2011) 702-722 716
clones (see Figure 12) as well as the remarkable finding
of a 33.6-hour doubling time in clonal subsets of clones
exhibiting biphasic growth rates. The 33.6-hour doubling
time is simply obtained when the Fibonacci series terms
are plotted on semi-log paper as a function of 24-hour
intervals (days) of culture.
5. HYPOTHESES
5.1. Circadian Cell Cycling Hypothesis
Various hypotheses have been proposed to account for
circadian clock control of cell division including gene
transcription of clock genes that have kinetics of synthe-
sis, and negative feedback regulation on gene transcrip-
tion to account for the oscillation in timing of mitosis
[11-13]. The circadian transcription clock is a determi-
nistic sequence; it predicts phase advances and phase
delays occur by restarting the transcription clock at ear-
lier or later entry points in the transcription loop of genes.
Uncoupling the transcription clock from the mitotic
clock probably is limited to restriction points in the G1
phase of the cell cycle and in this respect could account
for the multiple cell cycle “on-phase” coupling and un-
coupling of the transcription clock from the mitotic
clock. Finally, it would be hard to construct an interpre-
tation of the transcription clock that can account for
“cross-talk” between cells, leading to phase synchroni-
zation between sister cells or phase dispersion among a
cohort of cells in a clone based solely on the order of
appearance of mRNA transcripts or how this could ac-
count for the observed Fibonacci series in the total cell
numbers arising in committed clones. Although, mo-
lecular models provide a mechanism for sequential pro-
gression through the cell cycle, and provide a molecular
escapement component for the circadian control system
[8]. They all fail to account for the recurrent 24-hour
periods per se, nor deal with the effect of perturbations
to clock functioning, i.e., phase shifting and period al-
terations, and multiple cycle “on-phase” recurrent
rhythms.
5.2. Evidence for Biochemcial Oscillator:
Retrodictions and Predictions
Many results observed in these studies can be ex-
plained by the hypothesis of a biochemical oscillator
with underlying stable limit cycle dynamics [14] as the
temporal control system for human epidermal cell divi-
sion [15,16]. Earlier, we proposed a two-dimensional (X,
Y) biochemical oscillator of the sort previously de-
scribed as a model for explaining the behavior of the
mitotic division rhythm in the acellular slime mold,
Physarum polycephalum [11], and later extended to
mammalian cell cycles [12]. In particular, the proposed
mitotic oscillator model further associates this clonal
division pattern with events leading to the loss of cycling
among proliferating cells during their transition to G1/G0
arrest, and is ultimately involved in the mechanism of
commitment to terminal cell differentiation (see Figure
17). In addition, the puzzling modal frequencies of divi-
sion rates led us to consider that epidermal keratinocytes
may find an explanation in a mitotic oscillator-based
clock that controls cell division timing. Figure 17 arbi-
trarily maps the different cell cycle phases as sets of
neighboring states along the limiting trajectory. The lim-
iting trajectory moves smoothly through each of the
states in a counter-clockwise motion. In addition, it
means something dynamically to be at states of the sys-
tem that lie off the limiting trajectory. These are real
biochemical states that lie in the state space of the limit
cycle. Perturbations may carry the system to point off
the limit cycle through a destruction vector that drives
the system toward the origin (Xo, Yo), or by changing
the parameters of the constant kinetic scheme. In either
case, once transients die down, return from these states
occurs along trajectories that wind back on to the limit-
ing trajectory arrive at their ultimate phase according to
the isochron structure of the oscillator. The model ret-
rodicts that the temporal control of cyclins during the
cell cycle occurs as the closed path trajectory of the limit
cycle mitotic oscillator passes through biochemical
states that could trigger transcription of specific cyclin
genes and their gene products [19]. Figure 18 simulates
the results of a perturbation given at a critical phase and
critical intensity along the limiting trajectory that map to
sets of neighboring states in the G2 phase of the cell cy-
cle. As shown, the perturbation drives the system “in-
side” the limiting trajectory on a destruction vector that
points toward the origin. Upon release, the system re-
turns along a winding trajectory that crosses the Yc trig-
gering mitosis from the inside the limit cycle. In this
scenario, there is little or no delay to mitosis, and the
next cycle will occur in phase with identical copies of an
unperturbed oscillator. However, if the system is driven
further inside the limit cycle along the same isochron in
the G2 phase, upon release it will return on a “grazing”
or sub-threshold trajectory that does not cross the Y-axis
at the Yc value as required to trigger mitosis. These lat-
ter copies of the oscillator will not undergo mitosis/cell
division. Nevertheless, after a slightly less than one cy-
cle delay the next cell cycle will recur in phase with an
unperturbed copy of the oscillator, since the” grazing”
trajectory crosses the limiting trajectory in phase. A still
more intense perturbation given at the same critical
phase in G2 will drive the system toward the singularity.
Since this is a phase-less (timeless) locus where all

J. J. Wille / Natural Science 3 (2011) 702-722
717717
Figure 17. Diagram showing the effect of a perturbation given
at a state in the G2 phase (open shaded ellipse) that instantane-
ously drives the limiting trajectory “inside” the closed path
trajectory where it continues to cycle in phase (θ1) but follows
a new trajectory (dotted arrow) that fails to achieve sufficient
concentration of Y, Yc (shaded circle) to trigger mitosis result-
ing in a one or more full cycle delays.
Figure 18. Oscillator clock control of recurrent events of the
cell cycle. Different events of the cell cycle are driven when
the limiting trajectory of the oscillator pass through the arbi-
trarily drawn regions of state space (circles and boxes). Mitosis
is triggered at Yc, a critical concentration of active mitogen (Y).
Cytokinesis occurs when the trajectory reaches a near maxi-
mum of Y. The G1 phase of the cell cycle is associated with a
region of state space near a secondary steady state, SS1 that is
surrounded by a low amplitude oscillation. Cells cycle there
until reentering the high amplitude limit cycle oscillation, de-
pendent on growth contingencies (variable kinetics). Alterna-
tively cells in the SS1 region can drift into a tertiary steady
state labeled SS2 in the region of state space surrounded by an
anti-limit cycle (curved line) and become extremely slow cy-
cling in a resting state, G0. The S phase is denoted by the boxed
region with minimal Y and increasing concentration of X, the
inactive mitogen. Finally, the boxed region of state space de-
noted by G2 occurs when the limiting trajectory passes through
set of states with high concentration of X and increasing con-
centration of Y.
isochrons converge at or near the singularity (indetermi-
nate point), copies of the system that are at or near the
singularity can take up as many different ultimate phases
as the isochrons upon which they lie. Upon release, the
various copies of the system will appear to have random
phases with respect to one another, i.e., high phase dis-
persion. The account given above is in accord with the
observed phase resetting behavior of cells presumed to
be in the mid-G2 phase of the cell cycle. These cells ex-
hibit little inter-divisional delay, and their succeeding
cell divisions recur in phase. Still other cells behave as if
they were driven to a “grazing” or sub-threshold trajec-
tory and skip mitosis/cell division, but since they are on
a G2 isochron, their succeeding cell divisions recur in
phase with an unperturbed cell another, i.e., high phase
dispersion. This brief account has been provided to the
reader to facilitate understanding further predictions of
the model.
5.2.1. Model Confirmation: G2 Phase
Perturbation
The following are predictions if the perturbation
drives the initial state of the system to a new set of states
that return to the limiting trajectory in phase after one
cycle delay. By inference, cells exhibiting multiple cycle
delays prior to resuming in phase cell cycling are copies
of the oscillator that are driven yet deeper inside the state
space of the oscillator but are still on a G2 isochron, and
upon release follow a sub-threshold trajectory that re-
quires multiple cycles before winding onto the limiting
trajectory. The biochemical oscillator system used to
model these G2 perturbations had a “winding number of
three, which easily could explain multiple cycle delays
and return in phase for clones exhibiting 48, 72 and 96
hour delays before cycling, which, incidentally, was the
longest delays observed. In our model oscillator the an-
gular velocity is the same off the limiting trajectory as
drawn by straight-line isochrons. Otherwise, events would
get out of phase if the angular velocity is faster or slower
on isochrons that lie inside the state space of the oscilla-
tion. This does not appear to be the case for the underly-
ing oscillation preserving the cell division rhythm in
normal human epidermal keratinocytes.
5.2.2. Model Confirmation: G1 Perturbation
Cells presumed to be in the G1 phase at time of per-
turbation, were delayed 16 - 24 hours (i.e., a skipped one
cell cycle) before they started to divide, and then it took
them another 24 hours to complete this first inter-divi-
sion cycle, as if upon release the cells were randomly
arrayed at different phases of the cell cycle. In order to
account for the phase behavior cells perturbed during the
G1 phase of the cell cycle, it is necessary to explore the

J. J. Wille / Natural Science 3 (2011) 702-722 718
state space surrounding the G1 isochrons. A destruction
vector at G1 drives the oscillating system off the cycle in
the direction of the origin, but on a G1 isochron. There-
fore, identical copies of the oscillating system released
from these “off cycle” states should resume cell cycling
in phase with unperturbed copies of the oscillator that
were cycling from phases near a G1 isochron. This did
not happen. Why?
Previous work on mammalian cell cycles suggested
that G1 phase of the cell cycle may not be part of the
constant kinetic high amplitude oscillation, i.e., an inde-
terminate or phase-less portion of the cell cycle [11]. As
cells complete mitosis and cytokinesis, the synthesis of
many messages stops while chromosomes undergo a
chromatin condensation in prophase, and then decon-
densation in telophase. A realistic biochemical oscillator
model would posit that cells in early inter-phase enter a
G1 checkpoint and depending on the availability of pre-
formed precursors and the presence of active synthetic
machinery for synthesizing X and Y, temporarily exit off
the cell cycle to become G1/G0-arrested cells. It is pro-
posed that the high amplitude oscillation under variable
parameterization becomes a low amplitude oscillation,
existing entirely within the state space of G1 isochrons
(see Figure 18). Since constant kinetics no longer pre-
vail, the limit cycle amplitude would decrease and its
singularity drift toward the X, Y origin, at which point
the systems dynamics can undergo a bifurcation. One
parameterization of the coefficients of the state variables,
for example, is a decrease in the rate of synthesis of X.
Bifurcation can occur in one of two ways. The first is by
a “soft” bifurcation, occurring at the G1 checkpoint,
which would carry the oscillating system near to but not
within a neighboring basin of attraction. Simply by in-
creasing the rate of synthesis of X to the original rate
would return the system to the high amplitude oscillation.
Cells would then be back on the G1 isochrons, and stay
in phase. It is posited that unperturbed cells, having a
minimum 12 hour G1 phase, routinely undergo a soft
bifurcation in early inter-phase, and cycle with a 12 hour
period in the low amplitude oscillation before they gain
enough rate of synthesis of X to return to the high am-
plitude oscillation. The high amplitude oscillation is also
a 12-hour period. Together the small and large amplitude
oscillations constitute a 24-hour or circadian cell cycle
[11]. The second mode of exit from the cell cycle, also
occurring in early inter-phase, is by way of a “hard” bi-
furcation, which would carry the oscillating system into
an anti-limit cycle trapped within a basin of attraction
(see Figure 17). A “hard” bifurcation creates a “hystere-
sis effect” whereby a higher rate of synthesis of X is
required to restore the low amplitude oscillation than
that prevailing under constant kinetics of the high am-
plitude oscillation. Cells trapped in an anti-limit cycle
are non-cycling, i.e., phase-less. Hence, they exit
through a stochastic process in achieving sufficient syn-
thetic rate to restore the low amplitude oscillation.
Events beyond the restoration of the low amplitude limit
cycle oscillation are the same as in the soft bifurcation.
In summary soft to hard bifurcation switch accounts for
the variable dwell time of G1/G0 cells, while a hard bi-
furcation accounts for random process whereby G1 phase
cells return to the cell cycle. This modeling predicts that
there will be large phase dispersions in perturbed G1
cells.
5.3. Limit Cycle Dynamics Model:
Diffusively Coupled G1 and G2
Phase Cells
We now attempt to account for the fact that many
clones experienced a 33.6-hour exponential growth rate
upon resumption of clonal growth from a perturbed cell
cycle. This can be accounted for by considering the fact
that epidermal keratinocytes grow in a colony with each
cell in contact with one or more sister cells. The possi-
bility exists for neighboring sister cells within a colony
to interact diffusively with each other. Consider the case
where two independent copies of the oscillating system
are located in adjacent boxes connected by a semi-per-
meable membrane. If we let the variables of the dy-
namical system be the diffusible components, and de-
pending the allowed rate of diffusion, one can obtain
either synchrony (homogeneous solution) or persistent
asynchrony (inhomogeneous solution), i.e., phase cou-
pling results in synchrony if the two copies of the oscil-
lator are close in phase, while phase coupling between
far apart phases may fail to synchronize. Computer
simulations (data not given) of phase coupled limit cycle
oscillators that are diffusively coupled demonstrates that
a line connecting phases between an oscillator in G1
phase with a second oscillator in G2 phase may result in
the persistent sub-threshold amplitude oscillation in the
box containing the G2 oscillator while the oscillator in
the G1 box continues to cycle with a high amplitude os-
cillation. In this situation, the G2 cell oscillates below
the threshold (YC) required to trigger mitosis/cell divi-
sion. At greater diffusion rates, both oscillations may be
dampened to have sub-threshold oscillations, in which
case cell division would cease in both cells even though
the oscillation preserving rhythmicity of cell division
persists. Uncoupling of the oscillator pair would restore
the cell division rhythm. It is precisely, this phase cou-
pling by diffusion (electrical, chemical, etc) that is in-
voked to occur in epidermal cell colonies that account
for an alternating sequence of threshold and sub-thresh-
old oscillations between pairs of sister cells. Such an

J. J. Wille / Natural Science 3 (2011) 702-722
719719
mechanism would produce a pattern of alternating cycles
of division such that one member of the pair divides
while the other does not, but then on the succeeding cy-
cle the sister cell that failed to divide now divides, while
the other sister cell fails to divide. This situation is de-
picted in the dendrogram representing the case of clones
exhibiting a 33.6-hour AvGT (see Figure 13). Obviously,
uncoupling of sister cells results in a return to synchro-
nous 24-hour cell cycles.
5.3.1. Predictions (“p”)
p1: Phase compromise occurs at a phase off the limit-
ing trajectory of the oscillating system (θi, xi, yi) that
results in a one-cycle delay for the G2 cycling cell, and
to a phase advance for the G1 cycling sister cell.
p2: The G2 cycling sister cell misses one cell division
cycle (cycle 2), but it eventually winds back onto the
limiting trajectory of the large amplitude oscillation and
then re-enter G2 phase in phase with Cycle 3. Here it
again comes into a relationship to phase-couple with a
G1 cycling cell that has completed its second cycle and is
now in cycle 3. This reinitiates the phase compromise
situation and results in a perpetuated pattern of alternat-
ing sister cell divisions as witnessed by Fibonacci series
terms in total cell number per clone per day, i.e., GT =
33.6 hours (this model is further discussed below in dual
oscillator-probabilistic mitotic control model.
5.3.2. Confirmation
1) These predictions are confirmed in data presented
in Figures 2 and 3 (biphasic inter-division kinetics)
based on the expected proportion of G1 cells present at
the time of cloning from an asynchronous logarithmi-
cally dividing parent culture.
2) In asynchronous culture with a 24 hour cell cycle
time (data from clones of strains F and G).
The predictions are also fulfilled in data presented in
Table 2 (biphasic growth curves alternating from 24
hour to 33.6-hour GT slopes.
3) The prediction is also verified for clones exhibiting
the Fibonacci series of limited or “committed” prolifera-
tive potential (e.g., see data in Figure 6 and Figure 12
of “committed cell” clones of strain D.
5.4. Stochastic Cell Cycle Models: Evidence
for Probabilistic Control of G1 States of
the Cell Cycle
Although the stable limit cycle oscillator model does a
good job in accounting for “multiple” skipped cell cycles,
phase resetting of G2 perturbed cells, and phase com-
promise behavior in diffusively coupled G1 and G2 cells,
it requires an ad hoc hypothesis to explain G1 phase re-
setting following a perturbation, i.e., a stochastic com-
ponent as part of the cell cycle and collapse of the limit
cycle following a “hard bifurcation” in the G1 phase. In
addition, the mitotic oscillator hypothesis does not easily
explain period shortening without resort to an ad hoc
provision that would increase the size of the high ampli-
tude oscillation, e.g., by increasing the synthesis rates of
the one or more variables of the system. In the discus-
sion, a clone dividing exponentially at AvGT of 21.6
hours was explained by introducing a 10% increase in
the rate of cell division. Likewise, for clones that showed
a consistent delay that is an integral fraction of the
24-hour cycle, e.g., 28.8 hours, a 10% bias in lengthen-
ing the cell cycle relative to a standard 24 cell cycle was
introduced. We now attempt to show that this fits a
probabilistic mode for temporal control of the G1 phase
of the cell cycle in which there is a sensitive dependence
on initial conditions with resultant chaos in the oscillator
dynamics. Earlier, a theoretical model was examined for
the distribution of cell size and generation time that in-
corporated size control and random transition [17,21].
They found that a model with a random-exiting phase of
the cell cycle and a minimum size requirement for entry
into the random-exiting phase predicted exponential
beta-curves that are characteristic of sister cell genera-
tion times. They argue against unequal mother cell divi-
sion as the source of random fluctuations in determinis-
tic cell size models, and that transition-prob- ability
models with no feedback from cell size are unable to
account for the rapidity with which new, stable size dis-
tributions are reestablished. Indeed, deterministic cell
cycle models with transition probability like properties
have been proposed [19].
5.5. Daughter Cell Size/Cell Mass Variance
Can Perpetuate Lineage Differences
We begin this discussion with observations drawn
from two well-documented An example of cell lineage
differences due to a consistent bias in the division rate of
cell division products comes from prokaryotes in which
careful measurements of inter-division period indicated
that there was as much as a 20% variation in inter-divi-
sion times between sister cells, and further that there is a
correlation between filial and grand-parental generations
in inter-division timing and size (Kubitchek, per. com-
mun). These results have been interpreted as due to bio-
logical variation in the cell division process with smaller
daughter cells requiring longer inter-division period and
larger daughters requiring less time to reach the doubled
cell mass before dividing to form smaller cells. The lar-
ger notion is that there is inherent variation of the cell
division process that leads to cell size/mass variability,
which in turn is compensated for by a mass to DNA ratio
threshold mechanism. In this regard, unequal division in

J. J. Wille / Natural Science 3 (2011) 702-722 720
“conical,” a mutant of the ciliated protozoan Tetrahy-
mena, leads to sublines of different sizes. Regulation to
normal size occurs over several generations by slowing
the rate of cell division of the smaller cell with all phases
of the cell cycle being prolonged [22]. In the ciliated
protozoan Paramecium the two products of division are
readily recognized, separately cloned separately. The
clonal lineage of the anterior product, called the proter,
can be compared with that of the posterior product of
division, the opisthe. In essence each half-cell must build
a new half, the proter builds a new opisthe and the 0pithe
builds a new proter. Other asymmetries may also exist
with regard to the distribution of mass and organelles.
Proter lineages display shorter inter-division times rela-
tive to opisthe lineages (T. M. Sonneborn, per. commun).
These results indicate that consistent biases in cell divi-
sion can generate proportional cell cycle advances and
delays. Finally, new genetic and molecular studies in the
yeast Saccharomyces cerevisiae have provided evidence
in favor of the “critical cell size model” for coordination
of cell growth with division [21]. They reported that
both the expression and the activity of G1-phase cyclins
is modulated by growth rate and cell size in yeast. Other
data obtained in these studies suggested that the prolif-
erative capacity also correlates with cell size.
5.6. Probabilistic Model for G1 Phase
Duration
Variability in the duration of the G1 phase has been
well established [22] and G1-less cells occur during em-
bryogenesis, in mutant mammalian cells [23]. Here, we
propose that each cell division creates a proter-like and
opisthe-like cell line, and further that the proter-like cell
(arbitrarily) has an inherent bias in its expected in-
ter-division time while the opisthe-like cell line does not.
The reasoning for this has been given above, e.g., bias in
cell mass for anterior cell products. The bias is con-
strained to values that lie within the standard deviation
for the process of cell division as a whole. We then as-
sign probability values, p and q to the proter and opisthe
cell division rates, respectively, to reflect a fractional
deviation of the proter-like cells from the standard rate
(mean inter-division time). If there were no biases, the
value of p and q are both equal to one-half (0.5), then
according to the expansion of the binomial equation an
equilibrium distribution of proter and opisthe cell lines
are predicted to remain stable over succeeding genera-
tions. In the following example, we let the proter cell
line have a 10% decrease in mean inter-division time.
Table 6 compares the predicted number of proter and
opisthe cells having ± 10% bias in mean inter-division
rate with the combined proter or opisthe cell increase
without a bias in mean inter-division time, assuming the
24 hours cell cycle for unbiased rate. When the com-
bined number of proter and opisthe cells are plotted
against each successive mean inter-division interval, it
yields a logarithmic rate of increase at a GT = 21.6 hours.
Likewise, if we had let p remain constant at 0.5 and in-
stead let the value of q be 10% greater than the mean
inter-division time, the combined number of proter and
opisthe cells plotted at each successive mean inter-divi-
sion interval yield a logarithmic rate of increase clone
expanding at a GT = 28.8 hours. Another consequence of
a continuing bias in mass of the anterior versus the pos-
terior cell products is the alternating reappearance of the
anterior product in the opisthe of the preceding genera-
tion, perpetuating an alternating pattern of delays and
advances in each succeeding clonal line as detailed be-
low.
5.7. Dual Oscillator-Probabilistic Control of
Cell Cycle
Diffusive Coupling between Proter and Opisthe
Cells that are in the G1 and G2 Phases in
Epithelial Cell Colonies
Here we propose that soon after division, daughter
cells that have a cell mass 10% than the mean cell mass
at division, termed the Proter, leave the limit cycle by a
soft bifurcation undergo a 12 hour small amplitude os-
cillation and return to the limiting trajectory of the large
amplitude oscillation in the neighborhood of a G1 isoch-
ron. In this second cycle, it traverses the S phase and
then now becomes diffusively coupled to a cell in the G1
phase. As we indicated before, this coupling results in
phase compromise between the G1 - G2 pair, in which
the G2 phase cell is driven inside the oscillation to a
sub-threshold G2 trajectory where it fails to trigger mito-
sis/cell division. It now exits the limit cycle in the
neighborhood of G1 isochrons that cause it to undergo a
hard bifurcation. This drives it to an anti-limit cycle
where it must cycle at least twice before gaining enough
amplitude to renter the large amplitude oscillation. In
this way a proter cells in cycle 1 is converted to an opis-
the cell in cycle 3, and an opisthe cell in cycle 1 is con-
verted to a proter cell in cycle 3. This pattern of clonal
proliferation is illustrated by the dendrogram depicted in
Figure 19.
5.8. Deterministic versus Stochastic
Control of Cell Cycling or is it both?
As we showed, certain aspects of the cell cycle such
as G2 phase perturbation were well handled by the limit
cycle dynamics while other aspects such as G1-phase
duration seemed to be better accounted for by a probab-
listic model. This subject has recently been modeled as a

J. J. Wille / Natural Science 3 (2011) 702-722
721721
Table 6. Probabilistic model prediction of clone size of cell
lineages with examples of biased versus unbiased mean inter-
division times.
Successive
Generationsa (n)Clone Sizea GTb
Condition:
0 1 24
1 2
2 4
1. p = q = 0.5
3 8
0 1 21.6
1 2.1
2 4.4
2. p = 0.55, q = 0.5
3 10.2
0 1 19.2
1 2.2
2 4.8
3. p = 0.6, q = 0.5
3 12.3
aSuccessive generations are calculated from the terms of the binomial ex-
pansion, (p + q)n 1. bGT, average generation time derived from semi-log
plot of clone size versus mean inter-division time of unbiased cell lineages.
d
d
d
d
d
d
d
Figure 19. Hypothetical dendrogram illustrating diffusive cou-
pling (d) between the proter and opisthe products of cell divi-
sion which occur on a daily basis (days 1, 2 , 3, and 4) for each
proter descendant, but only on days 3 and 4 for each of the
opisthe descendants, resulting in a Fibonacci sequence of ac-
cumulated cells (N) and an AvGt = 33.6 hours.
complex network of regulatory protein (cyclin-depend-
ent kinases and their activator and inhibitors [26], where
by the stochastic fluctuations arise both from intrinsic
molecular noise and extrinsic noise from unequal divi-
sion. In particular, t the eukaryotic cell cycle is actually
evolved from a prokaryotic deterministic G1-less cell
cycle through acquisition of an environmentally-sen-
sitive stochastic transitional phase inserted between cy-
tokinesis and chromosome decondensation, i.e., a time-
less phase in the cell cycle. Here, we propose that the
epidermal keratinocyte cell cycle has both a determinis-
tic portion and a stochastic portion. The former appears
to be a fixed sequence of events that begins at the G1/S
checkpoint in S and to terminate with mitosis and cyto-
kinesis. We suggested earlier that this fixed sequence is
underlain by a biochemical oscillator that triggers events
during the S-G2/M phases in the acellular syncytial slime
mold [10]. In mammalian cells, however, cytokinesis not
only partitions the mother cell into two compartments,
but results in a sharp phase discontinuity between sister
cells, as well as halving the mass to DNA ratio, thus,
severing the steady-state conditions upon which the ex-
istence of limit cycle deterministic control system was
predicated. The void in mitotic control may be just that.
In its place we propose a stochastic system of control of
G1 phase control in which a critical threshold of cell
size/cell mass is required before reentry into the deter-
ministic portion of the cell cycle. In effect upon cell di-
vision, cells entering early G1 are faced with whims of
environmental vicissitudes. Mammalian cells have
“learned” to cope with this evolutionarily by inventing a
series of environmental checkpoints that limit entry into
the deterministic portion of the cell cycle. The plethora
of decision points converge on that portion of the deter-
ministic control system in the limit cycle dynamics where
the trajectory naturally collapses in the G1 isochron re-
gion of the limit cycle and where it may undergo transi-
tion to an unstable singularity with a much diminished
oscillation, or it but may rapidly return to the large am-
plitude limit cycle upon stochastically attaining thresh-
old cell size/cell mass. This situation appears to occur
routinely for cells meeting all of the environmental con-
tingencies, nutrients, growth factors, matrix support, but
to be lacking in cells that sink deeper into G1/Go arrest,
and cell death. Ultimately only continued cell cycling or
initiation of a terminal cell differentiation program can
assure cell survival.
6. DEDICATION AND
ACKNOWLEDGEMENTS
This paper is dedicated to Dr. Charles F. Ehret, who died February
24, 2007 at aged 83. He introduced me to the exotic yet ubiquitous
world of chronobiology. Theoretical and experimental work was con-
ducted on a biochemical oscillator model for the synchronous mitosis
in Physarum polycephalum at the University of Chicago in collabora-

J. J. Wille / Natural Science 3 (2011) 702-722 722
tion with Dr. Stuart A. Kaufman and Dr. John J. Tyson. The initial
laboratory work was undertaken at the Mayo Medical School in the
laboratory of Dr. Robert E. Scott. I wish to thank Ms. Nell Swanson for
her diligent technical assistance and my gratitude to Dr. Mark Pit-
telkow of the Mayo Medical School for his invaluable scholarship.
REFERENCES
[1] Riley, P.A. and Hola M. (1980) Clonal differences in
generation times of GPK epithelial cells in monolayer
culture. Experimental Cell Biology, 48, 310-320.
[2] Kitano, Y., Nagase, N., Okada, N. and Okano, M. (1982)
Cinemicrograhic study of cell proliferation patterns and
interdivision times of human keratinocytes in primary
culture. Normal and Abnormal Epidermal Differentiation,
University of Tokyo Press, Tokyo, 97-108.
[3] Barrandon, Y. and Green, H. (1985) Cell size as a deter-
minant of the clone-forming ability of human keratino-
cytes. Proceedings of the National Academy of Sciences
of the United States of America, 82, 5390-5394.
doi:10.1073/pnas.82.16.5390
[4] Barrandon, Y. and Green H. (1987) Three clonal types of
keratinocytes with different capacities for multiplication.
Proceedings of the National Academy of Sciences of the
United States of Amrica, 84, 2302-2306.
doi:10.1073/pnas.84.8.2302
[5] Wille, J.J. (1999) Heterogeneity in clonal potential and
life histories in individual keratinocytes propagated in
serum-free medium. Journal of Inventigative Dermatol-
ogy, 112, 573.
[6] Wille, J.J., Pittelkow, M.R., Shipley, G.R. and Scott, R.E.
(1984) Integrated control of growth and differentiation of
normal human prokeratinocytes cultured in serum-free
medium: clonal analyses, growth kinetics, and cell cycle
studies. Journal of Celluar Physiology, 121, 31-44.
doi:10.1002/jcp.1041210106
[7] Sheffey, C. and Wille, J.J. (1978) Cycloheximide-in-
duced mitotic delay in Physarum polycephalum. Experi-
mental Cell Research, 11 3, 259-262.
doi:10.1016/0014-4827(78)90365-8
[8] Bass, J. and Takahashi, J.S. (2010) Circadian integration
of metabolism and energetic. Science, 330, 1349-1354.
doi:10.1126/science.1195027
[9] Thompson, D.W. (1943) On growth and form. Cam-
bridge University press, New York.
[10] Hofstadter, D.R. (1980) Godel, esher, bach: An eternal
golden braid. Chapter V: Recursive Structures and Proc-
esses, Vintage Books. A Division of Random House,
New York, 131-137.
[11] Kauffman, S.A. and Wille, J.J. (1975) The mitotic oscil-
lator in Physarum Polycephalum. Journal Theory Biol-
ogy, 55, 47-93. doi:10.1016/S0022-5193(75)80108-1.
[12] Barnett, A. Ehret, C. and Wille, J.J. (1969) Testing the
chronic theory of circadian timekeeping, Biochronometry,
Proceeding of the Symposium on Biochronometry. Fri-
day Harbor, Washington, D.C.
[13] C.F. Ehret and E.Trucco E, (1967) “Molecular models for
the circadian clock. I. the chronic concept”. Journal The-
ory Biology, 15, 240-262.
doi:10.1016/0022-5193(67)90206-8
[14] Glass, L. and Mackey, M. (1988) From Clocks to Chaos.
The Rhythms of Life, Chapter 2. Princeton University
Press, Princeton.
[15] Tyson, J.J. and Novak, B. (2008) Temporal organization
of the cell cycle. Current Biology, 18, R759-R768.
doi:10.1016/j.cub.2008.07.001
[16] Novak, B. and Tyson, J.J. (2008) Design principles of
biochemical oscillators. Nature Reviews, 9, 981-991.
doi:10.1038/nrm2530
[17] Brooks, R.F. Bennett, D.C. and Smith, J.A. (1980) Mam-
malian cell cycles need two random transitions. Cell, 19,
493-504. doi:10.1016/0092-8674(80)90524-3
[18] Wille, J.J. and Scott, R.E. (1984) Cell cycle-dependent
integrated control of cell proliferation and differentiation
in normal and neoplastic mammalian cells. Cell Cycle
Clocks, New York, 433-453.
[19] Tyson, J.J. and Hannsgen, K.B. (1985) The distributions
of cell size and generation time in a model of the cell cy-
cle incorporating size control and random transitions.
Journal Theory Biology, 11 3, 29-62.
doi:10.1016/S0022-5193(85)80074-6
[20] Kar, S. Baumann, W.T. Paul, M.R. and Tyson, J.J. (2009)
Exploring the roles of noise in the eukaryotic cell cycle,
http://www.pnas.org/cgi/doi/10.1073/pnas.0819934106
[21] Mackey, M.C. Santavy, M. and Selepova, P. (1986) A
mitotic oscillator model for cell cycle with a strange at-
tractor, Non-linear Oscillations in Biology and Chemistry.
Springer-Verlag, Berlin, 354-454.
[22] Schafer, E. and Cleffmann, G. (1982) Division and
growth kinetics of the division mutant “conical” of Tetra-
hymena, Experimental Cell Research, 137, 277-284.
[23] Zhang, R. Aguila, D. Schneider, C. and Schneider, B.L.
(2005) The importance of being big. Journal Invest Der-
matol Symp Products, 10, 131-140.
doi:10.1111/j.1087-0024.2005.200414.x
[24] Mitchison, M. (1971) The Biology of the Cell Cycle.
Cambridge University press, London.
[25] Kubitschek, H.W.E. (1970) Evidence for the generality of
linear cell growth. Journal Theoretic Biology, 28, 15-29.
doi:10.1016/0022-5193(70)90061-5.
[26] Baserga R., (1976) Multiplication and Division in Mam-
malian cells. Marcel Dekker, New York.