Materials Sciences and Applicatio n, 2011, 2, 1139-1142
doi:10.4236/msa.2011.28154 Published Online August 2011 (http://www.SciRP.org/journal/msa)
1139
Alloying Behaviour of CuPd Liquid Alloy
Bhrigunandan Prasad Singh1, Devendra Adhikari1*, Indu Shekhar Jha2, Jitendra Kumar3,
Ram Prasad Koirala2
1University Department of Physics, T. M. Bhag. University, Bihar, India; 2Department of Physics, M. M. A. M. Campus, Biratnagar
Tribhuvan University, Biratnagar, Nepal; 3Metals and Ceramics Div., Research Institute, University of Dayton, Dayton, USA.
Received April 20th, 2011; revised May 23rd, 2011; accepted June 16th, 2011.
ABSTRACT
The mixing properties of CuPd alloy in molten state at 1350 K have been studied by assuming strong interaction be-
tween Cu and Pd atoms. Regular associated solution model has been used for the study. The asymmetry in integral ex-
cess free energy of mixing, heat of mixing and entropy of mixing has been well explained.
Keywords: Strong Interaction, Entropy of Mixing, Mole Fraction, Electronegativity, Size Factor
1. Introduction
The knowledge of thermodynamic properties of binary
liquid alloys is necessary for the design and development
of reliable materials for high temperature application.
Moreover, the properties of alloys in the melt are helpful
to understand the alloying behaviour of alloys in solid
state. Thus the determination of thermodynamic func-
tions for alloys in liquid state has been the subjects of
active research for many years.
Growing technological interest to the nonperiodicity in
the atomic arrangement of disordered materials has led to
an increasing need for a better description of their atomic
scale structures. In this paper, we intend to study the
thermodynamic properties of CuPd alloys in liquid state
at 1350 K on the basis of regular associated solution
model. Regular associated solution model has been
proved to study the thermodynamic and structural prop-
erties of weakly, moderately and highly interacting liquid
alloys [1-6] by assuming the formation of complex. Such
assumptions have been used in different models [7-12].
The phase diagram, which is the fundamental source to
understand the formation of complex, manifests the
presence of Cu3Pd, Cu4Pd and CuPd intermediate phases
in solid state [13]. It is well known fact that the size fac-
tor and electronegativity difference of the constituent
metals play an important role on the alloying behaviour
of the binary alloys. The size factor, which has been con-
sidered to explain the anomalous behaviour of liquid
alloy [14], is small (= 1.25) and does not seem responsi-
ble for asymmetry in such alloys. The electronegativity
difference of the constituent metals is also important for
making alloys. Researchers [15] have argued that when
metals of very different electronegativity are alloyed, a
change from metallic behaviour to, at least, partly ionic
behaviour is expected. But the electronegativity differ-
ence of Pd and Cu (= 0.25) is not large enough to account
the alloying behaviour of CuPd alloy. Thus, I have as-
sumed strong interaction between Cu and Pd atoms is
responsible for the the alloying behaviour of CuPd alloy
in liquid state. The Cu and Pd atoms are considered to be
energetically favoured to form chemical complexes
Cu3Pd. The basic formalism of regular associated solu-
tion model with the assumption of strong interaction is
presented in Section 2.
2. Regular Associated Solution Model
Suppose type A and type B metals are mixed in the melt
to form alloy AB. According to regular associated solu-
tion model, the melt consists of three species namely
unassociated A-atoms, unassociated B-atoms and com-
plex AB. Considering a solution of atoms of A and
2 atoms of B, the formation of complex re-
quires 1AApB
1
n
Ap
nnB
nn pn

 and 2
B
ApB for conser-
vation of mass in the partially associated solution, where
AB and ApB are concentration of unassociated
A-atoms, B- atoms and complex respectively where p is
small integer. When there is association, the thermody-
namic behaviour of complexes A and B components is
governed by their true mole fractions A
nnn
,nn,n
x
,
B
x
and ApB
x
rather than their gross mole fraction 1
x
and2
x
, where

Alloying Behaviour of CuPd Liquid Alloy 1140
1
1
12
n
xnn , 2
2
12
n
xnn  A
A
ABApB
n
xnnn  ,
B
B
ABApB
n
xnnn  and ApB
ApB
ABApB
n
xnnn  .
Using above relations we can show 12AApB
x
xpxx ,
22
(1 )
B
ApB
x
xpxx .
Following Lele and Ramchandrarao [2], the equilib-
rium constant for the reaction
A
pB pA B is given
by

 
12
13 23
ln ln1
11
P
AB
BBA
ApB
ApBA AApBB B
xx
kpxxx
xRT
pxx xxpx x
RT RT








 

 

(1)
and the integral excess free energy
x
s
G is given by







12 13
ln
ln
B
ApB
xx
x
RT k
23
112 2
1
1
ln ln
1
lnln 1
xs
A BA ApBApB
ApB
AABB ApB
ApB
ApB
ApB
Gxxxx
px
RT xxxx x
px
x
RT xxxxpx

  






(2)
where 12

, 13

and 23

are interaction energies for
the species A, B; A, ApB and B, ApB respectively, T the
temperature and R stands for the universal gas constant.
The pairwise interaction energies and equilibrium con-
stant are determined following Lele and Ramchandrarao
[2] as follows:
In a regular associated solution11 AA
x
x

  and
22
B
B
xx

 , where 1

and 2

are respective gross
activity coefficients of components 1 and 2. Thus
1
1
lnlnln A
A
x
x
  (3a)
and 2
2
lnln ln
B
B
x
x
  (3b)
Following the technique of Lee and Ramchandrarao [2]
the pairwise interaction energies, the equilibrium con-
stants and the activity coefficients at infinite dilution can
be written as
012
1
ln RT

  (4a)

12
13
12
exp
oo
oo
kRT



 (4b)
where1
o

and 2
o

are activity coefficients of compo-
nent A and that of B at zero concentrations.
Solving equations (2a) and (2b) we obtain
 
21
13
2
ln1 ln1
BBB
BA
ApB
aa
xxxx
12
B
x
xR
RT x
T


 
 
 
 

(5)
 
12
23
2
ln1 ln1
AAA
AB
ApB
aa
xxxx
12
A
x
xR
RT x
T


 
 
 
 

(6)
Using equations (4), (12) and (13), we can derive
13 1
212 12
1
ln ln
ln ln
A
ApB A
p
B
ApB BApB
xa
kRT xx
x
aa
xxRTx
















  




a
(7)
Now heat of mixing and entropy of mixing can be re-
lated to the free energy of mixing with the standard
thermodynamic relations as follows
,TP
G
HGTT


 



(8)
H
G
ST

 (9)
3. Results and Discussion
We have calculated the concentration of complex, equi-
librium constant and pairwise interaction energies fol-
lowing the method employed by Lele and Ramchancra-
rao [2] and D. Adhikari et al. [3,5,6] using Eqs. (3-7).
The equilibrium constant and interaction energies for the
alloy Cu3Pd in liquid state at 1350 K are found to be
k = 0.125, 12RT

= –0.467, 13 RT

= –1.9 and
23 RT

= –3.47
The calculated and observed value of integral excess
free energy of mixing is in excellent agreement (Figure
1). The calculated integral excess free energy of mixing
is minimum (– 6.57 kJ·mol–1) at xCu = 0.7 which almost
matches with the observed value [13]. The observed
asymmetry in integral excess free energy of mixing is
well explained by our theoretical model.
We have observed that if the interaction energies are
supposed to be independent of temperature, i.e.,
12 0
T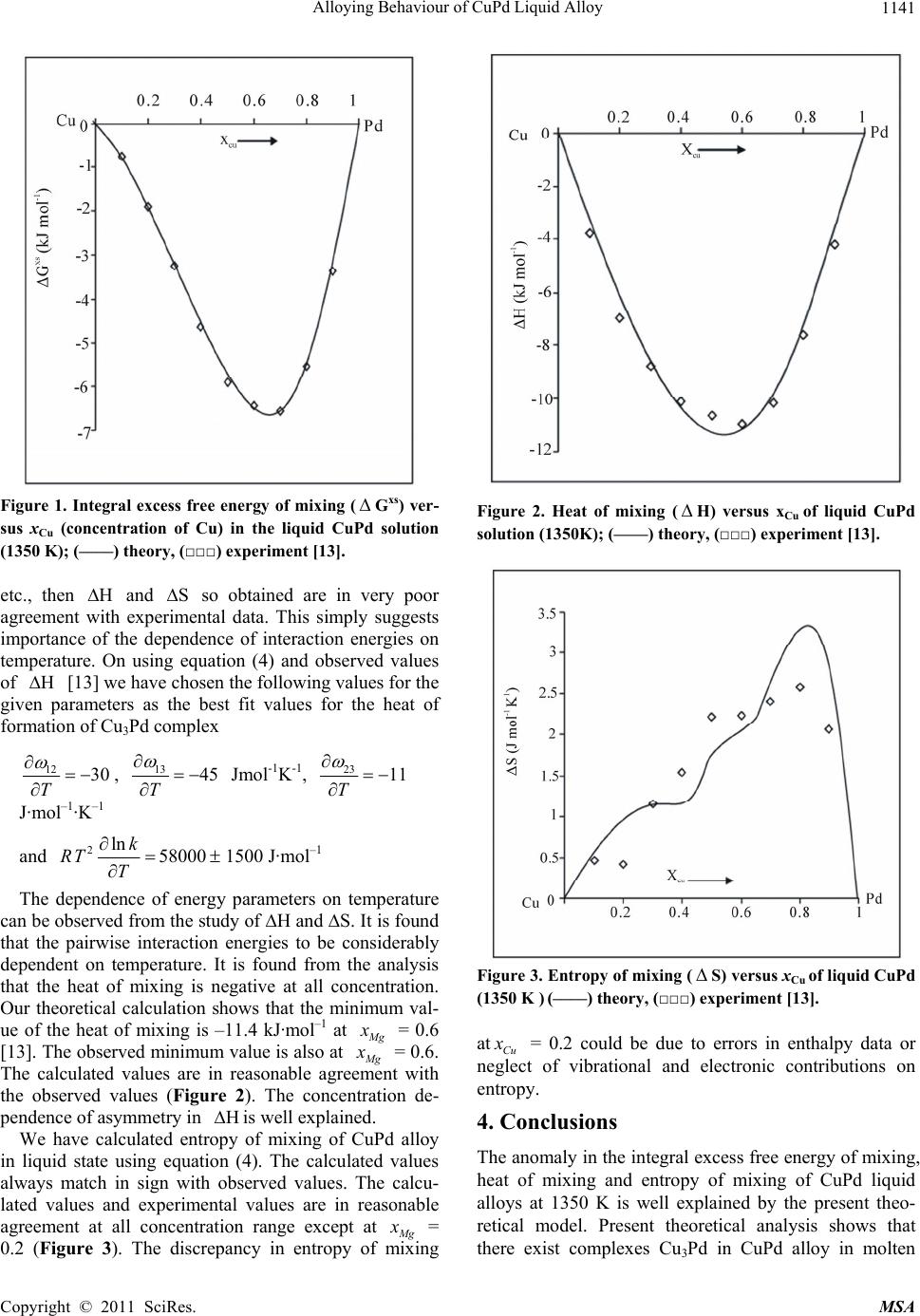
 


Alloying Behaviour of CuPd Liquid Alloy1141
Figure 1. Integral excess free energy of mixing (

Gxs) ver-
sus xCu (concentration of Cu) in the liquid CuPd solution
(1350 K); (––––) theory, (□□□) experiment [13].
etc., then and so obtained are in very poor
agreement with experimental data. This simply suggests
importance of the dependence of interaction energies on
temperature. On using equation (4) and observed values
of [13] we have chosen the following values for the
given parameters as the best fit values for the heat of
formation of Cu3Pd complex
HS
H
12 30
T
 
, 13 45
T
 
 Jmol-1K-1, 2311
T
 



J·mol–1·K–1
and 2lnk
RT T

58000 1500 J·mol–1

The dependence of energy parameters on temperature
can be observed from the study of ∆H and ∆S. It is found
that the pairwise interaction energies to be considerably
dependent on temperature. It is found from the analysis
that the heat of mixing is negative at all concentration.
Our theoretical calculation shows that the minimum val-
ue of the heat of mixing is –11.4 kJ·mol–1 at
M
g
x
= 0.6
[13]. The observed minimum value is also at
M
g
x
= 0.6.
The calculated values are in reasonable agreement with
the observed values (Figure 2). The concentration de-
pendence of asymmetry in is well explained.
H
We have calculated entropy of mixing of CuPd alloy
in liquid state using equation (4). The calculated values
always match in sign with observed values. The calcu-
lated values and experimental values are in reasonable
agreement at all concentration range except at
M
g
x
=
0.2 (Figure 3). The discrepancy in entropy of mixing
Figure 2. Heat of mixing (

H) versus xCu of liquid CuPd
solution (1350K); (––––) theory, (□□□) experiment [13].
Figure 3. Entropy of mixing (S) versus xCu of liquid CuPd
(1350 K ) (––––) theory, (□□□) experiment [13].

at Cu
x
= 0.2 could be due to errors in enthalpy data or
neglect of vibrational and electronic contributions on
entropy.
4. Conclusions
The anomaly in the integral excess free energy of mixing,
heat of mixing and entropy of mixing of CuPd liquid
alloys at 1350 K is well explained by the present theo-
retical model. Present theoretical analysis shows that
there exist complexes Cu3Pd in CuPd alloy in molten

Alloying Behaviour of CuPd Liquid Alloy 1142
state and the pairwise interaction energies are tempera-
ture dependent.
REFERENCES
[1] B. P. Singh, D. Adhikari and I. S. Jha, “Concentration
Dependence of the Structure and Thermodynamic Proper-
ties of Silver-Antimony Alloys,” Journal Non-Crystals
Solids, Vol. 356, No. 3-4, 2010, pp. 1730-1734.
doi:10.1016/j.jnoncrysol.2010.06.004
[2] S. Lele and P. Ramchandrarao, “Estimation of Complex
Concentration in a Regular Associated Solution,” Metal-
lurgical Transactions B, Vol. 12B, 1981, pp. 659-666.
doi:10.1007/BF02654134
[3] D. Adhikari, I. S. Jha and B. P. Singh, “Thermodynamic
and Microscopic Structure of Liquid Cu-Sn Alloys,” Phy-
sica B, Vol. 405, No. 7, 2010, pp. 1861-1865.
doi:10.1016/j.physb.2010.01.064
[4] A. S. Jordan, “A Theory of Regular Associated Solution
Applied to the Liquidus Curves of the Zn-Te and Cd-Te
Systems,” Metallurgical Transactions, Vol. 1, 1970, pp.
239-249.
[5] D. Adhikari, B. P. Singh, I. S. Jha and B. K. Singh,
“Thermodynamic Properties and Microscopic Structure of
Liquid Cd-Na Alloys by Estimating Complex Concentra-
tion in a Regular Associated Solution,” Journal Molecu-
lar Liquids, Vol. 156, No. 2-3, 2010, pp. 115-119.
doi:10.1016/j.molliq.2010.05.020
[6] D. Adhikari, I. S. Jha and B. P. Singh, “Structural
Asymmetry in Liquid Fe-Si Alloys,” Philosophical Mag-
azine, Vol. 90, No. 20, 2010, pp. 2687-2694.
doi:10.1080/14786431003745302
[7] V. Milekhine, M. I. Onsoien, J. K. Solberg and T. Skal-
and, “Mechanical Properties of FeSi (ε), FeSi2 (ζα) and
Mg2Si,” Intermetallics, Vol. 10, No. 8, 2002, pp. 743-750.
doi:10.1016/S0966-9795(02)00046-8
[8] A. B. Bhatia and D. E. Thornton, “Structural Aspects of
the Electrical Resistivity of Binary Alloys,” Physics Re-
viwes B, Vol. 8, No. 2, 1970, pp. 3004-3012.
doi:10.1103/PhysRevB.2.3004
[9] K. Hoshino and W. H. Young, “Entropy of Mixing of
Compound Forming Liquid Binary Alloys,” Journal
Physics F, Vol. 10, No. 7, 1980, pp. 1365-1376.
doi:10.1088/0305-4608/10/7/006
[10] S. P. McAlister and E. D. Crozier, “The Concentra-
tion-Concentration Correlation Function in the Long-
Wavelength Limit for Binary Liquid Alloys,” Journal of
Physics C, Vol. 7, No. 19, 1974, p. 3509.
[11] I. S. Jha, R. N. Singh, P. L. Shrivastava and N. R. Mitra,
“Stability of HgNa and HgK Liquid Alloys,” Philosophi-
cal Magazine, Vol. 61, No. 1, 1990, pp. 15-24.
doi:10.1080/13642819008208649
[12] R. N. Singh and F. Sommer, “Segregation and Immisci-
bility in Liquid Binary Alloys,” Reports Progress Physics,
Vol. 60, No. 1, 1997, p. 57.
doi:10.1088/0034-4885/60/1/003
[13] R. Hultgren, P. D. Desai, D. T. Hawkins, M. Gleiser and
K. K. Kelley, “Selected Values of the Thermodynamic
Properties of Binary Alloys,” ASM, Metal Park, 1973.
[14] A. B. Bhatia and N. H. March, “Size Effects, Peaks in
Concentration Fluctuations and Liquidus Curves of
Na-Cs,” Journal Physics F: Meterial Physics, Vol. 5, No.
6, 1975, p. 1100.
doi:10.1088/0305-4608/5/6/011
[15] W. B. Pearson, “The Crystals Chemistry and Physics of
Metals,” Wiley-International Science, 1972.