Materials Sciences and Applicat ion, 2011, 2, 1076-1082
doi:10.4236/msa.2011.28145 Published Online August 2011 (http://www.SciRP.org/journal/msa)
Indentation Creep Behavior and Microstructure of
Cu-Ge Ferrites
Hesham Mohaned Z ak i1,2*, A li Mohamed Abdel-Daiem1,2, Yah ia Ibrahim Swi lem1,2, Fari d El-Tantawy3,
Fahad Maso ud A l-Marzouki1, Ahmed Ab dall ah A l-Ghamdi1, S al e h A l-Heniti1, Far ag S aid Al-Hazmi1,
T alal Sad aka Al-Harbi1
1Department of Physics, Facul ty of Science, King Abdulaziz University, Jeddah, Kingdom of Saudi Arabia ; 2De par t ment of Physics,
Facult y of Science, Zagazi g Unive r sit y, Zaga zig, Egypt;3Depar tment of Physics, Faculty of Science, Suez Canal University, Ismailia,
Egypt Department of Physics.
Received Februar y 26th, 2011; re vise d May 5th, 2011; accepted May 20th, 2011.
ABS TRACT
Cu-Ge ferrite was prepared using the standard ceramic method. The creep rate of polycrystalline Cu1+xGexFe2-2xO4
ferrite has been measured as a function of time at room temperature. It is found that the indentation length increases
with the i ncrease of both time and applied load. A regime of indiv idual cree p curves i s observed f or the f i rst and second
stages. It is not possible to record the third stage of the curve as usually happened in an ordinary creep test, because
fracture of the samples does not occur. The slope is found to increase wi th i ncreasing germanium and copper content in
the steady state region. The high value of n (st re ss exponent f act or ) indicates that the disloc ati on c reep is the dominate
mechanism. The porosity arrangements developed within the specimens were examined using optical microscope. The
resul ts are di scus se d with regard to models de scribi ng the role of the steady state creep rate of metals. The morphology
of the samples shows that t he porosity is increased by increasing bot h copper and germanium i ons.
Keywords: Ferrit es, Indentati on Creep, Microstructure
1. Introduction
Ferri tes—ceramic ferromagnetic materials have been
considered as highly important electronic materials for
more than half a cen tury.
Ceramic like ferromagnetic materials, which are
mainly composed of ferric oxide, α-Fe2O3, are called
“ferrites”. Although the saturation magn etization for fer-
rites is less than half that of ferromagnetic alloys, they
have advantages, such as applicability at higher frequen-
cy, greater heat resistance, higher corrosion resistance
an d lower cost. The pr act ical a ppl icat i on s of fer ri tes ha ve
been expa nded by compl et ely utiliz ing th ese adv antage s .
Individual creep curves generally show primary, sec-
ondary, and tertiary creep. The majority of the primary
creep is not recoverable. The best representation of the
data is one where the creep rate depends exponentially on
the str es s , rath er tha n on the traditional po w e r law.
The steady state rate equation for creep of crystalline
materi als is often represent ed by
/npQ RT
Ade σ
−−
∈=

(1)
where
∈

is th e str ain rate,
σ
is the applied stress, A is
a material parameter, d is the grain size, Q is the activa-
tion energy for creep, R is the gas constant, T is the tem-
per at ur e, n den ot es th e stres s expon ent fa ctor and p i s the
grain size exponent.
Nishikawal et al. [1], Nish ikawa and okamoto [2] dis-
cuss ed the control of deformation mechanisms for creep
unde r s ome con dit i on s by a na l yz in g str ess, gr ain s ize an d
temperature dependency of steady state strain rate. Ac-
cording to their study, at intermediate stresses, power
low creep is th e dominant mechanism giving size of n =
3 to 5 , p = 0 and Q = QL, in which QL is the activation
energy of the lattice diffusion. There are three distinct
deformation mechanisms which exhibit the stress depen-
dence. Two of them include the flow of vacancies from
surface s and gr a in boundari es under r ela ti ve c ompress ion.
In th e ca se that va can cies fl ow t hrough th e cr yst a l lat ti ce ,
p = 2, Q = QL (Nabarro-Her ring creep) [3,4]. In the ca se
th at th e va can cies flow al ong gr a in bounda r ie s, p = 3 and
Q = Qb, where Qb is the activation energy for grain
boundary diffusion (Coble creep) [5]. The third process
was reported firstly by Harper and Dorn [ 6 ] on alumi-
num, in volves dislocation, multiplication and motion.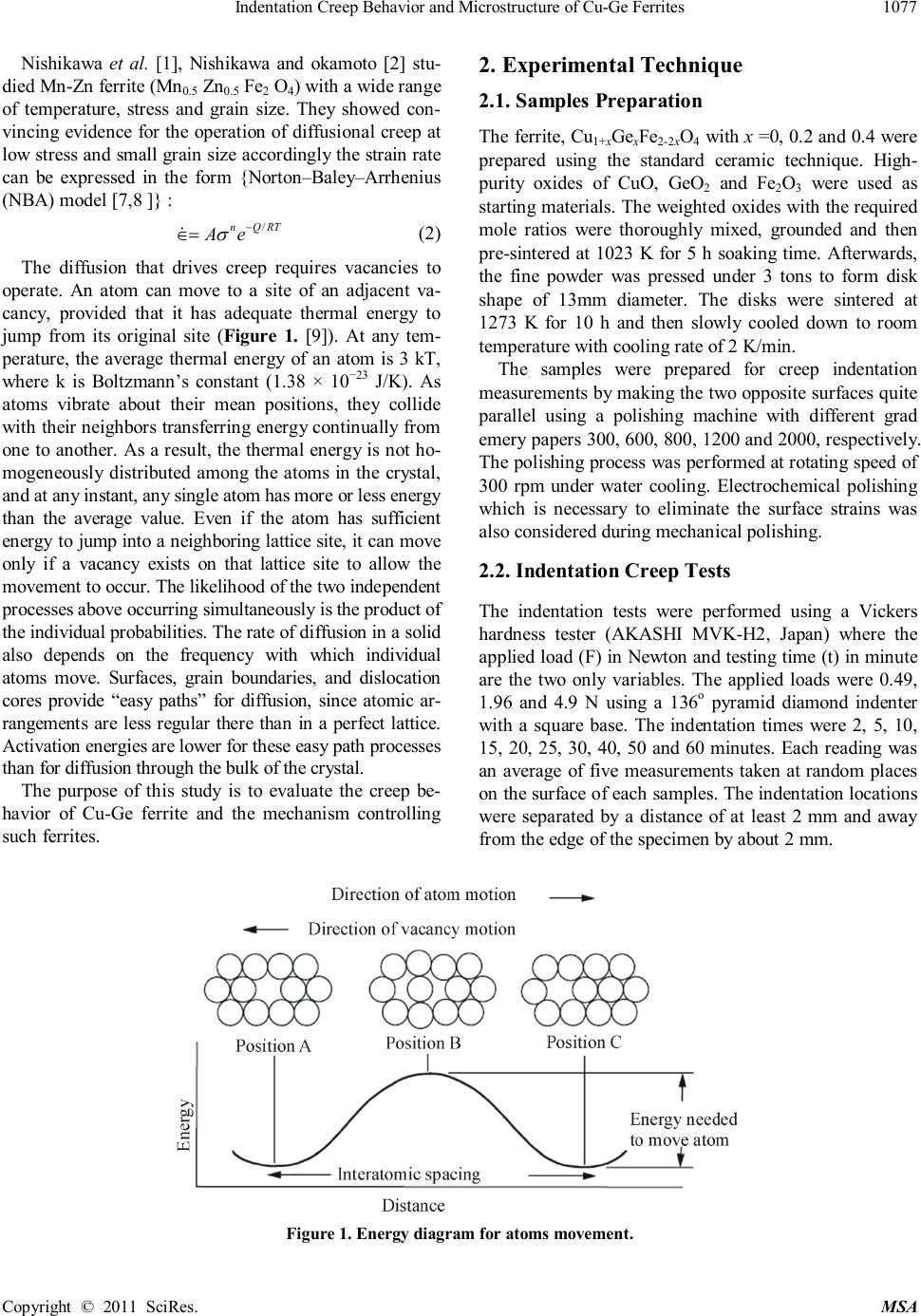

Indentation Cree p Behavior and Microstr ucture of C u -Ge F erri tes
1077
Nishikawa et al. [1], Nishikawa and okamoto [2] stu-
died Mn -Zn fer rite ( Mn0.5 Zn0.5 Fe2 O4) with a wide range
of temperature, stress and grain size. They showed con-
vin cing evidence for th e op eration of diffusional creep at
low st r ess an d sm al l grain siz e accor di ngly th e strain rate
can be expressed in the form {Norton–Baley–Arrhenius
(NBA) model [7,8 ]} :
/nQ RT
Ae σ
−
∈=

(2)
The diffusion that drives creep requires vacancies to
operate. An atom can move to a site of an adjacent va-
cancy, provided that it has adequate thermal energy to
jump from its original site (Figure 1. [9]). At any tem-
perature, the average thermal energy of an atom is 3 kT,
where k is Boltzmann’s constant (1.38 × 10−23 J/K). As
atoms vibrate about their mean positions, they collide
with their neighbors transferring energy continually from
one to another. As a result, the thermal energy is not ho-
mogeneousl y distributed among the atoms in the crystal,
and at any ins tant, any single a to m has more or less energy
than the average value. Even if the atom has sufficient
energy t o jump int o a n eighbor ing la ttice site, it can move
only if a vacancy exists on that lattice site to allow the
m ove ment to oc cur . The l ikelihood of the two independent
proces s e s above oc c urring si m ultaneous ly is t he product of
the individua l probabilities. The rate of diffusion in a s olid
also depends on the frequency with which individual
atoms move. Surfaces, grain boundaries, and dislocation
cores provide “easy paths” for diffusion, since atomic ar-
rangement s are less r egular ther e tha n in a perfect lattice.
Acti vati on energies are lower for these easy path processes
tha n for diff usion through the bu lk of the crysta l.
The purpose of this study is to evaluate the creep be-
havior of Cu-Ge ferrite and the mechanism controlling
such ferrites.
2. Experimental Technique
2.1. Samples Preparatio n
Th e ferrite, Cu1+xGexFe2-2xO4 with x = 0, 0. 2 and 0. 4 wer e
prepared using the standard ceramic technique. High-
purity oxides of CuO, GeO2 and Fe2O3 were used as
star tin g mat erial s. The wei ght ed oxides with th e requir ed
mole ratios were thoroughly mixed, grounded and then
pre-sintered at 1023 K for 5 h soaking time. Afterwards,
the fine powder was pressed under 3 tons to form disk
shape of 13mm diameter. The disks were sintered at
1273 K for 10 h and then slowly cooled down to room
temperature with cooling rate of 2 K/min.
The samples were prepared for creep indentation
measurements by makin g the two opposite surfaces quite
parallel using a polishing machine with different grad
emery papers 300, 600, 800, 1200 an d 2000, respectively.
Th e pol i shi n g pr oc ess wa s perform ed at rotating speed of
300 rpm under water cooling. Electrochemical polishing
which is necessary to eliminate the surface strains was
also considered during mechanical polishing.
2.2. Indentation Creep Tests
The indentation tests were performed using a Vickers
hardness tester (AKASHI MVK-H2, Japan) where the
applied load (F) in Newton and testing time (t) in minute
are the two only variables. The applied loads were 0. 49,
1.96 and 4.9 N using a 136o pyramid diamond indenter
with a square base. The indentation times were 2, 5, 10,
15, 20, 25, 30, 40, 50 and 60 minutes. Each reading was
an average of five measurements taken at ran dom places
on the surface of each samples. The indentation locations
were separated by a distance of at least 2 mm and away
fr om the edge of the speci m en by about 2 mm.
Figure 1. En ergy diagram for atom s movem ent.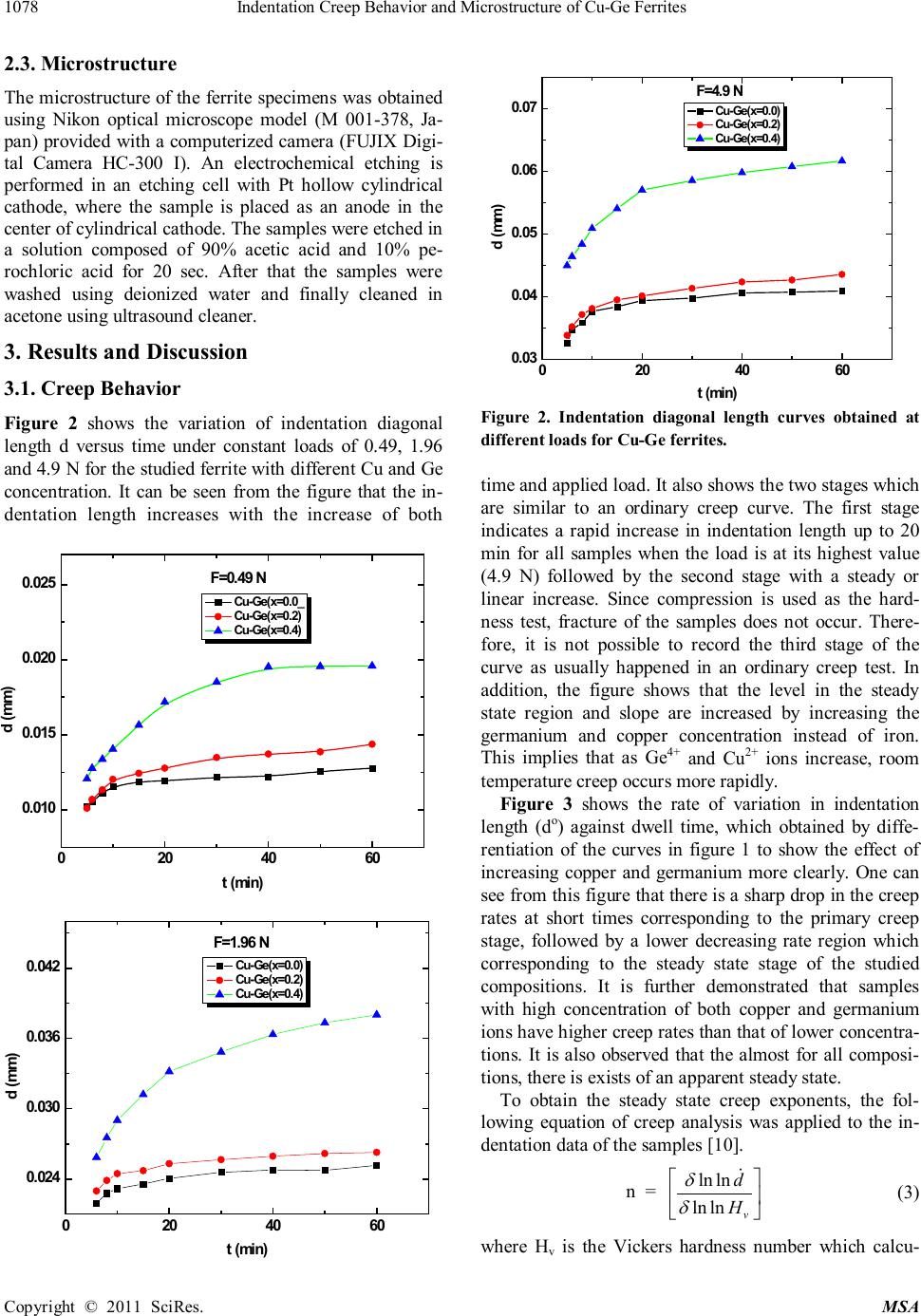

Indentation Cree p Behavior and Microstr ucture of C u -Ge F erri tes
1078
2.3. Micros t ructure
Th e mi cr ostr uctur e of the ferrite specimen s wa s obt ain ed
using Nikon optical microscope model (M 001-378, Ja-
pan) pr ovi ded with a computerized camera (FUJIX Digi-
tal Camera HC-300 I). An electrochemical etching is
performed in an etching cell with Pt hollow cylindrical
cathode, where the sample is placed as an anode in the
center of cylindrical cathode. Th e s amples were etched in
a solution composed of 90% acetic acid and 10% pe-
rochloric acid for 20 sec. After that the samples were
washed using deionized water and finally cleaned in
acetone using ul tras oun d cleaner.
3. Results and Disc ussion
3.1. Creep Behavior
Figure 2 shows the variation of indentation diagonal
length d versus time under constant loads of 0.49, 1.96
an d 4.9 N for the s tudi ed fer ri te with differ en t Cu and Ge
concen tr ation. It can be seen from the figure that th e in-
dentation length increases with the increase of both
Figure 2. Indentation diagonal length curves obtained at
different loads for Cu-Ge ferrites.
time an d applied load. It also shows th e two stages which
are similar to an ordinary creep curve. The first stage
indicates a rapid increase in indentation length up to 20
min for all samples when the load is at its highest value
(4.9 N) followed by the second stage with a steady or
linear increase. Since compression is used as the hard-
ness test, fracture of the samples does not occur. There-
fore, it is not possible to record the third stage of the
curve as usually happened in an ordinary creep test. In
addition, the figure shows that the level in the steady
state region and slope are increased by increasing the
germanium and copper concentration instead of iron.
This implies that as Ge4+ and Cu2+ ions increase, room
temperature creep occurs more rapidly.
Figure 3 shows the rate of variation in indentation
length (do) against dwell time, which obtained by diffe-
rentiation of the curves in figure 1 to show the effect of
increasing copper and germanium more clearly. One can
see from this figure that there is a sharp dr op in th e cr eep
rates at short times corresponding to the primary creep
stage, followed by a lower decreasing rate region which
corresponding to the steady state stage of the studied
compositions. It is further demonstrated that samples
with high concentration of both copper and germanium
ion s ha ve h igh er cr eep ra t es than tha t of lower concen tr a-
tions. It is also observed tha t the almost for all composi-
tion s, there is exists of an apparent steady state.
To obtain the steady state creep exponents, the fol-
lowing equation of creep analysis was applied to the in-
dentation data of th e sa mples [10].
n =
lnln
lnln
v
d
H
δ
δ




(3)
where Hv is the Vickers hardness number which calcu-
020 40 60
0.010
0.015
0.020
0.025
Cu- Ge (x=0.0_
Cu- Ge( x=0.2)
Cu- Ge( x=0.4)
d (mm)
t (min )
F=0.49 N
020 40 60
0.024
0.030
0.036
0.042
Cu- Ge( x=0.0)
Cu- Ge( x=0.2)
Cu- Ge( x=0.4)
d (mm)
t (min )
F=1.96 N
020 40 60
0.03
0.04
0.05
0.06
0.07
Cu- Ge( x=0.0)
Cu- Ge( x=0.2)
Cu- Ge( x=0.4)
d (mm)
t 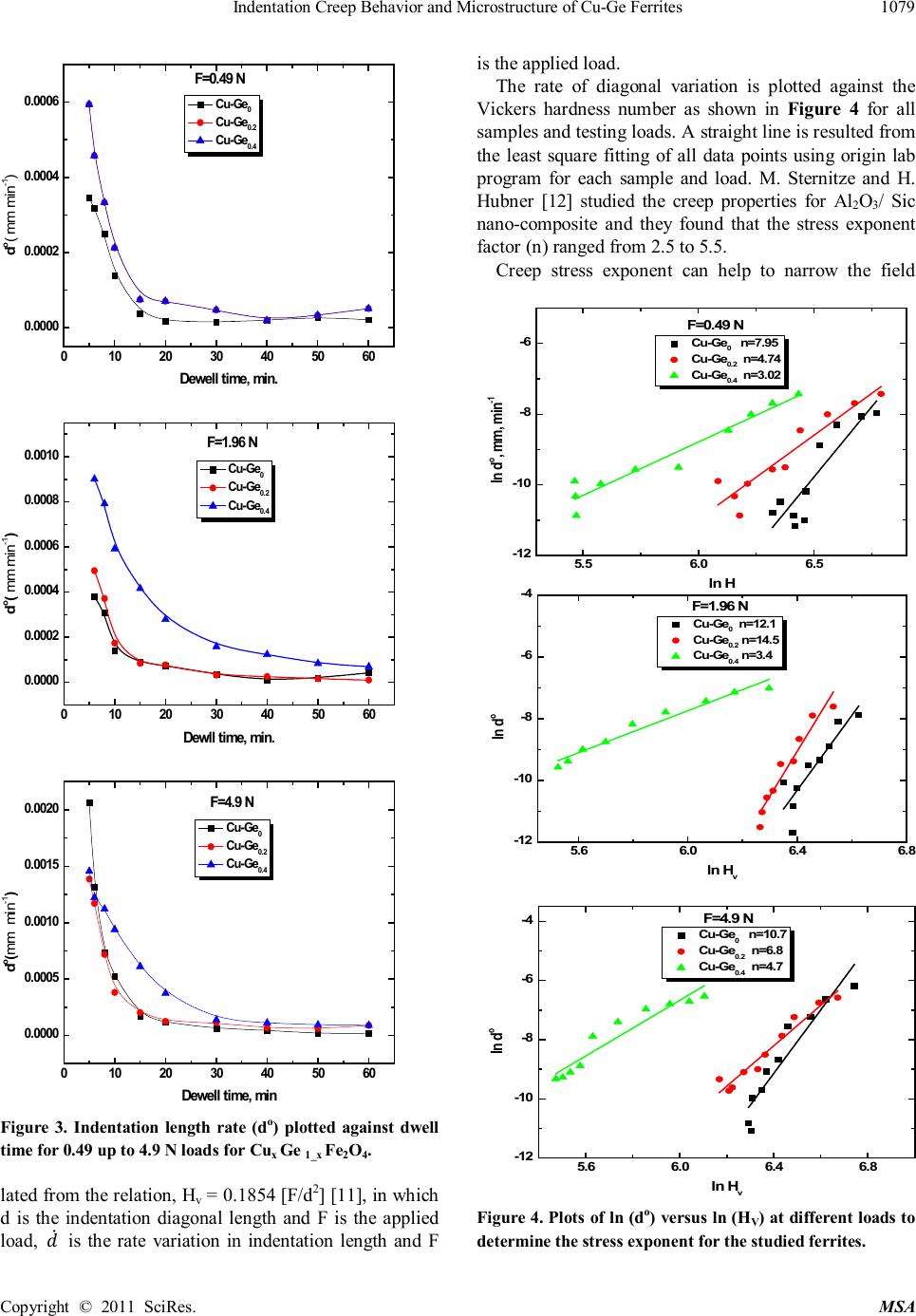(min )
F=4.9 N

Indentation Cree p Behavior and Microstr ucture of C u -Ge F erri tes
1079
Figure 3. Indentation length rate (do) plotted against dwell
t ime for 0.49 up t o 4.9 N loads for C ux Ge 1_x Fe2O4.
lated fr om the relation, Hv = 0. 1854 [F/d2] [11], in which
d is the indentation diagonal length and F is the applied
load, ̇ is the rate variation in indentation length and F
is the applied lo ad.
The rate of diagonal variation is plotted against the
Vickers hardness number as shown in Fi gure 4 for all
samples and testin g loads. A straight line is resulted from
the least square fitting of all data points usin g origin lab
program for each sample and load. M. Sternitze and H.
Hubner [12] studied the creep properties for Al2O3/ Sic
nano-composite and they found that the stress exponent
fa c tor (n ) r a nged fr om 2. 5 to 5. 5.
Creep stress exponent can help to narrow the field
Figure 4. Pl ots of ln (do) ve rs u s l n ( H V) at di ff eren t l oads to
determine the stress exponent for the studi ed ferrites.
010 20 30 40 50 60
0.0000
0.0002
0.0004
0.0006
d
o
( mm min
-1
)
D e w e ll time, min.
Cu-Ge
0
Cu-Ge
0.2
Cu-Ge
0.4
F=0.49 N
010 20 30 40 50 60
0.0000
0.0002
0.0004
0.0006
0.0008
0.0010
do( mm min-1)
D e w ll time, min.
Cu-Ge0
Cu-Ge0.2
Cu-Ge0.4
F=1.96 N
010 20 30 40 50 60
0.0000
0.0005
0.0010
0.0015
0.0020
do(mm min-1)
D e w e ll time, min
Cu-Ge0
Cu-Ge0.2
Cu-Ge0.4
F=4.9 N
5.5 6.0 6.5
-12
-10
-8
-6
ln d
o
, mm, min
-1
ln H
Cu-Ge0 n=7.95
Cu-Ge0.2 n=4.74
Cu-Ge0.4 n=3.02
F=0.49 N
5.6 6.0 6.4 6.8
-12
-10
-8
-6
-4
ln d
o
ln H
v
Cu-Ge
0
n=12.1
Cu-Ge
0.2
n=14.5
Cu-Ge
0.4
n=3.4
F=1.96 N
5.6 6.0 6.4 6.8
-12
-10
-8
-6
-4
F=4.9 N
Cu-Ge
0
n=10.7
Cu-Ge
0.2
n=6.8
Cu-Ge
0.4
n=4.7
ln d
o
ln H
v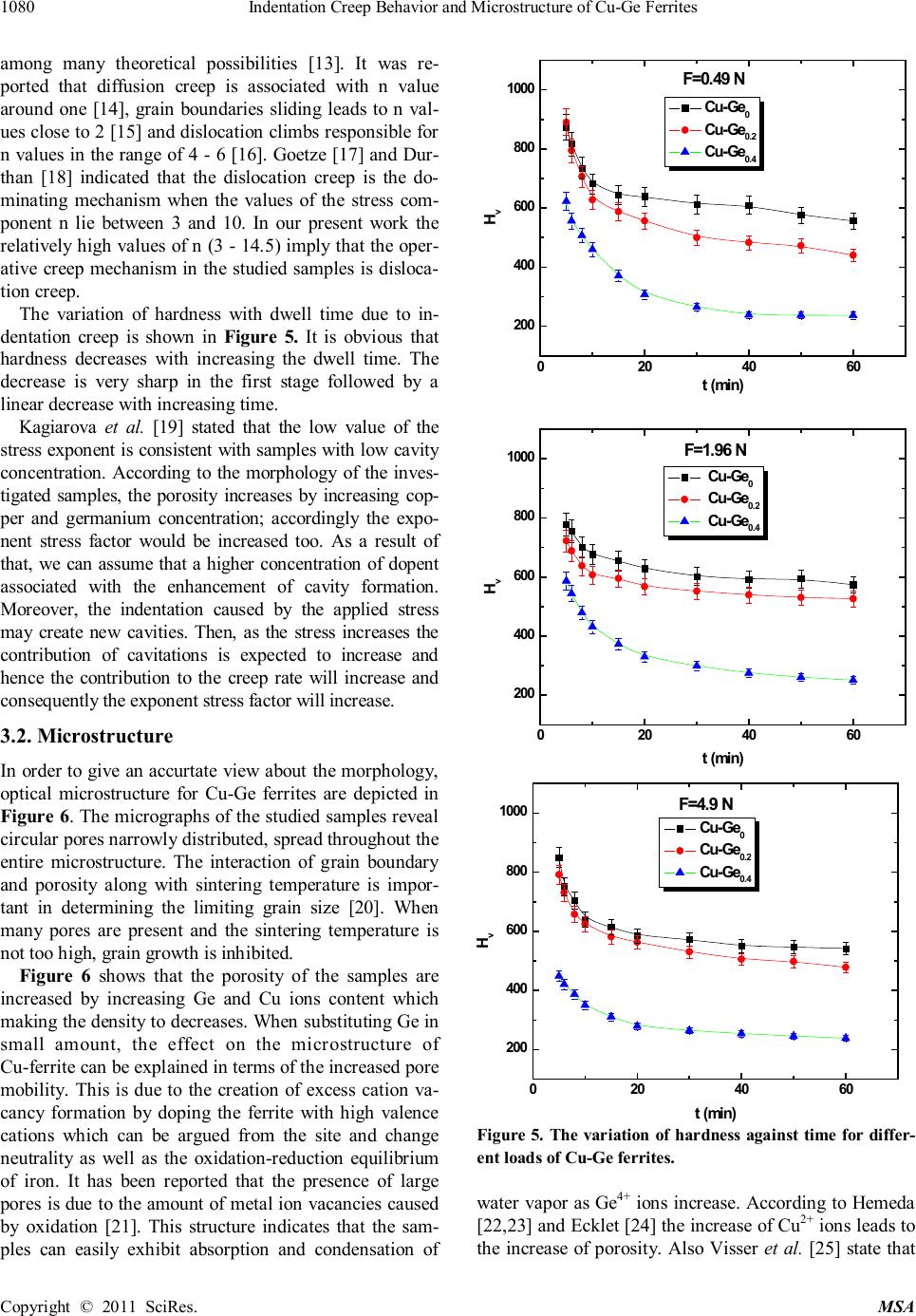

1080 Indentation Creep Behavior and Microstructure of Cu-Ge Ferrites
Copyright © 2011 SciRe s. M SA
among many theoretical possibilities [13]. It was re-
ported that diffusion creep is associated with n value
around one [14], grain boundaries sliding leads to n val-
ues close to 2 [15] an d dislocation climbs r esponsible for
n values in th e range of 4 - 6 [16]. Goetze [17] and Dur-
than [18] indicated that the dislocation creep is the do-
minating mechanism when the values of the stress com-
ponent n lie between 3 and 10. In our present work the
relatively high values of n (3 - 14.5) imply that the oper-
ative creep mechanism in the studied samples is disloca-
tion cr eep.
The variation of hardness with dwell time due to in-
dentation creep is shown in Figure 5. It is obvious that
hardness decreases with increasing the dwell time. The
decrease is very sharp in the first stage followed by a
linear de crea se wi th increasin g ti me.
Kagiarova et al. [19] stated that the low value of the
stress exponent is cons ist ent with samples with low ca vit y
concentration. According to the morphology of the inves-
tigated samples, the porosity increases by incr easing cop-
per and germanium concentration; accordingly the expo-
nent stress factor would be increased too. As a result of
that, we can assume that a higher concentration of dopent
associated with the enhancement of cavity formation.
Moreover, the indentation caused by the applied stress
ma y cr eate ne w cavi ties. Then, as the stress increases the
contribution of cavitations is expected to increase and
hence the contribution to the creep rate will increase and
con sequently the expon ent stress factor will increa se.
3.2. Micros t ructure
In order to give an accur t at e vi ew about the morphology,
optical microstructure for Cu-Ge ferrites are depicted in
Figure 6. Th e m icr ogra ph s of th e studied samples reveal
circular pores n arr owly distributed, sprea d throughout the
entire microstructure. The interaction of grain boundary
and porosity along with sintering temperature is impor-
tant in determining the limiting grain size [20]. When
many pores are present and the sintering temperature is
not too high, grain grow th is inhibited.
Figure 6 shows that the porosity of the samples are
increased by increasing Ge and Cu ions content which
making the density to decreases. When substituting Ge in
small amount, the effect on the microstructure of
Cu-fer r ite can be expl ained in term s of t he in creased pore
mobility. This is due to the creation of excess cation va-
cancy formation by doping the ferrite with high valence
cations which can be argued from the site and change
neutrality as well as the oxidation-reduction equilibrium
of iron. It has been reported that the presence of large
por es is due t o the am ount of met al ion vacan cies ca used
by oxidation [21]. This structure indicates that the sam-
ples can easily exhibit absorption and condensation of
Figure 5. The variation of hardness aga inst time for differ-
ent loads of Cu-Ge ferrites.
water vapor as Ge4+ ions increase. Accor din g t o Hemeda
[22,23] and E cklet [24] th e in cr ease of Cu2+ ion s leads to
the in crease of porosit y. Also Visser et al. [25] state th at
020 40 60
200
400
600
800
1000
Cu -Ge
0
Cu -Ge
0.2
Cu -Ge
0.4
H
v
t (min )
F=1.96 N
020 40 60
200
400
600
800
1000
C u-Ge
0
C u-Ge
0.2
C u-Ge
0.4
H
v
t (min )
F=4.9 N
020 40 60
200
400
600
800
1000
C u-Ge0
C u-Ge0.2
C u-Ge0.4
Hv
t (min )
F=0.49 N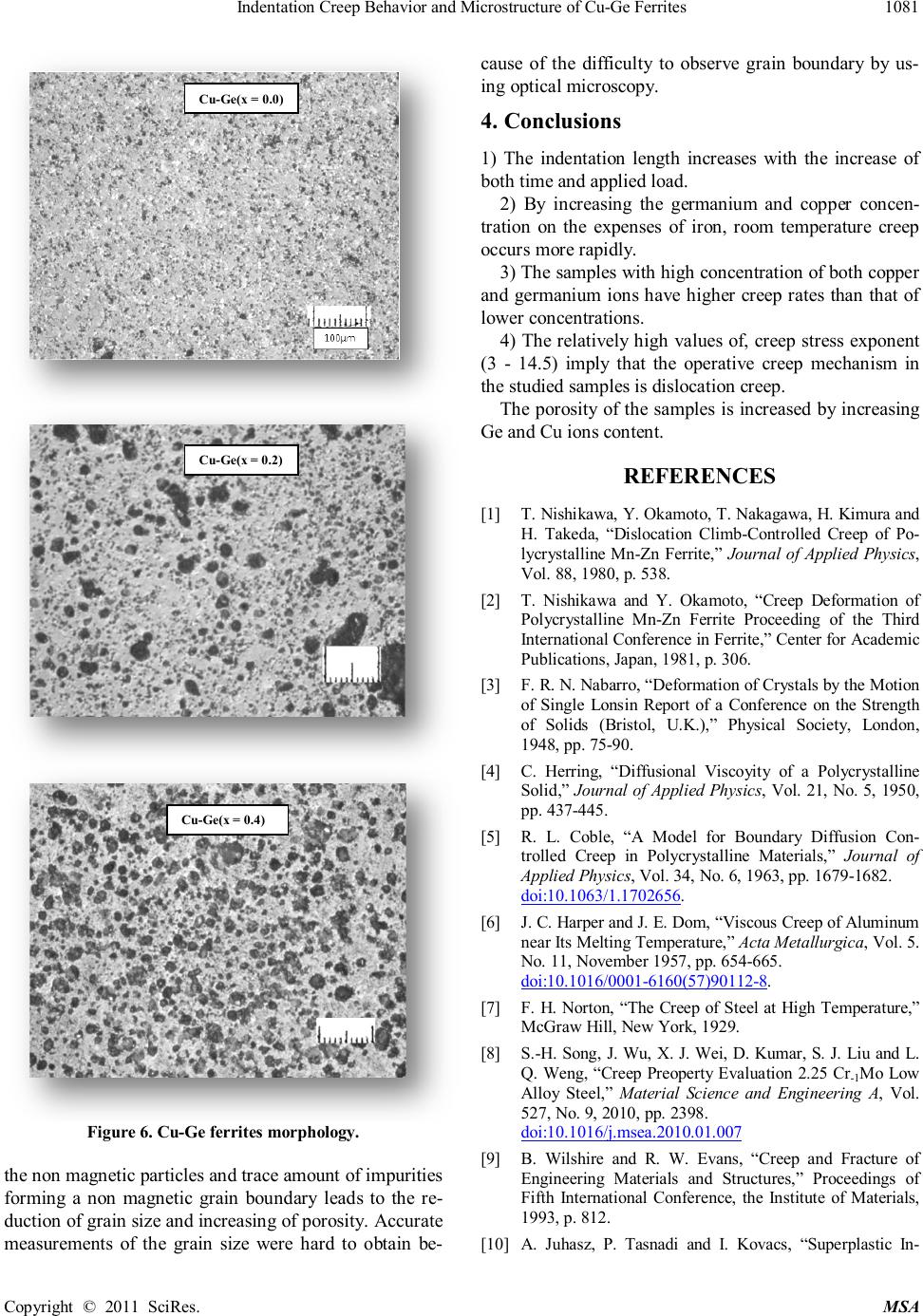

Indentation Cree p Behavior and Microstr ucture of C u -Ge F erri tes
1081
Figure 6. Cu-Ge ferrite s morphology.
the non magneti c particles an d trace am ount of im pu r ities
forming a non magnetic grain boundary leads to the re-
duction of grain size and in creasing of por osi ty. Accur at e
measurements of the grain size were hard to obtain be-
cause of the difficulty to observe grain boundary by us-
ing opti cal microscop y.
4. Conclusions
1) The indentation length increases with the increase of
both time and applied lo ad.
2) By increasing the germanium and copper concen-
trati on on the expenses of iron, room temperature creep
occurs mo re rapidly.
3) Th e sam ples wi th hi gh con cen trat ion of bot h coppe r
an d germanium i on s ha ve h igh er cr eep r at es than that of
lower con centration s .
4) Th e r elatively h igh values of, creep str ess exponent
(3 - 14.5) imply that the operative creep mechanism in
the studied samples is dislocation creep.
The porosity of the samples is increased by increasing
G e a nd Cu ions c ont ent.
REFERENCES
[1] T. Nishika w a, Y. Okamoto, T. Nakagawa, H. Kimura and
H. Takeda, “Dislocation Climb-Controlled Creep of Po-
lycrystalline Mn-Z n Fer rite,” Journal of Applied Physics,
Vol. 88, 1980, p. 538.
[2] T. Nishikawa and Y. Okamoto, “Creep Deformation of
Polycrystalline Mn-Zn Ferrite Proceeding of the Third
Inter national Conference i n Fer ri te ,” Ce nt er for Acade mi c
Publications, Japan, 1981, p. 306.
[3] F. R. N. Nabarro, “De f ormation of Cr ys tal s by the Mot ion
of Single Lons in Report of a Conference on the Strength
of Solids (Bristol, U.K.),” Physical Society, London,
1948, pp. 75-90.
[4] C. Herring, “Diffusional Viscoyity of a Polycrystalline
Solid,” Journal of Applied Physics, Vol. 21, No. 5, 195 0,
pp. 437-445.
[5] R. L. Coble, “A Model for Boundary Diffusion Con-
trolled Creep in Polycrystalline Materials,” Journal of
Applied Physi cs, V ol. 34 , No. 6, 1963, pp. 1679-1682.
doi:10.1063/1.1702656.
[6] J. C. Ha rper and J. E. D om , “ Viscous Cr eep of Aluminum
near Its Melting Temperature,” Ac ta Metal lurgica, Vol. 5.
No. 11, November 1957, pp. 654-665.
doi:10.1016/0001-6160(57)90112-8.
[7] F. H. Nort on, “T he Cr ee p of St eel at High Tempera tur e,”
McGr a w Hill, New York, 1929.
[8] S.-H. Song, J. Wu, X. J. Wei, D. Kumar, S. J. Liu and L.
Q. Weng , “Creep Pre oper ty Eva lua ti on 2.25 Cr -1Mo Low
Alloy Steel,” Material Science and Engineering A, Vol.
527, No. 9, 2010, pp. 2398.
doi:10.1016/j.msea.2010.01.007
[9] B. Wilshire and R. W. Evans, “Creep and Fracture of
Engineering Materials and Structures,” Proceedings of
Fifth International Conference, the Institute of Materials,
1993, p. 812.
[10] A. Juhasz, P. Tasnadi and I. Kovacs, “Superplastic In-
Cu-Ge(x = 0.0)
Cu-Ge(x = 0.2)
Cu-Ge(x = 0.4)

1082 Indentation Creep Behavior and Microstructure of Cu-Ge Ferrites
Copyright © 2011 SciRe s. M SA
dentation Creep of Lead-Tin Eutectic,” Journal of Ma-
terial Science Letters, Vol. 5, No. 1, 1986, pp. 535-536.
[11] R . Roumina, B. Ra e isinia and R. Mahmudi, “Room Tem-
perature Indentation Creep of Cast Pb-Sb Alloys,” Sci-
ence Material, Vol . 51, 2004, p. 497.
doi:10.1016/j.scriptamat.2004.05.048.
[12] M. Sternitzke and H. Hubner, “Creep Mechanisms Of
Alumina/Sic Nanocomposites Ceramic Engineering and
Science Proceedings,” Mrityunjay Singh & Todd Jessen,
The American Ceramic Society, 2001, p. 145.
[13] A. R. Geranmayeh, R. Mahmudi, “Room-Temperature
Indentation Creep of Lead Free Alloy,” Journal of Elec-
tricity Material, 2005, Vol. 34, pp. 1002-1009.
[14] T. G. Langdon, “Identifying Creep Mechanisms at Low
Stresses,” Material Science Engeneering, Vol. A283,
2000, pp. 266-273.
[15] B. Walser and O. D. Sherby, “Structure Dependence of
Power Law Creep,” Scripta Metall, Vol. 16 , No. 2, 1982,
pp. 213-219. doi:10.1016/0036-9748(82)90389-1
[16] G. Sharma, R. V. Ramanujan, T. R. G. Kutty and G. P.
Tiwari, “Hot Hardness and Indentation Creep Studies of a
Fe-28Al-3Cr-0.2C Alloy,” M aterial Science Engeneering,
Vol. A278, 2000, pp.106-112.
[17] C. Goetze and W. F. Brace, “Laboratory Obs ervations of
High-Temperature Rheology of Rocks,” Tectonophysics,
Vol. 113, No. 1-4, 1972, pp. 583-600.
doi:10.1016/0040-1951(72)90039-X
[18] D. L. Kohlstedt and C. Goetze, “Low-Stress High Tem-
perature Creep in Olivine Single Crystals,” Journal of
Geophysial Research, Vol. 79, 1974, p. 2045.
[19] M. Kagiarova, W. Z. Barbara, Y. Shollock, Y. A. Boc-
ca ccini and J. Dusza, “M icrostructure and C reep B ehavior
of a Si3N4-SiC Microna nocomp osite,” Journal of Ameri-
can Ceramic Society, Vol. 92, No. 2, 2009, pp. 439-444.
doi:10.1111/j.1551-2916.2008.02848.x
[20] W. D. Kingery, H. K. Bowen and D. R. Uhimann, “In-
troduction t o Cera mi c Science, ” Wile y, New York, 1975,
p. 474.
[21] J. Smith, “Magnetic Properties of Materials,” McGraw
Hill, New York, 1971.
[22] D. M. Heme da and O. M. Hemeda, “Electrical, Structural,
Magnetic and Transport Properties of Zn2BaFe 16O27
Doped with Cu2+,” Journal Magnetism Magnetic Matte-
rials, Vol. 320, No. 8, 2008, pp. 1557-1562.
doi:10.1016/j.jmmm.2008.01.006
[23] D. M. Heme da and O. M. Hemeda, “Electrical, S t ructural,
Magnetic and Transport Properties of Zn2 Ba Fe 16O27
Doped with C u2+,” American Journal of A pp li ed Sciences,
Vol. 5, No. 4, 2008, pp. 289- 295.
doi:10.3844/ajassp.2008.289.295
[24] J. Eckelt, C. Bottger and J. Hesse, “AC Susceptibility in
the Reentrant Spin-Glass System (Fe0.65Ni0.35)1-xMn,”
Journal Magnetism Magnetic Matterials, Vol. 104, No. 3,
1992, pp.1665-1667. doi:10.1016/0304-8853(92)91502-K
[25] E. G. Visser, M. T. Johnson, P. J. V. D. Zaag, “Ferrites:
Production,” International Flavour-6, Japan, 1992, p. 807.