 Applied Mathematics, 2011, 2, 1031-1038 doi:10.4236/am.2011.28143 Published Online August 2011 (http://www.SciRP.org/journal/am) Copyright © 2011 SciRes. AM On the Behavior of Solutions of the System of Rational Difference Equations 1 11 11 , , 11 nn nnn nnnn nn n x xy z xx z 1 1 1 Abdullah Selçuk Kurbanlı1, Cengiz Çinar2, Mehmet Emre Erdoğan3 1Mathematics Department, Education Faculty, Selçuk University, Konya, Turkey 2Mathematics Department, Education Faculty, Gazi University, Ankara, Turkey 3Huglu Vocational High School, Selçuk University, Konya, Turkey E-mail: agurban@selcuk.edu.tr, ccinar25@yahoo.com, m_emre448@hotmail.com Received April 29, 2011; revised May 16, 2011; accepted May 24, 2011 Abstract In this paper, we investigate the solutions of the system of difference equations 1 1 1 , 1 n nnn x xyx 1 1 1 , 1 n nnn y yxy 1 1 n nnn zyz where 010101 ,,,,,xxyy zz . Keywords: Difference Equation, Difference Equation Systems, Solutions 1. Introduction Recently, there has been great interest in studying diffe- rence equation systems. One of the reasons for this is a necessity for some techniques which can be used in investigating equations arising in mathematical models describing real life situations in population biology, economic, probability theory, genetics, psychology etc. Although difference equations are very simple in form, it is extremely difficult to understand throughly the global behavior of their solutions; for example, see Refs. [1-28]. In this paper, we investigated the periodicity of the solutions of the difference equation system 11 111 11 , , 11 nn nnn nn nnnn1 n yx xyz yx xy yz f (1.1) where the initial conditions are arbitrary real numbers. 2. Main Results Theorem 1. Let 010101 ,,,,,yay bxcx dzez ,, nnn be arbitrary real numbers and let yz be a solutions of the system (1.1). Also, assume that and 1ad 1cb , 0,0, 0abe and 0f . Then all solutions of (1.1) are , 1 1, n n n dnodd n even ad x c cb (1.2) , 1 1, n n n bn cb y a ad odd n even (1.3) 1 1 1 1 , ,4 ,4 ,4 1 1 1 1 14 3, 1 1,3, 1 13, 1 12,3, 1 n n n n nn n n n ccbnk af ad dcb nk be ad zfcb nk ad ecb nk ad 1, 2, 3, 2,1,2 1,1,2, ,1, k k k k (1.4)  1032 A. S. KURBANLI ET AL. Proof: For we have 1,2, 3,n 1 1 0111 xd xyx ad 1 1 0111 yb yxy cb 0 1 01 xc zyz af , 1 1 1 1 01 01 2 cbc c cb bc xy x x 0 2 10 1 11 1 ya ya d xy a ad ad 1 2 10 1 1 1 1 ddcb xad zb yzbe ad e cb , 1 32 21 1 11 11 1 d xd ad xd yx ad aadad , 1 32 21 1 11 11 1 b yb cb yb xy cb ccbcb 2 3 21 11 1 1 ccbf cb x zc yz ad aadaf , 2 4 32 2 2 11 111 1 1 1 1 ccbccb x xbc yx ccb cb cb ccb b 2 4 32 2 2 11 111 1 1 1 1 aadaad y yd xy aadad ad aad ad 2 3 4 32 2 11 11 1 1 d ade cb x zdcb yz ad b be ad cb for assume that nk 23 21 222311 k kk kk xd xyx ad 22 2 2122 1 1 k k kkk x xc yx cb 23 21 222311 k kk kk yb yxy cb 22 2 2122 1 1 k k kkk y ya xy ad and 1 44 43 1 4445 1 1 k k kk kk ccb x zyz af ad 43 42 434 4 1 1 k k kk kk dcb x zyz be ad 42 41 4243 1 1 k k kk kk fcb x zyzad 41 4 4142 1 1 k k kk kk ecb x zyz ad are true. Then 1nk we will show that (1.2), (1.3) and (1.4) are true. From (1.1), we have 21 21 221 1 1 111 1 1 k k kk kk k k d ad x xd yx aadad d ad 21 21 221 1 1 111 1 1 k k kk kk k k b cb y yb xy ccbcb b cb . Also, similarly from (1.1), we have 2 4 41 441 2 11 11 11 kk k kkk kk k k ccbccb x zyz fcb afad aad ad 21 1 41 42 1 414 21 11 11 11 kk k kkk kk kk d add cb x zyz ecb bead b cb ad Copyright © 2011 SciRes. AM  A. S. KURBANLI ET AL. 1033 Also, we have 2 22 2121 1 1 11 11 1 1 1 kk k kk kk k k ccbccb x xbb yx ccbc cb cb ccb 1 2 22 212 1 1 11 111 1 1 1 kk k kk kk k k aad aad y ydd xy aad a ad ad aad 1 and 21 42 43 4241 21 1 1 1 1 11 1 1 k k kk kk k k k k ccb x zyz ccb aad af ad fcb ad 22 43 44 1 4342 22 1 1 1 1 1 11 1 1 k k kk kk kk k k d ad x zyz dcb b cbbe ad ecb ad Corollary 1. Let be arbitrary real num-,,,,,abcde f n bers and let ,, nn yz be a ,,,,cde f solution of the system (1.1). If 0, 1a then we have my b 21 21 lim li nn nn x 43 0, lim , , n n cb ad zcbad 41 lim , , n nzc b ccb ad af , 0, cb ad ad cb ad and 22 lim lim 0 nn nn xy adcb, be dadcb, adcb,0 zlim 2n4 n , 4 lim , , n nzc b ecbad 0, cb ad ad Proof. From 1 we have 0 0,,,abcd 01ad 11 ad and 00111cb cb Hence, we obtain 21 1 lim n x lim lim 11 , ., nn nn n dd ad ad nodd dn even 21 1 lim limlim 11 , ., nnn nn n d yb cb cb nodd bn even and 11 43 1 11 lim limlim1 1 1 11 1 1 11 1 1 11 1 0, , , nn nn nn n ccbccb zaf ad af ad cb ad cbadcbad cb ad cbadcbad cb ad cbadcbad cb ad cb ad ccb ad af 1 1 1 41 11 lim limlim1 1 1 11 1 1 11 1 1 11 1 0, , , nn nn nn n fcb ab zf ad ad cb ad cbadcbad cb ad cbadcbad cb ad cbadcbad cb ad cb ad fcbad 1 1 1 Similarly, we have 2 limlim n xccd 1lim10 0 nn nn n ccdc Copyright © 2011 SciRes. AM 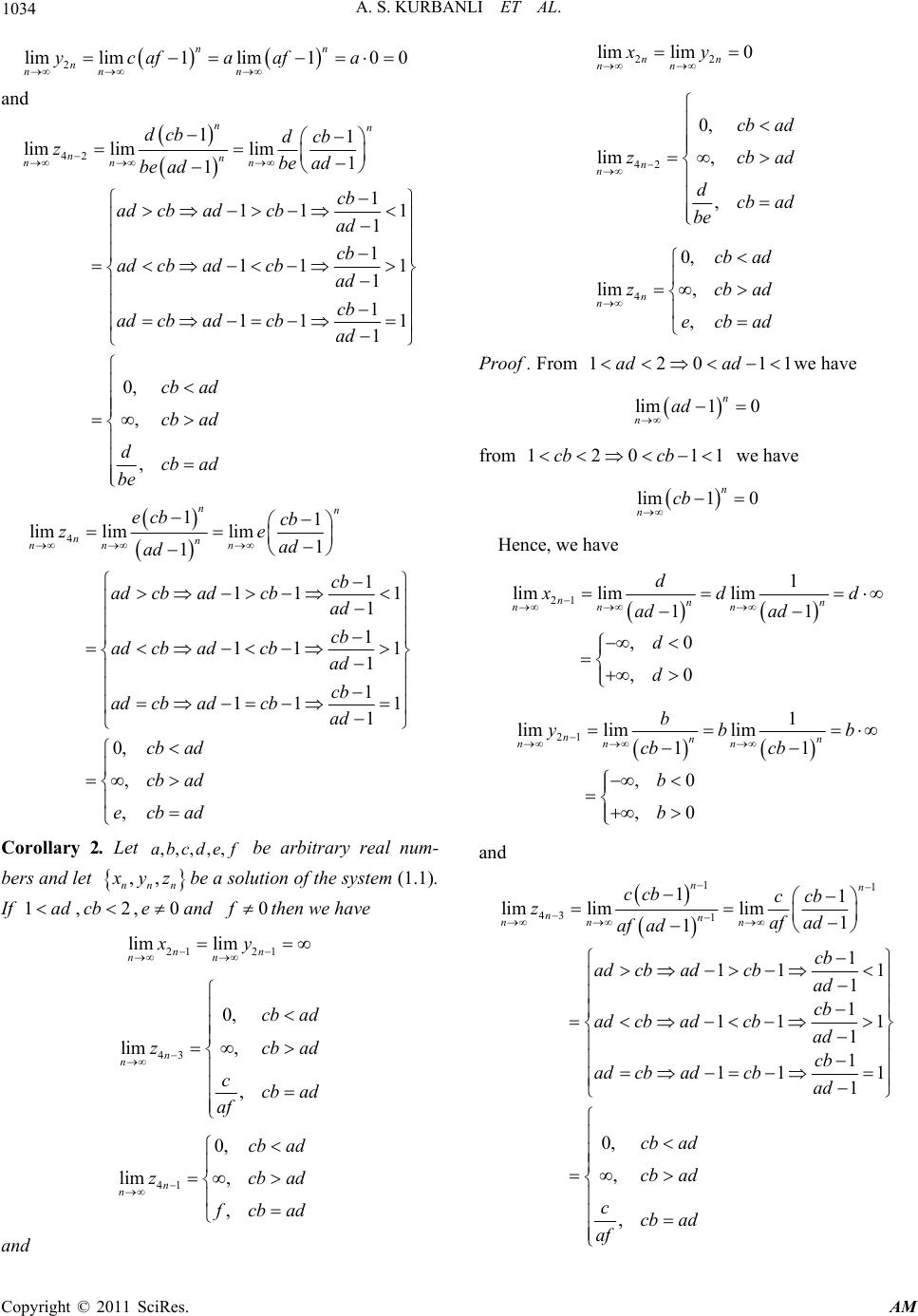 A. S. KURBANLI ET AL. 1034 and 2 limlim1lim10 0 nn n nn n ycafaafa 42 11 lim nn limlim 1 1 1 111 1 1 11 1 1 1 111 1 0, , , nn nnn dcb dcb zbe ad be ad cb ad cbadcbad cb ad cbadcbad cb ad cbadcbad cb ad cb ad dcb ad be 4 11 lim limlim1 1 1 11 1 1 11 1 1 11 1 0, , , nn nn nn n ecb cb ze ad ad cb ad cbadcbad cb ad cbadcbad cb ad cbadcbad cb ad cb ad ecbad 1 1 1 Corollary 2. Let be arbitrary real num- bers and let ,,,,,abcde f ,, nnn yz be a sol 0and f ution of the system (1.1). If 1ad,2cb ,e0 then we have 1 1 n 2 2 lim lim nn n xy 43 0, lim , , n n cb ad zcb ccb ad af ad ad 41 0, lim , , n n cb ad zcb cb ad 22 lim lim 0 nn nn xy 42 0, lim , , n n cb ad zcb dcb ad be ad ad Proof . From 1 4 0, lim , , n n cb ad zcb ecbad 1201ad ad we have from 1 lim1 0 n nad 1201cb cb we have Hence, we have lim1 0 n ncb 21 1 limlimlim 11 ,0 ,0 nnn nnn d dd ad ad d d 21 1 lim limlim 11 ,0 ,0 nnn nn n b yb cb cb b b b and 11 43 1 11 lim limlim1 1 1 11 1 1 11 1 1 11 1 0, , , nn nn nn n ccbccb zaf ad af ad cb ad cbadcbad cb ad cbadcbad cb ad cbadcbad cb ad cb ad ccb ad af 1 1 1 and Copyright © 2011 SciRes. AM  A. S. KURBANLI ET AL. 1035 41 11 lim limlim1 1 1 11 1 1 11 1 1 11 1 0, , , nn nn nn n fcb cb zf ad ad cb ad cbadcbad cb ad cbadcbad cb ad cbadcbad cb ad cb ad fcbad 1 1 1 Similarly, we have and 2 limlim1lim10 0 nn n nn n xccbccbc 2 limlim1lim100 nn n nn n yaadaada 42 11 lim limlim1 1 1 11 1 1 11 1 1 11 1 0, , , nn nn nnn dcb dcb zbe ad be ad cb ad cbadcbad cb ad cbadcbad cb ad cbadcbad cb ad cb ad dcb ad be 1 1 1 4 11 lim limlim1 1 1 11 1 1 11 1 1 11 1 0, , , nn nn nn n ecb cb ze ad ad cb ad cbadcbad cb ad cbadcbad cb ad cbadcbad cb ad cb ad ecbad ,, nnn yz 1 1 1 Corollary 3. Let be the solutions of (1.1). If 0,0e,,cbad and 0fthen 21 21 lim lim 0 nn nn xy 43 0, lim , , n n cb ad zcb ccb ad af ad ad 41 0, lim , , n n cb ad zcb cb ad and 22 lim lim nn nxy 42 0, lim , , n n cb ad zcb dcb ad be ad ad oof. From 1 4 0, lim , , n n cb ad zcb e c bad Pr cb 01cb and 101ad ad we have and Hence, we have , lim 1, n n nodd cb neven , lim 1, n n nodd ad n even 21 1 limlimlim0 0 11 nnn nnn d xd ad ad d 21 1 limlimlim00 11 nnn nn n b ybb cb cb and Copyright © 2011 SciRes. AM  1036 A. S. KURBANLI ET AL. 11 43 1 11 lim limlim1 1 1 11 1 1 11 1 1 11 1 0, , , nn nn nn n ccbccb zaf ad af ad cb ad cbadcbad cb ad cbadcbad cb ad cbadcbad cb ad cb ad ccb ad af 1 1 1 41 11 lim limlim1 1 1 11 1 1 11 1 1 11 1 0, , , nn nn nn n fcb cb zf ad ad cb ad cbadcbad cb ad cbadcbad cb ad cbadcbad cb ad cb ad fcbad 1 1 1 Similarly, we have 2 limlim1lim 1 ,0 ,0 ,0 ,0 nn n nn n ccb ccb c candnodd candnodd candn even candn even and 2 limlim1 lim 1 ,0 ,0 ,0 ,0 nn n nn n yaadaada aandnodd aandnodd aandn even aandn even 42 11 lim limlim1 1 1 11 1 1 11 1 1 11 1 0, , , nn nn nn n dcb dcb zbe ad be ad cb ad cbadcbad cb ad cbadcbad cb ad cbadcbad cb ad cb ad dcb ad 1 1 1 be 4 11 lim limlim1 1 1 11 1 1 11 1 1 11 1 0, , , nn nn nn n ecbcb ze ad ad cb ad cbadcbad cb ad cbadcbad cb ad cbadcbad cb ad cb ad ecbad 1 1 1 Corollary 4. Let , nn y be the solutions of (1.1). If and ad cb ,2, , and 0e 0f ,,ab ,cd then we have 21 21 lim lim 0 nn nn xy 43 0, lim , , n n cb ad zcb ccb ad af ad ad 41 0, lim , , n n cb ad zcb cb ad and 22 lim lim nn nn xy Copyright © 2011 SciRes. AM  A. S. KURBANLI ET AL. 1037 42 0, lim , , n n cb ad zcb dcb ad be ad ad Proof. The proof is clear from Corollary 3. 4. Acknowledgements to the a study. 5. References [1] A. S. Kurbanli, C. Cinar and I. Yalcinkaya, “On the Behavaior of Positive Solutions of the System of Rational Difference Equations 4 0, lim , , n n cb ad zcb ecbad We are grateful nonymous referees for their valuable suggestions that improved the quality of this 1 1 1 , 1 n nnn x xyx 1 1 11 n nnn y yxy ,” Mathematical and Computer Modelling, Vol. 53, No. 5-6, 2011, pp. 1261-1267. doi:10.1016/j.mcm.2010.12.009 [2] A. S. Kurbanli, “On the Behavior of Solutions of the System of Rational Difference Equations 1 1 1 , 1 n nnn x xyx 1 1 11 n nnn y yxy ,”World Applied Sciences Journal, 2010, in Press. . S. Kurbanli, “On the Behavior of Solutions of the System of Rational Difference Equations [3] A 1 1 11 n nnn x xyx , 1 1 11 n nnn y yxy , 1 1 11 n nnn z zyz ,” Discrete Dynamics in Nature and Society, 2011, in Press. doi:10.1155/2011/932362 [4] C. Cinar, “On the Solutions of the Difference Equation 1 1 1 1 n nnn x xax x ,” Applied Mathematics and Com- pulation, Vol. 158, 2004, pp. 793-797. [5] C. Çinar, “On the Positive Solutions of the Difference Equation System 1 1, nn xy 1 11 n nnn y y y ,” Applied 004, pp. Mathematics and Computation, Vol. 158, 2 303-305. doi:10.1016/j.amc.2003.08.073 [6] G. Papaschinopoulos and C Two Nonlinear Difference . J. Schinas, “On a System of Equations,” Journal of Mathematical Analysis and Applications, Vol. 219, No. 1998, pp. 415-426. doi:10.1006/jmaa.1997.5829 [7] G. Papaschinopoulos and C. J. Schinas, “On the System of Two Difference Equations,” Journal of Mathematical Analysis and Applications, Vol. 273, No. 2, 2002, pp. 0.1016/S0022-247X(02)00223-8294-309. doi:1 ] A. Y. Özban, “On the System of Rational Difference [8 Equations 3 1 3 , yn nn qnq aby xyxy nn ,” Applied Mathe- matics and Computa, 2007, pp. 833-837. tion, Vol. 188, No. 1 10.034doi:10.1016/j.amc.2006. uations,” Computers & Mathe- , 2002, pp. 49-867. nal Diserence Equation Mling Com Vol. 52, pp. 1765-1 4-8 [9] D. Clark and M. R. S. Kulenović, “A Coupled System of Rational Difference Eq matics with Applications, Vol. 43 [10] D. Clark, M. R. S. Kulenović and J. F. Selgrade, “Global Asymptotic Behavior of a Two-Dimensio odelpetition,” Nonlinear Analysis, , No. 7, 2003776. doi:10.1016/S0362-546X(02)0029 of Rate Equat [11] E. Camouzis and G. Papaschinopoulos, “Global Asymptotic Behavior of Positive Solutions on the System ional Differencions1, n nnm xy 1 x 11n nnm y yx ,” Applied Mathematics Letters, Vol. 17, No. 6, 2004, pp. 733-737. doi:10.1016/S0893-9659(04)90113-9 Liu and S. Bai, “On the System of High Order Rational Difference Equations [12] X. Yang, Y. , nnp a xy np n yby nq nq y 71, No. 2, 2005, pp ,” Applied Mathematics and Computation, Vol. 1. 853-856. doi:10.1016/j.amc.2005.01.092 [13] X. Yang, “On the System of Rational Difference Equations 1n nnp nq y xA y ,1n nnr ns x yA y ,” Journal of Mathematical Analysis and Applications, Vol. 307, No. 1, 2005, pp. 305-311. doi:10.1016/j.jmaa.2004.10.045 [14] M. R. S. Kulenović and Z. Nurkanović, “Global Behavior of a Three-Dimensional Linear Fractional System of Difference Equations,” Journal of Mathematical Analysis n, “On the Positive Rational Difference Equations and Applications, Vol. 310, No. 2, 2005, pp. 673-689. [15] A. Y. Özba Solutions of the System of 1 1 nnk y x, 1nn n y y mnmk xy al Analysis and Applications, Vol. 323, No. 1, 2006, pp. 26-32. ,” Journal of Mathematic doi:10.1016/j.jmaa.2005.10.031 [16] Y. Zhang, X. Yang, G. M. Megson and D. J. Evans, “On the System of Rational Difference Equations 1, nnp xA y 1n nnr ns y yA y ,” Applied Mathematics and Computation, Vol. 176, No. 2, 2006, pp. 403-408. doi:10.1016/j.amc.2005.09.039 Copyright © 2011 SciRes. AM  A. S. KURBANLI ET AL. Copyright © 2011 SciRes. AM 1038 stem Analysis, Vol. 16, No. 2, 2009, pp. 37-50. [23] R. Abu-Saris, C. Cinar and I. Yalcinkaya, “On the Asym- ptotic Stability of [17] Y. Zhang, X. Yang, D. J. Evans and C. Zhu, “On the Nonlinear Difference Equation Sy 1, n ny xA m n 1nnk naxx 1nm nn yA y nn k x x,” Computers & x Mathematics with Applications, Vol. 56, No. 5, 2008, pp. 1172-1175. doi:10.1016/j.camwa.2008.02.028 ,” Computers & Mathematics with Appli- cations, Vol. 53, No. 10, 200 [18] I. Yalcinkaya and C. Cinar, “Global A 7, pp. 1561-1566. symptotic Stability [24] R. P. Agarwal, W. T. Li and P. Y. H. Pang, “Asymptotic Behavior of a Class of Nonlinear Delay Difference Equa- tions,” Journal of Difference Equations and Applications, of two nonlinear Difference Equations 1, nn tza z 1 1 nnn tz 1 1 1 nn zt t ,” Fasciculi Mathematici, Vol. 43, 2010, pp. 171-180. E. M. Elsayed, “On the Sol nn na zt 21, No. 3-4, 2008, pp [20] I. Yalcinkaya, “On the Global Asymptotic Stability of a and Society, 2008, Article ID Vol. 8, 2002, pp. 719-728. doi:10.1080/1023619021000000735 [25] R. P. Agarwal, “Difference Equations and Inequalites,” 2nd Editions, Marcel Dekker, New York, 2000. [26] I. Yalcinkaya, C. Cinar and D. Simsek, “Global Asymp- totic Stability of a System of Difference Equations,” Applicable Analysis, Vol. 87, No. 6, June 2008, pp. 689- 699. doi:10.1080/00036810802140657 [19] utions of Higher Order Rational System of Recursive Sequences,” Mathematica Balkanica, Vol. . 287-296. Second-Order System of Difference Equations,” Discrete Dynamics in Nature 860152, 12 Pages. [21] E. M. Elabbasy, H. EI-Metwally and E. M. Elsayed, “On the Solutions of a Class of Difference Equations Sys- tems,” Demonstratio Mathematica, Vol. 41, No. 1, 2008, pp. 109-122. [22] E. M. Elsayed, “Dynamics of a Recursive Sequence of Higher Order,” Communications on Applied Nonlinear [27] E. M. Elsayed, “On the Solutions of a Rational System of Difference Equations,” Fasciculi Mathematici, Vol. 45 2010, pp. 25-36. [28] B. Irićanin and S. Stević, “Some Systems of Nonlinear Difference Equations of Higher Order with Periodic Solutions,” Dynamics of Continuous, Discrete and Impulsive Systems. Series A Mathematical Analysis, Vol. 13, No. 3-4, 2006, pp. 499-507.
|