Paper Menu >>
Journal Menu >>
 Applied Mathematics, 2011, 2, 1005-1010 doi:10.4236/am.2011.28139 Published Online August 2011 (http://www.SciRP.org/journal/am) Copyright © 2011 SciRes. AM Orbital Stability of Solitary Waves for Generalized Klein-Gordon-Schrödinger Equations Wenhui Qi, Guoguang Lin School of Mathematics and Statistic, Yunnan University, Kunming, China E-mail: gglin@ynu.edu.cn Received May 30, 201 1; revised June 13, 2011; accepted June 20, 2011 Abstract This paper concerns the orbital stability for exact solitary waves of the Generalized Klein-Gordon-Schrö- dinger equations. Since the abstract results of Grillakis et al. [1,2] can not be applied directly, we can extend the abstract stability theory and use the detailed spectral analysis to obtain the stability of the solitary waves. Keywords: Solitary Waves, Stability, Klein-Gordon-Schrödinger Equations 1. Introduction In this paper, we consider the the stability for the exact solitary waves of the Generalized Klein-Gordon-Schrö- dinger equations 2 2 2 pp txx pp tt xx i x R M (1.1) which describe a classical model of interaction of nu- cleon field with a meson field [3]. Here is a complex scalar nucleon field, is a real meson field, M is the mass of a meson. By applying the abstract stability the- ory and detailed spectral analysis in [4-6], we obtain the orbital stability of the solitary waves. This paper is organized as follows: in Section 2, we state the results of the existence of the exact solitary waves; in Section 3, we state the assumptions and the stability results. 2. The Exact Solitary Waves Consider the following system 2 2 2 pp txx pp tt xx i x R M (2.1) Let () ,ee , it icxct x tux xtv xct be the solitary waves of (2.1). Put (2.2) into (2.1) and suppose ,as ,,, 0uu vv x ,we obtain 2 22 2 22 21 0 0 10 pp pp cu uc cuvuu cvMvuvv (2.3) Let 1, 2ukv (2.4) satisfy (2.3) with constant determined later, then we have 0k 22 2 22 22 2 20 10 p p pp ucuu u k cvMvkvv (2.5) Let 1 1 1 sech p uc cx 2 satisfy (2.4)-(2.5) and con- stants will be determined later, then we obtain 12 ,cc 2 22 222 2 22 2 222 2 22 1 1 21 ,21 1 22 1 pp p Mp kcc c c cpcc p ct (2.2) Thus  1006 W. H. QI ET AL. 1 222 22 22 1 2 1 1 222 22 22 2 1 2 1 22 1 sech 21 22 1 21 sech 21 ppp p ppp p uxpc c cp x pcc vx c cp x (2.6) Finally, we have Theorem 1. For any real constants ω, c, p, M satisfy- ing 1 01, ,1, 2 cp 0M (2.7) there exist solitary wave of (2.1) in the form of (2.2),with satisfying (2.6). ,uv 3. Main Results Rewrite Equation (2.1) as 2 2 2 10 2, pp txx t pp txx i x R n nM (3.1) Let n u, and the function space in which we shall work is 11 realcomplex real 2 X HRH RL , with inner pro d uc t 11112 22233 ,Red xxx x R f gfg fgfgfgx fg , , X fg (3.2) The dual space of X is *112 realcomplex real X HH L * , there is a natural isomorphism : I XXdefined by ,,Igffg (3.3) where denotes the pairin g between X and ,* X . 112233 ,Red R By (3.2)-(3.4), it is obvious 2 2 1 1 I x Because the stability in view here refers to perturba- tions of the solitary-wave profile itself, a study of the initial-value problem for (1.1) is necessary. Lemma 1. Let 11 0realcomplex real 2 H RH RL u, there exists **0 0TTu and a unique solution 112 *0 [0, );,0CTHHL u * T u u. In addition, ei- ther or * ,X x ttuT. Let 12 be one-parameter groups of unitary opera- tor on X defined by ,TT 11 1 ,,TssXs R uu u (3.5) 2 22 ,e,,, is TsnXsR uu (3.6) Obviously 12 0 0,0 0 x TT x x i It follows from Theorem 1 and (3.1) that there exist solitary waves 12,, , ,, ccc TctTtxx nx with ,,, ,, cCc x xn x defined by , , , c icx c c xvx x eux nx cvx (3.7) Let ,,,, ,, cccc x xxn x In this and the following sections, we shall consider the orbital stability of solitary waves 12 ,c TctT tx of (3.1). Note that Equation (3.1) is invariant under 1 T and 2 T,we define the orbital stability as follows: Definition 1. The solitary wave 12 ,c TctT tx is orbitally stable if for all 0 there exists 0 with f gfg fgx fg (3.4) the following property. If 0,cX u and tu ) is a solution of (3.1) in some interval0with [0,t 0 0 uu, then tucan be continued to a solution in Copyright © 2011 SciRes. AM  W. H. QI ET AL. Copyright © 2011 SciRes. AM 1007 11 22 0, 0, 0 EtEQt Q QtQ uu u u uu 0t, and 12 11 2 2, 0sup t inf infcX sRsRtTsTsx u (3.12) Otherwise 12 ,c TctT tx is called orbitally unstable. Note that Equation (3.1) can be written as the follow- ing Hamiltonian system So long as ,c are fixed we write ,,n for ,, , ,, ccc d dJE t uu (3.13) x xn x . Define 222 2 11 11 22 24 2 d xx pp En M x where J is a skew-symmetric linear operator, E is a func- tional (the energy). 1 R p u However, by (2.4)-(2.6), we have (3.8) ,1, 2, 0 cc c EcQ Q (3.14) where ,EQ and 2 Q are the Frechet derivatives of E, and , with 1 Q2 Q 1u11 dI m d 22 xx x RR Qnnx x (3.9) 2 2 2 1 2 pp xx pp xx M E n u 2 21d 2R Q u x (3.10) It is easy to verify that Eu, and 1 Qu 2 Qu are invariant under 12 , and formally conserved under the flow of (3.1). Namely ,TT 2 212 1 2 2112 1 2 2212 ,, (),, (),, TsEforany s sR sT sQforanyssR sT sQforanyssR uu uu uu 1 x x x n Qi u , 2 0 0 Q u 11 11 21 E Ts QT QT * Define an operator from X to X ,,1,2ccc HE cQQ ,c (3.15) (3.11) with 123 ,, y yy X y, and and for any ,tRtu is a flow of (3.1) 22 212 2 224 2 ,1 2 2 13 12 12 22 2 pp p x ppppp c x p Mp yycy x pp 3 H py ic x x cy y ψy Observe that ,c H is self-adjoint in the sense that (3.17) *,c,c H H This means that 1,c I H is a bounded self- adjoint operator on X. The spectrum of ,c H consists of the real numbers such that ,c H I is not invert- ible. We claim that 0 belongs to the spectrum of By (3.16), Z is contained in the kernel of ,c H . Assumptio n 1. (Spectral decomposition of ,c H ) The space X is decomposed as a direct sum X NZP (3.18) ,c H . By (3.11-3.15), it is easy to prove that where Z is defined above, N is a finite-dimensional sub- space such that ,1 , ,2 , 00 0 cc cc HT x HTx 0 (3.16) ,, c H0 uu for (3.19) 0Nu Let and P is a closed subspace such that 11,2 2,12 00, cc Z kTxk TxkkR 2 ,, cX Huu u for (3.20) Pu  1008 with some constant W. H. QI ET AL. 0 indept of ,c (3.21) and define to be the Hessian of fu ear fo enden . u We define ,dc RR by :R 2 ,dc Q ,1,cc E cQ ,dc bilinnction d. It is a symmetricrm. In addition, we use pd to express the numbers of positive eigenv alue of d an ,c nH to express the numbers of negative eigealue d nv of ,c H . Theorem E 2. Suppose that there exist three function 12 ,,QQuu usatisfying (3.11) and (3.12), and 2 ,c tTtx satisfying (3.14). Moreover, suppr c H, given by (3.15) satisfies Assumption 1. If ,dc on-degen- erative, 13p and pd n then solitary waves solitary waves 1 Tc ose that the operato H is n c, , 12 tT f. According t , Tc t y stable. Proo )-(3.15), we only nee pr cx are orbit o (3.8 all d to mption 1 d For any ove that Assumption 1 and ,c pd nH hold. First of all, we prove that Assuhold an n,1 c H X y, let z (2) then 13 ,zzzy2221 22212 ,e ,,Re icx zziz z 3.2 2 22 , 1 2 2 22131 22 12 2 4222 2 13 3 2 22 113 2 22 1 1 121 ,Re 1 21 22 2 2 d 1 1 2d pp c R p x pp pp x xx R pp p p Mpz x pzz zczz p pzz x picz z x cz zzx czcz z Mp z pzzx yy H 1 21212 2222 2 22 131 21 2 11 21212 2222 ,, d, , p x R Lz zLzz czzp zz LzxL zzLzz where 2 22 2 121 p p Lc Mp x 2 2 12 11 22 pp c Lp x 2 22 12 11 22 pp pp c Lp p x 2 2 22 1 22 pp c Lx (3.23) Since 2 20c , note that 2 11 2 2 22 2 1 22 1 22 c LM x c LM x x x (3.24) with 1 M2 0, as;0,asxxMxx (3.25) Thus, by Weyl’s theorem on the essential spectrumee [5]), we have (s 22 1 22 2 [,), 22 [,), 22 ess ess cc L cc L 0 0 (3.26) Following from (2.3)-( 2.5 ) 12 0, 0LuL u (3.27) By (2.6) and (3.27), wehas a simple see that 0u zero at x , thiouvill theoen Sturm-L implies that 0 is the nd eigenvalue of rem seco 1 L,and 1 L has exactly on e strictly ve eigenvalue negati2, with eigenfunctionan 1 . In virtue of (3.24)), as[3], we lowing lemma. Lemma 2. For any real functions -(3.27 in have the fol- 1 21 zHR, sa- tisfying 21 121 ,,zzu 0 (38) there exists a positive number .2 such that 10 1 2 121 21121 , H Lz zz (3.29) 1 22 zHRLemma 3. For any real functions , sa- tisfying 22 ,0zu , there exists a ber positive num Copyright © 2011 SciRes. AM 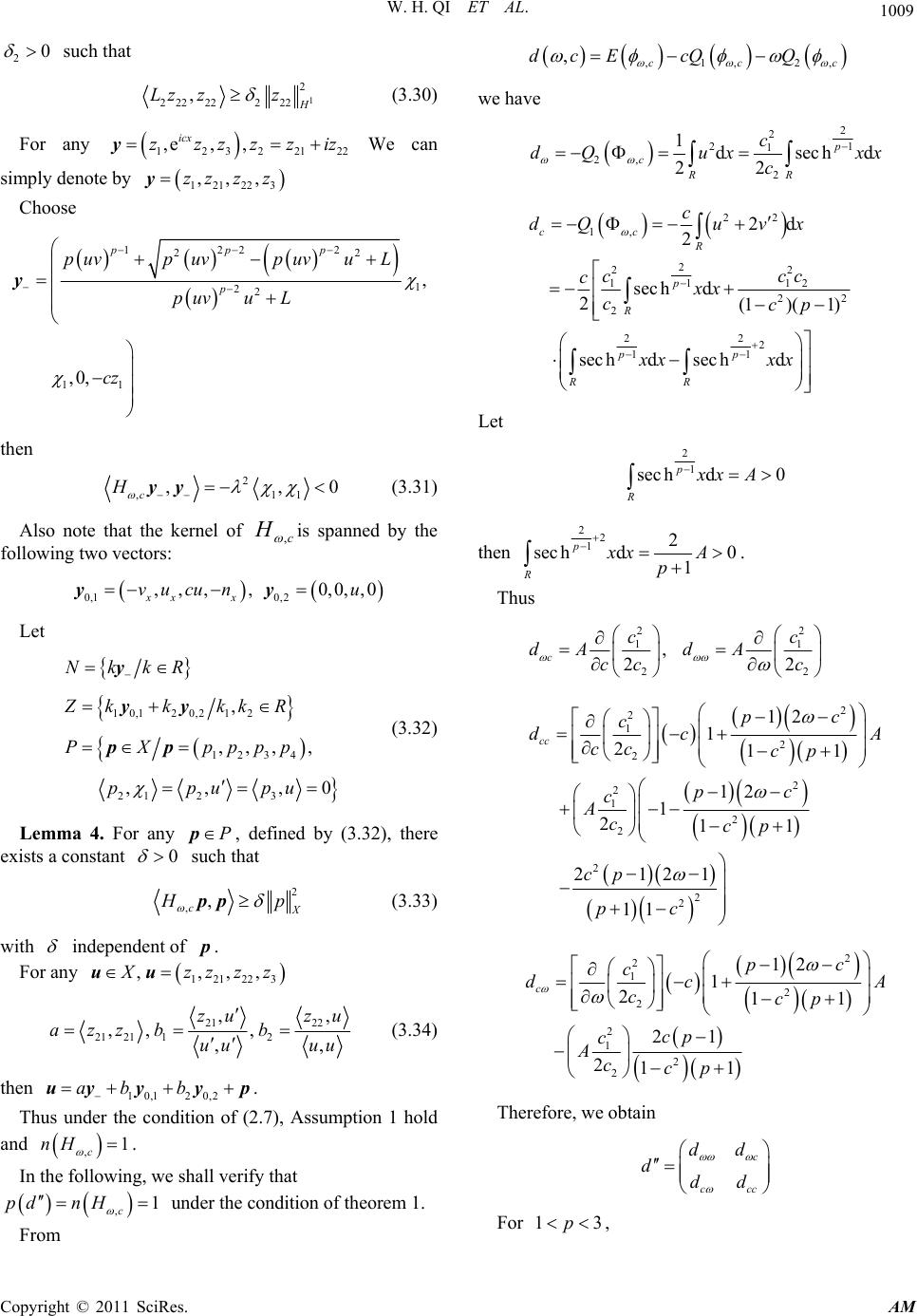 W. H. QI ET AL. 1009 20 such that 1 2 222 22222 , H Lz zz For any 22 We can se (3.30) 123221 ,e, , icx zzzzzizy 223 ,z sim Choo ply denote by 121 ,,zzzy 1222 22 pu1 22, ppp p puvpuvpuvuL uv L 11 ,0,cz y then 2 , 1 , 0 c H yy 1 , (3.31) note that the kernel of s s two vectors: Also following Let c H, ipanned by the 0,1 x0,2 ,,, ,0,0,,0 x x vucu nu yy 10,120,212 1234 PX p (3.32) 21 2 3 , ,,, , ,,,0 NkkR Zkk kkR pppp ppupu y yy p Lemma 4. For any , defined by (3.32), there exists a constant Pp 0 such that 2 ,, cX H ppp (3.33 ) with independent of ny p. For a 12122 3 ,,,, X zz z zuu 21 22 21 2112 ,, ,, , ,, zu zu azzbb uu uu (3.34) then u10,1 20,2 abb yy y p. Thus under the condition of (2Assumption 1 hold and 1nH. .7), the following, we shall verify that under the condition of theorem 1. ,c In p From d ,1 c nH ,1, 2 ,cc dcE cQQ ,c we have 2 2 21 1 2, 2 1dsech 22 p c RR c dQ uxx c d x 22 1, 2d 2 cc R dQ uvx 2 22 1 11 2 22 2 2 2 11 sech d 2(1)( 1) sech dsech p R pp RR c cc cxx ccp xxx Let 2 d x c 2 1 sech d0 p R xx A th en 22 12 sech d0 1 p R xx A p . Thus 22 11 22 , 22 c cc dAdA cc c 2 2 1 2 2 2 2 1 2 2 2 2 2 12 1 211 12 1 211 2121 11 cc pc c dc cc cp pc c Accp cp pc A 2 2 1 2 2 2 1 2 2 12 1 211 21 211 c pc c dc ccp cp c Accp A Therefore, we obtain For c ccc dd ddd 13p , Copyright © 2011 SciRes. AM 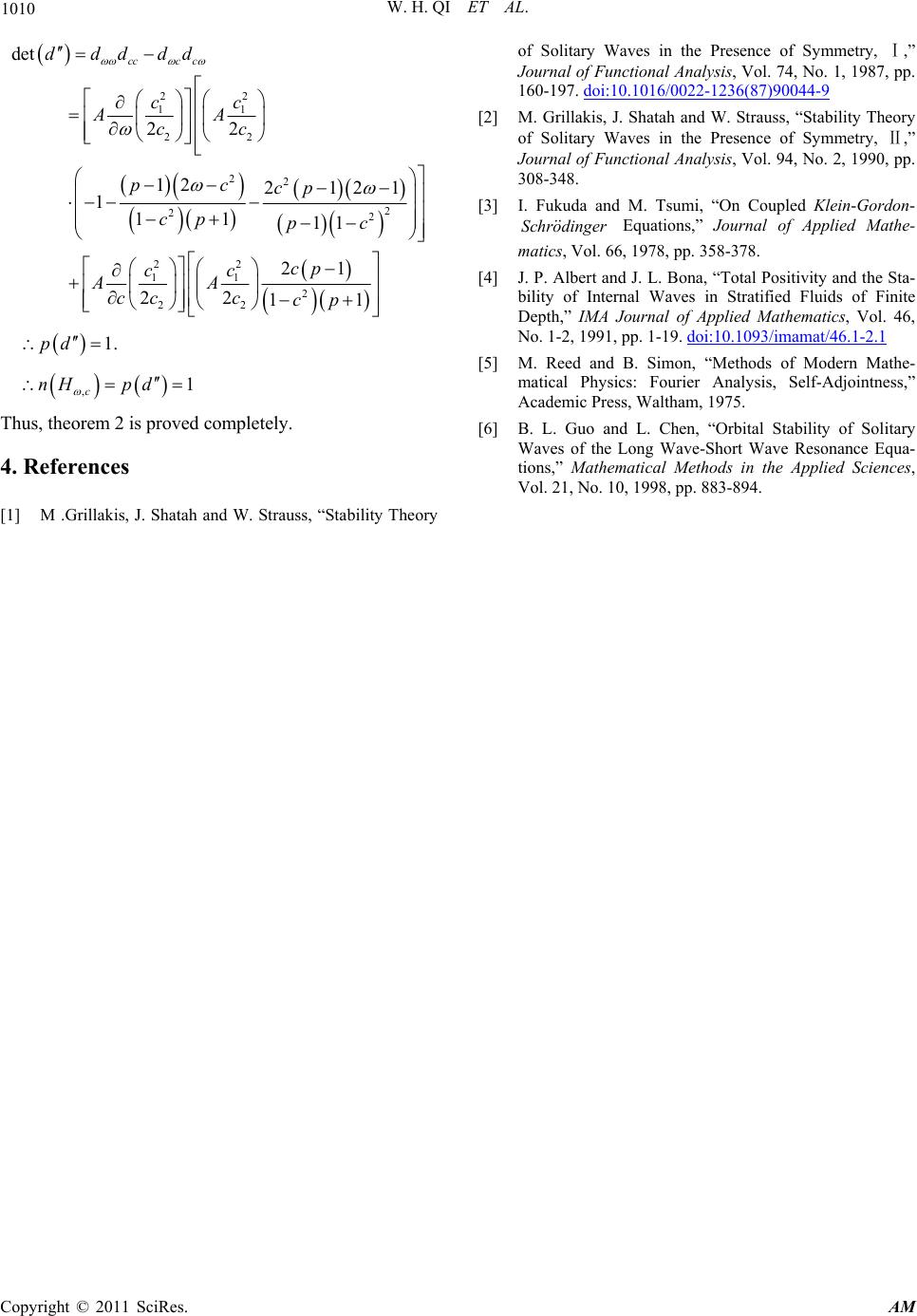 W. H. QI ET AL. Copyright © 2011 SciRes. AM 1010 22 11 22 22 2 22 22 11 2 22 det 22 12 2121 111 11 21 22 11 ccc c ddddd cc AA cc pc cp cp pc cp cc AA ccccp Thus, theorem 2 is proved completely. 4. References [1] M .Grillakis, J. Shatah and W. Strauss, “Stability Th of Solitary Waves in the Presence of Symmetry, Ⅰ,” Journal of Functional Analysis, Vol. 74, No. 1, 1987, pp. 0044-9 160-197. doi:10.1016/0022-1236(87)9 ] M. Grillakis, J. Shatah and W. Strauss, “Stability Theory aves in the Presence of Symmetry, Ⅱ,” Journal of Functional Analysis, Vol. 94, No. 2, 1990, pp. 991, pp. 1-19. doi:10.1093/imamat/46.1-2.1 [2 of Solitary W 308-348. [3] I. Fukuda and M. Tsumi, “On Coupled Klein-Gordon- Schrodinger Equations,” Journal of Applied Mathe- matics, Vol. 66, 1978, pp. 358-378. [4] J. P. Albert and J. L. Bona, “Total Positivity and the Sta- bility of Internal Waves in Stratified Fluids of Finite Depth,” IMA Journal of Applied Mathematics, Vol. 46, No. 1-2, 1 1.pd ,1 c nH pd eory m [5] M. Reed and B. Simon, “Methods of Modern Mathe- atical Physics: Fourier Analysis, Self-Adjointness,” Academic Press, Waltham, 1975. [6] B. L. Guo and L. Chen, “Orbital Stability of Solitary Waves of the Long Wave-Short Wave Resonance Equa- tions,” Mathematical Methods in the Applied Sciences, Vol. 21, No. 10, 1998, pp. 883-894. |

