Paper Menu >>
Journal Menu >>
 Advances in Pure Mathematics, 2011, 1, 201-203 doi:10.4236/apm.2011.14035 Published Online July 2011 (http://www.SciRP.org/journal/apm) Copyright © 2011 SciRes. APM Note on Laguerr e Transform in Two Variables Ajay Shukla, Ibrahim Abubaker Salehbhai Department of Mat hematics, S.V. National Institute of Technology , Surat, India E-mail: ajayshukla2@rediffmail.com, ibrahimmaths@gmail.com Received March 29, 2011; revised June 10, 2011; accepted June 10, 2011 Abstract An attempt is made to investigate the some new properties of Laguerre transform in two variables [1]. Keywords: Laguerre Transform, Laguerre Polynomials in Two Variables, Laguerre Transform in Two Variables 1. Introduction Debnath [2] introduced the Laguerre transform and de- rived some properties. He also discussed the applications in study of heat conduction [3] and to the oscillations of a very long and heavy chain with variable tension [4]. Glaeske generalized Laguerre transform of one vari- able as Laguerre-Pinney transformation [5], Wiener- Laguerre transformation [6] and derived its properties. Debnath et al. [7] reported all these work in their book. Recently Shukla et al. [1] introduced the Laguerre Transform of , f xy as , 00 ,,,,, e, n xy n Ffxyxyn ,dd x yKxyf xyxy L (1.1) where , f xy S be a Riemann integrable function defined on the set , 1 , 1 , is non- negative integer and n ,, 0 ,, ! r nrr nnr rr xy K xyL xy rn (1.2) Ragab [8] introduced Laguerre polynomials of two variables , which is defined as ,, n Lxy , 0 11 ,! !1 n k nnk k nn Lxy n Lxy knk k 1 n (1.3) Ragab [8] also obtained, ,, nn K xyL xLy (1.4) Therefore, the equivalent definition for the Laguerre Transform of , f xy is ,,fxyF 00 e, n xy nn dd x yLxLyf xyxy L (1.5) We also used following theorems based on Shukla et al. [1]: Theorem 1: If ,, n K xy is defined as (1.2), then , 00 nm K xyK , e,,dd xy nmn xyxy xy (1 ) where .6 mn (Kronecker delta symbol) is defined as 0, 1, mn m mn n , 2 11 ! n nn n ,1 and 1 . Srivastava and Manocha[9 ] rerted foing respollowults: 011 m mmm mmm Lx Lyt 0 2 ! 1!1 11 ;1,1;,,1 11 m m mmm m xyt tmt xt yt mm mt tt (1.7) wher e2 is defined as: 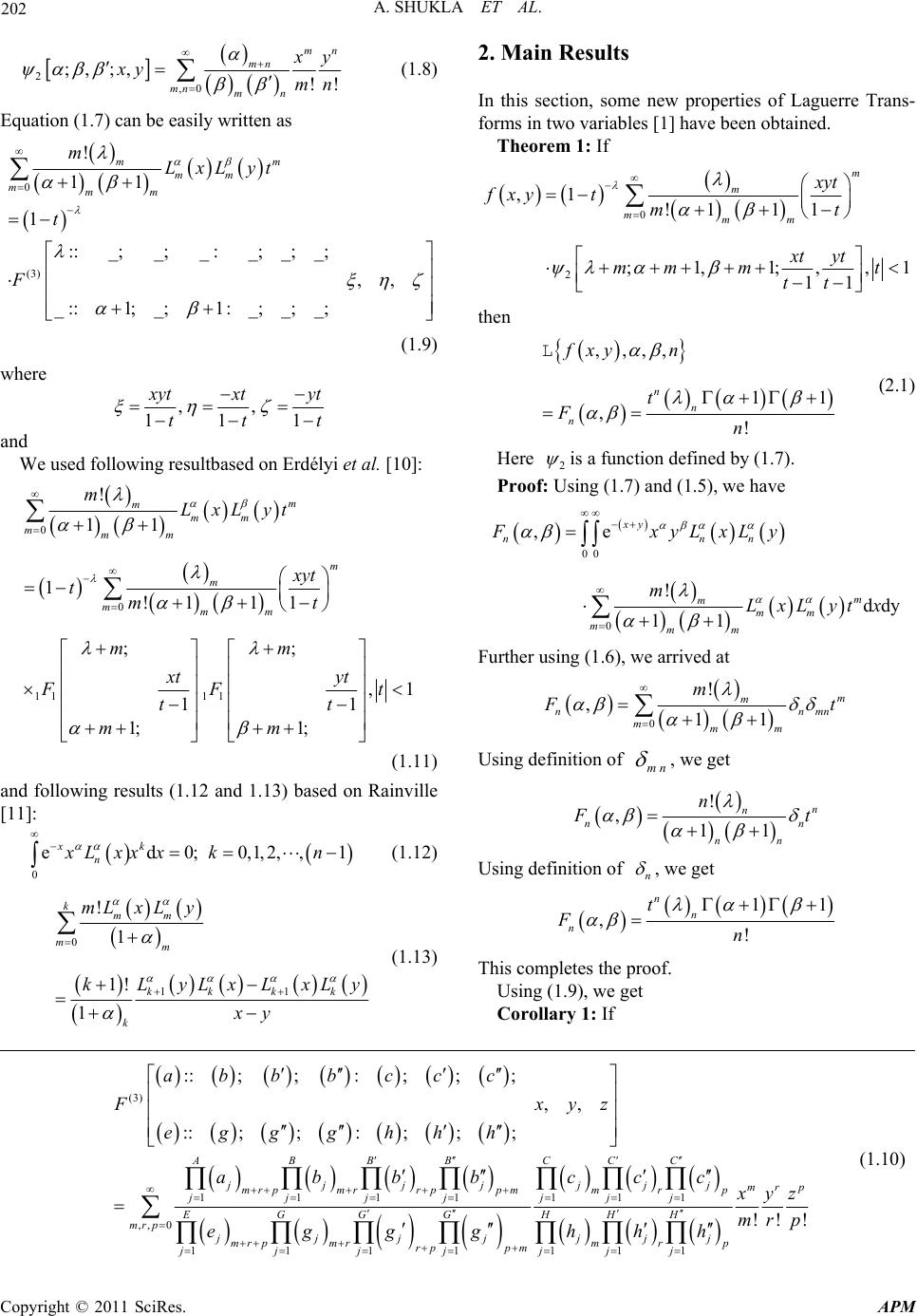 202 A. SHUKLA ET AL. 2,0 ;, ;,!! mn mn mn mn x y xy mn (1.8) Equation (1.7) can be easily written as 0 (3) ! 11 1 ::_;_;_:_;_; _; _:: 1;_;1:_;_;_; m mmm mmm mLxLyt t F ,, (1.9) where ,, 111 x yt xtyt tt t and We used following resultbased on Erdélyi et al. [10]: 0 0 1111 ,1 11 xt yt FFt tt ! 11 !1 11 ;; 1; 1; m mmm mmm m m mmm mLxLyt xyt tmt mm mm (1.11) and following results (1.12 and 1.13) based on Rainville [11]: (1.12) 1 0 ed0;0,1,2, ,1 xk n xLxx xkn 0 11 ! 1 1! kmm mm kk kk mL xLy kLyLxLxLy 1kxy 2. Main Results In this section, some new properties of Laguerre Trans- forms in two variables [1] have been obtained. Theorem 1: If 0 2 ,1 !1 11 ;1,1;,, 11 m m mmm xyt fxyt mt xt yt mm mt tt 1 then ,,,, 1 ,! n n n fxyn t Fn L 1 (2.1) Here 2 is a function defi ned by (1.7). Proof: Using (1.7) and (1.5), we have 00 0 ,e !ddy 11 xy nnn m mmm mmm FxyLxLy mLxLytx Further using (1.6), we arrived at 0 ! ,11 m m nn mmm m mn F t Using defi nition of mn , we get ! ,11 n n nn nn n F t Using defi nition of n , we get 11 ,! n n n t Fn (1.13) This completes the proof. Using (1.9), we get Corollary 1: If (3) 1111111 ,, 0 1111 :: ;;: ;cc ; ; ,, ::;;: ;;; ABBBCCC jj jjjj mr pmrpmmrp jjj jjj EGGG mr pjjjjj mr pmrrp pm jjjj abbb c Fxyz eggghhh ab bccc egggh j r pj b 111 !! ! mrp HHH jj mr p jjj x yz mr p hh (1.10) Copyright © 2011 SciRes. APM  A. SHUKLA ET AL. 203 (3) ,1 ::_;_;_:_;_; _; ,, _:: 1;_;1:_;_;_; fxyt F where ,, 111 x yt xtyt tt t then ,,,, 11 ,! n n n fxy n t Fn L (2.2) Here 3 F is a function defined by (1.10). Also, using (1.11) we have Corollary 2: If 0 11 11 , fx 1!1 11 ;; 11 1; 1; m m mmm xyt yt mt mm x ty FF tt mm , t (1)t then, ,,,, 11 ,! n n n fxyn t Fn L (2.3) Theorem 2: If ,kl f xy xy, ch that 0, 1 where and are positive numbers suk1l ,2, ,kn or (2.4) (1.12) we can obtain (2.4). orem 3: If then 0,1,2, ,1ln ,0fxy L Using The 11 1! ,1 kk k k kLyLxLx fxyxy an , k Ly d ,, n ,, F fxy n Lthen, 11 ,! n n Fn ( Proof: Using (1.13) and (1.5), we have 2.5) , 0 00 , ! e, 1 n k xy mm nmm F mL xLydd x yK xyxy Further using (1.6), we arrived at 0 ! ,1 k nn mm m Fmn Using definition of , we get mn ! ,1 n n n F n U sing defi n i tion of n , we get (2.5). 3. References [1] A. K. Shukla, I. A. Salehbhai and J. C. Prajapati, “On the Laguerre Transform in Two Variables,” Integral Trans- forms and Special Functions, V. 6, 2009, pp. 459-470. doi:10.1080/10652460802645818 ol. 20, No [2] L. Debnath, “On Laguerre Transform,” Bu cutta Mathematical Society, Vol. 55, 1960, [3] rsity di Ferrara, Sezione VII-ScienzeMathematiche, Vol. X, 1962, pp. 17- 19. [4] L. Debnath, “Application of Laguerre Transform to the Problem of Oscillations of a very Long and Heavy Chain,” Annali dell’ Univ. di Ferrara, Sezione VII-Scien- zeMathe-matiche, Vol. IX, No. 1, 1961, pp. 149-151. Pinney Transformation,” Aequationesmathematicae, Vol. 22, No. 1, 1981, pp. 73- 85. doi:10.1007/BF02190163 lletin of Cal- pp. 69-77. L. Debnath, “Application of Laguerre Transform to Heat Conduction Problem,” Annali dell’ Unive [5] H. J. Glaeske, “Die Laguerre- [6] H. J. Glaeske, “On the Wiener-Laguerre Transforma- ec Isity Zulia, Vol. 9, No. 1, 1986, pp. 27-34. [7] L. Debnath and D. Bhatta, “Integral Transforms and Their Applications,” Chapman & Hall, New York, 2007. n omls of Two Vari- of l Society, Vol. 83, 1991, pp. 253-262. ava and H. L. Manocha, “A Treatise on Generating Functions,” John Wiley and Sons, New York, tion,” Riview T´ng Univer [8] S. F. Ragab, “OLaguerre Polyn ables,” Bulletin Calcutta Mathematica ia [9] H. M. Srivast 1984. [10] A. Erdélyi, “Higher Transcendental Functions,” Vol. 2, McGraw-Hill, New York, 1953. E. D. Rainville, “Special Functions,” Mac[11] millan, New York, 1960. Copyright © 2011 SciRes. APM |

