Engineering, 2009, 2, 99-105
doi:10.4236/eng.2009.12011 Published Online August 2009 (http://www.SciRP.org/journal/eng/)
99
On an MC-CDMA System Operating with Distinctive
Scenarios of Antenna
Joy Iong-Zong CHEN, Hau-Wei HUANG, Kai Zhi ZHUANG
Department of Communication Engineering, Dayeh University, Dacun Changhua, Taiwan, China
Received June 10, 2009; revised July 12, 2009; accepted July 20, 2009
ABSTRACT
In this paper the impact of distinctive structure of antenna with branch correlation for an OFDM (orthogonal
frequency division multiplexing)-based system, MC-CDMA (multi-carrier coded-division multiple-access)
system, operating over the frequency selective fading environments is studied. For the reason of accordance
with the working environments in the real world applications (urban areas) the correlated-Nakagami-m fad-
ing is adopted. Furthermore, the performance evaluation with average BER (bit error rate) formulas of
MC-CDMA system with MRC (maximal ratio combining) diversity was derived with an alternative method
of the complementary error function. The illustrated results are not only discussing the effect that comes
from triangular, linear, and circular antenna array constructions, but the factors of branch correlation are also
analyzed. Generally, it is known that the more the received branch number is, the more superior system per-
formance of a multiple-access system will become. It is interesting to contrast to the geometric of the an-
tenna array, that is, the little shape changing of the antenna is, the worse inferior system performance arrive
at.
Keywords: Circular Antenna Array, Linear Antenna Array, MC-CDMA Systems, MRC Diversity, Naka-
gami-m Fading, Triangular Antenna Array
1. Introduction
For the purpose of overcoming ISI (inter-symbol inter-
ference) effect and reducing the channel fading in a
transmission channel, multi-carrier modulation scheme
has been adopted for high speed transmission applica-
tions. A number of multi-carrier modulation techniques
have been proposed during the pass decade [1]. To sup-
port a wide area of services and high data rate by using a
variety of techniques capable of achieving the highest
possible spectrum efficiency is the main objective for
future generations of wideband wireless communication
systems. The CDMA (coded-division multiple-access)
scheme has been applied as an attractive multiple access
technology in both 2G (second-generation) and 3G
(third- generation) wireless radio systems. In general, the
multicarrier DS systems have already been proposed and
can be categorized into two types: a parallel transmis-
sion-scheme of narrowband DS waveforms in the fre-
quency domain, and a combination of OFDM (orthogo-
nal frequency division multiplexing) and CDMA [1].
The available frequency spectrum of carrier wave is di-
vided into M equal band of subcarriers in the former
systems. These subcarriers are used to carry a narrow-
band DS waveform and the number of subcarriers is
usually much less than the processing gain. In the latter
system, each chip modulates a different carrier convey-
ing a narrowband waveform rather than a DS waveform,
and the number of carriers should be equal to the proc-
essing gain.
On the other hand, due to the advantages of spectrum
efficient, interference immune, high date rate, and insen-
sitivity to frequency selective channel, etc. Such that
multiple access system bases on direct sequence CDMA
(coded-division multiple-access) have drawn recently
interest in the application of wireless radio systems [1].
Especially, multi-carrier CDMA (MC-CDMA) appears
to be a considerable candidate for future mobile radio

J. I. Z. CHEN ET AL.
100
communication system. The MC-CDMA system based
on the spread spectrum techniques. There are a lot of
previous researches have been published for investiga-
tion about the issues of MC-CDMA system. Besides, the
BER (bit error rate) analysis of MC-CDMA based on
considering different kinds of assumptions, so far, have
been dedicated in numerous previously researches [1-3].
In [2] the authors analyzed the BER (bit error rate) per-
formance of uplink MC-CDMA system over frequency
selective Nakagami-m fading with MRC (maximal ratio
combining) and EGC receptions. The performance
evaluation of MC-CDMA over multipath fading chan-
nels was studied in [3]. The results presented in [4] are
for uplink channel using MRC with the assumed fre-
quency offsets condition in correlated fading. The per-
formance of MC-CDMA in non-independent Rayleigh
fading was studied in [5]. In [6], which by use of the
method of CF (characteristic function) and residue theo-
rem to calculate the performance for downlink
MC-CDMA system. Both of the envelopes and phases
correlation are considered in [7] to evaluate the per-
formance of a MC-CDMA system operates in Rayleigh
fading channel. The literature in [8] illustrated the error
probability for MC-CDMA systems assumed that the
transmission channel is in Nakagami-m fading, and the
postdetection of EGC (equal gain combining) is consid-
ered.
The different construction, linear, triangular, and cir-
cular antenna arrays are deployed as the environments
for evaluating the system performance of uplink
MC-CDMA systems in this paper. Moreover several
expressions of BER performance are derived not only for
the previously mentioned scenarios, but with also the
assumption of correlated fading channels, which is as-
sumed characterized as correlated-Nakagami-m statistics.
An average BER formula closed-form is obtained via the
pdf (probability density function) of Gamma variates
distribution to avoid the difficulty of explicitly obtaining
the pdf for the SNR (signal-to-noise ratio) at the MRC
output. The results from this paper partly analyze and
show how the channel correlation affects the system
performance of MC-CDMA systems, and explore the
phenomena of different incident angular of waveform
into an antenna array. The rest of this paper is organized
as follows: Section 2 gives a description of the
MC-CDMA system model, the correlated-Nakagami-m
fading channel model, and the receiver model of
MC-CDMA system. The performance of MC-CDMA
operating in uncorrelated and correlated fading cannel is
carried out in Section 3. There are numerically results
shown in Section 4. Finally, Section 5 draws briefly con-
clusions.
2. MC-CDMA System Models
2.1 Transmitter Model
An uplink MC-CDMA system model is considered for
the study. Assuming that exist K simultaneous users are
with N subcarriers within a signal cell. Any effect of
correlation among users is going to be ignored by as-
suming the number of users is uniformed of distribution.
As shown in Figure 1, a signal data symbol is replicated
into N parallel copies. The signature sequence chip with
a spreading code of length L is used to BPSK (binary
phase shift keying) modulated each of the N subscriers of
the k-th user. Where the subcarrier has frequencyb
F
/T
Hz, and where F is an integer number [1, 3]. The techni-
cal described above is same as to the performance of
OFDM (orthogonal frequency division multiplexing) on
a direct sequence spread-spectrum signal when set
1
F

. The larger values of
F
, the more transmit
bandwidth increase. The transmitted signal the resulting
transmitted baseband signal corresponding to the
M data bit size can be expressed as
()t
k
S
11
00
2
()[][] ()Re[]
n
ML
jt
kkkT
mn
P
StanbmPt e
N




(1)
where [] {1,1}
k
an


..., [1]
kk
aaL
, , the sequencer []{1,1}
k
bm
[0],

and represent the
signature sequence and the data bit of the k-th user, re-
spectively, P is the power of data bit, M denotes the
number of data bit, N denotes the number of subcarriers,
the T is defined as an unit amplitude pulse that is
non-zero in the interval of [0 , and Re []
[0],...,[
kk
bb
]
b
,T
1]M
P(t)

denotes
the real part of a complex number, 2( )
ncb
f
nF T

 
is the angular frequency of the n-th subcarrier.
2.2 Channel Model
A frequency-selective channel with 1bc b is
addressed in this paper, where is the coherence
bandwidth. This channel model means that each modu-
lated subcarrier does not experience significant disper-
sion and with transmission bandwidth of
TBWFT
c
BW
1b T, i.e.
, where
b
TTd1d T is the Doppler shift typically in
the range of 0.3~6.1 Hz [1] in the indoor environment,
and the amplitude and phase remain constant even the
symbol duration b. In addition to, the channel of inter-
est has the transfer function of the continuous-time fad-
ing channel assumed for the k-th user can be represented
as
T
,
,
[]ki
j
kc ki
b
F
Hf ie
T

  (2)

J. I. Z. CHEN ET AL.
101
where ,ki

and ,ki

are the random amplitude and
phase of the channel of the k-th user at frequency
()
cb
f
iFT. In order to follow the real world case, the
random amplitude, ,ki

are assumed to be a set of N
correlated not necessarily identically distributed in one
of our scenarios.
The equal fading severities are considered for all of
the channels, namely, . The pdf of
the fading amplitude for the k-th user with i-th chan-
nel,
, 1,,mm L

,k

, are assumed as r. v. (random variable) with the
Nakagami-m distribution, and given as [10]
21 2
2
()()(), 0
()
m
m
mm
Pexp
m




 
(3)
where is the gamma function defined by
()
1
0
x
() t
x
te
dt


, 2
[E]

 denoting expectation, the
parameter m of the amplitude distribution characterizes
the severity of the fading, and it is defined as
2
22
0.5
[() ]
mE




(4)
It is well known that 0.5m

(one-sided Gaussian
fading) corresponds to worst case fading condition,
and correspond to Rayleigh fading
(purely diffusive scattering) and the non-fading condition,
respectively. As what follows, we consider these two
cases.
1mm
First, with the assumption the propagation channels
are assumed as i.i.d (identically independent distributed),
then by using of the variable changing, the variable

is assigned as the fading intensity of the correlated
channel, and let 2

 , then the pdf of

is given eas-
ily following as a Gamma distribution, which can be
obtained by the processing of random stochastic as
 
1
1
,, exp
r
x
x
px



 

 

 
 

 

 
(5)
Let [], 0,,1
L

 

i
be a set of N correlated iden-
tically distributed, and all the figure parameters and the
average power are assumed equivalent, that
is,
j
mm m , and i
j
 , where
. The power at the output of the
MRC is a function of the sum of the squares of signal
strengths, and is given as

,ij for , 0,,1ij L
1
0
L
R





2.3 MC-CDMA Receiver Model
A slowly varying fading channel is considered in this
paper, that is, the channel parameters are unchanged over
one bit duration
b
T
)
. For K active transmitters, the re-
ceived signal can be written as
(rt
111
,
000
,
2
()[ ][]
()()()
KM L
mn kk
kmn
Tbknmn
P
rtanb m
N
Pt mTcostnt





 

(6)
where is the AWGN (additive white Gaussian
noise) with a double-sided power spectral density of
()nt
02N. We can evaluate, the local-mean power, ,
which is given as
,kn
P
2
,,
[]
kn kn
PE N

 
(7)
where the total-mean power of the k-th user is defined to
be
,
kkn
P
NP

, if the local-mean power of the subcarri-
ers is assumed equal. Assuming that acquisition has been
accomplished for the user of interesting (). In addi-
tion, the system operates synchronously with each user
having the same clock is assumed, and the MRC diver-
sity reception technique is considered in this paper. For
the reason of using MRC, it is assumed that perfect
phase correction can be obtained, i.e., 0
ii
0k

0,



. De-
modulating each subcarrier includes applying a phase
correction,

0,i

, and a gain correction factor
0,0, 0[] nn
dan


 is multiplied by the n-th subcarrier sig-
nal as shown in Figure 2.
All the signals at the output of the correlators are
combined with the MRC diversity scheme, and the re-
sults can be written as
1
0
L








(8)
where

 is the SNR at every branch. The branch
number is assumed that equal to the subcarrier number,
that is ,
L
N

, in this paper. With all the assumptions
for MRC combining, the decision variable of the
m-th data bit reference user, and given by
0
D
0,0
1
(1)
00
0
()
0
1() []
Re[ ]
U
b
b
l
L
mT
l
mT l
b
wt Q
sMAI
Drtal
T
e
I 








0,
d
dt (9)
where is the received signal shown in (10), is
the gain factor for MRC diversity. The first term,, in
previous equation represents the desired signal, can be
expressed as
()rt 0,i
d
S
U
1
2
0, 0
0
[]
2
L
S
P
Ua
N






m
(10)

J. I. Z. CHEN ET AL.
102
and the second term,
M
AI
I, is the MAI (multiple access
interference) contributed from all other users which can
be written as
11
0
10
,0, ,
[] [] []
2
cos( )
KL
MAIk k
kn
km nkn
P
I
ambmam
N
 


 


 (11)
where '
,0,knn kn,

 , where ,kn

is i.i.d uniformly dis-
tributed over [0,2 )

, and the last term, 0

, in (13) is
the AWGN.
3. Performance Analysis
A generalized average BER for the k-th user using co-
herent BPSK (binary phase shift keying) modulation
scheme is derived in this section. For coherent demodu-
lation in the presence of AWGN, the probability of error
conditioned on the instantaneously SNR can be expressed
as [11]

() 0.5
e
P
sQSNR
(12)
where the Gaussian Q-function is defined by

22
()12 t
x
Qxe dt 

,
and the received instantaneously SNR , which condi-
tioned on 2
0, 0,nn

, at output of the receiver is calcu-
lated as
0
1
2
20,
0
22 2
2
MAI
N
n
sn
TI
P
UN








(13)
where 2
M
AI
I

is the variance of
M
AI
I, which is shown in
(14). In the limiting case of large N and by the methods
of CLT (central limit theory), the MAI can be approxi-
mated by a Gaussian r.v. with zero mean and the vari-
ance, 2
M
AI
I

, can be determined as
22
,
2
,,
[] (1)[
2
[cos]( 1)
4
MAI
2
]
I
MAIk n
kn kn
P
EIk E
P
Ek


 

(14)
where 2
,,
[]
kn kn
E

 , 2
,
[]
kn
Ecos  12
. On the other
hand, the background noise term 0

is a random vari-
able with zero mean and the variance can be calculated
as
0
22
0
0
[]4b
NN
ET

 
(15)
By substituting (14) and (15) into (13), which can be
obtained as
2
1
0
2
1()
2
s
N
T
US
N




(16)
where
1
2
0, ,
0
N
Nn
n
S 


kn


,
and
0
0
,0
11
444
bkn
NN kNk
PT

4



,
where
0,0,bkn bkn
PTNEN0



is the SNR of each bit, and bb
E
PT denotes the bit
energy.
It is known that the decision variable in (13) has a
Gaussian distribution conditioned on the uncorrelated
and correlated channel power 2
0,n

, respectively, and the
AWGN, 0

, and the MAI,
M
AI

are mutually inde-
pendent. Therefore, the probability of error by means of
BPSK modulation conditioned on the instantaneously
SNR has been given in (16) can be evaluated as follows.
If the conditions of correlated channels are considered
as the impact factors for an MC-CDMA system, then the
average bit error probability for the case can be calcu-
lated by averaging (5) and (16), and yield as
2
/2
00 0
0
2
11
12sin
exp (1)(,)
2
(,, )
b
Lm
l
l
e
x
N
KdL
E
N
pxldxd






















P
(17)
Next, by using of the integral equivalent formula,

1
01
mm
ed

 


m
the average BER can be simplified and expressed as
00
0 2
11
/2
02
0
0
2
2 1()
(1)(,)
2
11
2
21
(1)(,) sin
N
e
b
Lm
l
ll
l
b
S
P
Qf
N
KdL
E N
d
N
KdL
EN









xdx

































(18)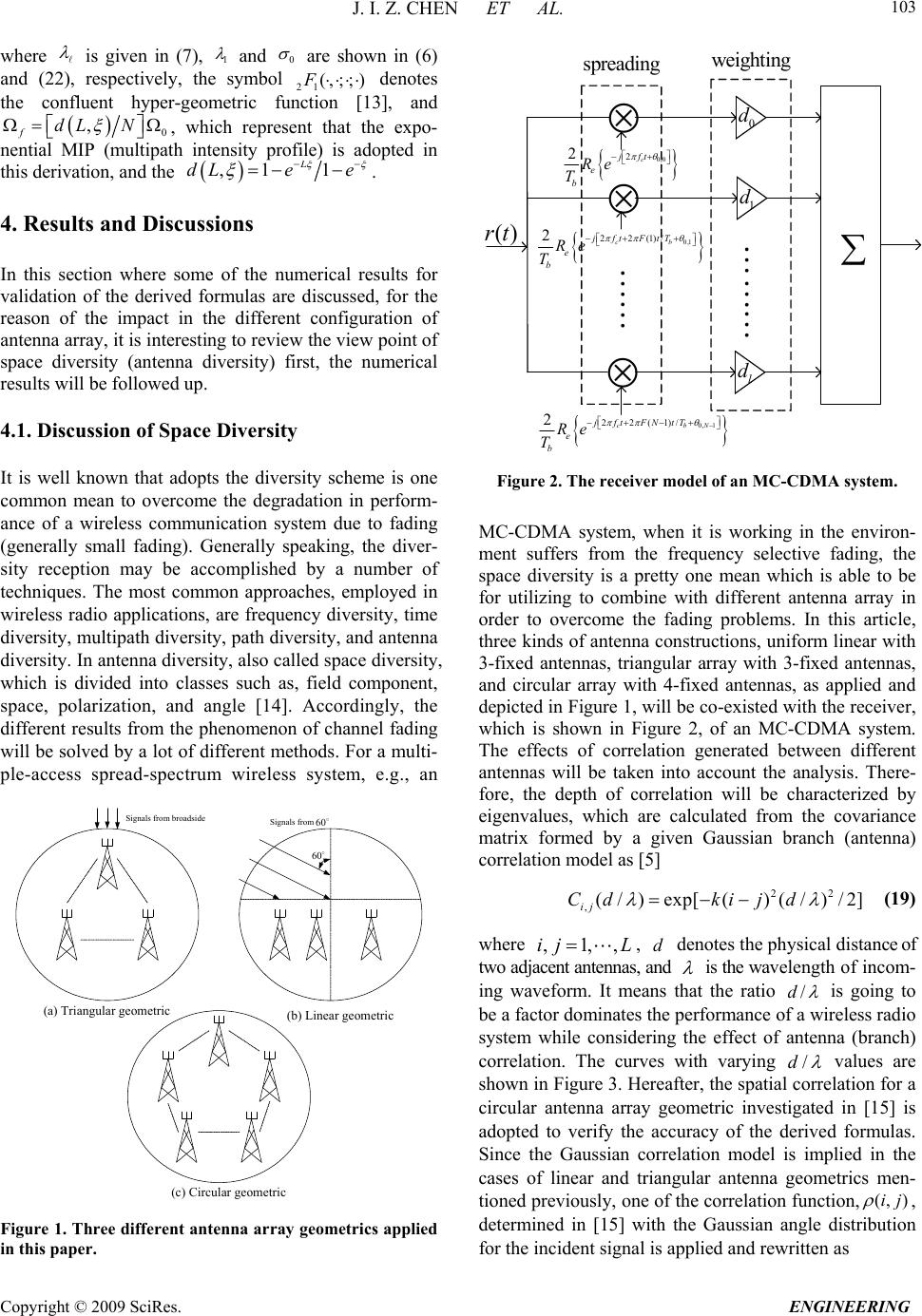

J. I. Z. CHEN ET AL.
103
where

 is given in (7), 1

and 0

are shown in (6)
and (22), respectively, the symbol 21 (,;;)
F
 denotes
the confluent hyper-geometric function [13], and


0
,
fdL N

 

, which represent that the expo-
nential MIP (multipath intensity profile) is adopted in
this derivation, and the


,1 1
L
dLee



.
4. Results and Discussions
In this section where some of the numerical results for
validation of the derived formulas are discussed, for the
reason of the impact in the different configuration of
antenna array, it is interesting to review the view point of
space diversity (antenna diversity) first, the numerical
results will be followed up.
4.1. Discussion of Space Diversity
It is well known that adopts the diversity scheme is one
common mean to overcome the degradation in perform-
ance of a wireless communication system due to fading
(generally small fading). Generally speaking, the diver-
sity reception may be accomplished by a number of
techniques. The most common approaches, employed in
wireless radio applications, are frequency diversity, time
diversity, multipath diversity, path diversity, and antenna
diversity. In antenna diversity, also called space diversity,
which is divided into classes such as, field component,
space, polarization, and angle [14]. Accordingly, the
different results from the phenomenon of channel fading
will be solved by a lot of different methods. For a multi-
ple-access spread-spectrum wireless system, e.g., an
60
60
Signals from
Signals from broadside
(b) Linear geometric
(c) Circular geometric
(a) Triangular geometric
Figure 1. Three different antenna array geometrics applied
in this paper.




0,0
2
2c
jft
e
b
Re
T








0,1
22(1)/
2cb
jftFtT
e
b
Re
T


  







0, 1
22(1)/
2cbN
jftFNtT
e
b
Re
T
 






0
d
1
d
l
d



spreading weight i ng

()rt
Figure 2. The receiver model of an MC-CDMA system.
MC-CDMA system, when it is working in the environ-
ment suffers from the frequency selective fading, the
space diversity is a pretty one mean which is able to be
for utilizing to combine with different antenna array in
order to overcome the fading problems. In this article,
three kinds of antenna constructions, uniform linear with
3-fixed antennas, triangular array with 3-fixed antennas,
and circular array with 4-fixed antennas, as applied and
depicted in Figure 1, will be co-existed with the receiver,
which is shown in Figure 2, of an MC-CDMA system.
The effects of correlation generated between different
antennas will be taken into account the analysis. There-
fore, the depth of correlation will be characterized by
eigenvalues, which are calculated from the covariance
matrix formed by a given Gaussian branch (antenna)
correlation model as [5]
22
,(/) exp[()(/)/2] ij
Cd kijd   (19)
where , 1,,ij L

, denotes the physical distance of
two adjacent antennas, and
d

is the wavelength of incom-
ing waveform. It means that the ratio /d

is going to
be a factor dominates the performance of a wireless radio
system while considering the effect of antenna (branch)
correlation. The curves with varying /d

values
shown in Figure 3. Hereafter, the spatial correlation for a
circular antenna array geometric investigated in [15] is
adopted to verify the accuracy of the derived formulas.
Since the Gaussian correlation model is implied in the
cases of linear and triangular antenna geometrics men-
tioned previously, one of the correlation function,(,)ij
are

,
determined in [15] we Gaussian angle distribution
for the incident signal is applied and rewritten as
ith th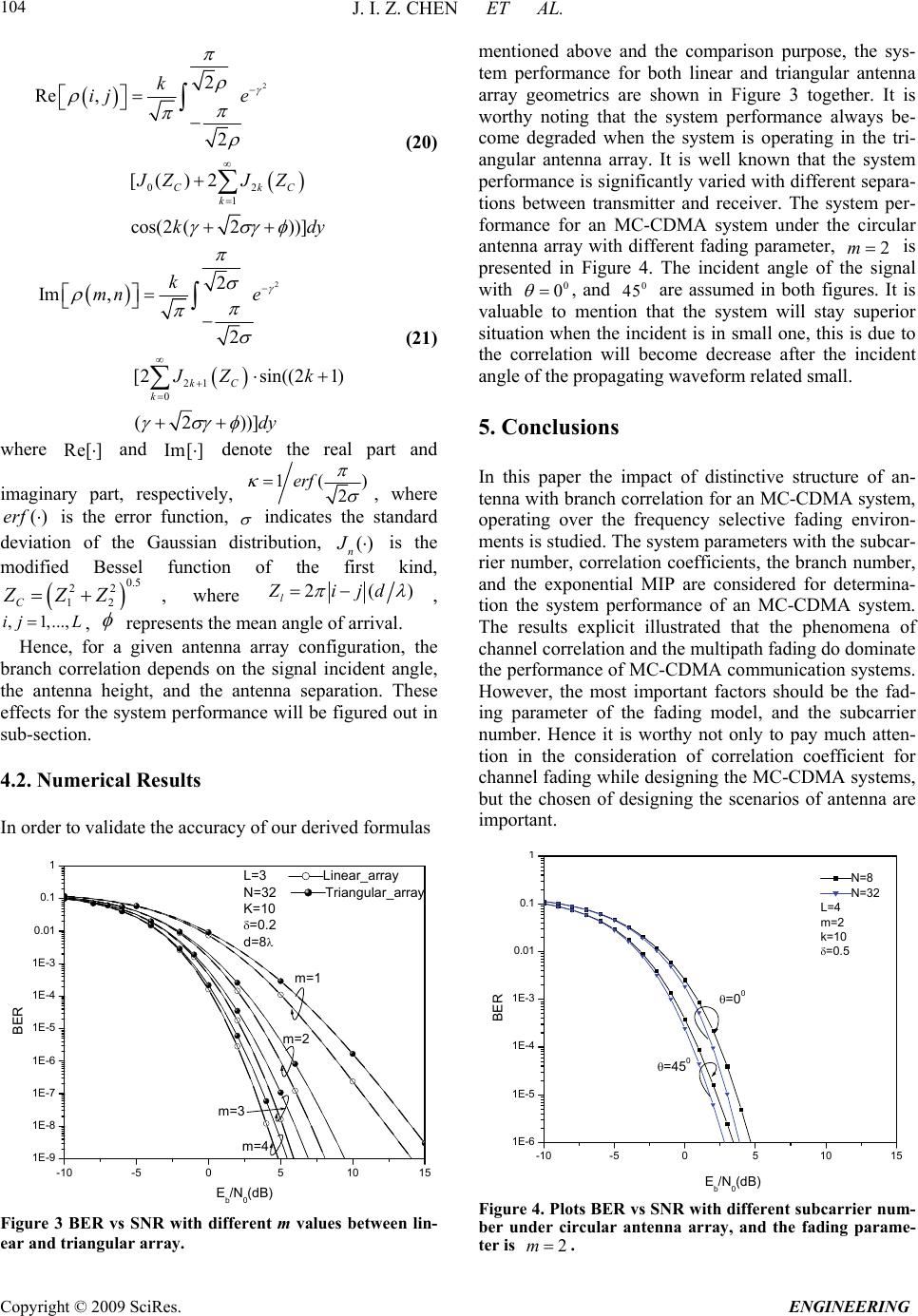

J. I. Z. CHEN ET AL.
104


2
02
1
2
Re ,
2
[( )2
cos(2 (2))]
CkC
k
k
ij e
JZJZ
kd












 




y
(20)


2
21
0
2
Im ,
2
[2sin((2 1)
( 2))]
kC
k
k
mn e
JZ k
dy


















(21)
where and denote the real part and
imaginary part, respectively,
Re[ ]Im[ ]
1(
2
erf )



, where
is the error function,
()erf 

indicates the standard
deviation of the Gaussian distribution, ()
n
J

is the
modified Bessel function of the first kind,


0.5
Z
22
12
ZZ
C, where 2(
l)
Z
ijd

  ,
,
,1ij,...,L

represents the mean angle of arrival.
Hence, for a given antenna array configuration, the
branch correlation depends on the signal incident angle,
the antenna height, and the antenna separation. These
effects for the system performance will be figured out in
sub-section.
4.2. Numerical Results
In order to validate the accuracy of our derived formulas
-10 -5051015
1E-9
1E-8
1E-7
1E-6
1E-5
1E-4
1E-3
0.01
0.1
1
m=4
m=3
m=2
m=1
BER
Eb/N0(dB)
L=3 Linear_array
N=32 Triangular_array
K=10
=0.2
d=8
Figure 3 BER vs SNR with different m values between lin-
ear and triangular array.
mentioned above and the comparison purpose, the sys-
tem performance for both linear and triangular antenna
array geometrics are shown in Figure 3 together. It is
worthy noting that the system performance always be-
come degraded when the system is operating in the tri-
angular antenna array. It is well known that the system
performance is significantly varied with different separa-
tions between transmitter and receiver. The system per-
formance for an MC-CDMA system under the circular
antenna array with different fading parameter,
2
m

is
presented in Figure 4. The incident angle of the signal
with 0
0


, and are assumed in both figures. It is
valuable to mention that the system will stay superior
situation when the incident is in small one, this is due to
the correlation will become decrease after the incident
angle of the propagating waveform related small.
0
45
5. Conclusions
In this paper the impact of distinctive structure of an-
tenna with branch correlation for an MC-CDMA system,
operating over the frequency selective fading environ-
ments is studied. The system parameters with the subcar-
rier number, correlation coefficients, the branch number,
and the exponential MIP are considered for determina-
tion the system performance of an MC-CDMA system.
The results explicit illustrated that the phenomena of
channel correlation and the multipath fading do dominate
the performance of MC-CDMA communication systems.
However, the most important factors should be the fad-
ing parameter of the fading model, and the subcarrier
number. Hence it is worthy not only to pay much atten-
tion in the consideration of correlation coefficient for
channel fading while designing the MC-CDMA systems,
but the chosen of designing the scenarios of antenna are
important.
-10 -50510 15
1E-6
1E-5
1E-4
1E-3
0.01
0.1
1
E
b
/N
0
(dB)
=450
=00
N=8
N=32
L=4
m=2
k=10
=0.5
BER
Figure 4. Plots BER vs SNR with different subcarrier num-
ber under circular antenna array, and the fading parame-
ter is 2m

.

J. I. Z. CHEN ET AL.
105
6. References
[1] N. Yee, J. P. Linnartz, and G. Fettweis, “Multi-carrier
CDMA in indoor wireless radio networks,” IEICE
Transactions on Communication, Vol. E77-B, No. 7,
pp. 900-904, July 1994.
[2] Z. J. Kang and K. Yao, “Performance comparison of
MC-CDMA over frequency-selective Nakagami-m and
Rayleigh fading channels,” Vehicular Technology
Conference, Vol. 6, pp. 4228-4232, September 2004.
[3] E. A. Sourour and M. Nakagami, “Performance of or-
thogonal multicarrier CDMA in a multipath fading
channel,” IEEE Transactions on Communication, Vol.
44, pp. 356-367, March 1996.
[4] T. Kim, Y. Kim, J. Park, K. Ko, S. Choi, C. Kang, and
D. Hong, “Performance of an MC-CDMA system with
frequency offsets in correlated fading,” IEEE Interna-
tional Conference on ICC 2000, Vol. 2, pp. 1095-1099,
June 2000.
[5] J. Park, J. Kim, S. Choi, N. Cho, and D. Hong, “Per-
formance of MC-CDMA systems in non-independent
Rayleigh fading,” IEEE on ICC’ 99, Vol. 1, pp. 6-10,
506-510, June 1999.
[6] Q. R. Shi and M. Latva-aho, “Exact error floor for
downlink MC-CDMA with maximal ratio combining in
correlated Nakagami fading channels,” 2002 Interna-
tional Zurich Seminar on Broadband Communications,
pp. 37-1-37-5, February 20, 2002.
[7] Q. Shi and M. Latva-aho, “Performance analysis of
MC-CDMA in Rayleigh fading channels with corre-
lated envelopes and phases,” IEE Proceedings: Com-
munication, Vol. 150, No. 3, pp. 214-220, June 2003.
[8] Z. Li and M. Latva-aho, “Erroe probability for
MC-CDMA in Nakagami-m fading channels using
equal gain combining,” IEEE International Conference
on ICC 2002, Vol. 1, pp. 227-231, April 28-May 2,
2002.
[9] M. S. Alouini, A. Abdi, and M. Kaveth, “Sum of
gamma variates and performance of wireless commu-
nication systems over Nakagami-m fading channels,”
IEEE Transactions on Vehicular Technology, Vol. 50,
No. 6, pp. 1471-1480, November 2001.
[10] M.Nakagami, “The m-distribution—A general formula
of intensity distribution of rapid fading,” Statistic
Methods of in Radio Wave Propagation, Pergamon
Press, New York, pp. 3-36, 1960.
[11] M. Schwartz, W. R. Bennett, and S. Stein, “Communi-
cation systems and techniques,” McGraw-Hill, New
York, 1966.
[12] M. K. Simon and M. S. Alouini, “A unified approach
to the performance analysis of digital communication
over generalized fading channel,” Proceedings of the
IEEE, Vol. 86, pp. 1860-1877, September 1998.
[13] I. S. Gradshteyn and I. M. Ryzhik. “Table of integrals,
series, and products,” CA: Academic Press, San Diego,
5th Edition, 1994.
[14] W. C. Jakes, “Microwave Mobile Communications,”
IEEE Press, Piscataway, Jersey, 1974.
[15] J. Zhou, S. Sasaki, S. Muramatsu, H. Kikuchi and Y.
Onozato, “Saptial correlation for a circular antenna
array and its applications in wireless communications,”
IEEE Global Telcommunications Conference, Vol. 2,
pp. 1108-1113, 2003.