Atmospheric and Climate Sciences, 2011, 1, 147-164
doi:10.4236/acs.2011.13017 Published Online July 2011 (http://www.SciRP.org/journal/acs)
147
Understanding the Variability of Z-R Relationships Caused
by Natural Variations in Raindrop Size Distributions
(DSD): Implication of Drop Size and Number
Abé D. Ochou1*, Eric-Pascal Zahiri1, Bakary Bamba1, Manlandon Koffi2
1Laboratoire de Physique de l’Atmosphère et Mécanique des fluides (LAPA-MF), Université de Cocody-Abidjan,
Abidjan, Côte d’Ivoire
2Institut National Polytechnique Houphouët-Boigny, Yamoussoukro, Côte d’Ivoire
Received May 9, 2011; revised June 13, 2011; accepted July 13, 2011
Abstract
In the issue of rainfall estimation by radar through the necessary relationship between radar reflectivity Z and
rain rate R (Z-R), the main limitation is attributed to the variability of this relationship. Indeed, several pre-
vious studies have shown the great variability of this relationship in space and time, from a rainfall event to
another and even within a single rainfall event. Recent studies have shown that the variability of raindrop
size distributions and thereby Z-R relationships is therefore, more the result of complex dynamic, thermody-
namic and microphysical processes within rainfall systems than a convective/stratiform classification of the
ground rainfall signature. The raindrop number and size at ground being the resultant of various processes
mentioned above, a suitable approach would be to analyze their variability in relation to that of Z-R relation-
ship.In this study, we investigated the total raindrop concentration number NT and the median volume di-
ameter D0 used in numerous studies, and have shown that the combination of these two ‘observed’ parame-
ters appears to be an interesting approach to better understand the variability of the Z-R relationships in the
rainfall events, without assuming a certain analytical raindrop size distribution model (exponential, gamma,
or log-normal). The present study is based on the analysis of disdrometer data collected at different seasons
and places in Africa, and aims to show the degree of the raindrop size and number implication in regard to
the Z-R relationships variability.
Keywords: Raindrop Size Distribution, Radar Reflectivity Factor, Rain Rate, Median Volume Diameter,
Total Number of Drops Per Unit Volume, Z-R Relationship, Convective Rain, Stratiform Rain,
Squall Lines, Thunderstorm
1. Introduction
In the study of rainfall, one parameter of interest to es-
timate with regard to rain drop size distributions (DSD)
is the rain rate R. It can be measured from the ground
using rain gauges and weather radar.The nature of
space-time radar measurements represented by the re-
flectivity factor Z has generated so much interest that
many studies have focused on finding connections to
bring it in intensity [1-12].Thus, numerous studies based
on the measurement of DSD in precipitation around the
world continue to show that empirical expression of the
form b
ARZ is a suitable relation to describe the rela-
tionship between these two parameters. The fundamen-
tal reason for the existence of such power law relation-
ships is the fact that Z and R are related to each other via
the raindrop size distribution. However, investigations
on the establishment of these relations have shown and
continue to show a great variability from a rainfall event
to another, within a given rainfall event and from a type
of precipitation to another [7-10,13]. For the case of
Africa, the variability of Z-R relationships is widely
documented by works of Sauvageot and Lacaux [4],
Nzeukou et al. [11], Moumouni et al. [12], Russell et al.
[14]. Thus, since Z-R relations proliferate in literature, it
is difficult to know if an instantaneous rain rate calcu-
lated with a fixed Z-R relation is necessarily correct,
even if rainfall accumulations should be reasonable

A. D. OCHOU ET AL.
148
when an appropriate climatological Z-R relation is used.
The rain rate R and the reflectivity factor Z being related
theoretically to the statistical moments of DSD, the mul-
tiplicity of their relationship would be primarily the re-
sult of the spatial and temporal variability of the DSD
[15]. Steiner et al. [16] also argued that the relationship
between Z and the rain rate R is very dependent on the
characteristics of drop size distributions and their evolu-
tion with rain rate. In other words, natural variations of
DSD characteristics between type of rain and from
storm to storm induce a variability in the Z-R relations
which affect the quantitative estimation of rain rate from
radar reflectivity.
To improve the accuracy of rainfall estimation by ra-
dar, numerous studies have given special attention to the
determination of Z-R relationships valid for different
climatic zones and particularly for different types of pre-
cipitation since many authors have strongly suggested
the coexistence of distinctly different convective and
stratiform DSDs within tropical systems [5]. In special
case of tropical Africa the convective and stratiform
modes of rainfall are especially important because pre-
cipitating clouds often organize into mesoscale convec-
tive systems containing the two distinctive environments.
Joss and Waldvogel [2] showed that application of Z-R
relationships for each type of precipitation (convective
or stratiform) would significantly increase the quality of
radar measurements of rainfall at daily time step. In that
scope, the precipitating systems are, on the basis of
rainfall patterns or defined criteria, generally classified
by type of precipitation with convective and stratiform
parts and sometimes a transition zone [5,17-21] between
both when considering rainfall events such as squall
lines.
Nevertheless, various investigations have revealed a
variety of situations: Yuter and Houze [17] attribute to
convective rains a multiplicative factor A higher than in
stratiform rain while Tokay and Short [5], Atlas et al.
[19], Narayana et al. [22], Maki et al. [7] show that the
factor A of the stratiform part is about twice higher than
the convective part, the exponent b varying a bit in both
cases. Such disparities, suspected to be a cause of under-
estimation or overestimation of rainfall from measured
radar reflectivity, implicitly highlight three important
issues:
 the fact that multiple rain rates can be associated
with the same reflectivity because of variations in
the drop size distribution;
 the problem of characterizing the nature of rainfall
and the reliability of partitioning algorithms in
stratiform/convective only according to the ground
signature of rainy events or following drop size dis-
tribution characteristics such as median volume di-
ameter [17];
 the difficulty of linking a Z-R relationship to a pre-
vailing physical or microphysical process which
leads to the formation or affects the DSD due to the
variety of processes in each type of rain.
The latter issue was addressed recently by Lee and
Zawadzki [10] through an analysis of the DSD variabil-
ity at different scales (climatologic, daily, within one day,
between physical processes and within a physical process)
and its implication on the Z-R relationship. Their work
showed that the DSD variability and therefore that of Z-R
relationship is more the result of complex dynamic,
thermodynamic and microphysical processes within
rainfall systems than a convective/stratiform classifica-
tion of the ground rainfall signature. Similar work has
been conducted by Rosenfeld and Ulbrich [23] through-
out a notable comprehensive review which ultimate goal
was to define the dominant properties of the drop size
distribution, their microphysical origin, and their asso-
ciation with the physical characteristics of the storm re-
sponsible for their generation. They explored the ques-
tion of the connections between raindrop size distribu
-tions and Z-R relationships from the combined approach
of rain-forming physical processes (such as coalescence,
breakup, evaporation, size sorting by drafts, ) that
shape the DSD, and a formulation of the DSD into the
simplest free parameters of the rain intensity R, rain wa-
ter content W and median volume diameter D0. Thereby,
they suggested that their results may be used to illustrate
the effects on the coefficient A and exponent b of each of
the various physical processes. Lee and Zawadzki [10] as
well as Rosenfeld and Ulbrich [23] came to the conclu-
sion that the classification or identification of various
physical processes within precipitating systems is essen-
tial to reduce the rainfall estimation errors through the
Z-R relationship.
In the present work, we do not discuss the physical
and microphysical processes underlying the variability of
Z-R relationship, which would require a description of
the vertical structure of precipitating systems from vol-
umetric radar measurements, for example. However, as
the raindrop number and size at ground are the result of
different processes mentioned above, a suitable approach
would be to analyze their variability in relation to that of
Z-R relationships at the rainfall event scales. Such a
study is interesting as some authors [10,24,25] reported
that the DSD variability explains 30% to 50% of errors
in the rain rate R estimation using the single traditional
Z-R method. Because of this DSD variability, the pre-
cipitations from different types of cloud may have simi-
lar intensities even though the associated couples “di-
ameter-number of raindrops” behave differently. Thus, it
appeared essential to study simultaneously the behavior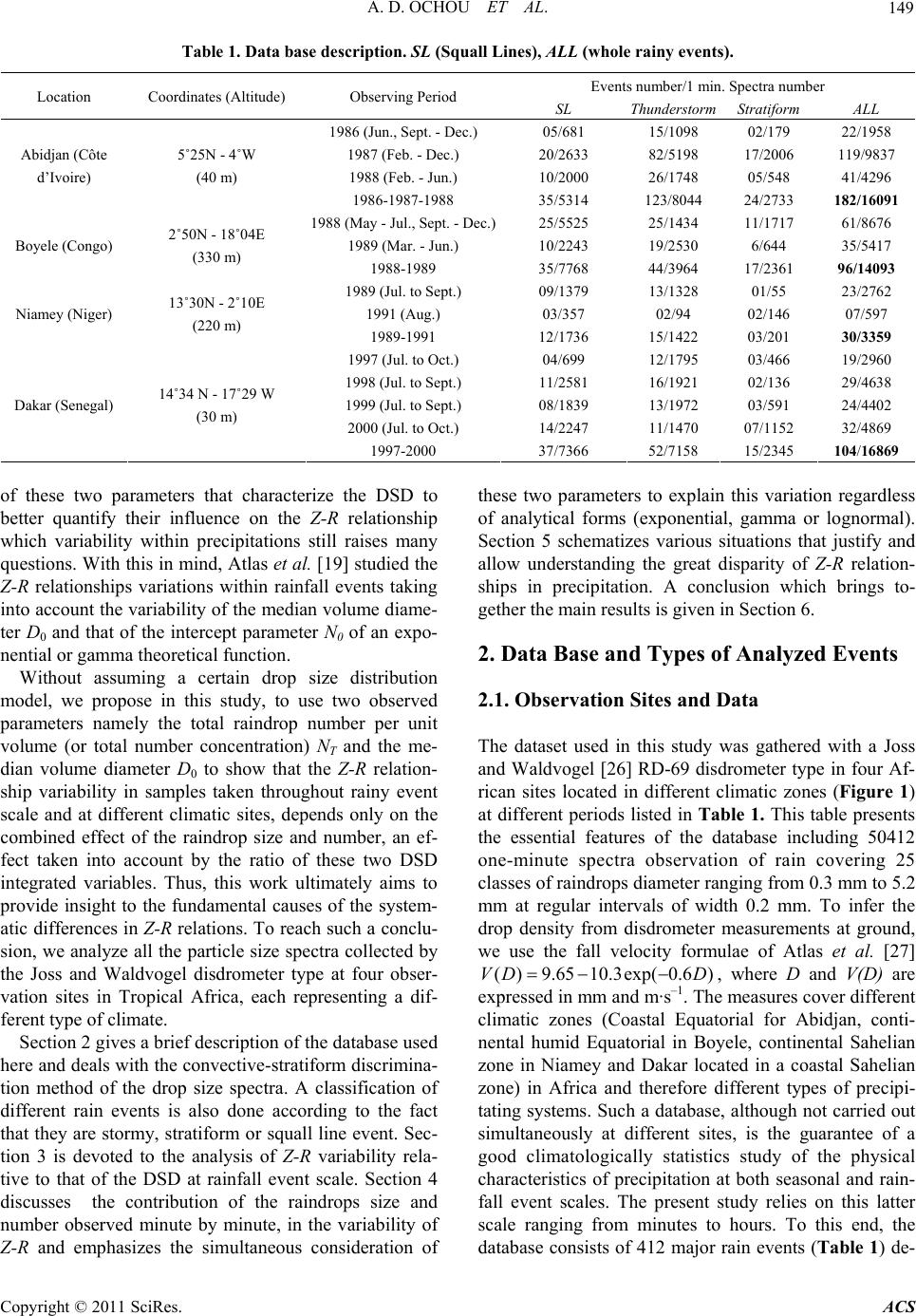

A. D. OCHOU ET AL.
149
Table 1. Data base description. SL (Squall Lines), ALL (whole rainy events).
Events number/1 min. Spectra number
Location Coordinates (Altitude) Observing Period SL Thunderstorm Stratiform ALL
1986 (Jun., Sept. - Dec.) 05/681 15/1098 02/179 22/1958
1987 (Feb. - Dec.) 20/2633 82/5198 17/2006 119/9837
1988 (Feb. - Jun.) 10/2000 26/1748 05/548 41/4296
Abidjan (Côte
d’Ivoire)
5˚25N - 4˚W
(40 m)
1986-1987-1988 35/5314 123/8044 24/2733 182/16091
1988 (May - Jul., Sept. - Dec.)25/5525 25/1434 11/1717 61/8676
1989 (Mar. - Jun.) 10/2243 19/2530 6/644 35/5417 Boyele (Congo) 2˚50N - 18˚04E
(330 m) 1988-1989 35/7768 44/3964 17/2361 96/14093
1989 (Jul. to Sept.) 09/1379 13/1328 01/55 23/2762
1991 (Aug.) 03/357 02/94 02/146 07/597 Niamey (Niger) 13˚30N - 2˚10E
(220 m) 1989-1991 12/1736 15/1422 03/201 30/3359
1997 (Jul. to Oct.) 04/699 12/1795 03/466 19/2960
1998 (Jul. to Sept.) 11/2581 16/1921 02/136 29/4638
1999 (Jul. to Sept.) 08/1839 13/1972 03/591 24/4402
2000 (Jul. to Oct.) 14/2247 11/1470 07/1152 32/4869
Dakar (Senegal) 14˚34 N - 17˚29 W
(30 m)
1997-2000 37/7366 52/7158 15/2345 104/16869
of these two parameters that characterize the DSD to
better quantify their influence on the Z-R relationship
which variability within precipitations still raises many
questions. With this in mind, Atlas et al. [19] studied the
Z-R relationships variations within rainfall events taking
into account the variability of the median volume diame-
ter D0 and that of the intercept parameter N0 of an expo-
nential or gamma theoretical function.
Without assuming a certain drop size distribution
model, we propose in this study, to use two observed
parameters namely the total raindrop number per unit
volume (or total number concentration) NT and the me-
dian volume diameter D0 to show that the Z-R relation-
ship variability in samples taken throughout rainy event
scale and at different climatic sites, depends only on the
combined effect of the raindrop size and number, an ef-
fect taken into account by the ratio of these two DSD
integrated variables. Thus, this work ultimately aims to
provide insight to the fundamental causes of the system-
atic differences in Z-R relations. To reach such a conclu-
sion, we analyze all the particle size spectra collected by
the Joss and Waldvogel disdrometer type at four obser-
vation sites in Tropical Africa, each representing a dif-
ferent type of climate.
Section 2 gives a brief description of the database used
here and deals with the convective-stratiform discrimina-
tion method of the drop size spectra. A classification of
different rain events is also done according to the fact
that they are stormy, stratiform or squall line event. Sec-
tion 3 is devoted to the analysis of Z-R variability rela-
tive to that of the DSD at rainfall event scale. Section 4
discusses the contribution of the raindrops size and
number observed minute by minute, in the variability of
Z-R and emphasizes the simultaneous consideration of
these two parameters to explain this variation regardless
of analytical forms (exponential, gamma or lognormal).
Section 5 schematizes various situations that justify and
allow understanding the great disparity of Z-R relation-
ships in precipitation. A conclusion which brings to-
gether the main results is given in Section 6.
2. Data Base and Types of Analyzed Events
2.1. Observation Sites and Data
The dataset used in this study was gathered with a Joss
and Waldvogel [26] RD-69 disdrometer type in four Af-
rican sites located in different climatic zones (Figure 1)
at different periods listed in Table 1. This table presents
the essential features of the database including 50412
one-minute spectra observation of rain covering 25
classes of raindrops diameter ranging from 0.3 mm to 5.2
mm at regular intervals of width 0.2 mm. To infer the
drop density from disdrometer measurements at ground,
we use the fall velocity formulae of Atlas et al. [27]
()9.6510.3exp( 0.6)VD D

, where D and V(D) are
expressed in mm and m·s–1. The measures cover different
climatic zones (Coastal Equatorial for Abidjan, conti-
nental humid Equatorial in Boyele, continental Sahelian
zone in Niamey and Dakar located in a coastal Sahelian
zone) in Africa and therefore different types of precipi-
tating systems. Such a database, although not carried out
simultaneously at different sites, is the guarantee of a
good climatologically statistics study of the physical
characteristics of precipitation at both seasonal and rain-
fall event scales. The present study relies on this latter
scale ranging from minutes to hours. To this end, the
database consists of 412 major rain events (Table 1) de-

A. D. OCHOU ET AL.
150
Figure 1. Geographical location of the rainfall measure-
ment data sites by the aid of disdrometer.
rived from the split of one-minute continuous data and
analyzed in this paper.
2.2. Precipitating Systems and Categorizing
Precipitation Types for DSD Data
In the study of precipitations, notably warm or liquid rain,
precipitation types are associated with clouds types in a
given climate zone. Since the nature of the precipitation
reflects the nature of the microphysical processes of
hydrometeor formation, growth, and transformation, it is
especially important to distinguish different modes of
rainfall occurring in Africa tropical regions. Thus, we
can analyze the similarities and differences between
raindrop size distributions in various precipitation re-
gimes and their impact on Z-R relationships. At surface,
considering single punctual measurements, as in case of
this study, the value of the rain rate R and its temporal
variability can be the simplest way to identify the type of
precipitation but did not provide information on micro-
structure of rain. Figure 2 shows the temporal evolution
of rainfall rate and DSD related to different types of pre-
cipitation. There are convective rainfall associated with
squall lines and isolated thunderstorms and rain associ-
ated with the non convective or stratiform clouds. Wide-
spread stratiform rains, usually from stratus clouds, are
characterized by low and moderate intensities less than
or equal to 10 mm·h–1, variable or stable over time and
can last several hours. They are usually observed in
monsoon periods or short dry season in tropical areas.
Figure 2(b) shows an example of these rains in Abidjan.
Rain from thunderstorm, are yielded by localized and
isolated precipitating convective clouds characterized by
one cell (Figure 2(c)) or more convective cells called
clusters (Figure 2(d)). The former (called unicellular
convective system) are relatively limited in time (about
20 minutes to an hour) when the latter, multi-cellular
may last several hours. These rainy convective systems
are characterized by high intensities which can reach
more than 100 mm·h–1 in the active phase of storm cells.
Figure 2(c) and Figure 2(d) show two typical cases of
stormy rainfall in Abidjan. Finally, squall lines consist of
precipitation generated by convective clouds multi-ce-
llular cumulonimbus, well organized. Their time signa-
ture at surface is characterized by two distinctive regions:
a leading part called convective of relatively short dura-
tion (20 - 30 minutes) and characterized by high rain
rates (up to 100 to 150 mm·h–1) and another part called
stratiform rain region which can last 1 to 6 hours with
little varying, moderate and low (below 10 mm·h–1) in-
tensities. Figure 2(a) shows a typical example of squall
lines observed in Abidjan with both convective (referred
by letter C) and stratiform region (referred by letter S).
Because characteristics of these regions of squall lines
are different, it is also important to categorize the DSD
data according to whether the spectra were obtained in
region of convective or stratiform precipitation. Accord-
ing to the standard convective-stratiform definition, pre-
cipitation type can be identified in the presence of si-
multaneous observations of vertical air velocities and the
terminal fall speed of hydrometeors [18,28] or based
upon reflectivity structure [17,29]. None of these two
methods of distinguishing convective from stratiform
rain is applicable in our study because neither radar data
nor draft magnitudes have been simultaneously collected
during measurements periods of raindrop size distribu-
tion used here. Since these observations are rare, Tokay
and Short [5] and Tokay et al. [18] determined the sepa-
ration between convective and stratiform precipitation on
the basis of a jump in the intercept parameter (N0) of the
gamma-fitted DSD, following the physical arguments of
Waldvogel [30]. Nevertheless, as we do not consider
specific theoretical DSD model in this study, precipita-
tion was classified as convective or stratiform basing on
hyetograph (time evolution of rain rate for a given rainy
event) according to the method proposed by Testud et al.
[6] and successfully applied by Moumouni et al. [12] and
Gosset et al. [31] on recent African rainfall observed in
northern Benin during the intensive campaign of the in-
ternational African Monsoon Multidisciplinary Analysis
(AMMA).
This approach is based on the fact that stratiform pre-
cipitations are low in intensity and have a large horizon-
tal extension. Thus, for a sequence of values {Ri} inten-
sities of rainfall from the hyetograph of a squall line, a
spectrum k is classified as stratiform only if its intensity
Rk and those of its 20 closest neighbors spectra (R10–k to
R10+k) are all smaller than the threshold value of 10
mm·h–1. Otherwise, this spectrum is classified convective.
In this latter case, the 20 adjacent spectra are also con-
sidered as convective. In this way, such a criterion allows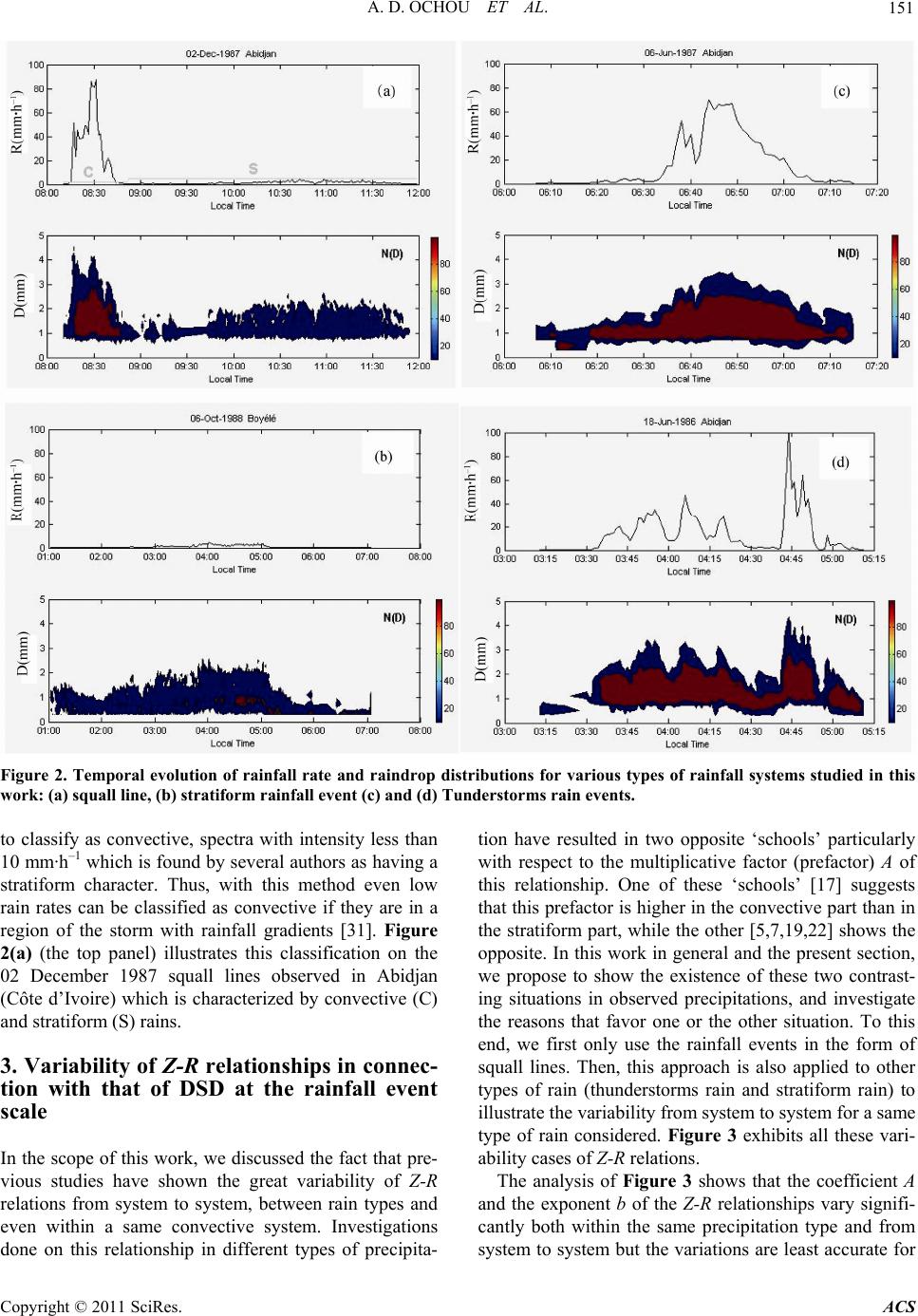

A. D. OCHOU ET AL.
151
Figure 2. Temporal evolution of rainfall rate and raindrop distributions for various types of rainfall systems studied in this
work: (a) squall line, (b) stratiform rainfall event (c) and (d) Tunderstorms rain events.
to classify as convective, spectra with intensity less than
10 mm·h–1 which is found by several authors as having a
stratiform character. Thus, with this method even low
rain rates can be classified as convective if they are in a
region of the storm with rainfall gradients [31]. Figure
2(a) (the top panel) illustrates this classification on the
02 December 1987 squall lines observed in Abidjan
(Côte d’Ivoire) which is characterized by convective (C)
and stratiform (S) rains.
3. Variability of Z-R relationships in connec-
tion with that of DSD at the rainfall event
scale
In the scope of this work, we discussed the fact that pre-
vious studies have shown the great variability of Z-R
relations from system to system, between rain types and
even within a same convective system. Investigations
done on this relationship in different types of precipita-
tion have resulted in two opposite ‘schools’ particularly
with respect to the multiplicative factor (prefactor) A of
this relationship. One of these ‘schools’ [17] suggests
that this prefactor is higher in the convective part than in
the stratiform part, while the other [5,7,19,22] shows the
opposite. In this work in general and the present section,
we propose to show the existence of these two contrast-
ing situations in observed precipitations, and investigate
the reasons that favor one or the other situation. To this
end, we first only use the rainfall events in the form of
squall lines. Then, this approach is also applied to other
types of rain (thunderstorms rain and stratiform rain) to
illustrate the variability from system to system for a same
type of rain considered. Figure 3 exhibits all these vari-
ability cases of Z-R relations.
The analysis of Figure 3 shows that the coefficient A
and the exponent b of the Z-R relationships vary signifi-
cantly both within the same precipitation type and from
system to system but the variations are least accurate for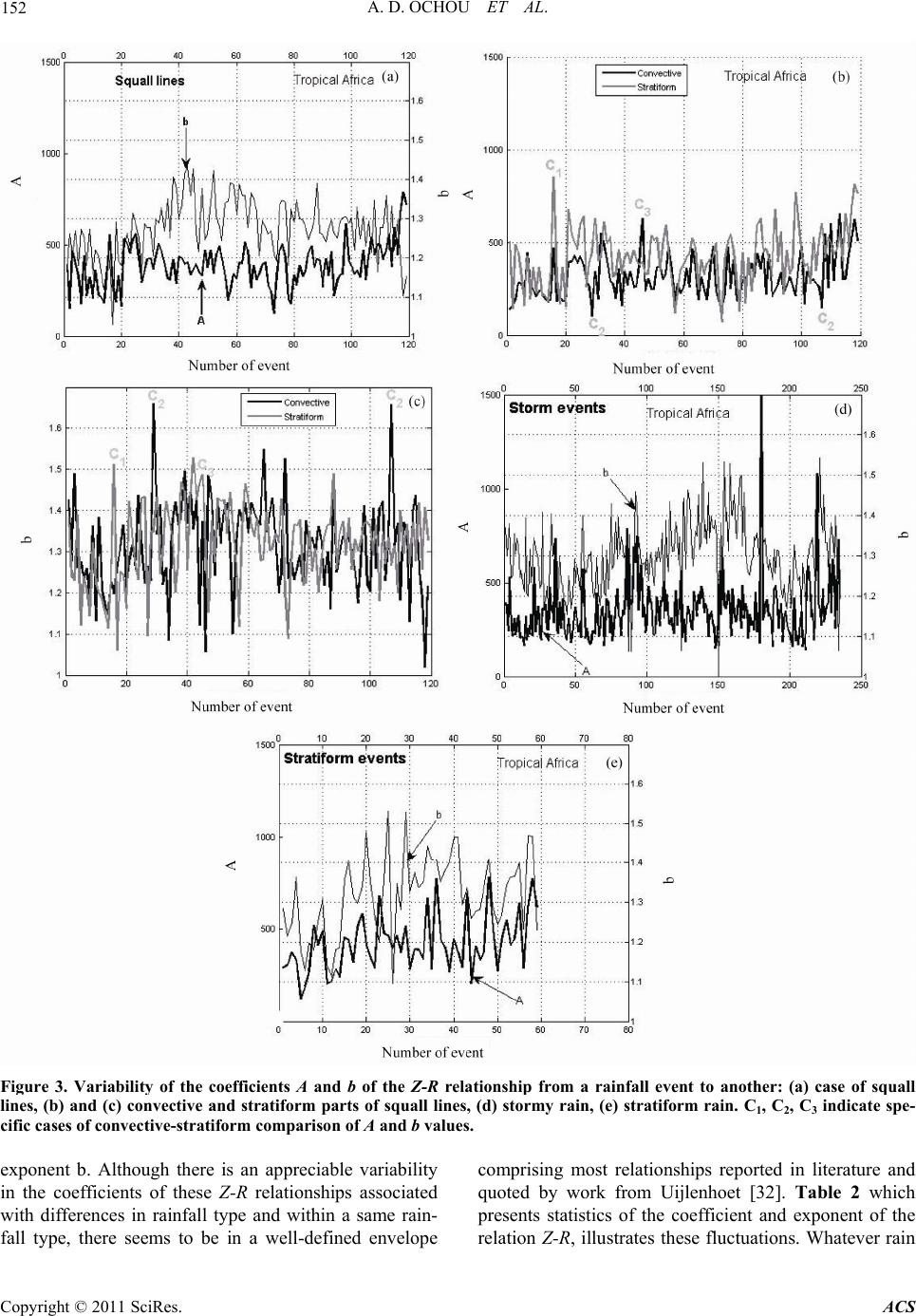

A. D. OCHOU ET AL.
152
Figure 3. Variability of the coefficients A and b of the Z-R relationship from a rainfall event to another: (a) case of squall
lines, (b) and (c) convective and stratiform parts of squall lines, (d) stormy rain, (e) stratiform rain. C1, C2, C3 indicate spe-
cific cases of convective-stratiform comparison of A and b values.
exponent b. Although there is an appreciable variability
in the coefficients of these Z-R relationships associated
with differences in rainfall type and within a same rain-
fall type, there seems to be in a well-defined envelope
comprising most relationships reported in literature and
quoted by work from Uijlenhoet [32]. Table 2 which
presents statistics of the coefficient and exponent of the
relation Z-R, illustrates these fluctuations. Whatever rain

A. D. OCHOU ET AL.
153
Table 2. Statistics of coefficient and exponent of the relation
between Z and R. Mean, Standard deviation, minimum, and
maximum values for various type of rainfall.
Rain types Z-R Coef.Mean Std dev MinMax
Squall lines (SL) A
b
384
1.28
116
0.07
127
1.03
788
1.43
Convective (SL) A
b
319
1.31
117
0.11
104
1.02
654
1.66
Stratiform (SL) A
b
420
1.31
150
0.10
72
1.06
852
1.53
Thunderstorms A
b
344
1.30
165
0.09
141
1.0
1924
1.54
Stratiform events A
b
417
1.32
153
0.10
118
1.09
777
1.53
All events A
b
366
1.30
153
0.09
118
1.0
1924
1.54
type, the coefficient A of the Z-R relationship shows a
great standard deviation corresponding to a variation
greater than 30% to the mean value of the related sample.
Specifically, Convective rainfall from squall lines is
characterized by prefactor A varying from 104 to 654,
with a mean value of 314 and a standard deviation of 117.
This standard deviation corresponds to a variation of
37% to the mean value. All the A values for stratiform
sample derived from squall lines are within the range 72
< A < 852 corresponding to a similar variation (36%) to
the related mean value as in the convective case in spite
of a distinctive mean value (420). An exceptional very
large A coefficient (A = 1924) is noted in thunderstorm
rainfall sample. Such values of the prefactor A (2754 and
1471) has been also reported by Ulbrich and Atlas [33]
analyzing DSD data from two major convective cells of a
continental storm observed at Arecibo, Puerto Rico (see
their Table 1). They probably refer to extreme rainfall
events.
In contrast to the coefficient A of the Z-R relationship,
the exponent b presents a relatively small variation (Ta-
ble 2), with mean value of 1.3 whatever rainfall type.
The standard deviation values of different rainfall types
account for variations under 9% to their respective mean
value.
Moreover, on the one hand, the results, especially in
the events described as squall lines, exhibit several situa-
tions illustrated by C1, C2 and C3 in Figures 3(b) and (c):
and
cS cs
A
Abb (case C1 in Figures 3(b) and (c))
and
cS cs
A
Abb (case C2 in Figures 3(b) and (c))
and
cS cs
A
Abb (case C3 in Figures 3(b) and (c)).
The coefficients Ac and bc are the values of factors A
and b of the Z-R relationship in the convective region
whereas As and bs are those for the stratiform part of the
squall lines. On the other hand, rainy events character-
ized by rain called stratiform (widespread and low rain-
fall) where the intensities hardly reach 10 mm·h–1, and
localized stormy rain have also different Z-R relations
from system to system considering each category of rain.
These examples confirm the great variability of Z-R
relations in precipitation and allow to accept the results
of Yuter and Houze [17] who obtained Ac > As and those
of Tokay and Short [5] who found on the contrary that Ac
< As. Their respective results can be justified by the fact
that they likely used squall lines related with different
intrinsic characteristics (raindrops number and size). In-
deed, Tokay and Short [5] observed two distinct groups
of DSD for the same rain rate (R = 6 mm·h–1), including
one being dominated by small drops and the other by
large drops, and both derived from convective and
stratiform parts respectively of a squall lines. Yuter and
Houze [17], unlike Tokay and Short [5], found that
stratiform precipitation contains a broad range of drop
spectra especially both large and narrow DSDs exist
within the same stratiform region of squall lines data.
From this, we can recognize, as argued Chandrasekar et
al. [34], the connection between DSD variability and the
values of A and b. However, the crucial issue to be con-
cerned in should be find an interpretation of the coeffi-
cients of resulting power law Z-R relationships in terms
of the parameters of the raindrop size distribution. In
other words, understand how the coefficients of such
relationships are related to the parameters of the raindrop
size distribution may help to explain their variability.
Previous works by Heinrich et al. [35], Rosenfeld and
Ulbrich [23], and Lee and Zawadzki [10], as mentioned
in introduction, have revealed the connection of domi-
nant physical processes with Z-R relationship. However,
the modifications of the DSD by these physical processes
generally lie in changes in drop size and number. For
instance, Heinrich et al. [35] have shown clear evidence
of a relationship between riming process, drops concen-
tration (represented by N0 parameter of a gamma func-
tion) and the median volume diameter, D0. They showed
that both N0 and D0 exhibit dramatic changes as riming
increased, at time without changes in rain rate. Rosenfeld
and Ulbrich [23] have shown the essential features of
DSD (in terms of drop size and number) resulting from
coalescence, break-up, accretion, size sorting by drafts,
wind shear, and evaporation. From this, obviously tack-
ling such connection between DSD variations and the
values of A and b implies simultaneous analysis of both
drops size and number.
The next section of this paper is devoted to the outline
of the approach to take into account the combined effect
of the DSD characteristics (drop size and number) and
understand its implication in the variability of Z-R rela-
tionships.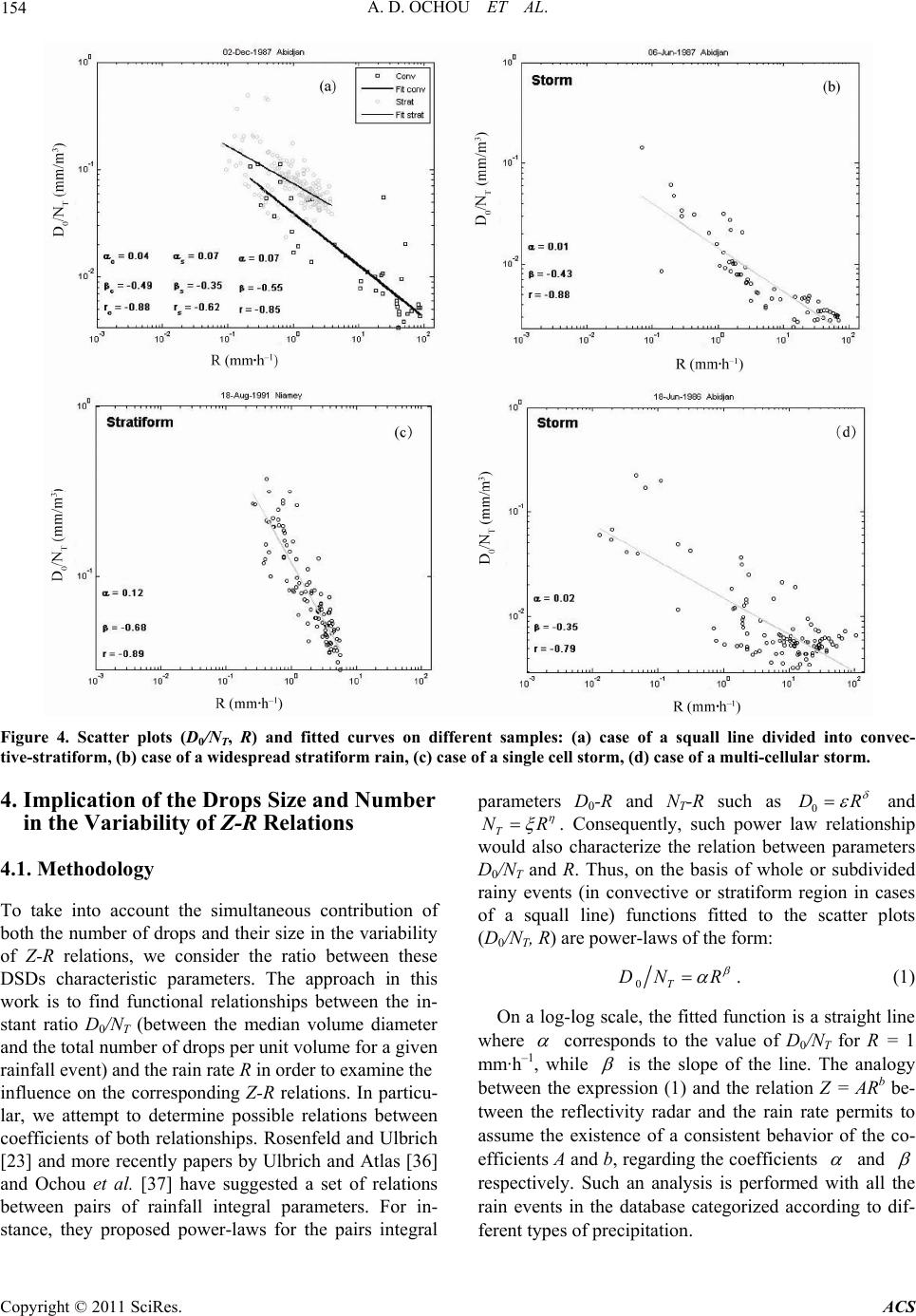

A. D. OCHOU ET AL.
154
Figure 4. Scatter plots (D0/NT, R) and fitted curves on different samples: (a) case of a squall line divided into convec-
tive-stratiform, (b) case of a widespread stratiform rain, (c) case of a single cell storm, (d) case of a multi-cellular storm.
4. Implication of the Drops Size and Number
in the Variability of Z-R Relations
4.1. Methodology
To take into account the simultaneous contribution of
both the number of drops and their size in the variability
of Z-R relations, we consider the ratio between these
DSDs characteristic parameters. The approach in this
work is to find functional relationships between the in-
stant ratio D0/NT (between the median volume diameter
and the total number of drops per unit volume for a given
rainfall event) and the rain rate R in order to examine the
influence on the corresponding Z-R relations. In particu-
lar, we attempt to determine possible relations between
coefficients of both relationships. Rosenfeld and Ulbrich
[23] and more recently papers by Ulbrich and Atlas [36]
and Ochou et al. [37] have suggested a set of relations
between pairs of rainfall integral parameters. For in-
stance, they proposed power-laws for the pairs integral
parameters D0-R and NT-R such as 0 DR


 and
T
NR


. Consequently, such power law relationship
would also characterize the relation between parameters
D0/NT and R. Thus, on the basis of whole or subdivided
rainy events (in convective or stratiform region in cases
of a squall line) functions fitted to the scatter plots
(D0/NT, R) are power-laws of the form:
0T
DN R

 . (1)
On a log-log scale, the fitted function is a straight line
where

corresponds to the value of D0/NT for R = 1
mm·h–1, while

is the slope of the line. The analogy
between the expression (1) and the relation Z = ARb be-
tween the reflectivity radar and the rain rate permits to
assume the existence of a consistent behavior of the co-
efficients A and b, regarding the coefficients

and

respectively. Such an analysis is performed with all the
rain events in the database categorized according to dif-
ferent types of precipitation.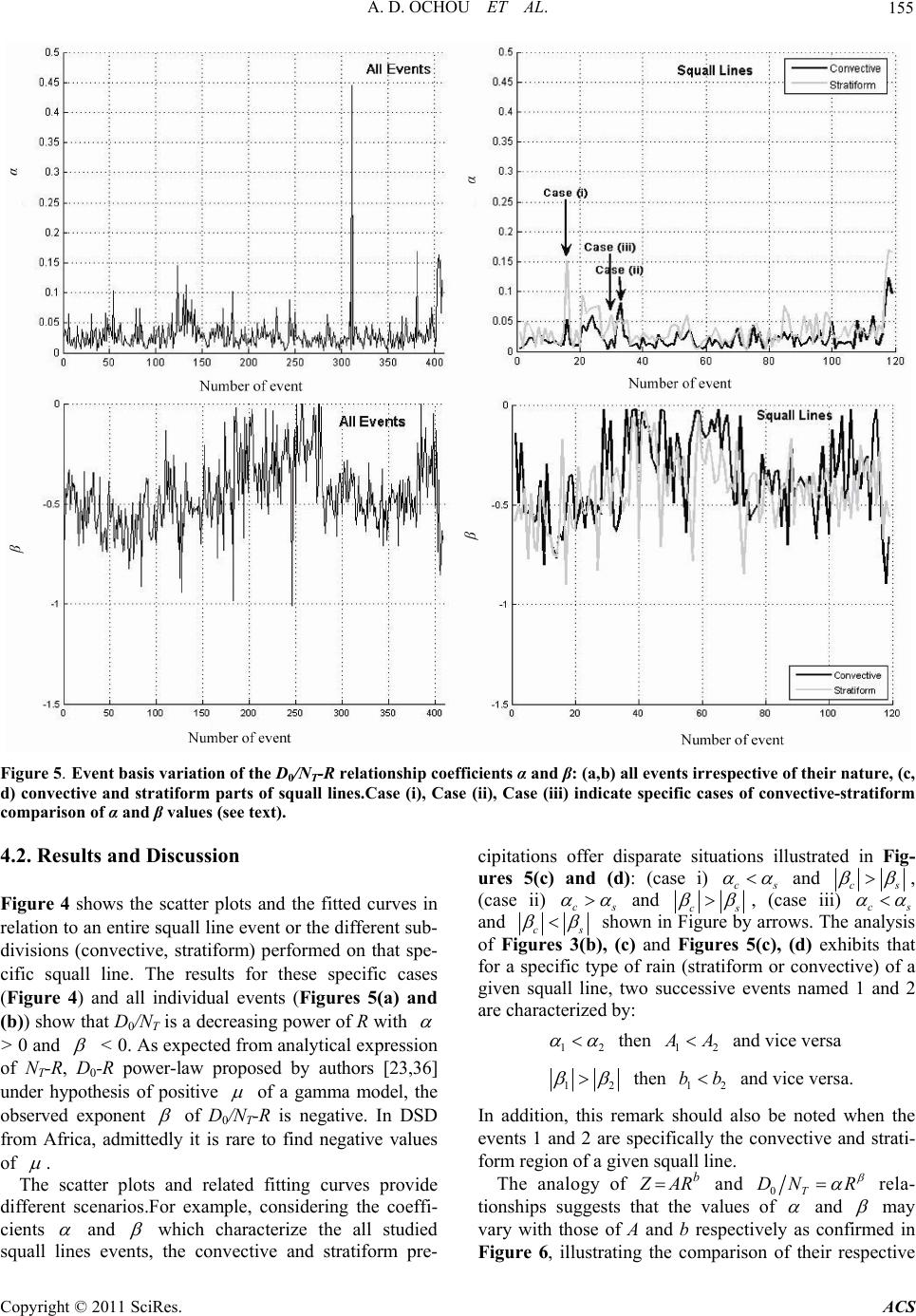

A. D. OCHOU ET AL.
155
Figure 5. Event basis variation of the D0/NT-R relationship coefficients α and β: (a,b) all events irrespective of their nature, (c,
d) convective and stratiform parts of squall lines.Case (i), Case (ii), Case (iii) indicate specific cases of convective-stratiform
comparison of α and β values (see text).
4.2. Results and Discussion
Figure 4 shows the scatter plots and the fitted curves in
relation to an entire squall line event or the different sub-
divisions (convective, stratiform) performed on that spe-
cific squall line. The results for these specific cases
(Figure 4) and all individual events (Figures 5(a) and
(b)) show that D0/NT is a decreasing power of R with

> 0 and

< 0. As expected from analytical expression
of NT-R, D0-R power-law proposed by authors [23,36]
under hypothesis of positive

of a gamma model, the
observed exponent

of D0/NT-R is negative. In DSD
from Africa, admittedly it is rare to find negative values
of

.
The scatter plots and related fitting curves provide
different scenarios.For example, considering the coeffi-
cients

and

which characterize the all studied
squall lines events, the convective and stratiform pre-
cipitations offer disparate situations illustrated in Fig-
ures 5(c) and (d): (case i) cs

  and cs

 ,
(case ii) cs

  and cs

 , (case iii) cs



and cs

  shown in Figure by arrows. The analysis
of Figures 3(b), (c) and Figures 5(c), (d) exhibits that
for a specific type of rain (stratiform or convective) of a
given squall line, two successive events named 1 and 2
are characterized by:
12



then 12
A
A

and vice versa
12

  then 12 bb and vice versa.
In addition, this remark should also be noted when the
events 1 and 2 are specifically the convective and strati-
form region of a given squall line.
The analogy of b ARZ  and 0T DN R

  rela-
tionships suggests that the values of

and

may
vary with those of A and b respectively as confirmed in
Figure 6, illustrating the comparison of their respective

A. D. OCHOU ET AL.
156
series at the scale of the rainfall event. The analysis of
these curves derived from miscellaneous rain events data
of different climatic zones shows those variations of A
and

on one hand and those of b and

on the other
Figure 6. Compared event basis variations of : (a) A and α,
(b) b and β.
hand, are remarkably similar: a strong variation of A
corresponds to a strong variation of

whereas a small
variation of b is associated with a low fluctuation of

.
We propose in following paragraph to determine the
functional relationship between A and

, then between
b and

.
Figure 7 and Figure 8 show the scatter plots of cou-
ples (A,

) and (b,

) respectively and the corre-
sponding regression curves. All the samples made with
various types of events (squall lines, thunderstorms,
widespread stratiform rain) from equatorial, tropical,
sahelian and guinean climatic zones, exhibit that the
multiplicative factor A of Z-R increases with

while
the exponent b decreases with

. As can be seen on
the graphs, fitting curves corresponding to each of the
relations are of the form:
2
1
a
Aa

, with 0

 (2)
12
bb b

, with 0

 (3)
The strong correlation coefficients greater than 0.80
demonstrates the close relationships existing between the
Table 3. Coefficients of the relationship 2
1
a
A=aα et b=
12
bβ+b for different types of précipitations.
Relationships
2
1
a
Aa

 12 bb b  
Rain types
a1 a
2 b 1 b 2
Squall lines (SL) 2172.9 0.486 0.4 1.46
Convective (SL) 1996.9 0.468 0.4 1.45
Stratiform (SL) 2332.6 0.499 0.5 1.52
Storms events 2187.8 0.508 0.4 1.49
Stratiform events 2448.8 0.521 0.5 1.53
All events 2273.7 0.510 0.4 1.49
Mean 2235.5 0.499 0.43 1.49

154.60 0.019 0.0516 0.0316
cv 0.069 0.04 0.12 0.02
multiplicative factors A and

and exponents b and

respectively. The quasi-similarity of functional relation-
ships obtained (listed in Table 3) using categorizing
samples of different rainfall events such as squall lines
and their convective and stratiform regions, thunder-
storms, widespread continuous light rain (stratiform rain),
taken from different latitudes, may be regarded as quasi
universal and thus applicable to other rain event samples.
The coefficients of variation (CV) of a1, a2, b1 and b2
parameters, lower than 15% (Table 3) confirm the al-
most constant character of those relations (2) and (3).
The unique relations should be of the form:
0.499
2235.5A   (4)
0.43 1.49b


 (5)
where the respective coefficients are the mean values of
individual ones derived from categorized precipitation
types (Table 3).
To emphasize the effect of the combination of NT and
D0 on the variability of Z-R relations through the coeffi-
cients A and b, we proposed to investigate the functional
relationships ()
A
f


and )(

fb  then ()Af


and ()bf


concerning the relationships b
Z
AR,
0
DR


 and T NR


.
This approach aims to show how A and b behave in
relation to the multiplicative factors

,

 and expo-
nents


,

 of D0 and NT separately in their relation-
ship with the rainfall rate R.
Figure 9 and Figure 10 present the scatter plots for
only convective and stratiform samples from squall lines.
Table 4 gathers the results for the six (6) samples studied
previously. Their comparison shows an uneven behavior
in the case of relations obtained with NT, while D0 be-
haves consistently (almost identical relations) as in the
case of the ratio D0/NT, whatever the type of rain. Indeed,
in the case of NT-R relation, we find sharp distinctive
relations between convective (A = 0.54 2981.6

; b =
0.48 1.55


) and stratiform (A = 0.63 5698.6

; b =
0.60 1.65


) sampled regions of squall lines. In addi-

A. D. OCHOU ET AL.
157
Figure 7. Relationship between the Z-R coefficient A and the D0/NT-R coefficient α: (a) combination of squall lines, (b) convec-
tive parts of squall lines, (c) stratiform parts of squall lines, (d) all rainfall systems, (e) storm water, (f) stratiform rain.
tion, the coefficient of variation in case of multiplicative
factors of A

  relative to NT is higher (16%) than in
the cases of A

 (4%) for D0, and A

 (7%) cor-
responding to the combination D0/NT.
Given the fact that Z and R both depend on the drops
size and number, this result shows that taking them into
account separately is not sufficient to explain the vari-
ability of A and b coefficients of Z-R relationship. Fur-

A. D. OCHOU ET AL.
158
Figure 8. Relationship between the coefficients b and β of D0/NT-R Z-R respectively: (a) combination of squall lines, (b) con-
vective part of squall lines, (c) stratiform part of squall lines, (d) all rainfall systems, (e) stormy rain events, (f) stratiform
rain events.
thermore, it shows that their variability is much more
dependent on the raindrops number (represented by NT)
than the size (represented by D0).
These results reasonably confirm that the Z-R rela-
tionships disparity and variability are not as random as it
seems, but depend closely on the precipitation intrinsic
characteristics such as the raindrops size and number,
taken into account here by

and

coefficients of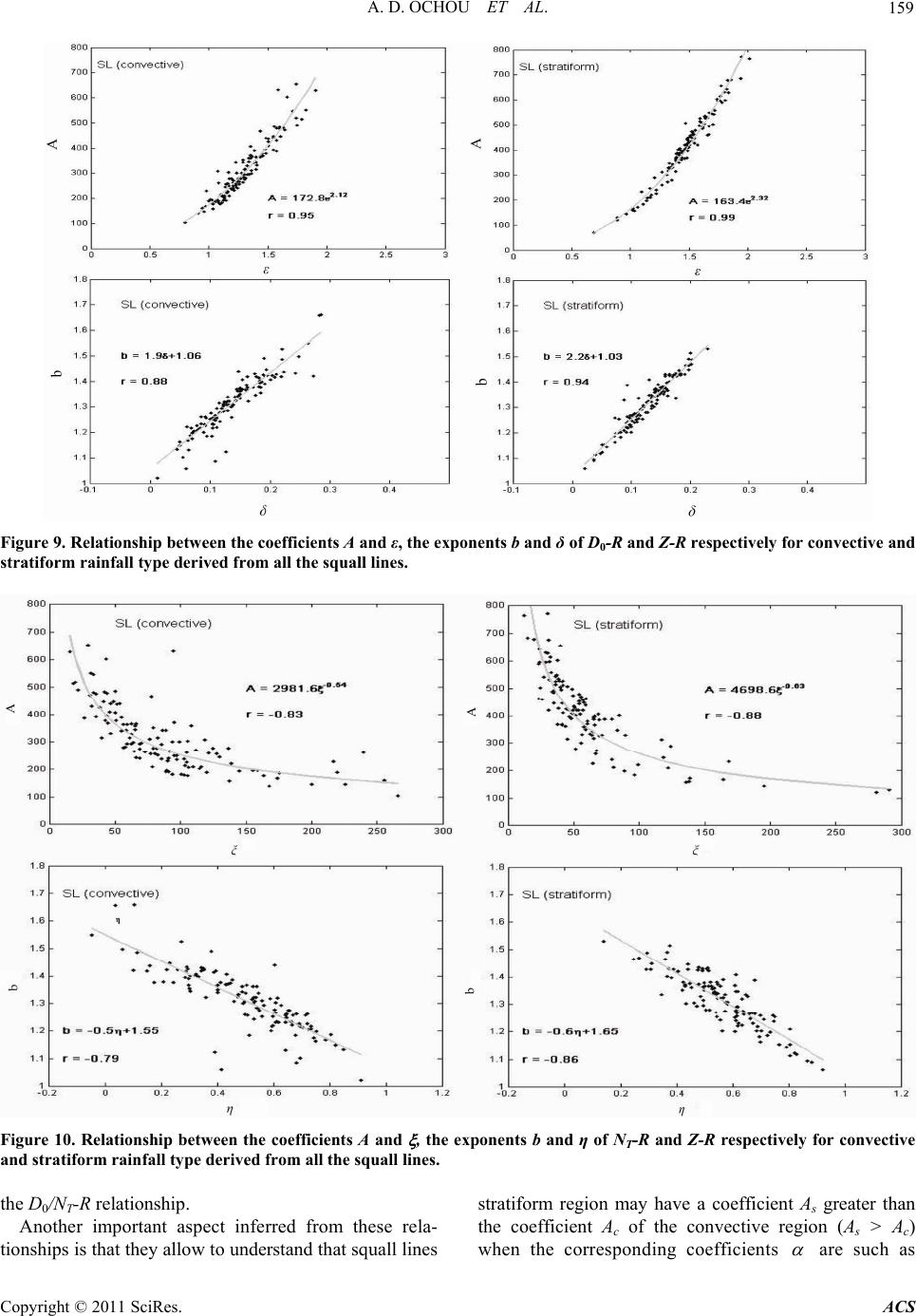

A. D. OCHOU ET AL.
159
Figure 9. Relationship between the coefficients A and ε, the exponents b and δ of D0-R and Z-R respectively for convective and
stratiform rainfall type derived from all the squall lines.
Figure 10. Relationship between the coefficients A and  , the exponents b and η of NT-R and Z-R respectively for convective
and stratiform rainfall type derived from all the squall lines.
the D0/NT-R relationship.
Another important aspect inferred from these rela-
tionships is that they allow to understand that squall lines
stratiform region may have a coefficient As greater than
the coefficient Ac of the convective region (As > Ac)
when the corresponding coefficients

are such as

A. D. OCHOU ET AL.
160
Table 4. Coefficients of the relationships A = f(x) and b = f(y) where x is the multiplicative factor and y the exponent of the
relationships between R and the parameters D0/NT, D0 and NT for different types of precipitations.
Relationships
D0/NT =
R


Relationships
D0 = R


Relationships
NT =
R


2
1
a
Aa

 12 bb b   2
1
c
A
c

 12 bd d 

 2
1
e
A
e

 12 bf f 


Rain types
a1 a
2 b 1 b 2 c 1 c 2 d 1 d 2 e 1 e 2 f 1 f 2
Squall lines (SL) 2172.9 0.486 0.4 1.46 161.52.338 2.0621.0404013.2 –0.596 –0.474 1.552
Convective (SL) 1996.9 0.468 0.4 1.45 172.82.122 1.8831.0552981.6 –0.536 –0.479 1.549
Stratiform (SL) 2332.6 0.499 0.5 1.52 163.42.317 2.2311.0294698.6 –0.628 –0.602 1.653
Storms events 2187.8 0.508 0.4 1.49 157.22.324 2.2041.0274344.4 –0.638 –0.534 1.602
Stratiform events 2448.8 0.521 0.5 1.53 158.32.367 2.2271.0334935.5 –0.650 –0.597 1.659
All events 2273.7 0.510 0.4 1.49 158.02.346 2.1891.0304489.8 –0.637 –0.534 1.601
Mean 2235.5 0.499 0.43 1.49 161.92.302 2.1331.0364234.8
–0.614 –0.537 1.603

154.60 0.019 0.0516 0.03165.86 0.0901 0.1370.011693.1 0.042 0.055 0.047
cv 0.069 0.04 0.12 0.02 0.0360.039 0.0640.0100.163 –0.069 –0.103 0.030
s
c

 . Similarly, if both regions are such as
s
c



,
we will then have As < Ac. These two situations are quite
possible since it depends on the simultaneous behavior of
the raindrops size and number in distinctive regions of
the squall line, taken in account through the ratio D0/NT
in this work. Indeed, if a stratiform part of a given squall
line is characterized by large but few drops (high D0,
small NT), the ratio D0/NT will be higher than in the con-
vective part which is characterized by large but more
numerous drops (high D0, high NT) providing lower
D0/NT ratios.The radar reflectivity factor Z depending on
the 6th power of the diameter, remains relatively high in
the stratiform region where R, which depends on the 3rd
power of the diameter, is very weak. Because of the high
values of Z in this part, the relationship s
b
sRAZ is
such as As (Z value for R=1 mm·h–1) must be high
enough to “compensate” for low values of R. On the
other hand, in the convective part where Z and R are
characterized by high values, the coefficient Ac, although
high, remains very often lower than As (As > Ac). Con-
versely if a squall line’s stratiform region is such as low
rain rates are due to small but more numerous drops
(small D0, high NT)―yielding a low D0/NT ratio value-
the radar reflectivity factor Z is low as well as the rain
rate R. Both parameters varying in the same sense, the
relationship s
b
sRAZ in this part is such as As would
be logically low. In the corresponding convective region,
because of high D0 and high NT, the ratio D0/NT, although
low, remains higher than in the stratiform region.The Z-R
coefficient Ac would be then higher than As of the strati-
form region (As < Ac). These two situations described
above are fairly well illustrated by the squall lines rain-
fall events observed respectively on 17 October 1987 in
Abidjan (Côte d’Ivoire) marked by As > Ac and on 1st
July 1988 in Boyele (Congo) where on the contrary we
have As < Ac (Figure 11).
5. Conceptual Schematization of Possible
Situations
The Z-R relationships, being characterized simultane-
ously by the coefficients A and b, their respective behav-
iors with respect to

and

suggests several possi-
ble combinations existing in the precipitations. In this
section we propose a schematization of different situa-
tions that justify and allow understanding the great dis-
parity of the Z-R relationships in the precipitations. To
do this, consider two rainfall events indexed 1 and 2
which can be independent rainfall events or convective
and stratiform regions of the same event (case of squall
lines). Let us assume a particular situation where the
relations D0/NT = R

 are such as the slopes

are
identical


12

  and the multiplicative factors
(the intercept in log-log scale) are different


12

 .
We have thus the following relations:
01 1
b
T
DNRZAR

  (6)
02 2
b
T
DNRZ AR

  (7)
There are two corresponding possibilities named P1
and P2 shown schematically in Figures 12(a) and (c) in
a log-log scale.
Let us now suppose another particular situation where
relations D0/NT = R

 are such as the multiplicative
factors are identical


12

  and the slopes dif-
ferent


12

 . We therefore have the following rela-
tions:
11
0
b
TARZRND 

 (8)
22
0
b
TARZRND 

 (9)
which also refer to two possibilities 1 P and 2 P

rep-
resented schematically in Figures 12(b) and (d).
However, it should be noted that the situations de-
scribed above are particular cases rarely observed. In-

A. D. OCHOU ET AL.
161
Figure 11. Time series of the rain rate R, the reflectivity factor Z, the raindrops number NT, the mean volume diameter D0
and their ratio D0/NT for two rainfall events observed in Abidjan ((a)-(c)) and Boyele ((d)-(f)).
deed, given the high variability of the raindrops size and
number in the precipitations, it is uncommon to see two
independent events and especially the convective and
stratiform regions of a single rainfall event, be charac-
terized by relations Z-R having the same multiplicative
factor A or the same exponent b. In the observed precipi-
tations, different parts of a rain event are characterized
by a common area (mixing) especially in low and mod-
erate rain rates, so that the intercepts (A or

) and
slopes (b or

) undergo more or less strong changes
depending
On the degree of mixing and the raindrops number and
size in attendance, the actual situations are therefore de-
scribed by the combinations (1
P + 1 P’ ), (1 P + 2 P’ ),
(2 P + 1 P’ ) and (2 P + 2 P’ ), represented in Figure 13.
These situations occur in the rainfall events observed in
this study. They show the great variability of Z-R rela-
tionships within a single rainfall event and between inde-
pendent events. Thus, in the case where samples 1 and 2
represent the convective and stratiform regions of a squall
line, this analysis gives the reasons why Yuter and Houze
[17] found that the coefficient A in the convective part is
higher than that of the stratiform part (Ac > As) and those
for which, Tokay and Short [5], conversely, obtained Ac <

A. D. OCHOU ET AL.
162
Figure 12. Conceptual schematization of specific situations
in observed rainfall events to understand the variability of
Z-R relationships.
Figure 13. Conceptual schematization of possible actual
situations showing Z-R relationships variability in observed
rainfall events.
As. In fact, their respective results are shown for the for-
mer by the situations (1 P + 1 P’ ) or (1 P + 2 P’ ) and for
the latter by the situations (2 P + 1 P’ ) or (2 P + 2 P’ ).
Each of these situations may exist predominantly in a
climatic zone with its own characteristics; we can there-
fore understand the two trends as well as the high vari-
ability and the disparity of Z-R relationships. These re-
sults suggest us not directly assign categories of Z-R re-
lationships to precipitation types called ‘convective’ and
‘stratiform’ considering the only rain rates which do not
always reflect the precipitating system intrinsic charac-
teristics, but to take into account simultaneously the
drops size and number. For example, using radar ob-
ervations, Yuter and Houze [17] showed that the strati-
form region characteristics of a squall line depend on the
degree and nature of dynamic and thermo-dynamic
processes prevailing in the convective part.Thus, taking
into account only drops diameters as Atlas et al. [19] and
Tokay and Short [5] did or the only drops number
[18,30], is not sufficient to discrimi nate precipitation.
From ground-based measurements, the simultaneous
consideration of both characteristic parameters of the
DSD, which are the raindrops number and size, allow to
better understand the reasons of the Z-R relationships
variability in the precipitations.
6. Conclusions
The variability of the relationship between the rain rate R
and the radar reflectivity factor Z, useful in estimating
rainfall by radar, has been a major concern in this work.
The study of relationships in independent rainfall
events confirmed their great variability from a given pre-
cipitation to another and within the same precipitation
type. Based on a sampling of whole and/or subdivided
events, it appeared that the convective (indexed c) and
stratiform (indexed s) regions of squall lines are charac-
terized by multiplicative factors such as Ac > As or Ac <
As as well as exponents such as bc > bs or bc < bs. The
analysis of the rain drops size and number, respectively
indicated by D0 and NT, showed that the behavior of Z-R
relationships is primarily due to their combined effect.
This contribution of both DSD integral parameters has
been taken into account considering their ratio D0/NT.
The comparative study of Z-R relationship and
D0/NT =


0R



showed a consistent behavior of
the coefficients A and b compared to the coefficients α
and

respectively. Indeed, this study achieved with
samples of different types whole events or subdivided
into convective/stratiform regions (in case of squall
lines), at all observation sites put together, allowed to
reveal relationships almost similar and independent of
the precipitation nature. It has been established
that A and b are power and linear functions of

and

respectively, such as A = 2235.5 0.499  and
b = 0.43

+ 1.49. This result, obtained with different
types of precipitation, shows that taking into account the
raindrops size and number through the simple ratio D0/NT
enable to predict how the Z-R relationship coefficients A
and b may vary in rain events. It has been also shown
that their variability is much more dependent on the
raindrops number than the size. In addition, while show-
ing the non-random variability of the Z-R relationships,
these results allow us to understand that the convective
region of a squall line may have a coefficient A higher or
lower than the stratiform region. We so understand that
the work of the ‘school Ac > As’ [17] and those of the
‘school Ac < As’ [5] are not contradictory, their results
being probably related to precipitations with different
physical characteristics.
We conclude that in a future study, we can use the
combination of rain drop size and number to attempt a
differentiation between stratiform and convective pre-
cipitation since Heinrich et al. [35] have shown clear
evidence of a relationship between riming processes (an
indication of updrafts and convection) and raindrop

A. D. OCHOU ET AL.
163
spectra’s intrinsic integrated parameters namely the in-
tercept parameter N0 of an exponential model fitting ob-
served DSD and the median volume diameter, D0.
7. Acknowledgements
The authors are grateful to all of those who contributed
to the data set used in this study. Greatly appreciated are
the advice, detailed comments on the manuscript and
discussions with Prof. Henri Sauvageot. We are also
grateful to the PASRES for their financial contribution.
8. References
[1] J. S. Marshall and W. M. K. Palmer, “The Distribution of
Raindrops with Size,” Journal of Meteorology, Vol. 5,
No. 51948, pp. 165-166.
doi:10.1175/1520-0469(1948)005<0165:TDORWS>2.0.
CO;2
[2] J. Joss and A. Waldvogel, “Raindrop Size Distribution
and Doppler Velocities,” Proceedings of the 14th Radar
Meteorology Conference, Boston, 1970, pp. 153-156.
[3] P. T. Willis, “Functional Fit to Some Observed Drop Size
Distributions and Parameterization of Rain,” Journal of
the Atmospheric Sciences, Vol. 41, No. 9, 1984, pp.
1648-1661.
doi:10.1175/1520-0469(1984)041<1648:FFTSOD>2.0.C
O;2
[4] H. Sauvageot and J. P. Lacaux, “The Shape of Averaged
Drop Size Distributions,” Journal of the Atmospheric
Sciences, Vol. 52, No. 8, 1995, pp. 1070-1083.
doi:10.1175/1520-0469(1995)052<1070:TSOADS>2.0.C
O;2
[5] A. Tokay and D. A. Short, “Convective vs Stratiform
Rain in the West Pacific during TOGA COARE: Evi-
dence from Raindrop Spectra,” Journal of Applied Mete-
orology, Vol. 35, No. 3, 1996, pp. 355-371.
doi:10.1175/1520-0450(2001)040<1118:TCONDT>2.0.C
O;2
[6] J. Testud, S. Oury, R. A. Black, P. Amayenc and X. Dou,
“The Concept of Normalized Distribution to Describe
Raindrop Spectra: A Tool for Cloud Physics and Cloud
Remote Sensing,” Journal of Applied Meteorology, Vol.
40, No. 6, 2001, pp. 1118-1140.
doi:10.1175/1520-0450(2001)040<1118:TCONDT>2.0.C
O;2
[7] M. Maki, T. D. Keenam, Y. Sasaki and K. Nakamura,
“Characteristics of the Raindrop Size Distribution in
Tropical Continental Squall Lines Observed in Darwin,
Australia,” Journal of Applied Meteorology, Vol. 40, No.
8, 2001, pp. 1393-1412.
doi:10.1175/1520-0450(2001)040<1393:COTRSD>2.0.C
O;2
[8] R. Uijlenhoet, J. A. Smith and M. Steiner, “The Micro-
physical Structure of Extreme Precipitation as Inferred
from Ground-Based Raindrop Spectra,” Journal of the
Atmospheric Sciences, Vol. 60, No. 10, 2003, pp. 1220-
1238.
doi:10.1175/1520-0469(2003)60<1220:TMSOEP>2.0.C
O;2
[9] R. Uijlenhoet, M. Steiner and J. A. Smith, “Variability of
Raindrop Size Distributions in a Squall Line and Implica-
tions for Radar Rainfall Estimation,” Journal of Hydro-
meteorology, Vol. 4, No. 1, 2003, pp. 43-61.
doi:10.1175/1525-7541(2003)004<0043:VORSDI>2.0.C
O;2
[10] G. W. Lee and I. Zawadzki, “Variability of drop size
distributions: Time-Scale Dependence of the Variability
and its Effects on Rain Estimation,” Journal of Applied
Meteorology, Vol. 44, No. 2, 2005, pp. 241-255.
doi:10.1175/JAM2183.1
[11] A. Nzeukou, H. Sauvageot, A. D. Ochou and C. M. F.
Kebe, “Raindrop Size Distribution and Radar Parameters
at Cape Verde,” Journal of Applied Meteorology, Vol. 43,
No. 1, 2004, pp. 90-105.
doi:10.1175/1520-0450(2004)043<0090:RSDARP>2.0.C
O;2
[12] S. Moumouni, M. Gosset, E. Houngninou, “Main Fea-
tures of Rain Drop Size Distributions Observed in Benin,
West Africa, with Optical Disdrometers,” Geophysical
Research Letters, Vol. 35, 2008, L23807.
doi:10.1029/2008GL035755
[13] L. J. Battan, “Radar Observation of the Atmosphere,”
University of Chicago Press, Chicago, 1973.
[14] B. Russell, E. R. Williams, M. Gosset, F. Cazenave, L.
Descroix, N. Guy, T. Lebel, A. Ali, F. Metayer and G.
Quantin, “Radar Rain-Gauge Comparisons on Squall
Lines in Niamey, Niger for the AMMA,” Quarterly
Journal of the Royal Meteorological Society, Vol. 136,
No. S1, 2010, pp. 290-304. doi:10.1002/qj.548
[15] A. D. Ochou, “Variabilité Spatio-Temporelle des Mo-
ments Statistiques des Distributions des Gouttes de Pluie
et Conséquences sur la Mesure des Précipitations par Té-
lédétection Micro-Ondes,” Ph.D. Dissertation, Université
Cocody-Abidjan, Abidjan, 2003.
[16] M. Steiner, J. A. Smith and R. Uijlenhoet, “A Micro-
physical Interpretation of Radar Reflectivity-Rain Rate
Relationships,” Journal of the Atmospheric Sciences, Vol.
61, No. 10, 2004, pp. 1114-1131.
doi:10.1175/1520-0469(2004)061<1114:AMIORR>2.0.C
O;2
[17] S. E. Yuter and R. A. Houze, “Measurements of Raindrop
Size Distribution over the Pacific Warm Pool and Im-
plementations for Z-R Relations,” Journal of Applied
Meteorology, Vol. 36, No. 7, 1997, pp. 847-867.
doi:10.1175/1520-0450(1997)036<0847:MORSDO>2.0.
CO;2
[18] A. Tokay, D. A. Short, C. R. Williams, W. L. Ecklund
and K. S. Gage, “Tropical Rainfall Associated with Con-
vective and Stratiform Clouds: Intercomparison of Dis-
drometer and Profiler Measurements,” Journal of Applied
Meteorology, Vol. 38, No. 3, 1999, pp. 302-320.
doi:10.1175/1520-0450(1999)038<0302:TRAWCA>2.0.
CO;2
[19] D. Atlas, C. W. Ulbrich, F. D. Marks Jr., E. Amitai and C.
R. Williams, “Systematic Variation of Drop Size and

A. D. OCHOU ET AL.
164
Radar-Rainfall Relations,” Journal of Geophysical Re-
search, Vol. 104, No. D6, 1999, pp. 6155-6169.
doi:10.1029/1998JD200098
[20] D. Atlas, C. W. Ulbrich, F. D. Marks Jr., R. A. Black, E.
Amitai, P. T. Willis and C. E. Samsury, “Partitioning
Tropical Oceanic Convective and Stratiform Rains by
Draft Strength,” Journal of Geophysical Research, Vol.
105, No. D2, 2000, pp. 2259-2267.
doi:10.1029/1999JD901009
[21] C. W. Ulbrich and D. Atlas, “On the Separation of
Tropical Convective and Stratiform Rains,” Journal of
Applied Meteorology, Vol. 41, No. 2, 2002, pp. 188-195.
doi:10.1175/1520-0450(2002)041<0188:OTSOTC>2.0.C
O;2
[22] T. Narayana Rao, D. Narayana Rao and K. Mohan,
“Classification of Tropical Precipitating Systems and
Associated Z-R Relationships,” Journal of Geophysical
Research, Vol. 104, 2001, pp. 17699-17711.
[23] D. Rosenfeld and C. W. Ulbrich, “Cloud Microphysical
Properties, Processes, and Rainfall Estimation Opportuni-
ties. Radar and Atmospheric Science: A Collection of
Essays in Honor of David Atlas,” Meteorological Mono-
graphs, the American Meteorological Society, Boston,
No. 52, 2003, pp. 237-258.
[24] J. W. F. Goddard and S.M. Cherry, “The Ability of Dual
Polarization Radar (Co-Polar Linear) to Predict Rainfall
Rate and Microwave Attenuation,” Radio Science, Vol.
19, No. 1, 1984, pp. 201-208.
doi:10.1029/RS019i001p00201
[25] N. Balakrishnan, D. S. Zrnic, J. Goldhirsh and J. Row-
land, “Comparison of Simulated Rain Rate from Disdro-
meter Data Employing Polarimetric Radar Algorithms,”
Journal of Atmospheric and Oceanic Technology, Vol. 6,
No. 3, 1989, pp. 476-486.
doi:10.1175/1520-0426(1989)006<0476:COSRRF>2.0.C
O;2
[26] J. Joss and A. Waldvogel, “Raindrop Size Distribution
and Sampling Size Errors,” Journal of the Atmospheric
Sciences, Vol. 26, No. 3, 1969, pp. 566-569.
doi:10.1175/1520-0469(1969)026<0566:RSDASS>2.0.C
O;2
[27] D. Atlas, R. C. Srivastava and R. S. Sekhon, “Doppler
Radar Characteristics at Vertical Incidence,” Reviews of
Geophysics, Vol. 11, No. 1, 1973, pp. 1-35.
doi:10.1029/RG011i001p00001
[28] R. A. Houze Jr., “Cloud Dynamics,” Academic Press,
New York, 1993.
[29] M. Steiner, R. A. Houze Jr. and S. E. Yuter, “Clima-
tological Characterization of Three-Dimensional Storm
Structure from Operational Radar and Rain Gauge Data,”
Journal of Applied Meteorology, Vol. 34, No. 9, 1995, pp.
1978-2007.
doi:10.1175/1520-0450(1995)034<1978:CCOTDS>2.0.C
O;2
[30] A. Waldvogel, “The N0 Jump of Raindrop Spectra,”
Journal of the Atmospheric Sciences, Vol. 31, No. 4,
1974, pp. 1068-1078.
doi:10.1175/1520-0469(1974)031<1067:TJORS>2.0.CO;
2
[31] M. Gosset, E.-P. Zahiri and S. Moumouni, “Rain Drop
Size Distribution Variability and Impact on X-Band Po-
larimetric Radar Retrieval: Results from the AMMA
Campaign in Benin,” Quarterly Journal of the Royal Me-
teorological Society, Vol. 136, No. S1, 2010, pp. 243-356.
doi:10.1002/qj.556
[32] R. Uijlenhoet, “Raindrop Size Distributions and Radar
Reflectivity-Rain Rate Relationships for Radar Hydrol-
ogy,” Hydrology and Earth System Sciences, Vol. 5, No.
4, 2001, pp. 615-627.
[33] C. W. Ulbrich and D. Atlas, “Radar Measurements of
Rainfall with and without Polarimetry,” Journal of Ap-
plied Meteorlogy Climate, Vol. 31, No. 4, 2008, pp.
1067-1078. doi:10.1175/2007JAMC1804.1.
[34] V. Chandrasekar, R. Meneghini and I. Zawadzki, “Global
and Local Precipitation Measurements by Radar. Radar
and Atmospheric Science: A collection of Essays in
honor of David Atlas,” Meteorology Monograohy, No. 52,
American Meteorology Society, 2003, pp. 215-236.
[35] W. J. Heinrich, J. Joss and A. Waldvogel, “Raindrop Size
Distributions and the Radar Bright Band,” Journal of Ap-
plied Meteorology, Vol. 35, No. 10, 1996, pp. 1688-1701.
doi:10.1175/1520-0450(1996)035<1688:RSDATR>2.0.C
O;2
[36] C. W. Ulbrich and D. Atlas, “Microphysics of Raindrop
Size Spectra: Tropical Continental and Maritime Storms,”
Journal of Applied Meteorology and Climatology, Vol.
46, No. 11, 2007, pp. 1777-1791.
doi:10.1175/2007JAMC1649.1
[37] A. D. Ochou, A. Nzeukou and H. Sauvageot, “Pa-
rametrization of Drop Size Distribution with Rain
Rate,” Atmospheric Research, Vol. 84, No. 1, 2007,
pp. 58-66. doi:10.1016/j.atmosres.2006.05.003