 Journal of Applied Mathematics and Physics, 2015, 3, 1207-1217 Published Online September 2015 in SciRes. http://www.scirp.org/journal/jamp http://dx.doi.org/10.4236/jamp.2015.39148 How to cite this paper: Bilgin, T. (2015) (f, p)-Asymptotically Lacunary Equivalent Sequences with Respect to the Ideal I. Journal of Applied Mathematics and Physics, 3, 1207-1217. http://dx.doi.org/10.4236/jamp.2015.39148 (f, p)-Asymptotically Lacunary Equivalent Sequences with Respect to the Ideal I Tunay Bilgin Department of Mathematics, Education Faculty, Yuzuncu Yil University, Van , Turk ey Email: tbilgin@yyu.edu.tr Received 6 Octo ber 20 14; accepted 27 September 2015; published 30 September 2015 Copyright © 2015 by author and Scientific Research Publishing Inc. This work is licensed under the Creative Commons Attribution International License (CC BY). http://creativ ecommon s.org/l icenses/by/4. 0/ Abstract In this study, we define (f, p)-Asymptotically Lacunary Equivalent Sequences with respect to the ideal I using a non-trivial ideal , a lacunary sequence , a strictly positive se- quence , and a modulus function f, and obtain some revelent connections between these notions . Keywords Asymptotically Equivalenc e, Ideal Convergence , Lacunary Seque nce, Modulus Function, Statistically Limit 1. Introduction Let denote the spaces of all real sequences, bounded, and convergent sequences,respectively. Any subspace of s is called a sequence space. Following Freedman et al. [1], we call the sequence lacunary if it is an increasing sequence of integers such that as . The intervals determined by will be denoted by and . These notations will be used troughout the paper. The sequence space of lacunary stron gly co nvergent s equences was defined by Freedman et al. [1], a s follows: ( ) 1 :lim0for some r irr i iI Nx xshxss θ − ∈ = =∈−= ∑ . The notion of modulus function was introduced by Nakano [2]. We recall that a modulus f is a function fro m to such that 1) if and only if , 2) for , 3) f is increasing and 4) f is continuous from the right at 0. Hence f must be continuous everywhere on .  T. Bilgin Connor [3], Ko lk [4], Maddox [5], Öztürk a nd Bi lgin [6], Pehliva n and Fi sher [7], Ruck le [8] and others used a modulus function to construct sequence spaces. Marouf presented definitions for asymptotically equivalent sequences and asymptotic regular matrices in [9]. Patterson extended these concepts by presenting an asymptotically statistical equivalent analog of these defi- nitions and natural regularity conditions for nonnegative summability matrices in [10]. Subsequently, many authors have shown their inter e st to solve differe nt p roblems arising in this area (see [11]-[13]). The concept of I-convergence was introduced by Kostyrko et al. in a metric space [14]. Later it was further studie d b y Dass e t al. [15], D e ms [16], Savas and Gumus [17], Kumar and Sharma [18], Ku mar and Mursaleen [19] and many others. Recently, Bilgin [20] used modulus function to define some notions of asymptotically equivalent sequences and studied some of their connections. Kumar and Sharma extended these concepts by presenting a non-trivial ideal I This paper presents introduce some new notions, (f, p)-asymptotically equivalent of multiple L, strong (f, p)- asymptoticall y equivalent of multiple L, and strong (f, p)-asympto tically lacunary equi valent of multip le L with respect to the ideal I which is a natural co mbinat ion of the d efinition for asymptoticall y equiva lent, a non -trivial ideal I, Lacunary sequence, a strictly positive sequence , and Modulus function. In addition to these definitions, we obtain some revelent connections between these notions. 2. Definitions and Notations Now we recall some definitions of sequence spaces (see [2] [4]-[6] [15], and [20]-[25]). Definition 2 .1. A sequence is statisticall y convergent to L if for every , (denoted by ), where the vertical bars denote the cardinality of the Enclosed set. Definition 2.2. A sequence is strongly(Cesaro) summable to L if , (denoted by ). Definition 2 .3. Let f be any modulus; the sequence is strongl y (Cesaro) summable to L with respect to a modulus i f , (denot ed by ). Definition 2.4. Two nonnegative sequences and are said to be asymptotically equivalent if , (denoted by ). Definition 2 .5. T wo nonne gat ive se q uence s and are said to be asymptotica lly statistic al e quiva le nt of multiple L provided that for every , 1 lim :0, k nk x kn L ny ε ≤−≥ = (denoted by ) a nd si mply asymptotically statistical equ ivalent, if . Definition 2 .6. T wo nonnegative sequences and are said to be strong asymptotically equivalent of multiple L provided that (denoted by ) and simply strong asymptotically equivalent, if . Definition 2.7. Let be a lacunary sequence; the two nonnegative sequences and are said to be asymptotically lacunary statistical equivalent of multiple L provided that for every , 1 lim :0, k rr rk x kI L hy ε ∈−≥ = (denoted by ) and simply asymptotically lacunary statistical equi- valent, if . Definition 2.8. Let be a lacunary sequence; the two nonnegative sequences and are said to be  T. Bilgin strong asymptotically lacunary equivalent of multiple L provided that (denoted by ) and simply strong asymptotically lacunary equivalent, if . Definition 2.9. Let f be any modulus; the two nonnegative sequences and are said to be f- asymptotically equivalent o f multiple L provided that, (denoted by ) and s imply s t ro ng f-asymptotically eq uiva lent, if . Definition 2.10. Let f b e an y modul us; the two nonne gative sequences and are said to be strong f- asymptotically equivalent of multiple L provided that, 1 1 lim 0 nk nkk x fL ny = −= ∑ (denoted by ) and simply strong f-asymptotic a lly equi valent , if . Definition 2.11. Let f be any modulus and be a lacunary sequence; the two nonnegative sequences and are said to be strong f-asymptotically lacunary equivalent of multiple L provided that 1 lim 0 r k rkI rk x fL hy ∈ −= ∑ (denoted by ) and simply strong f-asymptotically lacunary equivalent, if . For any non-empty set X, let denote the power set of X. Definition 2 .12. A family is said to be an ideal in X if 1) ; 2) imply and 3) imply . Definition 2 .13. A non-empty family is said to be a filter in X if 1) ; 2) imply and 3) imply . An ideal I is said to be non-trivial if and . A non-trivial ideal I is called admissible if it contains all the singleton sets. Moreover, if I is a non-trivial ideal on X, then is a filter on X and conversely. The filter is called the filter a ssociated with the ideal I. Definition 2 .14. Let be a non-trivial ideal in N and be a metric space. A sequence in X is said to b e I-co n ver ge nt to if for each , the set . In this case, we write Definition 2.15. A sequence of numbers is said to be I-statistical co nvergent or S(I)-conver gent to L, if for every and , we have { } 1 ;: . k n NknxLI n εδ ∈≤−≥≥ ∈ In this case, we write or Definition 2.16 Let be a non-trivial ideal in N. The two non-negative sequences and are said to be strongly asymptotically equivalent of multiple L with respect to the ideal I provided that for each 1 1 ;. nk kk x nNLI ny ε = ∈−≥ ∈ ∑ denoted by and simply strongly asymptotically equivalent with respect to the ide a l I, if . Definition 2.17. Let be a non-trivial ideal in N and be a lacunary sequence. The two nonne gative se quence s and are said to be asymptoticall y lacunary statistical equi valent of multiple L with respect to the id e a l I p r o vided that for each  T. Bilgin and , 1 ;: k r rk x rN kILI hy εγ ∈∈−≥≥ ∈ denoted by and simply asymptotically lacunary statistical equivalent with respect to the ideal I, if . Definition 2.18. Let be a non-trivial ideal in N and be a lacunary sequence. The two non-negative seque nces and are said to be strongly asymptotically lacunary equivalent of multiple L with respect to the id e a l I provided that for 1 ; r k kI rk x rNL I hy ε ∈ ∈−≥ ∈ ∑ denoted by and simply strongly asymptotically lacunary equivalent with respect to the ideal I, if . Definition 2.19. Let be a non-trivial ideal in N and f be a modulus function. The two non- negative sequences and are said to be f-asymptotically equivalent ofmultiple L with respect to the ideal I provided that for each ;k k x k NfLI y ε ∈− ≥∈ denoted by and simply f-asymptotically eq uivalent with resp e ct to the ideal I, i f . Definition 2.20. Let be a non-trivial ideal in N and f be a modulus function. The two non- negative sequences and are said to be strongly f-asymptotically equivalent of multiple L with respect to the ideal I provided that for each , 1 1 ;nk kk x nN fLI ny ε = ∈−≥∈ ∑ denoted by and simply strongly f-asymptotically equivale nt with respect to the ide a l I, if . Definition 2.21. Let be a non-trivial ideal in N, f be a modulus function and be a lacunary sequence. The two non-negative sequences and are said to be strongly f-asymptotically lacunary e quiva l ent of mult iple L with respect to the ideal I provided that for each , 1 ; r k kI rk x rN fLI hy ε ∈ ∈− ≥∈ ∑ denoted by and simply strongly f-asymptotically lacunary equivalent with respect to the ideal I, if . 3. Main Results We now con s ider our main results. We begin with the following definitions. Definition 3.1. Let be a non-trivial ideal in N, f be a modulus function, and be a sequence of positive real numbers. Two number sequences and are said to be strongly (f, p)- asymptotically equivalent o f multiple L with respect to the ideal I provided that for each , 1 1 ; k p nk kk x nN fLI ny ε = ∈− ≥∈ ∑  T. Bilgin denoted by and simply strongly (f, p)-asymptotically equi valent with respect to the ideal I, if . If we take for , we write instead of and simply strongly p-asympto- tically equivalent with respect to the ideal I, if . If we take for all , we write instead of Definition 3 .2. Let be a non-trivial ideal in N, f be a modulus function, be a lacunary sequence, and be a sequence of positive real numbers. Two number sequences and are said to be strongly (f, p)-asymptotically lacunary equivalent of multiple L with respect to the ideal I provided that for each , 1 ; k r p k kI rk x rNf LI hy ε ∈ ∈− ≥∈ ∑ denoted by and simply strongly (f, p)-asymptotically lacunary equivalent with respect to the ideal I, if . If we take for all , we write instead of Note that,we put , we write instead of . Hence is the same as the of Kumar and Sharma [15]. Also if we put for , we write instead of . Hence is the same as the of Savas and Gumus [25] We start this section with the following theorem to show that the relation between (f, p)-asymptotically equivalence and strong p-asymptotically equivalence with respect to the ideal I Theorem 3.1. Let be a non-trivial ideal in N, f be a modulus function, be a lacunary sequence and 0 infsup kk kkk h pppH< =≤≤=<∞ , then 1) if then , and 2) if , then ( ) ( ) () , . fp p Iw Iw x yxy ∼⇔ ∼ Proof. Part 1): Let and ε > 0. We choose such that for every u with . We can write 1 12 1 11 k kk p nk kk pp kk kk x fL ny xx fL fL ny ny = − =−+ − ∑ ∑∑ where the first summation is over and the second summation over By definition of f, we have { } ( ) ( ) { } 1 11 11 max,max 1, 21 kk pp nn H hH kk kk kk xx fLf L n yny εε δ − = = −≤ +− ∑∑  T. Bilgin Thus, { } ( ) ( ) { } 1 11 max , 11 ;; . max1,21 kk pphH nn kk H kk kk xx nN fLnNL n ynyf ε εε ε δ − = = − ∈−≥⊆∈−≥ ∑∑ Since , it follows the later set, and hence, the first set in above expression belongs to I. This prove s that Part 2): If , then for all . Let , clearly { } 1 1 =1 1 11 min , k kk p nk kk pp nn hH kk kk kk x fL ny xx LL n yny β ββ = = − ≥ −≥− ∑ ∑∑ it follows that for each , we have { } 11 11 ;;min , k k p p nn hH kk kk kk xx nNLnN fL nyn y εε ββ = = ∈− ≥⊆∈−≥ ∑∑ Since , it follows that the later set belong s to I, and therefore, the theorem is proved. Theorem 3.2. Let be a non-trivial ideal in N, f be a modulus function, be a lacunary sequence and 0 infsup kk kkk h pppH< =≤≤=<∞ , then 1) if then , and 2) if , then () ( ) , . fp p IN IN x yxy θ θ ∼⇔ ∼ Proof. The proof of Theorem 3.2 is very similar to the Theorem 3.1. Then, we omit it. The next theorem shows the relationship between the strong (f, p)-asymptotically equivalence and the strong (f, p)-asymptotically lacunary equivalence with respect to the ideal I. Theorem 3.3. Let be a non-trivial ideal in N, f be a modulus function, be a lacunary sequence and be a sequence of positive real numbers. Then 1) if then implies ; 2) if then implies ; 3) if 1 liminflimsup rr rr qq< ≤<∞ , then ( ) () ( ) , ,fp fp IN Iw x yxy θ ∼⇔∼ . Proof. Part (i): If then there exi st s such that for every r. Now suppose that and . Let 1 ; k r p k kI rk x A rNfL hy ε ∈ =∈ −< ∑ 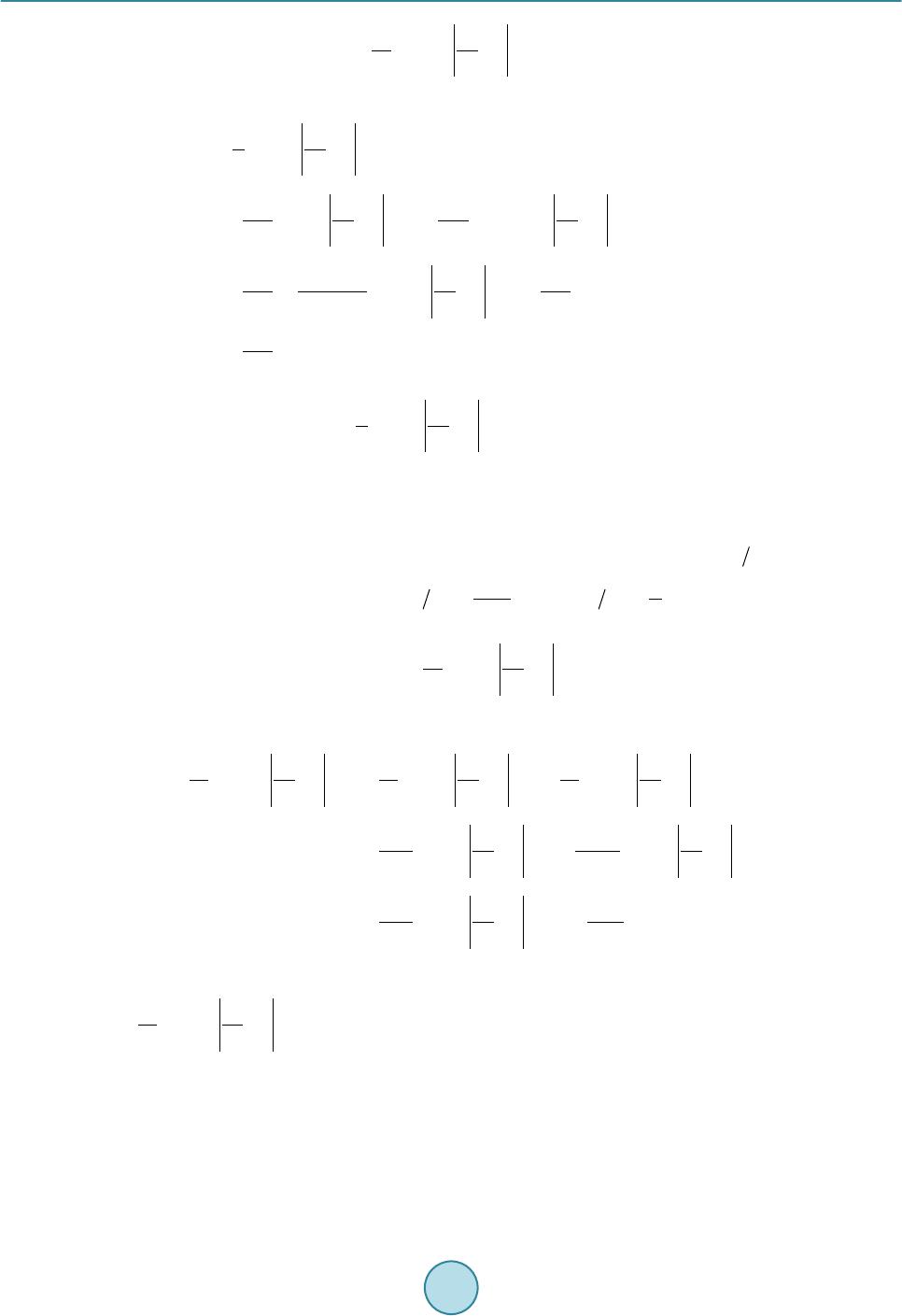 T. Bilgin Hence, for all we have 1. k j p k jkI jk x H fL hy ε ∈ = −< ∑ Let n be any integer with . Now write ( ) 1 11 11 11 11 11 1 1 11 11 sup sup k kk r m k m p nk kk pp kr kk km kI rk rk p rr mm kmmj jA m kIm rmk r r jA r x fL ny xx fL fL ky ky kkx f LkkH khy k kH k = ==∈ −− −−∈ =∈= −− ∈ − − ≤ −=− − =−= − = ∑ ∑ ∑∑ ∑∑ ∑ | sup jr j jA q HK εε ∈ = <= it follows that for any , ( ) | 1 1 ; k p nk kk x n NfLFI ny ε = ∈− <∈ ∑ which yields that . Because for any set , . Part (ii): Let and . There exists such that for all . We have, for sufficientl y lar ge r, that and . Let and define the set 1 1 ;. k r p kk rk rk x AkNf L ky ε = =∈ −< ∑ We have , which is the f ilte r of the ideal I, For each , we have 1 1 11 1 11 1 1 1 11 k kk rr r kk rr r ppp kk kkk kI kk r krkrk pp kk kk rr kk rr krrk k r k rr xxx fLfL fL h yhyhy xx kk fL fL khyhky k kh − − ∈== − = = − = −= −− − = −−− ≤ ∑ ∑∑ ∑∑ ∑ | 1 k p k k x fL y δεε δ + −<= it follows that for any , ( ) | 1 ; k r p k kI rk x r NfLFI hy ε ∈ ∈− <∈ ∑ which yiel ds that Part (iii): This immediatel y follows from (i) and (ii). Now we give relation between asymptotically statistical equivalence and strong (f, p) -asymptotically equi- valence with respect to the ideal I. Also we give relation between asymptotically lacunary statistical equivalence and strong (f, p)-asymptotically lacunary equivalence with respect to the ideal I. Theorem 3.4. Let be a non-trivial ideal in , be a modulus function, be a lacunary se quence and 0 infsup kk kkk h pppH< =≤≤=<∞ , then 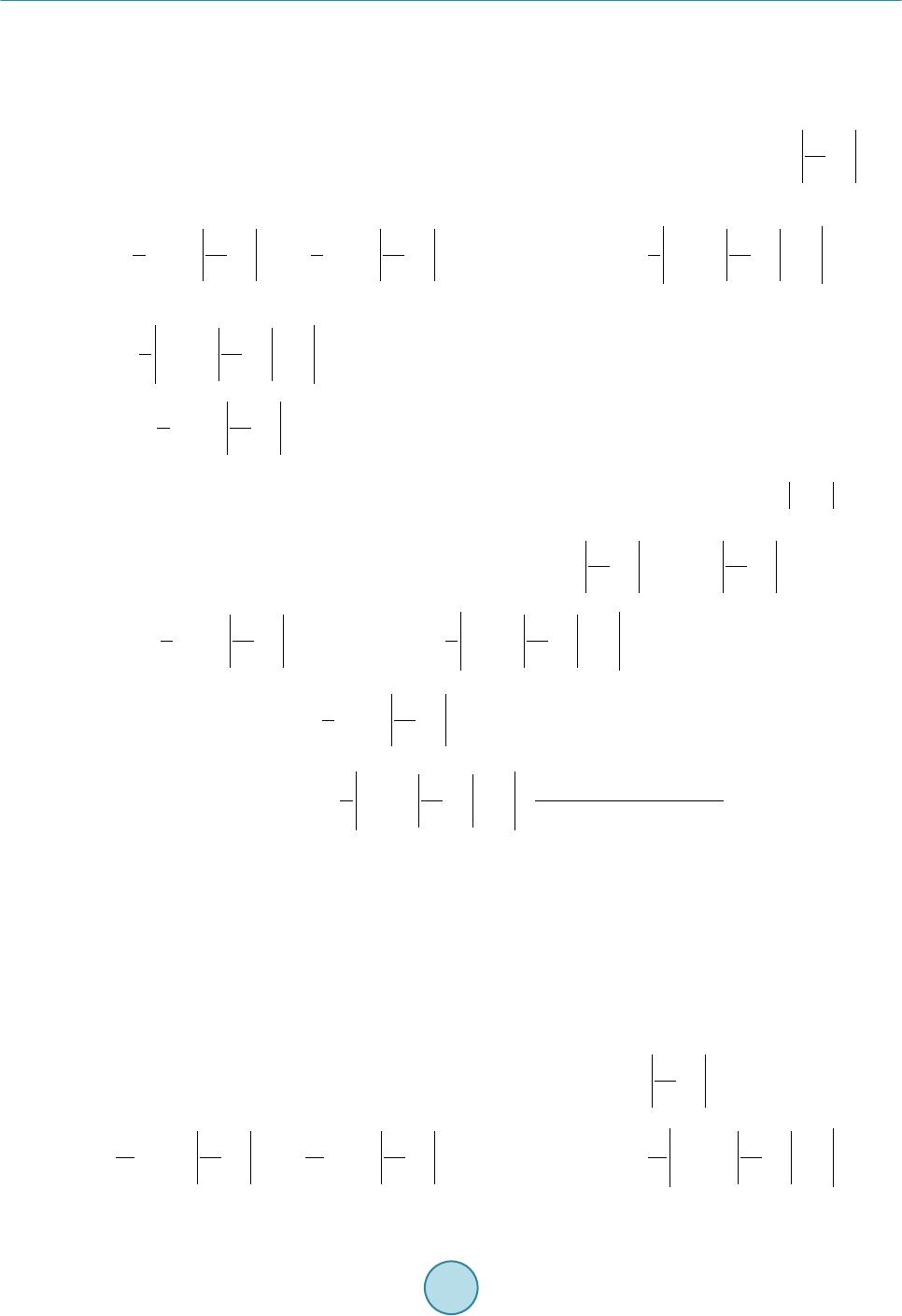 T. Bilgin 1) if then , 2) if f is bounded then . Proof. Part 1): Suppose , and let and denote the sum over with then we can write ( )( ) { } 11 11 1 min ,: kk pp nhH kk k kkkk xx x fLfLff knL nynyn y εε ε = −≥ −≥≤−≥ ∑∑ Consequently, for any , we have ( )( ) { } 1 1 ;: 1 ;min ,. k k k p nhH k kk x nN knL ny x nNfLf fI ny εγ γ εε = ∈≤−≥ ≥ ⊆∈− ≥∈ ∑ Therefore we have 2) Suppose f is bounded and . Since f is bo unded, there exists an integer T such t hat for all . Moreover, for , We split the s um for into sums over and . Then { } ( )( ) { } , 1 11 max ,:max, k p nhH hH kk kkk xx fLTTknLf f nyn y ε εε = −≤≤−≥ + ∑ Consequen tly, we have ( )( ) { } { } 1 , 1 ; max , 1 ;: . max , k p nk kk hH k hH k x nN fL ny ff x nN knLI ny TT ε ε εε ε = ∈ −≥ − ⊆ ∈≤−≥≥∈ ∑ Therefore we have Theorem 3.5. Let be a non-trivial ideal in N, f be a modulus function, be a lacunary sequence and 0 infsup kk kkk h pppH< =≤≤=<∞ , then 1) if then , 2) if f is bounded then ( ) ( ) ,fp IN IS xyx y θθ ∼ ⇔∼ . Proof. Part 1): Take and let denote the sum over with . Then ( )( ) { } 1 11 1 min ,:, kk r pp hH kk k r kI r krkrk xx x fLfLffkI L h yhyhy εε ε ∈ −≥ −≥∈−≥ ∑∑ 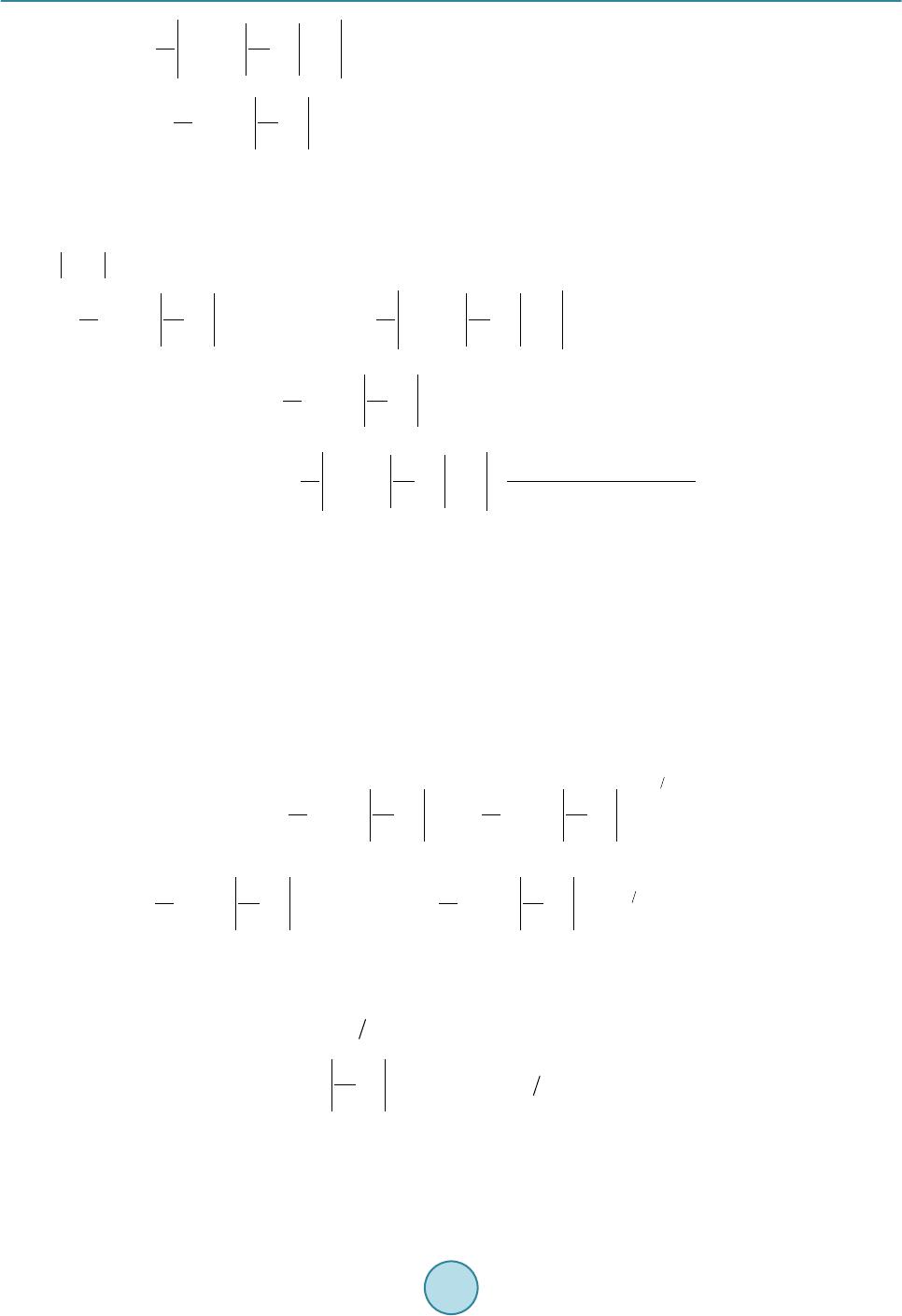 T. Bilgin and ( )( ) { } 1 ;: 1 ;min ,. k r k r rk p hH k kI rk x rN kIL hy x rNfLf fI hy εγ γ εε ∈ ∈∈−≥ ≥ ⊆∈−≥∈ ∑ But then, by definition of an ideal, later set belongs to I, and therefore Part 2): Suppose that f is bounded and . Since f is bounded, there exists an integer T such that for all . We see that { } ( )( ) { } , 11 max ,:max,, k r p hH hH kk r kI rkr k xx fLTTkILff hyh y ε εε ∈ −≤∈−≥ + ∑ so we have ( )() {} {} , 1 ; max , 1 ;: max , k r p k kI rk hH k rhH rk x rNfL hy ff x rN kILI hy TT ε ε εε ε ∈ ∈ −≥ − ⊆ ∈∈−≥≥∈ ∑ Therefore we have Let for all k, for all k and . Then it follows following T heorem. Theorem 3.6. Let be a non-trivial ideal in N, f be a modulus function, and be a lacunary sequence, then implies , Proof. Let It follo ws from Holder’s inequality 11 rr pt pt kk kI kI rk rk xx fL fL hy hy ∈∈ −≤ − ∑∑ and 11 ;; rr pt tp kk kI kI rk rk xx rNf LrNf LI hyhy εε ∈∈ ∈−≥⊆∈−≥∈ ∑∑ . Thus we have We now consider that and are not constant sequences. Theorem 3.7. Let be a non-trivial ideal in N, f be a modulus function, be a lacunary sequence, for all k and be bounde d, then implies Proof. Let . k t k kk x zfL y = − and , so that : We define the sequences and as follows: For ; let and and for ; let and . T hen we have ; Now it follows that and . There- fore  T. Bilgin ( ) 1 111 k kk r rrr kkkk k kIkIkIkI r rrr zuvz v h hhh λ λλλ ∈ ∈∈∈ =+≤+ ∑ ∑∑∑ Now for each r; 1 1 11 11 1 11111 rrr rr kk kk kI kIkIkIkI r rrrrr vv vv h hhhhh λλ λλ λ λλ λλ λ − − −− ∈∈∈ ∈∈ =≤< ∑∑∑ ∑∑ and so 1 111 11 , 1,1 11 1 ,12, 1 k k r rrr rr rr r p kk kk kIkI kI kI rk rrr kkkk kI kI rr kk kkk kI kIkI rrr x fLzzv hy hhh zzzz hh zzzzz hh h λ λ λλ ∈ ∈∈∈ ∈∈ ∈∈ ∈ −=≤ + ≥≥ = ≤ +< < ∑ ∑∑∑ ∑∑ ∑∑ ∑ If 1 k r p k kI rk x fL hy ε ∈ −≥ ∑ then 1 1,1 1, <1 2 k r k r t kk kI rk t kk kI rk x fL z hy x fL z hy λ ε ε ∈ ∈ −≥ ≥ −≥ ∑ ∑ Hence 1 11 ;;min ,. 2 kk rr pt kk kI kI rk rk xx rNf LrNf LI hy hy λ ε εε ∈∈ ∈−≥⊆∈−≥∈ ∑∑ Thus we ha ve Acknowledgements The wor k is s uppo rte d b y the P res ide ncy o f scientific Research P rojects of Y uz unc u Yil Univ ers ity ( No. KONGRE- 2014/94) and is presented at 3rd International Eurasian Conference on Mathematical Sciences and Applications (IECMSA-2014). References [1] Freedman, A.R, Sember, J.J. and Raphel, M. (1978) Some Cesaro-Type Summability Spaces. Proceedings London Mathematical Society, 37, 508-520. http://dx.doi.org/10.1112/plms/s3-37.3.508 [2] Nakano, H. (1953) Concave Modulars. Journal of the Mathematical Society of Japan, 5, 29-49. http://dx.doi.org/10.2969/jmsj/00510029 [3] Connor, J.S. (1989) On Strong Matrix Summability with Respect to a Modulus and St atistical Con vergence. Canadian Mathematical Bulletin, 32, 194-198. http://dx.doi.org/10.4153/CMB-1989-029-3 [4] Kolk, E. (1993) On Strong Boundedness and Summability with Respect to a Sequence Moduli. Tartu Ülikooli Toime- tised, 960, 41-50. [5] Maddox, I.J. (1986) Sequence Spaces Defin ed b y a Modulus. Mathematical Proceedings of the Cambridge Philosoph- ical Society, 100, 161-166. http://dx.doi.org/10.1017/S0305004100065968 [6] Öztürk, E. and Bilgin, T. (1994) Strongly Summable S equence S paces Defined b y a Modulus. Indian Journal of Pure and Applied Mathematics, 25, 621-625.  T. Bilgin [7] Pehlivan, S. and Fisher, B. (1994) On Some Sequence Spaces. Indian Journal of Pure and Applied Mathematics, 25, 1067-1071. [8] Ruckle, W.H (1973) FK Spaces in Which the Sequence of Coordinate Vectors Is Bounded. Canadian Journal of Ma- thematics, 25, 973-978. http://dx.doi.org/10.4153/CJM-1973-102-9 [9] Marouf, M. (1993) Asymptotic Equivalence and Summability. International Journal of Mathematics and Mathemati- cal Sciences, 16, 755-762. http://dx.doi.org/10.1155/S0161171293000948 [10] Patterson, R. F. (2003) On Asymptotically Statistically Equivalent Sequences. Demonstratio Mathematica, 36, 149-153. [11] Metin, B. and Selma, A. (2008) On δ-Lacunary Statistical As ymptotically Equivalent Sequences. Filomat, 22, 161-172. http://dx.doi.org/10.2298/FIL0801161B [12] Basarir, M. and Altundag, S. (2011) On Asymptotically Equivalent Difference Sequences with Respect to a Modulus Function. Ricerche di Matematica, 60, 299-311. http://dx.doi.org/10.1007/s11587-011-0106-0 [13] Patterson, R.F . and Savas, E. (2006) On Asymptotically Lacunary St atistically Equi valent Sequences. T hai Journ al of Mathematics, 4, 267-272. [14] Kostyrko, P., Salat, T. an d Wilczynski, W. (2001) I-Convergence. Real Analysis Exchange, 26, 669-686. [15] Das, P., Savas, E. and Ghosal, S. (2011) On Generalizations of Certain Summability Methods Using Ideals. Applied Mathematics Letters, 24, 1509-1514. http://dx.doi.org/10.1016/j.aml.2011.03.036 [16] Dems, K. (2004) On I-Cauch y Seq uences. Real Analysis Exchange, 30, 123-128. [17] Savas, E. and Gumus, H. (2013) A Generalization on Ι -Asymptotically Lacunary Statistical Equivalent Sequences. Journal of Inequalities and Applications, 2013, 270. [18] Ku mar, V. and Sharma, A. (2012) Asymptotically Lacunary Equivalent Sequences Defined by Ideals and Modulus Function. Mathematical Sciences, 6, 1-5. [19] Kumar, V. and Mursaleen, M. (2003) On Ideal Analogue of Asymptotically Lacunary Statistical Equivalence of Se- quences. Acta Universitatis Ap ule nsis, 36, 109-119. [20] Bilgin, T. (2011) f-Asymptoti cally Lacunar y Eq uivalent Sequen ces. Acta Universitatis Apulensis, 28, 271-278. [21] Connor, J.S. (1988) The Statistical and Strong p-Cesaro Convergence of Sequences. Analysis, 8, 47-63. http://dx.doi.org/10.1524/anly.1988.8.12.47 [22] Fast, H. (1951) Sur la convergence statistique. Colloquium Mathematicae, 2, 241-244. [23] Fridy, J.A. (1985) On Statistical Convergence. Analysis, 5, 301-313. http://dx.doi.org/10.1524/anly.1985.5.4.301 [24] Fridy, J.A. and Orhan, C. (1993) Lacunary Statistical Convergen t. Pacific Journal of Mathematics, 160, 43-51. http://dx.doi.org/10.2140/pjm.1993.160.43 [25] Fridy, J.A. and Orhan, C. (1993) Lacunary Statistical Summability. Journal of Mathematical Analysis and Applications, 173, 497-504. http://dx.doi.org/10.1006/jmaa.1993.1082
|