Applied Mathematics
Vol. 3 No. 11 (2012) , Article ID: 24378 , 5 pages DOI:10.4236/am.2012.311223
An Application of Linear Automata to Near Rings
School of Mathematics and Computer Science, Hubei University, Wuhan, China
Email: yousongfa@163.com
Received August 31, 2012; revised September 30, 2012; accepted October 7, 2012
Keywords: Linear Automata; Accessible; GSA-Homomorphism; Near-Ring
ABSTRACT
In this paper, we have established an intimate connection between near-nings and linear automata, and obtain the following results: 1) For a near-ring N there exists a linear GSA S with 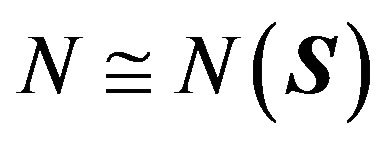 iff (a)
iff (a)  is abelian, (b) N has an identity 1, (c) There is some
is abelian, (b) N has an identity 1, (c) There is some 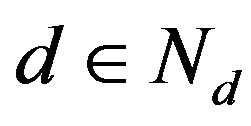 such that
such that  is generated by {1,d}; 2) Let
is generated by {1,d}; 2) Let 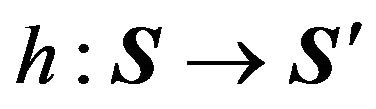 be a GSAepimorphism. Then there exists a near-ring epimorphism
be a GSAepimorphism. Then there exists a near-ring epimorphism 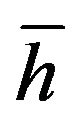 from
from 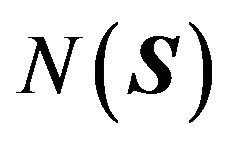 to
to 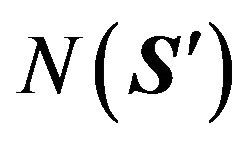 with
with 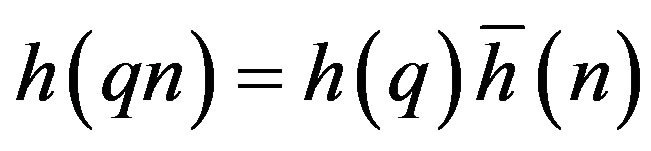 for all
for all 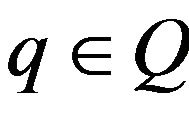 and
and ; 3) Let
; 3) Let  be a GA. Then (a)
be a GA. Then (a) 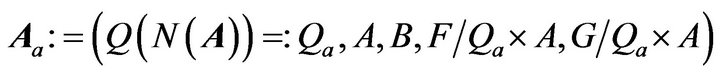 is accessible, (b) Q = 0N(A), (c)
is accessible, (b) Q = 0N(A), (c)  with
with 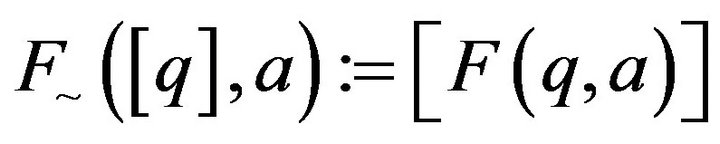 and
and  is reduced, (d)
is reduced, (d) 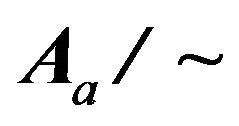 is minimal.
is minimal.
1. Introduction
Automata consist of inputs, states, and outputs, together with maps which describe how new inputs affect the state and the output. A semi-automation is a triple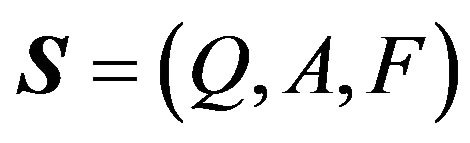 , where Q and A are sets, called the state set and input set, and F is a function from
, where Q and A are sets, called the state set and input set, and F is a function from 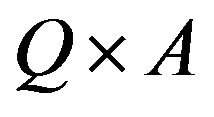 in Q, called the state-transition function. If Q is a group, we call
in Q, called the state-transition function. If Q is a group, we call 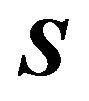 a group-semiautomaton and abbreviate this by GSA. Automata consist of inputs, states, and outputs, together with maps which describe how new inputs affect the state and the output. A semiautomaton is a triple
a group-semiautomaton and abbreviate this by GSA. Automata consist of inputs, states, and outputs, together with maps which describe how new inputs affect the state and the output. A semiautomaton is a triple , where Q and A are sets, called the state set and the input set, and F is a function from
, where Q and A are sets, called the state set and the input set, and F is a function from 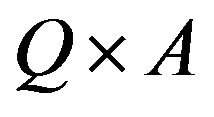 in Q, called the state-transition function. If Q is a group (we always write it additively), we call
in Q, called the state-transition function. If Q is a group (we always write it additively), we call 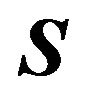 a group-semiautomaton and abbreviate this by GSA. For
a group-semiautomaton and abbreviate this by GSA. For 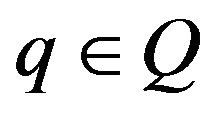 and
and 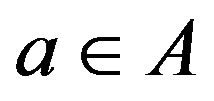 we interprete
we interprete 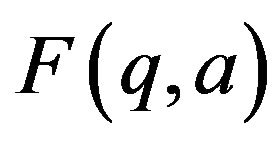 as the new state obtained from the old state q by mean of the input a [1].
as the new state obtained from the old state q by mean of the input a [1].
If 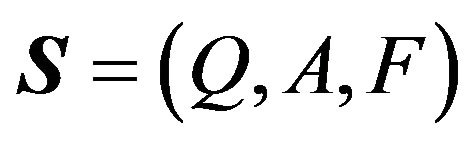 is a semiautomaton, we get a collection of mappings
is a semiautomaton, we get a collection of mappings 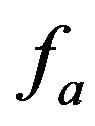 from Q to Q, one for each
from Q to Q, one for each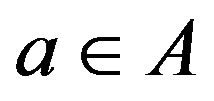 , which are given by
, which are given by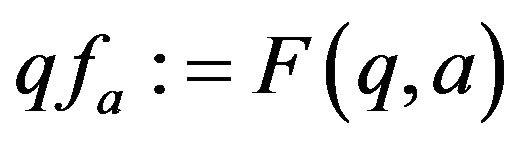 . Hence
. Hence 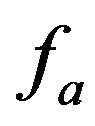 describes the effect of the input a on the state set Q of
describes the effect of the input a on the state set Q of .
.
If the input 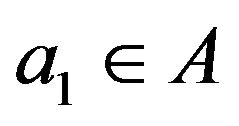 is followed by the input
is followed by the input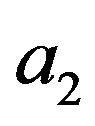 , the semiautomaton moves from the state
, the semiautomaton moves from the state 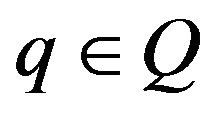 first into
first into 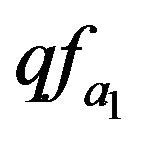 and then into
and then into . We extend (as usual) A to the free monoid
. We extend (as usual) A to the free monoid 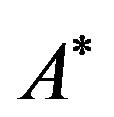 over A consisting of all finite sequences of elements of A, including the empty sequence
over A consisting of all finite sequences of elements of A, including the empty sequence , and get
, and get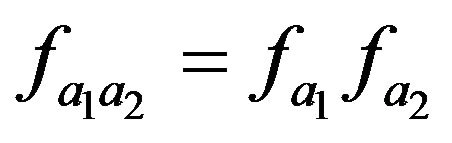 , i.e. the map
, i.e. the map 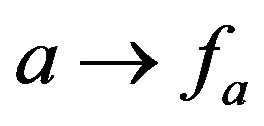 is a monomorphism from
is a monomorphism from 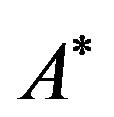 into the transformation monoid over Q with
into the transformation monoid over Q with . In the case of
. In the case of , we are also able to study the superposition
, we are also able to study the superposition  (defined pointwisely) of two simultaneous inputs
(defined pointwisely) of two simultaneous inputs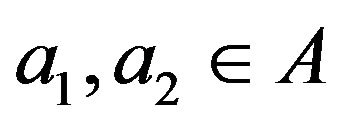 . Hence it is natural to consider
. Hence it is natural to consider 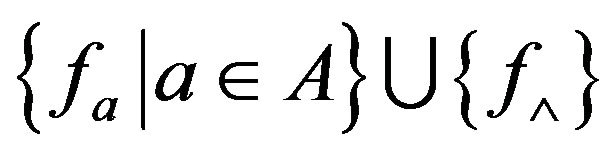 and all of its sums and products (composition of maps). The obvious framework for that is, of course, the structure of a near ring.
and all of its sums and products (composition of maps). The obvious framework for that is, of course, the structure of a near ring.
Let  be a
be a , The subnear-ring
, The subnear-ring  of
of  generated by
generated by  and all
and all 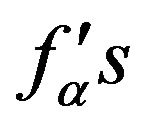 is called the syntactic near-ring of
is called the syntactic near-ring of . Thus
. Thus 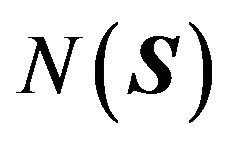 is always a near-ring with identity. If Q is finite, then
is always a near-ring with identity. If Q is finite, then  is finite, too [2].
is finite, too [2].
2. Discussion
1) The homomorphism case. Let Q and A be additive groups with zero 0 and F a homomorphism from the direct product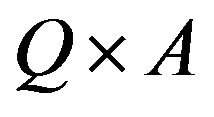 . We then call
. We then call 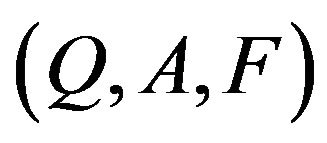 a homomorphic
a homomorphic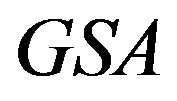 . Because of
. Because of 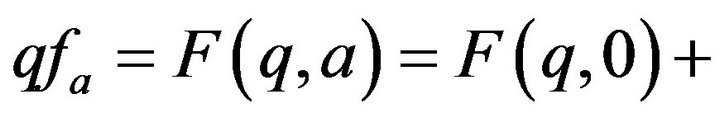
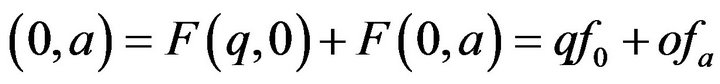 , we get
, we get 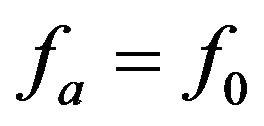
 , where
, where  is a homomorphism (i.e. a distributive element in N(Q)), while
is a homomorphism (i.e. a distributive element in N(Q)), while 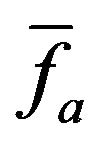 is the map with constant value
is the map with constant value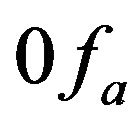 . If no input can change the zero state, i.e. if
. If no input can change the zero state, i.e. if  for all
for all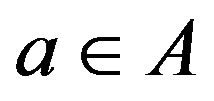 , then
, then 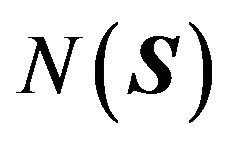 obviously is a distributively generated near-ring, consisting of
obviously is a distributively generated near-ring, consisting of 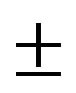 -sums of powers of
-sums of powers of 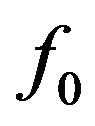 which are endomorphisms, we also get a distributively generated near-ring if F is additive in the first component. For homomorphic
which are endomorphisms, we also get a distributively generated near-ring if F is additive in the first component. For homomorphic  one sees by induction that
one sees by induction that  , where the map in brackets is constant. Each power
, where the map in brackets is constant. Each power 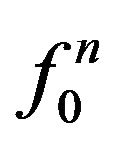 is a homomorphism [3].
is a homomorphism [3].
2) The linear case is a special case of the homomorphism case in which Q and A are Abelian groups (or more generally, R-modules for some ring R) and where F is linear. Let Q and A be free R-modules with finite base X, Y respectively. Let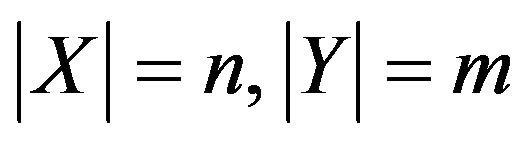 . Then the action of F can be described by an
. Then the action of F can be described by an 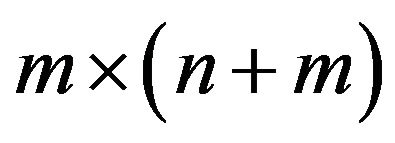 -matrix
-matrix  over R if we replace each element of Q and of A by its decomposition
over R if we replace each element of Q and of A by its decomposition  induces a decomposition of Z such that
induces a decomposition of Z such that
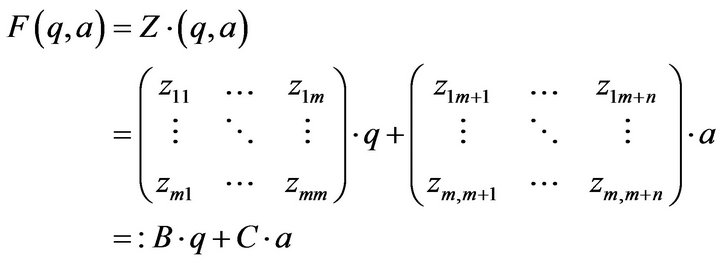
We then get
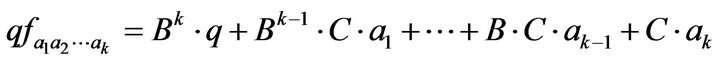 . If, in particular,
. If, in particular, 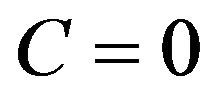 , we get
, we get 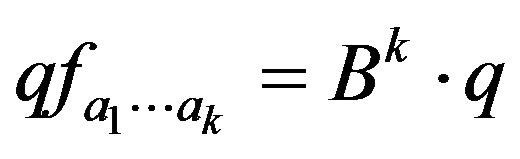 and
and 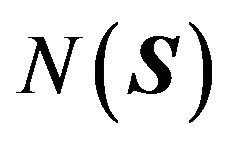 is a ring, generated by B and the unit matrix I [4]. on the other hand, if
is a ring, generated by B and the unit matrix I [4]. on the other hand, if , then
, then . We get
. We get 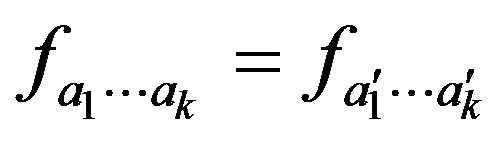 iff
iff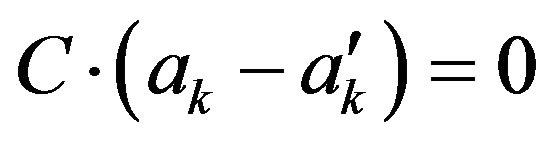 .
.
Anyhow, each fa (and hence each fa for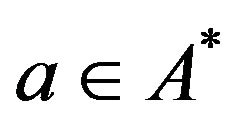 ) is an affine map from Q to Q. If Q is free on X with
) is an affine map from Q to Q. If Q is free on X with 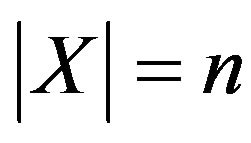 then we can extend the idea of matrix representations from linear maps to affine maps. Let f be an affine map. Then f decomposes as
then we can extend the idea of matrix representations from linear maps to affine maps. Let f be an affine map. Then f decomposes as  where f0 is a homomorphism and c is constant. Let F be the matrix for f0 with respect to X. Invent a symbol e with
where f0 is a homomorphism and c is constant. Let F be the matrix for f0 with respect to X. Invent a symbol e with 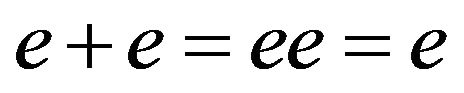 and
and 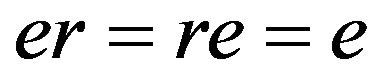 for all
for all . Then
. Then

Establishes an isomorphism between Maff(Q) (all affine of Q) and a subnear-ring of all 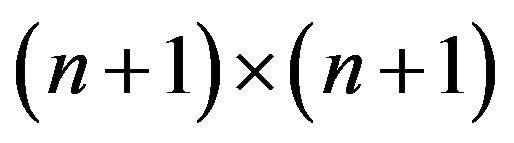 matrices over
matrices over 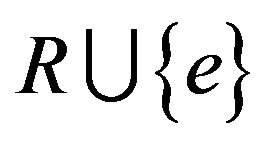 [3].
[3].
3. Main Results
Theorem 1. Let  be a homomorphic GSA, Then
be a homomorphic GSA, Then 
Proof. 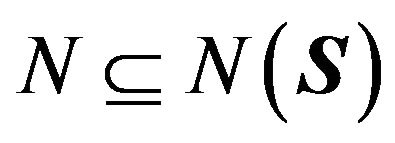 is clear. Conversely it suffices to show that N is a near-ring, since obviously N contains all
is clear. Conversely it suffices to show that N is a near-ring, since obviously N contains all  and
and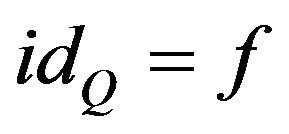 . In fact, we show that N is a subnear-ring of M(Q)
. In fact, we show that N is a subnear-ring of M(Q)
Take 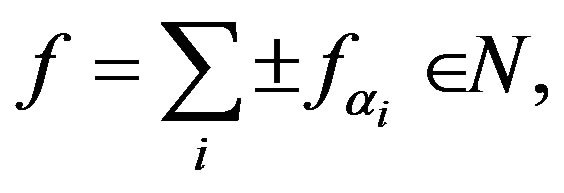
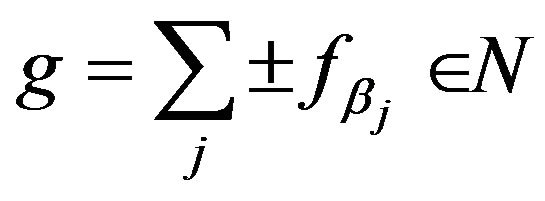 . It is clear that
. It is clear that . So consider
. So consider
 .
.
Hence we only look at the last expression in (a), let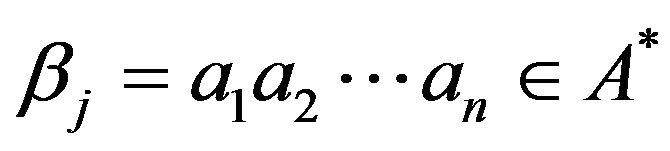 . Then
. Then
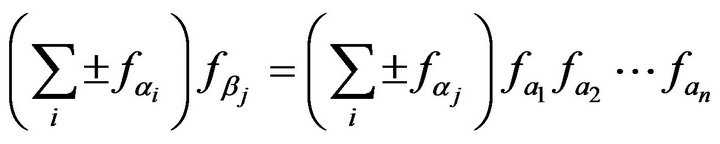
We first focus our attention to 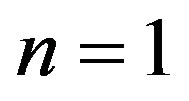 and put
and put 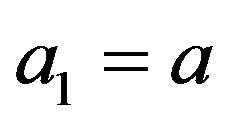 for a moment
for a moment
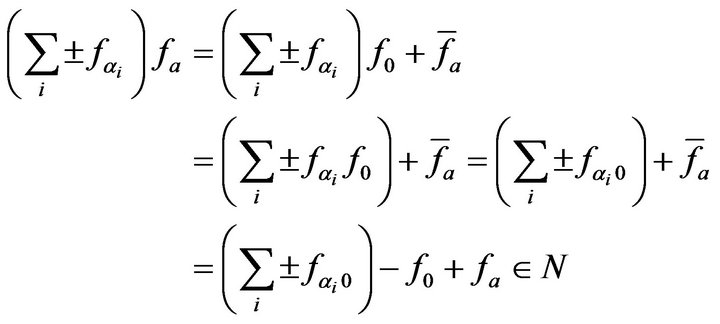
Therefore we get  with
with
 .
.
By induction, this is in N Let 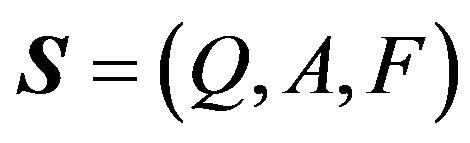 be homomorphic. The zero-symmetric part
be homomorphic. The zero-symmetric part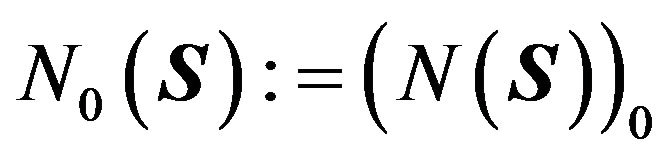 , and
, and  consists of all finite sums of elements of the form
consists of all finite sums of elements of the form  with
with  and
and .
.
In fact, all elements 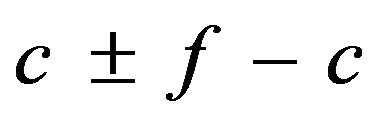 are in
are in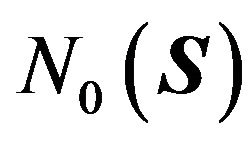 . Conversely, take
. Conversely, take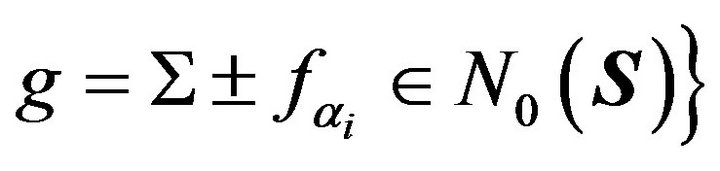 . Then
. Then
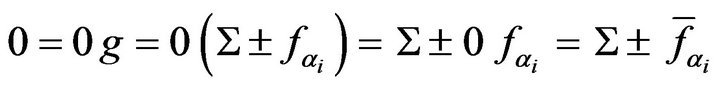 . By standard group theory, we can arrange
. By standard group theory, we can arrange
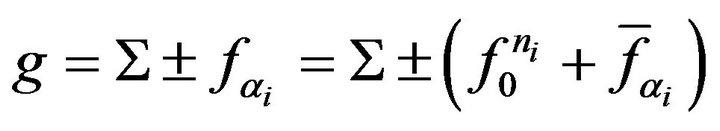 into sums and differences of elements of the form
into sums and differences of elements of the form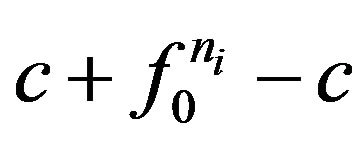 , where c is the sum of some
, where c is the sum of some  [5]. If
[5]. If 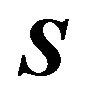 be linear. Then (with
be linear. Then (with )
)
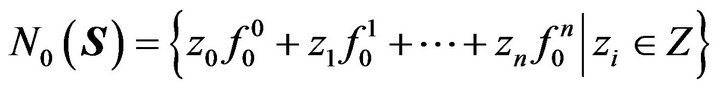 (n is non negative integer ), Hence
(n is non negative integer ), Hence 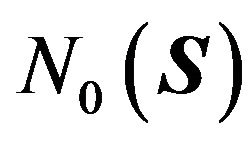 is the subnear-ring of
is the subnear-ring of  generated by
generated by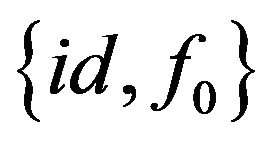 . Since
. Since 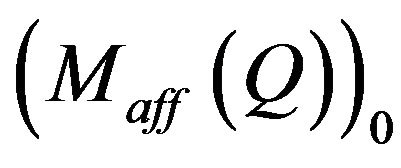 is a ring,
is a ring,  is a ring, too [6].
is a ring, too [6].
We can find a group Q such that N is isomorphic to a subnear-ring 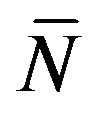 of
of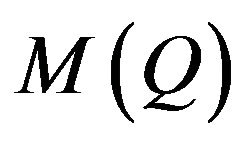 . Let A be an index set for
. Let A be an index set for 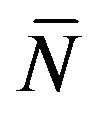 , i.e.
, i.e. . Let
. Let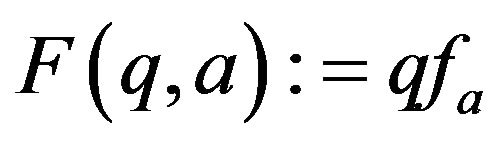 . Then
. Then 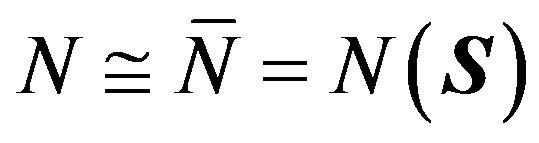 with
with . Since every nearring can be embedded in a near-ring with identity, we get every near-ring can be embedded in the near-ring of some GSA [7]
. Since every nearring can be embedded in a near-ring with identity, we get every near-ring can be embedded in the near-ring of some GSA [7]
Theorem 2. For a near-ring N there exists a linear GSA  with
with  iff (a)
iff (a) 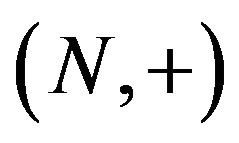 is Abelian, (b) N has an identity 1, (c) There is some
is Abelian, (b) N has an identity 1, (c) There is some 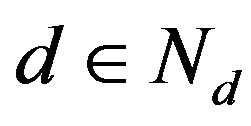 such that
such that 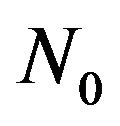 is generated by
is generated by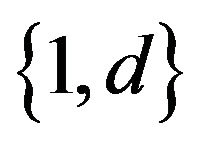 .
.
Proof. Let N be a near-ring with (a)-(c), we know that N is isomorphic to a subnear-ring 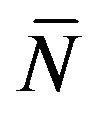 of
of 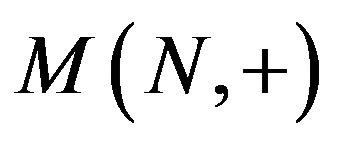 [2]. Let
[2]. Let 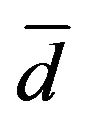 and
and 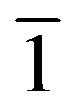 be the images of d and 1 in
be the images of d and 1 in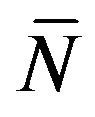 . Since d is distributive,
. Since d is distributive,  is an endomorphism of
is an endomorphism of  and
and 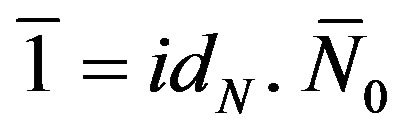 is generated by id and
is generated by id and , whence
, whence
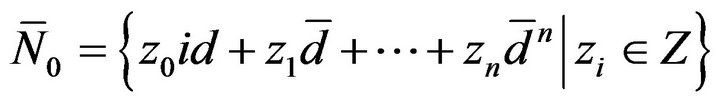 (n is non negative integer). Now let
(n is non negative integer). Now let  and
and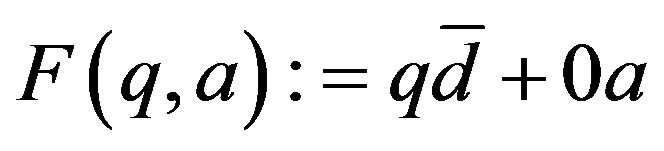 . Then
. Then 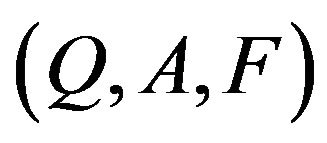 is a linear GSA, Since
is a linear GSA, Since 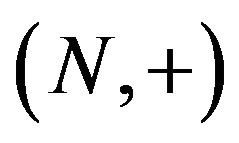 is abelian. Since
is abelian. Since 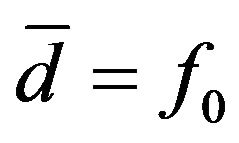 we get
we get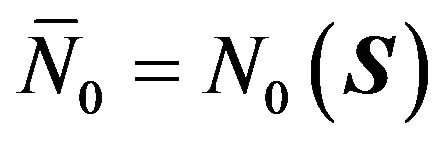 . Furthermore, take
. Furthermore, take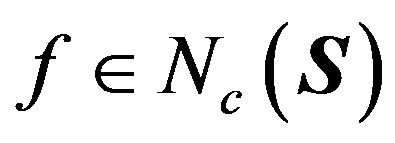 . We get
. We get
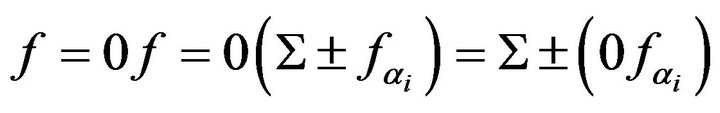 with
with
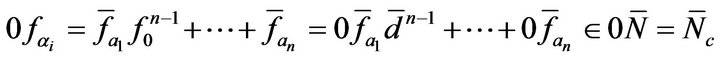 .
.
This shows . Conversely, every
. Conversely, every 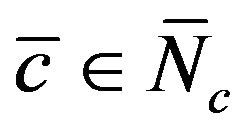 (with constant value c) is in
(with constant value c) is in 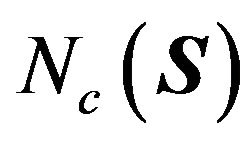 since
since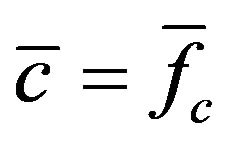 . Hence
. Hence .
.
It is customary in algebraic automata theory to consider the semigroup-epimorphism 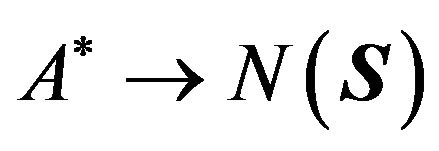 given by
given by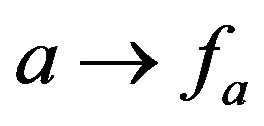 . The idea of simultaneous inputs enables us to transfer this epimorphism from semigroups to nearrings. We can, for instance, interpret
. The idea of simultaneous inputs enables us to transfer this epimorphism from semigroups to nearrings. We can, for instance, interpret 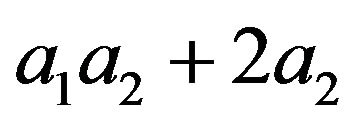 as being the complex input “input sequence
as being the complex input “input sequence  together with the simultaneous input
together with the simultaneous input 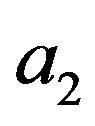 (in double strength)”. We extend A to the free near-ring A# over A. If
(in double strength)”. We extend A to the free near-ring A# over A. If 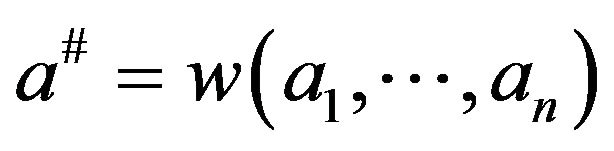 is a word in A# we define
is a word in A# we define  , and
, and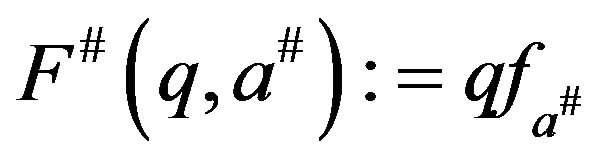 . Thus we get an extended simultaneous sequential GSA
. Thus we get an extended simultaneous sequential GSA  . Let I be {
. Let I be { is the zero map}. Then I is a near-ring ideal and we get by the homomorphism theorem:
is the zero map}. Then I is a near-ring ideal and we get by the homomorphism theorem: 
If we had used right near-rings, we would have 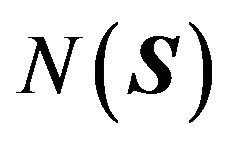 anti-isomorphic to
anti-isomorphic to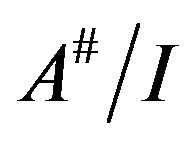 . Hence
. Hence 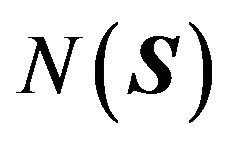 can be viewed as a homomorphic image of
can be viewed as a homomorphic image of . It is, however, impossible to give a nice canonical form for all elements of
. It is, however, impossible to give a nice canonical form for all elements of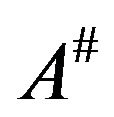 .
.
A possible relief comes from the observation that one might replace 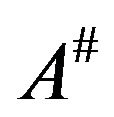 by
by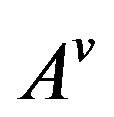 , the free algebra in a variety v of near-rings containing
, the free algebra in a variety v of near-rings containing 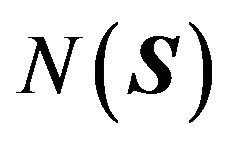 (for instance, one might take v as the variety generated by
(for instance, one might take v as the variety generated by ).
).
Attention! If A already bears some additive structure, this new addition can (and in most cases will) be different from the given addition in A! In particular, our new addition is one in  and not in
and not in .
.
In the linear case we saw that 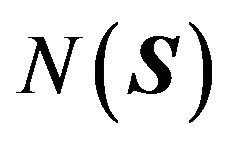 is an affine nearring. Since the class of all affine near-rings is known to form a variety, it makes sense to look at free affine nearrings, the more so since we know how this monsters look like.
is an affine nearring. Since the class of all affine near-rings is known to form a variety, it makes sense to look at free affine nearrings, the more so since we know how this monsters look like.
Let A be a set, A* the free monoid over A and  the free affine near-ring over A. Then every element of
the free affine near-ring over A. Then every element of 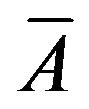 is a finite sum of elements
is a finite sum of elements 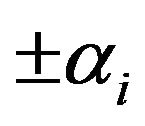 with
with . In fact. Since
. In fact. Since 
 and
and  are laws in the variety of affine near-rings, we can bring all expressions into
are laws in the variety of affine near-rings, we can bring all expressions into 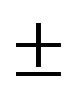 -sums of elements which are products of elements in
-sums of elements which are products of elements in 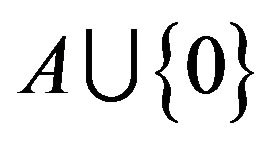 (observe that we use left near-rings!)
(observe that we use left near-rings!)
Let 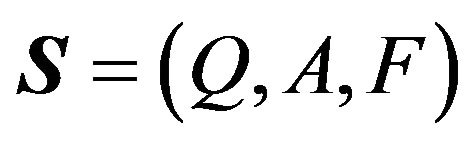 be a GSA and
be a GSA and  the free nearring on A.
the free nearring on A. 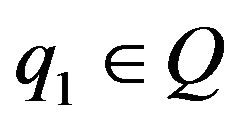 is accessible from
is accessible from 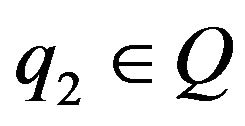 if there is some
if there is some 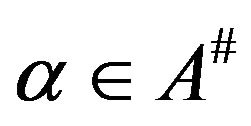 with
with .
. 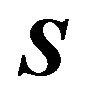 is accessible if each state q is accessible from each other state.
is accessible if each state q is accessible from each other state. 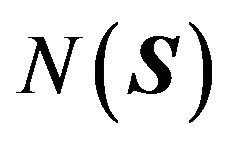 is not only a near-ring, but it also operates on Q. obviously Q is an
is not only a near-ring, but it also operates on Q. obviously Q is an 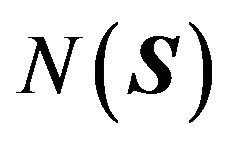 group via
group via 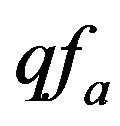 in the usual meaning.
in the usual meaning. 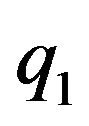 is accessible from
is accessible from 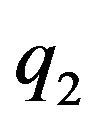 iff
iff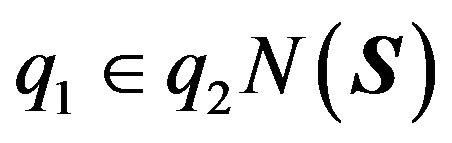 . Alternatively, Q can be viewed as an
. Alternatively, Q can be viewed as an 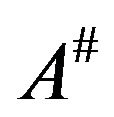 -group via
-group via . We have
. We have  is accessible iff Q is an
is accessible iff Q is an 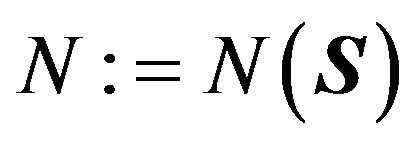 -group with
-group with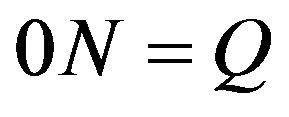 . In fact, if
. In fact, if  is accessible then obviously
is accessible then obviously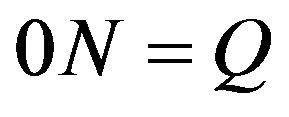 . Conversely, suppose that
. Conversely, suppose that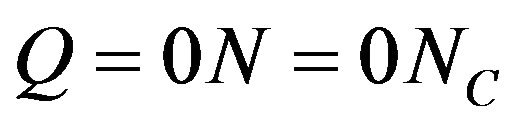 . If
. If 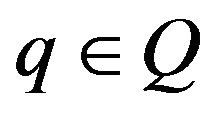 then
then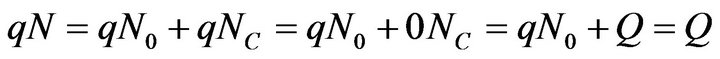 , and
, and  is shown to be accessible.
is shown to be accessible.
It might be most useful to examine the relationship between generators, primitivity and accessibility more closely. Now we look at constructions of semiautomata and their corresponding syntactic near-rings.
Let  and
and 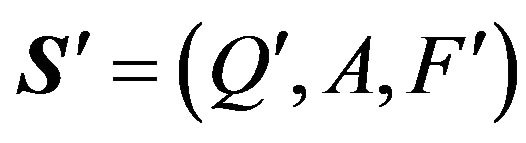 be GSA with identical input sets. A group homomorphism
be GSA with identical input sets. A group homomorphism  is called a GSA-homomorphism if
is called a GSA-homomorphism if 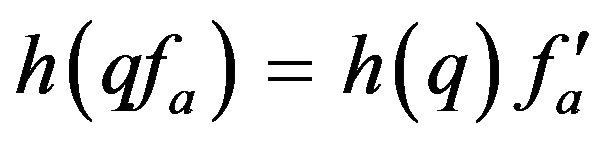 holds for all
holds for all 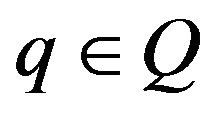 and
and 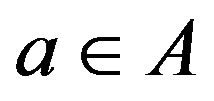 (with
(with  of course).
of course).
Theorem 3. Let  be a GSA-epimorphism. Then there exists a near-ring epimorphism
be a GSA-epimorphism. Then there exists a near-ring epimorphism 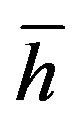 from
from 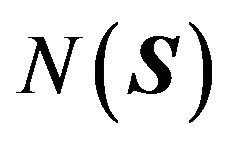 to
to 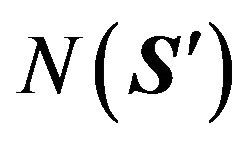 with
with 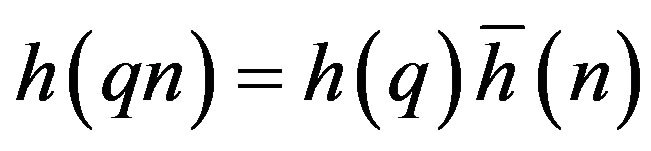 for all
for all 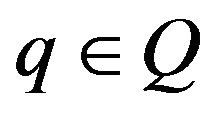 and
and .
.
Proof. If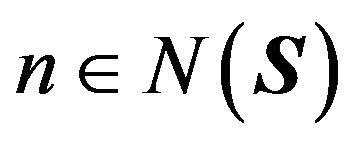 , n is a word
, n is a word
 in
in . Then
. Then
 by induction on the length of w. Define
by induction on the length of w. Define .
. 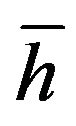 is well-defined since
is well-defined since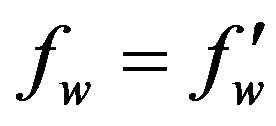 implies
implies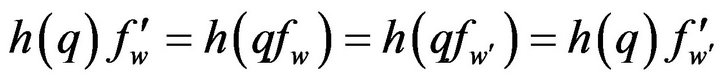 , for all
, for all
 . Since h is surjective,
. Since h is surjective, 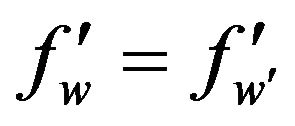 follows. Obviously,
follows. Obviously, 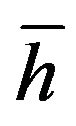 is a near-ring epimorphism and
is a near-ring epimorphism and 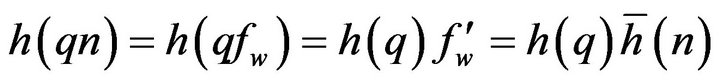 is also true for all
is also true for all 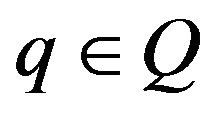 and
and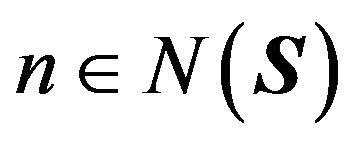 .
.
An automaton is a quintuple , where
, where 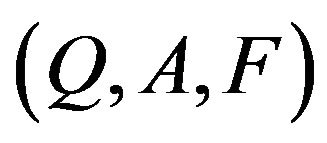 is a semiautomaton, B a set (the output set) and
is a semiautomaton, B a set (the output set) and  a function (called the output function of
a function (called the output function of ). If Q is a group,
). If Q is a group,  is called a groupautomaton (abbreviated by GA). We call
is called a groupautomaton (abbreviated by GA). We call  a homomorphic GA if Q, A, B are groups and F, G are homomorphisms.
a homomorphic GA if Q, A, B are groups and F, G are homomorphisms.  is called a linear GA or linear automaton or linear sequential machine if Q, A, B are R-modules for some ring R and F, G are R-linear maps [1].
is called a linear GA or linear automaton or linear sequential machine if Q, A, B are R-modules for some ring R and F, G are R-linear maps [1].
In many cases, however, outputs do play an essential role. For instance, if one wants to connect two (or more) automata in series. For doing that, consider 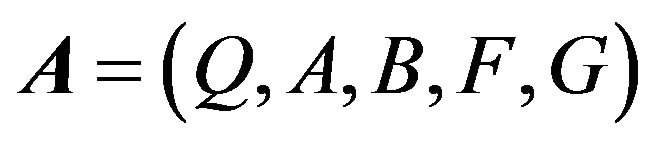 and
and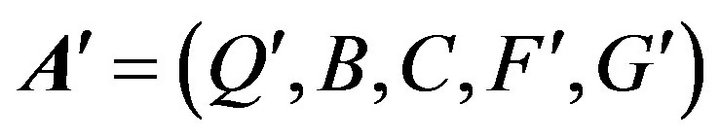 . The
. The
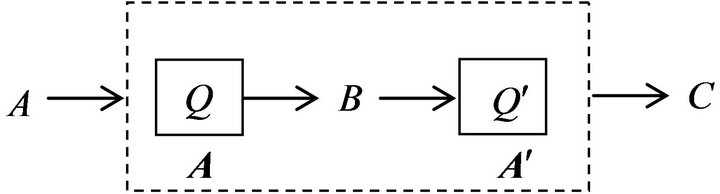
Series connection  s
s
outputs of  shall be the inputs of
shall be the inputs of 
More formally,  s
s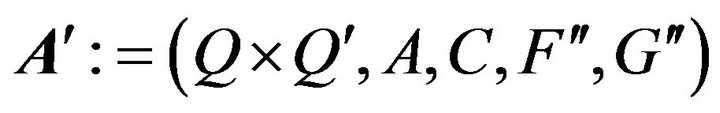 with
with
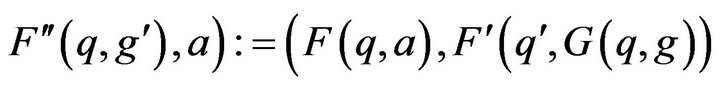 and
and
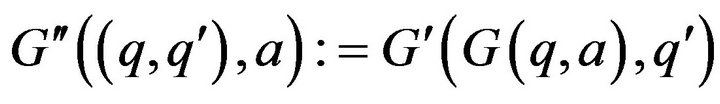 .
.
If  and
and  are linear GA then
are linear GA then  is the near-ring
is the near-ring 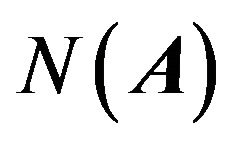
 additively generated by all pairs of the form
additively generated by all pairs of the form  (n is non negative integer)the constant-map-pairs
(n is non negative integer)the constant-map-pairs  and all
and all
 (n is non negative integer), with
(n is non negative integer), with 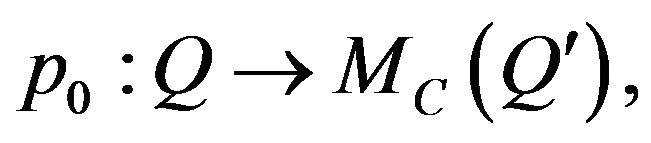
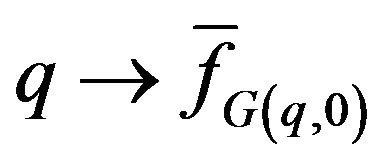 .
.
Let A* and B* denote the free monoids over A and B, respectively. For 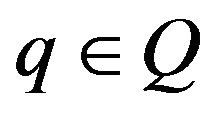 let
let 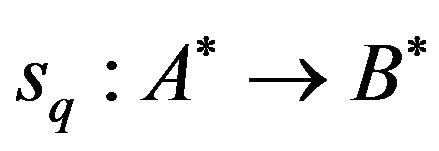 be defined by
be defined by
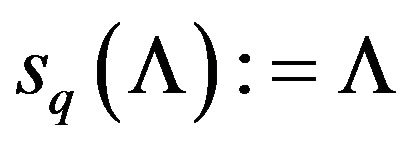 ,
, 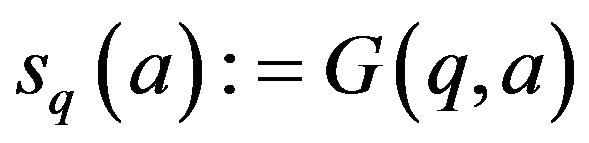 ,
,
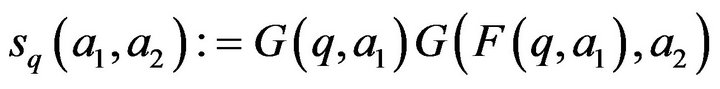
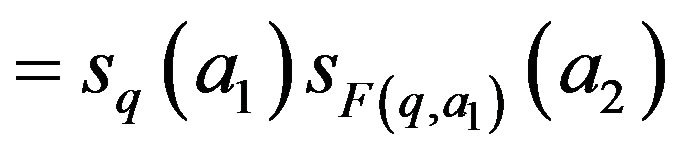 and proceed inductively with
and proceed inductively with
 .
.
 is called the sequential (input-output-) function of
is called the sequential (input-output-) function of  at q. If
at q. If  is a GA,
is a GA, 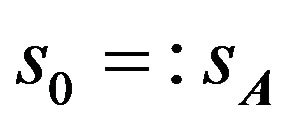 is called the sequential function of A. Furthermore, call
is called the sequential function of A. Furthermore, call 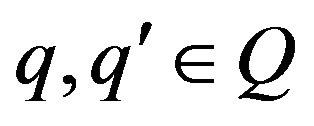 equivalent states (
equivalent states (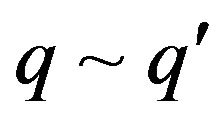 ) if
) if 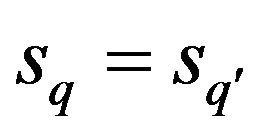 (i.e. if
(i.e. if 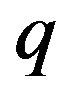 and
and  induce the same input-output-behaviour).
induce the same input-output-behaviour).
It might make sense to extend 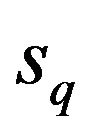 from
from 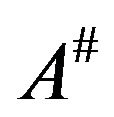 to
to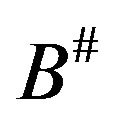 , where
, where  and
and 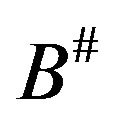 are the free near-rings [2] in a variety which contains the one generated by
are the free near-rings [2] in a variety which contains the one generated by 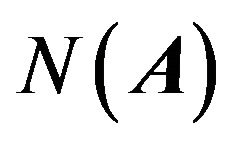 if we define
if we define
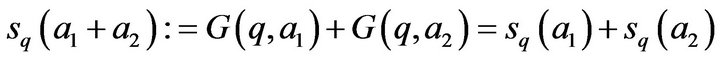 .
.
If 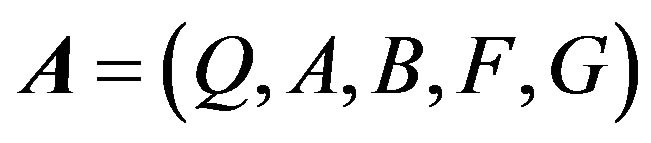 is homomorphic we get for
is homomorphic we get for 
If 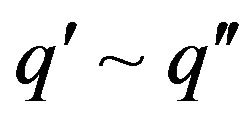 then
then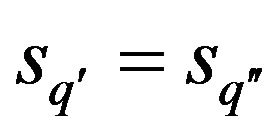 . Let
. Let . Then
. Then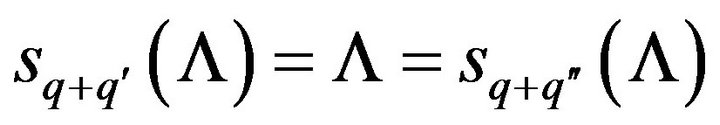 ;
;
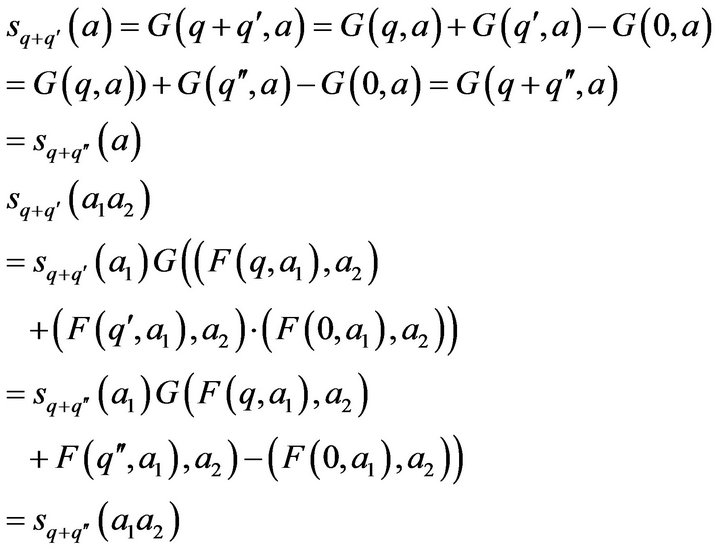
and so on, hence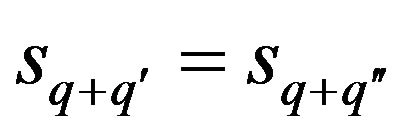 , whence
, whence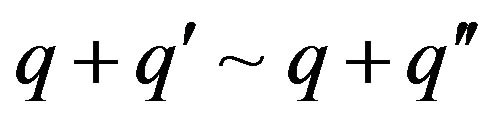 .
.
Similarly, if 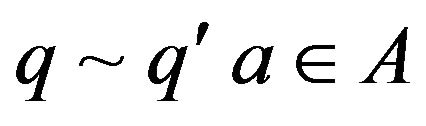 and
and 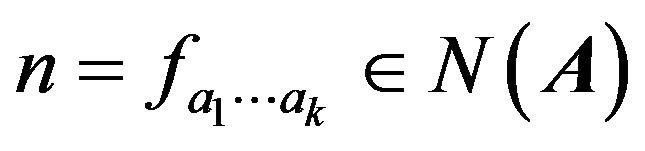 then
then
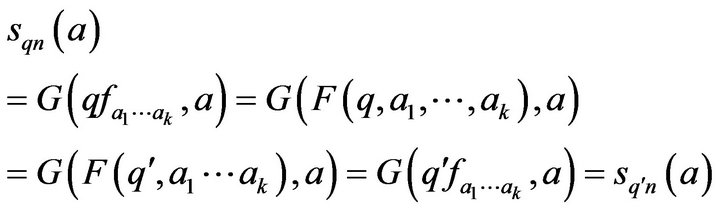
and induction shows . We there fore get Theorem 4. Let
. We there fore get Theorem 4. Let  be a homomorphic GA. Then ~ is a congruence relation in the
be a homomorphic GA. Then ~ is a congruence relation in the  -group Q. and (a)
-group Q. and (a) 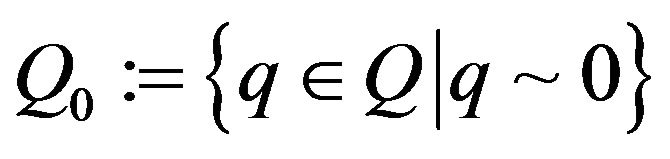 is an ideal of
is an ideal of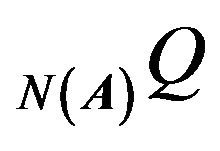 ; (b)
; (b) 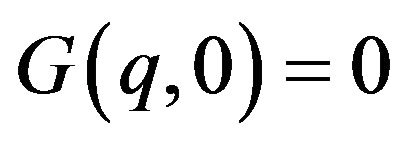 for all
for all .
.
We might ask what  means in detail Theorem 5. Let
means in detail Theorem 5. Let  be homomorphic and
be homomorphic and
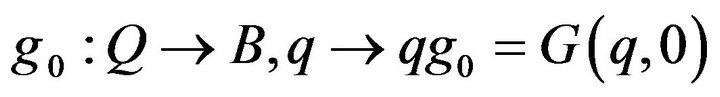 . Then
. Then 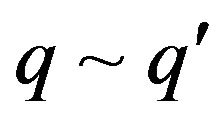
 For any non negative integer k,
For any non negative integer k, 
Proof. Let . We use induction on k and start with
. We use induction on k and start with . If
. If  then
then
 .
.
Since 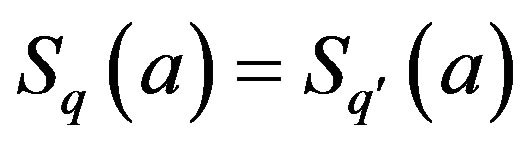 we get
we get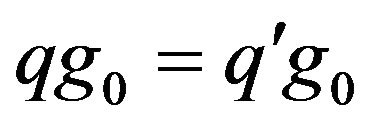 . Now suppose theorem 5 holds for all words
. Now suppose theorem 5 holds for all words 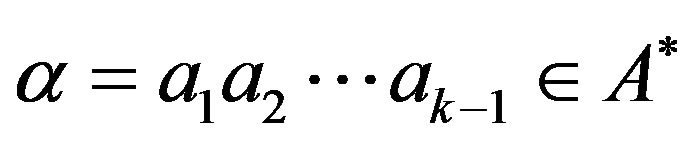 of length
of length . Then for all
. Then for all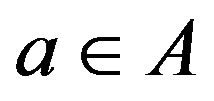 ,
, 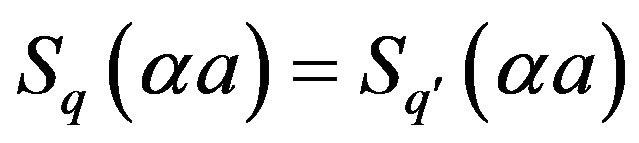 , hence
, hence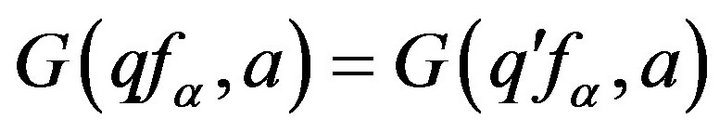 , we have,
, we have,

Similarly,
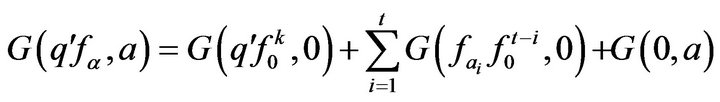 hence
hence 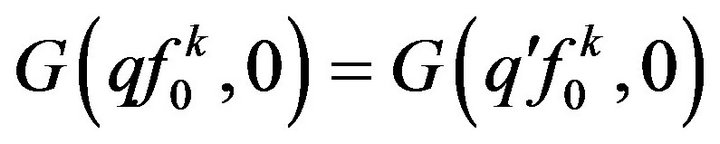 and we get
and we get
 . The converse is shown similarly.
. The converse is shown similarly.
A GA  is reduced if ~ is the equality. If
is reduced if ~ is the equality. If  is accessible (i.e. if (Q, A, F)is accessible) and reduced then
is accessible (i.e. if (Q, A, F)is accessible) and reduced then  is called minimal [1]. Obviously, a homomorphic GA is reduced iff
is called minimal [1]. Obviously, a homomorphic GA is reduced iff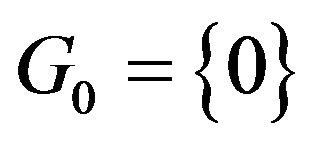 , we have Corollary 6. Let
, we have Corollary 6. Let 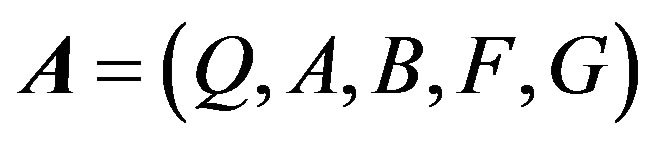 be a GA. Then
be a GA. Then
(a) 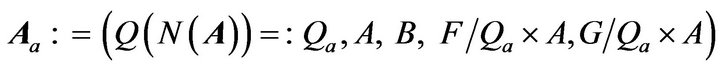
is accessible; (b) ; (c)
; (c)
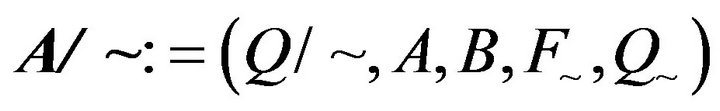 with
with
 and
and  is reduced; (d)
is reduced; (d)  is minimal.
is minimal.
The proofs are straightforward. In looking for criteria to decide if a given GA  is minimal or not, we obviously have to view Q not only as an
is minimal or not, we obviously have to view Q not only as an  -group but also have to care about B.
-group but also have to care about B.
Corollary 7. Let A be a homomorphic GA. Then A is reduced iff  has no non-zero ideals
has no non-zero ideals  with
with .
.
Proof. If  has no such ideals then
has no such ideals then  and
and  is reduced. So suppose that conversely
is reduced. So suppose that conversely  is reduced and that
is reduced and that 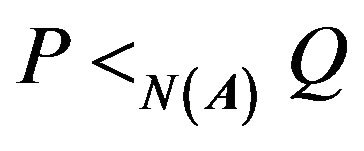 has
has 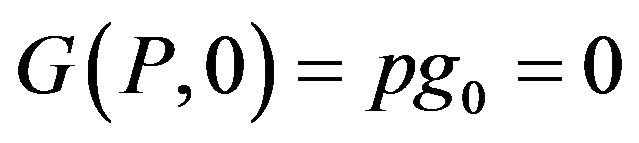 for all
for all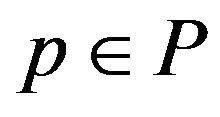 . If
. If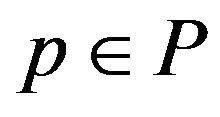 , we see by similar arguments that
, we see by similar arguments that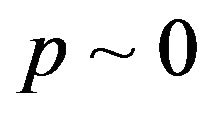 , hence
, hence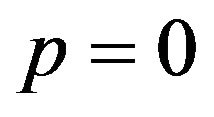 , whence
, whence .
.
From corollary 7 we get Corollary 8. Let  be a homomorphic GA. Then A is minimal iff
be a homomorphic GA. Then A is minimal iff 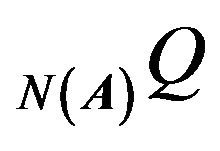 is generated by 0 and does not contain non-zero ideals which are annihilated by
is generated by 0 and does not contain non-zero ideals which are annihilated by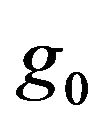 .
.
REFERENCES
- S. Eilenberg, “Automata, Language, and Machines,” Academic Press, New York, 1974.
- G. Pilz, “Near Rings,” North-Holland, Amsterdam, 1977.
- S. F. You, M. Cao and Y. J. Feng, “Semiautomata and Near Rings,” Quantitative Logic and Soft Computing, Vol. 5, 2012, pp. 428-431.
- S. F. You, H. Y. Zhao, Y. J. Feng and M. Cao, “An Application of Eulerian Graph to PI on Mn(C),” Applied Mathematics, Vol. 3, No. 7, 2012, pp. 809-811.
- S. F. You, “An Application of Eulerian Graph to Polynomial Identity,” IEEE Proceedings of the 2011 International Conference on Computational Intelligence and Software Engineering (CiSE 2011), Wuhan, 9-11 December 2011.
- S. F. You, et al., “Eulerian Graph and Polynomial Identities on Matrix Rings,” Advances in Mathematics, Vol. 32, No. 4, 2003, pp. 425-428.
- S. F. You, “The Primitivity of Extended Centroid Extension on Prime GPI-Rings,” Advances in Mathematics, Vol. 29, No. 4, 2000, pp. 331-336.

