Applied Mathematics
Vol.3 No.10(2012), Article ID:23372,9 pages DOI:10.4236/am.2012.310167
Retractions of One Dimensional Manifold
1Mathematics Department, Faculty of Science, Taibah University, Madinah, KSA
2Mathematics Department, Faculty of Science, Tanta University, Tanta, Egypt
Email: hk.mishra@juet.ac.in
Received July 23, 2012; revised September 10, 2012; accepted September 17, 2012
Keywords: Retractions; Deformation Retracts; Folding; One Dimensional Manifold
ABSTRACT
Our aim in the present article is to introduce and study types of retraction of one dimensional manifold. New types of geodesics in one dimensional manifold are presented. The deformation retracts of one dimensional manifold into itself and onto geodesics is deduced. Also, the isometric and topological folding in each case and the relation between the deformations retracts after and before folding has been obtained. New types of conditional folding are described.
1. Introduction
As is well known, the theory of retractions is always one of interesting topics in Euclidian and Non-Euclidian space and it has been investigated from the various viewpoints by many branches of topology and differential geometry El-Ahmady [1-3].
Most folding problems are attractive from a pure mathematical standpoint, for the beauty of the problems themselves. The folding problems have close connections to important industrial applications Linkage folding has applications in robotics and hydraulic tube bending. Paper folding has application in sheet-metal bending, packaging, and air-bag folding El-Ahmady [2,3]. Following the great Soviet geometer El-Ahmady [4], also used folding to solve difficult problems related to shell structures in civil engineering and aero space design, namely buckling instability El-Ahmady [4].
Isometric folding between two Riemannian manifold may be characterized as maps that send piecewise geodesic segments to a piecewise geodesic segments of the same length El-Ahmady [2-4]. For a topological folding the maps do not preserves lengths El-Ahmady [5,6], i.e. A map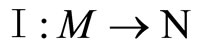 , where M and N are
, where M and N are  -Riemannian manifolds of dimension m, n respectively is said to be an isometric folding of M into N, iff for any piecewise geodesic path
-Riemannian manifolds of dimension m, n respectively is said to be an isometric folding of M into N, iff for any piecewise geodesic path , the induced path
, the induced path 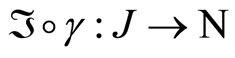 is a piecewise geodesic and of the same length as
is a piecewise geodesic and of the same length as , If
, If  does not preserve length, then
does not preserve length, then  is a topological folding El-Ahmady [7,8].
is a topological folding El-Ahmady [7,8].
A subset  of a topological space
of a topological space 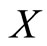 is called a retract of
is called a retract of 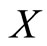 if there exists a continuous map
if there exists a continuous map 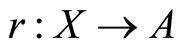 such that
such that 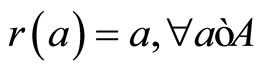 where A is closed and
where A is closed and 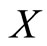 is open El-Ahmady [9-11]. Also, let
is open El-Ahmady [9-11]. Also, let 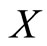 be a space and
be a space and 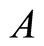 a subspace. A map
a subspace. A map 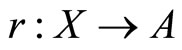 such that
such that 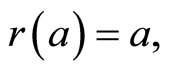 for all
for all  is called a retraction of
is called a retraction of 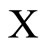 onto
onto  and
and  is called a retract of
is called a retract of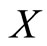 . This can be restated as follows. If
. This can be restated as follows. If 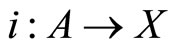 is the inclusion map, then
is the inclusion map, then 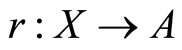 is a map such that
is a map such that 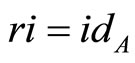 If, in addition,
If, in addition, 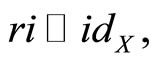 we call r a deformation retract and
we call r a deformation retract and 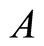 a deformation retract of
a deformation retract of 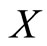 Another simple-but extremely useful-idea is that of a retract. If A,
Another simple-but extremely useful-idea is that of a retract. If A, 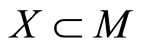 then
then 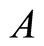 is a retract of
is a retract of 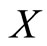 if there is a commutative diagram.
if there is a commutative diagram.
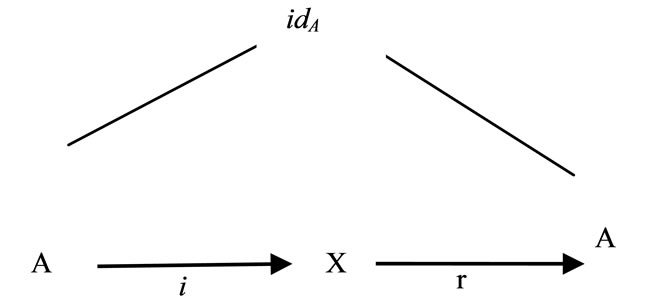
If  and
and  then f is a retract of g if there is a commutative diagram El-Ahmady [3,7], Arkowitz [12], Naber [13,14], Reid [15], Shick [16] and Strom [17].
then f is a retract of g if there is a commutative diagram El-Ahmady [3,7], Arkowitz [12], Naber [13,14], Reid [15], Shick [16] and Strom [17].
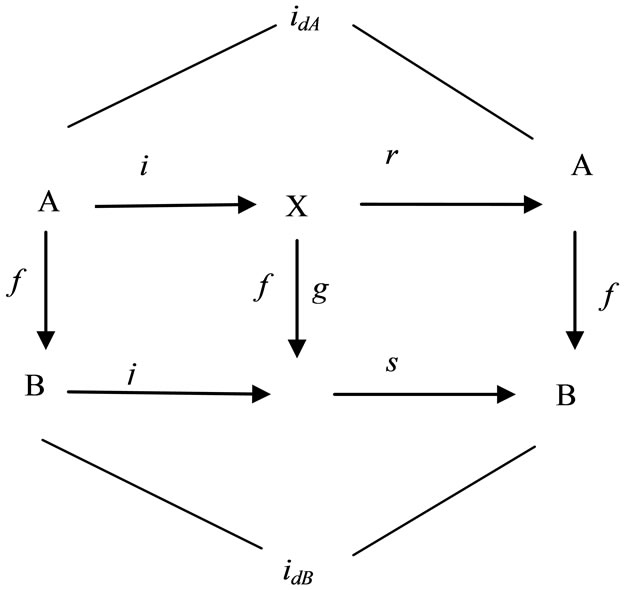
2. Main Result
2.1. On a Closed Interval
In what follows, we discuss the retractions, let the closed interval be 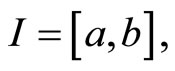 since the closed interval I is closed then firstly take a point
since the closed interval I is closed then firstly take a point 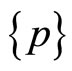 of it to make a retraction,
of it to make a retraction,  is open. Consider some types of retractions of
is open. Consider some types of retractions of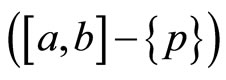 .
.
If 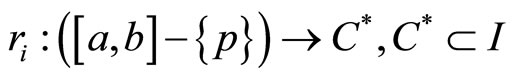
then we can get
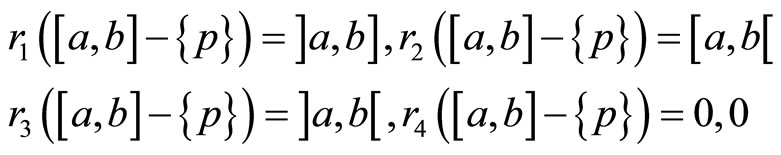 .
.
Now, we are in a position to formulate the following two theorems.
Theorem 1. All types of retraction of a closed interval are semi-open set or open set or zero-space.
Theorem 2. The limit of retraction of closed interval is a zero-manifold.
Now, we are going to discuss the deformation retract of the closed interval. Let 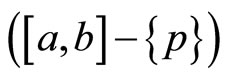 be the open interval, and then the homotopy map is defined as
be the open interval, and then the homotopy map is defined as
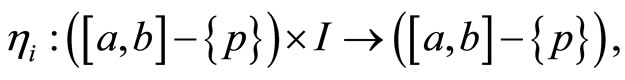 where
where 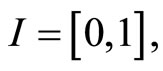
then we present the following cases of deformation retracts
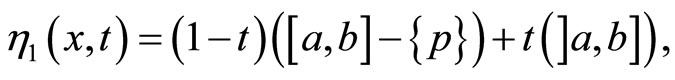
where 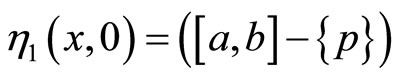
and
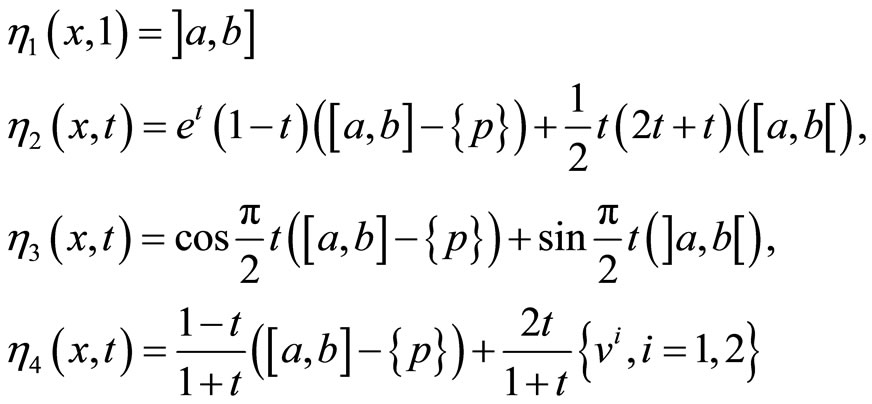
From the above discussion, we obtain the following theorem.
Theorem 3. The deformation retracts of a closed interval gives semi-open set, open set and zero-dimensional space.
Now, we are going to discuss the folding  of closed interval I.
of closed interval I.
Let , where
, where 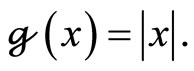
An isometric folding of closed interval I into itself may by
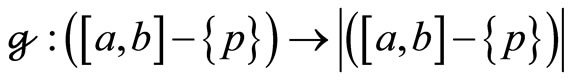
The deformation retracts of the folded closed interval 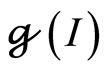 into the folded retraction
into the folded retraction 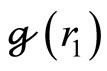 is
is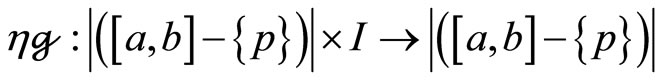 with
with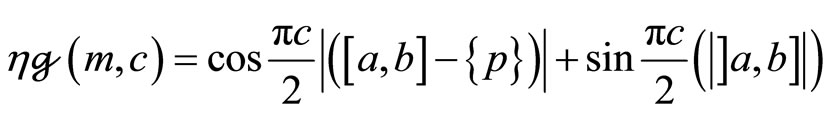 ,
, where
where 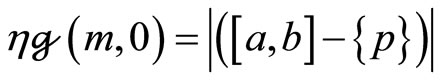 and
and 
The deformation retract of the folded closed interval  into the folded retraction
into the folded retraction 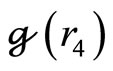 is
is
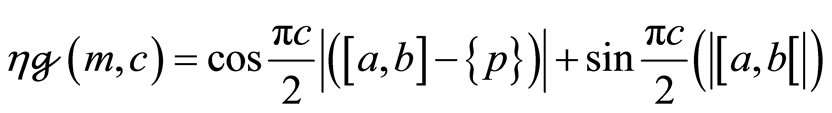
The deformation retract of the folded closed interval 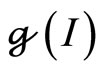 into the folded retraction
into the folded retraction 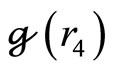 is
is
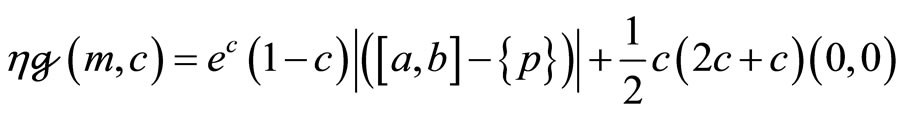
Then, the following theorem has been proved.
Theorem 4. The deformation retract of the folded closed interval into the folded retractions is the same as or different from the deformation retract of the closed interval into the retractions
Proposition 1. If the retraction of the closed interval 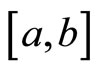 is
is 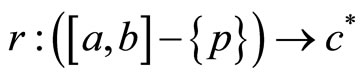 and the folding of (
and the folding of ( ) into itself is
) into itself is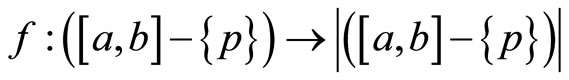 , then there are commutative diagram between retraction and folding such that
, then there are commutative diagram between retraction and folding such that
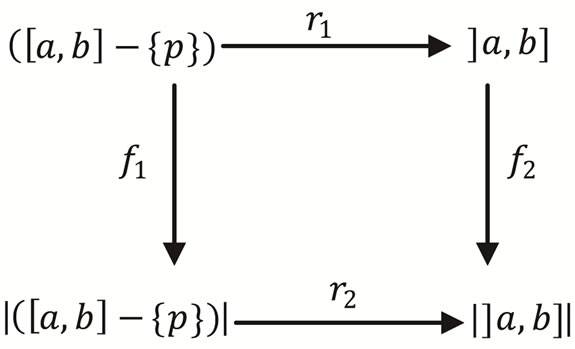
Proof. Let a retraction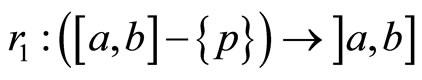 , be a retraction of
, be a retraction of 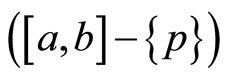 into
into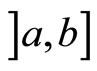 . Also, let the folding
. Also, let the folding 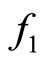 is
is 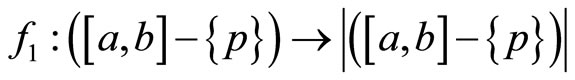 and the folding
and the folding 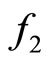 be
be . Then we have the retraction
. Then we have the retraction 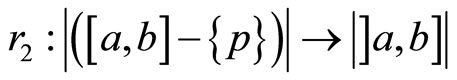 such that
such that
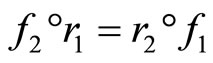
Proposition 2. The relation between the retraction of the closed interval and the limit of folding discussed from the following commutative diagram.
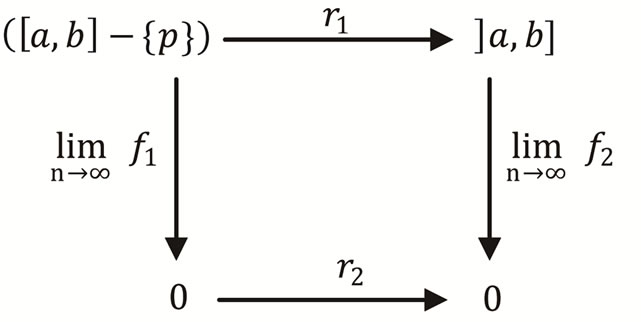
Proof. Let a retraction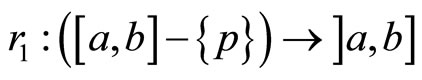 , be a retraction of
, be a retraction of 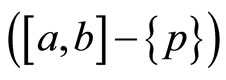 into
into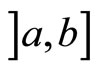 . Also, let the limits of folding are given by
. Also, let the limits of folding are given by
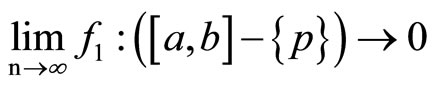 and
and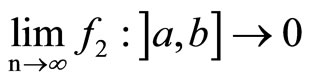 .
.
Then we have the retraction of the zero dimensional manifold is the identity map, i.e. 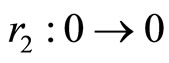 such that
such that
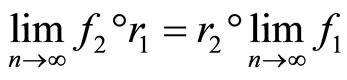
2.2. On a Cartesian Product of Closed Interval
In this position, we introduce the retraction of Cartesian product of closed interval. Consider two closed intervals . The Cartesian product is defined as
. The Cartesian product is defined as
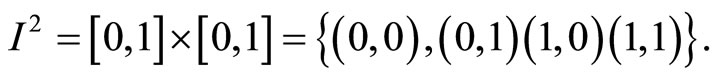
The retraction is defined as. Consider the square 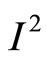 with vertices
with vertices 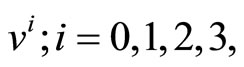 removing only one vertex
removing only one vertex 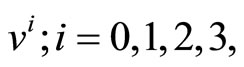 then the retraction is given by,
then the retraction is given by,
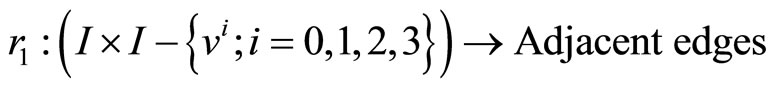 .
.
Also, removing two adjacent vertices is equivalent to removing an edge of the square, and then the retraction is defined as follows,
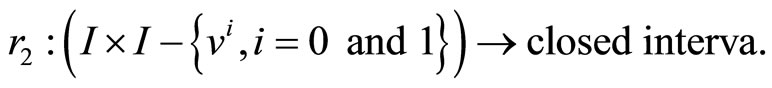
Moreover, removing two non-adjacent vertices gives a retraction, which is directly the zero-dimensional manifold,
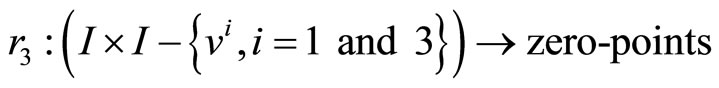
In what follows, we discuss the deformation retract of the square 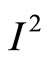 as follows. The deformation retract of the square
as follows. The deformation retract of the square  is defined by
is defined by
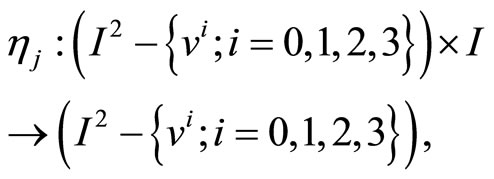
where 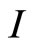 is the closed interval
is the closed interval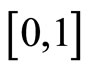 . Then we have the following cases of deformation retract. The deformation retract of the square
. Then we have the following cases of deformation retract. The deformation retract of the square  onto a
onto a 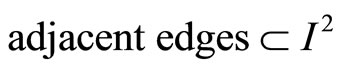 is given by
is given by
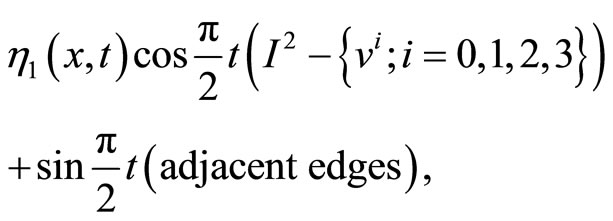
where 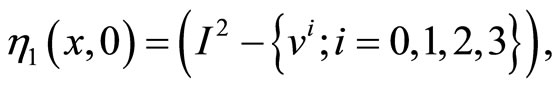
and 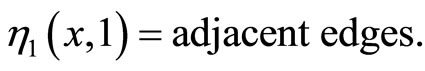
The deformation retract of the square  onto closed interval
onto closed interval 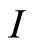 will be
will be
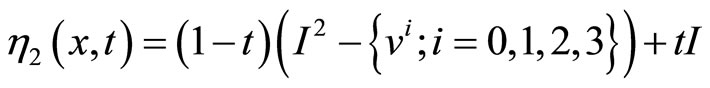
The deformation retract of the square  onto zero-points is
onto zero-points is
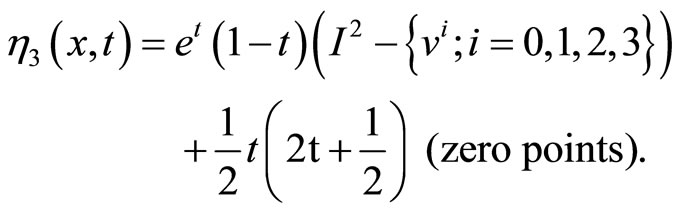
From all the above discussion, we arrive to the following theorem.
Theorem 5. The limit of retractions sequence of the square is the 0-dimensional manifold. Also, the deformation retract of the square is either subsquare or zero-dimensional manifold.
Proposition 3. If the retraction of the square 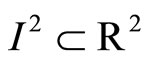 is
is
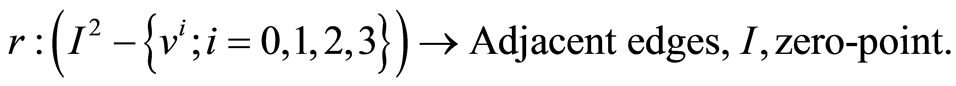
and the folding of 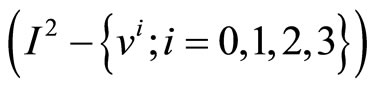 into itself is
into itself is
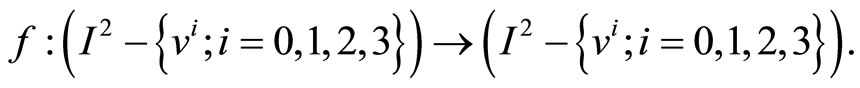
Then there are commutative diagrams between retractions and foldings such that
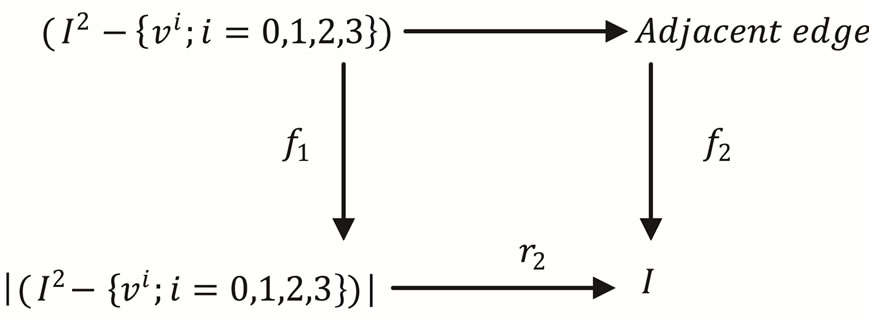
Also
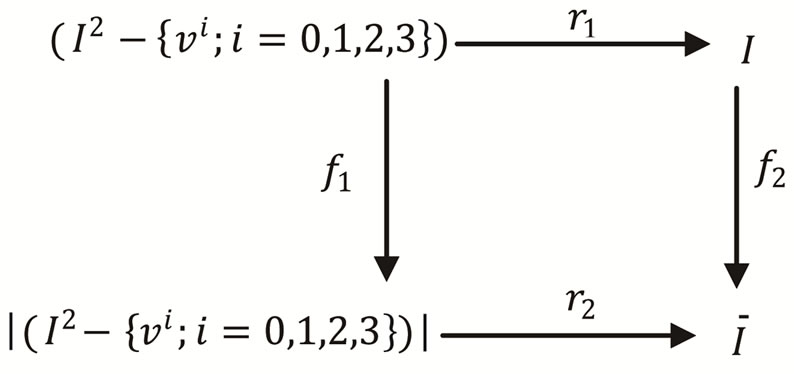
And also
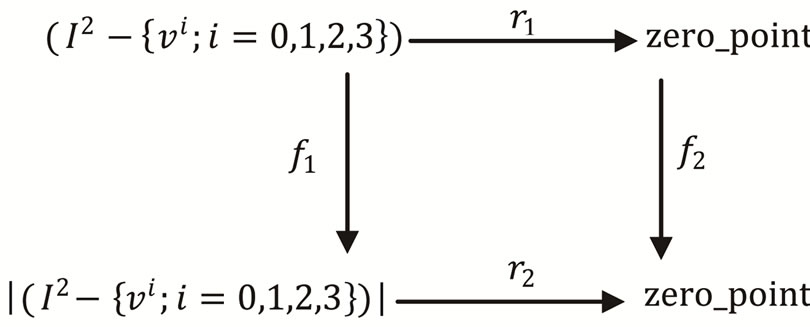
Proposition 4. The relation between the retraction of the square  and the limit of folding discussed from the following commutative diagrams.
and the limit of folding discussed from the following commutative diagrams.
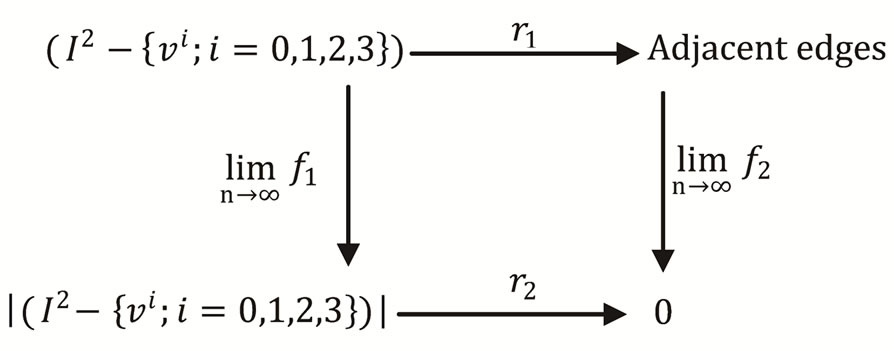
Also
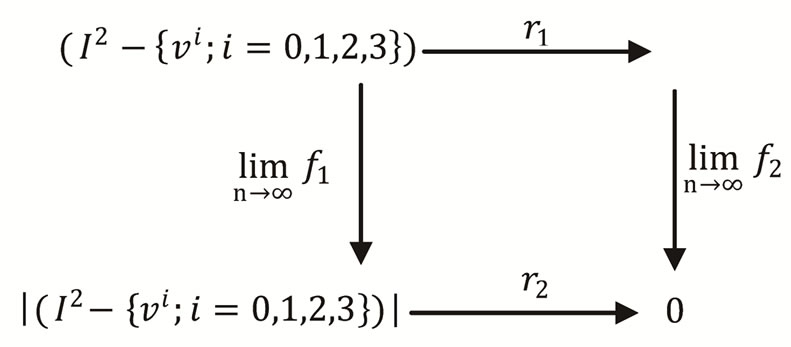
Again
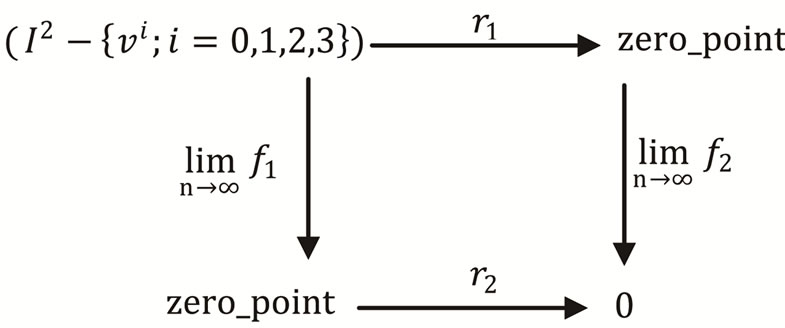
where the limit of the folding of the Cartesian product of 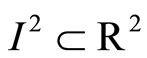 is not equal to the Cartesian product of their limits.
is not equal to the Cartesian product of their limits.
Proposition 5. If the deformation retract of 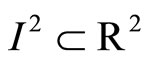
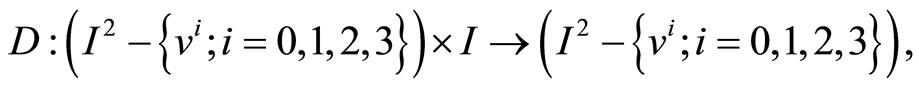
where 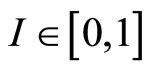 and the retraction of
and the retraction of 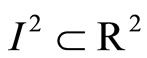 is
is 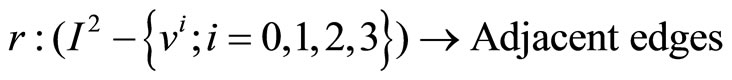 ,
,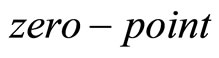 and the folding of
and the folding of 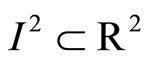 into itself is
into itself is 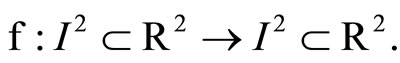 Then there are induces deformation retractions, and folding such that the following diagram is commutative.
Then there are induces deformation retractions, and folding such that the following diagram is commutative.
Proof. Let the deformation retract of 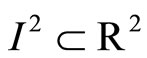 is
is
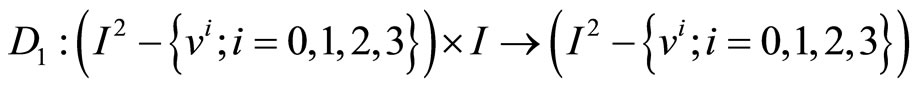 the folding of
the folding of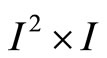 , and
, and
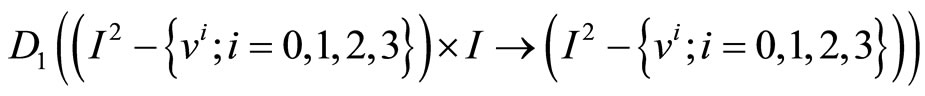
are defined by 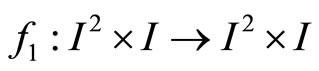 also
also
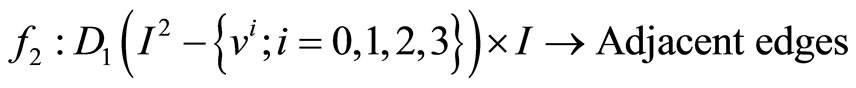 , the deformation retract of
, the deformation retract of 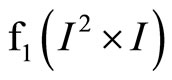 is
is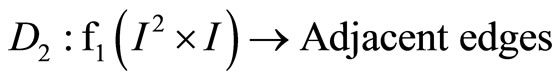 and the retractions of
and the retractions of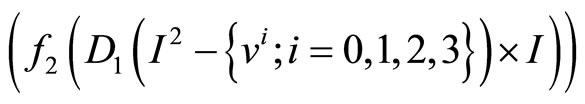 , and
, and 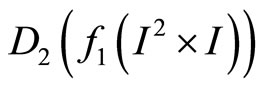
are given by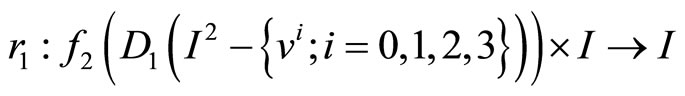 ,
,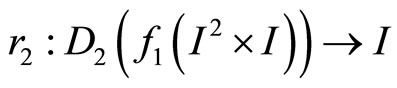 . Hence, the following diagram is commutative
. Hence, the following diagram is commutative
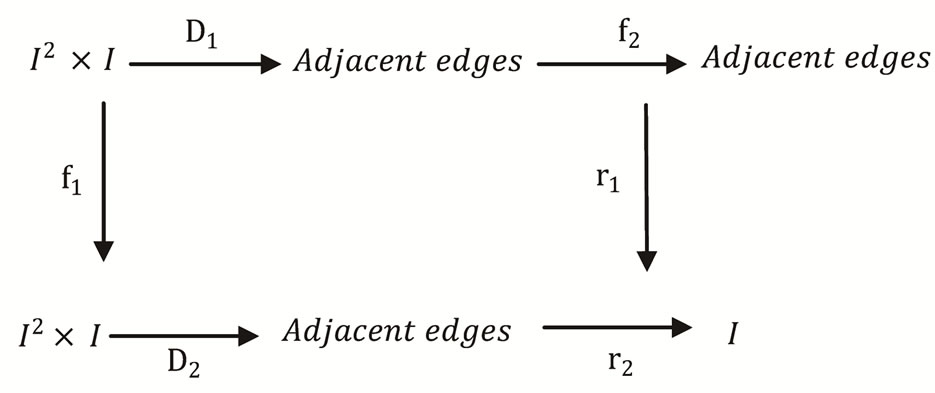
Also, the end of limits of the folding and the end of limits of retractions of 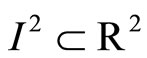 induces the 0-dimensional space which is a point and in this case the retraction and folding of 0-dimensional space coincide.
induces the 0-dimensional space which is a point and in this case the retraction and folding of 0-dimensional space coincide.
Proposition 6. The limit of the folding of 0-dimensional space M is 0-dimensional space.
Proof. Let 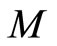 be an n-dimensional space, consider the limit of the folding
be an n-dimensional space, consider the limit of the folding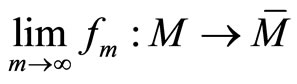 ,
,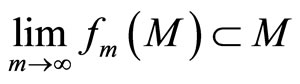 , then
, then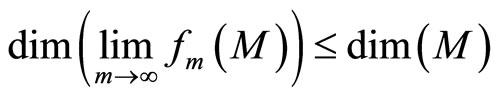 but if M has 0-dimension. Then
but if M has 0-dimension. Then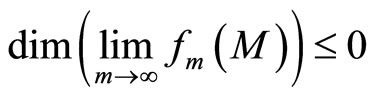 . Since
. Since 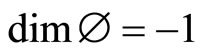 , then
, then  .
.
Theorem 6. 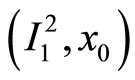 is a strong deformation retract of
is a strong deformation retract of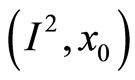 .
.
Proof. Let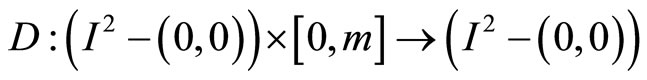 , where
, where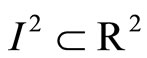 ,
, 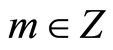 is a strong deformation retracting of
is a strong deformation retracting of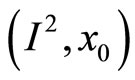 ,
, 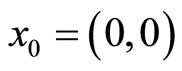 into
into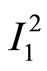 . To be specific, the k-homotopy D is assumed:
. To be specific, the k-homotopy D is assumed:
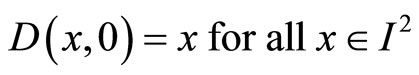 ,
, 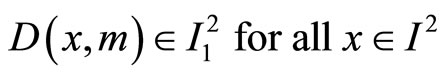 , and
, and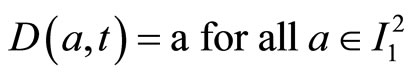 , and
, and .
.
Let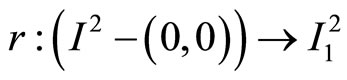 , be defined as
, be defined as
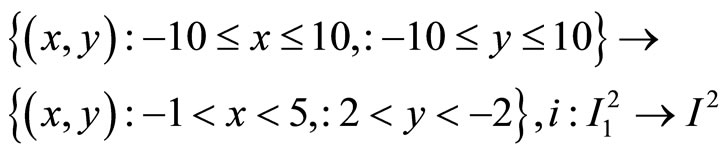
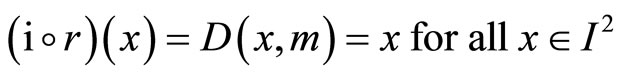 Also
Also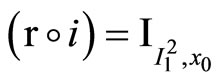 .
.
Then, 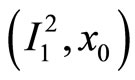 is a strong deformation retract of
is a strong deformation retract of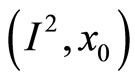 .
.
Proposition 7. The retraction of 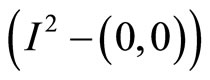 is a two-dimensional manifold and the limit of foldings is a one-manifold.
is a two-dimensional manifold and the limit of foldings is a one-manifold.
Proof. If A is a retraction of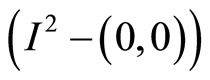 , then either dimension A = dimension
, then either dimension A = dimension 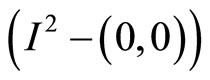 or dimension A ≠ dimension
or dimension A ≠ dimension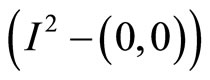 , but in this case dimension
, but in this case dimension 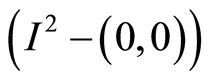 not invariant. Then
not invariant. Then 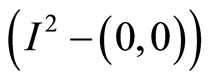 is the same dimension of I2. But, the limit of the foldings of 2-dimensional manifold into itself is a manifold of dimension n − 1. Then, the limit of foldings is a one-manifold.
is the same dimension of I2. But, the limit of the foldings of 2-dimensional manifold into itself is a manifold of dimension n − 1. Then, the limit of foldings is a one-manifold.
2.3. On a Circle
Theorem 7. If 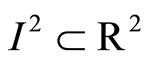 has the fixed point property, then
has the fixed point property, then 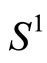 is not a retract of
is not a retract of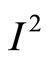 .
.
Proof. Let 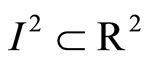 has the fixed point property. Observe that
has the fixed point property. Observe that 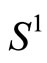 certainly does not have the fixed point property since, for example, the antipodal map-
certainly does not have the fixed point property since, for example, the antipodal map-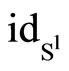 is continuous, but has no fixed points. Then
is continuous, but has no fixed points. Then 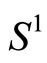 can therefore not be a retract of
can therefore not be a retract of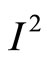 .
.
Proposition 8. If 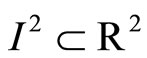 has the fixed point property, then
has the fixed point property, then 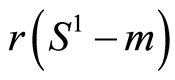 is not a retract of
is not a retract of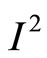 , where
, where
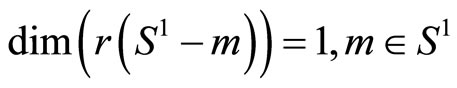 .
.
Proof. Since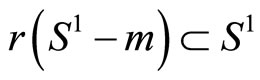 , and
, and 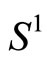 does not have the fixed point property. Then
does not have the fixed point property. Then 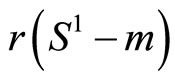 does not have the fixed point property,
does not have the fixed point property,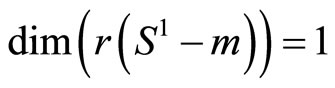 . Then
. Then 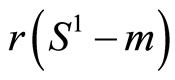 is not a retract of
is not a retract of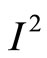 .
.
Theorem 8. If 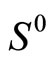 is a 0-manifold, then
is a 0-manifold, then 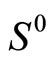 is a retract of
is a retract of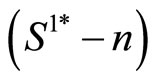 , such that
, such that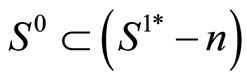 ,
,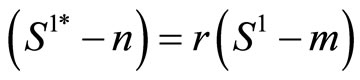 , and
, and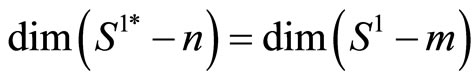 where
where 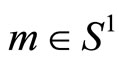 and
and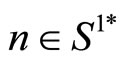 .
.
Proof. Now, let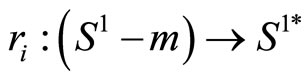 ,
, 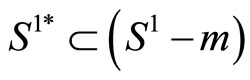 ,
,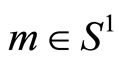 be the retraction map of
be the retraction map of 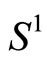 defined as
defined as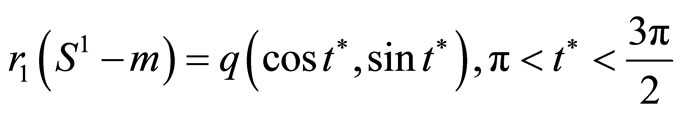 . Let the inclusion map of
. Let the inclusion map of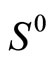 , where
, where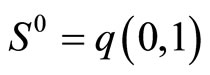 , is
, is , the retractions of
, the retractions of 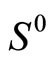 and
and  are defined by
are defined by 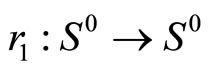 and
and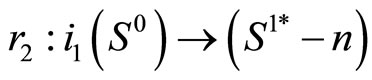 , where
, where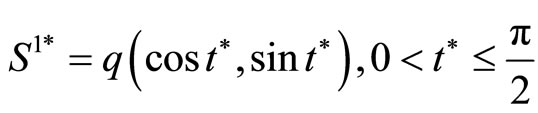 ,
,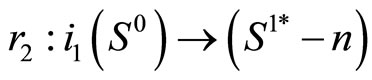 is the inclusion map of
is the inclusion map of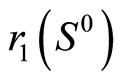 , the retraction of
, the retraction of 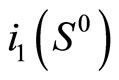 is
is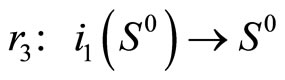 , also the retractions of
, also the retractions of 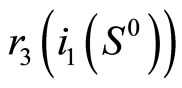 and
and 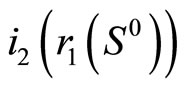 are given by
are given by 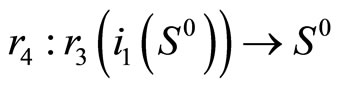 and
and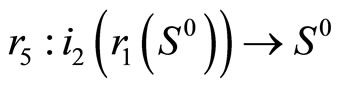 .
.
Hence the following diagram is commutative:
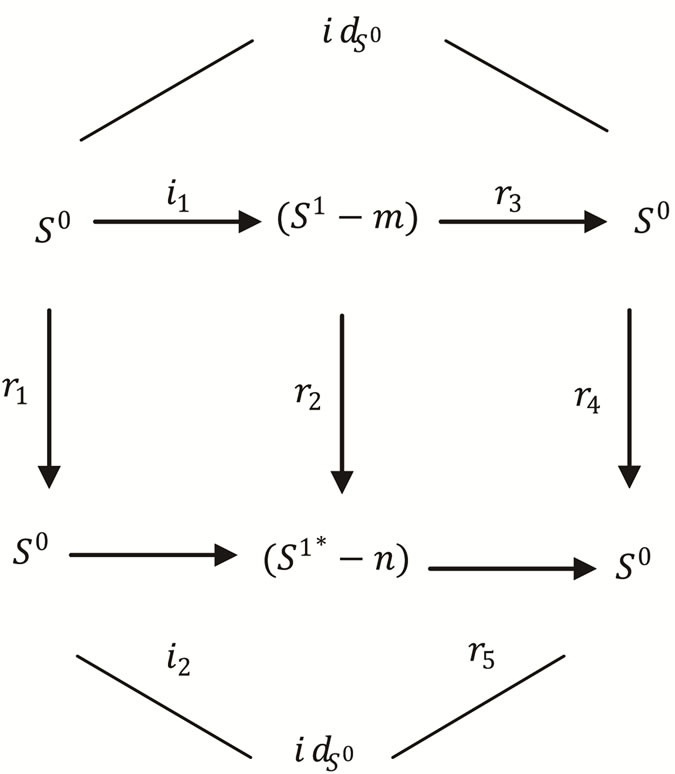
Proposition 9. The relation between the retraction, the limit of the folding and the inclusion map of circle  discussed from the following commutative diagram
discussed from the following commutative diagram
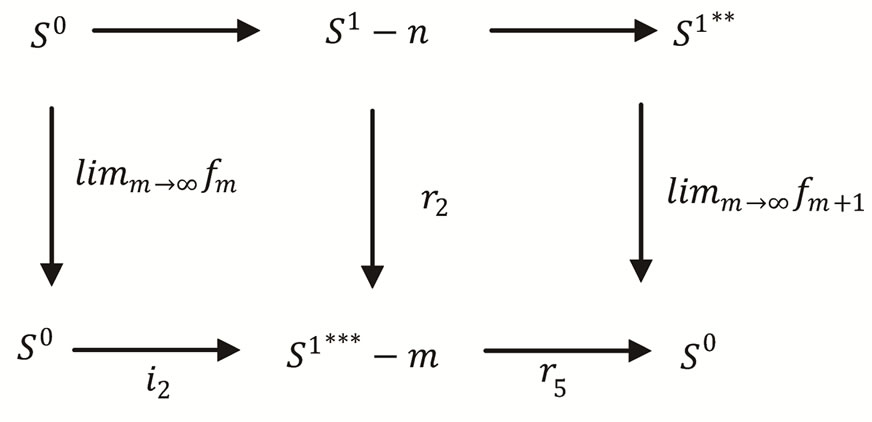
where
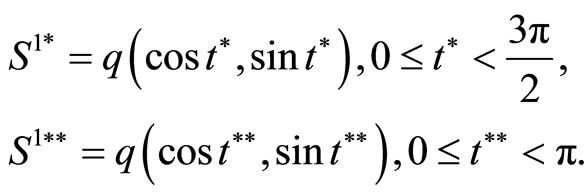
and
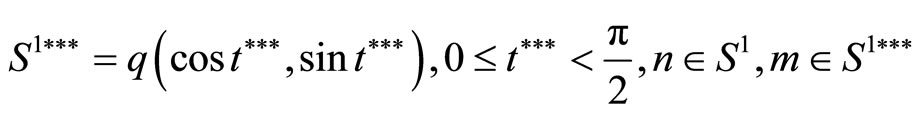
The purpose of this position is to introduce the relation between the deformation retract and folding of the circle, the parametric equation of the open circle in the plane is given by
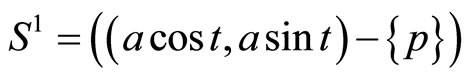 .
.
Now consider some types of retractions of the circle
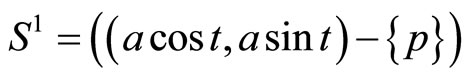 , if
, if
 then we can get
then we can get
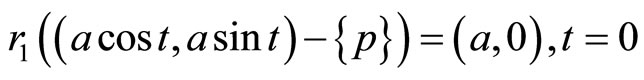 ,
,
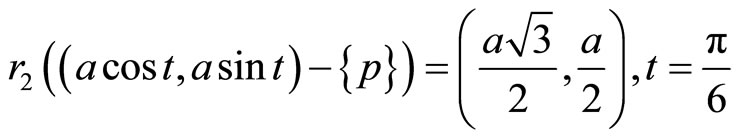 ,
,
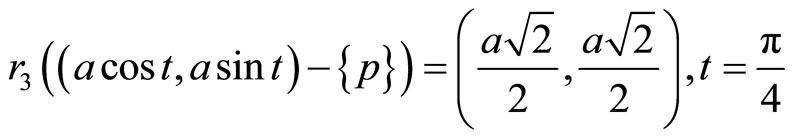 ,
,
 ,
,
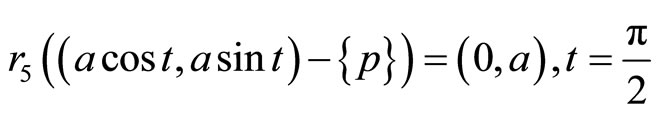 ,
,
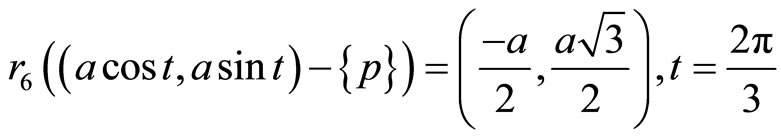 ,
,
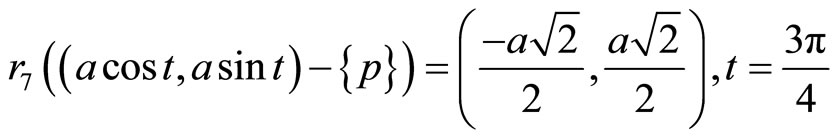 ,
,
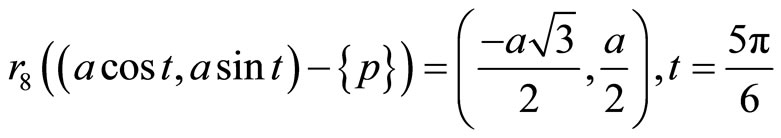 ,
,
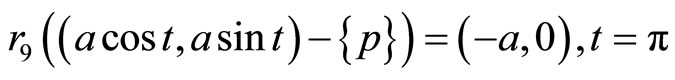 ,
,
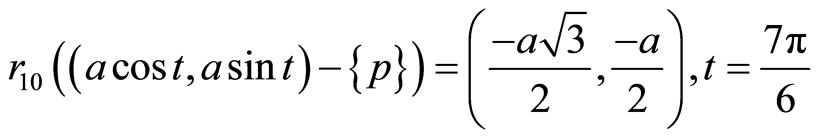 ,
,
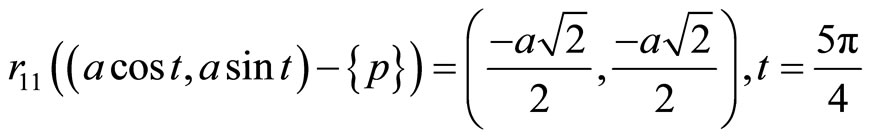 ,
,
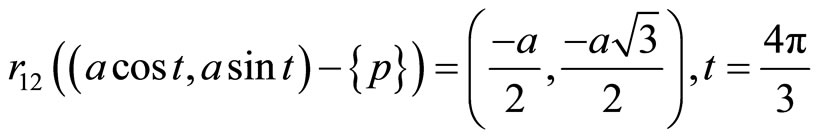 ,
,
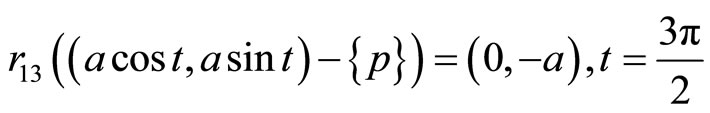 ,
,
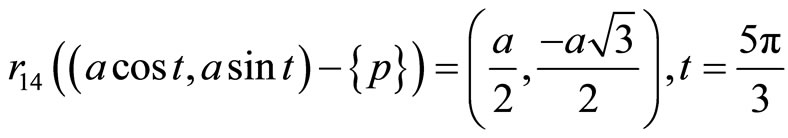 ,
,
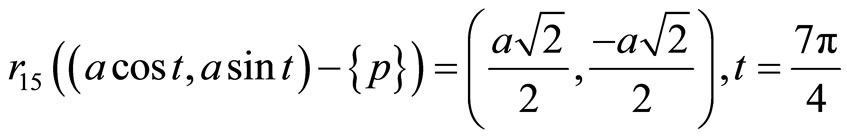 ,
,
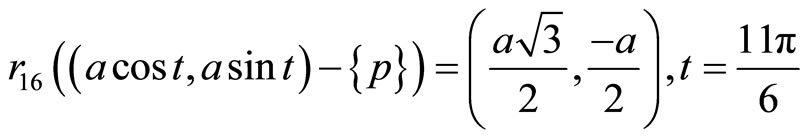 ,
,
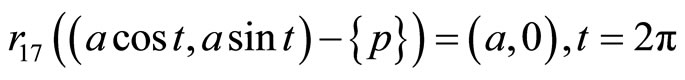 .
.
Now, we are going to discuss the deformation retract of the circle. Let 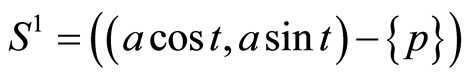 be the open circle, then the homotopy map is defined as
be the open circle, then the homotopy map is defined as
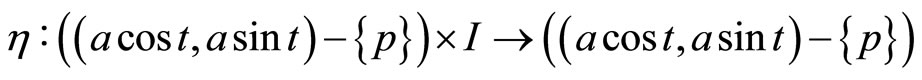 where
where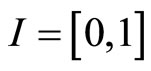 ,
,
Then we have the following cases of deformation retracts.
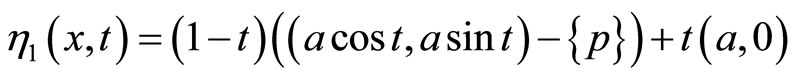 where
where 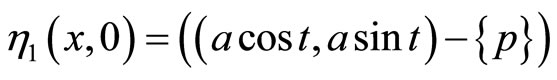
and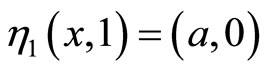 ,
,
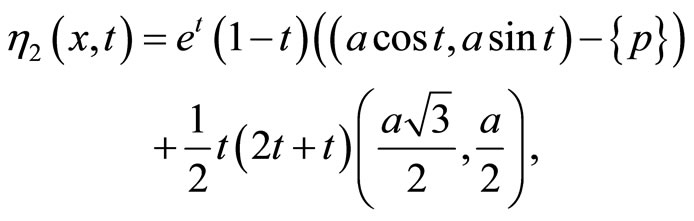
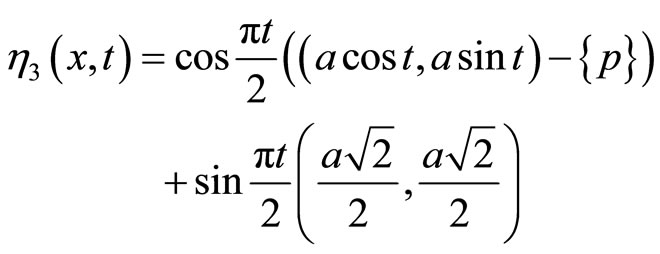
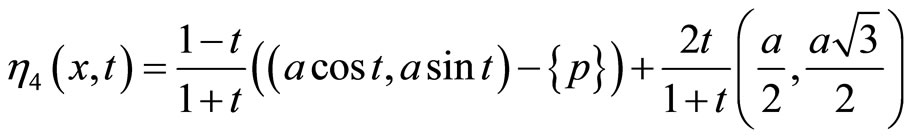 ,
,
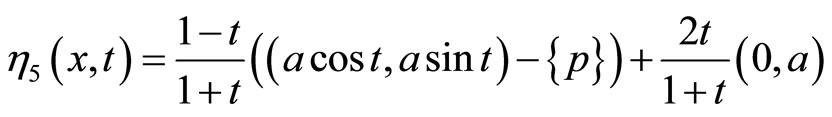 ,
,
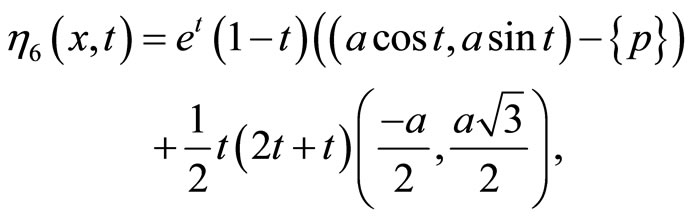

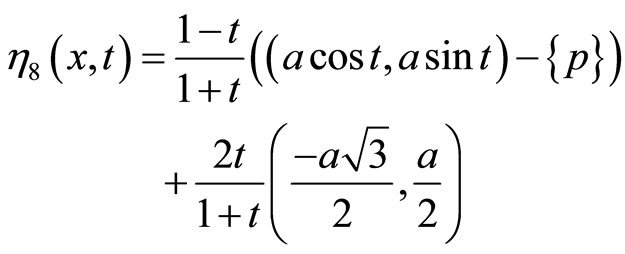
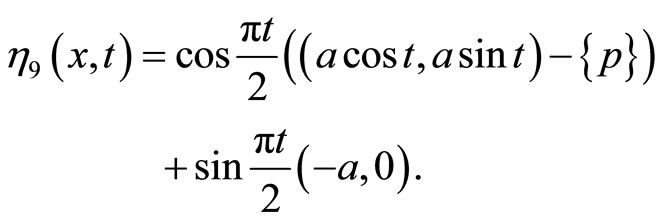
Now, we are going to discuss the folding  of the circle
of the circle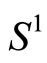 . let
. let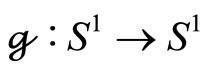 , where
, where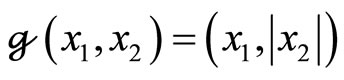 .
.
An isometric folding of the circle 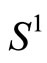 into itself may by defined by
into itself may by defined by
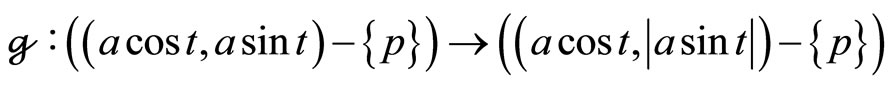
The deformation retracts of the folded circle 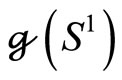 into the folded retraction
into the folded retraction 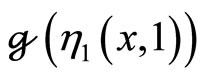 is
is
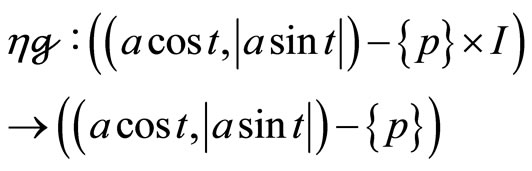
with
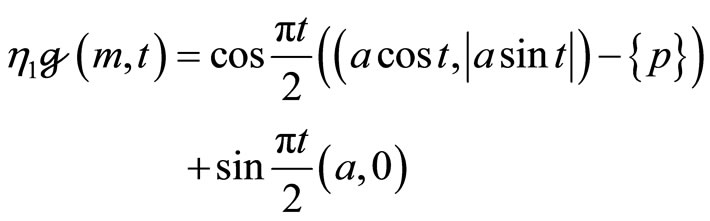
where
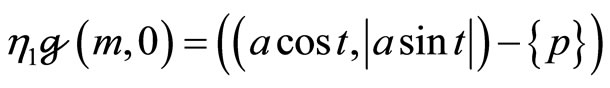
and 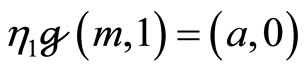
Then, the following theorem has been proved.
Theorem 9. Under the defined folding and any folding homeomorphic to this type of folding, the deformation retracts of the folded circle into the folded retractions  is the same as the deformation retracts of the circle into the retractions.
is the same as the deformation retracts of the circle into the retractions.
If the folding be defined by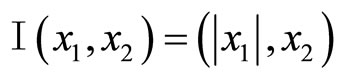 .
.
The isometric folded of the circle 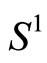 is defined as
is defined as
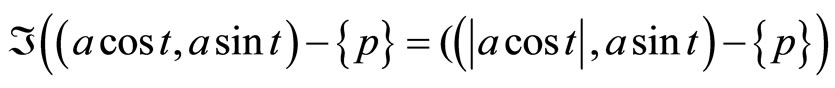
The deformation retract of the folded circle 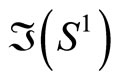 into the folded retraction
into the folded retraction 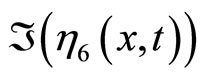 is
is
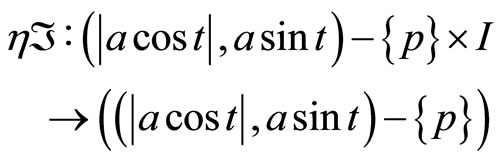
with
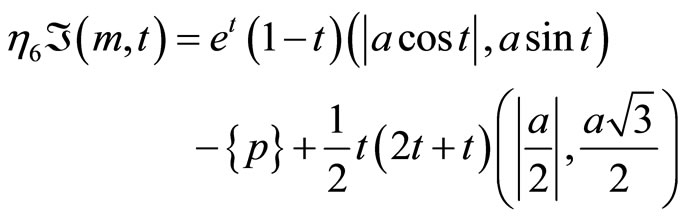
The deformation retract of the folded circle 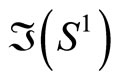 into the folded retraction
into the folded retraction 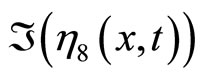 is
is
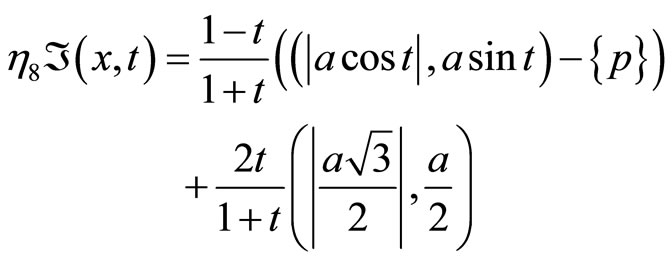
Then, the following theorem has been proved.
Theorem 10. Under the defined folding and any folding homeomorphic to this type of folding, the deformation retract of the folded circle into the folded retractions is different from the deformation retract of the circle into the retractions.
Proposition 10. If the retraction of the circle  is:
is: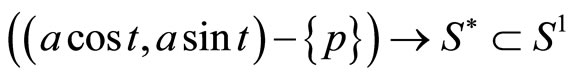 , and the folding of
, and the folding of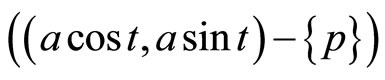 into itself is
into itself is
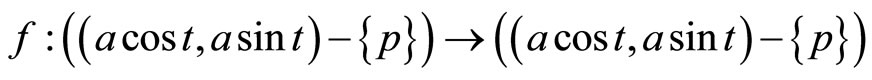 ).
).
Then there are commutative diagrams between retraction and folding such that:
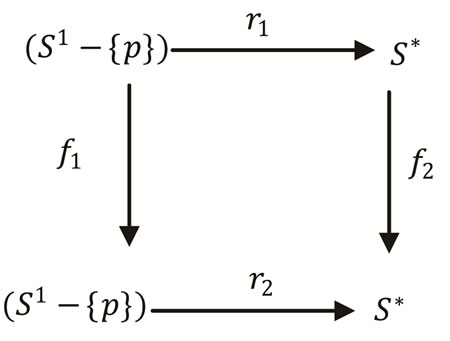
Proof. Let a retraction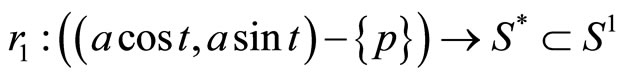 , be a retraction of
, be a retraction of 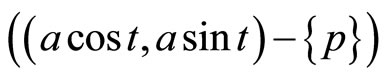 into
into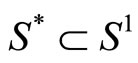 . Also, let the folding
. Also, let the folding  is given by
is given by
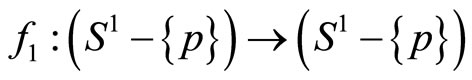 and
and , also,
, also,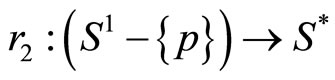 such that
such that
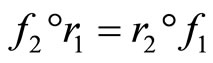
Let 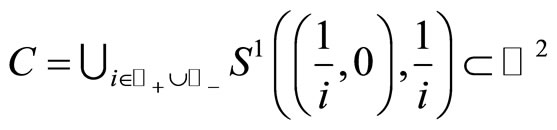 where
where
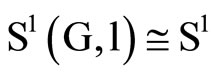 is the circle with center
is the circle with center  and radius
and radius . The intersection of all circles is denoted by 0. Let
. The intersection of all circles is denoted by 0. Let
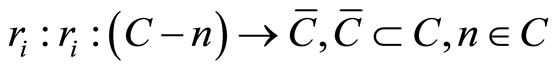 , be the retraction map of
, be the retraction map of 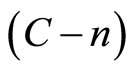 such that
such that
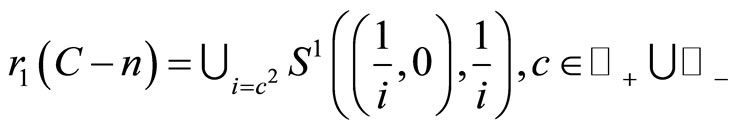 ,
,
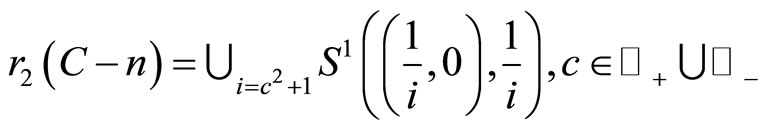 ,
,
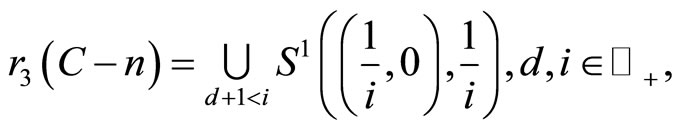
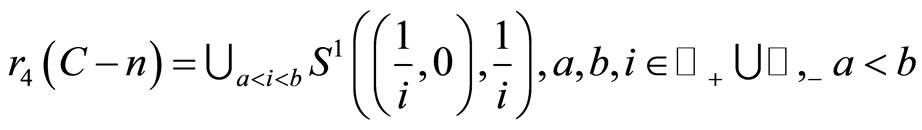 ,
,
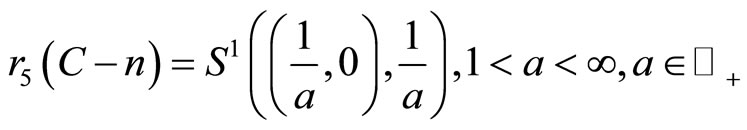 ,
,
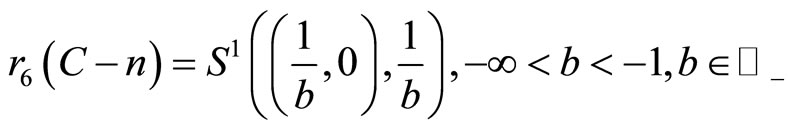
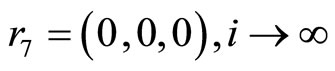 ,
,
 ,
,
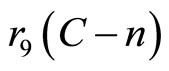 ,
,
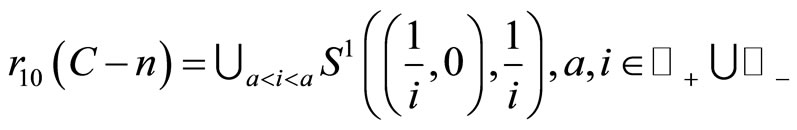 ,
,
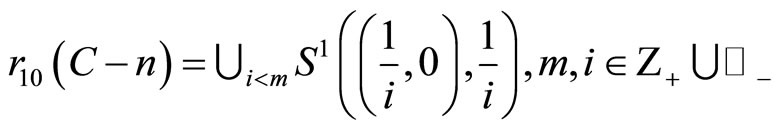
Hence, we can introduce the following theorems:
Theorem 11. Any circle  with center
with center 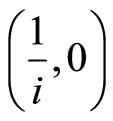 and radius
and radius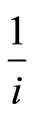 , where
, where , is a retract of
, is a retract of
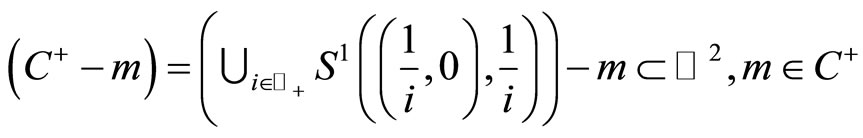 .
.
Proof. Let 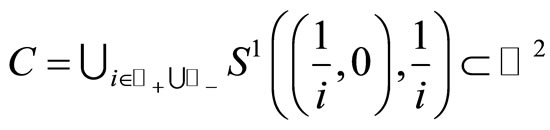 and
and  is a retract of
is a retract of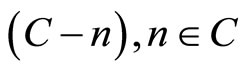 , where
, where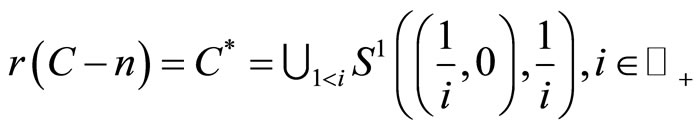 . Also, If
. Also, If 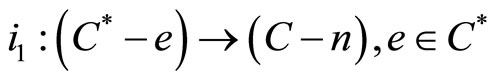 , is the inclusion map, the retractions of
, is the inclusion map, the retractions of 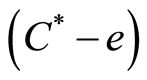 and
and 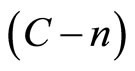 are defined by
are defined by and
and , the inclusion map of
, the inclusion map of 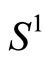 is
is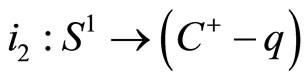 , and the retractions of
, and the retractions of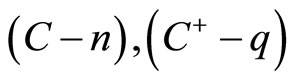 , and
, and 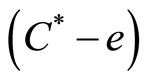 are given by
are given by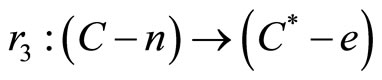 ,
, 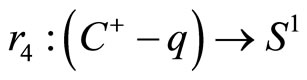 and
and
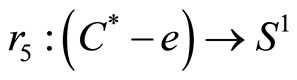 . Hence, the following diagram is commutative:
. Hence, the following diagram is commutative:
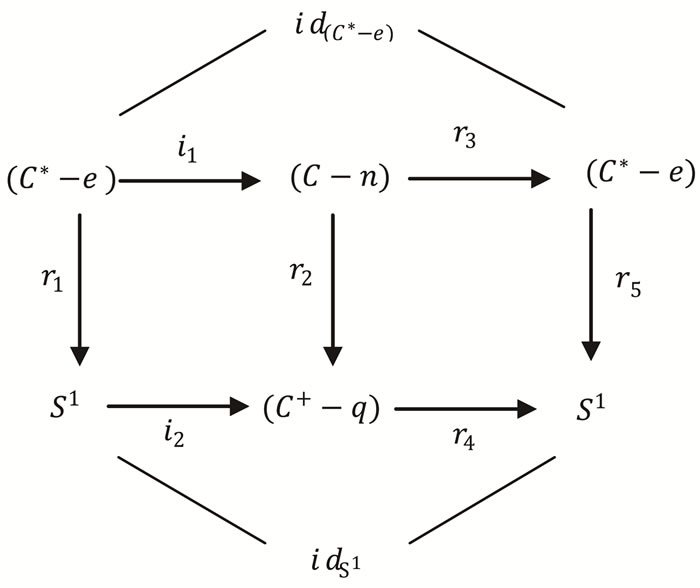
Proposition 11. Any circle 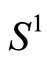 with center
with center 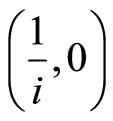 and radius
and radius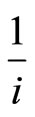 , where
, where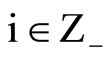 , is a retract of
, is a retract of
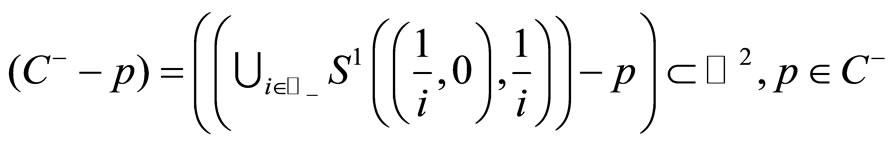 .
.
Theorem 12. Any circle 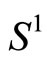 with center
with center 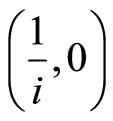 and radius
and radius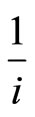 , where
, where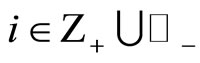 , is a retract of
, is a retract of
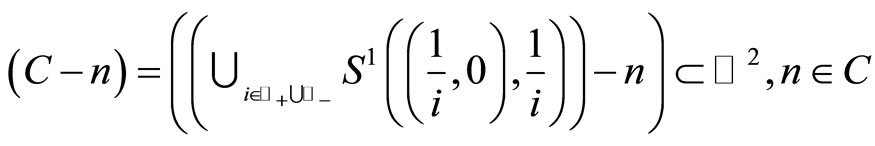 .
.
Proof. Since 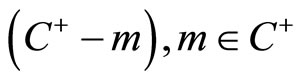 is a retract of
is a retract of 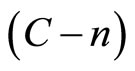 and any circle
and any circle 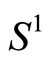 in
in  with center
with center 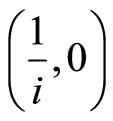 and radius
and radius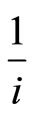 , where
, where , is a retract of
, is a retract of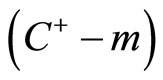 , then any circle
, then any circle 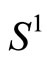 with center
with center  and radius
and radius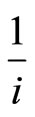 , where
, where , is a retract of
, is a retract of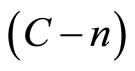 . Also, Since
. Also, Since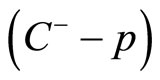 ,
, 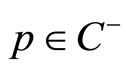 is a retract of
is a retract of  and any circle
and any circle 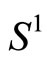 with center
with center 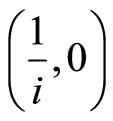 and radius
and radius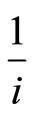 , where
, where , is a retract of
, is a retract of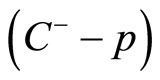 , then any circle
, then any circle 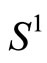 with center
with center 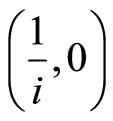 and radius
and radius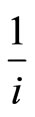 , were
, were , is a retract of
, is a retract of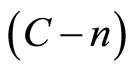 .
.
Theorem 13. Any retract of circle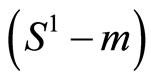 ,
, 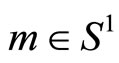 in
in 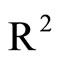 with center
with center 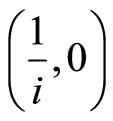 and radius
and radius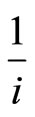 , where
, where
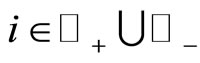 , is a retract of
, is a retract of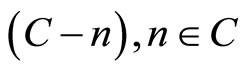 .
.
Proof. Let 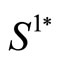 is a retract of
is a retract of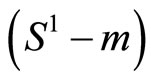 , then there is a continuous map
, then there is a continuous map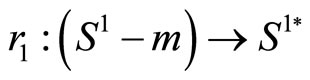 ,
, 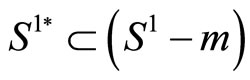 , where
, where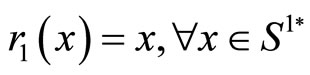 . Then the circle
. Then the circle 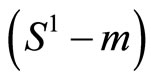 in
in 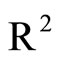 with center
with center 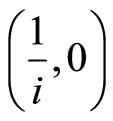 and radius
and radius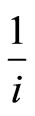 , where
, where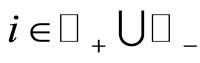 , is a retract of
, is a retract of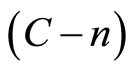 , then there is a continuous map
, then there is a continuous map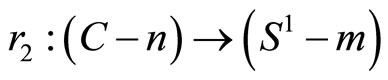 ,
,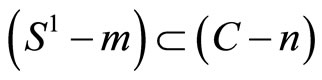 , where
, where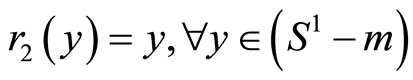 .Then,
.Then, 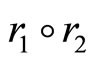 is a continuous map. Also,
is a continuous map. Also,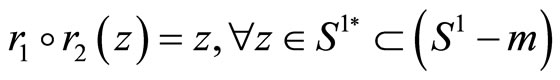 . Then any retract of circle
. Then any retract of circle 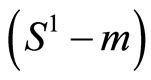 in with center
in with center 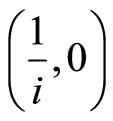 and radius
and radius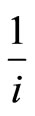 where
where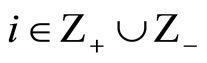 , is a retract of
, is a retract of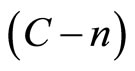 .
.
3. Conclusion
In this paper we achieved the approval of the important of the curves in the Euclidean space by using some geometrical transformations. The relations between folding, retractions, deformation retracts, limits of folding and limits of retractions of the curves in the Euclidean space are discussed. New types of minimial retractions on curves in the Euclidean space are deduced.
REFERENCES
- A. E. El-Ahmady, “The Variation of the Density Functions on Chaotic Spheres in Chaotic Space-Like Minkowski Space Time,” Chaos, Solitons and Fractals, Vol. 31, No. 5, 2007, pp. 1272-1278. doi:10.1016/j.chaos.2005.10.112
- A. E. El-Ahmady, “Folding of Fuzzy Hypertori and Their Retractions,” Proc. Math. Phys. Soc. Egypt, Vol. 85, No. 1, 2007, pp. 1-10.
- A. E. El-Ahmady, “Limits of Fuzzy Retractions of Fuzzy Hyperspheres and Their Foldings,” Tamkang Journal of Mathematics, Vol. 37, No. 1, 2006, pp. 47-55.
- A. E. El-Ahmady, “Retraction of Chaotic Black Hole,” The Journal of Fuzzy Mthematics, Vol. 19, No. 4, 2011, pp. 833-838.
- A. E. El-Ahmady, “Fuzzy Folding of Fuzzy Horocycle,” Circolo Matematico di Palermo Serie II, Tomo L III, 2004, pp. 443-450. doi:10.1007/BF02875737
- A. E. El-Ahmady, “Fuzzy Lobachevskian Space and Its Folding,” The Journal of Fuzzy Mathematics, Vol. 12, No. 2, 2004, pp. 609-614.
- A. E. El-Ahmady, “The Deformation Retract and Topological Folding of Buchdahi Space,” Periodica Mathematica Hungarica, Vol. 28, No. 1, 1994, pp. 19-30. doi:10.1007/BF01876366
- A. E. El-Ahmady and H. Rafat, “Retraction of Chaotic Ricci Space,” Chaos, Solutions and Fractals, Vol. 41, 2009, pp. 394-400. doi:10.1016/j.chaos.2008.01.010
- A. E. El-Ahmady and H. Rafat, “A Calculation of Geodesics in Chaotic Flat Space and Its Folding,” Chaos, Solutions and Fractals, Vol. 30, 2006, pp. 836-844. doi:10.1016/j.chaos.2005.05.033
- A. E. El-Ahmady and H. M. Shamara, “Fuzzy Deformation Retract of Fuzzy Horospheres,” Indian Journal of Pure and Applied Mathematics, Vol. 32, No. 10, 2001, pp. 1501-1506.
- A. E. El-Ahmady and A. El-Araby, “On Fuzzy Spheres in Fuzzy Minkowski Space,” Nuovo Cimento, Vol. 125B, 2010.
- M. Arkowitz, “Introduction to Homotopy Theory,” Springer-Village, New York, 2011.
- G. L. Naber, “Topology, Geometry and Gauge Fields,” Foundations, Springer, New York, 2011.
- G. L. Naber, “Topology, Geometry and Gauge Fields,” Interactions, Springer, New York, 2011.
- M. Reid and B. Szendroi, “Topology and Geometry,” Cambridge, New York, 2005. doi:10.1017/CBO9780511807510
- P. l. Shick, “Topology: Point-Set and Geometry,” Wiley, New York, 2007. doi:10.1002/9781118031582
- J. Strom, “Modern Classical Homotopy Theory,” American Mathematical Society, 2011.

