World Journal of Mechanics
Vol. 1 No. 2 (2011) , Article ID: 4673 , 7 pages DOI:10.4236/wjm.2011.12008
Attitude Stabilization Using Modified Rodrigues Parameters without Angular Velocity Measurements
Department of Mathematics, Faculty of Science, Mansoura University, Mansoura, Egypt
E-mail: aigohary@ksu.edu.sa, tawfikstm@yahoo.com
Received January 26, 2011; revised April 6, 2011; accepted April 7, 2011
Keywords: Attitude Control, Rotors with Frictions, Modified Rodrigues Parameters
ABSTRACT
The optimal stabilization of a rigid body motion without angular velocity measurements is considered with the help of three internal rotors that effected by internal frictions. In this paper, the orientation of the body will be described in terms of the Modified Rodrigues parameters (MRPs). The optimal control law which stabilizes asymptotically this motion and minimizes the require like-energy cost is obtained in terms of the MRPs. Numerical study and simulation are introduced.
1. Introduction
Most research into attitude motions of rigid bodies systems always has been and still remains one of the important problems of theoretical and applied mechanics. The controlling of a rigid body motion means how we can select the control law that ensures an asymptotic stability of this motion. Physically the control transfers the state of rigid body from an arbitrary initial state to the desired state. This control law is considered to be optimal if it minimizes a selecting performance index. This problem is considered one of the important problem in modern mechanics since the rigid body is a suitable mathematical and physical model for study the motion of satellite, aircraft, spacecraft and the like.
Many studies have derived the control laws in terms of the angular velocities of the rigid body and parameters attitude the orientation of the rigid body with respect to the inertial axes (El-Gohary, 2005a; Izzo and Pettazzi, 2007; Junfengy et al., 2000; Lovera and Astolfib, 2004; Tayebi and McGilvray, 2006; Tsiotras et al., 2001). The angular velocity measurement is noisy, that is it contains high-frequency and noises or random fluctuations. The controlling of a rigid body motion without angular velocity measurement using control torques is studied in (Akella, 2001; Costic et al., 2000; Lizarralde and Wen, 1996; Tayebi, 2006; Tsiotras, 1995). El-Gohary and Tawfik (2010) studied the optimal stabilization of a rotational motion of a rigid body using three rotors with internal friction moments. The control law which stabilizes asymptotically this motion is obtained in terms of Euler parameters. El-Gohary (2005b) studied the optimal stabilization of an equilibrium position using three rotors without inertial frictions. The control law which stabilizes asymptotically this position is obtained either in terms of the Cayley-Rodrigues parameters, or in terms of the MRPs. In this paper, the control law which stabilizes asymptotically a rotational motion of a rigid body in terms of the MRPs is derived. We will take into account the inertial frictions of the rotors. Moreover, a special case of the studied problem is obtained.
2. Equations of Motion
We consider the rotational motion of a rigid body carrying three symmetrical rotors attached to the principal axes of inertia of the body. The rotational motion of a rigid body about its centre of mass is described by the following equation:
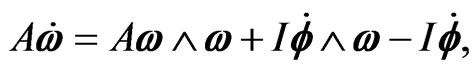 (1)
(1)
where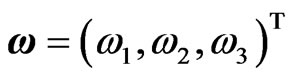 ,
,  , denote the angular velocity vector of the body the rotor angles of rotation vector referred to the principal axes of inertia of the body, A and I are the inertia matrices of the body and the rotors, respectively.
, denote the angular velocity vector of the body the rotor angles of rotation vector referred to the principal axes of inertia of the body, A and I are the inertia matrices of the body and the rotors, respectively.
The equation of the relative motion of the rotors with the inertial friction moments is:
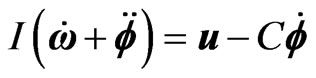 , (2)
, (2)
where 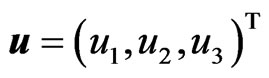 denote the control vector applied to the rotors and created by electric motors rigidly mounted on the body and
denote the control vector applied to the rotors and created by electric motors rigidly mounted on the body and 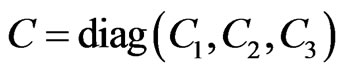 is diagonal friction coefficients matrix. The terms
is diagonal friction coefficients matrix. The terms 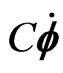 due to the rotors friction. Note that the friction coefficients depend upon various factors such as temperature, angular velocity and other factors. This friction is quasi-viscous.
due to the rotors friction. Note that the friction coefficients depend upon various factors such as temperature, angular velocity and other factors. This friction is quasi-viscous.
In this paper, the orientation of the body is described by using the MRPs. The kinematic equation in terms of the MRPs takes the form (Schaub and Junkins, 1996):
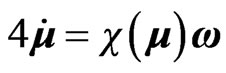 (3)
(3)
where
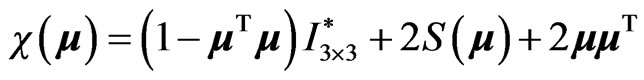 (4)
(4)
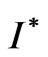 is the
is the  unit matrix. The matrix
unit matrix. The matrix 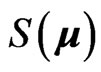 denotes the following skew symmetric matrix:
denotes the following skew symmetric matrix:
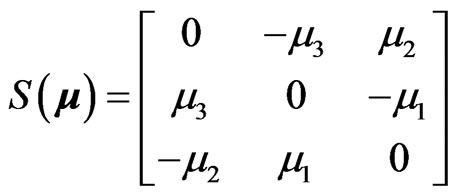 . (5)
. (5)
The direction cosines matrix of the inertial axes relative to the principal axes of inertia of the body in terms of the MRPs can be written in the form (Schaub and Junkins, 1996):
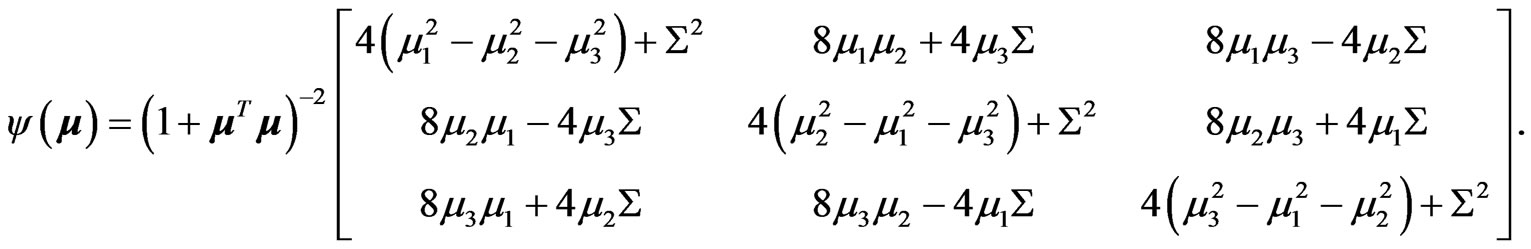 (6)
(6)
where
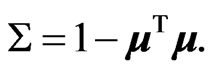
Modified Rodrigues parameters are three parameters which have the advantage over the Cayley-Rodrigues parameters that allow eigenaxis rotations greater than 180˚ but can’t be used to describe eigenaxis rotations of more than 360˚.
The system admits the first integrals
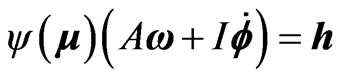 (7)
(7)
where 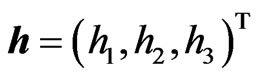 is the angular momentum vector of the whole system referring to the inertial axes can be regarded as constant.
is the angular momentum vector of the whole system referring to the inertial axes can be regarded as constant.
Solving Equation (7) with respect to 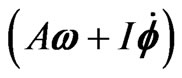 we obtain:
we obtain:
 (8)
(8)
The vectors 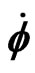 and
and 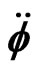 can be eliminated from Equations (1) by using Equations (8) and (2), we get
can be eliminated from Equations (1) by using Equations (8) and (2), we get
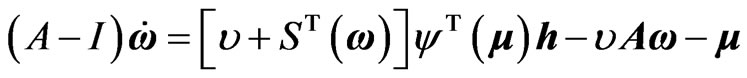 (9)
(9)
where 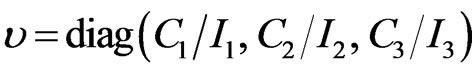 is positive diagonal matrix.
is positive diagonal matrix.
The system (9) and (3) admit the special solution
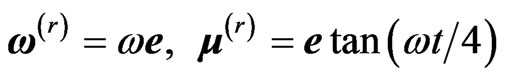 (10)
(10)
if the control vector take the values
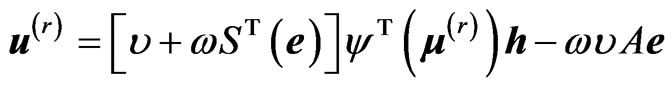 (11)
(11)
where 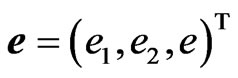 is the unit vector that the body rotates about it, referred to the principal axes of inertia of the body. The solution (10) represents a rotational motion of the body around an axis which is fixed in the body with a certain angular velocity
is the unit vector that the body rotates about it, referred to the principal axes of inertia of the body. The solution (10) represents a rotational motion of the body around an axis which is fixed in the body with a certain angular velocity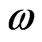 .
.
The optimal control law applied to the rotors which stabilizes asymptotically the rotational motion (10) of the rigid body can be determined only in terms of the MRPs and their estimates without angular velocity measurements. To derive these control law, we start by introducing the new variables which represent estimates attitude parameters. Assume that 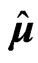 is an estimate of the kinematic attitude vector
is an estimate of the kinematic attitude vector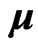 . Also we suppose that the kinematic attitude vector and its estimate satisfy the following auxiliary system of differential equations:
. Also we suppose that the kinematic attitude vector and its estimate satisfy the following auxiliary system of differential equations:
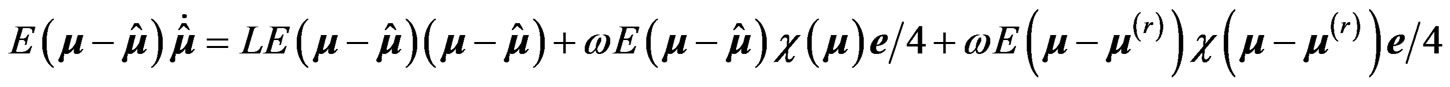 (12)
(12)
where 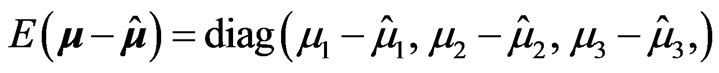
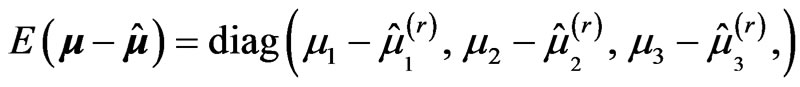 and
and
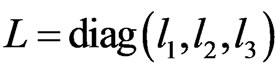 is positive diagonal matrix
is positive diagonal matrix 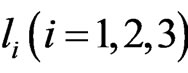
are called the stability constants.
This system is known as the auxiliary system. Using the kinematic Equation (3) the auxiliary system (12) can be written in the form:
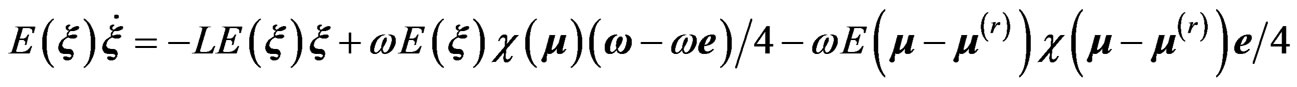 (13)
(13)
where
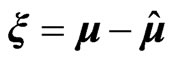 . (14)
. (14)
The problem is equivalent to find the optimal control law such that the rotational motion
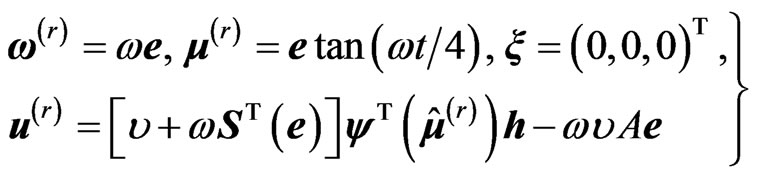 (15)
(15)
is asymptotically stable and minimize a selected performance.
To obtain the equations of the perturbed motion about the rotational motion (15) we introduce the following new variables
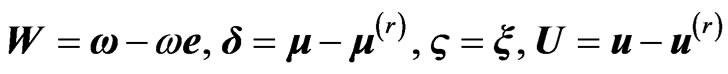 (16)
(16)
where 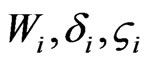 and
and 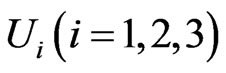 are the perturbation of the angular velocity of the body, MRPs, error attitude parameters and control moments about the rotational state (16) at respectively.
are the perturbation of the angular velocity of the body, MRPs, error attitude parameters and control moments about the rotational state (16) at respectively.
Substituting from (16) into (9), (3) and (13) we get the following systems:
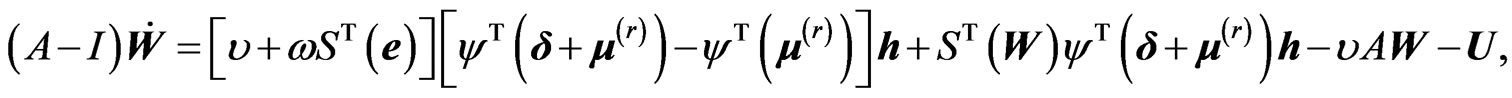 (17)
(17)
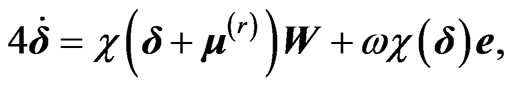 (18)
(18)
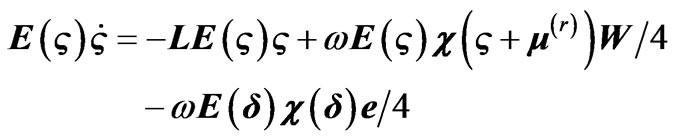 (19)
(19)
if 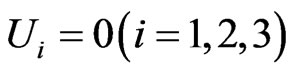 then the systems (17), (18) and (19) have an obvious unstable solution
then the systems (17), (18) and (19) have an obvious unstable solution
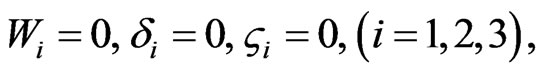 (20)
(20)
which must be stabilized. This stabilization can be achieved by using the control moments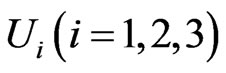 .
.
3. Optimal Stabilization Problem
In this section, the optimal control law U which stabilizes asymptotically the zero solution (20) and minimizes an integral performance index is determined on terms of the MRPs. The asymptotic stability of the zero solution (20) is derived. Moreover, a special case of the studied problem is obtained.
Theorem. The optimal control law
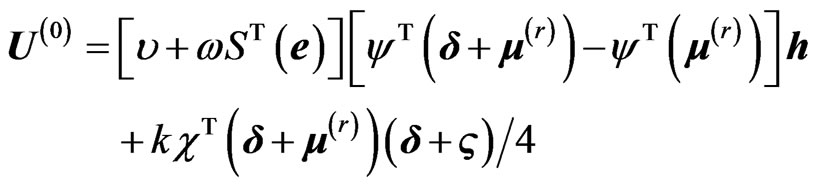 (21)
(21)
stabilizes asymptotically the zero solution (20) of the system described by Equations (17), (18) and (19) and minimizes the integral performance index:
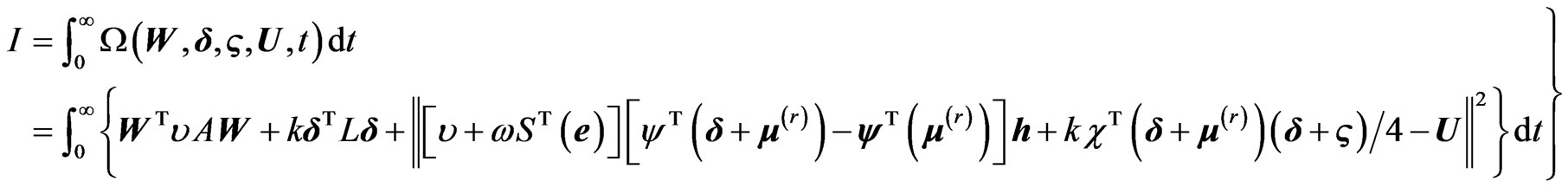 (22)
(22)
where k is positive control constant. It should be clear that the structure of optimal feedback control law depends upon the choice of the integral performance index.
Proof: Assume that, the optimal Liapunov function in the form
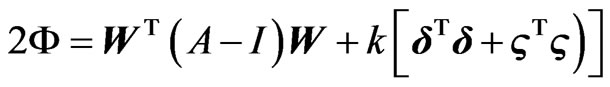 (23)
(23)
where 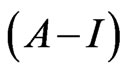 is positive diagonal matrix. This function is a positive definite with respect to stabilize variables since it consists of the sum of quadratic terms. Using the Krasovskii’s theorem (Krasovskii, 1966), we have
is positive diagonal matrix. This function is a positive definite with respect to stabilize variables since it consists of the sum of quadratic terms. Using the Krasovskii’s theorem (Krasovskii, 1966), we have
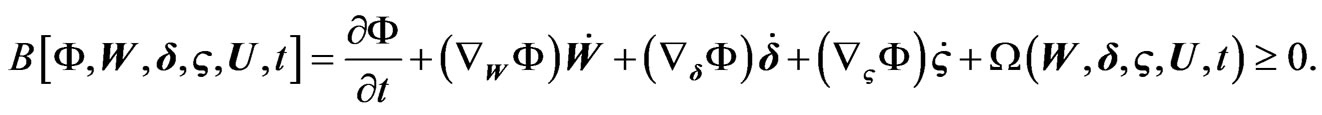 (24)
(24)
Using the conditions of optimality and assuming that
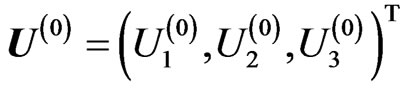 is the optimal control vector which stabilizes asymptotically the zero solution (20), the function
is the optimal control vector which stabilizes asymptotically the zero solution (20), the function 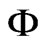 must satisfy the following partial differential equation:
must satisfy the following partial differential equation:
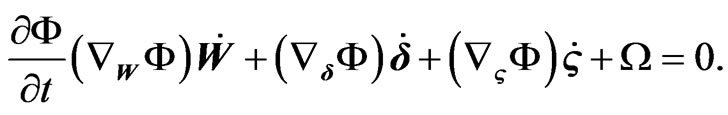 (25)
(25)
Substituting the function (17), (18), (19), (22) and (23) into the partial differential Equation (25), we get
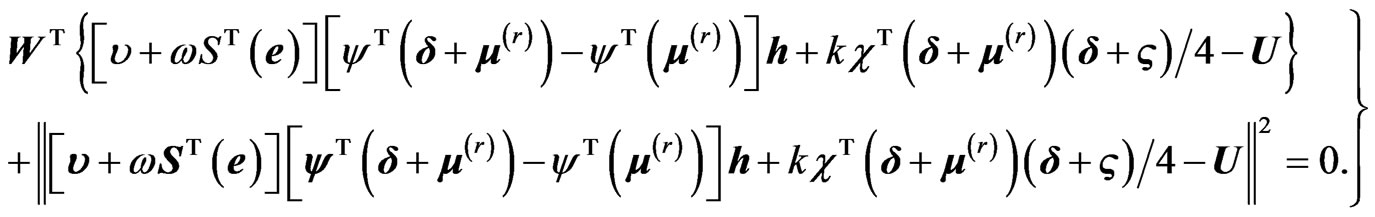 (26)
(26)
Thus, the optimal control vector 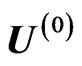 satisfies the Equation (26) are given by (21). Obviously, the present control law depend upon the kinematic attitude parameters and the friction coefficients. Moreover this control law do not require a knowledge of the rigid body inertial moments.
satisfies the Equation (26) are given by (21). Obviously, the present control law depend upon the kinematic attitude parameters and the friction coefficients. Moreover this control law do not require a knowledge of the rigid body inertial moments.
Now we will prove that the zero solution (20) is asymptotically stable under the control law (21). The total time derivative of the optimal Liapunov function (23) using (17), (18), (19) and taking into consideration the optimal control law (21) takes the form
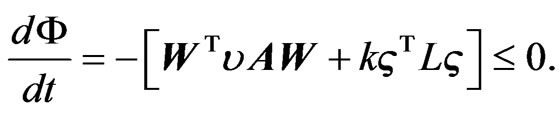 (27)
(27)
The function (23) is a positive definite with respect to the angular velocities of the body, the kinematic attitude parameters and the error attitude parameters. Furthermore, the total derivative of this function as given by (27) is a negative semi-definite function (constant sign function) only. Thus, under the optimal control law (21), the zero solution (20) is only stable in the Liapunov sense, but not necessarily asymptotic stable.
To prove the asymptotic stability of the zero solution (20) we consider the following function:
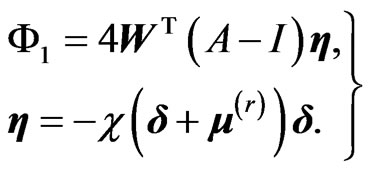 (28)
(28)
Using Equations (17) and (21) on the set (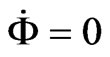 ,
, 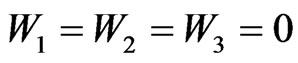 ,
,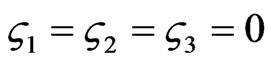 ) the derivative of the function (28) is given by
) the derivative of the function (28) is given by
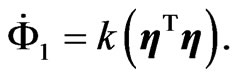 (29)
(29)
The function 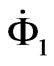 is a positive definite function. Thus by using theorem (1.2) in (Matrosov, 1962), the rotational motion (15) is asymptotically stable in the Liapunov sense.
is a positive definite function. Thus by using theorem (1.2) in (Matrosov, 1962), the rotational motion (15) is asymptotically stable in the Liapunov sense.
Similarly when neglected the friction of the rotors system i.e. 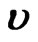 is zero matrix. Thus,
is zero matrix. Thus, 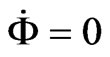 only if
only if 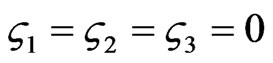 and
and 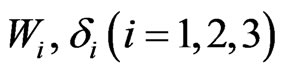 begin arbitrary. But in this case one can find that
begin arbitrary. But in this case one can find that 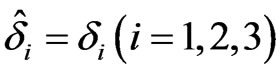 which leads to the kinematic attitude parameters
which leads to the kinematic attitude parameters 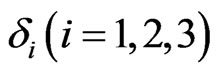 are constants. In this case the Equations (18) and (19) reduce to
are constants. In this case the Equations (18) and (19) reduce to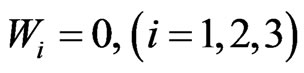 . Thus,
. Thus,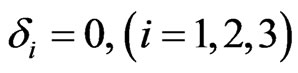 .
.
From the above analysis, we conclude that 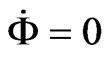 if and only if
if and only if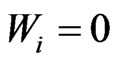 ,
, 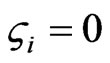 ,
, 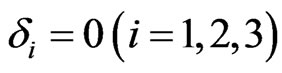 Thus the zero solution (20) is asymptotically stable in the Liapunov sense. □
Thus the zero solution (20) is asymptotically stable in the Liapunov sense. □
The equilibrium position of the rigid body which occurs when the principal axes of inertia of the body coincide with the inertial axes can be obtained as a special case of the studied problem by setting 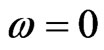 in Equation (15):
in Equation (15):
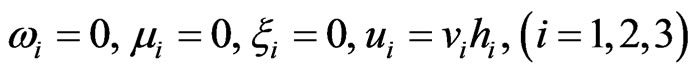 (30)
(30)
The optimal control law which stabilizes asymptotically this position can be obtained from Equation (21) by setting 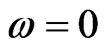 and
and 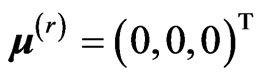
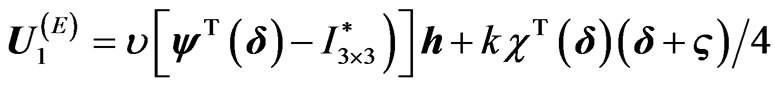 . (31)
. (31)
When the rotors system move without friction, that is, the coefficients of the friction 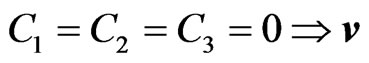 is zero matrix, the equilibrium position (30) reduce to
is zero matrix, the equilibrium position (30) reduce to
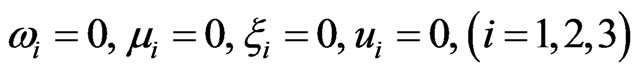 . (32)
. (32)
The optimal control law which stabilizes asymptotically this position can be obtained from Equation (31) by setting v zero matrix
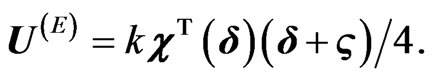 (33)
(33)
This result, as a special case of the obtained results for the considered problem, agrees with the result deduced by (El-Gohary, 2005b). This shows that the present method is more general than the method used by (El-Gohary, 2005b).
We compare the optimal control law (21) and the previous control law publication on this topic (Akella, 2001; Costic et al., 2000; Lizarralde and Wen, 1996; Tayebi, 2006; Tsiotras, 1995). The control law (21) stabilizes asymptotically the general rotational motion of a rigid body not an equilibrium position. Moreover, this control law applied to the internal rotors that effected by frictions not control torques.
4. Numerical Examples
The results of this paper are more useful for the rigid body application such as satellite, spacecraft, aircraft and others. In this section, we study the effect of the control constants and stability constants of the angular velocity, MRPs, error attitude parameters and control moments. We adopt the numerical values of a rigid body rotating around the third axis of inertia of the body with an angular velocity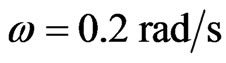 , the inertial moments of a rigid body A1, A2, A3, the inertial moments of the stabilizer rotors system I1, I2, I3, the constants of the angular momentum of the system h1, h2, h3, initial values of the angular velocities of the rigid body
, the inertial moments of a rigid body A1, A2, A3, the inertial moments of the stabilizer rotors system I1, I2, I3, the constants of the angular momentum of the system h1, h2, h3, initial values of the angular velocities of the rigid body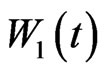 ,
, 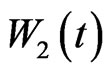 ,
, 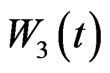 , MRPs
, MRPs 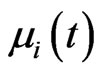 and their estimates
and their estimates 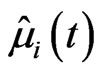 as follows:
as follows:
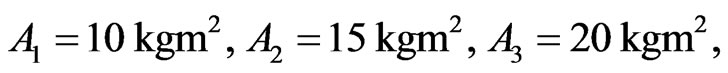
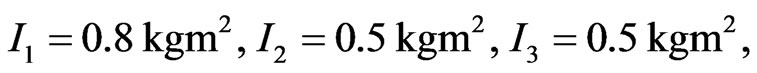
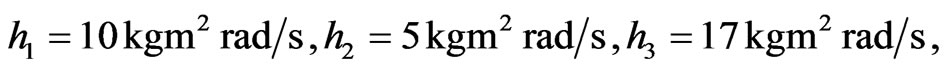
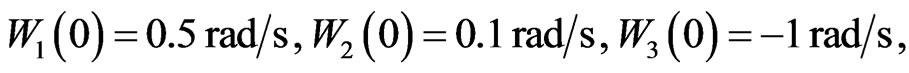
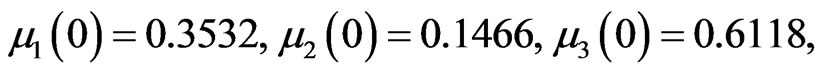
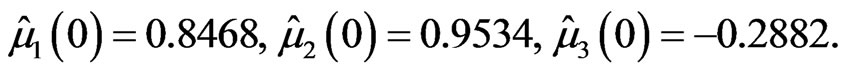 (34)
(34)
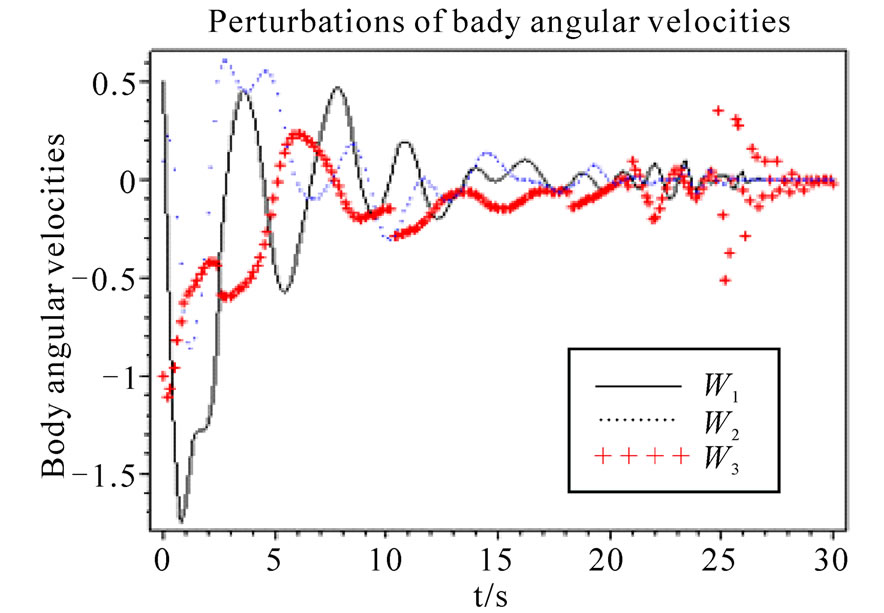
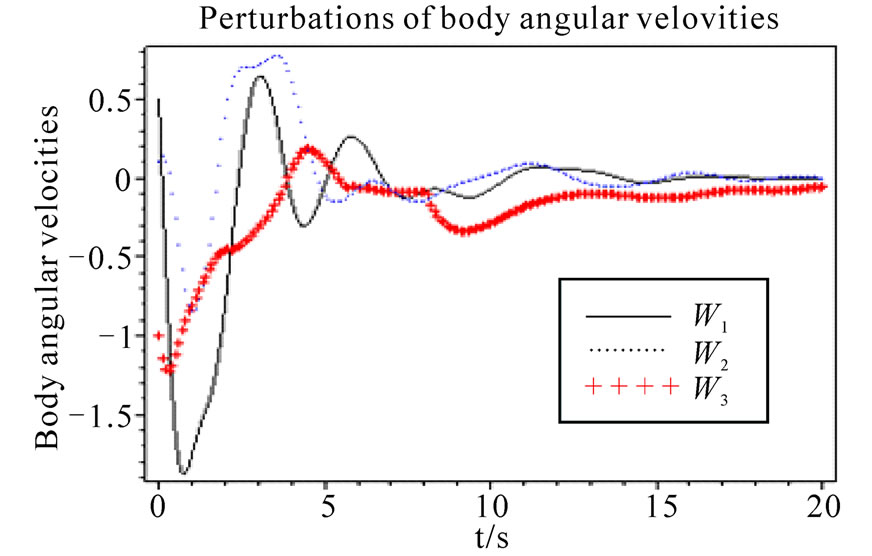
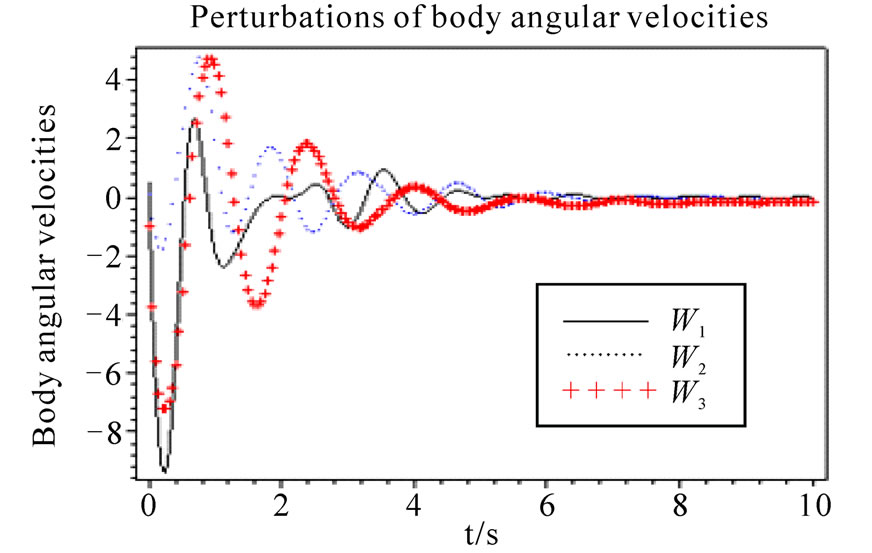
The results are shown in Figures 1(a)-3(d). Figures 1(a)-1(d) show the time histories of state variables, error attitude parameters and control moments for the values 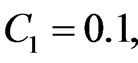
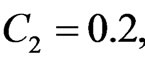
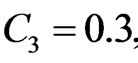
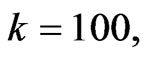
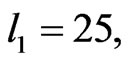
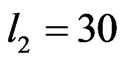 ,
,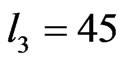 . Figures 2(a)-2(d) show the time histories of state variables, error attitude parameters and control moments for the values
. Figures 2(a)-2(d) show the time histories of state variables, error attitude parameters and control moments for the values ,
, 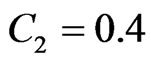 ,
, 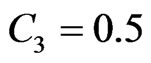 ,
, 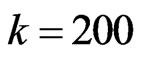 ,
,  ,
, 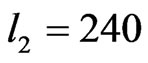 ,
, 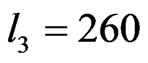 , Figures 3(a)- 3(d) show the time histories of state variables, error attitude parameters and control moments for the values
, Figures 3(a)- 3(d) show the time histories of state variables, error attitude parameters and control moments for the values 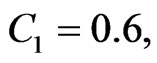
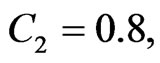
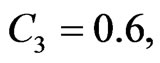
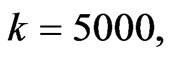
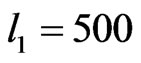 ,
, 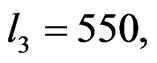
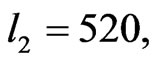 Recall that increasing the values of the friction coefficients of the rotors system, control constants and stability constants has the effect of decreasing the time for the control process, but these figures have the same behavior.
Recall that increasing the values of the friction coefficients of the rotors system, control constants and stability constants has the effect of decreasing the time for the control process, but these figures have the same behavior.
Based on the above numerical simulation study we conclude that, for the same initial state the control process depends on the friction coefficients of the rotors system, control constants and stability constants. The necessary time for the control process depend upon the friction coefficients, control constants and stability constants and becomes more effective for the large values of these control.
5. Conclusions
In this paper, the control law (21) which stabilizes asymptotically the rotational motion (15) of a rigid body in terms of the MRPs is derived. The inertial frictions of the rotors are taken into account. Global asymptotic stability is shown by applying Matrosov theorem. The equilibrium position (30) of the rigid body which occurs when the principal axes of inertia of the body coincide with the inertial axes is studied to be asymptotically stable as a spe-
 (a)
(a)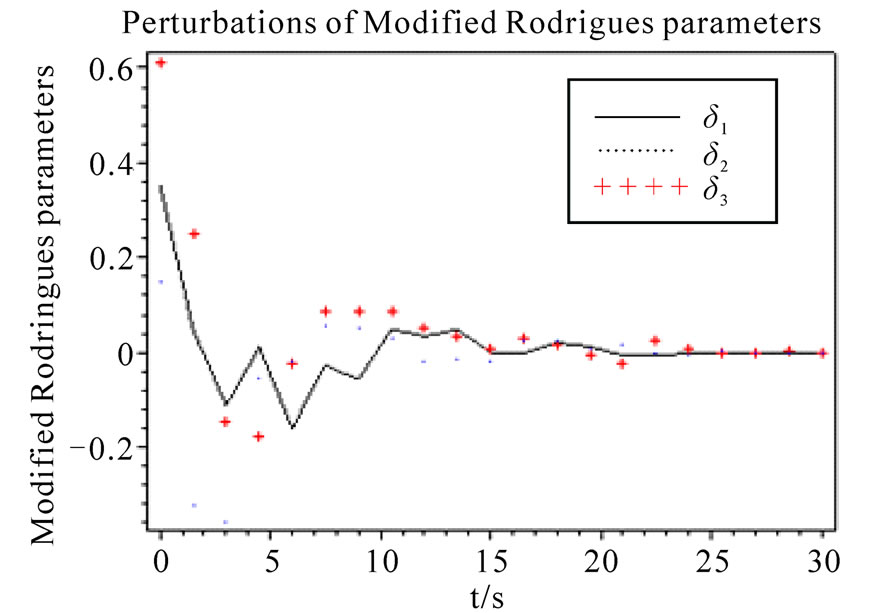 (b)
(b) (c)
(c)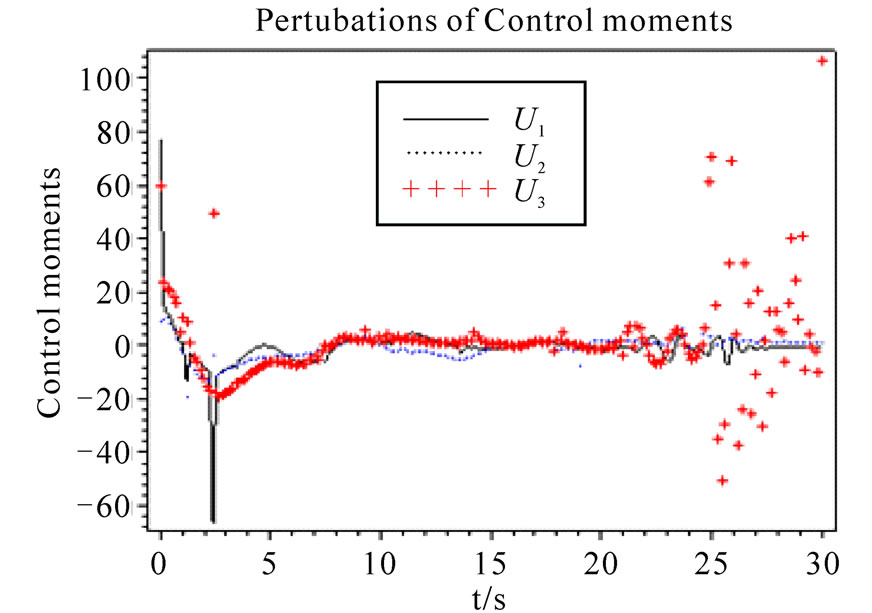 (d)
(d)
Figure 1. Show the above data at 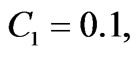
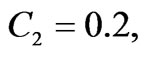
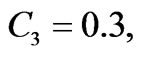
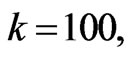
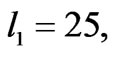
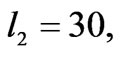
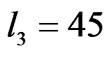 .
.
 (a)
(a)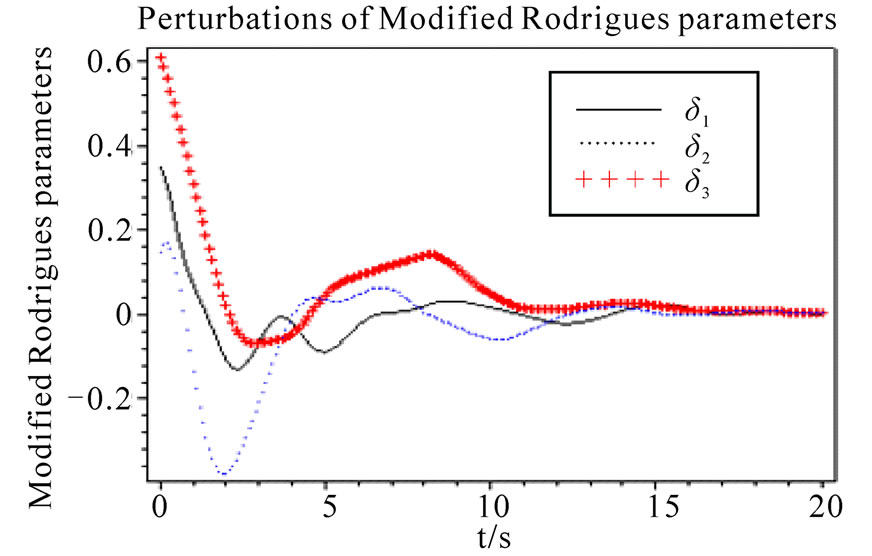 (b)
(b)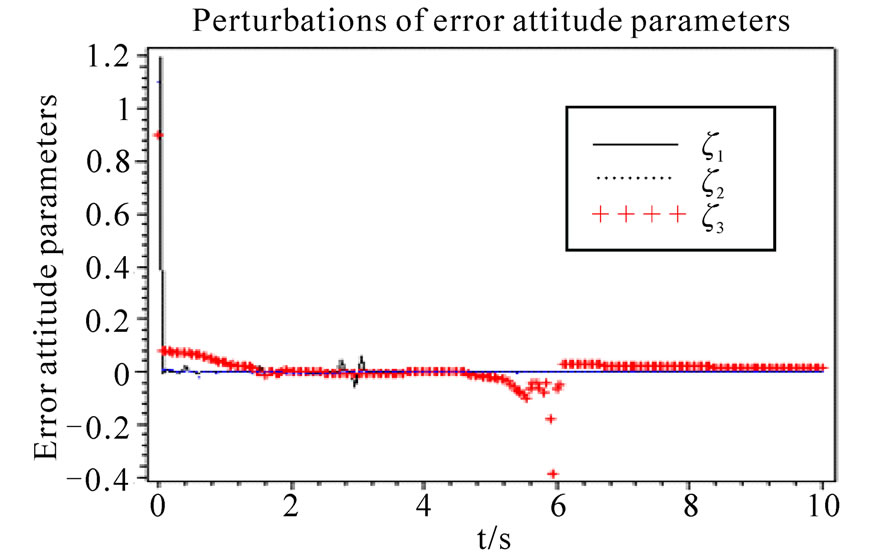 (c)
(c)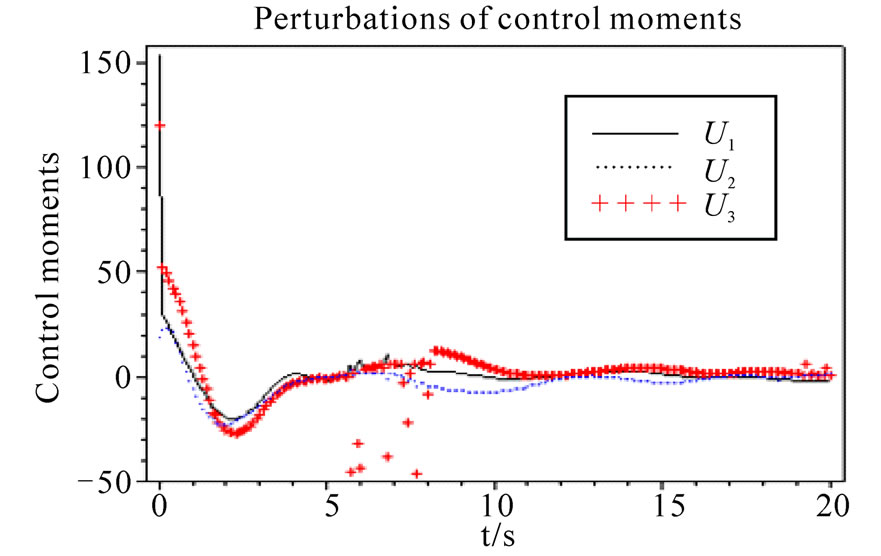 (d)
(d)
Figure 2. Show the above data at 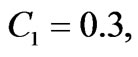
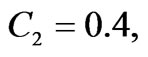
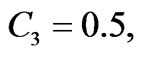
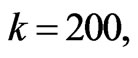
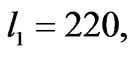
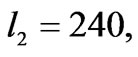
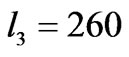 .
.
 (a)
(a)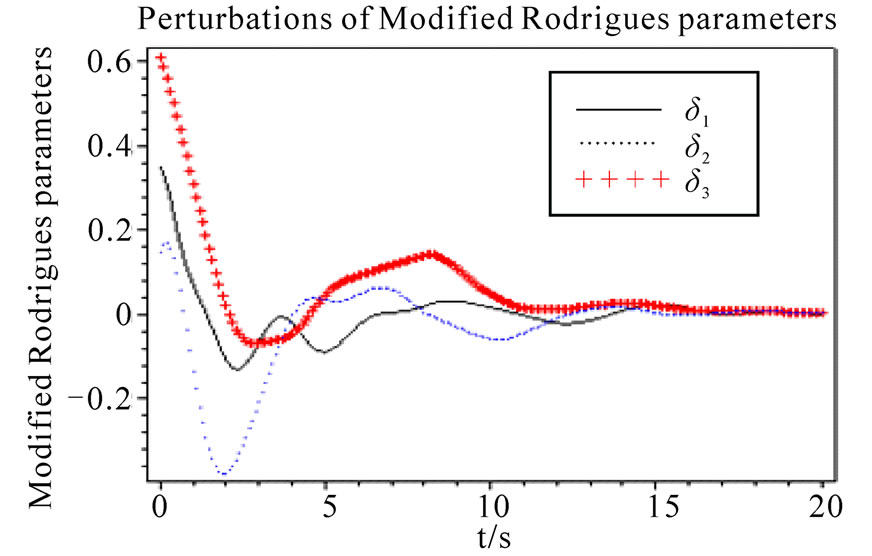 (b)
(b)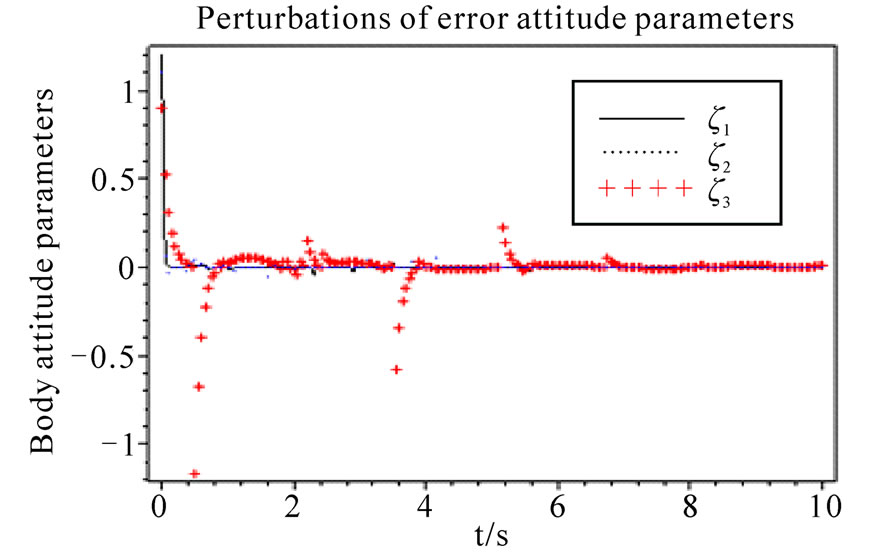 (c)
(c)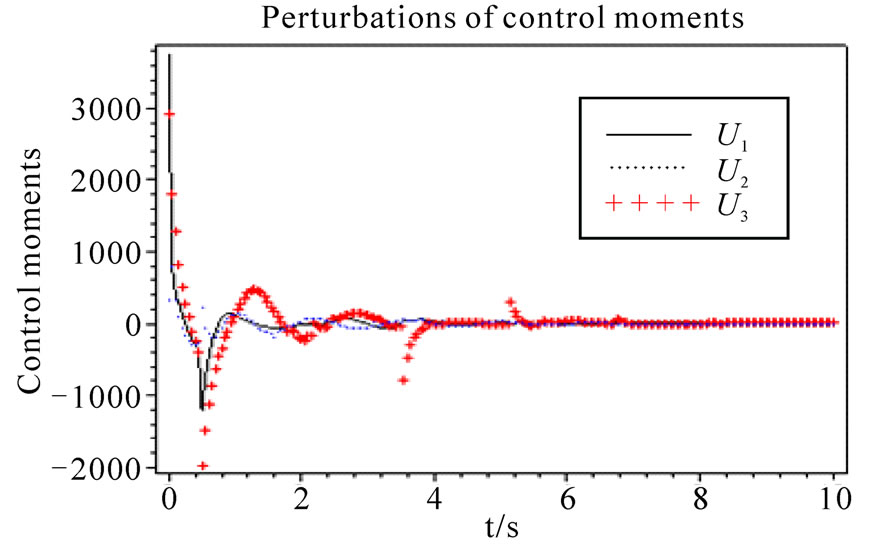 (d)
(d)
Figure 3. Show the above data at 
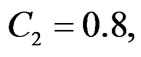
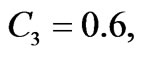
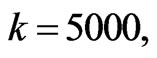
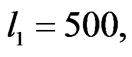
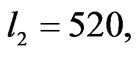
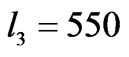 .
.
cial case of the studied problem. Numerical examples of the results are presented.
REFERENCES
- M. R. Akella, “Rigid Body Attitude Tracking without Angular Velocity Feed Back,” System & Control Letters, Vol. 42, No. 4, 2001, pp. 321-326. doi:10.1016/S0167-6911(00)00102-X
- B. T. Costic, D. M. Dawson, M. S. De Queiroz and V. Kapila, “A Quaternion-Based Adaptive Attitude Tracking Controller without Velocity Measurements,” Proceedings of the 39th IEEE Conference on Decision and Control, Sydeny, Vol. 3, 12-15 December 2000, pp. 2424- 2429.
- A. El-Gohary, “Optimal Control of the Rotational Motion of a Rigid Body Using Euler Parameters With the Help of Rotor System,” European Journal of Mechanics-A/Solids, Vol. 24, No. 1, 2005, pp. 111-125. doi:10.1016/j.euromechsol.2004.10.007
- A. El-Gohary, “New Optimal Control Law for Attitude of a Rigid Body without Angular Velocity Measurements,” Chaos, Solitons & Fractals, Vol. 25, No. 3, 2005, pp. 557-571. doi:10.1016/j.chaos.2004.12.008
- A. I. El-Gohary and T. S. Tawfik, “Optimal Control of a Rigid Body Motion Using Euler Parameters Without Angular Velocity Measurements,” Mechanics Research Communications, Vol. 37, No. 3, 2010, pp. 354-359. doi:10.1016/j.mechrescom.2010.02.004
- H. Schaub and J. L. Junkins, “Stereographic Orientation Parameters For Attitude Dynamics: A Generalization of the Rodrigues Parameters,” Journal of the Astronautical Sciences, Vol. 44, No. 1, 1996, pp. 1-19.
- G. R. Izzo and L. Pettazzi, “Command Shaping for a Flexible Satellite Platform Controlled by Advanced FlyWheels Systems,” Acta Astronautica, Vol. 60, No. 10-11, 2007, pp. 820-827.
- J. Li, M. Xu, Z. L.Wang and S. M. Wang, “Minimum-Torque Earth off-Nadir Pointing Control of Gravity-Gradient Stabilized Small Satellites,” Tsinghua Science and Technology, Vol. 5, No. 1, 2000, pp. 31-33.
- N. Krasovskii, “Problem of Stabilization of Controlled Motion,” In: I. G. Malkin, Ed., The Theory of Motion Stability, Nauka, Moscow, 1966, pp. 475-514.
- F. Lizarralde and J. T. Wen, “Attitude Control without Angular Velocity Measurement: A Passivity Approach,” IEEE transactions on Automatic Control, Vol. 41, No. 3, 1996, pp. 468-472. doi:10.1109/9.486654
- M. Lovera and A. Astolfib, “Spacecraft Attitude Control Using Magnetic Actuators,” Automatica, Vol. 40, No. 8, 2004, pp. 1405-1414. doi:10.1016/j.automatica.2004.02.022
- V. M. Matrosov, “On Stability of Motion,” Journal of Applied Mathematics and Mechanics, Vol. 26, No. 5, 1962, pp. 992-1002.
- A. Tayebi, “Unit Quaternion Observer Based Attitude Stabilization of a Rigid Spacecraft Without Velocity Measurement,” Proceedings of the 45th IEEE Conference on Decision and Control, San Diego, 13-15 December 2006, pp. 1557-1561.
- A. Tayebi and S. McGilvray, “Attitude Stabilization of a VTOL Quadrotor Aircraft,” IEEE Transactions on Control Systems Technology, Vol. 14, No. 3, 2006, pp. 562- 571. doi:10.1109/TCST.2006.872519
- P. Tsiotras, “A Passivity Approach to Attitude Stabilization Using Nonredundant Kinematic Parameterizations,” Proceedings of the 34th Conference on Decision and Control, New Orleans, 13-15 December 1995, pp. 13-15.
- P. Tsiotras, H. Shen and C. Hall, “Satellite Attitude Control and Power Tracking With Energy/Momentum Wheels,” Journal of Guidance, Control and Dynamics, Vol. 24, No. 1, 2001, pp. 23-34. doi:10.2514/2.4705

