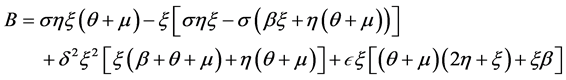Open Journal of Modelling and Simulation
Vol.05 No.01(2017), Article ID:73434,13 pages
10.4236/ojmsi.2017.51006
A Simulation Model for Sexual and Vectorial Transmission of Zika Virus (ZIKV)
Oscar Arias Manrique, Dalia M. Muñoz Pizza, Anibal Muñoz Loaiza, Julian A. Olarte García, Carlos A. Abello Muñoz, Steven Raigosa Osorio, Angie Johanna Osorio, Hans Meyer Contreras, John F. Arredondo Montoya
Grupo de Modelación Matemática en Epidemiología (GMME), Facultad de Educación, Universidad del Quindío, Quindío, Colombia

Copyright © 2017 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: September 7, 2016; Accepted: January 9, 2017; Published: January 12, 2017
ABSTRACT
Nowadays the Zika virus (ZIKV) has been one of the most studied vector-borne diseases due to the considerable outbreaks that have generated around the world as well as due to the new transmission mechanisms and health complications originated. According to statistics of the INS-Colombia for July 2016, 68% of the population infected by ZIKV (confirmed cases) are pregnant women. Furthermore, the Quindío department belongs to the states with more than 50% of the total infected persons being pregnant women. Taking into account those characteristics, a theoretical model is proposed and analyzed to describe the population dynamics considering the sexual and vectorial transmission of ZIKV, with special emphasis in the consequences of the non-vectorial transmission in the population. The obtained results with simulations through the beta parameter indicate that the probability of sexual transmission between susceptible women and infected men points out the importance of campaigns to inculcate prevention measures for the safe sexual relationships between ZIKV infected population.
Keywords:
ZIKV, Model, Dynamics, Sexual Transmission, Vectorial Transmission

1. Introduction
Recently the concern caused by the Zika virus (ZIKV) has increased, and this infection has been turned in a public health problem in several countries of the American continent. This is mainly due to the relationship with diseases such as: microcephaly and Guillain Barre Syndrome [1] [2] [3] and the discovery of new non-vectorial transmission mechanisms, among them, the vertical, sexual and by blood transfusion ways [4] .
It is well known that ZIKV is a disease transmitted by the bite of an infected mosquito (principally the Aedes aegypti gender). There are secondary ways of transmission, although the knowledge of them is limited. The virus has been detected in human saliva, semen and urine [5] [6] [7] . Also there exist documented cases referent to sexual transmission with clinical evidence and serological tests, in Senegal [8] , and afterwards in Tahiti and United Kingdom [9] [10] . More recently evidence has been found in France [6] , Italia [11] and United States [12] . Therefore, it is very important to give special attention to the non-vectorial transmission since it is being considered a sexually transmitted disease [13] , with repercussions in the outbreaks size and geographical expansion of the virus.
Particularly in Colombia, according to the statistics in the “Boletín Epidemiológico Semanal del Instituto Nacional de Salud” [14] , 68% of ZIKV infected population corresponds to pregnant women. Figure 1 highlighted the territorial extensions of the
Figure 1. Geospatial distribution of the percentage of pregnant women that are infected by ZIKV in Colombia (upper part). This image has been processed with ArcG is for office software with statistics of the INS [14] . Percentages of the infected pregnant women in each state (lower part).
country with the highest quantity of infected pregnant women (confirmed cases). We divide those percentages into three main groups, each group (sixteen states) with more than fifty percent of infected expectant women (58% - 87%). Then, it is especially important to study and analyze the sexual transmission in those states. Since the Quindío department is one of the most affected by this issue, we will consider it in our investigation.
Taking into account these facts, we propose a model in R3 which considers the vectorial transmission as well as the sexual transmission of the ZIKV. The basic reproduction number and the stability analysis of the system are obtained. After that, the simulations using the Maple software are carried out to observe the behavior of the epidemic threshold as function of several parameters, the behavior of the sensitivity indexes as well as the transmission probability by sexual way in the human populations. Unlike the model proposed by Gao et al. [15] , where it is developed a deterministic model of the vectorial and sexual transmission with statistics for Brazil, Colombia and El Salvador. The parameters used in that model include the population divided in symptomatic and asymptomatic populations on the other hand, our model contemplates the population divided in women and men infected by ZIKV, using the average temperature of Armenia-Quindío, Colombia. Also we focus in parameters which contemplate the susceptible women group, due to the possible complications that arise from the transmission to this fraction of the population [16] . The results of both models support the use of campaigns of sexual protection in the intimate relationships of ZIKV infected persons.
It is important to model this dynamics to offer useful information to the public health care institutions in the country, for the application of preventive measures to the sexual transmission of this virus, this is due to the potential propagation of this disease and the collateral effects that may cause. Note that in Colombia almost all the states present high percentages pregnant women infected by ZIKV (see Figure 1), emphasizing the relevance of the present study.
2. The Model
It is proposed a simulation model, in R3, based in non-linear ordinary differential equations following the Sir Ronald Ross formalism to describe the dynamics of the sexual and vectorial ZIKV transmission, accounting the following assumptions: closed populations, variations in time of the ZIKV infected pregnant women population, infected men populations, and the virus-carrier A. aegypti mosquitoes, and the constant recovery rate of infected women and infected men. Also, it is considered the ZIKV transmission from the infected men to the susceptible women, assuming promiscuity.
The variables of the model are:
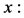 fraction of women infected by ZIKV at time
fraction of women infected by ZIKV at time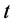 ,
,
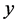 : fraction of men infected by ZIKV at time
: fraction of men infected by ZIKV at time 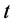 and
and
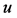 : fraction of virus-carrier mosquitoes at time
: fraction of virus-carrier mosquitoes at time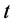 .
.
The parameters of the model are shown in Table 1.
The differential equations system to describe the infectious process is (Figure 2):
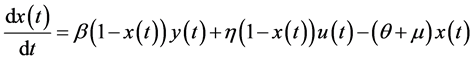 (1)
(1)
Table 1. Parameters of the model.
Figure 2. The infectious process diagram.
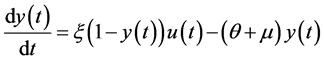 (2)
(2)
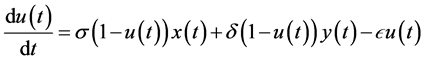 (3)
(3)
where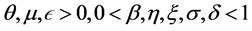 , with initial conditions
, with initial conditions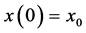 ,
, 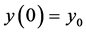 and
and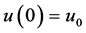 .
.
And, the region where the system trajectories have epidemiological sense is,
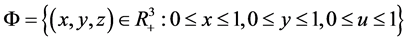
3. Stability Analysis
The stability analysis begins calculating the equilibrium points by solving the algebraic
system, which is associated to the non-linear differential equations, 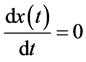 ,
, 




Obtaining 

Substituting (7) in (4), it is found:
Simplifying the previous equation, we have,

Replacing (7) and (8) in (6), we obtain:

where

For



Simplifying Equation (10), it is derived the following equation,
where,
Due to A > 0 and if we assume that at least one of the B, C and D is less than zero, it guarantees a change of sign in the coefficients of the quadratic equation. Following the signs rule of Descartes it is obtained a positive and real root (

In the linearization process for the non-lineal equations system (1)-(3), is calculated the Jacobian matrix at the generic equilibrium point

Evaluating the Jacobian matrix in the free of infection equilibrium point, we obtain:

So, the characteristic equation 

where,
Under the Routh-Hurwitz criterion, it should be accomplished that 


At the equilibrium point



where, 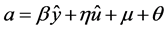


Solving by the first column and cofactors, we have,
with
Applying the Routh-Hurwitz criterion, this equation has 3 roots with negative real part if the inequalities are met 


4. Basic Reproduction Number
The so called epidemic threshold



Then doing 






so,
where;



For a best understanding of the basic reproduction number

The terms 



The term 

5. Numerical Analysis of Stability
For an average annual temperature in Armenia-Quindío, Colombia of 19.5˚C, were estimated the transmission probabilities 


where






The local stability analysis is carried out using the estimated parameters. We start with the calculation of the free of infection and prevalence equilibrium points, which are determined doing the differentiation of the system (1)-(3) equal to zero, and solving the non-linear algebraic system for each demographic variable.
Using the MAPLE software the equilibrium points are determined.
・ For
・ For
・ For
With information in the Table 2 and the calculated equilibrium points, we obtain the local sensitivity analysis. Also, using the Jacobian matrix the results of Table 3 are obtained.
6. Local Sensitivity Analysis
Another threshold is obtained using the local sensitivity analysis, this one represents a relative measure of the change in one variable when the value of one parameter changes
Table 2. Estimated parameters for the model.
Table 3. Local stability analysis for each
[20] [21] [22] . The sensitivity index for
where, 

The results of the sensitivity analysis of 
In Figure 3, it is presented the behavior of the basic reproduction number respecting
Table 4.Sensitivity index of 
Figure 3. Behavior of the threshold (

Figure 4. Behavior of the sensitivity indexes for
to the most sensitive parameters.
In Figure 4, it is shown the behavior for each sensitivity index as function of each parameter.
7. Simulations
The simulations of system (1)-(3) were obtained using the values of the parameters as reported in Table 1 and the MAPLE software.
From the results of the simulations, which are depicted in Figure 5 and Figure 6, it is show that the dynamic system, which describes the dynamics of the infectious process, stabilizes quickly in the first ten days for different values of the ZIKV transmission probability from the infected men to susceptible women, for small, medium and high values of probability. This is to analyze the behavior of the populations, see Table 3. If we consider some preventive measures to decrease the probability to 0.3, the stability level by sexual transmission decreases in almost an 84%.
Figure 6 displayed the behavior of the human and mosquitoes populations for a probability of sexual transmission of 0.8. These ones stabilize in the first ten days of the infectious process.
Figure 5. Behavior of the fraction of infected by ZIKV for different
Figure 6. Human and mosquitoes population behavior for
8. Conclusions
It is possible to see that upon beta increases, the infected women population increases in a time t. Then, it is extremely important to apply prevention measures in the sexual relationships of the infected population, and then help to stop the virus propagation at large scale. Together with these measures, it is expected to have a decrease in the diseases derived by this virus, as congenital microcephaly and Guillian-Barre syndrome.
Figure 6 shows the behavior of the infected women’s fraction, infected men’s fraction and virus-carrier mosquitoes fraction with a parameter 
The basic reproduction number is inversely proportional to the recovery rate of the infected persons and the mortality rate of the mosquitoes. That is to say, it is not appropriate to decrease the values of these parameters by treatment and control of the mosquito.
Actually, the Ross-Macdonald formalism is very important to model Vector-Host diseases as Malaria, Dengue, Chagas, Chikungunya and ZIKV, etc. In future works it is important to carry out the goblal stability analysis of the model and add the horizontal transmission in the man as well as the model without considering Sir. Ronald Ross formalism.
Acknowledgements
AML thanks to Grupo de Modelación Matemática en Epidemiología (GMME), Facultad de Educación, Vicerrectoría de investigaciones, Universidad del Quindío-Colombia.
Cite this paper
Manrique, O.A., Pizza, D.M.M., Loaiza, A.M., García, J.A.O., Muñoz, C.A.A., Osorio, S.R., Osorio, A.J., Contreras, H.M. and Montoya, J.F.A. (2017) A Simulation Model for Sexual and Vec- torial Transmission of Zika Virus (ZIKV). Open Journal of Modelling and Simulation, 5, 70-82. http://dx.doi.org/10.4236/ojmsi.2017.51006
References
- 1. Heymann, D.L., Hodgson, A., Sall, A.A., Freedman, D.O., Staples, J.E., Althabe, F., Baruah, K., Mahmud, G., Kandun, N., Vasconcelos, P.F.C., Bino, S. and Menon, K.U. (2016) Virus Zika y microcefalia: Por qué esta situación es un ESPII. The Lancet, 387, 719-721.
https://doi.org/10.1016/S0140-6736(16)00320-2 - 2. Rodríguez-Morales, A.J. (2016) Zika y microcefalia en América Latina: ¿una amenaza emergente para los viajeros embarazadas? Viajes Med Infect Dis., 14, 5-6.
- 3. Musso, D. and Baud, D. (2016) Zika Virus: Time to Move from Case Reports to Case Control. The Lancet Infectious Diseases, 16, 620-621.
https://doi.org/10.1016/S1473-3099(16)00096-7 - 4. European Centre for Disease Prevention and Control (2015) Zika Virus Epidemic in the Americas: Potential Association with Microcephaly and Guillain-Barre Syndrome. European Centre for Disease Prevention and Control, Stockholm.
- 5. Musso, D., Roche, C., Nhan, T.-X., Robin, E., Teissier, A. and Cao-Lormeau, V.-M. (2015) Detection of Zika Virus in Saliva. Journal of Clinical Virology, 68, 53-55.
https://doi.org/10.1016/j.jcv.2015.04.021 - 6. Mansuy, J.M., Dutertre, M., Mengelle, C., Fourcade, C., Marchou, B., Delobel, P., et al. (2016) Zika Virus: High Infectious Viral Load in Semen, a New Sexually Transmitted Pathogen? The Lancet Infectious Diseases, 16, 405.
https://doi.org/10.1016/S1473-3099(16)00138-9 - 7. Gourinat, A.C., O’Connor, O., Calvez, E., Goarant, C. and Dupont-Rouzeyrol, M. (2015) Detection of Zika Virus in Urine. Emerging Infectious Diseases, 21, 84-86.
https://doi.org/10.3201/eid2101.140894 - 8. Foy, B.D., Kobylinski, K.C., Chilson Foy, J.L., Blitvich, B.J., Travassos da Rosa, A., Haddow, A.D., Lanciotti, R.S. and Tesh, R.B. (2011) Probable Non-Vector-Borne Transmission of Zika Virus, Colorado, USA. Emerging Infectious Diseases, 17, 880-882.
https://doi.org/10.3201/eid1705.101939 - 9. Musso, D., Roche, C., Robin, E., Nhan, T., Teissier, A. and Cao-Lormeau, V.M. (2015) Potential Sexual Transmission of Zika Virus. Emerging Infectious Diseases, 21, 359–361.
https://doi.org/10.3201/eid2102.141363 - 10. Atkinson, B., et al. (2016) Detection of Zika Virus in Semen. Emerging Infectious Diseases,, 22, 940.
https://doi.org/10.3201/eid2205.160107 - 11. Venturi, G., et al. (2016) An Autochthonous Case of Zika Due to Possible Sexual Transmission, Florence, Italy 2014. Eurosurveillance, 21, Article Number: 30148.
https://doi.org/10.2807/1560-7917.ES.2016.21.8.30148 - 12. Armstrong, P., Hennessey, M., Adams, M., et al. (2016) Travel-Associated Zika Virus Disease Cases among US Residents. Morbidity and Mortality Weekly Report, 65, 286-289.
https://doi.org/10.15585/mmwr.mm6511e1 - 13. Patiño-Barbosa, A.M., Medina, I., Gil-Restrepo, A.F. and Rodriguez-Morales, A.J. (2015) Zika: Another Sexually Transmitted Infection? Sexually Transmitted Infections, 91, 359.
https://doi.org/10.1136/sextrans-2015-052189 - 14. Instituto Nacional de Salud. Dirección de Vigilancia y Análisis del Riesgo en Salud Pública.
- 15. Gao, D., Lou, Y., He, D., Porco, T.C., Kuang, Y., Chowell, G. and Ruan, S. (2016) Prevention and Control of Zika as a Mosquito-Borne and Sexually Transmitted Disease: A Mathematical Modeling Analysis. Scientific Reports, 6, Article Number: 28070.
- 16. Pizza, D.M.M., Loaiza, A.M., Montoya, J.F.A., Manrique, O.A., Sossa, V.A., Muñoz, C.A. and Enríquez, M.A. (2016) A Model for the Risk of Microcephaly Induced by the Zika Virus (ZIKV). Open Journal of Modelling and Simulation, 4, 109-117.
https://doi.org/10.4236/ojmsi.2016.43010 - 17. Van, P. and Watmough, J. (2002) Reproduction Numbers and Sub-Threshold Endemic Equilibria for Compartmental Models of Disease Transmission. Mathematical Biosciences, 180, 29-48.
https://doi.org/10.1016/S0025-5564(02)00108-6 - 18. Holland, J. (2007) Notes on R0. Department of Anthropological Sciences, Stanford University, Stanford.
- 19. Diekmann, O., Heesterbeek, J. and Roberts, M. (2010) The Construction of Next-Generation Matrices for Compartmental Epidemic Models. Journal of the Royal Society Interface, 7, 873-885.
https://doi.org/10.1098/rsif.2009.0386 - 20. Rodrigues, H., Monteiro, M. and Torres, D. (2013) Sensitivity Analysis in a Dengue Epidemiological Model. Conference Papers in Mathematics, 2013, Article ID: 721406.
- 21. Chitnis, N., Hyman, J. and Cushing, J. (2008) Determining Important Parameters in the Spread of Malaria through the Sensitivity Analysis of a Mathematical Model. Bulletin of Mathematical Biology, 70, 1272-1296.
https://doi.org/10.1007/s11538-008-9299-0 - 22. Hamby, D. (1994) A Review of Techniques for Parameters Sensitivity Analysis of Environmental Models. Environmental Monitoring and Assessment, 32, 135-154.
https://doi.org/10.1007/BF00547132