ABSTRACT

In the present paper, we prove some fixed point theorems of Hegedus contraction in some types of distance spaces, dislocated metric space, left dislocated metric space, right dislocated metric space and dislocated quasi-metric metric space which are generalized metrics spaces where self-distances are not necessarily zero.

1. Introduction

Fixed point theorems on metric spaces and generalized types of metric spaces have applications in the area of logic programming semantics (see, e.g., [1-3]). Dislocated metrics are also known as metric domains in the context of domain theory [4]. The slightly less general notation of partial metrics was also studied in [5].

The definition of a distance space was introduced by P. Waszkiewick [6,7].

Definition 1.1 [6,7]. Let X be a set. A distance on X is a map d:X × X → [0, ∞). A pair (X, d) is called a distance space.

Definition 1.2. Let (X, d) be a distance space. Consider the following conditions:

Following Waszkiewick [4], let X be a set. A distance on X is a map d:X × X → [0, ∞). A pair (X, d) is called a distance space. If d satisfies the following conditions:

   

   

   

   

   

   

   

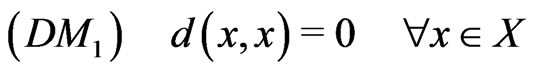
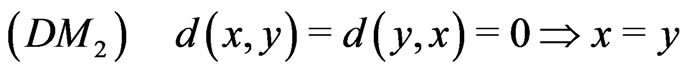
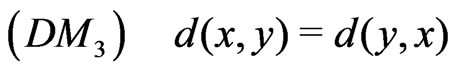
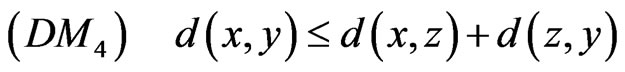
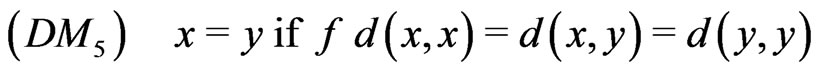
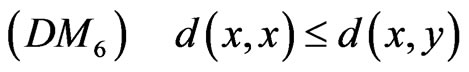
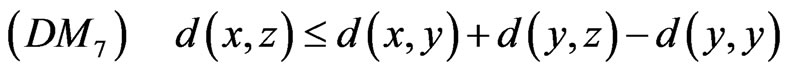
for all. If d satisfies (DM₁) – (DM₄), then it is called a metric on X. If it satisfies conditions (DM₂) – (DM₄) it is called a dislocated metric [1,2] (or simply d-metric) on X. If it satisfies conditions (DM₁), (DM₂), and (DM₄), It is called a quasi-metric (or simply q-metric) on X [8]. If it satisfies conditions (DM₂)) and (DM₄), It is called a dislocated quasi-metric (or simply dq-metric) on X [1,2]. If it satisfies conditions (DM₃), (DM₅), (DM₆) and (DM₇), It is called a partial metric on X [5].

It is clear that any partial metric is a d-metric and any d-metric is dq-metric.

Hitzler and Seda gave an alternative proof of Matthews’s Theorem [4] as follows.

Theorem 1.1 [1]. Let (X, d) be a complete d-metric space and let f:X → X be a Banach contraction function. Then f has a unique fixed point.

The plan of this paper is as follows. In Section 2, we introduce some definitions in distance spaces. In Section 3, we establish some fixed point theorems in some types of distance space. In Section 4, we establish some fixed point theorems in some types of dislocated metric space.

2. Some Basic Concepts and Results in Distance Spaces

In the following we proceed with definitions which are needed for our results in a distance space. As it turns out, these notions can be carried over directly from conventional metrics.

Definition 2.1. A sequence (x_n) in a distance space (X, d) ld-converges (resp. rd-converges, d-converges) to 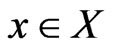if lim_{n → ∞}d(x_n, x) = 0 (resp. lim_{n → ∞}d(x_n, x) = 0, lim_{n → ∞}d(x_n, x) = lim_{n → ∞}d(x, x_n) = 0). In this case, x is called the ld-limit (resp. rd-limit, d-limit) of the sequence (x_n).

Is is obvious that a d-limit of a sequence (x_n) is ldlimit and rd-limit but the converse may not be true.

Definition 2.2. A sequence (x_n) in a distance space (X,d) is called Cauchy if.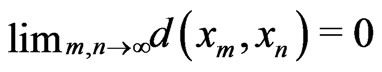

Definition 2.3. A distance space (X, d) is called ldcomplete (resp. rd-complete, d-complete) if every Cauchy sequence in it is ld-convergent (resp. rd-convergent, d-convergent).

Definition 2.4. Let (X, d₁) and (Y, d₂) be distance spaces. Then f:X → Y is sequentially l-continuous if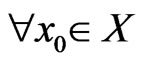, , 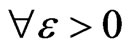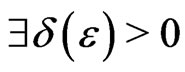such that

.

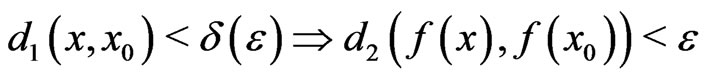Definition 2.5. Let (X, d₁) and (Y, d₂) be distance spaces. Then f:X → Y is sequentially r-continuous if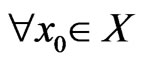, , 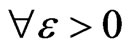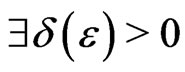such that

.

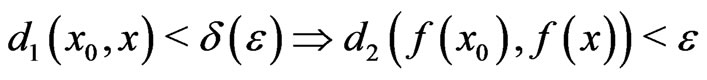We state the following lemmas without proof.

Lemma 2.1. Let (X, d₁) and (Y, d₂) be distance spaces. A function f:X → Y is sequentially ld-continuous (resp. rd-continuous, d-continuous) if and only if for each sequence (x_n) which is ld-convergent (resp. rd-convergent, d-convergent) to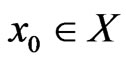, the sequence (f(x_n)) is ldconvergent (resp. rd-convergent, d-convergent) to.

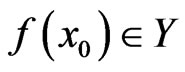Lemma 2.2. Let (X, d₁) and (Y, d₂) be distance spaces. Then f:X → Y is sequentially d-continuous if f is sequentially ld-continuous and sequentially rd-continuous.

The following counterexample illustrates the reverse of Lemma 2.2 need not be true.

Counterexample 2.1. Let d:N × N → [0, ∞) be defined by:

d(x, y) = 2, 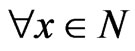, , 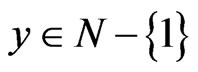d(1, 1) = 2.

Let f:N × N defined by f(x) = x, 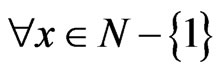, f(1) = 2. Since (x_n) (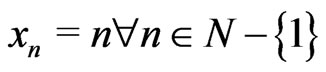) converges to 1 but (f(x_n) does not converges to f(1), then f is not sequentially l-continuous. But f is sequentially d-continuous.

Now, we give the following lemma without proof.

Lemma 2.4. Every subsequence of ld-convergent (resp. rd-convergent, d-convergent) sequence to x₀ is ld-convergent (resp. rd-convergent, d-convergent) to x₀.

It is obvious that the converse of Lemma 2.4 may not be true.

Definition 2.6. Let (X, d) be a distance space. Let N denote the set of positive integers and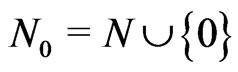. For any set 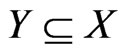the diameter of the set Y is defined by 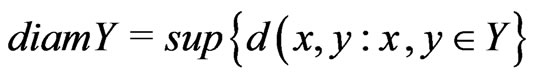and

is the f-orbit of x. The point 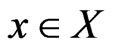is called regular (f-regular) if.

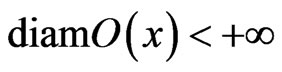A function f:(X, d) → (X, d) is called a Hegedus contraction [9] if there exists 0 ≤ λ ≤ 1 such that

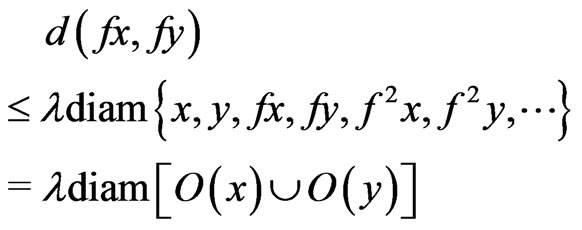where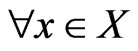, x is f-regular.

Lemma 2.2. Let (X, d) be a distance space. If f:(X, d) → (X, d) is a Hegedus contraction function, then for any 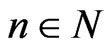and,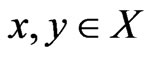

.

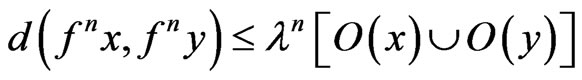Proof. Since

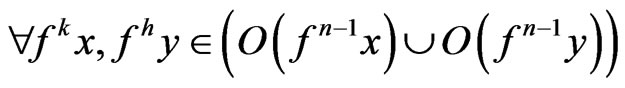
where k ≥ n – 1, h ≥ n – 1,

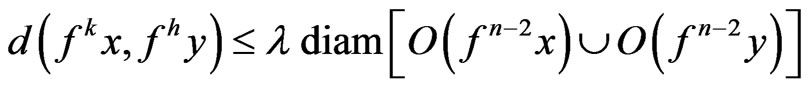then

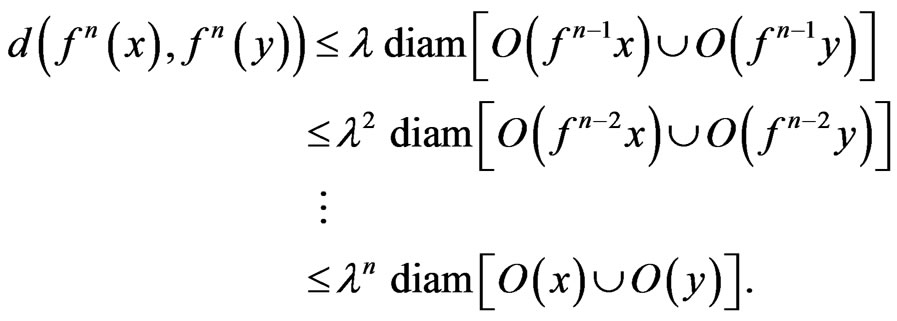
3. Fixed Point Theorems in Distance Spaces

In this section, we introduce some fixed point theorems in distance space.

Definition 3.1. A distance space (X, d) is called an ld-Hausdorff (resp. rd-Hausdorff, d-Hausdorff) space iff the ld-limt (resp. rd-limt, d-limt) of ld-convergent (resp. rd-convergent, d-convergent) sequence is unique. Such that,.

Lemma 3.1. Let (X, d) be a distance space such that,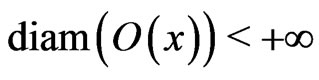. If a function f:X → X is a Hegedus contraction, then (fⁿ(x₀) is a Cauchy sequence for each.

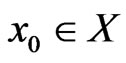Proof. Choose any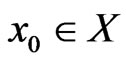, for any integer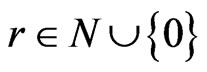. By lemma 2.2,

.

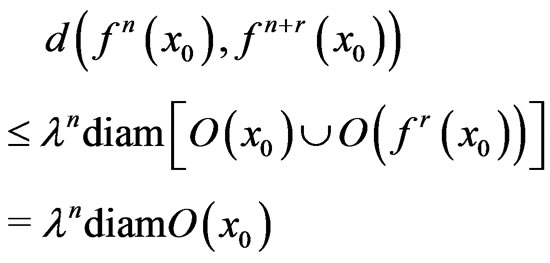The last term tends to zero as n tends to infinity. Also, we proceed similarly as above and obtain

.

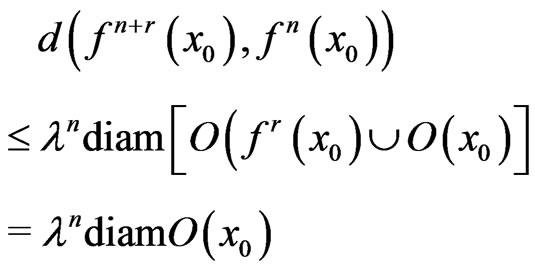The last term tends to zero as n tends to infinity. Thus, (fⁿ(x₀) is a Cauchy sequence.

Theorem 3.1. Let (X, d) be a ld-Hausdorff ld-complete distance space and a function f:X → X be a ld-continuous Hegedus contraction mapping such that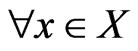, x is f-regular. Then f has a fixed point.

Proof. From Lemma 3.1, (fⁿ(x₀) is a Cauchy sequence. Since (X, d) is ld-complete distance space, then (fⁿ(x₀) is ld-convergent, say to. From the ld-continuity of the mapping f, (f^{n + 1}(x₀)) ld-converges to f(x). From Lemma 2.1, (f^{n + 1}(x₀) ld-converges to x. Since (X, d) is ld-Hausdorff, then f(x) = x.

In a similar manner of Theorem 3.1, we can prove the following theorems.

Theorem 3.2. Let (X, d) be a rd-Hausdorff rd-complete distance space and a function f:X → X be a rd-continuous Hegedus contraction mapping such that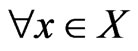, x is f-regular. Then f has a fixed point.

Theorem 3.3. Let (X, d) be a d-Hausdorff d-complete distance space and a function f:X → X be a d-continuous Hegedus contraction mapping such that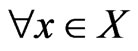, x is f-regular. Then f has a fixed point.

4. Fixed Point Theorems in Types of Dislocated Metric Spaces

In this section, we introduce the concept of left dislocated metric spaces and right dislocated metric spaces. Also, we state and prove some fixed point theorems in these spaces. Furthermore, we prove fixed point theorem in dislocated quasi-metric spaces and in dislocated metric spaces.

Definition 4.1. A left dislocated metric (simply ld-metric) is a distance d on a set X satisfying the following axioms, for all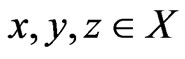(LD₁) if then x = y;

(LD₂).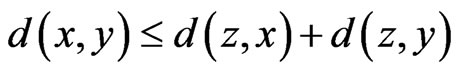

Lemma 4.1. ld-limits in ld-metric spaces are unique.

Proof. Let x and y be ld-limits of the sequence (x_n). By property (LD₂), it follows that

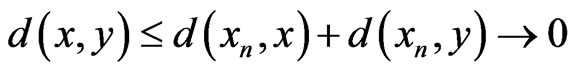as.

Hence, d(x, y) = 0. In a similar way, one can deduce that d(y, x) = 0. So, we obtain from Property (LD₁) that x = y.

Theorem 4.1. Let (X, d) be a ld-complete ld-metric space and a function f:X → X be ld-continuous Hegedus contraction function such that, x is f-regular. Then f has a fixed point.

Proof. Existence. From Lema 3.1, (fⁿ(x₀)) is a Cauchy sequence. Since (X, d) is ld-complete ld-metric then (fⁿ(x₀)) ld-convergent, say to x, f is ld-continuous and so from Lemmas 2.1 and 4.1,

.

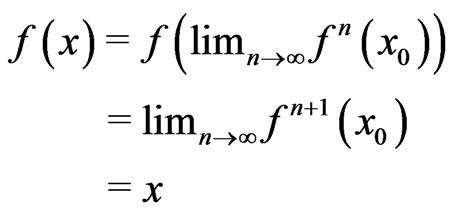Uniqueness. Suppose that there are two fixed points x and y. Then f(x) = x, f(y) = y and

If max{d(x, y), d(y, x), d(x, x), d(y, y)} is d(x, y) or d(y, x) or d(x, x) or d(y, y), then one can deduce that d(x, y) = d(y, x) = d(x, x) = d(y, y) = 0. Hence from (LD₁), x = y.

Definition 4.2. A right dislocated metric (simply rd-metric) is a distance d on a set X satisfying the following axioms, for all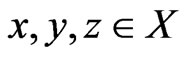(RD₁) if 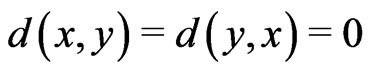then x = y;

(RD₂).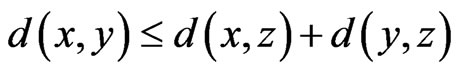

In a similar proof of Lemmas 4.1, we give the following lemmas:

Lemma 4.2. rd-limits in rd-metric spaces are unique.

Lemma 4.3. d-limits in dq-metric spaces are unique.

Lemma 4.4. d-limits in d-metric spaces are unique.

In a similar manner of Theorem 4.1, one can have the following theorems.

Theorem 4.2. Let (X, d) be a rd-complete rd-metric space and a function f:X → X be rd-continuous Hegedus contraction function such that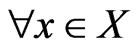, x is f-regular. Then f has a fixed point.

Theorem 4.3. Let (X, d) be a d-complete dq-metric space and a function f:X → X be d-continuous Hegedus contraction function such that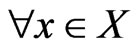, x is f-regular. Then f has a fixed point.

Theorem 4.4. Let (X, d) be a d-complete d-metric space and a function f:X → X be d-continuous Hegedus contraction function such that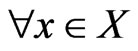, x is f-regular. Then f has a fixed point.

REFERENCES