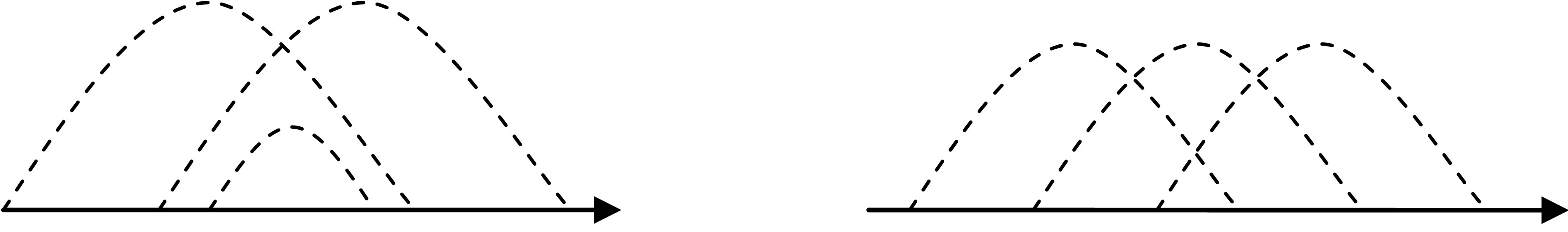American Journal of Analytical Chemistry
Vol.4 No.9(2013), Article ID:36897,7 pages DOI:10.4236/ajac.2013.49058
Theory of Triple Magnetopolarons in Quantum-Dimensional Nanostructures
1Samarkand branch of Tashkent Information Technology University, Samarkand, Uzbekistan
2Samarkand State Universiy, Samarkand, Uzbekistan
Email: eshkuvat@samdu.uz, eshquvat@mail.ru
Copyright © 2013 Barat Eshpulatov, Eshkuvat Arzikulov. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Received April 6, 2013; revised May 7, 2013; accepted June 10, 2013
Keywords: Triple Magnetopolarons; Quantum-Dimensional Nanostucrures; Electron; Phonons; Electron-Phonon Spectrum; Magnetooptical Absorption; Resonant Magnetic Field
ABSTRACT
A theory of triple magnetopolarons in an isolated quantum well in a strong magnetic field was developed. We study the behavior of the magnetooptical absorption peaks corresponding to the transitions of an electron at the Landau level with quantum numbers n ≥ 2. For n = 2 at the point of equality of cyclotron frequency and the frequency of optical phonon (LO), there is a cross of three terms of the electron-phonon system (the electron at the Landau level n = 2, the electron at n = 1, and the optical phonon and electron at n = 0 and two phonons), considered as a function of the cyclotron frequency. Interaction with phonons takes off the degeneracy of the terms and leads to three disjoint branches of the electron-phonon spectrum. The theory predicts that in the resonant magnetic field, the peak of magnetooptical absorption splits into three peaks, the intensity and position of which are dependent in a complex way on the magnitude of the magnetic field and the constant of the electron-phonon coupling.
1. Introduction
When the following condition is valid:
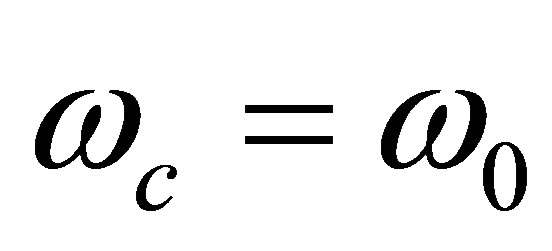 (1)
(1)
(where 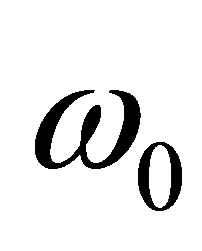 is maxima frequency of longitudinal optical phonon (OP),
is maxima frequency of longitudinal optical phonon (OP), 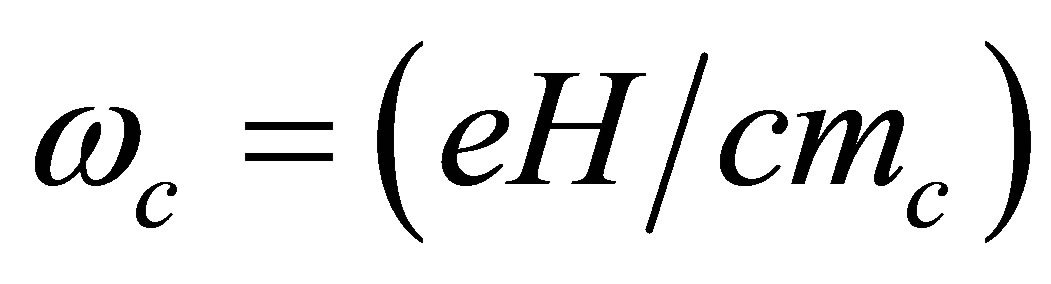 is the cyclotron frequency, е is the electron’s charge, Н is the magnetic field tension, с is the velocity of light in the vacuum, тс is the effective mass of electron), the magnetopolaron states are formed in semiconductors. This effect is called the effect of Johnson-Larsen [1,2] or magnetopolaron resonance.
is the cyclotron frequency, е is the electron’s charge, Н is the magnetic field tension, с is the velocity of light in the vacuum, тс is the effective mass of electron), the magnetopolaron states are formed in semiconductors. This effect is called the effect of Johnson-Larsen [1,2] or magnetopolaron resonance.
When the magnetic field satisfies the condition (1), there is a resonant relation between the levels with different Landau quantum numbers n (Figure 1). The electronphonon interaction leads to taking off the degeneracy in points of the level’s intercept which is manifested in magneto-optical phenomena. Magnetopolaron state was first discovered in a bulk InSb as the interband absorption of light [4,5].
Korovin and Pavlov showed [3] that in case of a bulk semiconductor, the magnetopolaron splitting is proportional to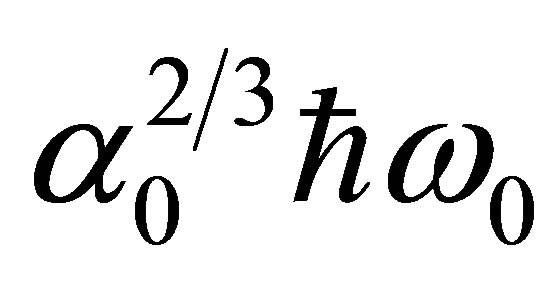 , where
, where  is the dimensionless con-
is the dimensionless con-

Figure 1. Schematic spectrum of electron 1—Landau level n = 2, 2—level n = 1 and optical phonon, 3—level n = 0 and two optical phonons. Dotted lines show the splitting of terms,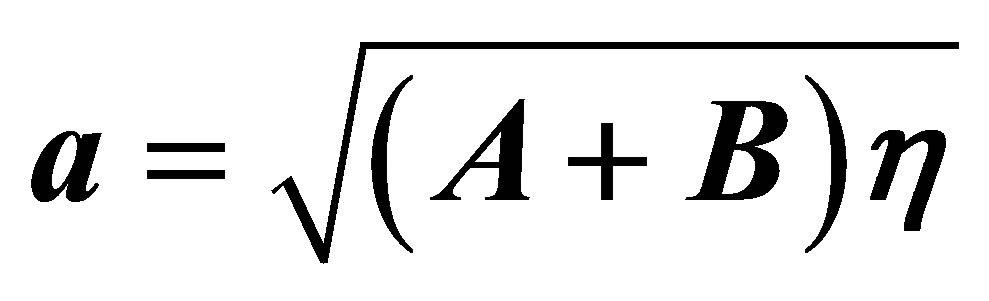 ;
;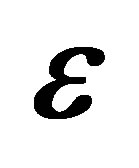 —term’s energy, 1' and 3'—upper and lower branches of the spectrum. Points of resonance
—term’s energy, 1' and 3'—upper and lower branches of the spectrum. Points of resonance 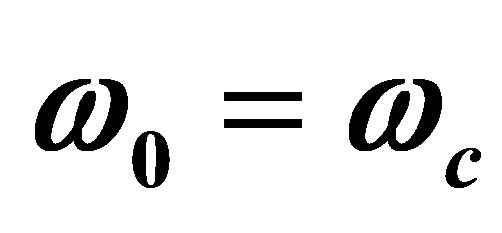 and break of the upper branch
and break of the upper branch 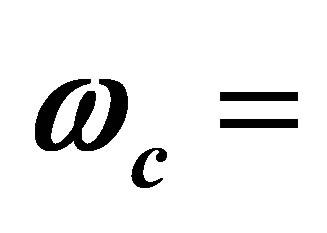
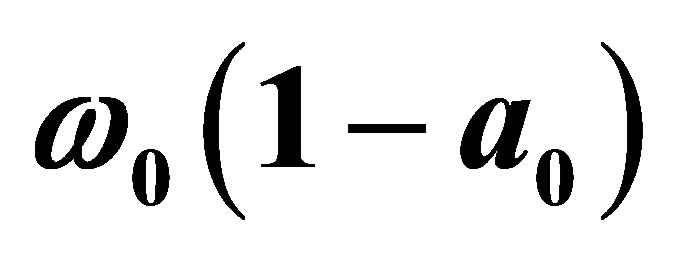 and lower branch
and lower branch 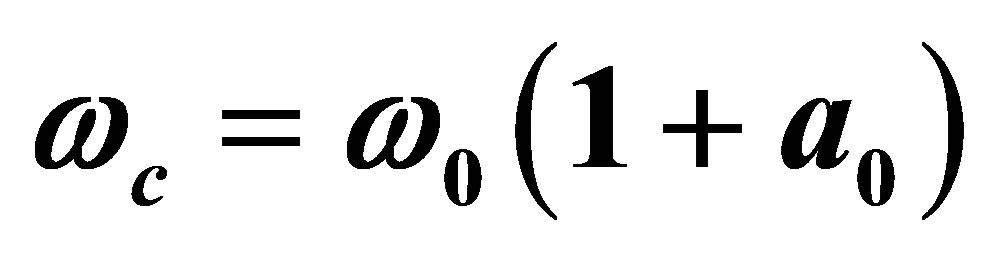 of the spectrum are shown.
of the spectrum are shown.
stant of the electron-phonon bonding, and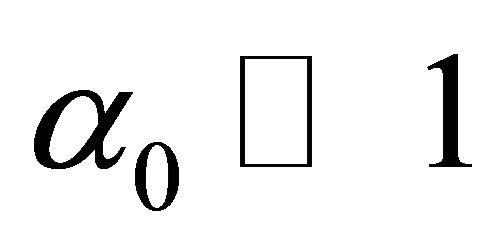 .
.
During the last several years, a new wave of interest to the Johnson-Larsen effect [4-22] was stimulated by appearance of semiconducting objects of reduced dimension in which the effect is increased due to dimensional quantization of the electron’s motion in perpendicular direction.
Formation of polaron states takes place both in threedimensional (3D) [1-3,22] and quasi-two-dimensional (2D) systems [4-22]. A difference between the systems is in electron’s spectra in the presence of quantizing magnetic field: in 3D-system these are one-dimensional Landau zones, and in 2D system these are discrete Landau levels. This difference leads to different magnitudes of pushing aside the levels of electron-phonon system [3,8,9].
In 3D and 2D systems, the magnetopolaron states play an important role in formation of frequency dependence of magnetooptical phenomena, such as the intarband absorption of light [1,2,6], the cyclotron resonance [4,5, 7,10] and Raman scattering of light [12-14].
In 2D-systems, the effect is enhanced, and the distance between the components of the splitting of the peak becomes proportional to 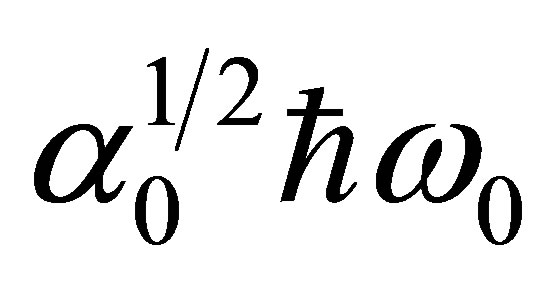
 [8-15].
[8-15].
When the Condition (1) is implemented, and when Landau levels n = 1 and n = 0 plus one LO-phonon are connected, the double polarons are formed [22]. If in this condition, three Landau levels n = 2, n = 1 plus one LO-phonon and n = 0 plus two LO-phonons are connected then triple polarons are formed [22]. The triple polarons in a bulk semiconductor were discussed in [23] and in the quantum wells they were considered in works [12-14,16].
The energy spectrum of magnetopoarons—both usual (classical) and combined [18]—was defined in two ways, which give the same results. One of them was first used in [3] and consisted in determining the poles of the single-particle Green’s function of an electron. It was also used in [8,17,18]. Another method was described in work [14] devoted to double magnetopolaron of A type. Wave functions of the polaron are represented as the superposetion of wave functions of unperturbed states (in the case of the A-polaron, this state (n = 1 and n = 0 plus one LOphonon) is that with yet unknown coefficients). Schrodinger equation reduces to a system of two equations for two coefficients. Equating the determinant to zero, we obtain a quadratic equation for the energies of polaron states p = a and p = b. The advantage, as compared with the first method, is that simultaneously with the calculation of the energies we find the wave functions of magnetopolarons, and these functions are necessary to describe a lot of magneto-optical effects. In [22], the results of [14] for the A-polaron were generalized to the case of all double polarons, including regular combined and special polaron states.
In this paper, we present the results of theoretical investigation of the energy spectrum of a triple mangotopolaron, and the influence of the triple magnotopolaron spectrum on the frequency dependence of magneto-optical interband peaks in semiconductor quantum wells.
2. Problem Statement and Analysis of the Mass Operator in Case of a Low Temperature
We consider a semiconductor in which in a surface layer there is a single-dimensional potential well that quantizes the motion of electrons in a direction normal to the interface (we mean InSb in MDS system and GaAs heterojunction). In the magnetic field, which is normal to the interface, the energy levels in the well become discrete ones (with infinitely fold degeneracy), and their classification depends on the ratio of the energy in the well and the cyclotron energy. Below we assume that the energy of quantization in the well is high as compared with the cyclotron one, and only the lowest level with adjacent Landau levels is taken into account We assume that the valence (V) and conductance (C) bands are located in the center of Brillouin zone, and the direct dipole transition between them is allowed. Interaction with LO-phonons, which determines in this case the splitting of peaks, we assume to be a weak one. In many semi-conductors the following condition is valid: 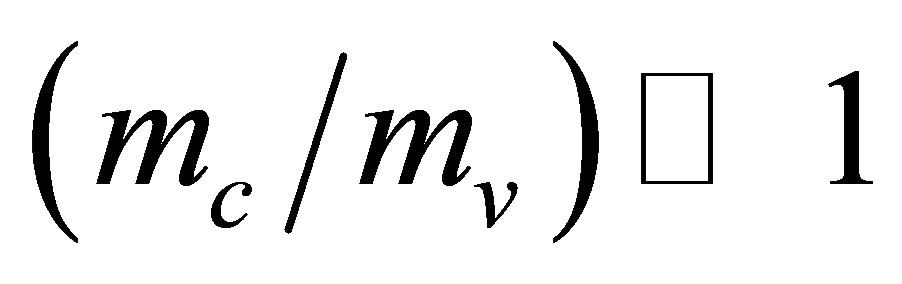 (where mv is the effective mass of the hole). We consider in this case the interband optical transitions, which creates an electron in a potential well of the conduction band and a hole near the peak of the Landau band or in a potential well in the valence band in the case of GaAs heterojunction (possibility of formation of exciton states is neglected). If the temperature is low, and the magnetic field is close to the resonance (see (1)), then the hole states will be stationary ones in the chosen mechanism of interaction, because the hole can not actually emit the LO-phonon due to a lack of energy. The hole cannot also absorb phonons because of their absence. An electron in the conductance band can, in this case, actually emit the LO-phonon and move to the level with quantum number n – 1.
(where mv is the effective mass of the hole). We consider in this case the interband optical transitions, which creates an electron in a potential well of the conduction band and a hole near the peak of the Landau band or in a potential well in the valence band in the case of GaAs heterojunction (possibility of formation of exciton states is neglected). If the temperature is low, and the magnetic field is close to the resonance (see (1)), then the hole states will be stationary ones in the chosen mechanism of interaction, because the hole can not actually emit the LO-phonon due to a lack of energy. The hole cannot also absorb phonons because of their absence. An electron in the conductance band can, in this case, actually emit the LO-phonon and move to the level with quantum number n – 1.
Wave functions of electron in this case can be written as
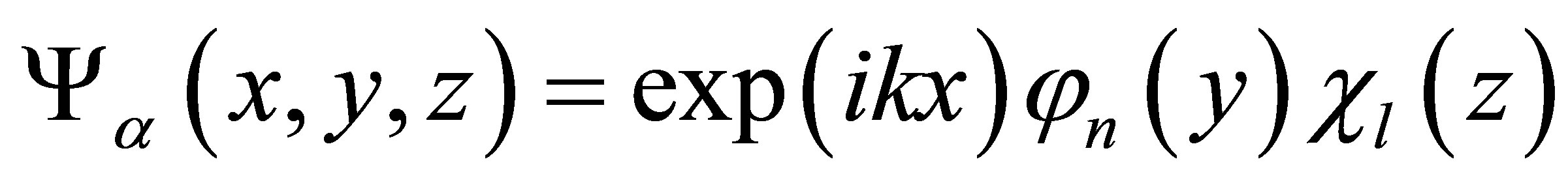 (2)
(2)
where 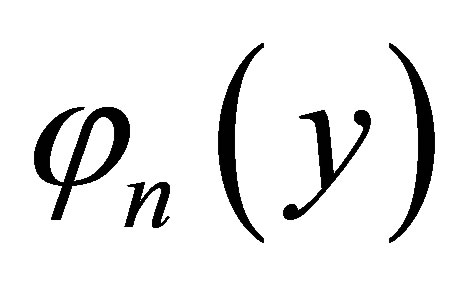 is a wave function of a harmonic oscillator in the magnetic field,
is a wave function of a harmonic oscillator in the magnetic field, 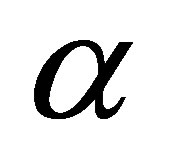 represents l, n and k quantum numbers that characterize the state of electron in the potential well in the magnetic field (l is a number of the quantization level along z axis, i.e. along the normal direction to the interface, that describes the levels of dimensional quantization in the well; n is the number of Landau level; k is the continuous quantum number, which describes the degeneration of discrete levels).
represents l, n and k quantum numbers that characterize the state of electron in the potential well in the magnetic field (l is a number of the quantization level along z axis, i.e. along the normal direction to the interface, that describes the levels of dimensional quantization in the well; n is the number of Landau level; k is the continuous quantum number, which describes the degeneration of discrete levels). 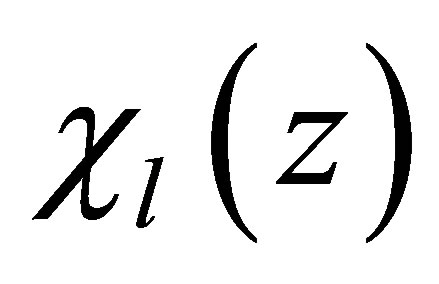 describes the quantum states in the well. In case when only the lowest level l = 0 is occupied, the function
describes the quantum states in the well. In case when only the lowest level l = 0 is occupied, the function 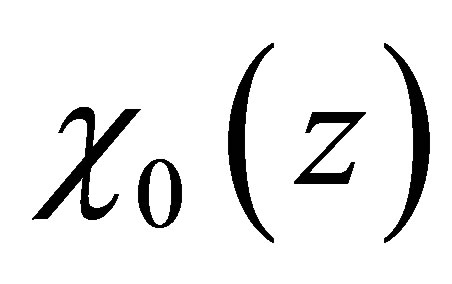 can be well approximated by the following expression:
can be well approximated by the following expression:
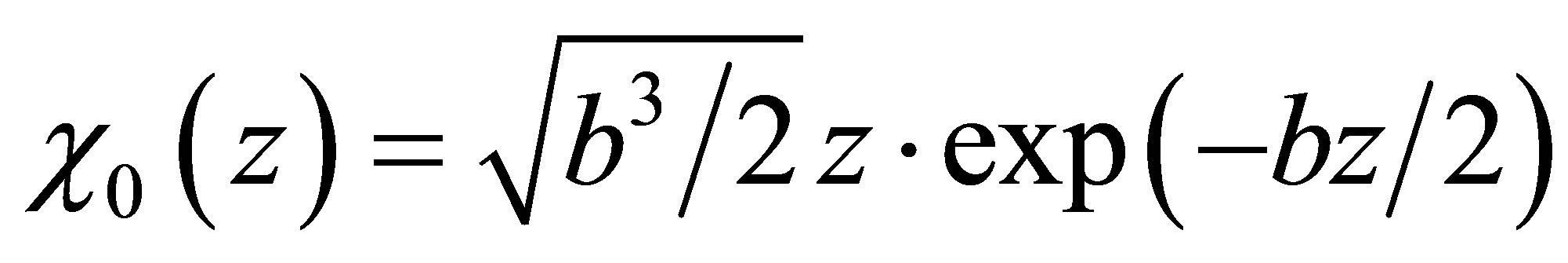 (3)
(3)
where
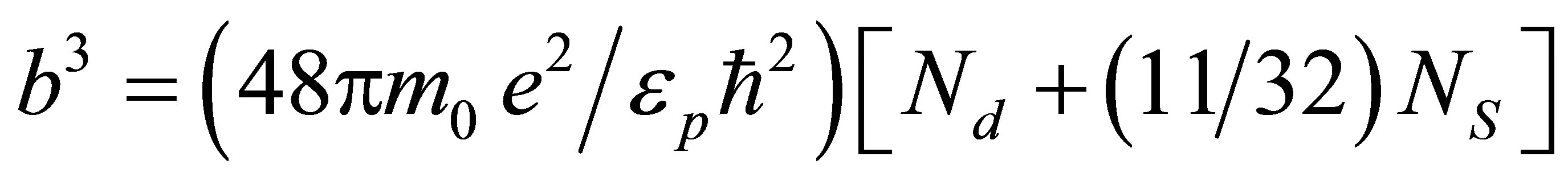 , (4)
, (4)
Here, mo is the mass of a free electron, Nd is the concentration of ionized impurities in the depleted layer, 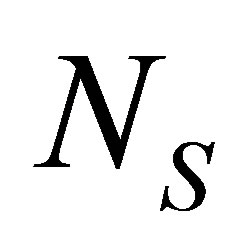 is the concentration of electrons (cm−2) on l = 0 level,
is the concentration of electrons (cm−2) on l = 0 level, 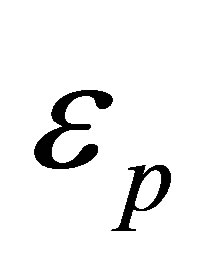 is the static dielectric constant of a semiconductor.
is the static dielectric constant of a semiconductor.
The absorption will be characterized by a fraction of the absorbed energy [24]
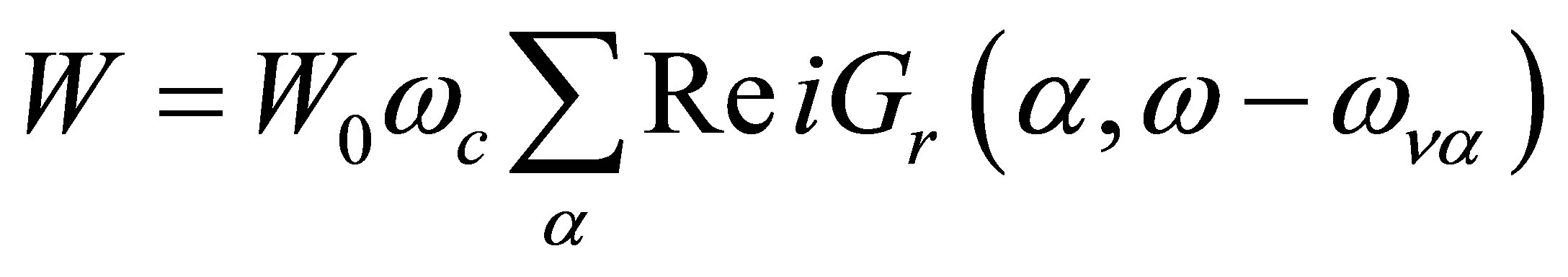 (5)
(5)
where
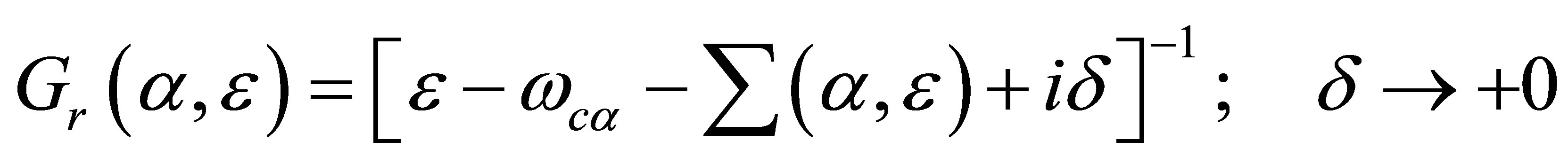 (6)
(6)
is the single-particle retarded Green’s function of the electron
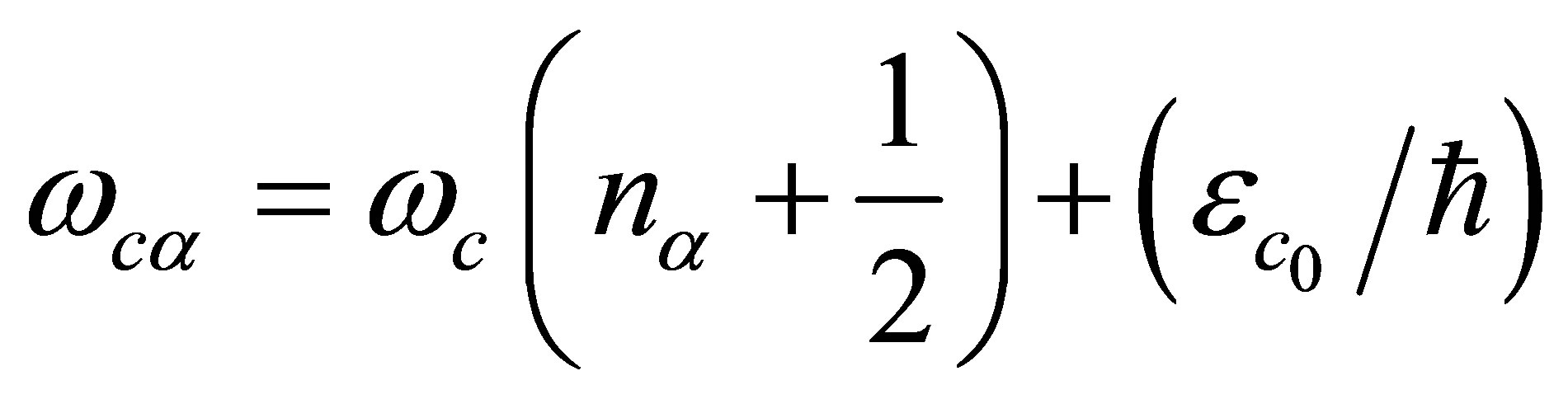 (7)
(7)
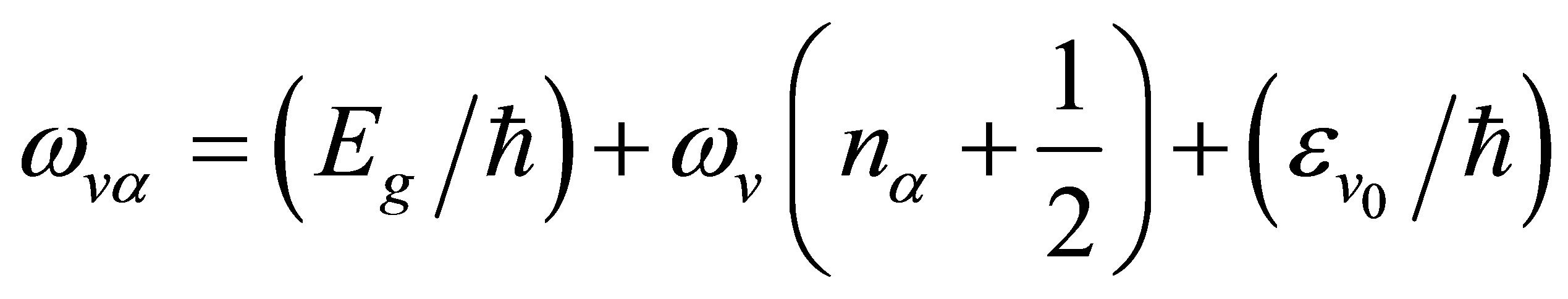 (8)
(8)
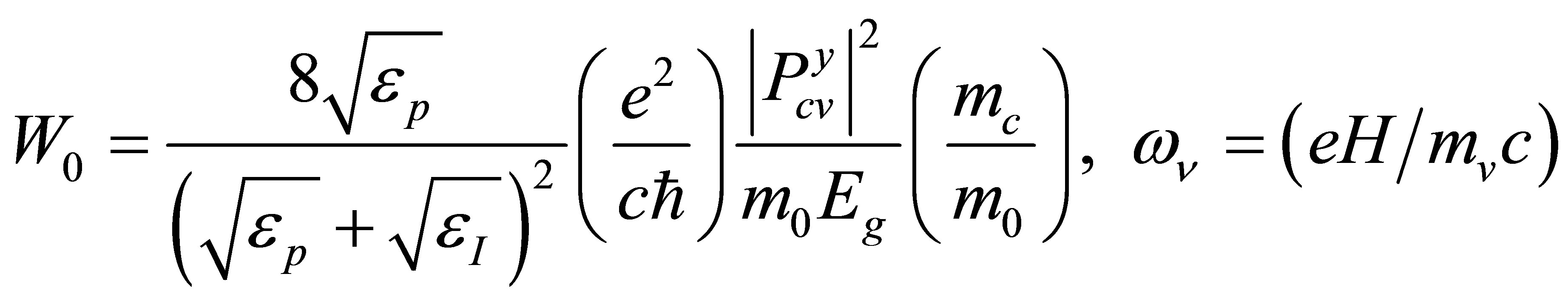 (9)
(9)
Here, 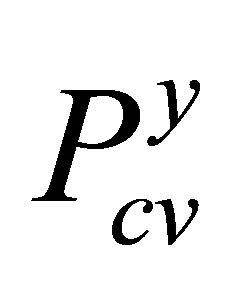 is the interband momentum matrix element, evaluated at the Bloch modulating factors,
is the interband momentum matrix element, evaluated at the Bloch modulating factors, 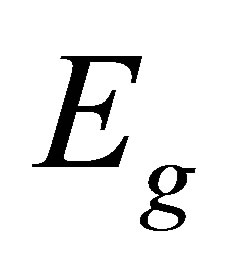 is the band gap,
is the band gap, 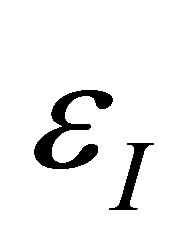 is the statistical permittivity of the dielectric,
is the statistical permittivity of the dielectric,  is the energy of l = 0 level in the potential well.
is the energy of l = 0 level in the potential well.
Below we consider in detail the transition of electron under the influence of light on Landau level with n = 2 in the conductance band. In this case, three terms of electron-phonon system (electron at n = 2 level, electron at n = 1 level and one LO-phonon, and electron at n = 0 level and two LO-phonons), which are considered as functions of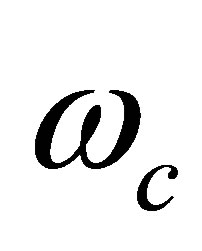 , intersect in the point
, intersect in the point 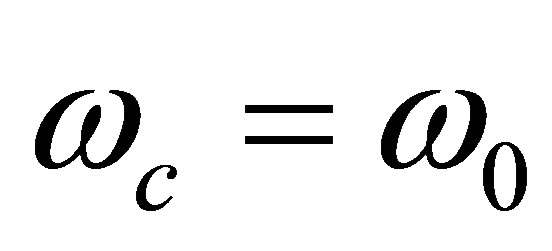 (Figure 1). The electron-phonon interaction leads to break of degeneracy in the point
(Figure 1). The electron-phonon interaction leads to break of degeneracy in the point  and, consequently, to appearance of three non-intersecting branches of the spectrum.
and, consequently, to appearance of three non-intersecting branches of the spectrum.
The problem of calculation of the spectrum is complicated by the fact that emission of phonons is connected with transitions of electrons between Landau levels, where the density of states is high. Formally, it means the impropriety of usual series expansion of the mass operator with respect to coupling constant, and necessity of selective summation of the series.
We start the analysis of the self-energy part of 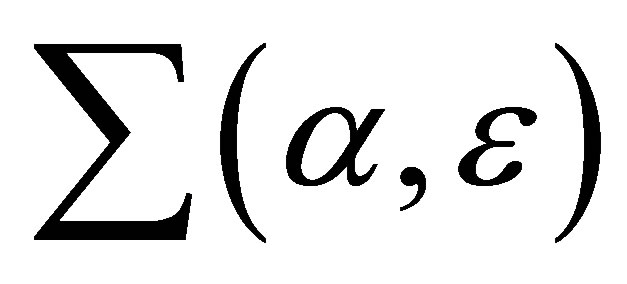 with consideration of the graph in Figure 2, a, which is
with consideration of the graph in Figure 2, a, which is
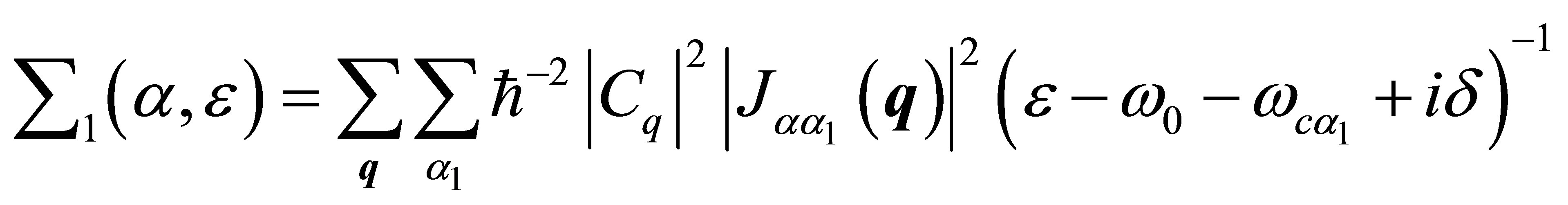 (10)
(10)
where
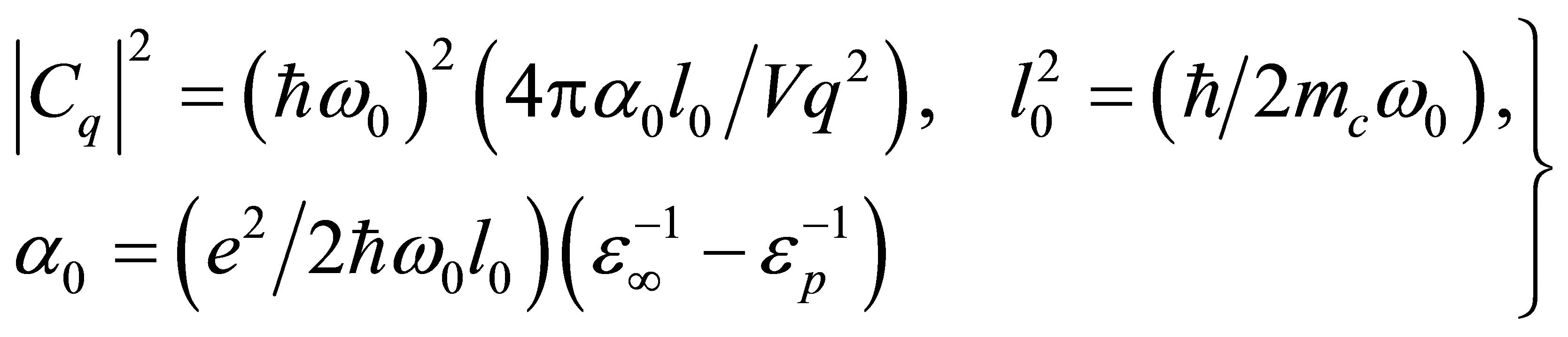 (11)
(11)
and 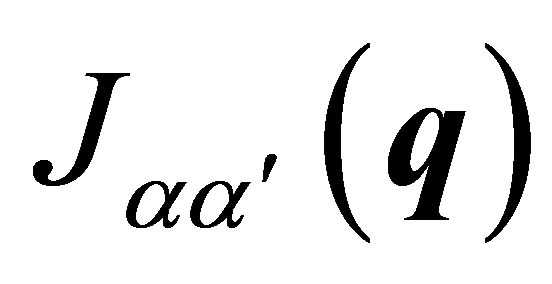 is the matrix element of
is the matrix element of 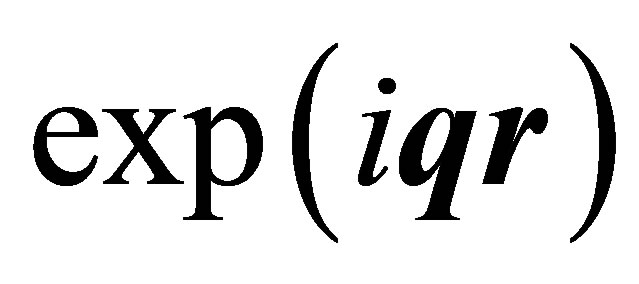 operator, evaluated at the wave functions (3)
operator, evaluated at the wave functions (3)
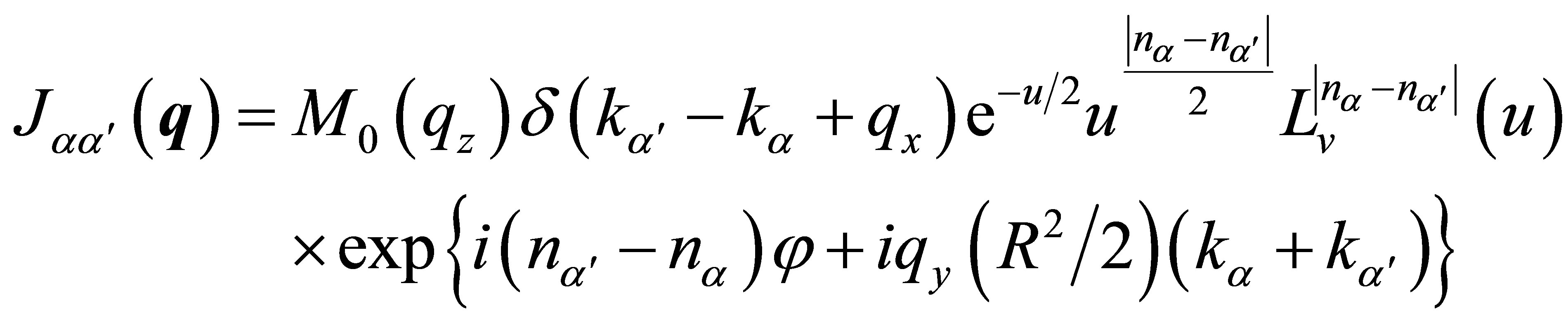 (12)
(12)
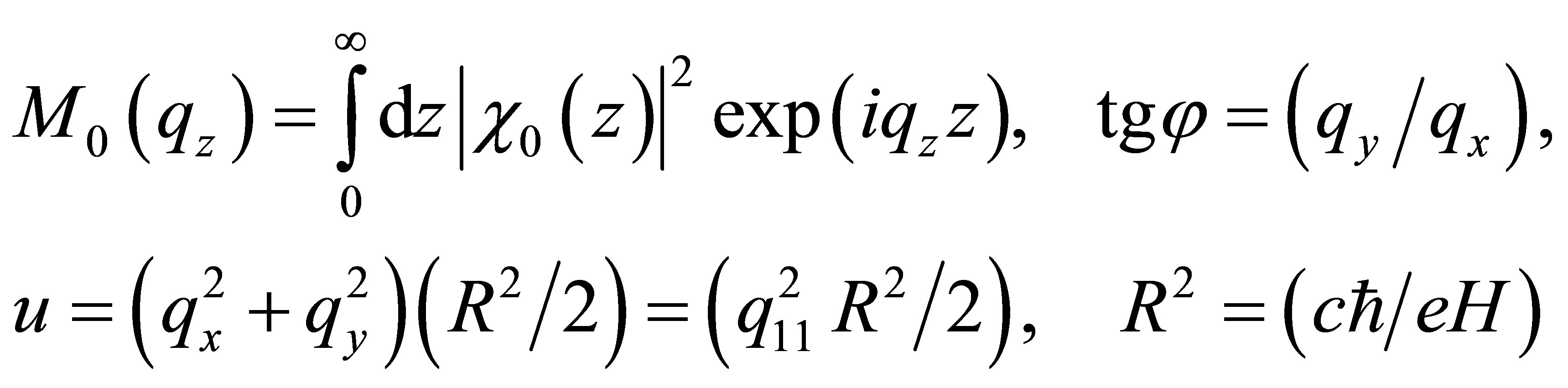 (13)
(13)
Here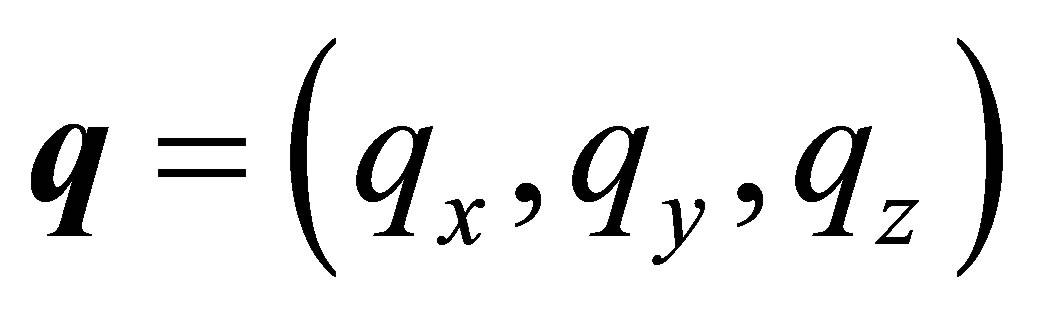 —is the wave-vector of phonon,
—is the wave-vector of phonon,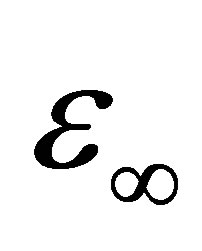 —is the high-frequency dielectric constant,
—is the high-frequency dielectric constant,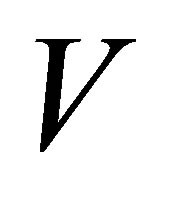 —is the normalization volume,
—is the normalization volume, 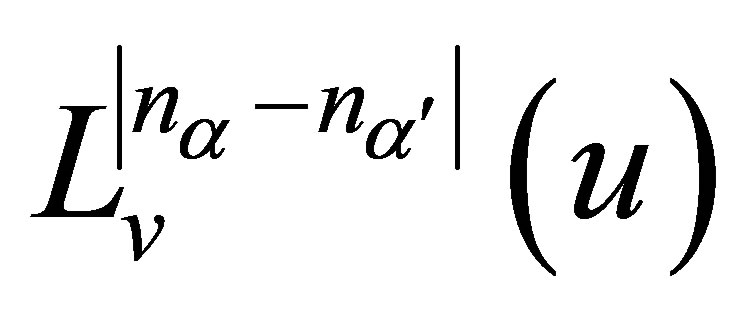 is Laguerre polynomial [25],
is Laguerre polynomial [25],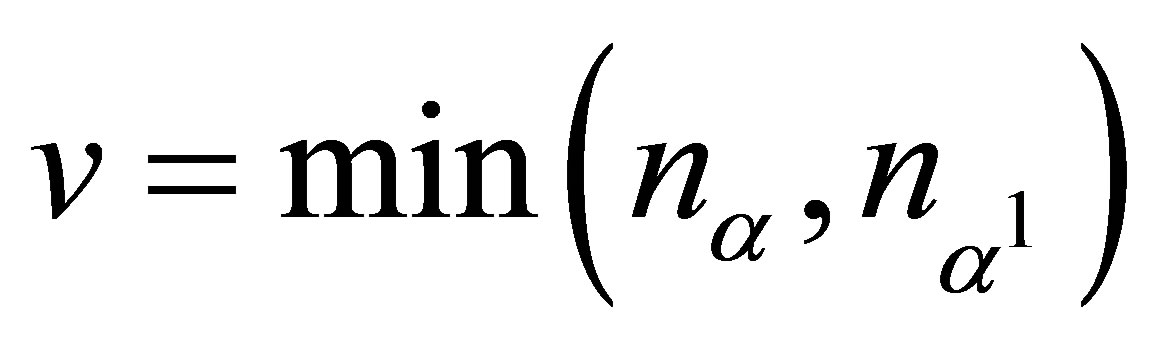 .
.
In case then the electron is thrown by light to Landau level n = 2 in the sum by 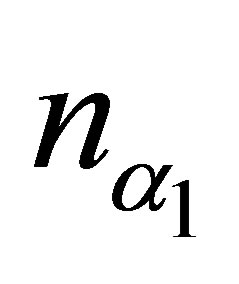 the term with
the term with 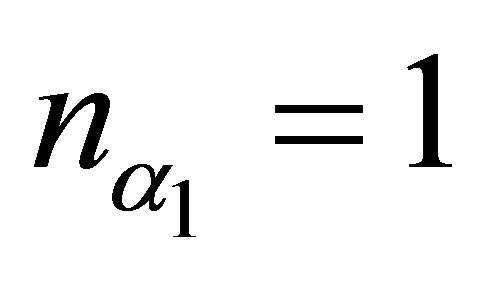 will be a resonant one since it corresponds to a real resonant transition between the neighboring Landau levels. Assuming
will be a resonant one since it corresponds to a real resonant transition between the neighboring Landau levels. Assuming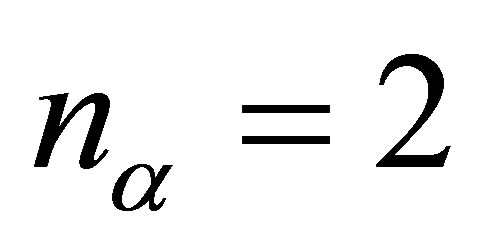 ,
, 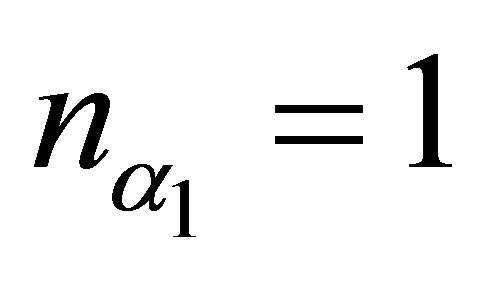 for the resonant term we will have
for the resonant term we will have
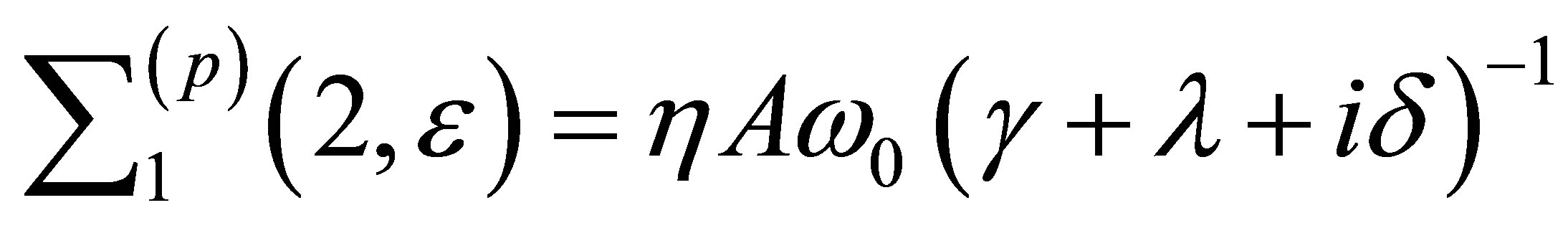 (14)
(14)
where
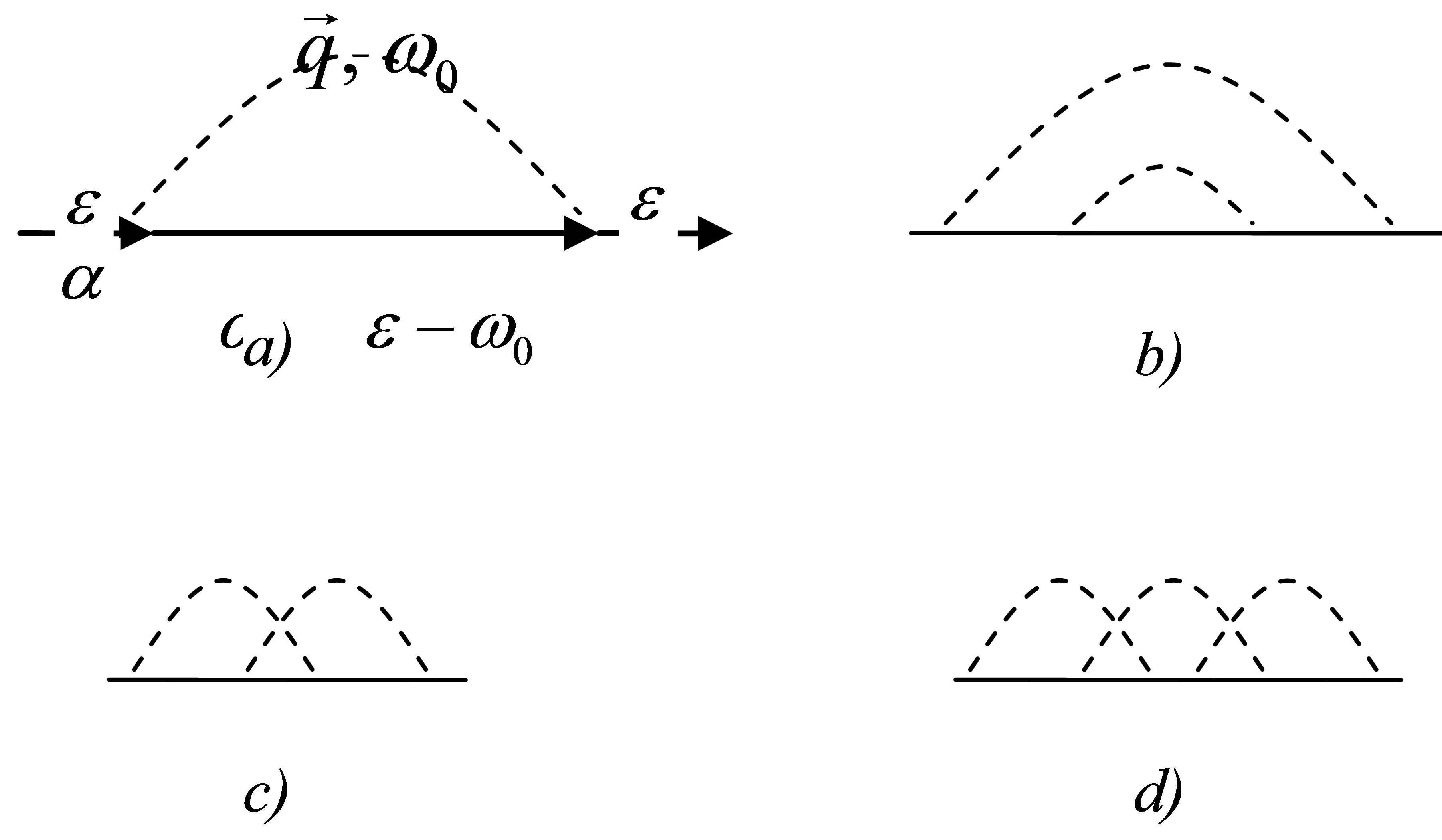
Figure 2. Examples of graphs, which are essential for calculation of the self-energy part.
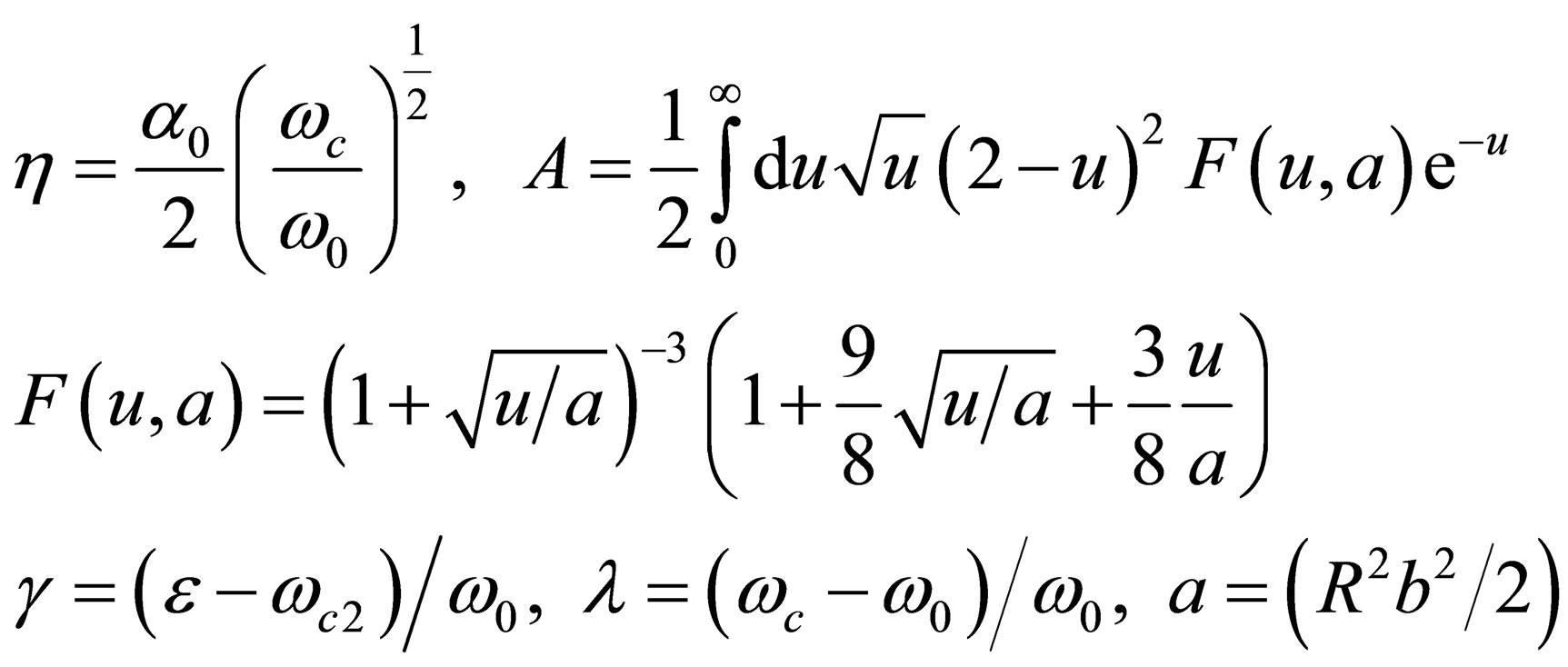 (15)
(15)
Graphs with two phonon lines, which are presented in Figures 2(b) and (c) in the area of resonance when 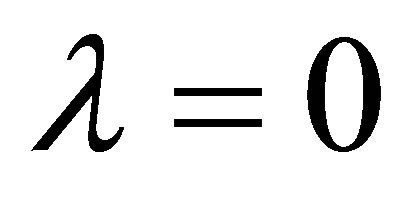 are, correspondingly, equal to
are, correspondingly, equal to
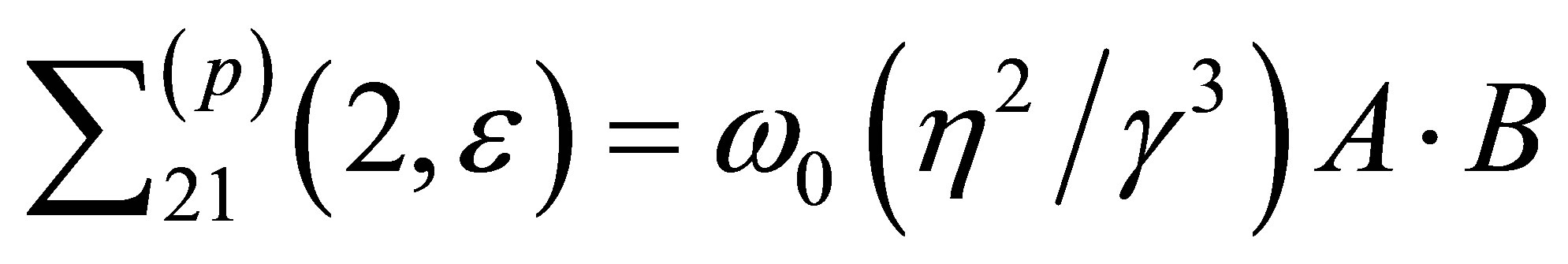 (16)
(16)
 (17)
(17)
where
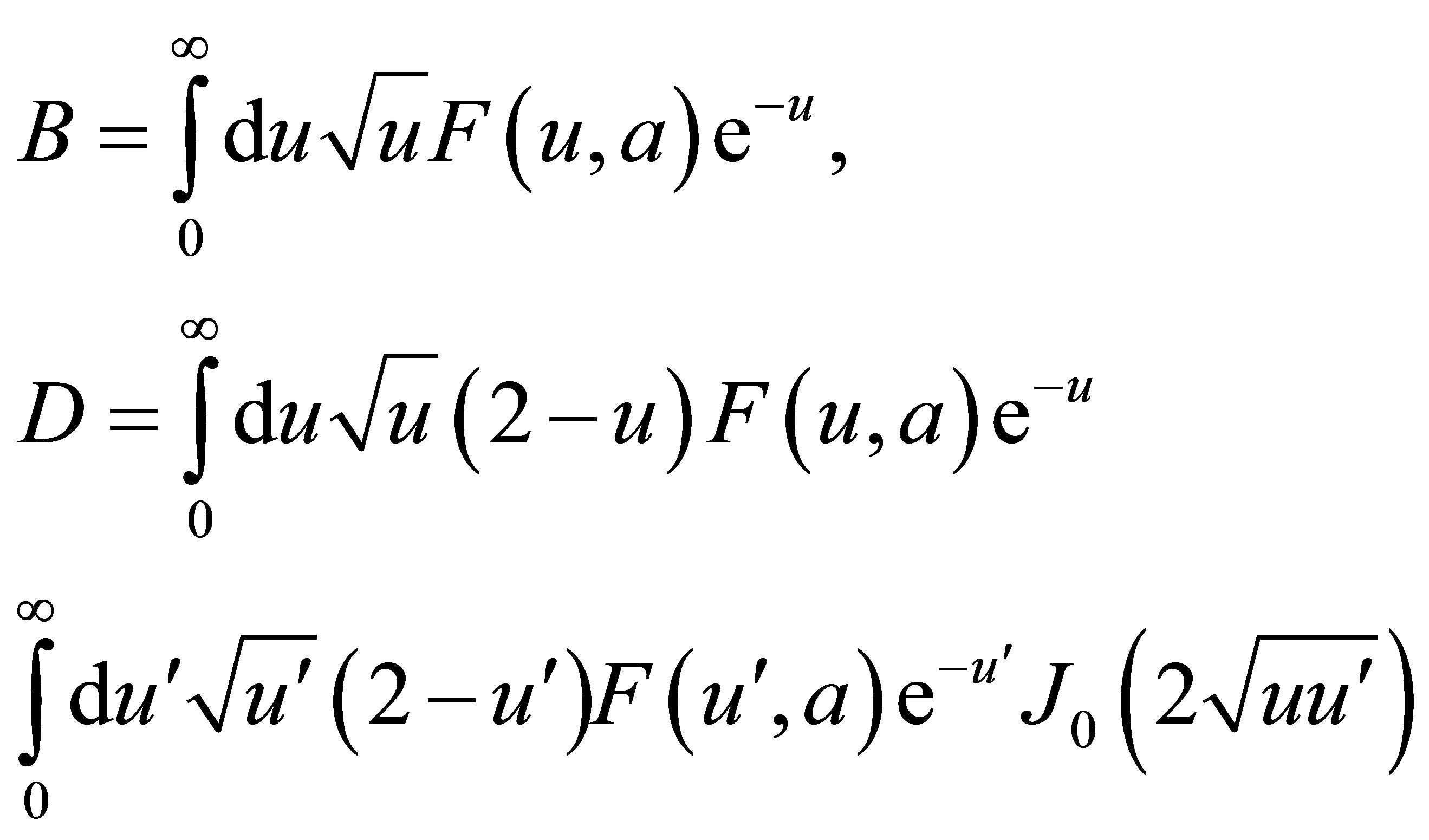 (18)
(18)
The general selection rule of the diagrams for the considered case of low temperatures and 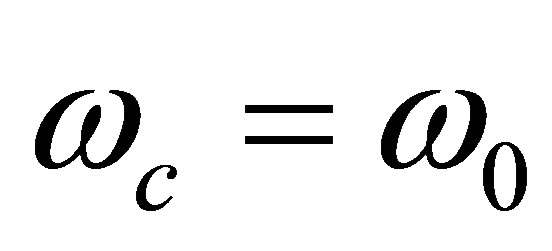 is as follows. For the Landau level with quantum number n the essential graphs will be those ones in which n-multiple resonant transition with emission of LO-phonons and serial transitions of the electron between the neighboring Landau levels is allowed. Graphs in which number of emitted phonons is greater than the number of possible resonant transitions will have smallness with respect to bonding constant, which is greater, the more “extra” phonon lines in the graph. That is why, for the level n = 2, besides graphs in Figures 2(b) and (c), another graphs will be essential, for example in Figure 2(d). Graphs presented in Figure 3 are small with respect to the bonding constant since they contain non-resonant transitions and, correspondingly, non-resonant denominators.
is as follows. For the Landau level with quantum number n the essential graphs will be those ones in which n-multiple resonant transition with emission of LO-phonons and serial transitions of the electron between the neighboring Landau levels is allowed. Graphs in which number of emitted phonons is greater than the number of possible resonant transitions will have smallness with respect to bonding constant, which is greater, the more “extra” phonon lines in the graph. That is why, for the level n = 2, besides graphs in Figures 2(b) and (c), another graphs will be essential, for example in Figure 2(d). Graphs presented in Figure 3 are small with respect to the bonding constant since they contain non-resonant transitions and, correspondingly, non-resonant denominators.
3. Evaluation of the Spectrum of Electron-Phonon System
In order to obtain a qualitative picture, we neglect first of all graphs, which contain apical parts. In this case it is enough to take into account a series of graphs presented in Figure 4. Non-resonant terms in all graphs are neg-
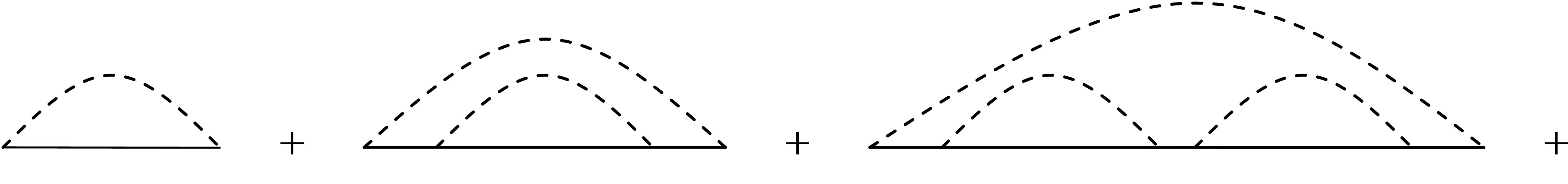
Figure 4. A series of graphs that leads to Equation (19).
lected due to their smallness with respect to the resonant ones. In this case, the summation of the series in Figure 4 leads to the following equation
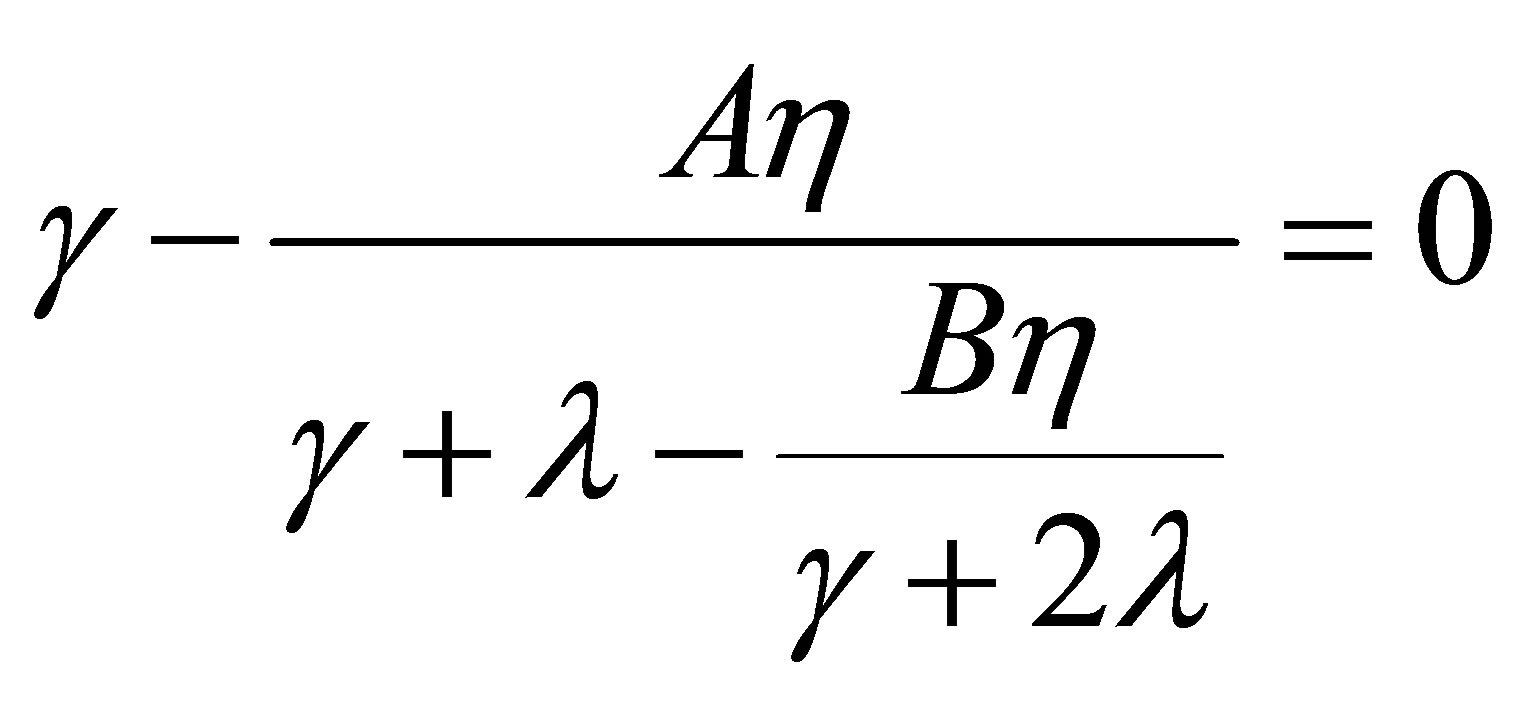 (19)
(19)
If we make a substitution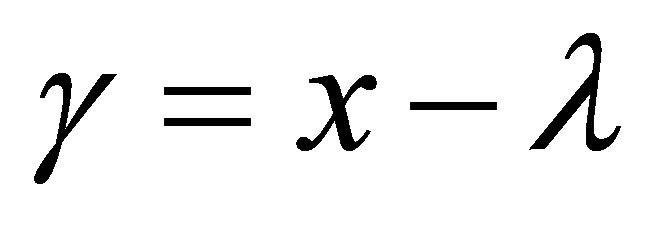 , then (19) can be presented as follows
, then (19) can be presented as follows
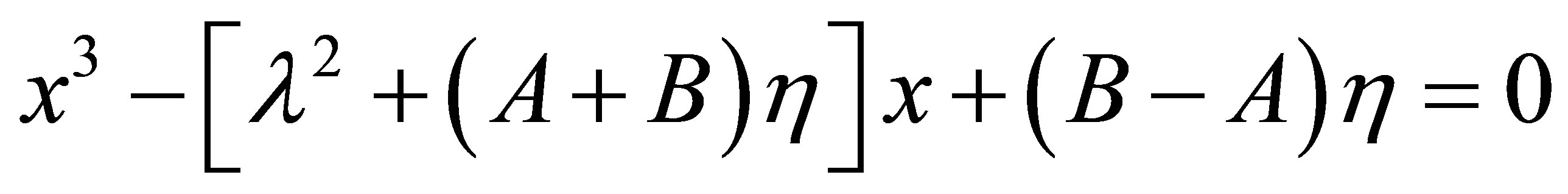 (20)
(20)
The roots of (20) are
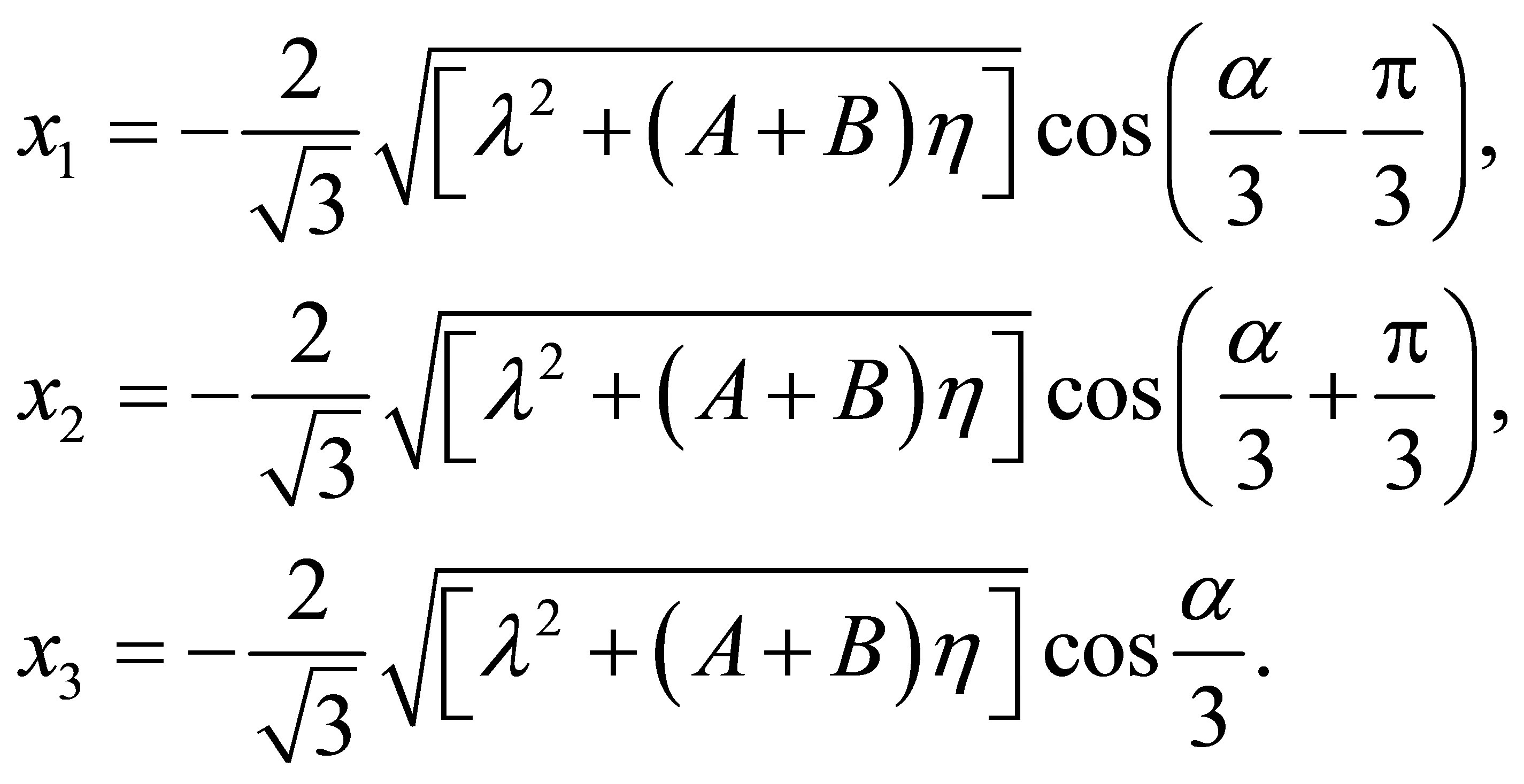 (21)
(21)
where
 . (22)
. (22)
Taking into account the made substitution we have for the spectrum of the electron-phonon system
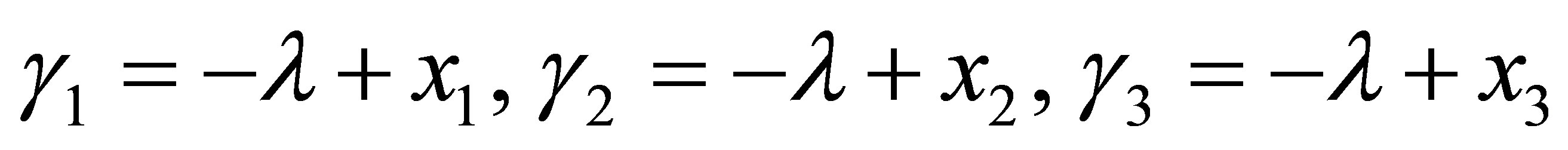 (23)
(23)
and when 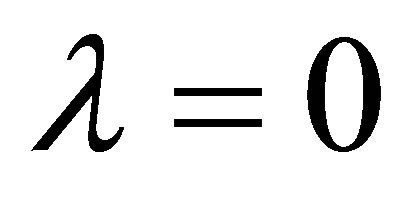 we have
we have
 (24)
(24)
When 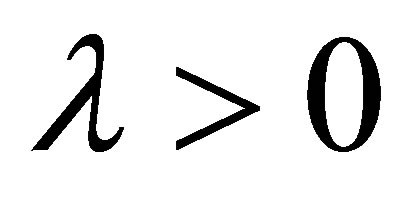 and
and 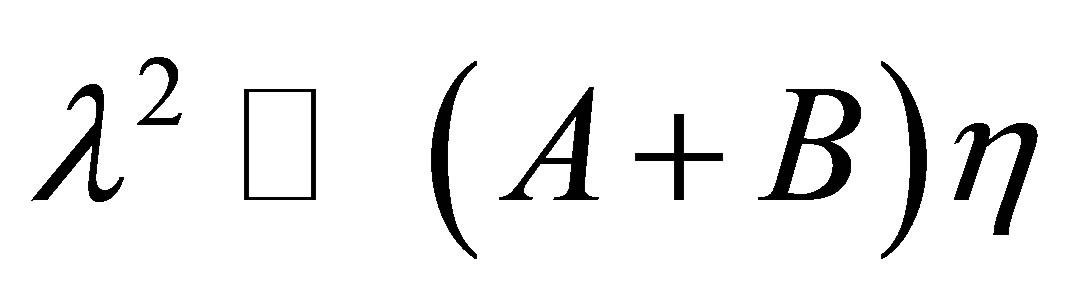 from (23) we have
from (23) we have
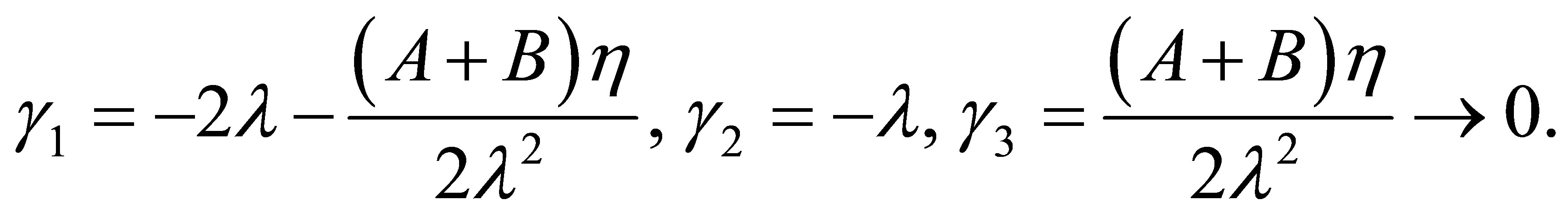 (25)
(25)
i.e., the lower branch of the spectrum disappears, at high magnetic fields two branches remain (see Figure 1).
If 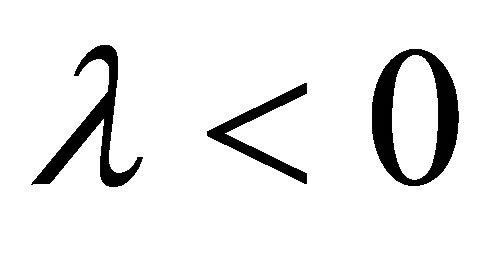 and
and , then we have a reverse picture
, then we have a reverse picture
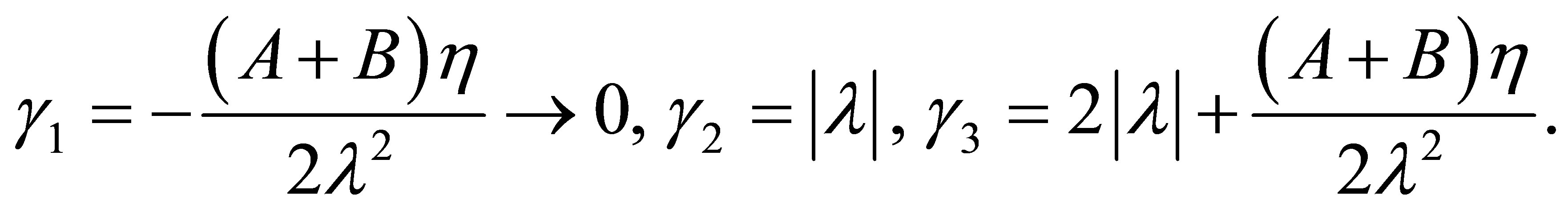 (26)
(26)
i.e., upper branch of the spectrum disappear, and also two branches remain (see Figure 1).
Under disappearance of the upper branch of the spectrum 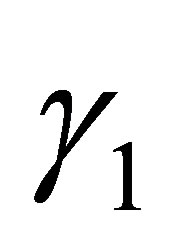 we should understand disappearance of the bond between states of electron with n = 1 and n = 0. Actually, when the magnetic field decreases the cyclotron frequency becomes so much lesser than
we should understand disappearance of the bond between states of electron with n = 1 and n = 0. Actually, when the magnetic field decreases the cyclotron frequency becomes so much lesser than 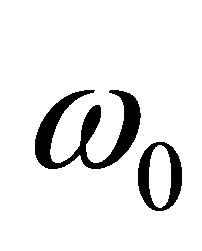 that the transition of electron thrown by light to level n = 2 trough level n = 1 becomes impossible. Since the energy levels are discrete ones the energy conservation law is not valid. With rising the magnetic field the cyclotron frequency becomes so much higher than
that the transition of electron thrown by light to level n = 2 trough level n = 1 becomes impossible. Since the energy levels are discrete ones the energy conservation law is not valid. With rising the magnetic field the cyclotron frequency becomes so much higher than 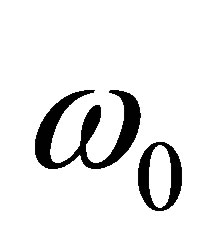 that the transition of electron from level n = 2 to level n = 1 and then to level n = 0 becomes impossible, and in this case the bond between the levels n = 2 and n = 1 disappears and two levels n = 1 and n = 0 remain, and, correspondingly, two branches
that the transition of electron from level n = 2 to level n = 1 and then to level n = 0 becomes impossible, and in this case the bond between the levels n = 2 and n = 1 disappears and two levels n = 1 and n = 0 remain, and, correspondingly, two branches 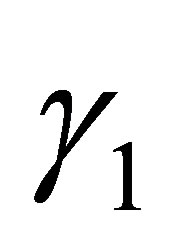 and
and 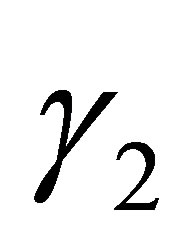 of the spectrum.
of the spectrum.
Now we account in Equation (19) the apical points. For Landau level n = 2 it is necessary to take into account the main graphs of those diverging in the resonance point, which are presented by the series presented in Figure 5(a), where the bold line presents the series in Figure 5(b).
The general term of the series is equal to
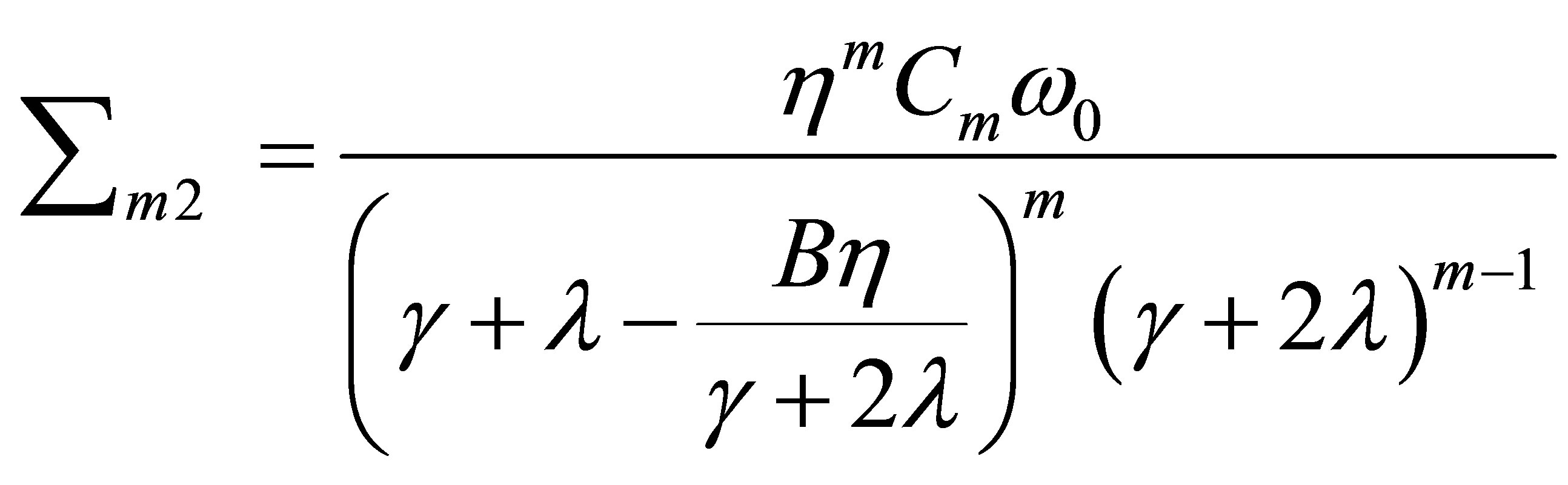 (27)
(27)
where m = 2, 3, …
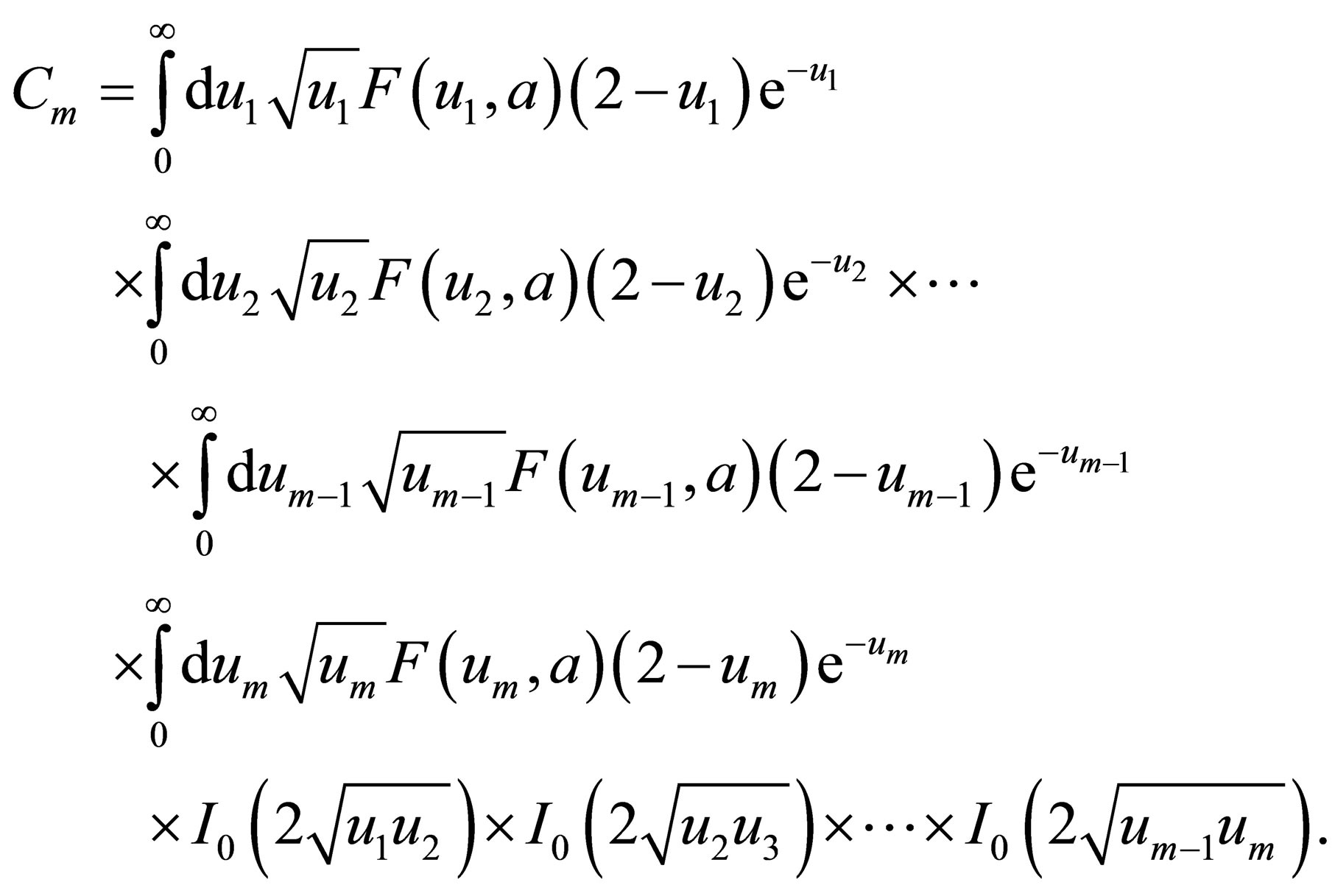 (28)
(28)
(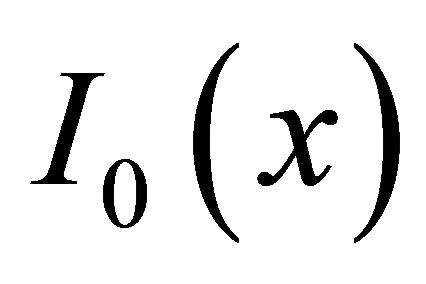 is the first order Bessel function [25]). When
is the first order Bessel function [25]). When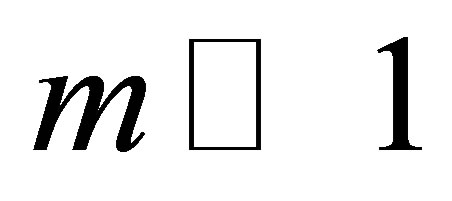 ,
,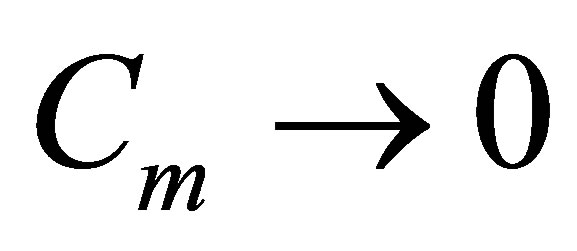 . For InSb (
. For InSb (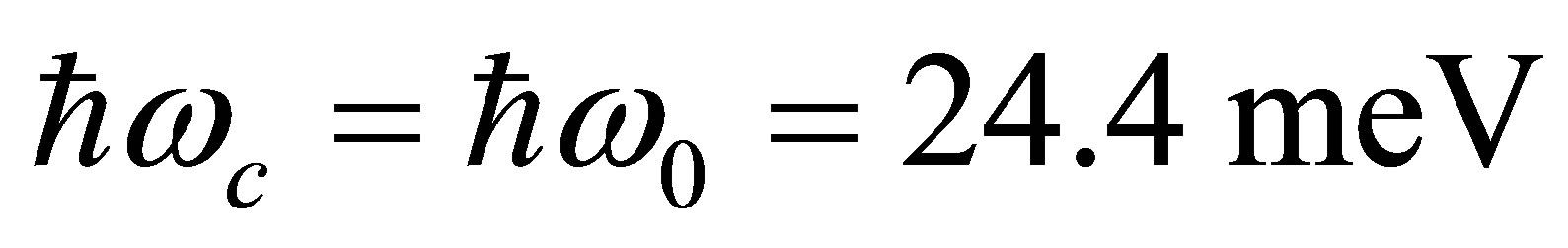 ,
,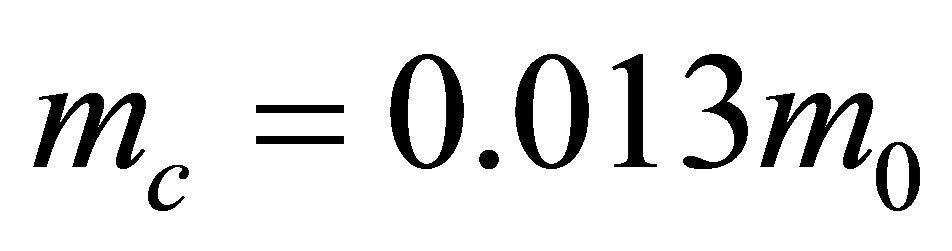 ) а = 1.8 and С2 = 0.175, С3 = 0.013 etc.
) а = 1.8 and С2 = 0.175, С3 = 0.013 etc.
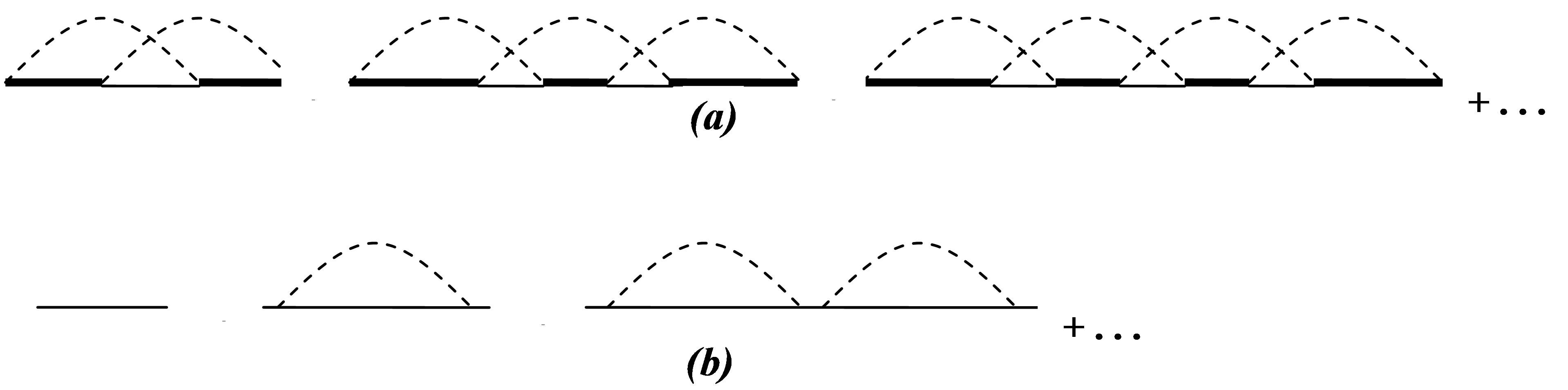
Figure 5. Essential apical parts.
Taking into account the term 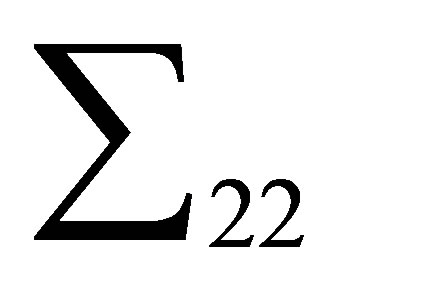 from (17) in the dispersion Equation (19) leads to addition of term (17) to the left side of the equation. Solution of (19) by taking into account this addition gives respectively small change in roots, which can be neglected.
from (17) in the dispersion Equation (19) leads to addition of term (17) to the left side of the equation. Solution of (19) by taking into account this addition gives respectively small change in roots, which can be neglected.
4. Splitting of Magnetooptical Absorption and Results Discussion
Three terms of the electron-phonon system, which in the absence of interaction, are interceptions in point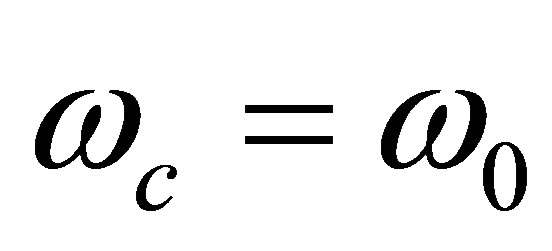 , after accounts of electron-phonon bonding are splitting, according to results of Section 2, into three branches of electron-phonon spectrum. The peak of magnetooptical absorption, which corresponds to excitation of electron by light to Landau level with n = 2, will also split at
, after accounts of electron-phonon bonding are splitting, according to results of Section 2, into three branches of electron-phonon spectrum. The peak of magnetooptical absorption, which corresponds to excitation of electron by light to Landau level with n = 2, will also split at 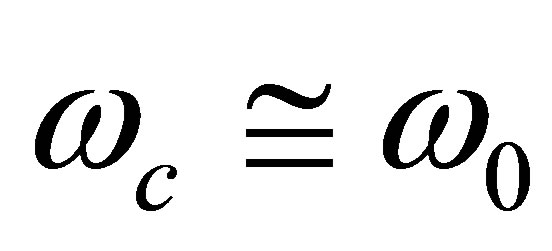 into three
into three  -like peaks. The absorption will be determined by three branches of electron-phonon spectrum in the conduction band since when the condition
-like peaks. The absorption will be determined by three branches of electron-phonon spectrum in the conduction band since when the condition 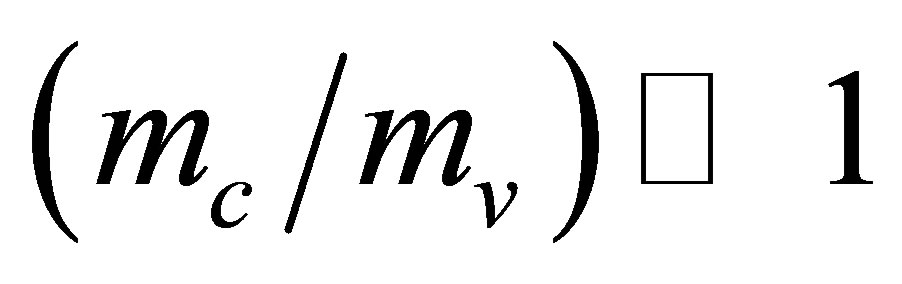 is valid, the holes do not contribute into non-stationarity of the levels.
is valid, the holes do not contribute into non-stationarity of the levels.
Now we calculate the absorption as a function of light frequency in the region of the studied peak by neglecting the small contribution of apical parts.
By taking into account Expressions (5), (6) and (19), as well as inequality of effective masses we have
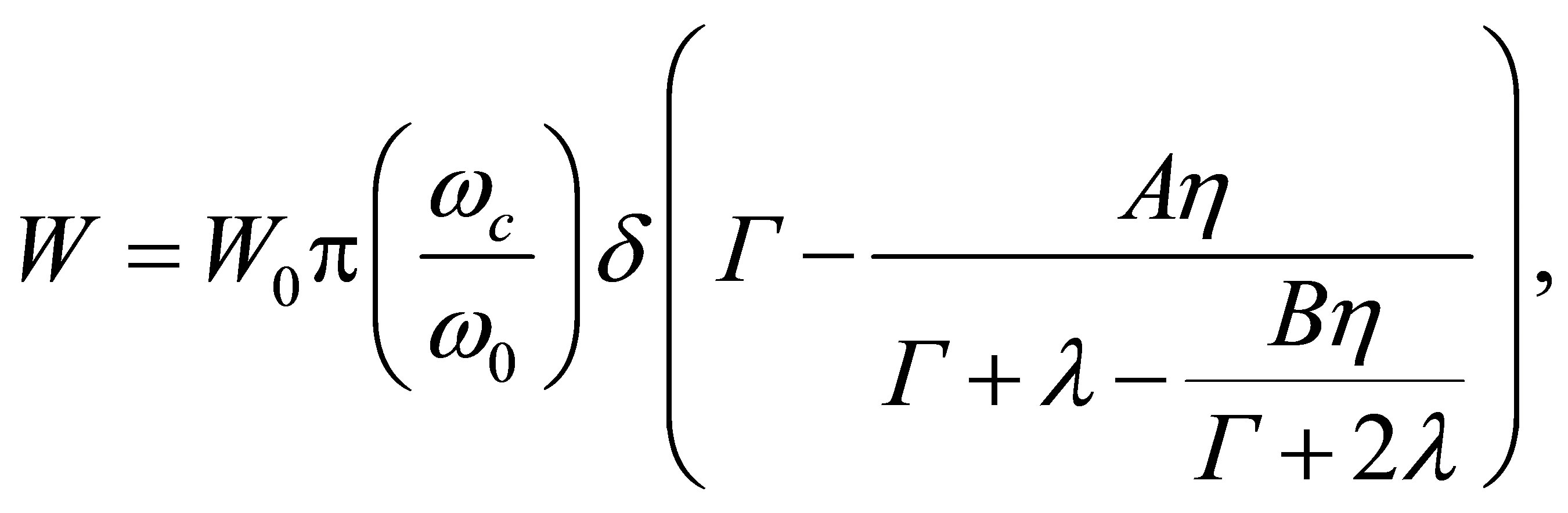 (29)
(29)
where
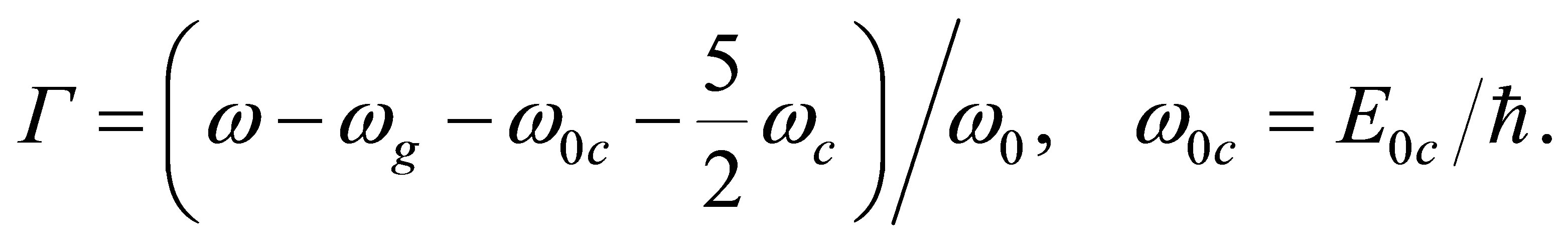 (30)
(30)
Using the properties of  -function [26], from (29) we have
-function [26], from (29) we have
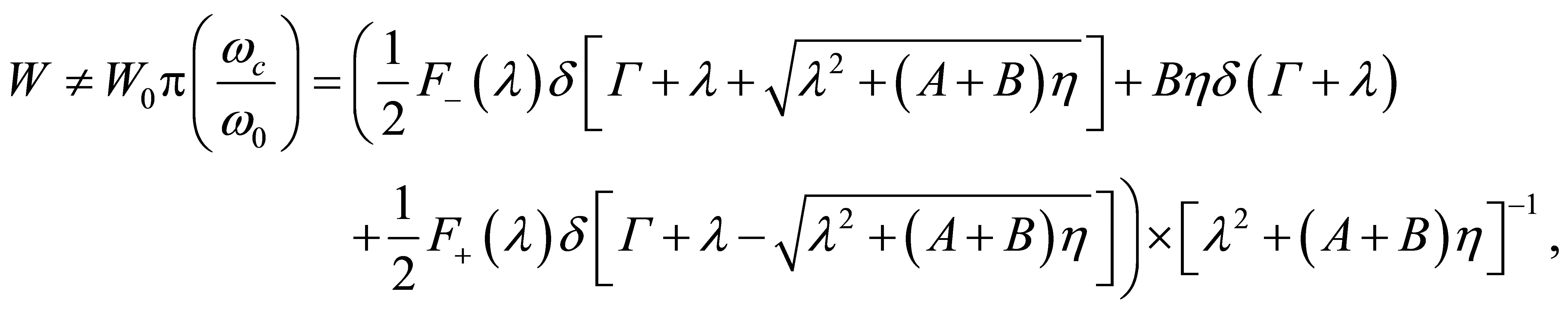 (31)
(31)
where
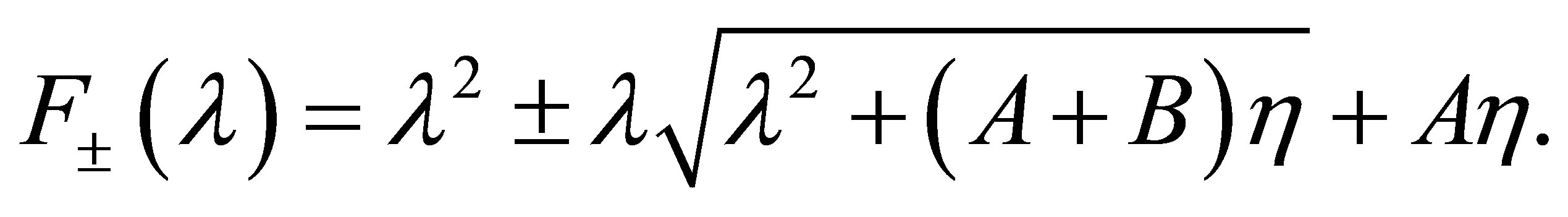 (32)
(32)
As one can see from (32) when 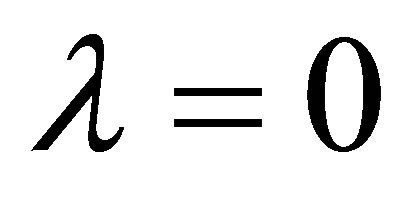 the integral intensities of left and right peaks are equal to each other
the integral intensities of left and right peaks are equal to each other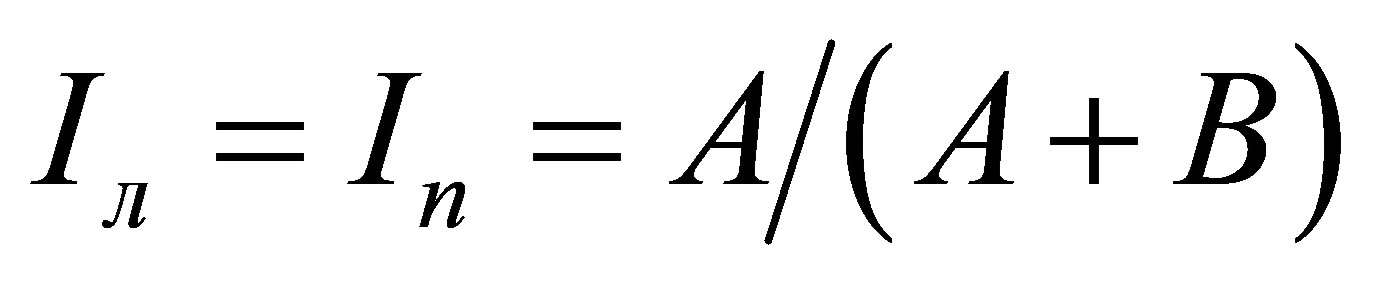 , since the central peak has the integral intensity equal to
, since the central peak has the integral intensity equal to 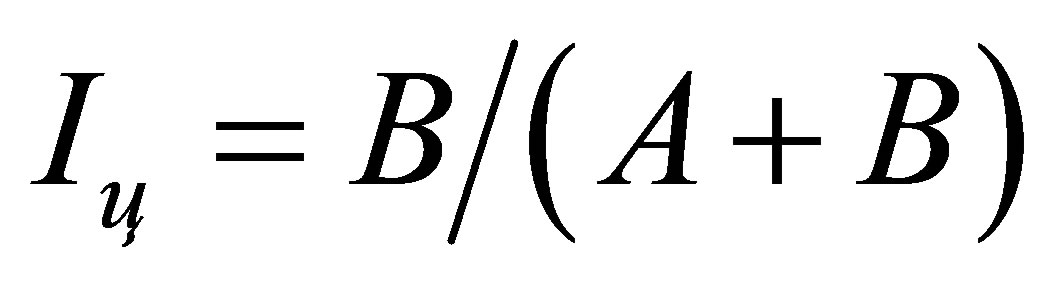 and it is
and it is 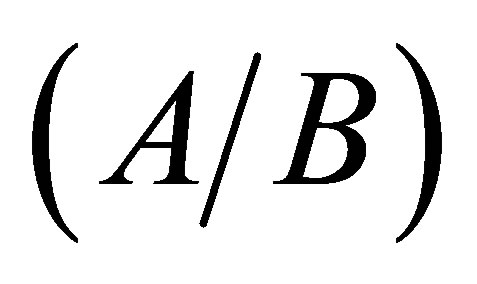 times lesser than the intensity of lateral peaks. The peaks are located in points
times lesser than the intensity of lateral peaks. The peaks are located in points 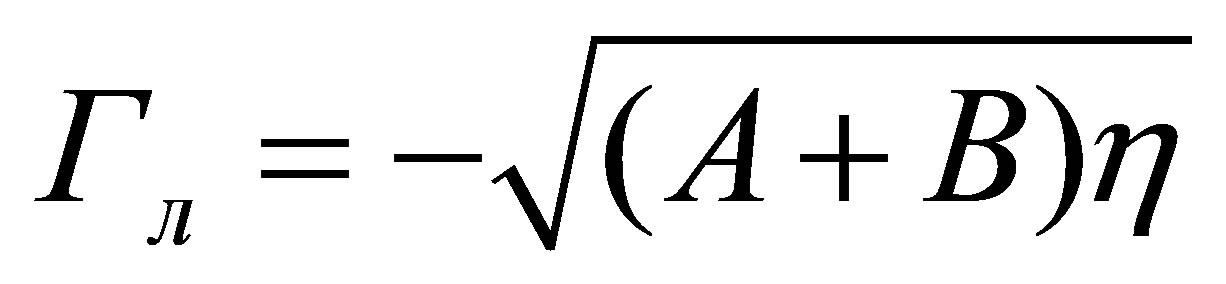 (left peak),
(left peak), 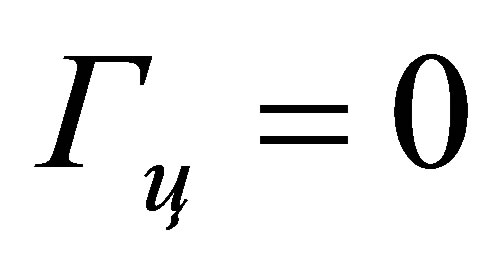 (central peak) and
(central peak) and 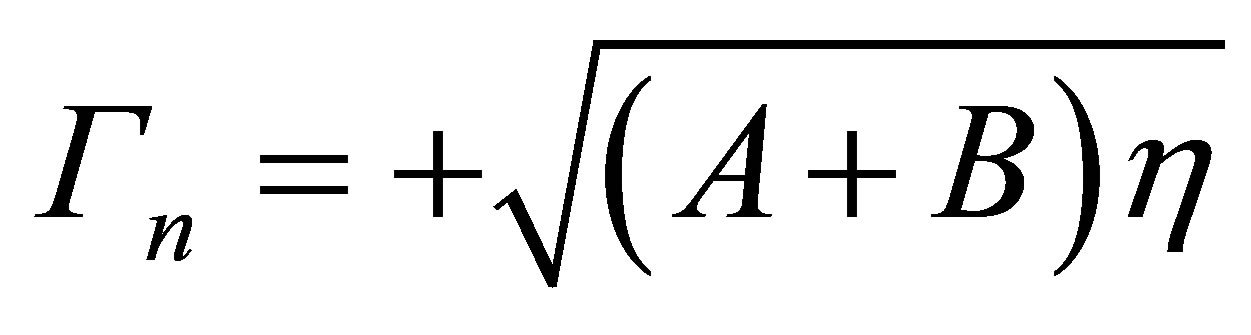 (right peak). The distance between peaks in this case is the smallest one. If
(right peak). The distance between peaks in this case is the smallest one. If 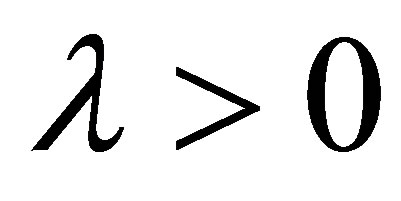 and
and , then
, then
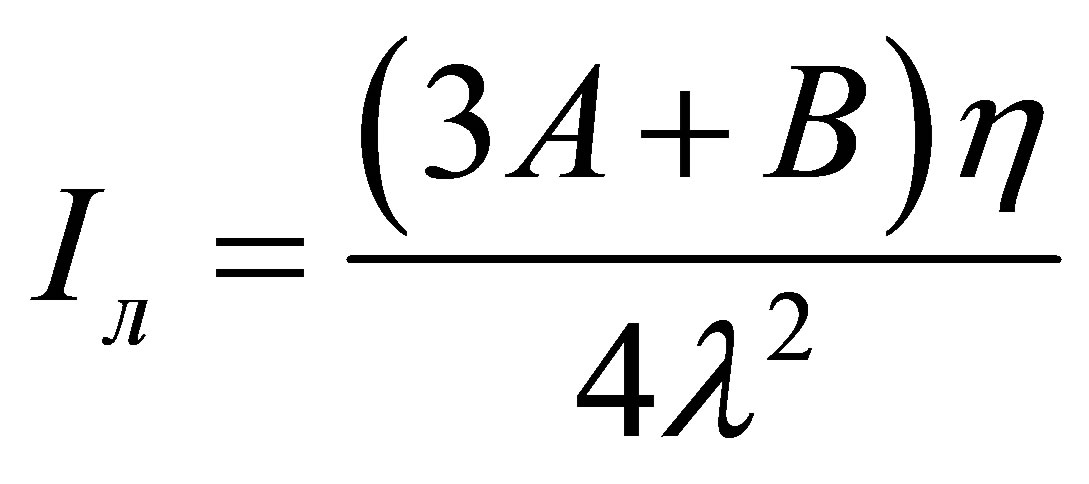 ,
, 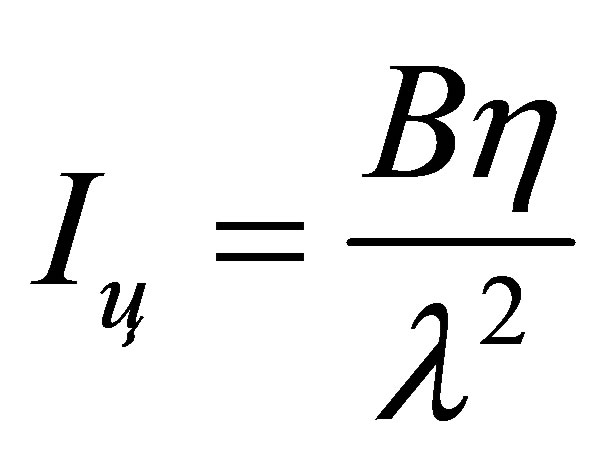 ,
, 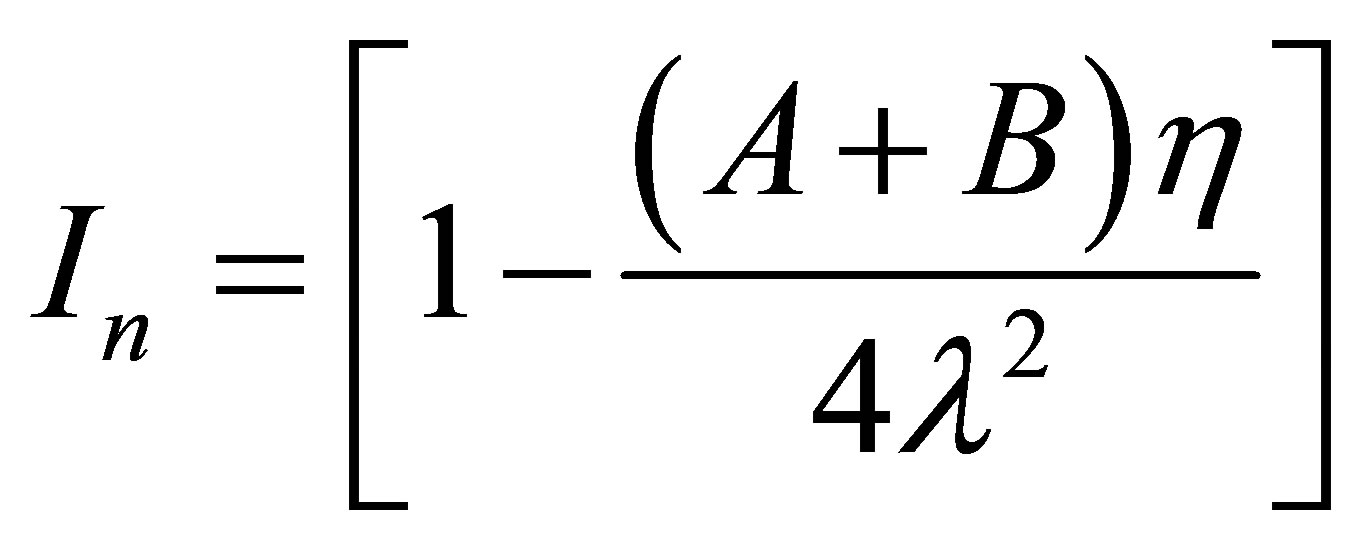 , i.e.
, i.e.
the right peak becomes dominant, 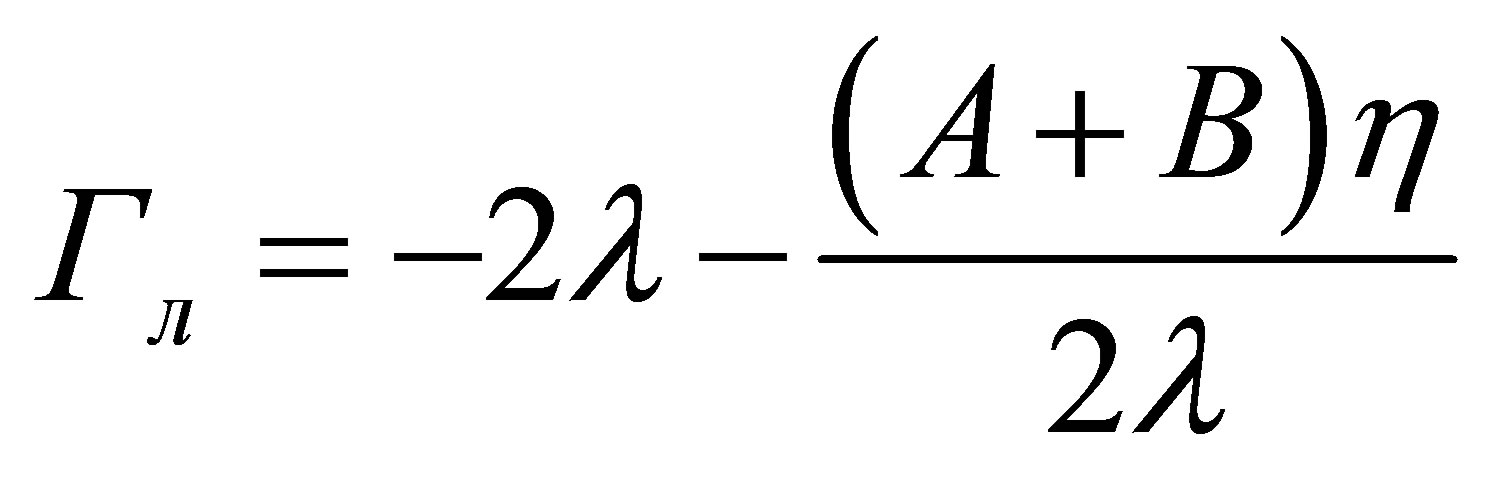 ,
, 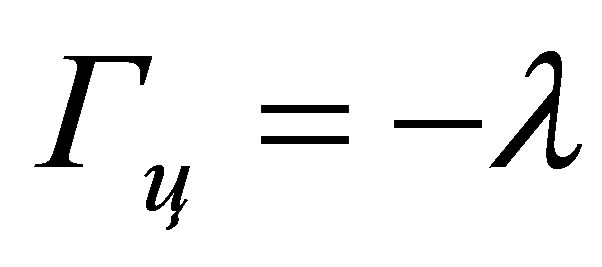 ,
,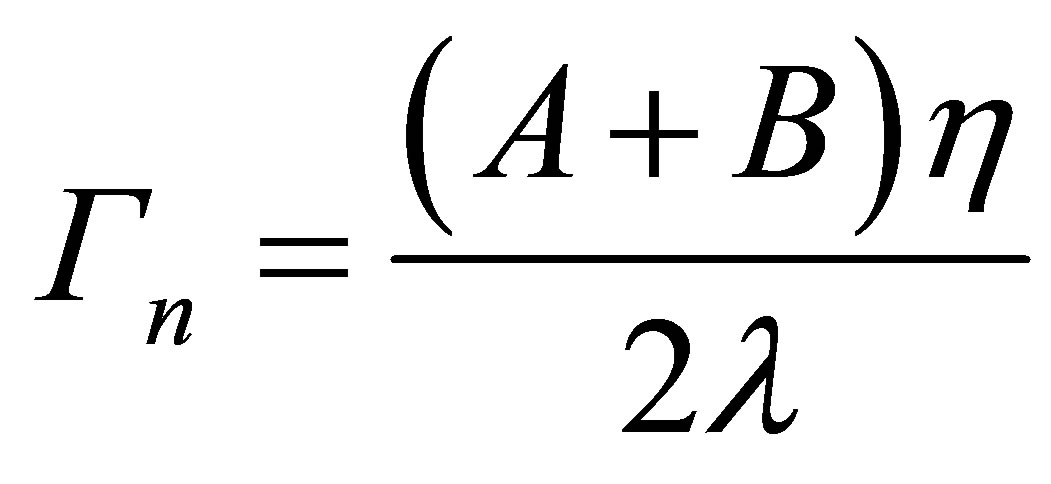 . If
. If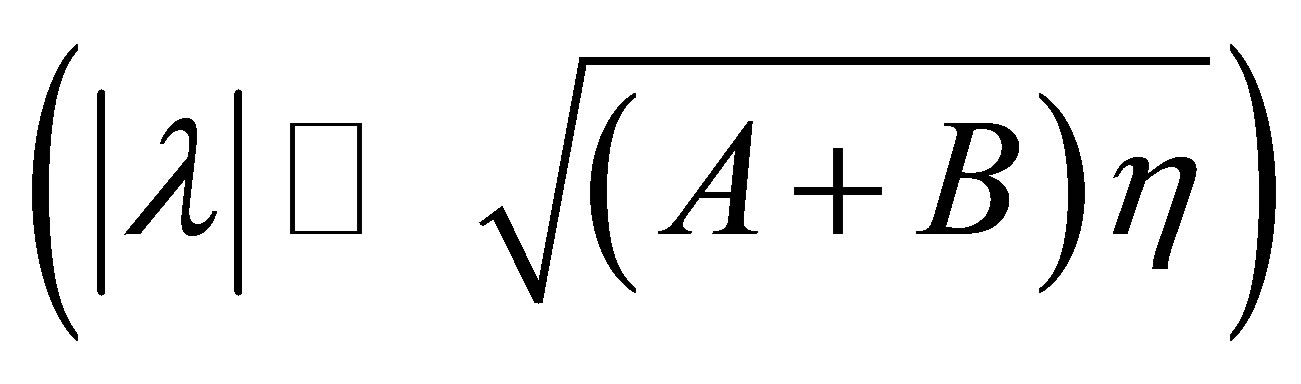
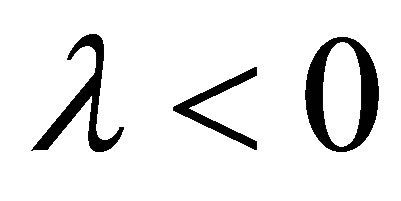 , then the intensity of the left and the right peaks shuffle, and the intensity of the central peak remains unchanged:
, then the intensity of the left and the right peaks shuffle, and the intensity of the central peak remains unchanged:
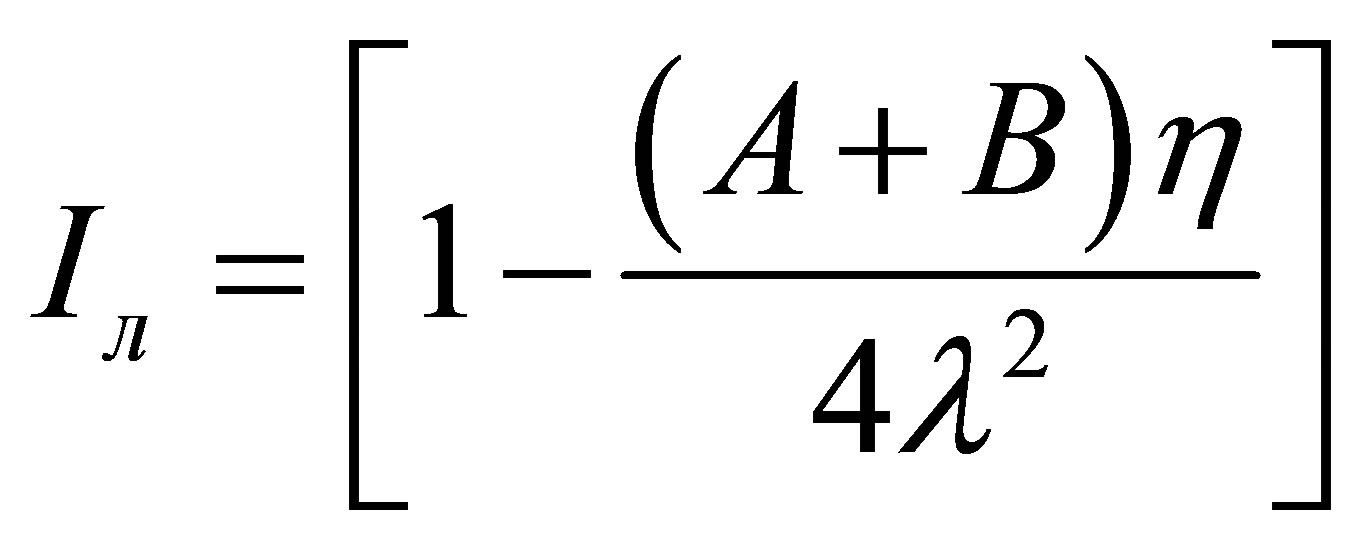 ,
, 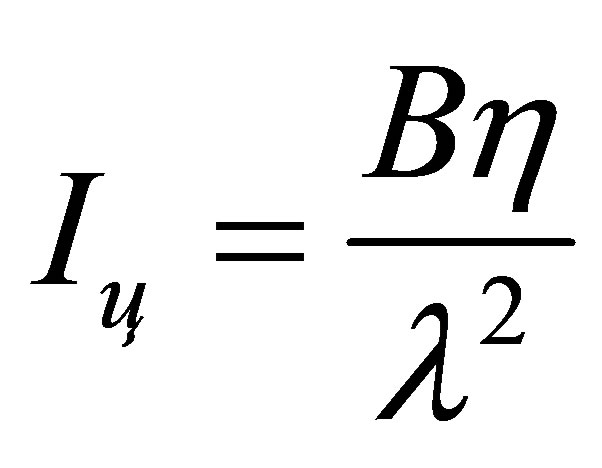 ,
, 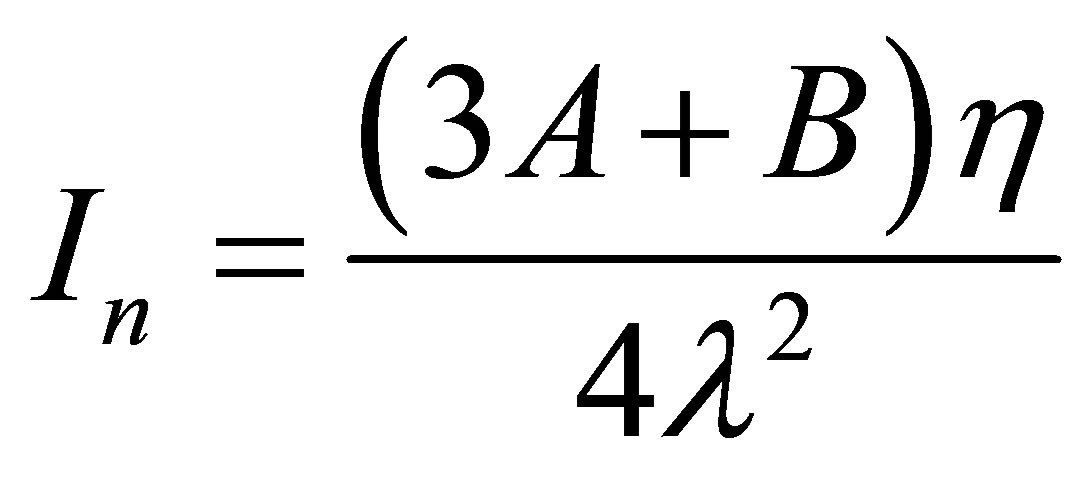 , and
, and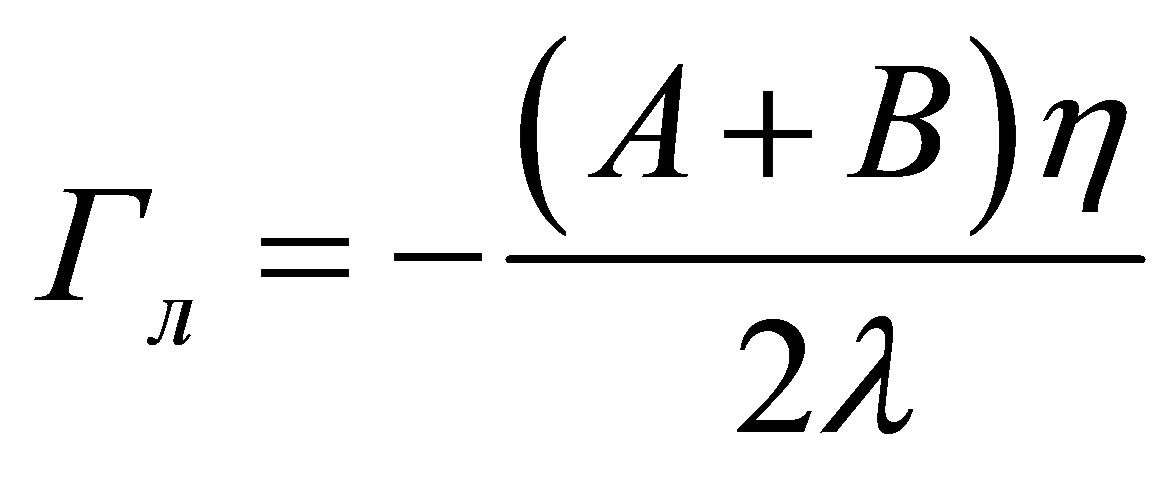 ,
, 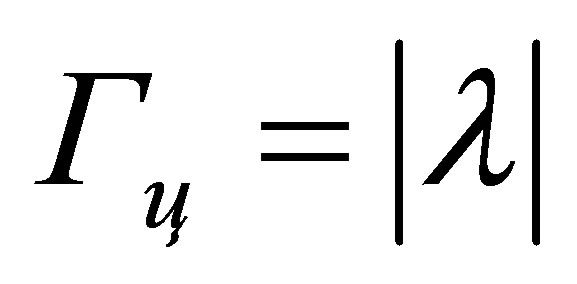 ,
,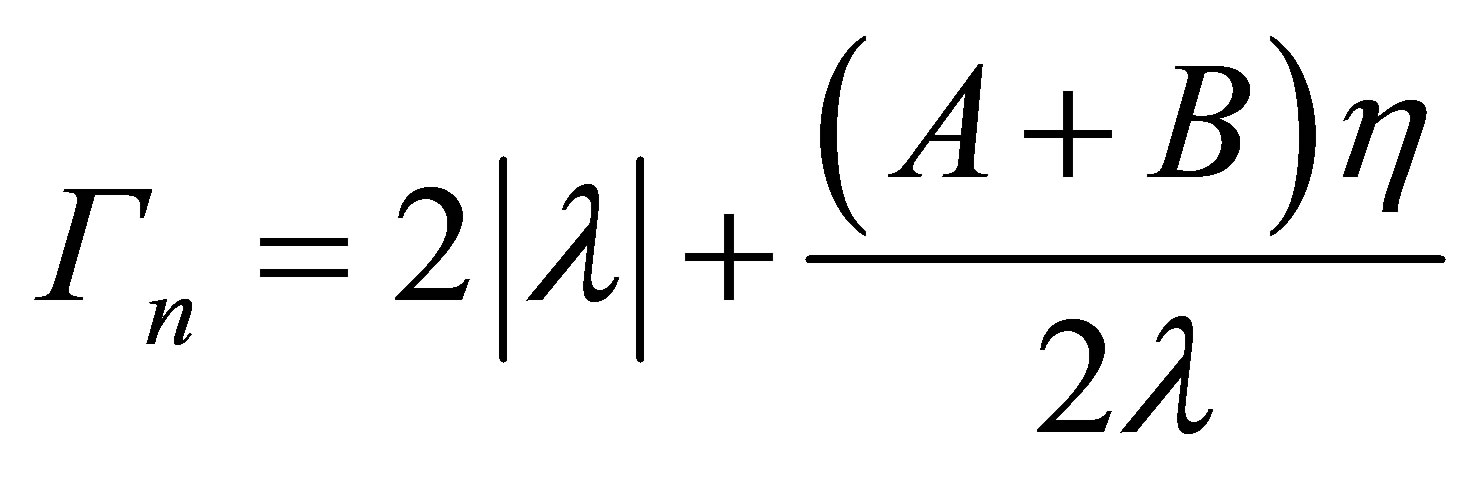 .
.
Above we assumed that the resonant transition with emission of optical phonon occurs between Landau levels, which correspond to dimension-quantized level with zero number l = 0. This limitation is not principled, the difference is just in the magnitude of the matrix element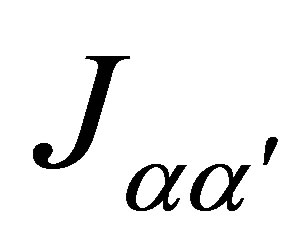 , which determines the magnitude of the levels’ splitting. On the other hand, the real depths of quantum-dimensional potential wells in quantum-dimensional nanostructures are quite enough for formation at least two or three Landau levels, which correspond to one dimensional-quantized sub-level with number l = 0 and space from each other on the magnitude
, which determines the magnitude of the levels’ splitting. On the other hand, the real depths of quantum-dimensional potential wells in quantum-dimensional nanostructures are quite enough for formation at least two or three Landau levels, which correspond to one dimensional-quantized sub-level with number l = 0 and space from each other on the magnitude 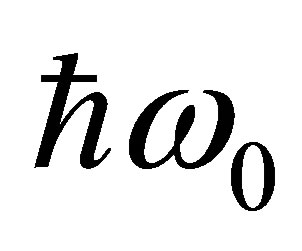 [27].
[27].
If we choose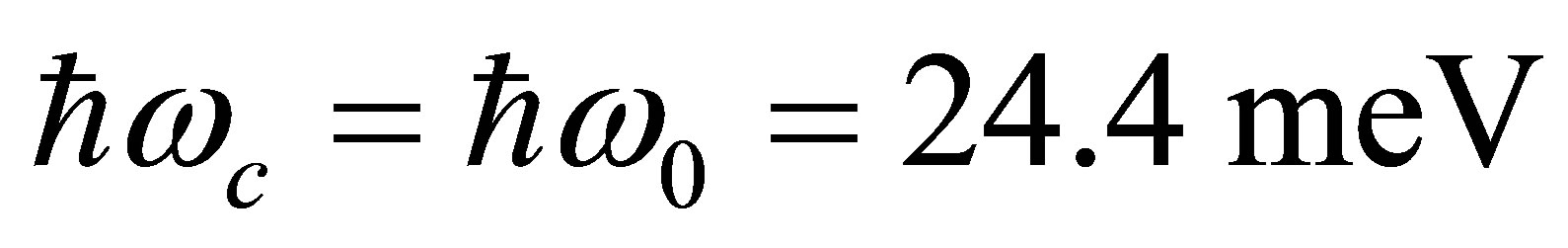 ,
, 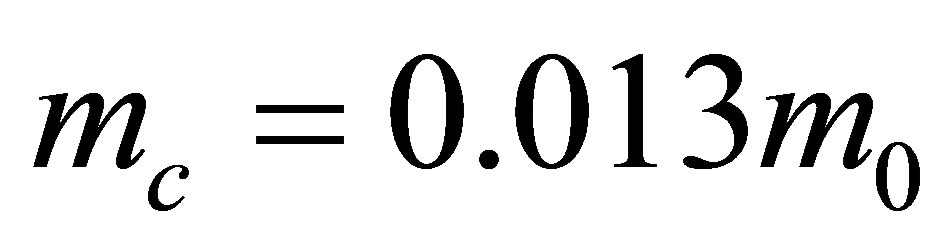 ,
, 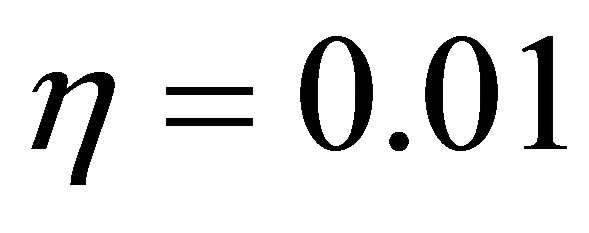 (the chosen parameters correspond to InSb), then
(the chosen parameters correspond to InSb), then 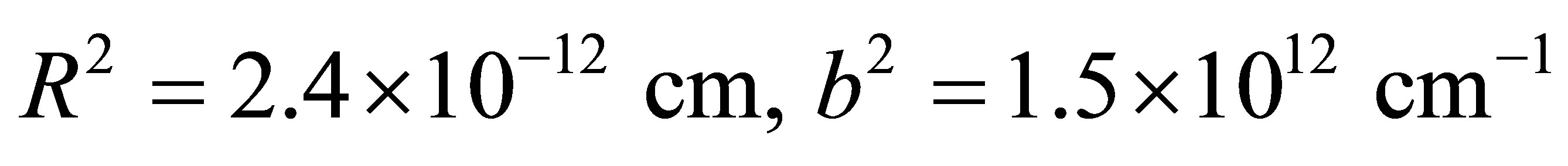 (variation parameter b coincides by order of magnitude with inverse thickness of the quantum-dimensional potential well),
(variation parameter b coincides by order of magnitude with inverse thickness of the quantum-dimensional potential well), 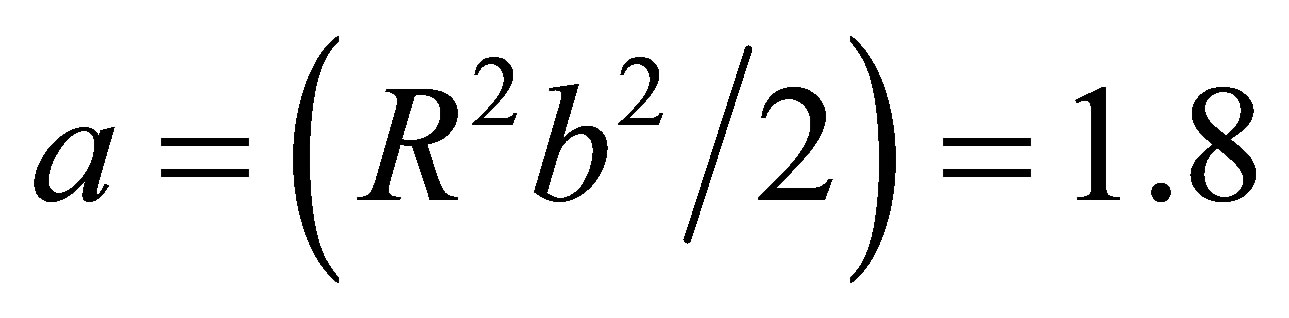 , A = 0.353, B = 0.336. The magnitude of splitting is
, A = 0.353, B = 0.336. The magnitude of splitting is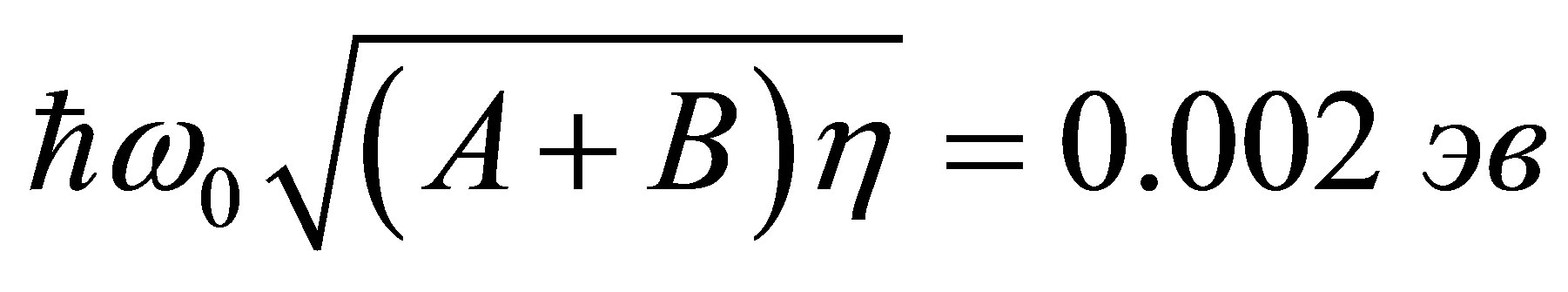 , which is possible to be measured.
, which is possible to be measured.
Since dissipative mechanisms of scattering (for example, by acoustic phonons or impurities) are neglected, the levels of the electronic sub-system are discrete ones, and the lines of absorption appear to be  -like ones. Naturally, in a real situation the lines are broadened and the picture of splitting becomes less pronounced. However, the considered mechanism of scattering due to LO-phonons is sharply non-monotonous one (in contrast with dissipative mechanisms, which depend monotonically on magnetic field and external frequency) and can be extracted on their background.
-like ones. Naturally, in a real situation the lines are broadened and the picture of splitting becomes less pronounced. However, the considered mechanism of scattering due to LO-phonons is sharply non-monotonous one (in contrast with dissipative mechanisms, which depend monotonically on magnetic field and external frequency) and can be extracted on their background.
Strictly speaking, the developed theory is valid if the energy of ionization of exciton in the magnetic field is small as compared with the energy of electron-phonon bonding, and the magnetic field is restricted by condition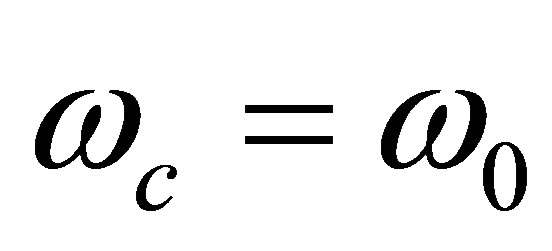 . For example, the ionization energy of exciton in the field H = 3.5 × 104 Oe for GaAs is 1.5 × 10–5 eV [28], and this corresponds to the level n = 0. With the rising number of Landau levels the ionization energy sharply decreases such, for n = 2 it is significantly lesser than above magnitude. On the other hand, splitting effects, as shown above, are 0.002 эВ, since the neglect of exciton states is possible.
. For example, the ionization energy of exciton in the field H = 3.5 × 104 Oe for GaAs is 1.5 × 10–5 eV [28], and this corresponds to the level n = 0. With the rising number of Landau levels the ionization energy sharply decreases such, for n = 2 it is significantly lesser than above magnitude. On the other hand, splitting effects, as shown above, are 0.002 эВ, since the neglect of exciton states is possible.
The work was supported by the fundamental research grant of SCST of the Republic of Uzbekistan (Project No. F2-FK-0-47339-F2-015).
REFERENCES
- E. J. J. Johnson and D. M. Larsen, “Polaron Induced Anomalies in the Interband Magnetoabsorption of InSb,” Physical Review Letters, Vol. 16. No. 15, 1996, pp. 655- 659. doi:10.1103/PhysRevLett.16.655
- D. M. Larsen and E. J. J. J. Johnson, “Polaron Induсed Anomalies in InSb,” Journal of the Physical Society of Japan, Vol. 21, No. 5, 1966, pp. 443-447.
- L. I. Korovin and S. T. Pavlov, “On the Role of Optical Phonons in Interband Megnetooptical Absorption of Semiconductors,” Journal of Experimental and Theoretical Physics, Vol. 53. No. 5. 1967, pp. 1708-1716.
- M. Horst, U. Merkt and J. P. Kotthaus, “Magneto-Polarons in a Two-Dimensional Electron Inversion Layer on InSb,” Physical Review Letters, Vol. 50, No. 10, 1983, pp. 754-757. doi:10.1103/PhysRevLett.50.754
- M. Horst, U. Merkt, W. Zawadzki, J. C. Maan and K. Ploog, “Resonant Polarons in a GaAs-GaAlAs Heterostructures,” Solid State Communications, Vol. 53, No. 4, 1985,pp. 403-405. doi:10.1016/0038-1098(85)90995-0
- D. C. Rogers, R. J. Nicholas, S. Ben Amor, J. C. Portal, A. Y. Cho and D. Sivco, “Inter-Band Magneto-Absorption in a Ga0.47In0.53As-Al0.48In0.52 as Quantum Well,” Solid State Communications, Vol. 60, No. 2, 1986, pp. 83-86. doi:10.1016/0038-1098(86)90534-X
- W. Seidenbuch, “Cyclotron-Resonance Study of Polarons in GaAs-AlxGa1-xAs Heterostructures,” Physical Review B, Vol. 36, No. 4, 1987, pp. 1877-1884.
- L. I. Korovin and S. T. Pavlov, “Eshpulatov B.E. Magnetooptical Absorption of Semiconductor in Surface Layer with Participation of Optical Phonons,” Physics of Solids, Vol. 20, No. 12, 1978, pp. 3594-3598.
- S. Das Sarma and A. Madhukar, “Study of ElectronPhonon Interaction and Magneto-Optical Anomalies in Two-Dimensionally Confined Systems,” Physical Review B, Vol. 22, No. 6, 1980, pp. 2823-2836.
- L. I. Korovin and B. E. Eshpulatov, “Theory of Polaron Effect in Cyclotron Resonance in Two-Dimension Electron Gas,” Physics of Solids, Vol. 29, No. 7, 1987, pp. 1963-1969.
- M. H. Degani and O. Hipolito, “Electron-Interfase-Phonon Interation in GaAs/AlAs and InAs/GaSb Heterostructures,” Surface Science, Vol. 196, No.1-3, 1988, pp. 459- 465. doi:10.1016/0039-6028(88)90726-1
- L. I. Korovin, S. T. Pavlov and B. E. Eshpulatov, “Twoand Three Phonon Resonant Raman Scattering of Light in Quasi-Two-Dimensional Electron Structure in Strong Magnetic Fields,” Physics of Solids, Vol. 35, No. 6, 1993, pp. 1562-1576.
- L. I. Korovin, S. T. Pavlov and B. E. Eshpulatov, “TwoPhonon Resonant Raman Scattering in a QW in a Quantizing Magnetic Field,” Physics of Low-Dimensional Structures, Vol. 4, No. 5, 1994, pp. 83-87.
- I. G. Lang, V. I. Belitsky, A. Cantarera, L. I. Korovin, S. T. Pavlov and M. Cardona, “Magnetopoloron Induced Increase of the Efficiency in Two-LO-Phonon Raman Scattering from Quantum Wells,” Physical Review B, Vol. 54, No. 24, 1996, p. 17768.
- V. Lopez, F. Comas, C. Trallero-Giner, T. Ruf and M. Cardona, “Resonant Electron-Phonon Coupling: Magnetopolaronos in InP,” Physical Review B, Vol. 54. No. 15, 1996, p. 10502.
- I. G. Lang, V. I. Belitsky, A. Cantarera, L. I. Korovin, S. T. Pavlov and M. Cardona, “Triple Magnetopolorons in Quantum Wells,” Physical Review B, Vol. 56. No. 11. 1997, p. 6880.
- L. I. Korovin, I. G. Lang, S. T. Pavlov, “Magnetopolaron States with Participation Confined Phonons in a Semiconductor Quantum Well,” Journal of Experimental and Theoretical Physics, Vol. 115. No. 1. 1999, pp. 187-204.
- L. I. Korovin, I. G. Lang, S. T. Pavlov, “Combined polaron states in magnetooptic effects in a quantum wells,” Journal of Experimental and Theoretical Physics, Vol. 116, No. 4, 1999, pp. 1419-1439.
- L. I. Korovin, I. G. Lang and S. T. Pavlov, “About Influence Phonon Dispertion and Exiton Spectrum on a Energy Spectrum Magnetopolarons in a Quantum Well,” Journal of Experimental and Theoretical Physics, Vol. 118, No. 2, 2000, pp. 388-398.
- I. G. Lang, L. I. Korovin, D. A. Cantareras-Solario and S.T. Pavlov, “Conventional of Magnetopolaron Effect in Reflection and Absorption of Light by Three-Level System in a Quantum Well,” Physics of Solids, Vol. 44, No. 11, 2002, pp. 2084-2096.
- F. Сomas and N. Studart, “Electron-Phonon Interaction in Quantum Dot/Quantum Well Semiconductor Heterostructures,” Physical Review B, Vol. 69, 2004, pp. 235321- 235329.
- I. G. Lang, L. I. Korovin and S.T. Pavlov, “Wave-Functions and Energies of Magnetopolarons in Semiconductor Quantum Wells,” Physics of Solids, Vol. 47, No. 9, 2005, pp. 1704-1710.
- L. I. Korovin, “Splitting of Interband Oscillations of Absorption Coefficient of Light in Magnetic Field,” Physics of Solids, Vol. 13, No. 3, 1971, pp. 842-848.
- L. I. Korovin, B. E. Eshpulatov, “Interband Megnetooptical Absorption by Surface Layer of Semiconductor,” Physics of Solids, Vol. 21, No. 12, 1979, pp. 3703-3712.
- I. S. Gradshtein and I. M. Rijik, “Tables of Integrals, Sums, Series and Products,” Nauka, Moscow, 1971.
- A. S. Davidov, “Quantum Mechanics,” GIFML, Moscow, 1963
- T. Ando, A. Fauler and F. Stern, “Electronic Properties of Two-Dimensional Systems,” Mir, Moscow, 1985.
- S.-R. Eric Yang, L. J. Sham, “Theory of Magnetoexcitons in Quantum Wells,” Physical Review Letters, Vol. 58, No. 24, 1987, p. 2598-2601. doi:10.1103/PhysRevLett.58.2598