Journal of High Energy Physics, Gravitation and Cosmology
Vol.02 No.01(2016), Article ID:62585,9 pages
10.4236/jhepgc.2016.21006
Gedanken Experiment for Degree of Flatness, or Lack of, in Early Universe Conditions
Andrew Walcott Beckwith
Physics Department, Chongqing University Huxi Campus, Chongqing, China

Copyright © 2016 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 19 October 2015; accepted 4 January 2016; published 7 January 2016
ABSTRACT
This document will from first principles delineate the degree of flatness, or deviations from, in early universe models. We will, afterwards, make comparison with recent results we have looked at concerning metric tensor fluctuations and comment upon the role of what early universe gravitational energy may play a role in the presumed deviation from flat space results. Note that 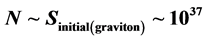 will be tied into the presumed results for initial state density, in ways we will comment upon, leading to observations which are supporting the physics given by Equation (26) of this document as with regards to Gravitational waves, from relic conditions. The deviations from flat space may help confirm the conclusions given by Buchert, Carfora, Kolb, and Wiltshire allegedly refuting the claim by Green and Wald that “the standard FLRW model approximates our Universe extremely well on all scales, except close to strong field astrophysical objects”, as well as give additional analysis appropriate for adding detail to expanding experimental procedures for investigating non FLRW models such as the Polynomial Inflation models as given by Kobayashi, and Seto, as well as other nonstandard cosmologies, as brought up by Corda, and other researchers. As well as improve upon post Bicep 2 measurements which will avoid GW signatures from interstellar dust, as opposed to relic GW. We hope that our approach may help in the differentiation between different cosmology models. Most importantly, our procedure may help, with refinement of admissible frequency range, avoid the problem of BICEP 2, which had its presumed GW signals from presumed relic conditions identical to dust induced frequencies, as so identified by the Planck collaboration in reference [25] which we comment upon in the conclusion.
will be tied into the presumed results for initial state density, in ways we will comment upon, leading to observations which are supporting the physics given by Equation (26) of this document as with regards to Gravitational waves, from relic conditions. The deviations from flat space may help confirm the conclusions given by Buchert, Carfora, Kolb, and Wiltshire allegedly refuting the claim by Green and Wald that “the standard FLRW model approximates our Universe extremely well on all scales, except close to strong field astrophysical objects”, as well as give additional analysis appropriate for adding detail to expanding experimental procedures for investigating non FLRW models such as the Polynomial Inflation models as given by Kobayashi, and Seto, as well as other nonstandard cosmologies, as brought up by Corda, and other researchers. As well as improve upon post Bicep 2 measurements which will avoid GW signatures from interstellar dust, as opposed to relic GW. We hope that our approach may help in the differentiation between different cosmology models. Most importantly, our procedure may help, with refinement of admissible frequency range, avoid the problem of BICEP 2, which had its presumed GW signals from presumed relic conditions identical to dust induced frequencies, as so identified by the Planck collaboration in reference [25] which we comment upon in the conclusion.
Keywords:
HUP, Stress Energy Tensor, Quantum Bounce, Infinite Quantum Statistics, Heavy Gravity

1. Introduction
We will start off first, with a description of the following equation which we will derive in the next section. We discuss the implications of a deviation from flat space, with a description of what  in the aftermath of a quantum bounce implies [1] , and what we should be looking forward in terms of structure formation afterwards. The relevant equation we will be working with is from the time component of the Stress energy Tensor which we will write up as, if
in the aftermath of a quantum bounce implies [1] , and what we should be looking forward in terms of structure formation afterwards. The relevant equation we will be working with is from the time component of the Stress energy Tensor which we will write up as, if  is a statement of volume and if
is a statement of volume and if  for the cosmological “constant”.
for the cosmological “constant”.
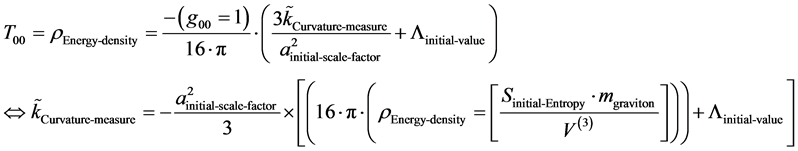 (1)
(1)
In picking this, we are using Ng infinite quantum statistics [1] as a counting factor and likely for 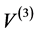 have a Planck length cubed, volume as a starting point, if so then, the mass of the graviton, will be important as well as some considerations given if
have a Planck length cubed, volume as a starting point, if so then, the mass of the graviton, will be important as well as some considerations given if  stays the same, to the present era, or if it has quintessence [2] [3] , a topic we will bring up. In delineating Equation (1) above, we will examine the following from first principle, while keeping in mind that [4]
stays the same, to the present era, or if it has quintessence [2] [3] , a topic we will bring up. In delineating Equation (1) above, we will examine the following from first principle, while keeping in mind that [4]
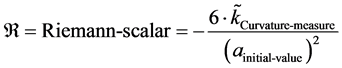 (2)
(2)
Then
 (3)
(3)
If we make the substitution of . The consequences afterwards follow for Equation (1).
. The consequences afterwards follow for Equation (1).
Our supposition is, that if  [1] [5] is used, as well as
[1] [5] is used, as well as 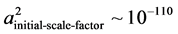 [5] , and
[5] , and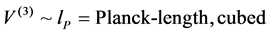 , and
, and  grams [6] then we have an almost but not zero negative value for the
grams [6] then we have an almost but not zero negative value for the 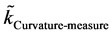 value, we will from here discuss its implications and what it says physically.
value, we will from here discuss its implications and what it says physically.
2. Implications as to Choosing 
First of all, this non zero initial value of the entropy is consistent with a quantum bounce, as can be postulated through LQG, as by [5] [7] but it says more than that. In reality the very small value for the 



Which is a 2nd order perturbative term for the equation for the evolution of h, if 

Then setting a conformal time as approaching early universe conditions requires that

Our supposition is, then that we have the following for well behaved GW and early cosmological perturbations being viable, in the face of cosmological evolution with modifying the formalism of Turok [8] to obtain

In practical terms near the initial expansion point it would mean that near the beginning of cosmological expansion we would have an initial energy density of the order of

If so then, if we assume that gravitons, of initial mass about 10−62 grams, i.e. and that we have Planck mass of about 10−5 grams, if gravitons were the only “information” passed into a new universe, making use of the following expression for the initiation of quantum effects, i.e. by Haggard and Rovelli [7]

Then, we would have, the initiation of quantum effects as of about [8]

Then by making use of Equation (10) we could, by dimensional analysis, start the comparison by setting values from Equation (7) and Equation (10) to obtain

So that to first order, a graviton count, for a radii of about the order of 

Depending upon

Equation (13) would put restrictions upon the following, namely
3. Considerations of What Could Lead to Equation (4), i.e. 2nd Order Perturbation to Cosmological Evolution, Vanishing
The simple short course as to the radius achieving its starting point to being quantum mechanical in its effects, from the big bang initiating from a quantum bounce is to have the following threshold for quantum effects to be in action, to the vanishing of Equation (1). Here the quantum effects start with a value of

If Equation (4) is zero due to 


It means that there is the following interval may be our best Quantum Mechanical perturbative indicator in terms of Equation (4), that is

4. Comparing the Variance in Position Given in Equation (16) with Modified HUP
Note this very small value of x comes from a scale factor, if [9] 

We will next discuss the implications of this point in the next section, of a nonzero smallest scale factor
We will be using the approximation given by Unruh [10] [11] , of a generalization we will write as

If we use the following, from the Roberson-Walker metric [3] [4] .

Following Unruh [5] , write then, an uncertainty of metric tensor as, with the following inputs

Then, if 

5. The Questions of Nonstandard Cosmologies, before Delving into What Our GW Generation Implies
It is noteworthy that, there have been numerous attempts to vet and prove a modification [12] for the Friedman Walker cosmology, which has been correction to the large scale inhomogeneity raised as a possibility by [13] - [17] , as the approach taken by Wald, and Green, a summary can be seen in the statement that as given by [14] that,
“We develop a new, mathematically precise framework for treating the effects of nonlinear phenomena occurring on small scales in general relativity. Our approach is an adaptation of Burnett’s formulation of the “shortwave approximation”, which we generalize to analyze the effects of matter inhomogeneities as well as gravitational radiation. Our framework requires the metric to be close to a “background metric”, but allows arbitrarily large stress-energy fluctuations on small scales”.
In the case of [12] the statement is that
“The large-scale homogeneity and isotropy of the universe is generally thought to imply a well defined background cosmological model. It may not. Smoothing over structure adds in an extra contribution, transferring power from small scales up to large. Second-order perturbation theory implies that the effect is small, but suggests that formally the perturbation series may not converge”.
We have the situation in defining 


Having said that, the issues of the nature of determining if there is or not if there are conditions allowing for quantization in the genesis of GR, as given by [18] in the quotation that
“On the other hand, one can define Extended Theories of Gravity those semiclassical theories where the Lagrangian is modified, in respect to the standard Einstein-Hilbert gravitational Lagrangian, adding high-order terms in the curvature invariants (terms like


In Equation (22), inputs into the terms





“the curvature invariants (terms like

do not play a large role, and that we do not have to talk about extended gravity. If Equation (1) is not small, then it is likely that extended gravity will have to be taken seriously with contributions to the Curvature, and the Lagrangian as seen for Equation (2) have to be painstakingly calculated. Having said that, let us now consider the matter of Gravity waves, and their implications
6. The Matter of GW, as Ascertained through Reference [19] plus Distinguishing between More Cosmologies than Just Extended Gravity
We start off with a quote from [19] which neatly summarizes up the interesting issues of GW research we should keep in mind;
“Omni-directional gravitational wave background radiation could arise from fundamental processes in the early Universe, or from the superposition of a large number of signals with a point-like origin. Examples of the former include parametric amplification of gravitational vacuum fluctuations during the inflationary era, termination of inflation through axion decay or resonant preheating, Pre-Big Bang models inspired by string theory, and phase transitions in the early Universe; the observation of a primordial background would give access to energy scales of
First off, are we considering contribution from a multitude of point like origins for GW generation, or do we have fundamental processes in the early universe to consider? We are trying to obtain GW which are Primordial, in origin. Not only is the above, as alluded to in the quote, there is one other complication which is in the next paragraph, namely [20] has, in page 66 the datum that if there exist an inflaton field or fields, that as stated
“The bubble like structure of the Fields is reflected in their statistics. Perhaps more surprisingly, the statistics of both fields remain non-gaussian for a long time after preheating. At the end of our simulation, at t = 300 m the fields were noticeably non Gaussian.”
As stated in [20] this leads to a rapid increase in turbulent interacting scalar waves. i.e. one could, unless we are very, very careful still, even if we have a primordial signal, have through the turbulence, due to preheating a stochastic background, and we go through the needle in a haystack problem with a vengeance. The author in [21] wrote also that the problem is complicated by the following
In the section called “technical problems which need to be addressed in order to improve the quality of research for relic signals” the author wrote in [21]
An important, direct connection between the strain of relic gravitational waves and the inflaton field has been released by Dr. Corda [22] as far as the formula he derived for an inflaton and inputs of strain upon the inflaton field. This was given by Dr. Corda as [22]

Here, H is given as the evolving Hubble parameter, and 








The upshot with the frequency, to this range, 

7. Conclusions
Equation (21) may, with refinements of r = x, in the four-dimensional Volume, give the new HUP, in our problem, its impact upon GW generation and its relevance to Bicep 2, the search for validation of nonstandard cosmologies, and GW searches.
If from Massimo Giovannini [23] we can write

Refining the inputs from Equation (26) means more study as to the possibility of a non zero minimum scale factor, as well as the nature of 
use 


This Equation (19) will be put into



nifying the spatial range of x for which quantum mechanics is valid, with three times that value connected as to when the perturbative methods break down. Thereby influencing the range of values for 

gravitational spin off according to


If the contribution from Pre-Planckian to Planckian is due to the stress energy tensor as given in 


The importance of Equation (29) is in giving a compliment to [23] as to the problem of relic gravitational waves, which the author views, is extremely important. A correct rendering of Equation (25) would be to determine additional experimental constraints which may determine if detection of early universe gravitational waves is feasible with LIGO technology, or if there is a requirement for other detectors. Certainly, what is wished by this inquiry is to avoid the problems associated with BICEP 2, through a refinement of methods as given in [24] . In addition, of special note would be to avoid picking up interstellar dust effects upon Gravitational waves, which has been a primary reason for the development of methodologies as given in [25] . Note also that BICEP2 only observed in one wavelength, which made it difficult for them to prove the B-modes they saw were truly from gravitational waves. Ascertaining Equation (29) properly, may help alleviate that problem.
Note that also the value of a correct rendering of Equation (29) would be to ascertain the axial tilt as would be expected in early universe cosmology, and relic Gravitational waves, with greater precision than which showed up in the BICEP 2 results.
This value of Equation (29) would have, as its origins, the near flat space physics given by Equation (1) as its genesis with this to consider, as the start [5] .

Equation (30), if confirmed experimentally, is of potential decisive importance to the problem of discriminating between different cosmology models. Note in the case of Bicep 2 the Planck collaboration had that the frequency of dust signals was about the same as what was reported by Bicep 2 presumed gravitational waves.
Hence, the conclusion is inescapable. The value of the flatness calculation as of Equation (1) and of getting a range of

There are some other issues to consider in addition to avoiding having presumed GW frequency being the same as for dust induced signals.
As [27] has brought up, the presence of massive gravity, which is a byproduct of the derivation of this paper, and in particular as stated in [26] “the solution for the massive mode arising from the Starobinsky’s high order gravity theory” may be akin to the problems of not only the round off done in Equation (26) but also of giving further meaning to the physics of Equation (4) and Equation (5), as brought up by the author. This is a topic which the author will investigate on his own in the next sequels to this document. Note that [28] has a distinct nonlinear component as to gravity, and so does this document, especially in the spin offs of Equation (4) and Equation (5).
Further refinements may be due to [5] [29] where we consider as given in [30] details of a quantum bounce which may give more information, as well as additional investigations into what Turok brought up [3] . The issues as to Equation (30) and what they imply are quite different from [30] for reasons we will go into in a future publication.
Acknowledgements
This work is supported in part by National Nature Science Foundation of China grant No. 11375279.
Cite this paper
Andrew WalcottBeckwith, (2016) Gedanken Experiment for Degree of Flatness, or Lack of, in Early Universe Conditions. Journal of High Energy Physics, Gravitation and Cosmology,02,57-65. doi: 10.4236/jhepgc.2016.21006
References
- 1. Jack Ng, Y. (2007) Holographic Foam, Dark Energy and Infinite Statistics. Physics Letters B, 657, 10-14. http://dx.doi.org/10.1016/j.physletb.2007.09.052
- 2. Will, C. (2015) Was Einstein Right? A Centenary Assessment. In: Ashtekar, A., Berger, B., Isenberg, J. and MacCallum, M., Eds., General Relativity and Gravitation, A Centennial Perspective, Cambridge University Press, Cambridge, UK, 49-96. http://dx.doi.org/10.1017/cbo9781139583961.004
- 3. Will, C. (2014) The Confrontation between General Relativity and Experiment. http://relativity.livingreviews.org/Articles/lrr-2014-4/download/lrr-2014-4Color.pdf
- 4. Katti, A. (2013) The Mathematical Theory of Special and General Relativity. CreateSpace Independent Publishing, North Charleston.
- 5. Beckwith, A. (2015) Geddanken Experiment for Refining the Unruh Metric Tensor Uncertainty Principle Via Schwartzshield Geometry and Planckian Space-Time with Initial Non Zero Entropy and Applying the Riemannian- Penrose Inequality and the Initial Kinetic Energy. http://vixra.org/abs/1509.0173
- 6. Goldhaber, A. and Nieto, M. (2010) Photon and Graviton Mass Limits. Reviews of Modern Physics, 82, 939-979. http://arxiv.org/abs/0809.1003 http://dx.doi.org/10.1103/RevModPhys.82.939
- 7. Haggard, H.M. and Rovelli, C. (2014) Black, Hole Fireworks: Quantum Gravity Effects outside the Horizon Spark Black to White Hole Tunneling. http://arxiv.org/abs/1407.0989
- 8. Turok, N. (2015) A Perfect Bounce. http://www.researchgate.net/publication/282580937_A_Perfect_Bounce
- 9. Kolb, E.W. and Turner, M.S. (1990) The Early Universe. Addison-Wesley Publishing Company, The Advanced Book Program, Redwood City.
- 10. Unruh, W.G. (1986) Why Study Quantum Theory? Canadian Journal of Physics, 64, 128-130. http://dx.doi.org/10.1139/p86-019
- 11. Unruh, W.G. (1986) Erratum: Why Study Quantum Gravity? Canadian Journal of Physics, 64, 1453. http://dx.doi.org/10.1139/p86-257
- 12. Adamek, J., Clarkson, C., Durrer, R. and Kunz, M. (2015) Does Small Scale Structure Significantly Affect Cosmological Dynamics? Physical Review Letters, 114, Article ID: 051302. http://arxiv.org/abs/1408.2741 http://dx.doi.org/10.1103/PhysRevLett.114.051302
- 13. Buchert, T., Carifora, M., Kolb, E. and Wilkshire, D. (2015) Is There Proof That Backreaction of Inhomogeneities Is Irrelevant in Cosmology? Quantum Gravity, 32, Article ID: 21521. http://arxiv.org/abs/1505.07800,15,Oct,2015,Class
- 14. Green, S.R. and Wald, R.M. (2011) A New Framework for Analyzing the Effects of Small Scale Inhomogeneities in Cosmology. Physical Review D, 83, Article ID: 084020. http://arxiv.org/abs/1011.4920
- 15. Green, S.R. and Wald, R.M. (2012) Newtonian and Relativistic Cosmologies. Physical Review D, 85, Article ID: 063512. http://arxiv.org/abs/1111.2997
- 16. Green, S.R. and Wald, R.M. (2013) Examples of Backreaction of Small Scale Inhomogeneities in Cosmology. Physical Review D, 87, Article ID: 124037. http://arxiv.org/abs/1304.2318
- 17. Green, S.R. and Wald, R.M. (2014) How Well Is Our Universe Described by an FLRW Model? Classical and Quantum Gravity, 31, Article ID: 234003. http://arxiv.org/abs/1407.8084
- 18. Corda, C. (2009) Interferometric Detection of Gravitational Waves: The Definitive Test for General Relativity. International Journal of Modern Physics D, 18, 2275-2282. http://arxiv.org/abs/0905.2502 http://dx.doi.org/10.1142/S0218271809015904
- 19. Van Den Broeck, C. (2015) Gravitational Wave Searches with Advanced LIGO and Advanced Virgo. LIGO Document Number P1500064. http://arxiv.org/abs/1505.04621
- 20. Kofman, L. (2008) Preheating after Inflation. In: Lemoine, M., Martin, J. and Peter, P., Eds., Inflationary Cosmology (Lecture Notes in Physics 738), Springer Verlag, Heidelberg, 55-78.
- 21. Beckwith, A. (2011) Detailing Minimum Parameters for the Red Shift, Frequency, Strain, and Wavelength of Gravitational Waves/Gravitons, and Impact upon GW Astronomy for Experimentally Falsifiable Measurements to Evaluate Current Models of Cosmology. Apeiron, 18, 321-351
- 22. Corda, C. (2010) Information on the Inflaton Field from the Spectrum of Relic Gravitational Waves. General Relativity and Gravitation, 42, 1323-1333. http://dx.doi.org/10.1007/s10714-009-0895-6
- 23. Giovannini, M. (2008) A Primer on the Physics of the Cosmic Microwave Background. World Press Scientific, Hackensack.
- 24. Das, S. and Souradeep, T. (2014) SCoPE: An Efficient Method of Cosmological Parameter Estimation. http://arxiv.org/abs/1403.1271
- 25. Li, S.Y., Xia, J.Q., Li, M.Z., Li, H. and Zhang, X.M. (2015) Primordial Gravitational Waves Measurements and Anisotropies of CMB Polarization Rotation. http://arxiv.org/pdf/1506.03526.pdf
- 26. Planck Collaboration, Adams, R., et al. (2014) Planck Intermediate Results. XXX. The Angular Power Spectrum of Polarized Dust Emission at Intermediate and High Galactic Latitudes. http://arxiv.org/abs/1409.5738
- 27. Corda, C. (2008) Massive Gravitational Waves from the R2 Theory of Gravity: Production and Response of Interferometers. International Journal of Modern Physics A, 23, 1521-1535. http://arxiv.org/abs/0711.4917 http://dx.doi.org/10.1142/S0217751X08038603
- 28. Starobinsky, A.A. (1980) A New Type of Isotropic Cosmological Models without Singularity. Physics Letters B, 91, 99-102. http://dx.doi.org/10.1016/0370-2693(80)90670-X
- 29. Rovelli, C. and Vidotto, F. (2015) Covariant Loop Quantum Gravity: An Elementary Introduction to Quantum Gravity and Spinfoam Theory. Cambridge University Press, Cambridge.
- 30. Galloway, G., Miao, P. and Schoen, R. (2015) Initial Data and the Einstein Constraints. In: Ashtekar, A., Berger, B., Isenberg, J. and MacCallum, M., Eds., General Relativity and Gravitation, A centennial Perspective, Cambridge University Press, Cambridge, UK, 412-448.

