Received 10 October 2014; revised 12 November 2014; accepted 19 November 2014

ABSTRACT

The equations of Euler-Lagrange elasticity describe elastic deformations without reference to stress or strain. These equations as previously published are applicable only to quasi-static deformations. This paper extends these equations to include time dependent deformations. To accomplish this, an appropriate Lagrangian is defined and an extrema of the integral of this Lagrangian over the original material volume and time is found. The result is a set of Euler equations for the dynamics of elastic materials without stress or strain, which are appropriate for both finite and infinitesimal deformations of both isotropic and anisotropic materials. Finally, the resulting equations are shown to be no more than Newton's Laws applied to each infinitesimal volume of the material.

Keywords:

Elasticity, Stress, Strain, Infinitesimal Deformations, Finite Deformations, Discrete Region Model

1. Background

Virtually all modern theories of elasticity [1] - [4] build the equations to describe elasticity using stress and/or strain. Hardy [5] proposed to return to the approach of Euler, Lagrange, and Poisson [6] to build the equations of elasticity using point locations and forces instead of stress and strain. Hardy called these equations the equations of Euler-Lagrange elasticity. The equations of Euler-Lagrange elasticity are appropriate for quasi-static defor- mations, but do not include dynamics. Dynamics will be added in this paper.

Hardy defined an elastic material as one which when deformed, stores energy; and when it is returned to its original state, the stored energy is returned to its surroundings. This is known as hyper-elasticity [7] . Hardy followed the notation of Spencer [8] by defining the initial position of each point in an elastic material to be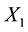, , 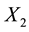and 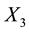corresponding to the x, y, and z coordinates of that point. The parameters, 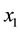, , 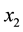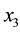were defined as the x, y, z coordinates of the corresponding point after the deformation. The final position of each point depends upon the initial position, so that each component of each point, , is a function of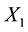, , 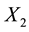and. The energy of the material is a function of the final positions of each point 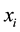(i = 1, 2, 3) and the

relative change in distances between points, 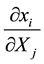(i and j = 1, 2, 3). This energy is expressed in terms of the energy per unit original volume, 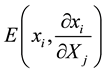, which can be divided into the energy associated with body forces, 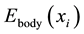, plus the energy associated with the deformation of the body, ,

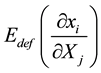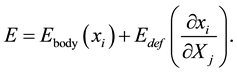(1)

To obtain the Euler-Lagrange differential equations, Hardy minimized the total energy, ,

, (2)

which resulted in three Euler equations,

(3)

The advantage of Hardy’s approach is that Equation (3) is applicable to both infinitesimal and finite defor- mations as well as being appropriate for both anisotropic and isotropic materials. The disadvantage of this approach is that it is only appropriate for quasi-static deformations, since time dependence is not included. In this paper, I will extend this approach to include dynamics.

2. Adding Dynamics

To add dynamics to the Euler-Lagrange elasticity equations several changes are needed to the quasi-static approach. First define each as a function of time as well as, , and. Second define an appro- priate Lagrangian. Third minimize the integral of the Lagrangian over both space and time. Lagrangians for particle dynamics are defined as the kinetic energy minus the potential energy of the particle. To extend this to a distributed material, our “particle” will be an infinitesimal volume of the elastic material. Define the kinetic energy per original volume of the material as

, (4)

with the mass per original volume of the material and the velocity of any point in the material, , is

(5)

Define the potential energy per unit original volume as in Equation (1) and the Lagrangian, as

(6)

Substitute Equation (1) into Equation (6) with and T from Equation (4) to express as

(7)

Now find the extrema of

(8)

Since, the following three Euler equations result from setting:

(9)

Substituting from Equation (7) gives

, (10)

or

(11)

Equation (11) are the equations of dynamics for deformation of elastic materials. All that is required is to define of the material experimentally. The must be invariant under coordinate rotations and translations. One method is to define in terms of invariants of the matrix (e.g. Ogden [9] , Hardy [10] ).

Note that no assumptions of infinitesimal deformation or isotropy have been made to derive Equation (11), so they are applicable for both infinitesimal and finite deformations of both isotropic and anisotropic materials. The most surprising thing about Equation (11) is that each term in Equation (11) can be given a simple physical interpretation.

3. Physical Interpretation of the Terms in Equation (11)

In order to give a physical interpretation to the individual terms in Equation (11) consider a small cuboid defined as. The term on the left hand side of Equation (11), , is the change in momentum per unit original volume of this cuboid with respect to time in the limit as, and approach 0. The first term on the right hand side, , is the force of gravity per unit original volume of this cuboid in the same limit. The second term on the right hand side, , is shown below to be the net surface force per unit original volume applied to all the surfaces of the cuboid as the volume of the cuboid shrinks to zero. In other words, Equation (11) is just an expression of Newton’s laws for each infinitesimal volume of the material.

To see that is indeed the net surface force per unit original volume acting on the cuboid, recall that Hardy [5] found that the external force acting on a surface can be written as

(12)

Let represent a particular plane during deformation, where the magnitude of is the current infinitesimal area of the plane and the direction of is perpendicular to the plane of interest and pointing away from the material receiving the force. To calculate the force on this plane using Equation (12), find the original magnitude and direction of before the deformation. Call this. Define the components of be, , and in the, , and directions respectively. The three components of the force exerted on the plane at any time during the deformation are then calculated from Equation (12) as

(13)

For our cuboid, defined as, the component of the force on a plane of the cuboid originally perpendicular to is, where

. (14)

For example, is the component of the force on plane. Divide the body into cuboids along the direction as shown in Figure 1(a). As shown in this figure, is the component of force on region a from region b in the direction. is the component of force on region b from region c. If we wish to express the net force on region b alone, this would be as shown in Figure 1(b). The net force in the direction on region b along the direction when divided by the cuboid’s original volume is

(15)

Taking the limit as the dimensions of the cube go to zero gives the net force per unit original volume on region b in the direction on the faces of the cube, , to be

(16)

A similar argument using and yields the net forces normal to the and faces, and, to be

, (17)

and

(18)

Next consider. Using Figure 2 and an argument similar to the one used in Figure 1 gives

, (19)

and in general

(20)

Combining these results, we have the total force in the direction to be

, (21)

for i = 1, 2, 3, and summed over j = 1, 2, 3, which is the third term in Equation (11). Thus

is the net surface force per unit original volume in the direction on any cuboid in the limit as the cuboid dimensions shrink to zero.

Figure 3 summarizes this result by illustrating the forces summed in each direction to calculate the net surface force on a cuboid of material. Note that in Figure 3 only the forces on the “front” faces of the cuboid are

shown. There are forces on the rear surfaces that also contribute to each term.

4. Some Details

The procedure outlined in the last section to calculate the force on a plane after a deformation seems a bit convoluted in that the location of the plane before any deformation must be found in order to find the force on the plane after deformation. However, Equation (12) are excellent for applying Neumann boundary conditions to Equation (11). As an example, consider the case of deforming a rectangular body as shown in Figure 1(a) by applying some force on the face of the cuboid. If we know the components of the applied force from boundary conditions as a function of time, we can write

(22)

If the force is applied uniformly over the area, is simply the applied force divided by a constant, the origial area. Therefore the Neumann boundary condition using Equation (12) is defined using just a rescaled version of the applied force on the surface of the material.

Finite deformations may displace and distorted planes in the cuboid from their original positions, but as long as inversions are not allowed, the same bounding surfaces of the cuboid are found regardless of how the material is deformed. The values of change from point to point as the material is deformed, but the vectors are unchanged by the deformation. Thus the forces shown in Figures 1-3 may be displaced due to the finite deformation, but the orientation of each component of each force from each surface is the same and the form of the sum of the forces, , is unchanged by the displacement.

Lastly, it is tempting to consider the second order tensor quantity to be stress, but it is only stress for infinitesimal deformations. This is because must be multiplied by the orignal surface vector, not the current one to get the force at the current location.

5. Conclusion

The equations for dynamics in Euler-Lagrange elasticity have been derived. These equations are shown to be a simple statement of Newton’s Law for each infinitesimal volume of the material. The derived equations, Equation (11), are applicable to infinitesimal and finite deformations for both isotropic and anisotropic materials.

References