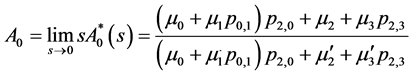Received 29 February 2016; accepted 18 July 2016; published 21 July 2016

ABSTRACT

The service facility or server is the key constituent to keep a system operational for desired period of time. As any eventuality with the system necessitates immediate presence of it (server) so the time point of arrival and treatment of server significantly affects the system performance. This paper works out the steady state behavior of a cold standby system equipped with two similar units and a server with elapsed arrival and treatment times following general probability distributions. It practices the theory of semi-Markov processes, regenerative point technique and Laplace transforms to derive the expressions for state transition probabilities, mean sojourn times, mean time to system failure, system availability, server busy period and expected frequencies of repairs and treatments. The profit function is also developed taking different costs and revenue in to account. For tracing wider applicability of the model for different reliability and cost-effective systems, a particular case study is also presented as an illustration.

Keywords:

Stochastic Model, Cold-Standby System, Server Failure, Regenerative Point, Arrival and Treatment Times

1. Introduction

The reliability analysis is an essential practice for the installations where failure may turn out hazardous either in terms of huge financial loss or threat to human life. These causes inspired the literature to a greater extent [1] - [8] . Further, with the pioneer works of Smith [9] and Pyke [10] , the use of semi-Markov regenerative processes became popular for developing reliability models of probabilistic systems [11] - [14] . To assure stable system performance both in terms of reliability as well as availability, the proviso of standby redundancy is widely considered in the literature [15] - [19] . The repairable systems are described with the feature that as soon as any component/unit fails it is either repaired or replaced by the service facility. So the service facility plays a key role in keeping a repairable system operational for longer period of time. In such cases, the failure of service facility interrupts the system performance in terms of availability, reliability and profit [20] - [22] . So the modeling of arrival and treatment times of server becomes essentially important to marginalize the loss due to system down time. Keeping these facts in view, the present paper investigates a cold-standby system taking account of waiting time for arrival and treatment of server subject to failure. The semi-Markov processes and regenerative point technique are used to obtain following measures of system performance in steady state:

a) Transition probabilities and mean sojourn times in different states.

b) MTSF and reliability of the system.

c) System availability.

d) Server busy period.

e) Expected number of repairs and treatments.

f) And expected profit.

2. System Assumptions & States Description

2.1. Assumptions

To provide ease to the computational work, the model is developed using the following set of assumptions:

a) The model consists of two identical units. Initially, one unit is in operation and another as cold-standby.

b) The unit in standby mode can’t fail.

c) Upon failure of the operative unit the standby becomes operative instantly.

d) All the failures are repairable to be repaired by the server but the server takes some time to arrive.

e) The server may fail while working but curable.

f) The server restoration subjects to treatment with some elapsed time.

g) All the repairs, treatments and switching are perfect.

h) The system works as long as at least one unit remains working.

i) All the random variables are assumed to be statistically independent.

j) All the random variables follow general probability distribution with different distribution functions.

2.2. States of the System

The system model comprises of regenerative and non-regenerative states. The states 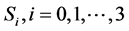are regenerative whereas the states 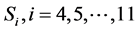are non-regenerative. The detailed description of all possible states is as follows:

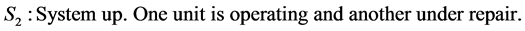
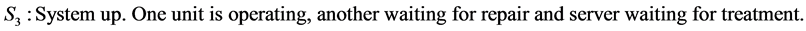
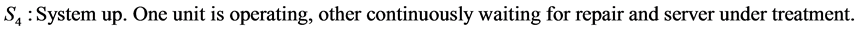
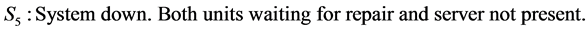
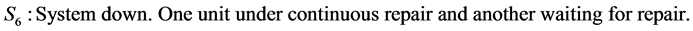
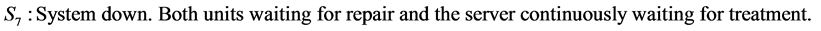
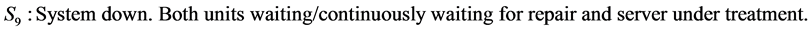
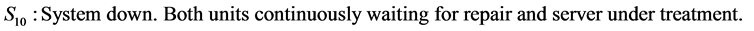
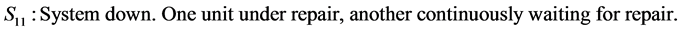
3. Notations & Acronyms

: pdf/cdf of failure time of the unit.

: pdf/cdfof failure time of the server.

: pdf/cdf of repair time of the failed unit.

: pdf/cdf of the treatment time of the server.

: pdf/cdf of the waiting time of the server for treatment.

: pdf/cdf of the arrival time of the server.

: pdf/cdf of direct transition time from a regenerative state i to a regenerative state j without visiting any other regenerative state.

: pdf/cdf of first passage time from a regenerative state i to a regenerative state j or to a failed state j visiting state k once in (0, t].

: pdf/cdf of first passage time from regenerative state i to a regenerative state j or to a failed state j visiting state k, r once in (0, t].

: pdf/cdf of first passage time from regenerative state i to a regenerative state j or to a failed state j visiting state k, r and s once in (0, t].

: Probability that the server is busy in the state S_i up to time ‘t’ without making any transition to any other regenerative state or returning to the same state via one or more non-regenerative states.

: Contribution to mean sojourn time (µ_i) in state S_i when system transit directly to state j.

: Contribution to mean sojourn time (µ_i) in state S_i when system transit to state j via k and n times between

(s)/(c): Stieltjes convolution/Laplace convolution.

: Laplace Stieltjes Transform (LST).

Laplace Transform (LT).

: Inverse Laplace Transform.

4. The Model Development

4.1. The State Transition Diagram

Taking account of all possible transitions and the re-generative points a system schematic state transition diagram is constructed as given in Figure 1. The solid dots denote the regenerative epochs for various states of the model. The probability density functions of various random variables are also shown in Figure 1.

4.2. State Transition Probabilities

Simple probabilistic considerations, yields the following expressions for the non-zero elements

(1)

For these Transition Probabilities, it can be verified that

4.3. Mean Sojourn Times

The Mean sojourn time µ_i in state S_i are given by:

(2)

The unconditional mean time taken by the system to transit from any state S_i when time is counted from epoch at entrance into state S_j is stated as:

5. Stochastic Analysis

5.1. Reliability Measure

Let be the c.d.f of the first passage time from regenerative state S_i to a failed state. Regarding the failed state as absorbing state, we have the following recursive relations for:

(3)

where S_j is an un-failed regenerative state to which the given regenerative state S_i can transit and S_k is failed state to which the state S_i can transit directly.

Taking LST of Equation (3) and solving for, we get MTSF as follows:

The reliability R(t) is given by

5.2. Economic Measures

Let the system entered the regenerative state S_i at t = 0. Considering S_j as a regenerative state to which the given regenerative state S_i transits, the recursive relations for various profit measures in (0, t] are given as follow:

(4)

(5)

(6)

(7)

Here,

And

Using LT/ LST, of Equations (4)-(7) and solving we get the results in steady state as below:

Further, using the values of above performance measures, the profit incurred to the system model in steady state is given as below.

6. Example (Particular Case of Exponential Distribution)

For the sake of convenience, let us suppose all the random variables follow the exponential distribution with the probability density functions given below.

We assume some particular values for various time rates and costs i.e.

Failure rate of server (g) = 0.02 per unit time, Failure rate of unit (λ) = 0.008 per unit time.

Repair rate of unit (α) = 0.3 per unit time, Treatment rate of server (β) = 0.05 per unit time.

Server arrival rate (y) = 0.08 per unit time, Waiting treatment time (x) = 0.08 per unit time.

And.

For this, we obtained the values for different measures of system performance as follows:

MTSF = 1110.909 unit time, Availability = 0.977238, Busy period of server = 0.023716,

Expected number of repairs = 0.000918, Expected number of treatments =0.000364 and

System profit = 19532.31.

The detailed results are given in tabular form. Here Tables 1-3 respectively, illustrate the effect of server treatment rate for various combinations of parameters on Mean Time to System Failure (MTSF), availability and profit assuming.

7. Discussion and Concluding Remark

A stochastic model for a repairable cold standby system, with waiting arrival and treatment times of server, is discussed in this paper. The theory of semi-Markov process and regenerative point technique is used to derive expressions for measures of reliability and profit. An example is given under the setup of exponential distribution by assigning distinct values to various parameters and costs considered for the system model. The further numerical results (as given in Tables 1-3) indicate that MTSF, availability and the profit rise with increasing server treatment rate (β), repair rate of units (α) and server arrival time (ψ) but the trend reverts with increasing the failure rates of server (g) and unit (λ). The persisting trend reveals that the waiting arrival and treatment times impact a lot on the system performance. Therefore, the study re-iterates the practicalities that a cold standby system served by a repairable server can be kept reliable and profitable by:

1) Using standard units with low failure rates.

2) Deploying efficient server with high repair rates.

3) Planning for higher arrival rate of server and,

4) Arranging rapid after failure treatment of the server.

The re-iteration of the true facts evidently proves the acceptability of the probabilistic model developed in this paper. The study may be inspiring and useful for system planners and reliability engineers for developing highly reliable and profitable systems to earn users’ satisfaction.

The study finds its application in diverse areas such as power generating systems with standby reservoirs, communication systems with redundant channels, remote sensing systems with alternate power backups etc.

Acknowledgements

This work is a part of Major Research Project F. N. 42-34/2013(SR) financially supported by UGC under MHRD, Govt. of India. The authors are grateful to anonymous referee for their valuable comments and suggestions.

Cite this paper

Rohtash K. Bhardwaj,Ravinder Singh, (2016) Stochastic Model of a Cold-Stand by System with Waiting for Arrival & Treatment of Server. American Journal of Operations Research,06,334-342. doi: 10.4236/ajor.2016.64031

References