Open Journal of Social Sciences
Vol.03 No.09(2015), Article ID:59705,6 pages
10.4236/jss.2015.39015
A Game-Theoretic Model for Bystanders’ Behaviour in Classes with Bullying
Yuriko Isada1, Nobuko Igaki1, Aiko Shibata2
1School of Policy Studies, Kwansei Gakuin University, Hyogo, Japan
2Board of Trustee, International Christian University, Tokyo, Japan
Email: yuriko@kwansei.ac.jp


Received 29 August 2015; accepted 15 September 2015; published 18 September 2015

ABSTRACT
In this paper, the behaviour of bystanders in a classroom in which bullying is occurring is analyzed using Game theory. We focus on bystander’s behaviour and formulate a threshold model. Our analysis shows that as class sizes become smaller, the probability of bullying being stopped increases.
Keywords:
Bullying, Free Rider, Non-Corporative Game

1. Introduction
According to a survey on problematic behaviours by the Ministry of Education, Culture, Sports, Science and Technology (MEXT) in Japan, the number of recognized incidents of bullying at all grade levels nationwide in 2012 was 198,108, 2.82 times than that of the previous year. This represents the largest number of recorded incidents since the survey began [1]. Additionally, there were 196 student suicides in 2012, of which 3.1% were understood to have resulted from bullying; this made the issue of bullying a serious social problem that must be solved.
Morita [2] emphasised the importance of bystanders’ behaviour. Shibata et al. [3] conducted an economic analysis on the behaviour of bystanders. Glass and Smith [4] established that a reduced class size can be expected to produce academic achievement. Additionally, Smith and Glass [5] have shown that small class size is effective in improving student attitudes and behaviour.
This paper comprises several sections in which different aspects of bullying analyses are discussed. Section 2 explains our model in detail. Section 3 analyses the Nash equilibrium within the model. Section 4 discusses numerical experiments with changes to class size and the impact of these changes on behaviour. Section 5 summarizes our results.
2. A Game-Theoretic Model for Bullying
There are three kinds of people in this situation: the bully, the bullied child and bystanders. In this paper we only focus on bystanders’ behaviour in a class where there is bullying. Suppose that there are  bystanders in the class each bystander can take behaviour R, where a student reports bullying to a teacher, or behaviour S, where a student does not report the bullying. Bullying is resolved when more than
bystanders in the class each bystander can take behaviour R, where a student reports bullying to a teacher, or behaviour S, where a student does not report the bullying. Bullying is resolved when more than 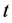 students report the bullying. All players are initially granted a utility level
students report the bullying. All players are initially granted a utility level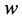 . When bullying occurs, players incur a negative externality (disutility)
. When bullying occurs, players incur a negative externality (disutility)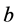 . Cost
. Cost 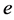 is constantly incurred for student who selects behaviour R, regardless of whether bullying is stopped or not.
is constantly incurred for student who selects behaviour R, regardless of whether bullying is stopped or not.
Then, a non-cooperative n-person game model [6] [7] is formulated, shown in Table 1 . Each value shows the player’s gain in each case, where X denotes the number of reporters other than himself of herself.
3. Nash Equilibrium in the Bullying Model
Each bystander play this game according to Table 1 . Suppose each bystander has the same probability of reporting, q. When players other than oneself select behaviour R with a probability q, the probability of case 1, 2 and 3 are 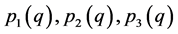 respectively as shown below:
respectively as shown below:
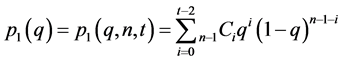 (1)
(1)
 (2)
(2)
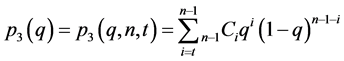 (3)
(3)
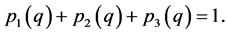 (4)
(4)
The Expected utility of 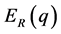 when a player selecting behaviour R, and the Expected utility of
when a player selecting behaviour R, and the Expected utility of 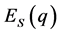 when a player selecting behaviour S are expressed with the following equations.
when a player selecting behaviour S are expressed with the following equations.
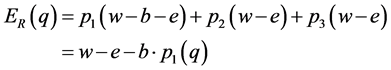 (5)
(5)
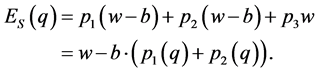 (6)
(6)
 is the state when all players select behaviour R and
is the state when all players select behaviour R and  is the state when all players select behaviour S. When
is the state when all players select behaviour S. When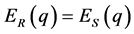 , the result is as
, the result is as
From Equation (2), we have


It follows that

where
Figure 1 shows that there are two values of which hold 



Figure 2 shows the relationship between Expected utilities 


As we saw in Figure 2, 



Table 1. Changes to player gain by the number of reporters when selecting either behaviour R or S.
Figure 1. Two intersections of 

Figure 2. Expected utility when there are two intersections of 

Proposition 1
1) A pure strategy Nash equilibrium always exists in which no player reports bullying. Only when 
2) When



When examining Figure 1, we see that we can expand the range 




Proposition 2
An increase in b or a decrease in 


By differentiating both sides of 









shion.
4. Behavior Resulting from Changing the Number of Bystanders in the Class
Let us examine changes in 












Figure 4 is a graph that shows the value of 















Proposition 3.
1) For

2) For i such as 


Figure 3. A graph of 

Figure 4. A graph of



Since

where 
using the relation 
it is left for us to prove 


From relations

we obtain
Proposition 3 (2) shows that adopting smaller class sizes is effective for reducing bullying. It gives the effectiveness of small-group education.
5. Conclusion
In this paper we modelled the behaviour of bystanders of students in a non-cooperative n-player game. We showed by making



Furthermore, to expand the range 


Finally, this study has demonstrated the existence of the “free riding” phenomenon: if the majority of other people report, it is advantageous for any given person not to do so.
Acknowledgements
This work was supported by JSPS KAKENHI Grant Numbers 25350468.
Cite this paper
Yuriko Isada,Nobuko Igaki,Aiko Shibata, (2015) A Game-Theoretic Model for Bystanders’ Behaviour in Classes with Bullying. Open Journal of Social Sciences,03,97-102. doi: 10.4236/jss.2015.39015
References
- 1. MEXT (2011) Heisei 23 nendo “Jidou seito no mondai koudou nado seito shidoujou no shomondai ni kan suru chousa kekka ni tsuite” [Results of the 2011 Survey of Problem Behavior of Students and Various Issues with Instructing Students]. http://www.mext.go.jp/b_menu/houdou/24/09/__icsFiles/afieldfile/2012/09/11/1325751_01.pdf.
- 2. Morita, Y. (2010) Ijime to ha nani ka [What Is Bullying]. Chuko Shinsho, Tokyo.
- 3. Shibata, A., Mori, T., Okamura, M. a Soyama, N. (2008) An Economic Analysis of Apathetic Behavior: Theory and Experiment. The Journal of Socio-Economics, 37, 90-107. http://dx.doi.org/10.1016/j.socec.2006.12.026
- 4. Glass, G.V. and Smith, M.L. (1979) Meta-Analysis of Research on Class Size and Achievement. Educational Evaluation and Policy Analysis, 1, 2-16. http://dx.doi.org/10.3102/01623737001001002
- 5. Smith, M.L. and Glass, G.V. (1980) Meta-Analysis of Research on Class Size and Its Relationship to Attitudes and Instruction. American Educational Research Journal, 17, 419-433. http://dx.doi.org/10.3102/00028312017004419
- 6. Nash, J. (1950) Equilibrium Points in n-Person Games. Proceed-ings of the National Academy of Sciences of the United States of America, 36, 48-49. http://dx.doi.org/10.1073/pnas.36.1.48
- 7. Nash, J. (1951) Non-Cooperative Games. Annals of Mathematics Second Series, 54, 286-295. http://dx.doi.org/10.2307/1969529






