 Applied Mathematics, 2011, 2, 846-853 doi:10.4236/am.2011.27114 Published Online July 2011 (http://www.SciRP.org/journal/am) Copyright © 2011 SciRes. AM Multiple Periodic Solutions for Some Classes of First-Order Hamiltonian Systems Mohsen Timoumi Department of Mat hematics, Faculty of Sciences, Monastir, Tunisia E-mail: Mohsen.Timoumi@fsm.rnu.tn Received April 15, 2011; revised May 19, 2011; accepted May 22, 2011 Abstract Considering a decomposition 2 AB of 2 , we prove in this work, the existence of at least geometrically distinct periodic solutions for the first-order Hamiltonian system 1dimA ,t xte t0Jx tH when the Hamiltonian ,Htuv is periodic in and its growth at infinity in v is at most like or faster than ,tu a v, 0a1 , and is a forcing term. For the proof, we use the Least Action Principle and a Generalized Saddle Point Theorem. e Keywords: Hamiltonian Systems, Partial Nonlinearity, Multiple Periodic Solutions, Critical Point Theory 1. Introduction Consider the nonautonomous first-order Hamiltonian system ,0JxtHt x te t where , is a continuous function, periodic in the first variable and differentiable with respect to the second 2 :N H T ,, ,tx Htx 0T variable with continuous derivative ,, tx tx , 2 :eN is a continuous Tperiodic function with mean value zero and J is the standard symplectic matrix: 0 0 N N JI being the identity matrix of order . N Using variational methods, there have been many pa- pers devoted to the existence of periodic solutions for (), we refer the readers to [1-5] and the references therein. However, there are few papers discussing the multiplicity of periodic solutions for () (see [6-9]). Under the assumptions that H is periodic in 1,, x, where , 12pN 1 12 ,,N xx and there exists such that 0, ;T 2 fL 2 ,,,..0 N Htxft xaet ,1. (1.1) 2 0,d, 0, T p Htxtasxx (1.2) the author has shown in [9] that system () possesses at least 1p geometrically distinct periodic solutions. The first goal of this note is to generalize the existence result of multiple periodic solutions obtained above to the sublinear case. Precisely, consider a decomposition 2N B of 2 with 12 1 space, ,,space, , pp ii AeeBee N i 1 where 02pN 2N and 12 iiN is the standard basis of and let us denote A P esp. e (r P) the pro- jection of 2N to in (resp. B). We obtain the fol- lowing result Theorem 1.1 Asse that H satisfies um 0 H is periodic the variables. in1,, ii x; 1 There exist α א [0, 1] and two T–periodic functions 1(0, ;) 1 aL T and such that 20, ;bLT 2 ,,, N B Htx atPxbt xaet ..0,1, (H2) Either 1) 20 1,d , T tx tasxx B x or 2) 20 1,d , T tx tasxx B x Then the Hamiltonian system () possesses at least 1pT periodic solutions geometrically distinct.  M. TIMOUMI 847 Example 1.1 Let be a periodic and continuously differentiable function. Consider the Ham- iltonian: 2 :N a 3 2 12π ,, sin 2 trptp ar T (1.3) Then H satisfies conditions 02 H with and 0 N A 0 B. It is easy to see that conditions 12 , H don’t cover some sublinear cases like 2 2 2 12π ,, cos, 2ln 2 ,, N par Htrp t Tpar trp (1.4) The second goal of this paper is to study the existence of multiple periodic solutions for () when the Hamilto- nian satisfies a nonlinearity condition which covers the cases like (1.4). Precisely, we will require the nonlinearity to have a partial growth at infinity faster than ,0 1x Our second main result is: Theorem 1.2 Consider a nonincreasing positive func- tion 0, ,C with the properties: lim inf0 s s , 0, ,sssass and assume that H atisfies 0 and the following as- sumptions 3 There exist a positive constant a d a function such that for all and ;T 20,gL 2N x .. 0aet,1 , BB , txaPxP xgt 4 Either 1) 20,d as 1, T tx txx B xx , 2) 20,d as 1, T tx txx B xx . Then the system () possesses at least 1p geo-metrically distinct Tperiodic solutions. Remark 1.1 The Hamiltonian defined in (1.4) satisfies the conditions 3 , 4 introduced above with 2 1,0 ln 2 ss s , 2. Preliminaries Firstly, let us recall a critical point theorem due to G. Fournier, D. Lupo, M. Ramos and M. Willem [10]. Given a Banach space E and a complete connected Finsler manifold V of class , we consider the space 2 C ExV . Let EW Z (topological direct sum) and (n EZ n ) be a sequence of closed subspaces with n Z, nWimW, 1dn W . Define nn ExV . For ,X, w/n nX 1 Cfe denote by f. Then we have 1,CX for nn Definition 2.1 Let fall 1. n 1 ,CX. The function f satis- fies the Palais-Smale condition with respect to n at a level c if every sequence n satisfying ,, nn nnn xXfx cfx0 has a subsequence which converges in to a critical point of f. The above property will be referred as the condition with respect to * PS c n . Theorem 2.1 (Generalized Saddle Point Theorem). Assume that there exist constants and 0r such that 1) f satisfies the c condition with respect to * PS n for every ,c , 2) ,fwv for every such that ,wvW V wr , 3) ,fzv for every , ,zvZ V 4) ,fwv for every such that ,wv WV wr . Then 1,f contains at least cuplength 1V critical points of f. Consider the Hilbert space 1 12 2, EHS where T and the continuous quadratic form Q efined in E y 0 1 (). d 2 T QxJx txtt where , y 2 inside the sign integral is the inner product of , xy. Let us denote by , , 0 E EE respec- tively the subspaces of on which Q is null, negative definite and positive definite. It is well known that these sub-spaces are mutually orthogonal in E 221 , LS and in with respect to the bilinear form: E 0 1 (, )().()d 2 ,, T BxyJxt yttxyE associated to Q. If E and E then 0(, )Bxy and ())(Qx yQy(Qx) . For 0 xxxE , the expression 1 2 2 0 xQxQx x is an equivalent norm in E. Moreover, the space is compactly embedded in E 212 , LS for all 1,s . In particular for all 1,s , there exists a constant 0 s such that for all E , Copyright © 2011 SciRes. AM 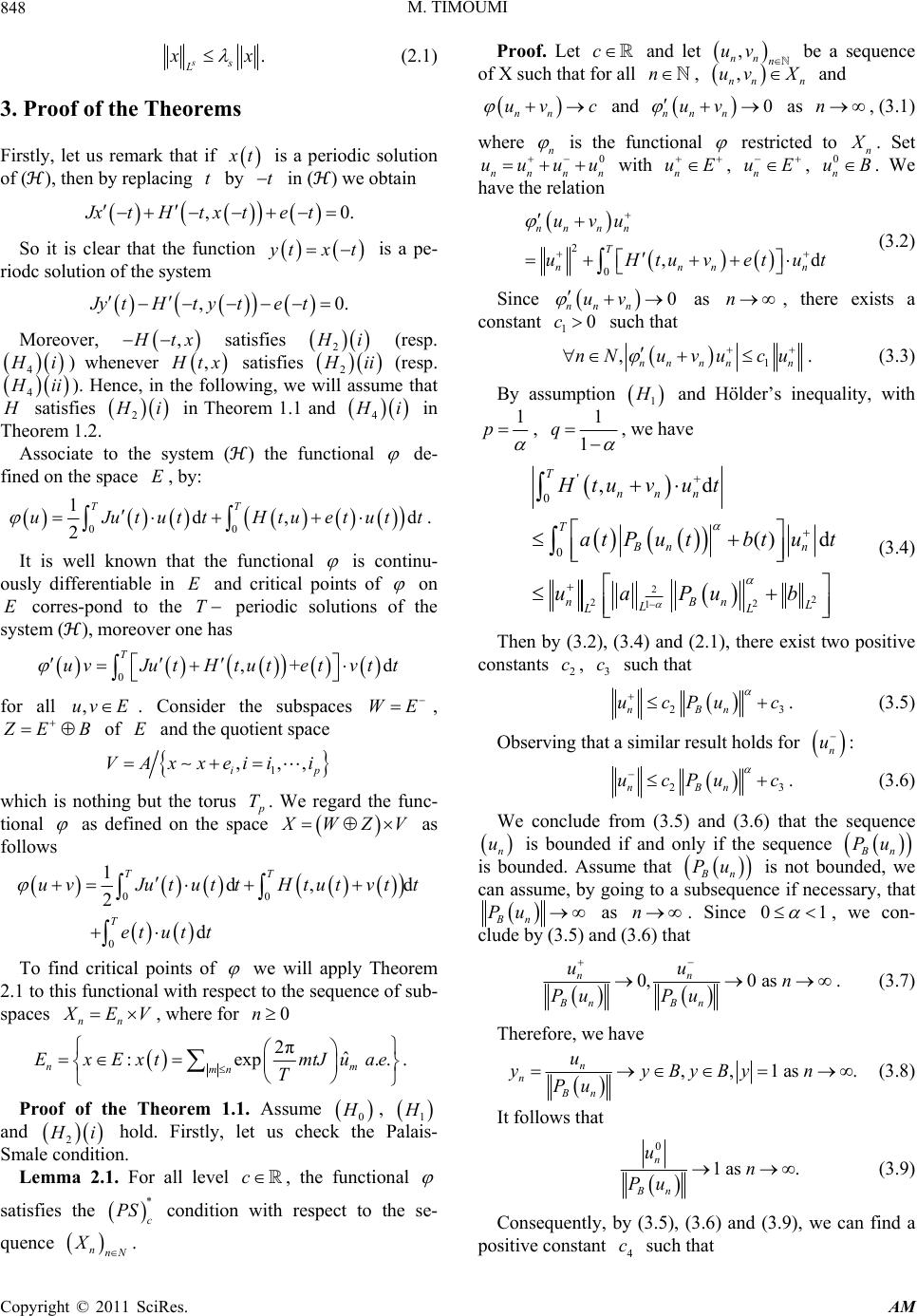 M. TIMOUMI 848 . ss L x (2.1) 3. Proof of the Theorems Firstly, let us remark that if tt is a periodic solution of (), then by replacing by in () we obtain t ,0JxtHt xtet . So it is clear that the function txt is a pe- riodc solution of the system ,0JytHt ytet . Moreover, , tx satisfies 2 i (resp. 4 i) whenever , tx satisfies 2 ii (resp. 4 ii ). Hence, in the following, we will assume that satisfies 2 i in Theem 1.1 and or 4 i in Theorem 1.2. Associate to the system () the functional de- fined on the space , by: E 00 1d, 2 TT uJut uttHtuet utt d . It is well known that the functional is continu- ously differentiable in and critical points of E on corres-pond to the T periodic solutions of the system (), moreover one has E 0,+ T uvJu tHtutetvtt d for all . Consider the subspaces ,uv EWE , E B of and the quotient space E 1 ,,, ip VAxxeii i which is nothing but the torus T. We regard the func- tional as defined on the space XZWV as follows 00 0 1d, 2 d TT T uvJututtHtutvt t et utt d To find critical points of we will apply Theorem 2.1 to this functional with respect to the sequence of sub- spaces nn EV, where for 0n 2πˆ :exp nm mn ExExt mtJuae T .. . Proof of the Theorem 1.1. Assume 0 , 1 and 2 i hold. Firstly, let us check the Palais- Smale condition. Lemma 2.1. For all level , the functional c satisfies the condition with respect to the se- quence . * c PS nN n X Proof. Let c and let be a sequence of X such that for all , nn n uv n , and , nn uv Xn nn uvc and 0 nn n uv as , (3.1) n where n is the functional restricted to n . Set n 0 nnn uuuu with n uE , , n uE 0 n uB . We have the relation 2 0,d nn nn T nnn uvu uHtuvetu n t (3.2) Since 0 nn n uv 10c as , there exists a constant such that n 1 ,nnnnn nNu vucu . (3.3) By assumption 1 and Hölder’s inequality, with 1 p , 1 1 q , we have 2 2 22 1 ' 0 0 ,d () d T nn n T Bn n nBn L L LL Htuvut at Putbtut uaPu b (3.4) Then by (3.2), (3.4) and (2.1), there exist two positive constants , such that 2 c3 c 2nBn ucPuc 3 . (3.5) Observing that a similar result holds for n u : 2nBn ucPu c 3 . (3.6) We conclude from (3.5) and (3.6) that the sequence n u is bounded if and only if the sequence Bn Pu is bounded. Assume that Bn Pu is not bounded, we can assume, by going to a subsequence if necessary, that Bn Pu as . Since n 01 , we con- clude by (3.5) and (3.6) that 0,0 as nn Bn Bn uu n Pu Pu . (3.7) Therefore, we have ,,1 as n n Bn u yyByBy Pu .n (3.8) It follows that 0 1 as . n Bn un Pu (3.9) Consequently, by (3.5), (3.6) and (3.9), we can find a positive constant such that 4 c Copyright © 2011 SciRes. AM  M. TIMOUMI 849 0 4,, i nn ucui . (3.10) Now, we apply the fact that nn uv is bounded to get 22 22 0 00 5 22 000 ,d .d T nn nn nn Tnn nn uu Htuvt uu et uuc t uu (3.11) where 3 is a positive constant. Using (3.10) and (3.11), we can find a constant satisfying c 6 c 0 22 00 00 0 2 00 0 62 00 ,, dd ,, d ,, d TT nnn nn Tnnn n Tnnn n Htu Htu v tt uu HtuHtu vt u HtuHtu v ct u (3.12). On the other hand, by the Mean Value Theorem and assumption 1 , we have 2 12 22 0 0 0 0 0 0 0 ,d ,. d . T nnn T nnnnnn T Bnnn nnn Bnnn LL nnn LL HtuHuvt n tuu u vu u v at P uuubt uuvt aPuuu buuv (3.13) By considering (3.13) and Sobolev’s embedding E 22 0, ; LT we can find a constant such that 70c 0 0 0 7 ,d || T nnn nn nnn HtuHuvt cuuuu u 1 (3.14) After combining (3.10), (3.12) and (3.14), we get 0 8 2 00 ,d Tn n Htu tc u (3.15) for some positive constant . However, the condition (3.15) contradicts 8 c 2 i because 0 n u as . Consequently, n n u v is bounded in X. Going if necessary to a subsequence, we can assume that and . Notice that , n uu 0 n u0 un v d nnn uu vuu Htv et nn nn u v t 0 T n Qu ,, n u v Htu uu uu (3.16) which implies that n in E. Similarly, n uu in E. It follows that ,, nn uv uv in X and 0uv . So satisfies the condition for all * c PS c . The Lemma 3.1 is proved. Now, let us prove that the functional satisfies the conditions a), b) and c) of Theorem 2.1. a) Let W,uv V . By using the Mean Value Theo- rem, assumptions 0 , 1 and (2.1), we have 2 00 00 00 , dd dd TT TT TT L Htu t et t ut t ut tu 2 00 2 0 2 0 2 0 2 0 1 22 2 0 || ||dd || ||,d ,.d d ,d ,d ,d d TT T B T B T T T uvuvt etu uHtv Htvu utut uHtvatPu btutet uHtvatPu btutet uHtv atu t t (3.17) 2 91 0 L c 0 2 11 d T betut uucuc where , 10 , are three positive constants. Since 9 c c 1 11 c 0 , then as ,uv uWu uniformly in vV . (3.18) b) Let ,uvZV , with . By using the Mean Value Theorem, we get 0 uu u 20 0 0 20 0 0 00 ,d d || ||,d ,d T T T TT uvuHtuu vt et ut uHtut tuuvuvet u t (3.19). Copyright © 2011 SciRes. AM  M. TIMOUMI 850 By assumption 1 and (2.1), we can find a con- stant such that 12 0c 22 22 0 0 0 0 1 22 20 0 0 12 ,()d , d 11 TT T B LL L T LL 0 d tuuvuvt etut at Ptuuvbt uvteu uv atu ube cuu u (3.20) Therefore, by using (3.19) and (3.20) we obtain 20 0 0 12 ,d 11 T uvuHtu t cuu u . (3.21) Now let 2 12 2 c d. By assumption 2 i, there ex- ists a constant such that 13 0c 2 00 13 0,dd T tu tuc (3.22). So by (3.21) and (3.22), we have 22 0 13 0 12 21 12 2 22 00 12 12 0 1212 13 11 1 2 1 22 . uv uduc cuu u ucu uu c ucudu cuc c (3.23) Since 2 12 2 c d and 01 , then as ,,uv uZu uniformly in vV . (3.24) Hence by Lemma 3.1 and properties (3.18), (3.24), we deduce that the functional satisfies all the assump- tions of Theorem 2.1. Therefore the Hamiltonian system () possesses at least 1p T periodic solutions geometrically distinct. The proof of Theorem 1.1 is com- plete. Proof of Theorem 1.2. Assume 0 , 1 and 4 i hold. The following lemma will be needed for the study of the geometry of the functional . Lemma 3.2. There exist a non-increasing positive function (]0, ,C and a positive constant satisfying the following conditions: 0 c i) ()0, as ,sss s ii) 20 (,)1 ,, BB L tucP uP uuE iii) 00 asu 20 00 1,d . THtu t uu Proof : For uE , let 1 2 0, :. BB AtTPut Pu By 3 , we have 2 2 2 2 1 22 0 1 22 0 2 2 1 22 2 0, 12 22 1 2 2 0 ,d d d d|||| d sup( )||||. T BB L T BB L BB A BB L TA BB A sB L tuaP utPutgtt aPutPut tg aPutPutt Put Puttg aPuPutt TsPug So, by (2.1) there exists a positive constant such th 0 c at 2 1 12 2 22 0 (,) 1. L BBB Htu cPuPuPu Take 1 12 221,0ss s s , satisfies (ii) and it is clear to see that then satis- ). et us define fies (i Next, l 2 1 22 lim inf. s 4 i, for any 0 , there exists a positive By Copyright © 2011 SciRes. AM  M. TIMOUMI 851 constant s 14 c uch that 2 14 0,d T. tx txxc (3.25) which imat for plies th0 uB, 00u, 2 0 T00 14 0 21 00 2 20 00 2 ,d . uu c Ht u t uu uuu (3.26) By the definition of , there exists such that fo 0R r all R 22 1 22 2 () . 2 ss ss s (3.27) Therefore 0 014 21 00 2 20 00 2 ,d 2 THtu tc T uu uuu (3.28) as 0 uR and then 0 0 0 2 00 ,d lim . 2 T u Htu t T uu (3.29) Since is arbitrary choosen, condition (iii) holds. et onal Now, l us prove the Palais-Smale condition. Lemma 3.3. For all level c, the functi e- that sa be ance in X such fo . (3.30) Set n and n By Hö inLemma 2 tisfies the * PS condition respect to the s quence n X Proo u c . ,v with seque n f. Letnn n , nn uv r all ,n n and u v X and 0 as nn nnn v cun 0 nnn uuuu lity, (2.1) and nn uuu . .2(ii), we lder’s equaget a positive constant 15 c such that 2 2 0 1 2 2 0 15 ,d , 1. Tu v nn n n T nnnnL L nBnBn Htetuut uu Htuve c uPuPu (3.31) Thus, for n large enough 2 15 21 nn nnBn Bn u ucuPuPu (3.32) So there exists a positive constant such that . nnn uvuu 16 c 16 1. nBnB u cPuPu n (3.33) By (3.33) and the properties (i) of , we deduce that n u is bounded if and only Bn Pu is bounded. since Now, is nonincreasing and Bn uPu 0 max, B uP , we get u m, B uuPu (3.34) Combining (3.32) and (3.34), yields f en 0 in or n large ough 2 00 15 2 1 n un nBnBn nn u c uPuPuuu which implies 15 15 21. n Bn uc Puc (3.35) Assume that 00 15 nn cuu Bn Pu is unbounded, then b to y going a subsequence, if necessary, we can assume that Bn Pu as n. Since 0s as , wcm (3at there exositiv stant 17 c such that e dedue fro.35) thists a pe con- 00 17n u n n cuu (3.36) for large enough. Since the mapn ss is con- tinuos in u 0, and goes to as , then 0 n u as. by the M Theorem n Now, ean Value ity , Hölder’s inequal- and Lemma 3.2(ii), we get 2 2 0 ,,d T nn n Htu vHtut 0 10 00 1 2 12 0 00 100 00 ,. dd ,dd 1d . T nn n n T nnn nn L nnnBnn Bn L Ht usuvuvst uvHtusuvt s cuvusP uusP us (3.37) Since 00 nBn usPu u n for all 0,1s there ex , we deom (2.1), (3.36) and (3.37) that du-ce frists a positive constant 18 c such that 2 0 0,,d T nn n Htu vHtut 00 0 0 22 000 00 18 00 || [ 1] 1, nn nnnBn L nnn nn nn cuvuuu Pu cuuuuu uu (3.38) which with (2.1) and (3.36) imply that there exists a positive constant such that 19 c Copyright © 2011 SciRes. AM  M. TIMOUMI 852 22 0 0,d T nn nnn T uv uuHtut 0 00 2 00 0 19 0 2 000 00 2 00 0 19 00 0 0 22 0000 19 ,, d.d ,d 1 1 1 ,d 1 T nn n n T nnn nnn nn nn n nn T n nn nn Ht uvHtutetut Htut cuu uuu uu cuuu uu Htu t uuc uu (3.39) which, with Lemma 3.2 (iii), imply that as . This contradicts the boundedn nn uv ess of n nn uv . So Bn Pu is bounded Ass that . ume 0 u, then up to a n is unboundedsub- sequence, if sary, we canecesn assume that 0 n u as .38), n. As in (3and using (2.1), (3.34) and the fact that ()0s as , we can find atant such that cons 21 0c 2 0 0 00 0 0 00 21 ,,d T nn u vt 1 1. n nnnnnBn L nn nn Ht Htu cuvu uuPu cuvu u (3.40) Now, since ()0 as ss , then combining (3.33) and (3.34) yields 000 00 22 1 n n nn uuuu P cuu (3.41) for a positive constant . Therefore there exists tive constant such 1 nn nBnn vu v 22 c that a posi- 23 c 0 0,,.d T nnnn 2 00 23 1. nn tuvH tuetut cu u (3.42) We deduce from (3.41) and (3.42) that ther constant such that e exists a 24 0c 2 00 0 1,d T nn nn uv cuuHtut 24 0 0 2 00 0 24 2 00 ,d 1 1 n T n nn nn tut uuc uu which implies by Lemma 3.2 (iii) that as . This contradicts the boundedness of nn uv n nn uv . Then 0 n u is also bounded and therefore n u is bounded. By a standard argument, we con-clude that n u f of possesses a convergent subse The proo Lemma 3.3 is complete. let - quence. Now, 0 ,,uvuvZ V u , then as in (3.38) that there exists a positive constant 25 c such 0 00 0 25 , .)d 11. T nnn n B vHtuetu t cuuu uPu 0(,Htu (3.43) So, we have for a positive constant c 26 00 26 0 1,d. T n u uuc Htut (3.44) Let 200 26 uvucuu 01 , we have 22 0000 2 26 26 2nn c cuuu uu 2. u (3.45) By combining (3.44) and (3.45), we get 2 20 26 26 0 2 2 00 260 22 00 1 ,d 1 T n nn nn uvcuuc u tut c uu uu which implies that uv as uZ , u, uniformly in vV . (3.46) On the other hand, let bB, 0b. By the Mean Value Theorem, we have for uW E 2 12 '2 00 1 (,| dd T L T uvbHtbvt s 2 2 2 1 00 1 00 1 22 1 00 1 22 d ,.dd ) dd dd T T B L B T B L BL tb t Htbsu uvbs su b uvbabsPu b bsPu bgtts uvbabsPu b bsPu bts g 0,,Htu vH vbt (3.47) Copyright © 2011 SciRes. AM  M. TIMOUMI Copyright © 2011 SciRes. AM 853 Take for 0,1s, 0,1 :. B st bsPubb Mathematical Analysis Application, Vol. 323, No. 15, 2006, pp. 854-863. doi:10.1016/j.jmaa.2005.11.004 [2] P. L. Felmer, “Periodic Solutions of Superquadratic Ha- miltonian Systems,” Journal of Diffuation, Vol. 102, No. 1, 1993, pp. 188-207. By a similar calculation as in the proof of Lemma 3.2, we get for some positive constants and 27 c cb 0 2 27 ,, 1 T erential Eq doi:10.1006/jdeq.1993.1027 [3] Z. Q. Ou and C. L. Tang, “Periodic and Subharmonic Class of Superquadratic Hamiltonian Sys- ar Analysis, Vol. 58, No. 3-4, 2004, pp. d tuvH tbetut cbucbu Solutions for a tems,” Nonline which implies that (3.48) 245-258. doi:10.1016/j.na.2004.03.029 [4] C. L. Tang and X. P. Wu, “Periodic Solutions for Second Order Systems with Not Uniformly Coercive Potential,” Journal of Mathematical Analysis Application, Vol. 259, No. 2, 2001, pp. 386-397.doi:10.1006/jmaa.20 22 27 0 1, T uvu cbu cbuHtb t d. (3.49) Since 00.7401 [5] M. Timoumi, “Periodic Solutions for Noncoercive Ham- iltonian Systems,” Demonstratio Mathematica, Vol. 35, No. 4, 2002, pp. 899-913. 0asss , there exists 0b such that 27 1 || 2 cb , which implies that [6] K. C. Chang, “On the Periodic Nonlinearity and the Mul- tiplicity of Solutions,” Nonlinear Analysis, Vol. 13, No. 5 1989, pp. 527-537. doi:10.1016/0362-546X(89)90062-X [7] S. X. Chen, X. Wu and F. Zhao, “New Exis 2 uv 0 11,d. 2 T ucb uHtbt So we have tence and Multiplicity Theorems of Periodic Solutions for Non- Autonomous Second Order Hamiltonian Systems,” Mathematical and Computer Modeling, Vol. 46, No. 3-4, 2007, pp. 550-556. doi:10.1016/j.mcm.2006.11.019 uv as uW, u, uniformly in vV (.3.50) Thus, Lemma 3.3 and properties (3.46), ( that the functional [8] I. Ekeland and J. M. Lasry, “On the Number of Periodic Trajectories for a Hamiltonian Flow on a Convex Energy Surface,” Annals of Mathematics, Vol. 112, No. 2, 1980, pp. 283-319.doi:10.2307/1 3.50) imply ) satisfies all the assum Generalized Saddle Point Theorem. Therefore the Ham possesses at least peri- etrically distinct. Theheo- rem 1.2 is complete. 4. References [1] tions Autonomous Hamiltoned Period,” Journal of ptions of the iltonian system ( odic solutions geom 971148 [9] M. Timoumi, “On the Multiplicity of Periodic Solutions of a Hamiltonian System,” Demonstratio Mathematica, - 1pT proof of TVol. 35, No. 4, 2002, pp. 899-913. [10] G. Fournier, D. Lupo, M. Ramos and M. Willem, “Limit Relative Category and Critical Point Theory,” Dynamics Reported, Vol. 3, 1994, pp. 1-24. T. An, “Periodic Soluof Superlinear ian Systems with Prescrib
|