Received 31 March 2015; accepted 23 June 2015; published 30 June 2015

ABSTRACT

Let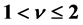,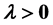. We study the existence and multiple positive solutions of n-th nonlinear discrete fractional boundary value problem of the form 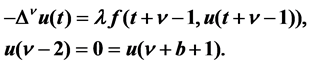By using a fixed-point theorem on cone, the parameter intervals of problem is established.

Keywords:

Fractional Difference Equations, Parameter Intervals, Positive Solution, Fixed-Point Theorem

1. Introduction

There have been of great interest recently on fractional difference equations. It is caused by the development of the theory of fractional calculus and discrete fractional calculus, also by its applications, see [1]-[7]. We noted that most papers on discrete fractional difference equation are devoted to solvability of linear initial fractional difference equations [8] [9]. Recently, there are some papers dealing with the existence of solutions of nonlinear boundary value problems, we also refer the readers to [10] [11]. However, there are few papers consider parameter intervals of fractional difference boundary value problems. In the present work, our purpose is to the parameter intervals of the following fractional difference boundary value problem

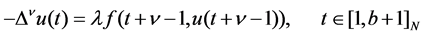(1.1)

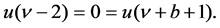(1.2)

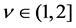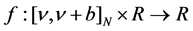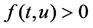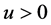where, is an integer, is continuous, for and. For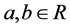, define.

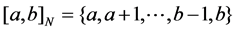F. M. Atici and P. W. E. [10] studied fractional difference boundary value problem

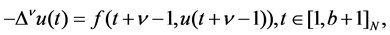(1.3)

with the boundary value condition (1.2). By using Krasnosel’skii fixed point theorem under condition

(H1),;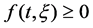

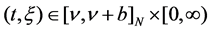(H2), where is a positive function, is a non-negative function and

(H3), where is a positive function, is a non-negative function and

They get the following.

Theorem 1.1[10] Assume that conditions (H1) and (H2) are satisfied, then problem (1.1) and (1.2) has at least one solution. Assume that conditions (H1) and (H3) are satisfied, then problem (1.1) and (1.2) has at least one solution.

The following conditions will be used in the paper

(A1), where is a positive function, is continuous, and there exist such that;

(A2).

2. Preliminaries

Recall the factorial polynomial where denotes the special Gamma function and if for some, we assume the product is zero. We shall employ the convention that division at a pole yields zero. For arbitrary, define We also appeal to the convention that

is a pole of the Gamma function and is not a pole, then. Let, and defined on, Miller and Ross [12] have defined the -th fractional sum of by

(2.1)

where, also define the -th fractional difference

where and with,.

Lemma 2.1 [10] Let, , the unique solution problem

(2.2)

is where

(2.3)

Lemma 2.2 [10] The Green’s function in Lemma 2.1 satisfies the following conditions:

(i) for and;

(ii) for;

(iii) There exists a positive number such that for

(2.4)

where

(2.5)

In the rest of the paper, we will use the fixed point index theory in cones to deal with (1.1) and (1.2).

Lemma2.3 [12] Let be a Banach space, be a cone, and suppose that are bounded open balls of centered at the origin with. Suppose further that is a completely continuous operator such that either

(i), and, , or

(ii), and,

holds, then has a fixed point in.

We will need the following notations. Let

Then is a Banach space with the norm

So, is a solution of (1.1) and (1.2) if, and only if is a fixed point of the operator defined by

Note, let be defined by (2.5) and define cones in by For some, Since is finite dimensional, we have the is compact. Obviously,.

Lemma 2.4 Suppose that conditions (A1) hold, and there exist two different positive numbers and such that

where.

Then, problem (1.1), (1.2) has at least one positive solution such that.

Proof. We can suppose that. For, , there is, then

these mains that for, there is. For, , there is, then

these mains that for, there is. By using Lemma 2.3, there exist such that. This means that, is a solution of problems (1.1), (1.2) and. Also, because,

so for, taking into account that conditions(A1) and (A2) hold and, we have that for, i.e. is a positive solution of (1.1), (1.2).

3. Main Results

For some, denote

By using Lemma 2.4, we get

Theorem 3.1 Assume that (A1) hold, and and, then, there exist, for every, problem (1.1) and (1.2) has at least two positive solutions.

Theorem 3.2 Assume that (A1) hold, and or, then, for every, problem (1.1) and (1.2) has at least one positive solutions.

Theorem 3.3 Assume that (A1) hold, and and, then, for every, problem (1.1) and (1.2) has at least two positive solutions.

Theorem 3.4 Assume that (A1) and (A2) hold, and or, then, for every, problem (1.1) and (1.2) has at least one positive solutions.

Acknowledgements

Author was supported by the NSF of Gansu Province (No. 2013GS08288).

Cite this paper

Youji Xu, (2015) Existence and Multiple of Positive Solution for Nonlinear Fractional Difference Equations with Parameter. Journal of Applied Mathematics and Physics,03,757-760. doi: 10.4236/jamp.2015.37091

References