Materials Sciences and Applications, 2011, 2, 537-545
doi:10.4236/msa.2011.26072 Published Online June 2011 (http://www.SciRP.org/journal/msa)
537
Strain Energy Release Rate Analyse of Matrix
Micro Cracking in Composite Cross-Ply
Laminates
Jean-Luc Rebière1, Denys Gamby2
1Laboratoire d’Acoustique de l’Université du Maine, UMR CNRS, Université du Maine, Le Mans, France; 2Institut P’, Département
Physique et Mécanique des Matériaux, Branche Mécanique des Matériaux ENSMA, Futuroscope Chasseneuil Cedex, France.
Received January 31st, 2011; revised March 10th, 2011; accepted April 6th, 2011.
ABSTRACT
The stress field distribution in composite cross ply laminates damaged by matrix cracking is analyzed through an ap-
proach which uses several hypotheses to simplify the damage state. The proposed cracking criterion involves the partial
components of the strain energy release rate associated with transverse and longitudinal cracking. The respective con-
tributions of the 0˚ and 90˚ layers to the damage process are also investigated. The initiation of transverse and longitu-
dinal cracking mechanisms is predicted. We also give an assessment of the influence of each individual component of
the stress tensor on the strain energy release rate of the damaged laminate.
Keywords: Composite Laminates, Matrix Cracking, Damage Mechanics, Failure Criterion
1. Introduction
Composite laminates are used in structural applications
thanks to their high strength to weight ratio; however,
their durability still needs to be carefully assessed. For a
composite cross-ply laminate subjected to uniaxial static
or fatigue tensile loading, the first type of damage ob-
served is usually transverse cracking. This damage
causes an interlaminar stress concentration at the crack
tips. On one hand high interlaminar stress levels may
entail the debond ing of layers at the interface of the plies
with different orientations and/or on the other they may
also cause matrix cracking between fibres in the layers
parallel to the loading axes. It is well known and experi-
mentally observed that the stiffness of a composite
structure is reduced by the growth of the transverse crack
damage. Moreover, a composite structure damaged by
incipient delamination or longitudinal cracking must be
repaired. The main objective of this work is to study the
initiation and evolution of transverse and longitudinal
cracking damage.
1.1. Experiment Observations
This study was prompted by experimental results re-
ported in [1-3]. Under the loading conditions (monotonic
and fatigue tests) the first damage mode is usually trans-
verse cracking. Two notable stages are characteristic of
this damage mod e: its initiation or occurrence of the first
transverse crack called “first ply failure (FPF)” on one
hand and the limiting state when no more transverse
crack can be created, named “characteristic damage state
(CDS)” on the other. Afterwards, it was observed that the
nature of the second damage depends on the following
parameters: the laminate geometry, for example the
thicknesses of the 0˚ or 90˚ layers, the nature of the fi-
bre/matrix constituents, the loading history and the
manufacturing cycle. For instance, the authors of [1,2]
observed the initiation and growth of delamination in a
thick laminate. Ply separation is caused by the increase
of interlanimar normal and shearing stresses, σzz and σxz
respectively. In thin laminates, the damage mode succes-
sion is different. Some authors [1,3] observed that the
second damage mode, which follows transverse cracking,
is longitudinal cracking. In this case, local delamination
appears between 0˚ and 90˚ layers, near the crossing of
longitudinal and transverse cracks, only when longitudi-
nal cracks are widespread. In each case, the accumulation
of the different damage modes causes fibre breaking in
the 0˚ layers. All fibre breaks entail “splitting” which

Strain Energy Release Rate Analyse of Matrix Micro Cracking in Composite Cross-Ply Laminates 538
appears just before the ultimate failure of the laminate.
1.2. Damage Models
For modelling the strain/stress relationship during dam-
age growth, analytical and numerical approaches have
been proposed. Several models describe the initiation of
the first damage mode. They mainly rely on some stress
field distribution and a relationship between loading and
crack density is usually proposed. The simplest models,
called “shear lag analyses” [4-6], usually involve ele-
mentary assumptions regarding the displacement and
stress distributions. Other models such as variational
approaches, whose principles are explained in [7,8], use
the principle of minimum complementary energy [9-12].
Other studies rely on the finite element method [13,14].
Alternative models are based on phenomenological ap-
proaches [15,16], self-consistent analyses [17,18] or ap-
proaches relying on specific aspects of the cracks pat-
terns [19]. Hashin [20] analyses longitudinal and trans-
verse cracking through a variational model, with a re-
strictive hypothesis of constant trough-thickness normal
stress distribution in each damaged layer. Binienda et al.
[21], who propose a finite element approach, use an en-
ergy criterion to analyze the influence of the material on
the strain energy release rate.
Longitudinal cracking is similar to transv erse cracking ,
but appears in the layers where fibres are parallel to the
main loading direction, and longitudinal cracks are not
always continuous [11,22]. For some laminates, longitu-
dinal cracking does not occur before the end of life of the
structure. In other laminates, longitudinal cracks can ap-
pear before the ultimate failure of the laminate. The ob-
served behaviour principally depends on the nature of the
material constituents, th e layer stacking sequence and the
type of loading. For these reasons, the investigation of
longitudinal cracking is often ignored by many models.
In the present approach, relying on experimental obser-
vations, we suppose that the longitudinal cracks are con-
tinuous and that they span the whole length of the studied
specimen.
1.3. Failure Criterion
In the literature, several approaches have been proposed
to investigate the development of the different types of
damage in cross-ply laminates and several kinds of crite-
ria have been proposed [23], among them maximum
stress based approaches. Other kinds of criteria [12,24,25]
rely on the energy release rates associated with each type
of damage. Our interest in damage mechanism evolution
and succession lead us to bring out the respective contri-
butions of the transverse or longitudinal damage mecha-
nism development which can be found in the strain en-
ergy release rate [26,27]. In this article, the strain energ y
release rate is expressed through an appropriate semi-
analytical model and decomposed into individual com-
ponents related to damage mechanisms. Only the most
significant components need to be retained to obtain a
good approximation of the whole strain energy release
rate. In contrast to the simplest models encountered in
the literature, which only take into account the normal
stress in the loading direction, the general model used
here allows a thorough investigation of the strain energy
release rate to be achieved. In the energetical model pro-
posed in [27,28], the strain energy properties is investi-
gated for laminates with arbitrary stacking sequence. The
present study is restricted to damage growth in cross ply
laminates. Here, we again use the decomposition of the
strain energy of the whole laminate already proposed in
[26]. This analysis relies upon some estimate of the role
of each strain energy component in the initiation and
propagation of a given damage mechanism, such as
transverse cracking or longitudinal cracking. Thus each
component of the stress tensor can be associated with one
pair of damage mechanism. After numerous numerical
simulations, it could be established that the influence of a
given component of the stress field on some of the dam-
age mechanisms can often be neglected [29]. In this arti-
cle we use some further results to v alid ate this hypo th esis.
The present study also gives a critical assessment of sev-
eral simple cracking models; namely, the proposed ap-
proach provides a justification for using the only normal
stress in the loading direction in damage criteria. Con-
cerning the initiation of transverse cracking, this kind of
assumption gives good results. However, when the evo-
lutions of transverse cracking or the transition to other
types of damage are of interest, the simplest damage
models cannot be used. In these cases, a more accurate
description of the stress field is necessary. Besides inves-
tigating transverse crack growth, the present paper aims
at analyzing the influence of longitudinal cracking on
strain energy release rate. All numerical simulations are
performed for a thin 8-ply cross ply laminate. Balanced
laminates correspond to a constraining parameter equal
to 1 (λ = 1); rigid laminates are obtained for λ < 1; and
soft laminates are in the range λ < 1. The computation of
the strain energy release rate associated to the different
damage types, transverse cracking, longitudinal cracking
and delamination is developed in [26]. In the article [29],
the proposed results concern the decomposition of the
strain energy release rate of each layer of the laminate. In
the article [29] we presented the strain en ergy release rate
associated to the different types of damage associated
with the three damage modes (mode I (opening mode),
mode II (sliding mode) and mode III (tearing mode)).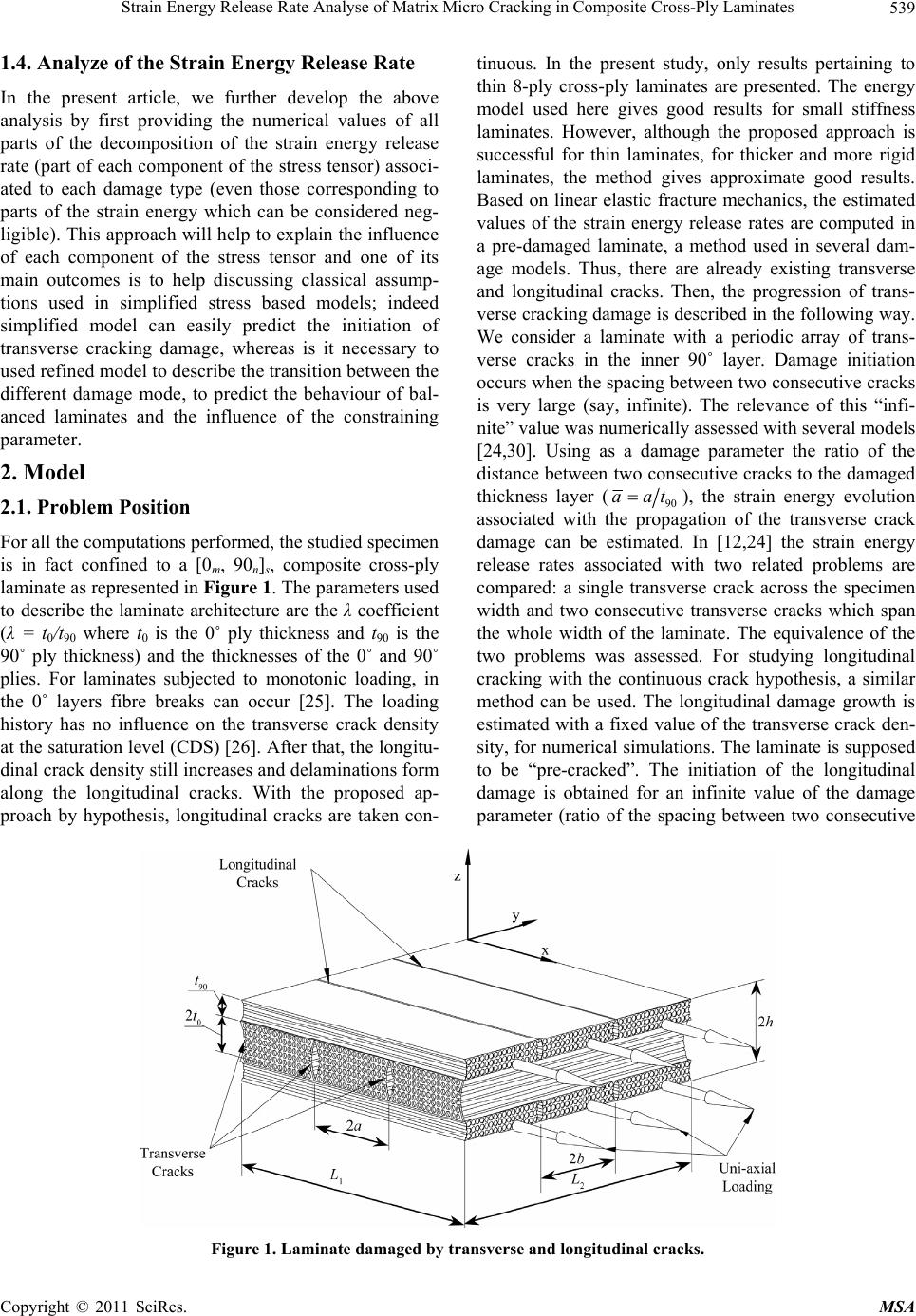

Strain Energy Release Rate Analyse of Matrix Micro Cracking in Composite Cross-Ply La minates 539
1.4. Analyze of the Strain Energy Release Rate
In the present article, we further develop the above
analysis by first providing the numerical values of all
parts of the decomposition of the strain energy release
rate (part of each component of the stress tensor) associ-
ated to each damage type (even those corresponding to
parts of the strain energy which can be considered neg-
ligible). This approach will help to explain the influence
of each component of the stress tensor and one of its
main outcomes is to help discussing classical assump-
tions used in simplified stress based models; indeed
simplified model can easily predict the initiation of
transverse cracking damage, whereas is it necessary to
used refined model to describe the transition between the
different damage mode, to predict the behaviour of bal-
anced laminates and the influence of the constraining
parameter.
2. Model
2.1. Problem Position
For all the computations performed, the studied specimen
is in fact confined to a [0m, 90n]s, composite cross-ply
laminate as represented in Figure 1. The parameters used
to describe the laminate architecture are the λ coefficient
(λ = t0/t90 where t
0 is the 0˚ ply thickness and t 90 is the
90˚ ply thickness) and the thicknesses of the 0˚ and 90˚
plies. For laminates subjected to monotonic loading, in
the 0˚ layers fibre breaks can occur [25]. The loading
history has no influence on the transverse crack density
at the saturation level (CDS) [26]. After that, the longitu-
dinal crack density still increases and delaminations form
along the longitudinal cracks. With the proposed ap-
proach by hypothesis, longitudinal cracks are taken con-
tinuous. In the present study, only results pertaining to
thin 8-ply cross-ply laminates are presented. The energy
model used here gives good results for small stiffness
laminates. However, although the proposed approach is
successful for thin laminates, for thicker and more rigid
laminates, the method gives approximate good results.
Based on linear elastic fracture mechanics, the estimated
values of the strain energy release rates are computed in
a pre-damaged laminate, a method used in several dam-
age models. Thus, there are already existing transverse
and longitudinal cracks. Then, the progression of trans-
verse cracking damage is described in the following way.
We consider a laminate with a periodic array of trans-
verse cracks in the inner 90˚ layer. Damage initiation
occurs when the spacing between two consecutive cracks
is very large (say, infinite). The relevance of this “infi-
nite” value was numerically assessed with several models
[24,30]. Using as a damage parameter the ratio of the
distance between two consecutive cracks to the damaged
thickness layer (90
aat

), the strain energy evolution
associated with the propagation of the transverse crack
damage can be estimated. In [12,24] the strain energy
release rates associated with two related problems are
compared: a single transverse crack across the specimen
width and two consecutive transverse cracks which span
the whole width of the laminate. The equivalence of the
two problems was assessed. For studying longitudinal
cracking with the continuous crack hypothesis, a similar
method can be used. The longitudinal damage growth is
estimated with a fixed value of the transverse crack den-
sity, for numerical simulations. The laminate is sup posed
to be “pre-cracked”. The initiation of the longitudinal
damage is obtained for an infinite value of the damage
parameter (ratio of the spacing between two consecutive
Figure 1. Laminate damaged by transver se and longitudinal cracks.

Strain Energy Release Rate Analyse of Matrix Micro Cracking in Composite Cross-Ply Laminates 540
cracks to the central damaged layer thickness). The
problem at hand is thus to compute the strain energy re-
lease rate for a laminate which is supposed to be previ-
ously damaged by transverse and longitudinal cracks, see
Figure 1 for the whole cross-ply laminate.
The accepted assumptions for the crack geometries in
the two types of layers of the laminate are as follows.
The cracks surfaces are supposed to have a rectangular
plane geometry. Each crack extends over the whole
thickness and the whole width of the 90˚ damaged ply.
Similar assumptions are made for the longitud inal cracks
in the two 0˚ layers. Moreover, the crack distribution is
supposed to be unif orm along both x and y directions, i.e.
for transverse cracks and for longitudinal cracks. With
these assumptions, it is sufficient to study the only “unit
damaged cell”. This “unit damaged cell” thus lies be-
tween two consecutive transverse and longitudinal cracks.
We give in [22] the summary of the method used to es-
timate the stress field distribution in the cracked laminate.
The proposed analytical model is based on a variational
approach relying on the proper choice of a statically ad-
missible stress field [22].
In the damaged laminate, the stress field in the two
layers has the following form:
 
0Tkk Pk
ijij ij
  (1)
In the undamaged laminate loaded in the x direction,
the layers experience a uniform plane stress state
obtained by the laminate plate theory (where k is the ply
index, k = 0˚, 90˚). The orthogonal cracks induce stress
perturbations in the 0˚ and 90˚ layers which are denoted
[22]. In the present approach, for the sake of
simplicity, thermal stresses are not taken into account.

0k
ij


Tk
ij

2.2. Strain Energy Release Rate
As explained in the previous section, the laminate is
supposed to be damaged by “pre-existing” transverse and
longitudinal cracks. The size of the unit damaged cell
depends on the transverse and longitudinal damage levels
in the 90˚ and 0˚ layers. The strain energy release rate G
associated with the initiation and development of intra
ply cracking for a given stress state is defined by the fol-
lowing expression:

d, with
ddd
GUA UNMU
A
 

cel
 (2)
where is the strain energy of the whole laminate
d
U

and A is the cracked area. Let L1 denote the laminate
length in the x direction and L2 its width in the y direction
(Figure 1). The strain energy in the damaged unit cell is
denoted by cel
UN

(190 2atNL

) is the number of
transverse cracks and M (290
2
M
Lbt) is the number
of longitudinal cracks. Dimensionless quantities are
defined by, 90
x
xt

, 90 yyt

, 90 zzt, 90 hht,
90
aat

, 90
bbt and the constraining parameter is
090
tt


. The transverse crack density is defined by
dt (dt = 1/2a) and the longitudinal crack density is d(d =
1/2b). The crack area is l l

121

A
LL ab  
. We will
now distinguish between the strain energy release rate
associated with different damage mechanisms. The strain
energy release rates associated with transverse and
longitudinal cracking are denoted GFT and GFL respecti vely .
The transverse (resp. longitudinal) cracking growth is
characterized by the increase of the transv erse (resp . lon-
gitudinal) crack surface initiated in the 90˚ (resp. 0˚) lay-
ers.
All details are given in [25]. Then:
dd d
ddd
dd d
dd
d
dd
FT
dd
FL
UU a
G
A
aA
UU
b
G
A
A
b




(3)
whence
2
90
2
90
d
1
d 2
d 1
d 2
cel
FT cel
cel
FL cel
U
GUa
a
bt
U
GUb
b
at 









(4)
2.3. Decomposition of the Strain Energy
Release Rate
The strain energy (2) is decomposed to display the
contribution of each the stress product in the strain
energy. The following quantities are related to the
products in pairs of the components of the stress tensor,
they are given by the expression (8):
k
ij
U

90 090 0 12
90
12
90
22
2
ijij ijij ij
ij
LL
UNMUU UU
abt
LL U
abt
 


(5)
where:
  

 

  
22 2
2 2
22
0
90
00 0
0 0
12 00 000
022
2
yy zzyz
xx xz LT TT
ijxxyyzzyy zz
V
LT LTTTLT
vv
LL
Uxyz
EE EEGG
abt
 

 










ddd
(6)

Strain Energy Release Rate Analyse of Matrix Micro Cracking in Composite Cross-Ply La minates541
  


 

  
22
2 2
2
22
90
90
90 90
90 90
90
12 90909090 90
90 22
2
xx zz
yy yz
xz LT TT
ij yyxxzzxx zz
V
LTLTTT LT
vv
LL
Uxyz
EE EEGG abt



 


 

 

 
 
 

ddd
(7)
where V0 is the half volume of the 0˚ plies (
x
a

,
y
b, and 90 zt) and V90 is the half volume of the
90˚ plies (
x
a,
y
b, and 0 tzh) in the unit
cell. It can be remarked that in [26], cross-terms involv-
ing Poisson’s ratios were not taken into account.
We can deduce the 16 components by:
k
ij
U







2
90
2
0
2
90
2
0
2
90
2
0
90
90 90
1
0
00
1
90
90 90
2
0
00
2
90
90 90
3
0
00
3
90 90
4,
1ddd
2
1ddd
2
1ddd
2
1ddd
2
1ddd
2
1ddd
2
xx xx
V
T
xx xx
V
L
yy yy
V
L
yy yy
V
T
zz zz
V
T
zz zz
V
T
xx yy
UU xyz
E
UU xyz
E
U Uxyz
E
UU xyz
E
UU xyz
E
UU xyz
E
UU



















 
 
 
 
 
90
0
90
0
90
90 90
00
00
4,
90 90
90 90
5,
00 00
5,
90 90
90 90
6,
00
6,
ddd
ddd
ddd
ddd
ddd
LT
xx yy
V
L
LT
xx yyxxyy
V
L
LT
yy zzyyzz
V
L
LT
yy zzyyzz
V
L
LT
xx zzxxzz
V
L
xx zz
v
x
yz
E
v
UU xyz
E
v
UU xyz
E
v
UU xyz
E
v
U Uxyz
E
v
UU
















 




0
2
90
2
0
2
90
2
0
00
90
90 90
7
0
00
7
90
90 90
8
0
00
8
ddd
1ddd
2
1ddd
2
1ddd
2
1ddd
2
LT
xx zz
V
L
xz xz
V
TT
xz xz
V
LT
yz yz
V
LT
yz yz
V
TT
x
yz
E
UU xyz
G
UU xyz
G
UU xyz
G
UU xyz
G














(8)
The contribution of each selected component pair to
the strain energy release rate is such that:
d
d
k
ij
k
ij
U
G
x


where i = x, y, z and k = 0˚, 90˚ (9)
2.4. Respective Influence of Each Layer of the
Cross Ply Laminate
The whole strain energy release rate of the damaged
cross ply laminate can alternatively be decomposed into
two parts. The first part represents the contribution to the
strain e nergy of th e 0˚ layers on one h and and th e second
one is the contribution of the 90˚ layer to the strain en-
ergy release rate.
09
GG G
0
0
(10)
Using the present approach, we now estimate the re-
spective contribution of each damaged layer to the whole
strain energy release rate. This unfamiliar procedure will
help us to understand how damage evolves in cross ply
laminates and clarify the domain of validity of some
simplified approaches. We also applied this type of de-
composition () to six other models [12,24]
in order to predict the initiation of transverse matrix
cracking in the central layer of a cross ply laminate. The
present study allows assumptions used in several
simplified models to be validated. Analytical models
were used and a 3D finite element analysis was per-
formed [24]. In some models, when strong hypothses are
used to model the stress field distribution, the strain
energy is only attributable to the normal stress in the
damaged layer. Such models, which use simplified stress
field distributions give good results for the initiation of
the transverse cracking damage but they cannot describe
the characteristic damage state (CDS).
09
GG G
3. Results
The parameters involved in the present study are the
constraining parameter, the thickness of the two 0˚ and
90˚ layers and the nature of the material constituent sys-
tem. The constraining parameter is λ (λ = m/n), where n
is the number of plies with 90˚ orientation and m is the
number of plies with 0˚ orientation. Let us recall that the
hypothesis of continuous longitudinal cracks is used and
that the distributions of all transverse and longitudinal
cracks are supposed to be uniform. In all the displayed
results, the numerical simulations are carried out for a
prescribed uni-axial loading of 150 MPa. Only one
T300-914 graphite/epoxy material system is studied in
the following numerical computations (see Table 1). In

Strain Energy Release Rate Analyse of Matrix Micro Cracking in Composite Cross-Ply Laminates 542
Table 1. Mechanical properties and ply thickness of T300/
914 graphite epoxy system.
Graphite epoxy system T300/914
ELT (GPa) 140
ETT’ (GPa) 10
GLT (GPa) 5.7
GTT’ (GPa) 3.6
vLT 0.31
vTT’ 0.58
Ply thickness (mm) 0.125
Figures 2-4, the variation of the initiation of the strain
energy release rates is presented as a function of the con-
straining parameter λ for this graphite epoxy system. The
variation of the strain energy release rates is similar for
others materials systems not presented in this article. The
only difference lies in the numerical values of the strain
energy release rates.
3.1. Respective Contribution of Each Layer of
the Cross Ply Laminate
The first result concerns the respective contribution of
each layer of the damage laminate to whole strain energy
release rate. The numerical results are computed versus
the constraining parameter λ (λ = m/n) with 8-ply
cross-ply laminate. For studying damage initiation, we
used “a” (90 aat) as a damage parameter with a very
large numerical value. In [12], the computed results show
that when parameter “a” is greater than 8, the strain en-
ergy release rate associated with the initiation of trans-
verse cracking remains stable. Selvarathinam et al. [31]
use similar values suggested by experimental observa-
tions. Thus, in the present paper, we use this a-value to
predict damage initiation. In all the results displayed, the
strain energy release rate GFT (evaluated from Equation
(3)) associated with transverse crack damage has the most
important value. For the 8 ply cross-ply laminate at hand,
transverse cracking is thus the first observed dam age.
We can also remark that the strain energy release rates,
GFT or GFL (evaluated from Equations (3) and (4)) have
similar variations with the constraining parameter λ. All
the strain energy release rates are decreasing functions of
parameter λ. For instance, in an 8-ply laminate, when the
value of parameter λ is increased, the thickness of the 0˚
plies becomes greater. In this case, the fibers in the 0˚
plies carry most of the tensile loading and damage initia-
tion is delayed. Although no experimental data are re-
ported in Figures 2(a) and 2(b), the results of the nu-
merical simulations confirm two main points: the pro-
posed approach agrees with experimental data for the
initiation of transverse cracking as the first damage
mechanism. It also predicts the readiness to initiate other
types of damage in the case of an 8 ply laminate con-
taining a thick 90˚ layer. All results show that the main
part of the strain energy release rate is provided from the
90˚ damaged layer. This result can explain that simplified
models which only take into account the damaged 90˚
layer give correct results for damage initiation [26].
3.2. Contribution of Each Individual Stress
Component to the Strain Energy Release
Rate
The second result shows numerical simulations of the
variation of partial strain energy release rates which rep-
resent the contribution of each individual stress compo-
nent to the whole strain energy release rate. The results
provided by the different components are associated with
transverse cracking in the 90˚ layer (Figure 3(a)), trans-
verse cracking in the 0˚ layer (Figure 3(b)), longitudinal
cracking in the 90˚ layer (Figure 4(a)) and longitudinal
cracking in the 0˚ layer (Figure 4(b)). All the partial
values of the strain energy release rates are presented as
functions of the constraining parameter λ. All the results
are made dimensionless by dividing by the value of the
strain energy release rate GFT in the 90˚ layer or GFL in
the 0˚ layer respectively.
Concerning transverse damage, Figures 3(a) and 3(b)
show the contribution of the normal stress in the loading
direction. In both layers, this normal stress always has
the most important value. In the 90˚ layer, the contribu-
tion of this normal stress is practically constant and is
about 60% of total GFT. The rest is shared between the
contribution of the shearing component (σxz) and that of
the normal stress (σzz) in the direction normal to the
thickness of the laminate. For λ-values less than unity,
the influence of the shear stress is the most important and
for other λ-values, the normal stress contribution prevails.
All the other contributions (evaluated from Equation (8))
are practically zero. Concerning the 0˚ layers, only the
normal stress (σxx) and the shear stress (σxz) have a nota-
ble influence; the others stress components have no in-
fluence.
For longitudinal damage, Figures 4(a) and 4(b) show
different results. In the 90˚ layer, for λ-values less than 2,
the main contribution is due to the normal stress in the
thickness direction (σzz). For other λ-values, the contribu-
tion of (σyy) becomes the most important. One can notice
that the shear component (σyz) always has a small
non-zero contribution. All the other stress components
have no influence. In the 0˚ layer, the main influence is
that of the (σyy) component and it is a decreasing function
of the constraining parameter λ. We can also notice some

Strain Energy Release Rate Analyse of Matrix Micro Cracking in Composite Cross-Ply La minates 543
(a) (b)
Figure 2. Respective contribution of 0˚ and 90˚ layers to the strain energy release rate as functions of constraining parameter
λ. (a) Transverse crack (
090
F
TFTF
GGG
T
0
); (b) Longitudinal crack (
09
F
LFLF
GGG
L
).
(a) (b)
Figure 3. Variation of the portion of the strain energy release rate responsible for transverse cracking in the 90˚ layer (a) and
in the 0˚ layer (b) with constraining parameter λ.
(a) (b)
Figure 4. Variation of the portion of the strain energy release rate responsible for longitudinal cracking in the 90˚ layer (a)
and in the 0˚ layer (b) with constraining parameter λ.

Strain Energy Release Rate Analyse of Matrix Micro Cracking in Composite Cross-Ply Laminates 544
contributio n of the shear component (σyz). These numeri-
cal simulations leads us to a partial conclusion: when
studying the development of transverse and longitudinal
cracking, the contribution of the 90˚ layers stems from
the normal stresses (σxx), (σyy), (σzz) and the shear stress
(σxz), whereas the contribution of the 0˚ layers stems from
the normal stresses (σyy), (σzz) and the shear stress (σyz).
3.3. Transverse and Longitudinal Cracking
Initiation and Evolution
The third result (Figure 5) shows the variation of the GFT
and GFL strain energy release rates. The strain energy
release rate is plotted versus crack density (cm–1) for two
carbon epoxy 8-ply laminates; results are given for [02,
902]s and [0, 903]s laminates.
Both laminates exhibit similar variations with the
crack density. Laminate [0, 903]s which has a thick 90˚
layer, gives the most important GFT value. This is in good
agreement with experimental results, which show that it
is easier to initiate a crack in a compliant laminate. Using
the above re sult, one can study the variation of the strain
energy release rates GFT or GFL associated with the mul-
tiplication of transverse cracks or the initiation of the
longitudinal cracking respectively, with the transverse
crack density. The critical transverse crack density cor-
responding to the crack initiation in the 0˚ layer is ob-
tained at the intersection of the GFT and the GFL curves (if
the Gc of the transverse and longitudinal cracking value
are equal). This is confirmed by experimental results and
previous numerical results relying on the analyse of the
strain energy release rate. For the two laminates investi-
gated, the curves generally intersect for a transverse
crack density equal to 15 cm–1. At this stage of the trans-
verse damage, longitudinal cracking appears.
4. Conclusions
The laminate at hand is supposed to be “pre-damaged”
by transverse and longitudinal cracks to investigate the
initiation and development of transverse and longitudinal
cracking. Simplifying assumptions on the crack geometry,
crack distribution and continuous longitudinal cracks are
used. The respective contributions of the 0˚ and 90˚ plies
to the longitudinal crack damage are computed. Another
investigation displays the contributions of the different
components of the stress tensor. It is shown that, for
properly describing the longitudinal cracking process, a
refined computation of all the normal stresses and the
inclusion of some shear stress components are necessary.
Only one material system is studied. For other materials,
similar variations of the strain energy release rate G
would be obtained, with different numerical values. The
G-values are presented as functions of the constraining
parameter λ. Distinguishing between the strain energy
Figure 5. Partial strain energy release rates GFT and GFL
versus transverse crack density for two [02, 902]s and [0,
903]s laminates.
release rate in the 90˚ and 0˚ layers allows to show that for
small λ-values, it is easier to initiate damage. The curves
displayed confirm that transverse cracking first occurs in
the 90˚ layers. The results obtained for the contribution of
each component of the stress tensor show that only 5 terms
pertaining to the 3 normal stresses and 2 shearing stresses
have to be estimated, and that it is not necessary to take
into account the cross-terms involving Poisson’s ratios.
These results give an interpretation of Figure 5 for inves-
tigating the initiation of longitudinal cracks.
REFERENCES
[1] E. Urwald, “Influence de la Géométrie et de la Stratifica-
tion sur l’Endommagement par fatigue de Plaques Com-
posites Carbone/Époxyde,” Ph.D. Thesis, Université de
Poitiers, Poitiers, 1992.
[2] A. S. D. Wang and F. W. Crossman, “Initiation and
Growth of Transverse Cracks and Edge Delamination in
Composite Laminates. Part I: An Energy Method,’’ Jour-
nal of Composite Materials, Vol. 14, No. 1, 1980, pp.
71-87. doi:10.1177/002199838001400106
[3] R.-D. Jamison, K. Schulte, K.-L. Reifsnider and W.-W.
Stinchcomb, “Characterization and Analysis of Damage
Mechanisms in Tension-Tension Fatigue of Graph-
ite/Epoxy Laminates,” American Society for Testing and
Materials, ASTM STP 836, 1984, pp. 21-55.
[4] P. S. Steif, “Parabolic Shear-Lag Analyses of a [0/90]s
Laminate,” In: S. L. Ogin, P. A. Smith and P. W. R.
Beaumont Eds., Transverse Crack Growth and Associ-
ated Stiffness Reduction during the Fatigue of a Simple
Cross-Ply Laminate, Cambridge University, London,
1984, pp. 40-41.
[5] N. Law and G. J. Dvorak, “Progressive Transverse
Cracking in Composite Laminates,” Journal of Composite
Materials, Vol. 22, 1988, pp. 900-916.

Strain Energy Release Rate Analyse of Matrix Micro Cracking in Composite Cross-Ply La minates545
[6] S. G. Lim and C. S. Hong, “Effect of Transverse Cracks
on the Thermomechanical Properties of Cross-Ply Lami-
nated Composites,” Composites Science and Technology,
Vol. 34, No. 2, 1989, pp. 145-162.
doi:10.1016/0266-3538(89)90102-4
[7] E. Reissner, “On a Variational Theorem in Elasticity,”
Journal of Mathematical Physics, Vol. 29, No. 2, 1950,
pp. 90-95.
[8] J. Lemaître and J.-L. Chaboche, “Mechanics of Solid Ma-
terials,” Cambridge University Press, Cambridge, 1994.
[9] V. V. Vasil’ev and A. A. Duchenco, “Analysis of the
Tensile Deformation of Glass-Reinforced Plastics,” Me-
chanics of Composite Materials, Vol. 6, No. 1, 1970, pp.
127-130. doi:10.1007/BF00860460
[10] Z. Hashin, “Analysis of Cracked Laminates: A Varia-
tional Approach,” Mechanics of Materials, Vol. 4, No. 2,
1985, pp. 121-136. doi:10.1016/0167-6636(85)90011-0
[11] J. A. Nairn, “The Strain Energy Release Rate of Compos-
ite Micro Cracking: A Variational Approach,” Journal of
Composite Materials, Vol. 23, No. 11, 1989, pp. 1106-
1129. doi:10.1177/002199838902301102
[12] J.-L. Rebière, “Modélisation du Champ des Contraintes
Créé par des Fissures de Fatigue dans un Composite
Stratifié Carbone/Polymère,” Ph.D. dissertation, Univer-
sité de Poitiers, Poitiers, 1992.
[13] C. T. Herakovich, J. Aboudi, S. W. Lee and E. A. Strauss,
“Damage in Composite Laminates: Effects of Transverse
Cracks,” Mechanics of Materials, Vol. 7, No. 2, 1988, pp.
91-107. doi:10.1016/0167-6636(88)90008-7
[14] D. Gamby, C. Henaff-Gardin and J.-L. Rebière, “Model-
ling of the Damage Distribution along the Width of a
Composite Laminate Subjected to a Tensile Fatigue
Test,” 2nd International Conference on Computer Aided
Assessment and Control, Localized Damage II, South-
ampton, 1-3 July 1992, pp. 315-325.
[15] D. H. Allen, S. E. Groves and C. E. Harris, “A Ther-
momechanical Constitutive Theory for Elastic Compos-
ites with Distributed Damage. Part I: Theoretical Devel-
opment,” Mechanics and Materials Center, Texas A & M
University, Report MM-5023-85-17, 1985.
[16] O. Allix, P. Ladevèze and E. Le Dantec, “Modélisation de
l’Endommagement d’un Pli Élémentaire des Composites
Stratifiés,” Proceedings of 7th Journées Nationales des
Composites, Paris, November 1990, pp.715-724.
[17] S. Adali and R. K. Markins, “Effect of Transverse Matrix
Cracks on the Frequencies of Unsymmetrical, Cross-Ply
Laminates,” Journal of the Franklin Institute, Vol. 329,
No. 4, 1992, pp. 655-665.
doi:10.1016/0016-0032(92)90078-U
[18] J. R. Yeh, “The Mechanics of Multiple Transverse Crack-
ing in Composite Laminates,” International Journal of
Solids and Structures, Vol. 25, No. 12, 1989, pp. 1445-
1455.doi:10.1016/0020-7683(89)90111-X
[19] A. K. Kaw and G. H. Besterfield, “Mechanics of Multiple
Periodic Brittle Matrix Cracks in Unidirectional Fi-
ber-Reinforced Composites,” International Journal of
Solids and Structures, Vol. 29, No. 10, 1992, pp.
1193-1207. doi:10.1016/0020-7683(92)90231-H
[20] Z. Hashin, “Analysis of Orthogonally Cracked Laminates
under Tension,” Journal of Applied Mechanics, Vol. 54,
No. 4, 1987, pp. 872-879. doi:10.1115/1.3173131
[21] W. K. Binienda, A. Hong and G. D. Roberts, “Influence
of Material Parameters on Strain Energy Release Rates
for Cross-Ply Laminate with Pre-Existing Transverse
Crack,” Composites Engineering, Vol. 4, No. 12, 1994,
pp. 1167- 1210. doi:10.1016/0961-9526(95)91390-3
[22] J.-L. Rebière, M.-N. Maâtallah and D. Gamby, “Initiation
and Growth of Transverse and Longitudinal Cracks in
Composite Cross-Ply Laminates,” Composite Structures,
Vol. 53, No. 2, 2001, pp. 173-187.
doi:10.1016/S0263-8223(01)00002-2
[23] N.-V. Akshantala and R. Talreja, “A Micromechanics
Based Model for Predicting Fatigue Life of Composite
Laminate,” Materials Science and Engineering A, Vol.
285, No. 1-2, 2000, pp. 303-313.
doi:10.1016/S0921-5093(00)00679-1
[24] D. Gamby, J. Martins, S. Maison and J.-L. Rebière, “In-
fluence du Confinement sur le Taux de restitution
d’Énergie Associé à la Fissuration Transverse d’un Strati-
fié. Comparaison de Deux Simulations Numériques,”
Proceedings of 7th Journées Nationales des Composites,
Lyon, November 1990, pp. 609-617.
[25] J.-L. Rebière, M.-N. Maâtallah and D. Gamby, “Analy sis
of Damage Mode Transition in a Cross-Ply Laminate un-
der Uniaxial Loading,” Composite Structures, Vol. 55,
No. 2, 2002, pp. 115-126.
[26] J.-L. Rebière and D. Gamby, “A Criterion for Modelling
Initiation and Propagation of Matrix Cracking and De-
lamination in Cross-Ply Laminates,” Composites Science
and Technology, Vol. 64, No. 13-14, 2004, pp. 2239-2250.
doi:10.1016/j.compscitech.2004.03.008
[27] L. N. McCartney, “Energy-Based Prediction of Progres-
sive Ply Cracking and Strength of General Symmetric
Laminates Using an Homogenisation Method,” Compos-
ites Part A, Vol. 36, No. 2, 2005, pp. 119-128.
[28] E. J. Barbero and D. H. Cortes, “A Mechanistic Model for
Transverse Damage Initiation, Evolution, and Stiffness
Reduction in Laminated Composites,” Composites Part B,
Vol. 41, No. 2, 2010, pp. 124-132.
doi:10.1016/j.compositesb.2009.10.001
[29] J.-L. Rebière and D. Gamby, “A Decomposition of the
Strain Energy Release Rate Associated with the Initiation
of Transverse Cracking, Longitudinal Cracking and de-
lamination in Cross-Ply Laminates,” Composite Struc-
tures, Vol. 84, No. 2, 2008, pp. 186-197.
doi:10.1016/j.compstruct.2007.12.004
[30] K. L. Reifnider and R. D. Jamison, “Fracture of Fa-
tigue-Loaded Composite Laminates,” International Jour-
nal of Fatigue, Vol. 4, No. 4, 1982, pp. 187-197.
doi:10.1016/0142-1123(82)90001-9
[31] A. S. Selvarathinam and Y. J. Weistman, “A Shear Lag
Analysis of Transverse Cracking and Delamination in
Cross-Ply Carbon-Fibre/Epoxy Composites under Dry,
Saturated and Immersed Fatigue Conditions,” Composites

Strain Energy Release Rate Analyse of Matrix Micro Cracking in Composite Cross-Ply Laminates 546
Science and Technology, Vol. 59, No. 14, 1999, pp. 2115-2123. doi:10.1016/S0266-3538(99)00069-X