Paper Menu >>
Journal Menu >>
 Advances in Pure Mathematics, 2011, 1, 49-53 doi: 10.4236/apm.2011.13011 Published Online May 2011 (http://www.SciRP.org/journal/apm) Copyright © 2011 SciRes. APM New Results on Oscillation of even Order Neutral Differential Equations with Deviating Arguments Lianzhong Li1, Fanwei Meng2 1School of Mathematical and System Sciences, Taishan College, Tai’an, China 2School of Mathematical Sciences, Qufu Normal University, Qufu, China E-mail: llz3497@163.com , fwmeng@qfnu.edu.cn Received January 19, 2011; revised March 15, 2011; accepted Ma rc h 20, 2011 Abstract In this paper, we point out some small mistakes in [6] and revise them, we obtain some new oscillation re- sults for certain even order neutral differential equations with deviating arguments. Our results extend and improve many known oscillation criteria because the article just generalizes Meng and Xu’s results. Keywords: Oscillation, Neutral Differential Equation, Deviating Argument 1. Introduction Oscillation of some even order differential equations have been studied by many authors. For instance, see [1-7] and the references therein. We deal with the oscil- latory behavior of the even order neutral differential eq- uations with deviating arguments of the form 1 1 0 0, n m ii i l jj j j xtp txt qtfx t tt (1) where 2n is even, throughout this paper, it is as- sumed that: (A1) 0 ,,,,,,0 ijj j pqC tRfCRR ufu for 0uand j f uis non-decreasing on R, 1, 2,,,1, 2,,;imjl (A2) 0,, , ii CtRttt and lim , i tt 1, 2,,im; (A3) 1,, ,,lim jjj t CtRt tt and 0 jt , 1, 2,,;jl (A4) There exists a constant M0 such that sgn M j f xx x for 0,1,2, , x jl; (A5) 1 ,0,1 m i i pt pp and there exists a func- tion 0,,qtC tR such that, min : j qtq t 1, 2,,jl. By a solution of Equation (1) we mean a function x twhich has the pro perty that 1 m ii i x tptxt ,, nx Ct R for some 0x tt and satisfies Equa- tion (1) on , x t . We restrict our attention to those solutions x t of Equation (1) which exist on some half-line , x t with sup :0xttT for any x Tt. A nontrivial solution of Equation (1) is called oscillatory if it has arbitrarily large zeros, otherwise it is said to be nonoscillatory. Equation (1) is said to be os- cillatory if all of it’s nontrivial solutions are oscillatory. Recently, Meng and Xu [6] studied Equation (1) and obtained some sufficient conditions for oscillation of the Equation (1), we list the main results of [6] as follows. Following Philos [5], we say that a function , H Hts belongs to a function class W, denotes by H W , if , H CDR , where 0 ,:Dtstst, which satisfies: (H1) ,0Htt and ,0Hts for 0 tst ; (H2) H has a continuous non-positive partial derivative H S satisfying the condition: ,,, H tsk s hts Hts Sks for some , loc hL DR 10 ,,,0,kCt is a non-decreasing function. Theorem A ([6, Theo re m 2.1]). Assume that (A1) - (A5) hold, let the functions ,, H hksatisfy (H1) and (H2), suppose 12 1 limsup ,, 4 tCFtrGtr C (2)  L. Z. LI ET AL. Copyright © 2011 SciRes. APM 50 holds fo r every 01 2 ,0,0,rtC C where 1 2 2 1 ,,d, , , 1 ,d ,, l t j rj t n rjj F trH tsk sqss Htr kshts Gtr s Htr Htss s and 1p , then every solution of Equation (1) is oscillatory. Theorem B ([6, The orem 2.2]). Assume that (A1)-(A5) hold, and ,, H hk are the same as in Th eorem A, suppose that 00 , inf 0 , liminf st t Hts Htt (3) and 0 limsup , tGtt (4) If there exists a function 0,,mCt R such that for all 0 tTt. 12 1 ,, 4 liminf tCFtTGtTm T C (5) and 0 22 limsupd,1,2,, n tjj t t m s jl k sss s (6) where max ,0mtmt , then every solution of Equation (1) is oscillatory. In Theorem A and B, function ,Gtr should be , j Gtr, so each of the condition (2), (4), (5) and (6) has as many as l conditions. Meanwhile, the Riccati func- tion t is not well-defined and there exist some small errors in the proof of the theorems. The purpose of this paper is further to strengthen oscillation results ob- tained for Equation (1) by Meng and Xu [6]. In our paper, we redefine the functions ,,,,tr tFrGt and provide some new oscillation criteria for oscillation of Equation (1). 2. Main Results In the sequel, we need the following lemmas: Lemma 2.1 ([1]). Let x t be a n times differentiable function on 0,t of one sign, 0 n xt on 0,t which satisfies () 0 n xtxt. Then: (I1) There exists a 10 tt such that ,1,2,,1 i x ti n are of one sign on 1,t; (I2) There exists a number 1, 3, 5,,1hn when n is even, or 2,4,6, ,1hn when n is odd, such that 0 i xtxt for 0,1, ,ih,1;tt 1 10 ni i xtx t for 1 1,2,,,ihhn tt. Lemma 2.2 ([1]). If x t is as in Lemma 2.1 and 10 nn xxtt for 0 tt, then for every 01 , there exists a constant 0N, such that 1 1n n Nt txt X for all large t. Lemma 2.3([7]). Suppose that x t is an eventually positive solution of Equation (1), let 1 m ii i zx pxttt t , then there exists a number 10 tt such that 0,zt 1 0, 0 n zztt and 1 0, nttzt. Theorem 2.1 Assume that (A1) - (A5) hold, let the functions ,, H hk satisfy (H1) and (H2), suppose limsup ,, 4 tMFtrGt r N (7) holds fo r every 0 rt and for some 1 , where 2 2 1 1 ,,d , , 1 ,d ,, t r t l rn jj j FtrHtsksqs s Htr ksh ts Gtr s Htr Htsss and 1p , then every solution of Equation (1) is oscillatory. Proof. Suppose to the contrary that x t is a nonoscillatory solution of Equation (1) and that x t is even- tually positive (when x t is eventually negative, the proof is similar). Let zt be defined as in Lemma 2.3, then following the proof of Theorem 2.1 in [6], without loss of generality, assume there exists a 10 tt such that (1) 0, 0,0, 0, 0 njj xt tt tt z zzt z z 1 2n n jj tNtzt z (by lemma 2.2) and 1 l njj j ttzMqzt for all 1 tt. Let 1 1 n l j j z k z t tt t (not as [6]), then we have 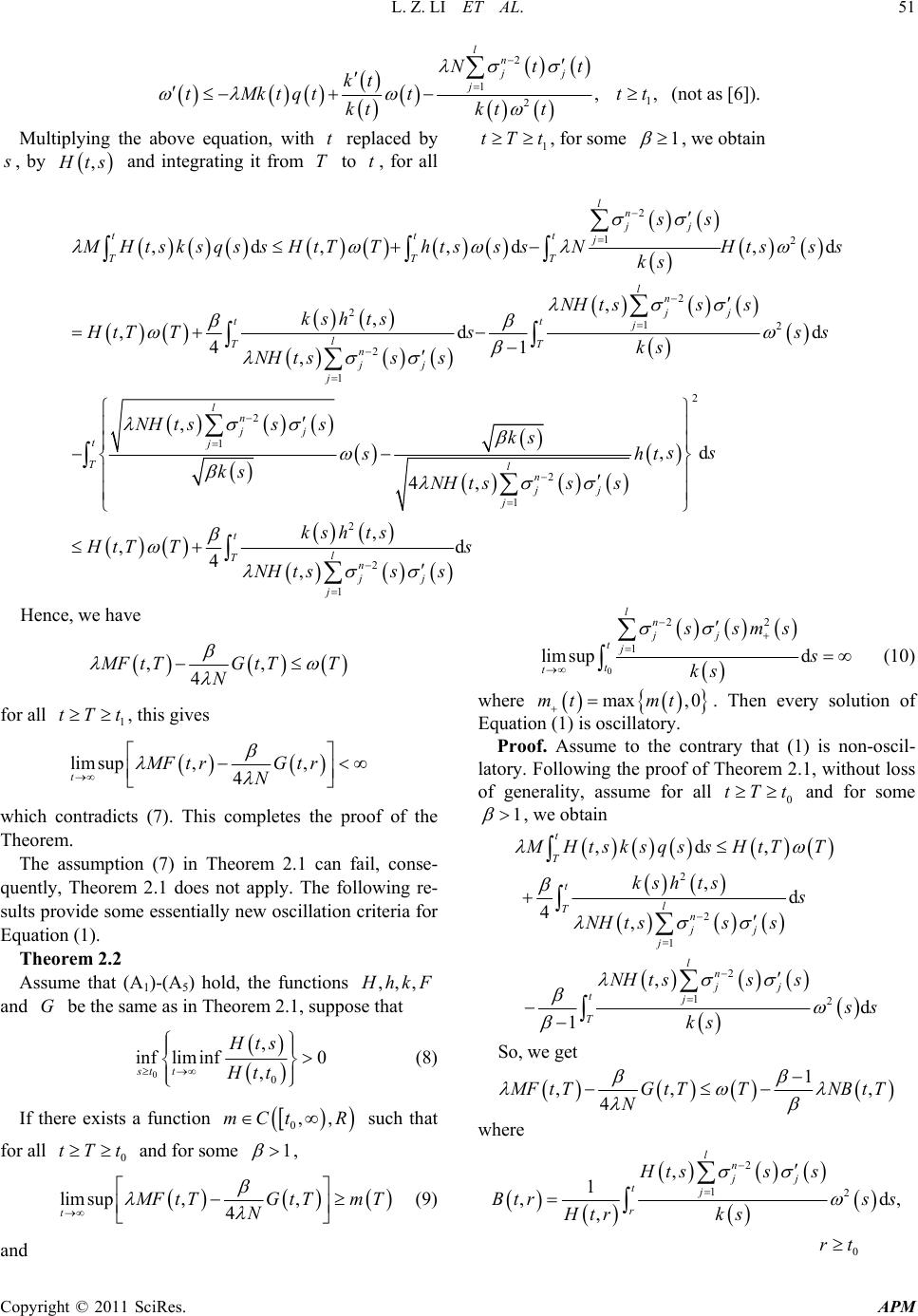 L. Z. LI ET AL. Copyright © 2011 SciRes. APM 51 2 11 2,, ln jj j N k Mk qt tt t tt ttt kt kt t (not as [6]). Multiplying the above equation, with t replaced by s , by ,Hts and integrating it from T to t, for all 1 tTt, for some 1 , we obtain 2 12 2 212 2 1 2 1 2 1 ,, , , , , , d, dd ,d d 41 ,4 ln jj ttt j TTT ln jj tt j l TT n jj j ln jj j ln jj j M HkqsHtTTh sNHs k NH kh H tT Tss k NH ss ts s stsstss s tss s sts s s tss s ts sNH kh ss st stss s kNH 2 2 2 1 d , , , ,d 4 t T t l Tn jj j s k s sh Ht ts ts s TT s NH s Hence, we have ,, 4 M FtTGtT T N for all 1 tTt, this gives limsup ,, 4 tMFt rGtr N which contradicts (7). This completes the proof of the Theorem. The assumption (7) in Theorem 2.1 can fail, conse- quently, Theorem 2.1 does not apply. The following re- sults provide some essentially new oscillation criteria for Equation (1). Theorem 2.2 Assume that (A1)-(A5) hold, the functions ,,, H hkF and G be the same as in Theorem 2.1, suppose that 00 , inf 0 , liminf st t Hts Htt (8) If there exists a function 0,,mCt R such that for all 0 tTt and for some 1 , l,m,isup4 tMtT tTFmTG N (9) and 0 22 1 limsup d ln jj tj t t msss ss k (10) where max ,0mt mt . Then every solution of Equation (1) is oscillato ry. Proof. Assume to the contrary that (1) is non-oscil- latory. Following the proof of Theo rem 2.1, without loss of generality, assume for all 0 tTt and for some 1 , we obtain 2 2 1 2 12 , , , , d, d 4 d 1 t T t l Tn jj j ln jj tj T tsss sts tss s tss s s s MHk qsHtTT kh s NH NH s k So, we get 1 ,, , 4 M FtTGtT TNBtT N where 2 12 0 , ,, 1d, ln jj tj r H Bs Hk tss s tr s tr s rt  L. Z. LI ET AL. Copyright © 2011 SciRes. APM 52 then ,, 4 1limin ms , lup f i t t M FtT GtT N TNBtT For all 0 Tt and for any 1 , by (9) we have 1liminf , t TmTN BtT So TmT (11) and especially 000 liminf ,(1) tBttt mt N (12) Now, we claim that 0 2 12 limsup d ln jj tj t t ss ss sk (13) Suppose to the contrary that 0 2 12 limsup d ln jj tj t t ss ss sk (14) By (8), there is a positive constant satisfying 00 , inf liminf0 , st t Hts Htt (15) Let be any arbitrary positive number, from (14) there exists a 10 tt such that, 0 2 12d ln jj tj t ss s ss k for all 1 tt Then, for 1 tt, we have 10 10 2 12 2 0 0 0 1 12 0 , , , , , 1d 1 , , dd ln jj ts j tt ln jj ts j tt tt vv tsd v tt v vv ts v tt v t B H v Hk H vs t Hk H tHt By (15), there exists a 21 tt such that, for all 2 tt, 1 0 , , Htt Htt , which implies 0 ,Btt for all 2 tt. Since is arbitrary, we have 00 liminf,lim , tt Btt Btt which contradicts (12), thus (13) holds. Then by (11) and (13) we get 0 0 2 12 2 12 limsu p limsu p d d, ln jj tj t ln jj t t t j t ms k s k ss s s ss s s which contradicts (10). This completes the proof. Remark 1 Let 1 in Theorem 2.1, Theorem 2.1 reduces to Theorem A [6]; we obtain the same result in Theorem 2.2 in which we omit the assumption (4) in Theorem B [6]. Therefore, Theorem 2.1 and 2.2 are gen- eralizations and improvements of the results obtained in [6]. Remark 2 With an appropriate choices of the func- tions , H h and k, one can derive a number of oscilla- tion criteria for Equation (1) from our theorems. Let ()1,0kt is a constant, ,tssHt , 1 ,,ts sht 0 tst , and we have 00 ,, limlim 1 ,, tt Hts ts Htt tt for any 0 s t. Consequently, let 2 , using Theorem 2.2, we have: Corollary 2.1 Assume that (A1)-(A5) and (8) hold, suppose that there exists a function 0,,mCt R such that, fo r some 1 , 2 2 0 2 1 1 limsup d , l tn jj j t T M tsqs s tNss mTt Tt (16) and (10) (with 1ks ) hold. Then every solution of Equation (1) is oscillatory. Example 1 Let 4,t , consider the following second order neutral differential equation 0xt ptx tqtxt (17)  L. Z. LI ET AL. Copyright © 2011 SciRes. APM 53 where 1,max21sin,0, 2 ptqtttf xx , 4 4d 12sin t ts ss , in this case 1 M , Let 1, 2N , by direct calculation, we get 2 2 2 2 1 limsup d 1 limsup1sin12 sind cossincos 1 t T t t T t Mt s qss Ns t tsssss s t mTTT TT It is easy to verify that (10) holds, therefore, Equation (17) is oscillatory by Corollary 2.1. However, we can easily find that 0 2 01 limsup limsupd,12sin t t tt Gstts s t so condition (4) in Theorem B is not satisfied, these show that Theorem B cannot be applied to Equatio n (17 ). Obviously our results are superior to the resu lts obtained before. 3. Acknowledegments The authors are very grateful to the referee for his/her valuable suggestions. 4. References [1] R. P. Agarwal, S. R. Grace and D. ORegan, “Oscillation Theory for Differential Equations,” Kluwer Academic, Dordrecht, 2000. [2] R. P. Agarwal and S. R. Grace, “The Oscillation of High- er Order Differential Equations with Deviating Argu- ments,” Computers & Mathematics with Applications, Vol. 38, No. 3-4, 1999, pp. 185-199. doi:10.1016/S0898-1221(99)00193-5 [3] Y. Bolat and O. Akin, “Oscillatory Behavior of Higher Order Neutral Type Nonlinear Forced Differential Equa- tion with Oscillating Coefficients,” Journal of Mathe- matical Analysis and Applications, Vol. 290, No. 1, 2004, pp. 302-309. doi:10.1016/j.jmaa.2003.09.062 [4] W. N. Li, “Oscillation of Higher Order Delay Differential Equations of Neutral Type,” The Georgian Mathematical Journal, Vol. 7, No. 2, 2000, pp. 347-353. [5] Ch. G. Philos, “Oscillation Theorems for Linear Differ- ential Equations of Second Order,” Archiv der Mathe- matik, Vol. 53, No. 5, 1989, p. 483. doi:10.1007/BF01324723 [6] F. Meng and R. Xu, “Kamenev-Type Oscillation Criteria for Even Order Neutral Differential Equations with Devi- ating Arguments,” Applied Mathematics and Computa- tion, Vol. 190, No. 2, 2007, pp. 1402-1408. doi:10.1016/j.amc.2007.02.017 [7] Yu. V. Rogovchenko and F. Tuncay, “Oscillation Criteria For Second-Order Nonlinear Differential Equations with Damping,” Nonlinear Analysis: Theory, Methods & Ap- plications, Vol. 69, No. 1, 2008, pp. 208-221. doi:10.1016/j.na.2007.05.012 |

