Theoretical Economics Letters, 2011, 1, 1-2
doi:10.4236/tel.[phone] Published Online May 2011 (http://www.SciRP.org/journal/tel)
A New Method of Estimating the Asset Rate of Return
Moawia Alghalith, Tracy Polius
Economics, University of the West Indies, St Augustine, USA
Received April 19, 2011; revised April 21, 2011; accepted April 25, 2011
Abstract
We present a new consumption-based method of estimating the asset rate of return.
Keywords: Return, Investment, Portfolio, Asset, Stochastic, Consumption CAPM
In this note, we present a new model that links the
stock/portfolio rate of return to consumption. Our app-
roach is more general than the existing models such as
the consumption-CAPM models, that are based on very
restrictive assumptions [1]. In so doing, we utilize a more
advanced and appropriate theoretical and empirical
framework than the ones used by previous literature. It is
worth noting that previous literature mainly used simple
linear regressions without a rigorous theoretical basis.
We use a stochastic factor model, which includes a
risky asset (portfolio, a risk-free asset and a stochastic
external economic factor [2,3]. Thus, we have a two-
dimensional standard Brownian motion



12
,,
sss
tsT
WW   on the probability space

,,
sP,
where

stsT
 is the augmentation of filtration. The
risk-free asset price process is

d
0=e ,
T
rZs
s
t
S where
 
2
sb
rZC R, is the rate of return and
s
Z
is the sto-
chastic economic factor.
The dynamics of the risky asset price is given by
 

1
d=dd ,
stss s
SS ZtZW   (1)
where

t
Z
 and

t
Z
 are the rate of return and the
volatility, respectively. The stochastic economic factor
process is defined as

2
12
d=dd1d ,=,
ss sst
Z
aztWW Zz   (2)
where <1 
is the correlation factor between the two
Brownian motions,

is a parameter, and
 
1
s
aZC R has a bounded derivative.
The wealth process is given by
  


π,,
1
=πd
πd,
T
cc
Tssssss
t
T
ss s
t
X
xrYXYrY cs
YW







(3)
where
x
is the initial wealth,

π,
ts
tsT
 is the
portfolio process and


,
ts
tsT
c  is the consumption
process, with 2 πd<
T
s
t
s

, d<
T
s
t
cs 
 and 0c. The
trading strategy

 
π,,
ss
cAxy is admissible.
The investor’s objective is to maximize the expected
utility of terminal wealth and consumption



π,
12
π,
,, =Supd,
T
c
Tst
ct
tt
vtxyEU XUcs









 (4)
where


.v is the (smooth) value function,


.U,
bounded and strictly concave utility function.
The value function satisfies the Hamiltonian-Jacobi-
Bellman PDE
 
 



22
π,
2
1
2
1
Sup ππ
2
0,
txyyy
txxt tx
c
t
txyt
vryxvazv v
zvzrzcv
zv uc

 















,, =,vTxzU x (5)
Hence, the optimal solutions are






 
2
π=,
xxy
t
x
x xx
zrzv v
zv zv

 


(6)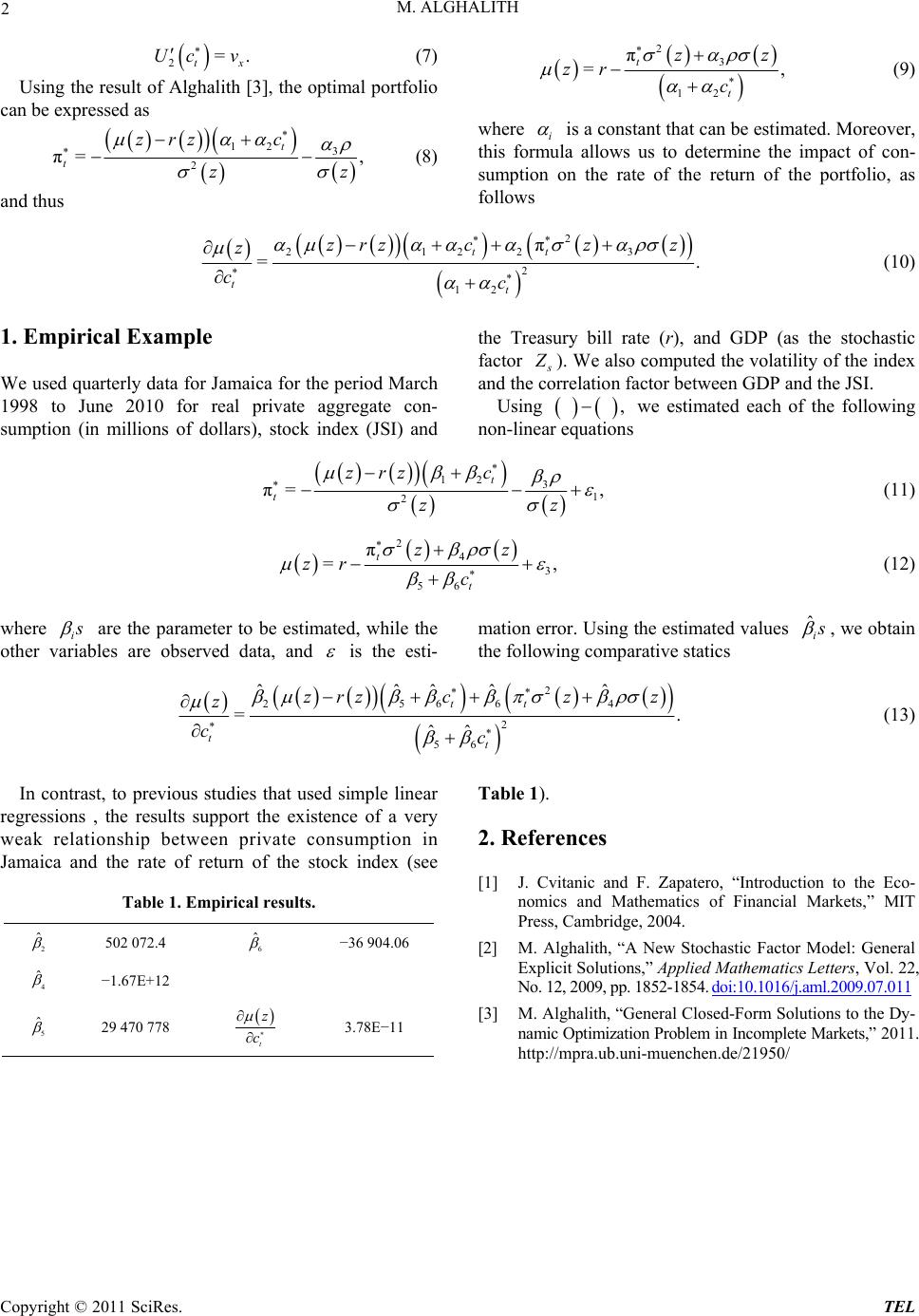

M. ALGHALITH
2

2=. tx
Uc v

 (7)
Using the result of Alghalith [3], the optimal portfolio
can be expressed as
  

 
12 3
2
π=,
t
t
zrz c
z z







 (8)
and thus




2
3
12
π
=,
t
t
zz
zr c





 (9)
where i

is a constant that can be estimated. Moreover,
this formula allows us to determine the impact of con-
sumption on the rate of the return of the portfolio, as
follows
 

 









2
21223
2
12
π
=.
tt
tt
zrz czz
z
cc
 






 (10)
1. Empirical Example
We used quarterly data for Jamaica for the period March
1998 to June 2010 for real private aggregate con-
sumption (in millions of dollars), stock index (JSI) and
the Treasury bill rate (r), and GDP (as the stochastic
factor
s
Z
). We also computed the volatility of the index
and the correlation factor between GDP and the JSI.
Using




, we estimated each of the following
non-linear equations


 


 
12 3
1
2
π=,
t
t
zrz c
z z








(11)





2
4
3
56
π
=,
t
t
zz
zr c








 (12)
where i
s

are the parameter to be estimated, while the
other variables are observed data, and

is the esti-
mation error. Using the estimated values ˆi
s

, we obtain
the following comparative statics
  



 



2
25664
2
56
ˆˆˆˆˆ
=.
ˆˆ
tt
tt
zrz cz z
z
cc
 




 


(13)
In contrast, to previous studies that used simple linear
regressions , the results support the existence of a very
weak relationship between private consumption in
Jamaica and the rate of return of the stock index (see
Table 1. Empirical results.
2
ˆ

502 072.4 6
ˆ

−36 904.06
4
ˆ

−1.67E+12
5
ˆ

29 470 778

t
z
c



 3.78E−11
Table 1).
2. References
[1] J. Cvitanic and F. Zapatero, “Introduction to the Eco-
nomics and Mathematics of Financial Markets,” MIT
Press, Cambridge, 2004.
[2] M. Alghalith, “A New Stochastic Factor Model: General
Explicit Solutions,” Applied Mathematics Letters, Vol. 22,
No. 12, 2009, pp. 1852-1854. doi:10.1016/j. aml.2009 .07.011
[3] M. Alghalith, “General Closed-Form Solutions to the Dy-
namic Optimization Problem in Incomplete Markets,” 2011.
http://mpra.ub.uni-muenchen.de/21950/