Journal of Modern Physics
Vol.08 No.08(2017), Article ID:77482,17 pages
10.4236/jmp.2017.88077
Quanta of the Phase-Space Areas Given by Intervals of Energy and Time Associated with Electron Transitions
Stanisław Olszewski
Institute of Physical Chemistry, Polish Academy of Sciences, Warsaw, Poland

Copyright © 2017 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: May 9, 2017; Accepted: July 4, 2017; Published: July 7, 2017
ABSTRACT
The paper examines the energy of electron transitions in an emission process and the time intervals necessary for that process. For simple quantum systems, the both parameters―that of energy and time―depend on the difference  of the quantum numbers n labelling the beginning and end state of emission. It is shown that the phase-space areas formed by products of energy and time involved in the emission can be represented as a quadratic function of
of the quantum numbers n labelling the beginning and end state of emission. It is shown that the phase-space areas formed by products of energy and time involved in the emission can be represented as a quadratic function of 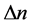 multiplied by the Planck constant h.
multiplied by the Planck constant h.
Keywords:
Electron Transitions, Their Energies and Transition Times, Quanta of the Phase-Space Areas of Energy and Time

1. Introduction
The phase space of energy and time seems to be much less discussed and applied in physics than the phase space based on the particle momentum and position. Nevertheless, in classical mechanics, the existence of the phase space of energy and time can be noticed for example in the case of the solar system; see e.g. [1] [2] [3] [4] .
In the quantum theory some ideas of the phase space of energy and time were applied many years ago by Ehrenfest [5] . Characteristically, the same author presented the use of the Hamilton equations entering the classical theory also in the case when the motion of the quantum wave packets should be described [6] [7] . More explicitly, the phase-space elements have been introduced into the quantum theory by Heisenberg [7] [8] whose uncertainty principle stated that any product of the energy interval 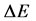 and corresponding time interval
and corresponding time interval 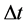 should satisfy the relation
should satisfy the relation
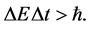 (1)
(1)
The presentation of (1) was done together with an introduction of the mo- mentum-position uncertainty relations
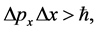 (2)
(2)
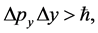 (2a)
(2a)
 (2b)
(2b)
satisfied by the Cartesian coordinates of the momentum and position intervals of a particle.
There exists a difference in considering the physical background of the Formula (1) and those of (2)-(2b); see [9] [10] . In fact the validity of (1) was objected in [11] [12] [13] , in some textbooks the presentation of (1) is neglected at all, see e.g. [14] [15] . A modification of (1) which occurred to be helpful in defining minimal distances of two Fermion particles in space and time, has been introduced in [16] [17] [18] [19] .
In fact, the energy and time coupled in (1) are much different parameters also from the point of view of the quantum theory. The energy seems to be a most commonly considered observable also for the quantum transitions. On the other hand, the time is not a much welcomed parameter in quantum theory, especially when a physical object is submitted to some change. A reason of this second behaviour is due to a probabilistic foundation of the theory. This situation, however, does not facilitate the answer to a rather natural question concerning the duration of any quantum process in the matter.
Such a process can be, for example, the change of the occupation of one of quantum states, say that of a higher energy , to another state whose energy
, to another state whose energy 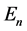 is lower than in the first state. How long is the duration time of the emission process? We tried to answer this question basing on a classical Joule- Lenz formula for the dissipated energy and found that if transition is going between two neighbouring quantum states, say having the indices
is lower than in the first state. How long is the duration time of the emission process? We tried to answer this question basing on a classical Joule- Lenz formula for the dissipated energy and found that if transition is going between two neighbouring quantum states, say having the indices 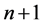 and
and , so
, so
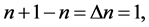 (3)
(3)
the transition time
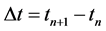 (4)
(4)
is coupled with transition energy
 (5)
(5)
by the formula
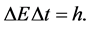 (6)
(6)
The accuracy with which (6) is satisfied increases with the number n [19] [20] [21] [22] . The aim of the present paper is to extend the Formula (6) valid for the case
 (6a)
(6a)
to the situation when the product
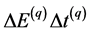 (7)
(7)
is considered. Here q is assumed to be an integer number larger than unity. Simultaneously we shall assume that

In brief, the aim of the paper is to present the calculations of the phase-space areas (7) done―with the aid of condition (7a)―for simple quantum systems: the free-electron particle enclosed in a one-dimensional potential box, electron in the hydrogen atom and electron motion considered as a linear harmonic oscillator. Following the formalism outlined in Section 2 the task is accomplish- ed in Sections 3-5. The formula summarizing the phase-space results obtained for energy and time valid for all quantum systems mentioned above is given in Equation (11). Additionally, the case of a free particle in the potential box is supplemented by the phase-space areas and their quanta calculated for the momentum and position coordinates of the electron particle; see Section 3.
Formally the calculations concern the parameters entering the Heisenberg formula for the uncertainty principle of energy and time [see (1)] and―for a free particle―also the Heisenberg formula of uncertainty concerning the momentum and position coordinates; see (2). However in the present paper a general uncertainty-like treatment of physical parameters given by Heisenberg does not apply, but is replaced by a concrete approach to the size of energy and time intervals entering the electron transition process between two definite quantum levels. In quantum mechanics such an approach is regularly avoided because of a probabilistic footing of the formalism applied in the treatment of electron transitions; in effect the quantum-mechanical calculations of a definite time interval connected with an individual electron transition can never take place.
The gain of the present paper was to demonstrate how the intervals of time associated with transitions could be obtained on a semiclassical reasoning and next applied giving an extension of a former approach done for the case when the electron transitions only between the nearest-neighbouring quantum levels are considered [19] [20] [21] .
2. Transition Energy and Transition Time for
In principle any interval of energy

can be decomposed into a sum of successive energy intevals




A full transition energy (8) is a sum of (8a), (8b), (8c), (8d).
But any of the component intervals (8a), (8b), (8c), (8d) provides us―accor- ding to (4) and (5)―to the transition time between level n and level

This is so because the whole time interval (9) is a sum of the component time intervals:




Evidently the interval (9) becomes a sum of (9a), (9b), (9c), (9d).
The method outlined above has been successfully applied in calculating the emission intensity in the hydrogen atom [23] [24] . In the next Sections we show that for

the phase-space areas of energy and time attain the result

where q is given by the Formula (7a).
Moreover it will be shown for the free particles (electrons) that the Cartesian intervals 


formally similar to (11).
3. The De-Excitation Energy of a Free Electron Examined for a One-Dimensional Potential Box
In a one-dimensional box of length 


For the case of a transition between the nearest quantum levels 

where the last step is dictated by the relation
By assuming that

we obtain the electron velocity:

The time period 


The quantum aspects of the Joule-Lenz law imply that [22] [23] [24]

In effect we obtain

see (6). But since

we have

The change of the electron position in course of the time 

Therefore

which is identical with the result obtained in (18).
In the next step let us consider the case of

and the time interval corresponding to (23) is:

on the basis of (9), (14) and (23). Simultaneously with the definition of (19)

In effect of (14), (23) and (24) we obtain

But the Formulaes (24) and (15) give

so from (25) and (27)

which gives on the right the result identical with that calculated in (26).
The next step concerns

In this case the decrease of energy is

obtained within the time interval

In effect Equations (30) and (31) provide us with product

The momentum change in course of transition (29) is

and from (15) and (31)

Therefore

This is a product equal to (32).
As a final step let us take

The time interval corresponding to transition (36) is

see (9).
The momentum increment for 

The change 


In effect from (36) and (37) we obtain

But a result identical to that presented in (40) gives also the product of (38) and (39):

Therefore both Formulae (40) and (41) are satisfied for the one-dimensional free-electron case. The calculations can be easily prolongated to an arbitrary size of
4. Phase-Space Areas of Energy and Time Characteristic for the Electron Transitions in the Hydrogen Atom
The electron energy in state n of the hydrogen atom is in virtue of the virial theorem equal to

where

is the kinetic electron energy. This holds because the electron velocity on the orbit n is [26]

In the last step of (44) we have introduced the radius 

and the circulation time period 

which is a known result [26] .
Let us take into account the energy difference

where at the end of (47) the large n are considered. In [19] [20] [21] [22] we have pointed out that

therefore from the Formulaes (43), (46) and (48) we obtain the product

This is a result identical with (6) and (18). Let us note that

and

because 

This formula is similar to that obtained in the astronomy of the solar system. For G equal to the gravitational constant, M-solar mass, m-planet mass, T-the circulation period of a planet, and a-the larger semiaxis of the planetary orbit, we have [1] [2]

where E is the energy of the planetary motion and a can be roughly represented by the radius r of a circle, so expression

is approximately equal to the angular momentum of a planet; v is the planet velocity.
In the next step let us show that the phase-space result (26) for 

see (47). Therefore

because of (47). On the other side

This gives

which is a result presented before in (26).
Similar identity of the phase-space areas can be obtained for larger energy differences. For 

and

The product of (59) and (60) provides us with the result

obtained already in (32) for a free electron.
Also the case of 

and

The last two formulae give the product

obtained earlier in (40).
5. Phase-Space Areas for Energy and Time Characteristic for Electron Transitions in a Linear Harmonic Oscillator
The calculations are very simple if we note that the oscillator energy in state n is

where 

which is a result independent of n.
On the other hand the time period of the oscillation associated with any state n, viz.

is also independent of n. In effect

The Formula (66) multiplied by (67) gives

which is a result identical to that in (6), (18) and (49). For 

and

so the product of (69) and (70) becomes

The case of 

and the transition time interval is

From (72) and (73) their product becomes

Finally the case of 

for the energy change and

for the transition time, so the product of (75) and (76) is equal to

The results (68), (71), (74) and (77) find their counterparts in the formulae (18) and (49), (26) and (58), (32) and (61), and (40) and (63a), respectively. This kind of parallelism seems to be readily attainable also for
6. Classical Hamilton Equations and the Phase-Space Areas
Ehrenfest [6] [7] has pointed out that the Hamilton equations for a classical particle remain valid also for the motion of the electron wave packets applied in the quantum theory. But from the point of view of the motion description the Hamilton equations seem to be not of an equal importance. The first equation

defines the particle velocity with respect to the dependence of energy on the particle momentum. For 


eliminates the notion of the particle motion at all. In this case Equation (79) provides us only with a condition for minimalization of the particle energy which can be valid also for a static system. A slight dependence of 

Approximately Equation (78) can be transformed into

which gives

The last equation indicates equivalence of two kinds of the phase-space areas examined in the present paper. In case of calculations on a quantum system the right-hand side of (81) may occur to be more easy to access than the left-hand side. An explicit equivalence of the both sides of (81) has been demonstrated in the present paper only in the free-electron case; see Sec. 3. Nevertheless, because of (81) and the fact that E in the hydrogen atom can be represented as a position-independent variable [see (42)], the validity of (81) seems to be justified also in this case.
On the next step, for a linear harmonic oscillator the dependence on x is eliminated in (78) because of a partial derivative

since E is a function having the dependence on 

7. The Check of Calculations: Reference of Energies Belonging to the Various States Considered in the Equations for Electron Transitions
Our aim is to eliminate, in the first step, the dependence of transition equations on the time parameter and find, in the next step, that such transformation gives a correct reference between different energies.
Let us consider, for example, two phase-space areas representing transitions: (i) between two lowest quantum states and (ii) between two states one of which is the lowest, but the higher state is separated from the lowest one by one empty state. In this case we have the equations pair:


because


Equation (83) gives

and Equation (84) gives

where

In the next step

and

The last result substituted to (88) gives the equation

or

We check that Equation (93) is satisfied by the energy intervals belonging to any of the quantum systems examined in the present paper.
For the particle in a one-dimensional potential box we obtain for (93) the relation

This can be transformed into

so

For the hydrogen atom the Formula (93) gives

or

In fact for large n we obtain from (98) the equality

Finally for a linear harmonic oscillator we have


Therefore (93) is transformed into the expression

which completes the proof.
8. Simple Example: The De-Excitation Time of a Free Electron in a One-Dimensional Potential Box
It seems of interest to examine an example of a spontaneous de-excitation time of an electron enclosed in a one-dimensional potential box. Let us assume that the Fermi level of electrons in the box is about 1 eV and the box length is 1 cm, so a kind of a one-dimensional metal is considered. In the first step the quantum number 

for
This is a realistic result because the sample can be considered as built up of the chain of atoms which are separated approximately by a distant of 1 Å 
Let us assume that an excited electron is just one level above


This time seems to be much longer than a typical relaxation time in three- dimensional metals which is of the size of 
9. Partition of the Phase-Space Areas into Contributions Due to the Individual Quanta of Action Equal to the Planck Constant h
We demonstrate in this Section that the quanta of energy and time, as well those of momentum and position (this second kind of quanta is obtained in the case of a particle moving in a one-dimensional potential box), are distributed uniformly in each of the considered phase-spaces with the same constant contribution h given within an individual quantum area. The data are taken from Sections 3-6.
Beginning with the energy-time phase space we obtain:

and

for a particle enclosed in a one-dimensional potential box, giving the product of the final terms in (105) and (106) equal to

In the next step we obtain for the electron moving in the hydrogen atom.


and product of the final terms in (108) and (109) becomes

In the third step we have for the harmonic oscillator

and

which gives a product of the final steps in (111) and (112) equal to

valid for a linear harmonic oscillator.
A similar partition of the phase-space area we obtain for the momentum- position phase space considering the motion in a one-dimensional potential box:


and product of the final terms in (114) and (115) is equal to

10. Degeneracy of the Emission Intensity for Electron Transitions Having Different Dn
It is easy to note that relation (11) can be obtained also as the result of dependencies

and

For, in virtue of (117) and (118), we have the relation

where the last step is equivalent to that derived before; see (6).
Evidently because of (117) and (118) the emission intensity becomes

The last step in (120) is the emission intensity concerning the electron transitions between two neighbouring electron levels.
Therefore, though according to Bohr [28] [29] , the radiation emitted during a transition between two stationary states is unifrequentic and possess the frequency 

the intensity of emission is not unifrequentic but can be degenerated, or almost degenerated, with the intensities of transitions

having different 

11. Summary
Conventionally―since its very beginning―the quantum theory descibed the phenomenon of electron transitions between the quantum levels on a probabilistic footing. In consequence, the size of the time intervals connected with transitions was systematically neglected in the mentioned description.
The aim of the present paper was to make a step towards a change of a such kind of approach. For simple quantum systems taken as examples, we found that in fact the energy interval and time interval taking part in an electron transition can be coupled together into a phase-space area whose size depends on the separation between two quantum levels. The size of the area is expressed by a multiple number of the Planck constant h; see Formula (11).
This very simple complementary description of transitions done with the aid of energy and time does hold on condition the quantum number n characteristic for the levels involved in the transition process is large. Simultaneously, the change Dn of the quantum number denoting the levels taking part in transitions should be small in comparison with n.
For a free electron enclosed in a one-dimensional potential box the phase- space areas of energy and time have their counterparts in the phase-space areas of the particle momentum and position; see Formula (12). For a given quantum transition Dn and large n the sizes of both the energy-time and momentum- position areas become equal; cf. (11) and (12).
A discussion on the sense of the classical Hamilton equations allows us to expect that―at least at some special conditions―a similar equality concerning the areas belonging to two kinds of the phase space should exist also for other quantum systems than that represented by the free-electron case.
Cite this paper
Olszewski, S. (2017) Quanta of the Phase-Space Areas Given by Intervals of Energy and Time Associated with Electron Transitions. Journal of Modern Physics, 8, 1158-1174. https://doi.org/10.4236/jmp.2017.88077
References
- 1. Sommerfeld, A. (1943) Mechanik, Akademische Verlagsgesellschaft, Leipzig.
- 2. Lass, H. (1950) Vector and Tensor Analysis. McGraw-Hill, New York.
- 3. Olszewski, S. (1996) Earth, Moon and Planets, 74, 151-173.
https://doi.org/10.1007/BF00056411 - 4. Olszewski, S. and Kwiatkowski, T. (2012) Journal of Modern Physics, 3, 1142-1151.
https://doi.org/10.4236/jmp.2012.329149 - 5. Ehrenfest, P. (1917) Philosophical Magazine, 33, 500-513.
https://doi.org/10.1080/14786440608635664 - 6. Ehrenfest, P. (1927) Zeitschrift fuer Physik, 45, 455-457.
https://doi.org/10.1007/BF01329203 - 7. Schiff, L.I. (1968) Quantum Mechanics. 3rd Edition, McGraw-Hill, New York.
- 8. Heisenberg, W. (1927) Zeitschrift fuer Physik, 43, 172-198.
https://doi.org/10.1007/BF01397280 - 9. Landau L. and Peierls, R. (1931) Zeitschrift fuer Physik, 69, 56-69.
https://doi.org/10.1007/BF01391513 - 10. Jammer, M. (1974) The Philosophy of Quantum Mechanics. The Interpretations of Quantum Mechanics in Historical Perspective. Wiley, New York.
- 11. Schommers, W. (1989) Space-Time and Quantum Phenomena. In: Schommers, W., Ed., Quantum Theory and Pictures of Reality, Springer, Berlin, 217-277.
https://doi.org/10.1007/978-3-642-95570-9_5 - 12. Bunge, M. (1970) Canadian Journal of Physics, 48, 1410-1411.
https://doi.org/10.1139/p70-172 - 13. Allcock, G.R. (1969) Annals of Physics, 53, 253-285.
- 14. Isaacs, A. (1990) Concise Dictionary of Physics. Oxford University Press, Oxford.
- 15. Weinberg, S. (2013) Lectures on Quantum Mechanics. Cambridge University Press, Cambridge.
- 16. Olszewski, S. (2011) Journal of Modern Physics, 2, 1305-1309.
https://doi.org/10.4236/jmp.2011.211161 - 17. Olszewski, S. (2012) Journal of Modern Physics, 3, 217-220.
https://doi.org/10.4236/jmp.2012.33030 - 18. Olszewski, S. (2012) Quantum Matter, 1, 127-133.
https://doi.org/10.1166/qm.2012.1010 - 19. Olszewski, S. (2016) Reviews in Theoretical Science, 4, 336-352.
https://doi.org/10.1166/rits.2016.1066 - 20. Olszewski, S. (2015) Journal of Modern Physics, 6, 1277-1288.
https://doi.org/10.4236/jmp.2015.69133 - 21. Olszewski, S. (2016) Quantum Matter, 5, 664-669.
https://doi.org/10.1166/qm.2016.1360 - 22. Olszewski, S. (2016) Journal of Modern Physics, 7, 162-174.
https://doi.org/10.4236/jmp.2016.71018 - 23. Olszewski, S. (2016) Journal of Modern Physics, 7, 827-851.
https://doi.org/10.4236/jmp.2016.78076 - 24. Olszewski, S. (2016) Journal of Modern Physics, 7, 1004; (2016) ibid. 7, 2314 (erratum).
- 25. Eyring, H., Walter, J. and Kimball, G.E. (1957) Quantum Chemistry. Wiley, New York.
- 26. Sommerfeld, A. (1931) Atombau und Spektrallinien. Vol. 1, 5th Edition, Vieweg, Braunschweig.
- 27. Mott, N.F. and Jones, H. (1958) The Theory of the Properties of Metals and Alloys. Oxford University Press-Dover Publications, New York.
- 28. Bohr, N. (1918) Memoires de l’Academie Royale des Sciences et des Lettres de Denmark. Section des Sciences, 8eme serie, Tome IV, No. 1, fasc. 1-3.
- 29. Van der Waerden, B.L. (1968) Sources of Quantum Mechanics. Dover Publications, New York.





