Journal of Modern Physics
Vol.05 No.07(2014), Article ID:45452,5 pages
10.4236/jmp.2014.57065
Quantum-Mechanical Information Content of Multiples Hartree-Fock Solutions. The Multi-Reference Hartree-Fock Configuration Interaction Method
Luiz Augusto Carvalho Malbouisson1,2*, Antonio Moreira de Cerqueira Sobrinho1, Micael Dias de Andrade1
1Departamento de Física do Estado Sólido do Instituto de Física da UFBA, Salvador, Brazil
2Departamento de Físico-Química do Instituto de Química da UFRJ, Rio de Janeiro, Brazil
Email: *lmalboui@ufba.br
Copyright © 2014 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 7 February 2014; revised 6 March 2014; accepted 3 April 2014
ABSTRACT
The Hartree-Fock equation is non-linear and has, in principle, multiple solutions. The 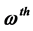 HF ex- treme and its associated virtual spin-orbitals furnish an orthogonal base
HF ex- treme and its associated virtual spin-orbitals furnish an orthogonal base 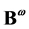 of the full configura- tion interaction space. Although all
of the full configura- tion interaction space. Although all 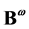 bases generate the same CI space, the corresponding con- figurations of each
bases generate the same CI space, the corresponding con- figurations of each 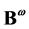 base have distinct quantum-mechanical information contents. In previous works, we have introduced a multi-reference configuration interaction method, based on the mul- tiple extremes of the Hartree-Fock problem. This method was applied to calculate the permanent electrical dipole and quadrupole moments of some small molecules using minimal and double, triple and polarized double-zeta bases. In all cases were possible, using a reduced number of con- figurations, to obtain dipole and quadrupole moments in close agreement with the experimental values and energies without compromising the energy of the state function. These results show the positive effect of the use of the multi-reference Hartree-Fock bases that allowed a better ex- traction of quantum mechanical information from the several
base have distinct quantum-mechanical information contents. In previous works, we have introduced a multi-reference configuration interaction method, based on the mul- tiple extremes of the Hartree-Fock problem. This method was applied to calculate the permanent electrical dipole and quadrupole moments of some small molecules using minimal and double, triple and polarized double-zeta bases. In all cases were possible, using a reduced number of con- figurations, to obtain dipole and quadrupole moments in close agreement with the experimental values and energies without compromising the energy of the state function. These results show the positive effect of the use of the multi-reference Hartree-Fock bases that allowed a better ex- traction of quantum mechanical information from the several 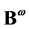 bases. But to extend these ideas for larger systems and atomic bases, it is necessary to develop criteria to build the multi- reference Hartree-Fock bases. In this project, we are beginning a study of the non-uniform distri- bution of quantum-mechanical information content of the
bases. But to extend these ideas for larger systems and atomic bases, it is necessary to develop criteria to build the multi- reference Hartree-Fock bases. In this project, we are beginning a study of the non-uniform distri- bution of quantum-mechanical information content of the 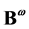 bases, searching identify the fac- tors that allowed obtain the good results cited above.
bases, searching identify the fac- tors that allowed obtain the good results cited above.
Keywords:
Multiple Hartree-Fock Solutions, Quantum Mechanical Information Content, Multi-Reference Hartre-Fock Configuration Interaction Method

1. Introduction
The repulsive coulomb term in the electronic Hamiltonian of atoms, molecules and solids introduces a hard difficult to determine stationary electronic states. It is possible only to obtain approximate solutions of the Schrö- dinger’s equation and there are several procedures for this goal. Two important and general approaches to achieve this purpose are the variational and perturbative methods. In this essay, we are primarily concerned with the approximate variational methods, in particular, with the configuration interaction (CI) methods.
An initial variational approach to study electronic structure of atoms and molecules is the Hartree-Fock (HF) approximation. This approximation can be used as starting-point for other variational and perturbative methods. Although the HF approximation provides the most part of the electronic ground state energy of the system, the rest of this energy is very important to understand several phenomena involving these systems. Rigorously, in practice it is impossible to determine the HF limit as well the exact electronic ground state energy because the atomic bases are finite. Thus, the quantity of correlation energy that is possible to rescue is limited by the atomic base and other truncations or approximations employed. But it is possible for some systems to obtain very accu- rate values. Beside this, an error in the electronic energy means an error in the correspondent state vector with consequences on the calculated values of other dynamical variables, in particular on the non-variational dynam- ical variables.
In molecular electronic problems, there is a variety of observables that depend of the charge distribution and that are not variational dynamical variables as, for instance, the electrical multipolar moments. Non-variational dynamical variables do not exhibit the decrescent monotonical behavior of the energy with the augmentation of the variational class of functions. In contrast, they display an irregular behavior. This means that in the scope of the approximate variational methods better values for electronic energy do not implicate in better values of non- variational observables. This introduces difficulties in the determination of non-variational properties in the ambit of these approximate methods. Over the last twenty years, multi-reference configuration interaction (MRCI) methods have been developed and used in molecular quantum mechanics to study this question [1] . In this communication we present some aspects of the content of quantum information of multiples HF solutions re- lated with this problem using a MRCI method based on multiple HF solutions.
2. Theory
The traditional CI method uses a single reference, the HF ground state, and your excited configurations to ex- pand the state function. However, the HF equation is non-linear and has, in principle, multiple solutions [2] and each HF extremum and its associated virtual spin-orbitals, generate the same full Configuration Interaction (full CI) space. Thus with each HF extremum we can formulate an orthogonal CI problem. It is possible also to for- mulate a non-orthogonal multi-reference CI using these multiple HF references. In the CI problem the variation- al class of functions to search the extrema is a vector space. So the equation of necessary condition to obtain the extrema is linear. It is a usual eigenvalue problem or a generalized eigenvalue problem if the base employed of the vector space is orthogonal or not, respectively. This equation is the matrix representation of the Schrödinger’s stationary equation in this base.
In previous works [3] - [5] we have introduced a new multi-reference configuration interaction method, based on the multiple solutions of the HF problem, called the multi-reference Hartree-Fock configuration interaction (MRHFCI) method. In the MRHFCI method, instead of a single reference one uses several HF extremes as ref- erences in order to expand the state function. The detailed formulation of the MRHFCI method to study a singlet ground state is presented in the references above. The basic aspects of the method can be summarized as follow.
For the restricted HF (RHF) problem of a molecule with  electrons, represented in the atomic base
electrons, represented in the atomic base , the
, the  HF solution is a set of functions
HF solution is a set of functions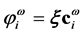 ;
;  where
where 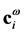 is a column vector with components
is a column vector with components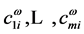 . These functions,
. These functions,  , are the occupied molecular orbitals (MOs). It is po- ssible to generate an additional set of functions
, are the occupied molecular orbitals (MOs). It is po- ssible to generate an additional set of functions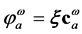 ;
; 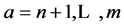 called virtual MOs of the
called virtual MOs of the 



In a molecular HF problem, formulated in any class of symmetry of point and spin, it is possible, in principle, to obtain several HF solutions. And from these solutions to construct several HF references and your corre- spondent excited configuration state functions (CSFs)


sub-bases


















system of generators of the full CI space. From the set 
The motivation for using the MRHF bases lies on the following consideration. Let 


or as,
where,
However,
Thus, in spite of the fact that the bases 



3. Results
3.1. Previous Results
The MRHFCI method was applied to calculate the permanent electrical dipole moment of some small molecules, LiH, BH, FH, CO and H2O and the quadrupole moment of the FH [3] - [5] , using the standard STO-6G and a new minimal-6G bases [6] . For LiH molecule were performed MRHFCI calculations with the double-zeta (dz), triple- zeta (tz) and polarized dz (dzp) bases. In all cases was possible, using a reduced number of CSFs, to obtain di- pole and quadrupole moments in close agreement with the experimental values and energies without compro- mising the energy of the state function. The corresponding full CI calculations do not provide good results for the dipole and quadrupole moments. Two other interesting aspects were observed. It was possible to obtain good dipole and quadrupole values using minimal bases considered as “poor” bases in the literature. These bases are much smaller than the vast majority of the atomic bases used in the calculations of the dipole and quadrupole moments. In the LiH calculations was verified a significant relative reduction of the size of the MRHF base as the dimension of the full CI base increases.
3.2. New Results
In the current stage of development of the MRHFCI method, the results are still obtained through trials but this requires too much computational effort. To extend these calculations for larger systems and atomic bases it is necessary to develop criteria to build the MRHF bases. With this purpose, we begin this project with a systemat- ic study of the non-uniform distribution of QMIC of the 
Table 1. (a) Non-uniform distribution of QMIC of the HF solutions of the LiH molecule; (b) Non-uniform distribution of QMIC of the HF solutions of the LiH molecule.
S, D, T and Q indicate single, double, triple and quadruple excited CSFs; Li–H distance: 3.015 bohr; All the HF solutions are 
(b)
In the last column R indicates HF reference; S, D, T and Q indicate single, double, triple and quadruple excited CSFs; Li-H distance: 3.015 bohr; All the HF solutions are 
Table 2. MRHFCI calculations of the dipole moment for the LiH molecule.
Total energies (electronic + nuclear repulsion) of the HF solutions: A = −7.98066895 hartree; B = −7.42164900 hartree; D = −5.46835770 hartree; L = 3.09788624 hartree; All the HF solutions are 
Table 3. MRHFCI calculation of the energy of the Be atom.
Energies of the HF solutions: A = −14.57125146 hartree; B = −13.78788831 hartree; C = −3.47143646 hartree; All the HF solutions are 1S and were obtained using the new double-zeta base [7] .
Table 3 the first column indicates the number of CSFs employed in the MRHFCI calculation. The second and third columns indicate the HF solutions used and the MRHFCI energy obtained, respectively. The fourth column in the Table 2 presents dipole moment value obtained by the MRHFCI method. The last row in the Table 2 and Table 3 presents the full CI calculation. All the calculations presented in the Table 1(a) and Table 1(b), Table 2 and Table 3 were performed using the new dz base.
4. Conclusions
As observed in Section 2, in spite of the fact that the bases 

Table 2 and Table 3 show the possibility to make good use of this non-uniform distribution of QMIC using the reduced MRHF bases. As presented in Table 2 is possible to obtain the dipole moment of the LiH molecule in close agreement with the experimental value without prejudice to the energy of the state function. The MRHFCI energies differ less than 

A second step in this project, now in progress, is to study the composition of the CSFs of the system of generators 
Acknowledgements
L. A. C. Malbouisson thanks Professor M. A. Chaer Nascimento (Instituto de Química—Universidade Federal do Rio de Janeiro—Brazil) for his hospitality during the written of this work.
References
- (a) Barbosa, A.G.H. and Nascimento, M.A.C. (2002) Generalized Multistructural Method: Theoretical Foundations and Applications. In: Cooper, D.L., Ed., Valence Bond Theory, Elsevier Science BV, Amsterdam. (b) Bundgen, P., Grein, F. and Thakkar, A.J.J. (1995) Molecular Structure Quantum Chemistry, 334, 7. (c) Palmieri, P., Tarroni, R., Mitrushenkov, A.O. and Rettrup, S. (1998) The Journal of Chemical Physics, 109, 7085. http://dx.doi.org/10.1063/1.477391 (d) Ayala, P.Y. and Schlegel, H.B. (1998) The Journal of Chemical Physics, 108, 7560. http://dx.doi.org/10.1063/1.476190
- Malbouisson, L.A.C. and Vianna, J.D.M. (1990) Journal de Chimie Physique et de Physico-Chimie Biologique, 87, 2017.
- Malbouisson, L.A.C., Martins, M.G.R. and Makiuchi, N. (2006) International Journal of Quantum Chemistry, 106, 2772. http://dx.doi.org/10.1002/qua.21035
- Sobrinho, A.M.C., Nascimento, M.A.C., de Andrade, M.D. and Malbouisson, L.A.C. (2008) International Journal of Quantum Chemistry, 108, 2595. http://dx.doi.org/10.1002/qua.21672
- Malbouisson, L.A.C., de Andrade, M.D. and Sobrinho, A.M.C. (2012) International Journal of Quantum Chemistry, 112, 3409. http://dx.doi.org/10.1002/qua.24272
- de Andrade, M.D., Nascimento, M.A.C., Mundim, K.C., Sobrinho, A.M.C. and Malbouisson, L.A.C. (2008) Interna- tional Journal of Quantum Chemistry, 108, 2486. http://dx.doi.org/10.1002/qua.21666
- New Double-Zeta Bases for Li and Be Not Yet Published.
- Multiple HF Solutions for LiH Not Yet Published.
- Chen, M.S., Han, J. and Yu, P.S. (1996) IEEE Transactions on Knowledge and Data Engineering, 8, 866. http://dx.doi.org/10.1109/69.553155
- Witten, I.H. and Frank, E. (2005) Data Mining: Practical Machine Learning Tools and Techniques. 2th Edition, Mor- gan Kaufmann, San Francisco.
NOTES
*Corresponding author.







