Journal of Modern Physics
Vol.3 No.9(2012), Article ID:22613,4 pages DOI:10.4236/jmp.2012.39120
Algebras of Hamieh and Abbas Used in the Dirac Equation*
Department of Mathematics, The University of Texas at San Antonio, San Antonio, USA
Email: gwene@utsa.edu
Received June 16, 2012; revised July 26, 2012; accepted August 3, 2012
Keywords: Dirac Equation; Jordan Algebra; Quadratic Algebra
ABSTRACT
Hamieh and Abbas [1] propose using a 3-dimensional real algebra in a solution of the Dirac equation. We show that this algebra, denoted by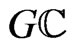 , belongs to a large class of quadratic Jordan algebras with subalgebras isomorphic to the complex numbers and that the spinor matrices associated with the solution of the Dirac equation generate a six-dimensional real noncommutative Jordan algebra.
, belongs to a large class of quadratic Jordan algebras with subalgebras isomorphic to the complex numbers and that the spinor matrices associated with the solution of the Dirac equation generate a six-dimensional real noncommutative Jordan algebra.
1. Introduction
Let 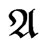 be an algebra over a field F not of characteristic two. The associator is a trilinear mapping
be an algebra over a field F not of characteristic two. The associator is a trilinear mapping
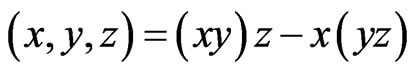
of 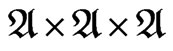 into
into  that measures the lack of associativity in
that measures the lack of associativity in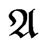 .
.
One scheme of classifying nonassociative algebras involves placing conditions on the associator of certain sets of elements. Some of the better known algebras are:
1) Alternative algebras. In this variety of algebras, all elements x and y satisfy
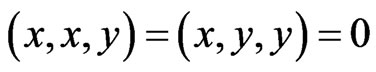
for all elements x and y. The octonion division ring is an alternative algebra. An interesting variation is psuedooctonion algebra (Okubo [5,9]).
2) Jordan algebras. These are commutative algebras in which all x and y satisfy
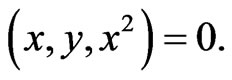
A Type D Jordan algebra is the Jordan algebra of the symmetric bilinear form q on a vector space . Albert [3] has shown that any algebra of Type D has a basis
. Albert [3] has shown that any algebra of Type D has a basis 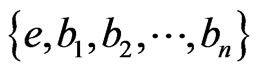 with multiplication given by
with multiplication given by
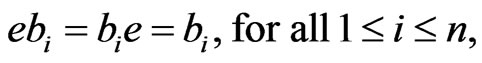
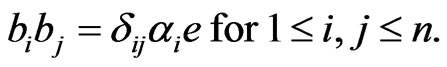
The algebra will be semisimple if 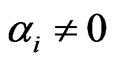 for all
for all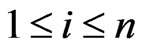 .
.
3) Noncommutative Jordan algebras. A generalization of the alternative and Jordan algebras that requires all x and y satisfy a generalization of the commutative law
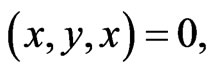
that is, the algebras are flexible, and
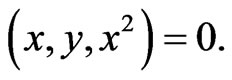
The book by Zhevlakov, Slin’ko, Shestakov and Shirshov [10] provides a detailed analysis of the alternative and Jordan rings.
The above algebras are all power associative since each element a generates an associative subalgebra; equivalently, 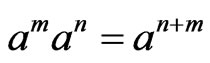 for positive integers
for positive integers . In any power associative algebra
. In any power associative algebra 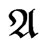 with unit element we can introduce the series
with unit element we can introduce the series
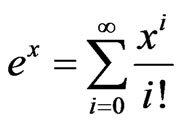
for 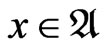 ignoring the question of convergence.
ignoring the question of convergence.
An algebra 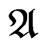 over a field F is called quadratic if, for every x in
over a field F is called quadratic if, for every x in 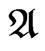

where  are in F and e is the identity of
are in F and e is the identity of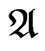 . The quantities
. The quantities 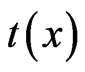 and
and 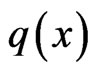 are called the trace and norm of the element x, respectively. The trace is a linear functional on
are called the trace and norm of the element x, respectively. The trace is a linear functional on  see Schafer [7]. The norm
see Schafer [7]. The norm 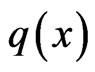 defines a symmetric bilinear form
defines a symmetric bilinear form 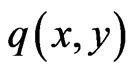 on
on 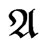 via
via

Say 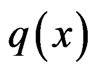 is nondegenerate if
is nondegenerate if 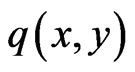 is. Any quadratic algebra is power associative and any flexible, quadratic algebra is a noncommutative Jordan algebra.
is. Any quadratic algebra is power associative and any flexible, quadratic algebra is a noncommutative Jordan algebra.
A quadratic algebra 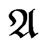 is flexible if and only if the trace is associative; that is,
is flexible if and only if the trace is associative; that is, 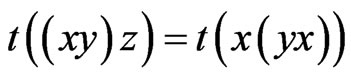 for all
for all 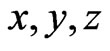 in
in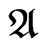 . If
. If  is flexible then the mapping
is flexible then the mapping 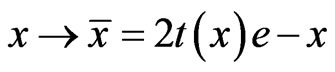 is an involution in
is an involution in  (see Braun and Koecher [11], p. 216).
(see Braun and Koecher [11], p. 216).
Lemma 1. The Hamiltonian division ring is a quadratic algebra.
Proof. Let 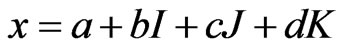 be an element of the Hamitonian division ring. Direct computation shows that
be an element of the Hamitonian division ring. Direct computation shows that
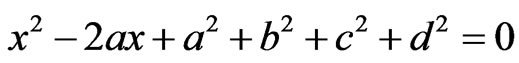 .
.
Example 1. The octonion division ring is a quadratic algebras.
Example 2. Domokos and Kövesi-Domokos [12] propose a quadratic algebra, the “algebra of color” as a candidate for the algebra obeyed by a quantized field describing quarks and leptons (see also Wene [13,14], and Schafer [15]).
2. Construction of the Algebras
The elements of the algebra 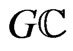 are the elements of the real vector space with basis
are the elements of the real vector space with basis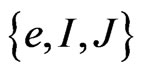 . The addition is the vector space addition and multiplication is defined by
. The addition is the vector space addition and multiplication is defined by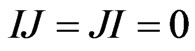 ,
, 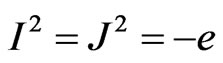 ,
, 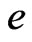 is the identity and the distributive laws. We note that the algebra is commutative and has divisors of zero.
is the identity and the distributive laws. We note that the algebra is commutative and has divisors of zero.
An immediate generalization of this algebra has a basis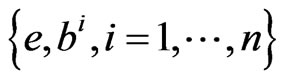 ,
,  over the field
over the field 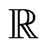 of real numbers and multiplication defined by
of real numbers and multiplication defined by 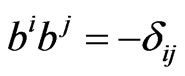 where
where 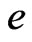 is the identity. For want of a better name called these the Abbas algebras. As noted above, these algebras are Type D Jordan algebras. Note that the
is the identity. For want of a better name called these the Abbas algebras. As noted above, these algebras are Type D Jordan algebras. Note that the  algebra is the construction for
algebra is the construction for ; the results for the Abbas algebras apply to the
; the results for the Abbas algebras apply to the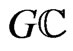 . Each Abbas algebra contains a copy of the complex numbers.
. Each Abbas algebra contains a copy of the complex numbers.
Lemma 2. The Abbas algebras are quadratic algebras.
Proof. Let H denote a Abbas algebra. Then if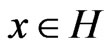 ,
, 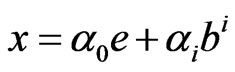 , Einstein summation convention where
, Einstein summation convention where  Then
Then

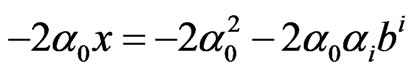
Adding both sides gives
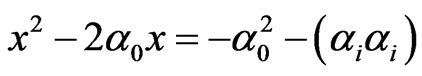
and we see that 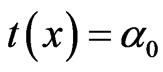 and
and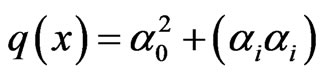 .
.
A commutative quadratic algebra will be a Jordan algebra.
Since the algebra is commutative the trace is associative; the norm is symmetric.
Lemma 3. The norm of a Abbas algebra is nondegenerate.
Proof. Let H denote a Abbas algebra. Then if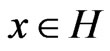 ,
, 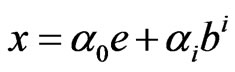 is arbitrary and
is arbitrary and 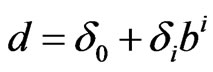 is fixed, then
is fixed, then
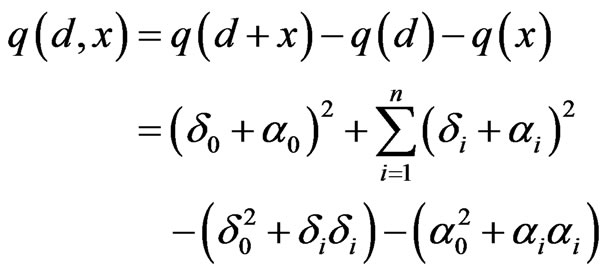
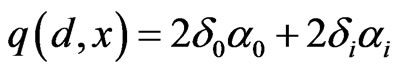
3. Special  Algebras
Algebras
Hamieh and Abbas [1] pass to a representation of the point 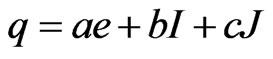 of the algebra
of the algebra  in spherical coordinates,
in spherical coordinates, 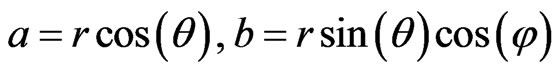 and
and 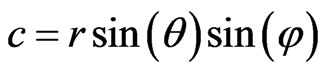 . The subalgebras, called special
. The subalgebras, called special  algebras and denoted by
algebras and denoted by 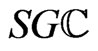 are the subalgebras spanned by all elements in which the “azimutal phase angle
are the subalgebras spanned by all elements in which the “azimutal phase angle 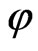 is constant”. Each of these subalgebras is (isomorphic to) the complex numbers.
is constant”. Each of these subalgebras is (isomorphic to) the complex numbers.
Lemma 4. The algebra 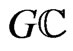 is isomorphic to an algebra of two by two matrices
is isomorphic to an algebra of two by two matrices
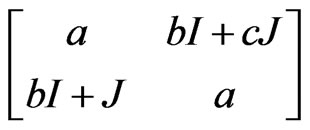
under the usual matrix operation of addition and multiplication.
Proof. The straight forward verification that the mapping 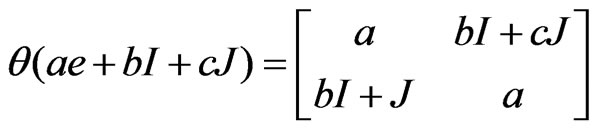 is an isomorphism is left to the reader.
is an isomorphism is left to the reader.
Lemma 5. Each of the algebras  is isomorphic to the complex numbers.
is isomorphic to the complex numbers.
Proof. We note that if 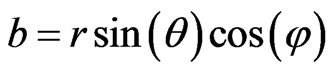 then
then
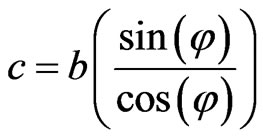 if
if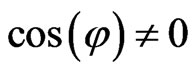 . If
. If 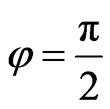 or
or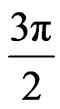 , then
, then 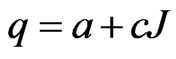 and the subalgebra
and the subalgebra  is (isomorphic to) the complex numbers. Otherwise,
is (isomorphic to) the complex numbers. Otherwise, 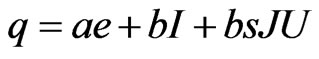 or
or 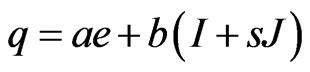 for some
for some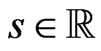 . Let
. Let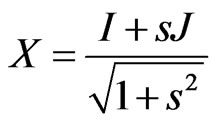 , then
, then
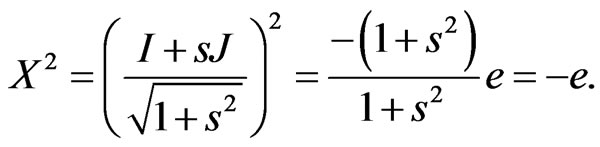
The multiplication, using the basis  will be given by
will be given by

4. The Spinor Matrices
The classical reference on spinors and wave equations is the book by Corson [16].
The associator spinor matrices are
 ,
, 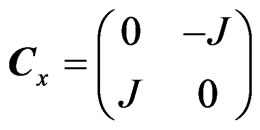 ,
, 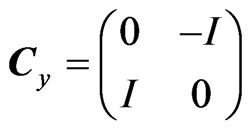 ,
, 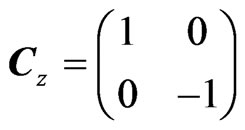
where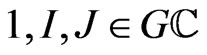 . Denoting the 2 by 2 identity matrix by
. Denoting the 2 by 2 identity matrix by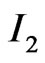 , these matrices satisfy
, these matrices satisfy
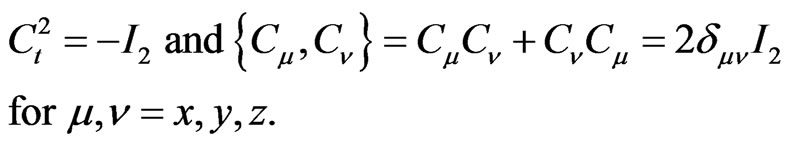
The spinor matrices generate a 6-dimensional real algebra with elements
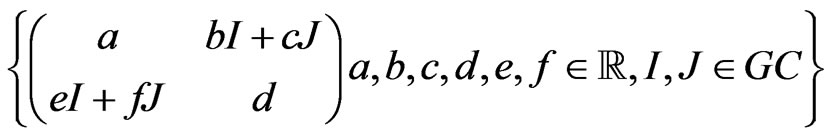
that contains the matrix representation of the 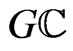 algebra. Denote this algebra by
algebra. Denote this algebra by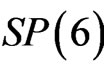 .
.
Lemma 6. The algebra 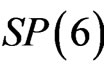 is a quadratic algebra.
is a quadratic algebra.
Proof. If  is an element of
is an element of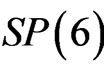 , then
, then
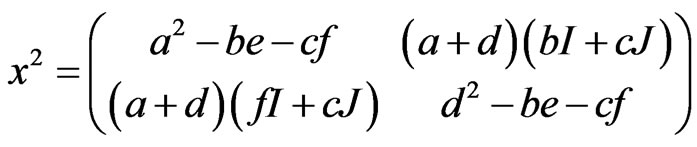
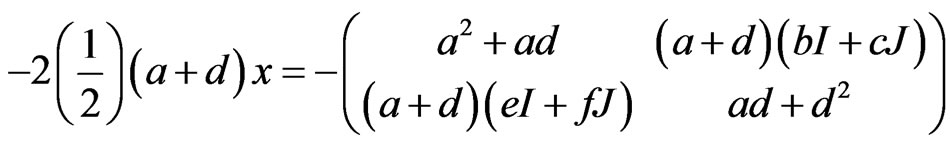
Adding the left and right sides gives
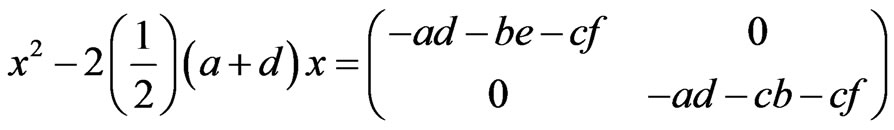
Lemma 7. The algebra 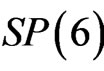 is flexible.
is flexible.
Proof. Because of the trilinearlty of the associator, we can write the elements x and y of the associator 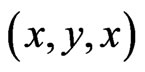
as 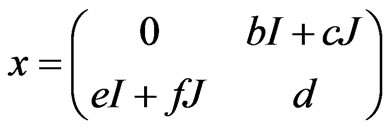 and
and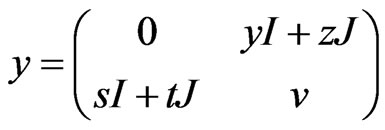 . Then
. Then
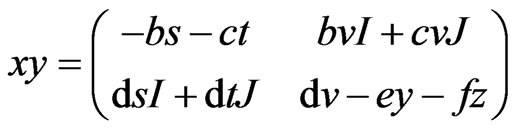
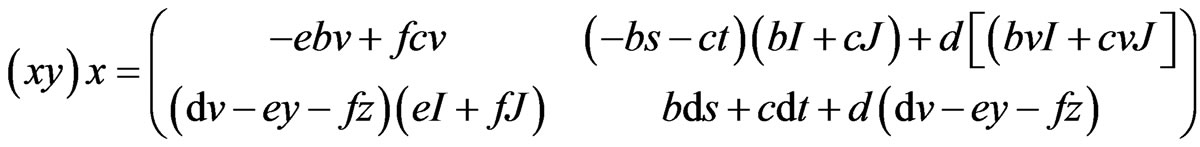
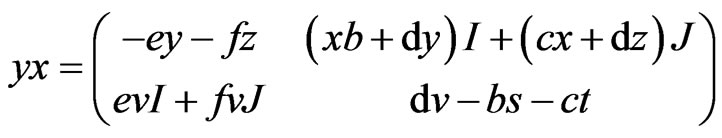
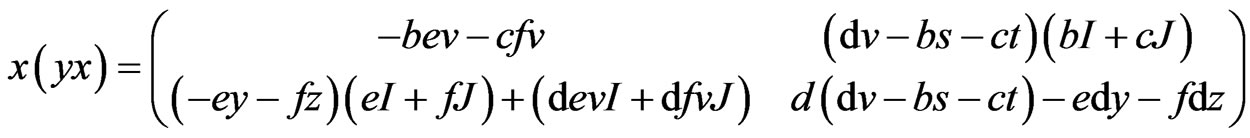
Theorem 1. The algebra  is a quadratic noncommutative Jordan algebra.
is a quadratic noncommutative Jordan algebra.
5. The Dirac Equation
We proceed as in Hamieh and Abbas [1]. The Dirac equation over the complex numbers is often written as
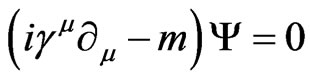
utilizing the Einstein summation convention for 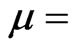
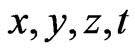 . A more general form is, setting
. A more general form is, setting
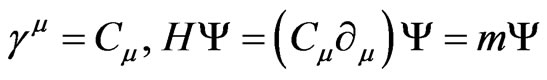
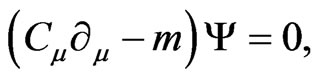
where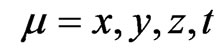 .
.
Upon substituting the matrices for  and simplifying we get
and simplifying we get
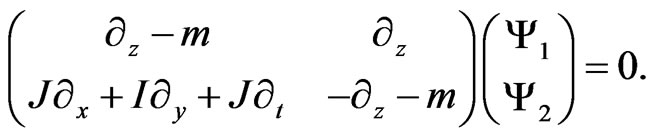
In dimensions x and t, the solution is given by
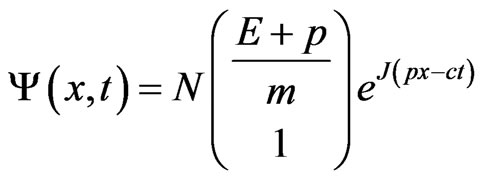
p and 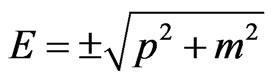 are respect the momentum and energy. N is a normalization factor.
are respect the momentum and energy. N is a normalization factor.
6. Conclusion
We have shown that the 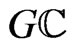 algebra belongs to a large class of Jordan algebras and have examined a few of the algebraic properties of these algebras and, like the Jordan algebra and the algebra of color, there is a very rich mathematical structure to further explore.
algebra belongs to a large class of Jordan algebras and have examined a few of the algebraic properties of these algebras and, like the Jordan algebra and the algebra of color, there is a very rich mathematical structure to further explore.
REFERENCES
- S. Hamieh and H. Abbas, “Two Dimensional Representation of the Dirac Equation in Non-Associative Algebra,” Journal of Modern Physics, Vol. 3, No. 2, 2012, pp. 184- 186. doi:10.4236/jmp.2012.32025
- P. Jordan, J. von Neumann and E. Wigner, “On an Algebraic Generalization of the Quantum Mechanical Formalism,” Annals of Mathematics, Vol. 35, No. 1, 1934, pp. 29-64. doi:10.2307/1968117
- A. A. Albert, “On Jordan Algebras of Linear Transformations,” Transactions of the American Mathematical Society, Vol. 59, No. 3, 1946, pp. 524-555. doi:10.1090/S0002-9947-1946-0016759-3
- J. Lõhmas, E. Paal and L. Sorgsepp, “Nonassociative Algebras in Physics,” Hadronic Press, Palm Harbor, 1994.
- S. Okubo, “Introduction to Octonion and Other NonAssociative Algebras in Physics,” Cambridge University Press, New York, 1995. doi:10.1017/CBO9780511524479
- M. L. Tomber, “A Short History of Nonassociative Algebras,” Hadronic Journal, Vol. 1, 1979, pp. 1252-1387.
- R. D. Schafer, “An Introduction to Nonassociative Algebras,” Academic Press, New York, 1966.
- N. Jacobson, “Structure and Representations of Jordan Algebras,” American Mathematical Society, Providence, 1968.
- S. Okubo, “Pseudo-Quaternion and Pseudo-Octonion Algebras,” Hadronic Journal, Vol. 1, 1978, pp. 1250-1278.
- K. A. Zhevlakov, A. M. Slin’ko, I. P. Shestakov and A. I. Shirshov, “Rings That Are Nearly Associative,” Academic Press, New York, 1982.
- H. Braun and M. Koecher, “Jordan-Algeben,” SpringerVerlag, Berlin, 1966. doi:10.1007/978-3-642-94947-0
- G. Domokos and S. Kövesi-Domokos, “The Algebra of Color,” Journal of Mathematical Physics, Vol. 19, No. 6, 1978, pp. 1477-1481. doi:10.1063/1.523815
- G. P. Wene, “An Example of a Flexible, Jordan-Admissible Algebra of Current Use in Physics,” Hadronic Journal, Vol. 1, 1978, pp. 944-954.
- G. P. Wene, “A Little Color in Abstract Algebra,” The American Mathematical Monthly, Vol. 89, 1982, pp. 417- 419. doi:10.2307/2321659
- R. D. Schafer, “A Generalization of the Algebra of Color,” Journal of Algebra, Vol. 160, No. 1, 1993, pp. 93-129. doi:10.1006/jabr.1993.1180
- E. M. Corson, “Introduction to Tensors, Spinors, and Relativistic Wave-Equations,” Chelsea Publishing Company, New York, 1982.
NOTES
*This paper is in final form and no version of it will be submitted for publication elsewhere.

