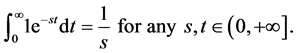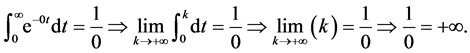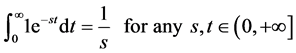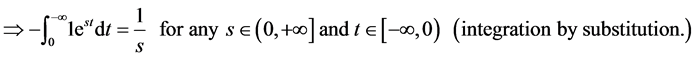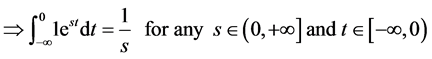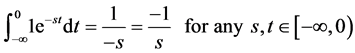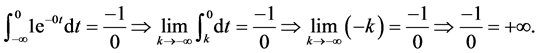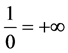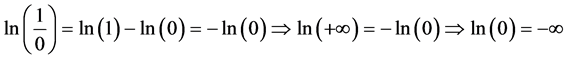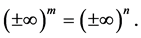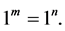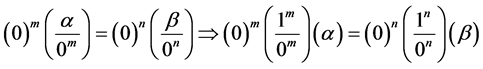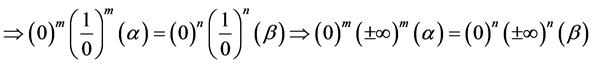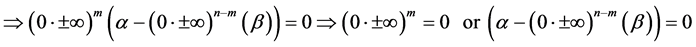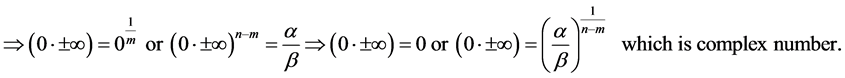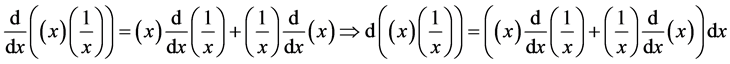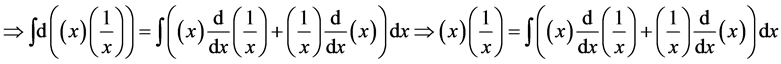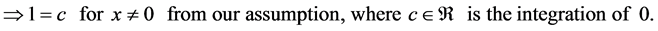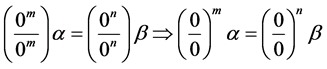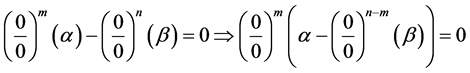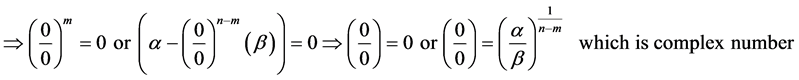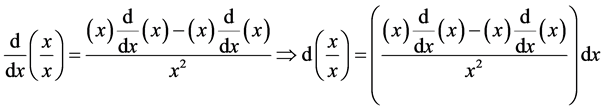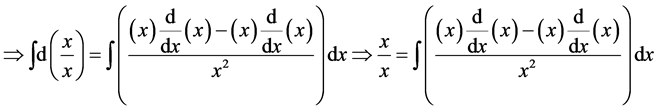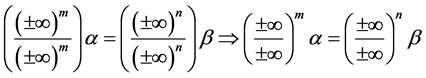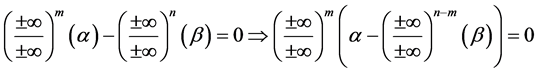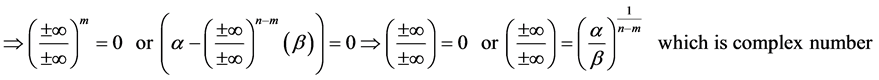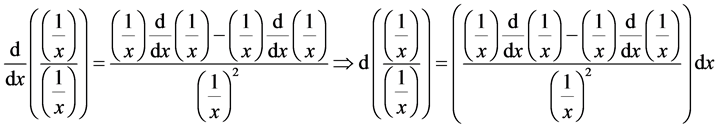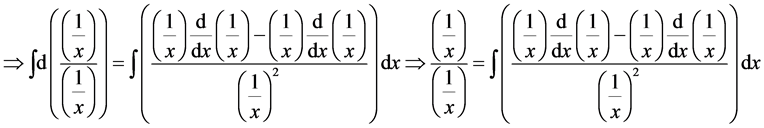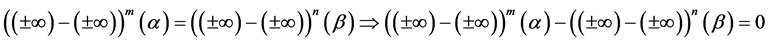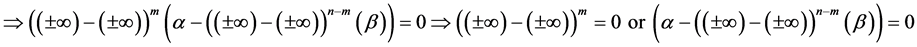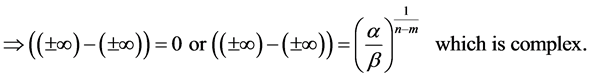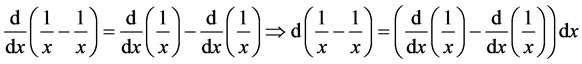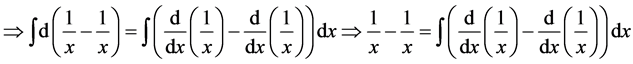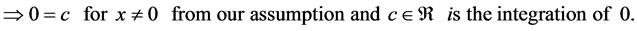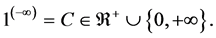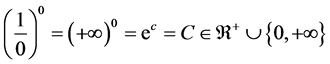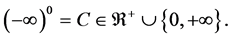Received 4 April 2016; accepted 22 July 2016; published 25 July 2016

ABSTRACT

In this paper, I’m going to discuss on why are, , 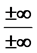, , , , 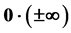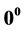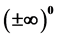and indeterminate form using simple calculus and algebra.

Keywords:

Integration, Differentiation, Simple Algebra

1. Introduction

There are so many things that they are undetermined/indeterminate. Our universe is too complex to model mathe- matically because most of them are undetermined/indeterminate, but we have mathematical model for indeter- minate things itself called indeterminate forms. For example, the weight and height of human beings is indeter- minate because we can’t say the weight or height of human being is this much.

Everything we don’t know is infinitely many times more than everything we know. From something we know we can creat something we don’t know and that gonna be something we know and using this something we know we can creat another something we don’t know and it will continue and never ending. For example, let the only thing we know is hydrogen and oxgen but if we combine two hydrogen and one oxgen we can creat another thing, say water and by combining alot of water together we can creat another thing, say river, lake, ocean etc and also if we add something in to the river or lake or ocean we may creat another living things that we haven’t seen them before and we can continue in such away that creating something we don’t know from something we know.

Someone may ask the main question, “What is the thing before something ?”. In other words, where is our universe comes from? Is our universe comes from something or from nothing? If we assume that our universe is created by God and then some one may ask, “Where God comes from and where he was before he creat the universe?” and Some one may answer for this question like “Before God there is nothing and God comes from nothing”. This may indicates that there is something from nothing [1] - [13] . If there is something from nothing and we know that after something we will have an endless thing, so what is the common thing from nothing and an endless thing? according to our hypothesis it should be something. Therefore if we denote nothing by 0 and

something by c, then 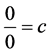since there is something from nothing and if there is something and using algebra, 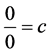implies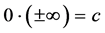, where 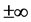is the notation for an endless thing/idea and in mathematics language

which means doesn’t exist. Here we can’t say anything about nothing while nothing exists but invisible. Till

now the first and the only thing we imagine about c is, c might be God or Big-bang. To be something exist there should be nothing exist first. Empty-space doesn’t imply that nothing and vice versa since nothing is not mea- surable and not observable but existance [1] - [13] . In this paper, I am going to discuss on the proof of indeter- minate things in our universe mathematically called indeterminate forms.

In calculus and other branches of mathematical analysis, limits involving algebraic combination of functions in an independent variable may often be evaluated by replacing these functions by their limits; if the expression obtained after this substitution does not give enough information to determine the original limit, it is said to take on an indeterminate form. The term was originally introduced by cauchy’s student Moigno in the middle of the 19th century.

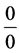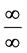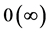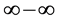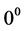
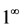
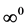
The indeterminate forms typically considered in the literature are denoted, , , , , and [14] [15] .

2. Why Is 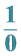Doesn’t Exist?

Definition: The function 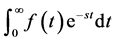is called the Laplace transform of the function. The variable s is, in general, a complex variable.

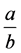
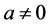
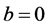
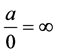
Theorem 1. The number is doesn’t exist for any positive real numbers and, that is,.

Proof. We know that the laplace transform of 1 is equal to for, that is,

But if, the laplace transform of 1 becomes doesn’t exist, that is,

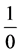
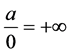
Therefore is doesn’t exist and if we multiply this equation both sides by any non zero positive real numbers a, we get.

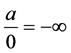
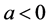
Corollary 1. for.

Proof. 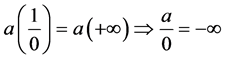for any non zero negative real number a.

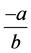
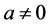
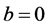
Theorem 2. The number is doesn’t exist for any positive real numbers and, that is,.

Proof. We know that the laplace transform of 1 is equal to for, that is,

Let’s replace s by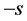, then we get

But if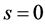, the laplace transform of 1 becomes doesn’t exist, that is,

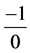
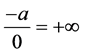
Therefore is doesn’t exist and if we multiply this equation both sides by any non zero positive real numbers a, we get.

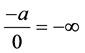
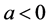
Corollary 2. for.

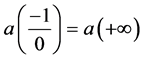
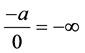
Proof. or for any non zero negative real numbers a.

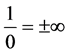
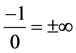
Theorem 3. and.

Proof. Easily sketched from Theorem 1-2 and Corollary 1-2.

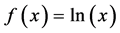
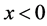
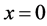
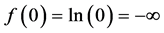
Theorem 4. The function is not defined for and doesn’t exists for, that is,.

Proof. From above Theorem-1 we have,

If we take natural logarithm both sides of this equation we get,

3. Why Is Indeterminate?

Remark: For every natural numbers m and n such that 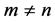we have,

1.

2.

3.

4.

Theorem 5. The number is defined, exists but not unique for, that is, , where.

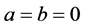
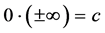
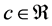
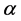
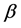
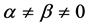
Proof. Let’s take any arbitrary constants and such that.

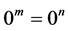
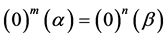
We know that then, if we divide the left and right hand side of this equation by and respectively, then we get

this implies that for every natural numbers m and n

From this we must therfore conclude that 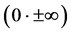is defined, exists but not unique so indeterminate.

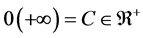
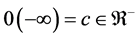
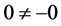
Theorem 6. If and, then.

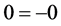
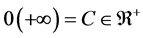
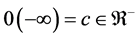
Proof. Suppose, and, then

Therefore.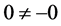

This theorem shows that and or since we know that.

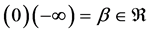
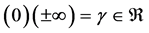
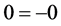
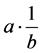
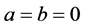
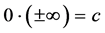
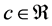
Theorem 7. The number is defined, exists but not unique for, that is, , where.

Proof. Suppose 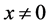is any given real number, then

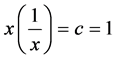
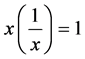
Therefore or for is defined, exists and unique.

Let’s suppose that then our equation becomes where c is the integration of 0 which is any real number and this indicates that is defined, exists but not unique.

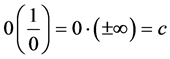
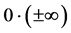
Thus is indeterminate form.

4. Why Is Indeterminate?

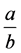
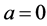
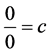
Theorem 8. The number is defined, exists but not unique for and, that is, where.

Proof. Let’s take any arbitrary constants and such that.

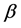
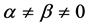
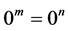
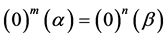
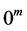
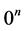
We know that then, if we divide the left and right hand side of this equation by and respectively, then we get

this implies that for every natural numbers m and n,

From this we must therefore conclude that 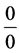is defined, exists but not unique so indeterminate.

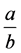
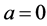
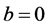
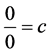
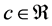
Theorem 9. The number is defined, exists but not unique for and, that is, where.

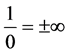
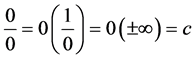
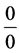
Proof. Since, then this indicates that the number is defined, exists but not unique since c is any arbitrarary constant so indeterminate form.

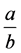
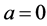
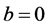
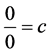
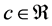
Theorem 10. The number is defined, exists but not unique for and, that is, where.

Proof. Suppose 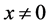is any given real number, then

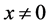
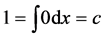
but from our assumption, then we have.

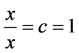
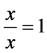
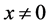
Therefore or for is defined, exists and unique.

Let’s suppose that, then our equation becomes where c is the integration of 0 which is any real number and this indicates that is defined, exists but not unique.

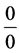
Thus 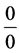is indeterminate form.

5. Why Is Indeterminate?

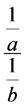
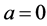
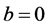
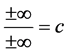
Theorem 11. The number is defined, exists but not unique for and, that is, where.

Proof. Let’s take any arbitrary constants and such that.

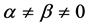
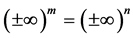
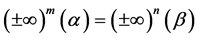
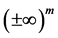
We know that then, if we divide the left and right hand side of this equation by and respectively, then we get

this implies that for every natural numbers m and n such that,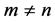

From this we must therefore conclude that 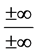is defined, exists but not unique so indeterminate.

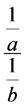
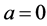
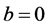
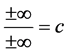
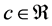
Theorem 12. The number is defined, exists but not unique for and, that is, where.

Proof. Suppose 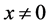is any given real number, then

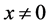
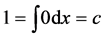
but from our assumption, then we have.

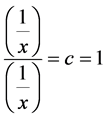
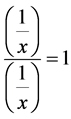
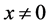
Therefore or for is defined, exists and unique.

Let’s suppose that, then our equation becomes where c is the integration of 0 which is any real number and this indicates that is defined, exists but not unique.

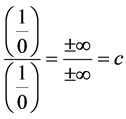
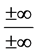
Thus 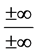is indeterminate form.

6. Why Is 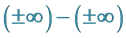Indeterminate?

Theorem 13. The number is defined, exists but not unique for, that is, , where.

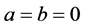
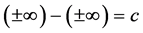
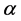
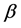
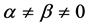
Proof. Let’s take any arbitrary constants and such that.

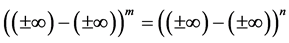
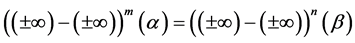
We know that then.

Thus for every natural numbers m and n such that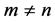, we have

From this we must therefore conclude that 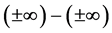is defined, exists but not unique so indeterminate.

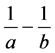
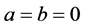
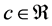
Theorem 14. The number is defined, exists but not unique for, that is, , where.

Proof. Suppose 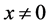is any given real number, then

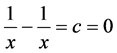
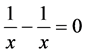
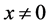
Therefore or for is defined, exists and unique.

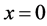
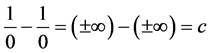
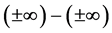
Let’s suppose that then our equation becomes where c is the integration of 0 which is any real number and this indicates that is defined, exists but not unique.

Thus is indeterminate form.

7. Why Is Indeterminate?

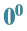
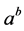
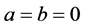
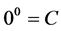
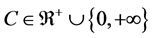
Theorem 15. The number is defined, exists but not unique for, that is, , where.

Proof. We know that 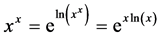for any positive real numbers x.

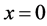
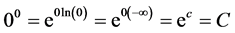
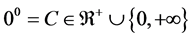
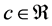
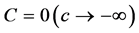
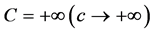
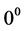
But if, then or for, where and this indicates that the number is defined, exists but not unique so indeterminate form.

8. Why Is 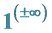Indeterminate?

Theorem 16. The number is defined, exists but not unique for and, that is, , where.

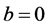
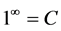
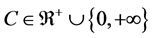
Proof. We know that 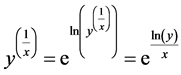for any real numbers x and positive real numbers y.

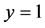
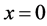
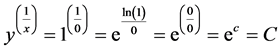
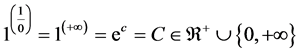
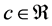
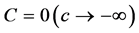
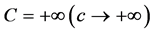
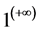
But if and, then or for, where and this indicates that the number is defined, exists but not unique so indeterminate form.

Corollary 3.

Proof. We know that 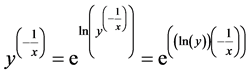for any real numbers x and positive real numbers y.

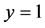
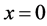
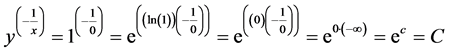
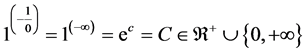
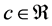
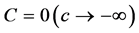
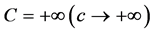
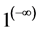
But if and, then or for, where and this indicates that the number is defined, exists but not unique so indeterminate form.

9. Why Is 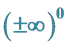Indeterminate?

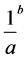
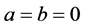
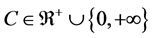
Theorem 17. The number is defined, exists but not unique for, that is, , where.

Proof. We know that 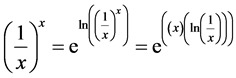for any positive real numbers x.

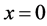
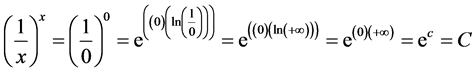
But if, then

or

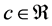
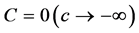
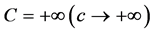
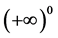
for, where and this indicates that the number is defined, exists but not unique so indeterminate form.

Corollary 4.

Proof. We know that 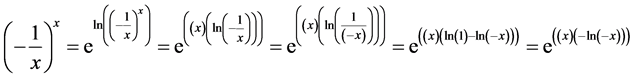for any negative real numbers x.

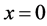
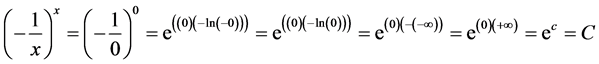
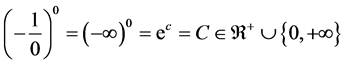
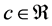
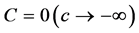
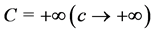
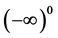
But if, then or for, where and this indicates that the number is defined, exists but not unique so indeterminate form.

Cite this paper

Dagnachew Jenber, (2016) Indeterminate Forms. Advances in Pure Mathematics,06,546-554. doi: 10.4236/apm.2016.68043

References