Advances in Physical Education
Vol.4 No.2(2014), Article ID:45612,7 pages
DOI:10.4236/ape.2014.42011
Research on the Speed-Time (V-T) State Characteristics Curves of 4 × 100 m Relay Baton Transition Period
Daoling Fu, Ming Yang, Jindong Chang*
The College of Physical Education Southwest University, Chongqing, China
Email: *changlai@swu.edu.cn
Copyright © 2014 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 17 January 2014; revised 17 February 2014; accepted 24 February 2014
ABSTRACT
Based on the v-t (speed-time) curves in physics, this study analyzes 4 × 100 m relay baton transition period and describes 4 different relay baton transition period v-t state characteristics curves. We can calculate from the curves that the speed of the first three athletes at the moment of transition is above the 96% of the average speed of 100-meter. The research reveals that the ideal v-t curve is a smooth semi-circular curve (without the launching phase). The stability and continuity during the baton transition period are the key factors in 4 × 100 m relay race.
Keywords:v-t Curve, 4 × 100 m Relay, Transition Period

1. Significance of the Study
During Beijing Olympic Games in 2008, Chinese men’s 4 × 100 m relay team reached the finals for the first time. However, the ranking of them was cancelled for the mistake that happened during the anchor. The crack U.S. team and defending champion England also had some problem in the phase, and for this reason they were all eliminated in the preliminaries (Wang et al., 2009). The cases clearly show that the baton transition period is the key factor in 4 × 100 m relay race (Baeg & Cho, 2005). In the 4 × 100 m relay race, the average speed is higher than the hundred meters because of the saves of launching time. That is the fascination of 1 + 1 + 1 + 1 > 4 in the relay race in which the secret is at the baton transition period (Berthelot et al., 2008). Based on the physics formula of v = v0 + at (Zhang, 1999, 2000; Zhu, 2004) …... the present study employed the v-t curves to show the relationship between speed and time at the different baton transition periods (Du & Lin, 2005).
2. Research Purpose and Methodology
2.1. Research Purpose
The purpose of the study is to analyze 4 × 100 m relay baton transition period through using the v-t (speed-time) curves in physics.
2.2. Methodology
2.2.1. To Search Literature Review
Using the CNKI and to search and categorize the related information which will provide the theoretical evidence for the research.
2.2.2. To Analyze v-t Curves
The v-t curves in physics were employed to analyze 4 × 100 m relay baton transition period, and the researcher advanced 4 different relay baton transition period v-t state characteristics curves.
2.2.3. Comprehensive Analysis
Based on the actual case of Track and Field World Championships, the present study calculated the specific values of the speed in each separated period. Then, the researcher compared and analyzed them. At last, the graphics and reference data were found in the qualitative and quantitative research.
3. Test Results and Discussion
3.1. Simplify the Processing and Description of v-t Curve
Firstly, based on the formula (1) v = v0 + at and to make the v-s curves.
The speed of each team member is represented by v = v0 + at, v0 is for initial velocity, a is for acceleration, it is for time, and v is for terminal velocity that is the final velocity after a period of acceleration time. In a relay race, the entire runner should start from zero speed, and accelerate the process of running to reach the maximum speed which should be kept by the runner till baton transition or sprint finish (Chollet et al., 1996, 1997, 1999).
The baton transition (Messerli, 1952) is the most critical link in relay race. In order to highlight this main contradiction the researcher suppose that the 4 team members’ hundred meters rate and the process of running is almost same. The v-t curves also for the schematic diagram (Tourny et al.,1992), and then the present study exaggerates the baton transition period, and supposes the last three team members’ speed as hundred meter rate (Salo & Bezodis, 2004). As t1, t2, t3 for the baton transition period speed of the 1st, 2nd, and 3rd team members, respectively. tc is for the total time of the full competition (c represents the runner’s score).
In figures 1-4, the v-s curve represents the 4
× 100 m relay under different kinds of baton transition. In the mathematic, the
area under the v-s curve represents distance, and expressed by x. the full game
can be expressed by a formula of
 . The areas under the 4 figures are equal, because the
full distance is 400 m. Integral limit tc represents the total
time of the game which is the more quickly the better. The 4 × 100 m relay is reflected
in the v-t curves. In other words, we pursuit of the smallest tc
under the circumstance that the areas are equal in figures
1-4. It requires that v-t curve is a smooth motion high line (except the
scratch line), in this way we can only guarantee the areas under the curves are
equal, and the time tc is the most short.
. The areas under the 4 figures are equal, because the
full distance is 400 m. Integral limit tc represents the total
time of the game which is the more quickly the better. The 4 × 100 m relay is reflected
in the v-t curves. In other words, we pursuit of the smallest tc
under the circumstance that the areas are equal in figures
1-4. It requires that v-t curve is a smooth motion high line (except the
scratch line), in this way we can only guarantee the areas under the curves are
equal, and the time tc is the most short.
The first runner’s initial velocity is represented by v01, and v01 = 0. the runner is required to have fast response in scratch line, great acceleration, and should reach the vw (speed way) in the short time (see the first part of skew lines in figures 1-4), and then to keep the speed vw till the baton shall be passed within the take-over zone.
The second runner must start from zero speed (this part should be expressed by dotted line for not carrying baton, and it cannot be included in the tc). The second runner should start running before the first runner enter take-over zone (Barbosa et al., 2008). When the speed nearly equals to vw the second runner should take over the baton quickly. We suppose that at the time the baton is passed the second runner’s speed is transfer speed (vT), and vT as his initial speed v02. Obviously, v02 ≠ 0. thus, the second runner’s speed can be expressed as the formula v = v02 + at = vT + at. In the same way, the third and fourth runners’ initial speed all equal to vT, and vT ≈
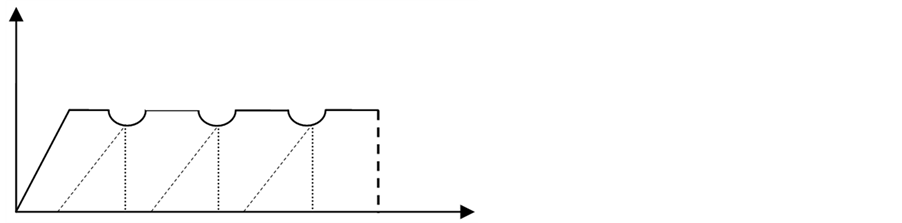
Figure 1.the ideal state of baton transition

Figure 2.the general level team’s baton transition state
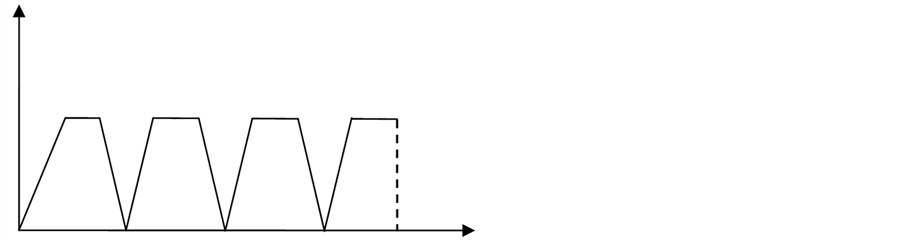
Figure 3. baton transition in a state of rest
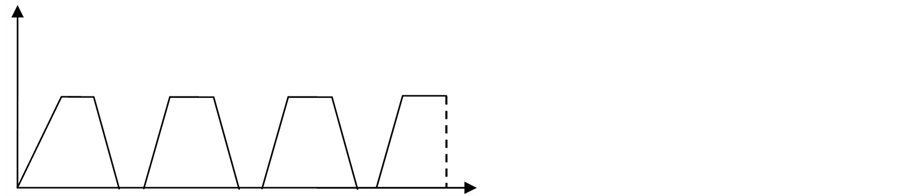
Figure 4.baton transitions in the state of losing the baton
vw. In this way the team can keep the continuity of the baton transition period speed, and the team can keep the speed vw throughout the race. Besides, the transmitter and receiver should keep the same speed in order to maintain stable transmitting in the baton transition period. In other words, when the transmitter and receiver have no relative speed the baton transition is the most ideal.
3.2. Types of v-t Curves under the Different Baton Transition States
figure 1 shows the ideal state of baton transition: as baton transmit, the initial speed should be quick (vT ≈ vw ) and stable, and the speed should be keep vw throughout the race. The curve in the transition period is slight decrease in punctuate, and the transmitter and receiver’s related speed is almost zero. The whole curve should be coherent. When the value of v is high and flat, the time tc is the most short.
figure 2 shows general level team’s baton transition state: in order to maintain the stability of baton transition the team should cost some baton transition period speed. At first, the transmitter should slow down the speed, then the receiver run slowly even though they already start to run. The receiver should look back while waiting the transmitter, and they would receive the baton when the speed vT ≈ vw 50%, than they should speed up to vw. Therefore, it will produce a large displacement in the long baton transition period, and which will influence the total score tc. In this curve, the value v has larger fluctuations which are not like figure 1 in which the value of v is high and flat. Thus, the total time tc is larger than that is in figure 1. However, it has some benefits, such as high stability, low risk in baton transition failure. Thus, this is the common method that average level of team may use As shown in figure 3, in a state of rest, the baton receiver holds still on the line until he receives the baton. This kind of baton transition would greatly slow down the speed even though baton transition is very stable. Because the receiver’s speed should start from zero and then he should accelerate. In this way, total time in the relay race means to add up 4 the hundred meter results. In addition, for transmitter’s slowing speed, the formula would become 1 + 1 + 1 + 1 < 4. Then, it will lose the meaning of relay race, so this method is not acceptable.
Figure 4 is the state of losing the baton but no violation of game rules: when the baton is recovered by the runner it does not produce displacement, even sometimes it may produce negative displacement. Furthermore, the runner should start from zero speed after recover baton, thus, the total time in the relay race will be greatly increased. Generally, if the baton dropped many times, the team will be eliminated (Arellano et al., 1994). So, we should try to avoid this. At the Beijing Olympic Games in 2008, the American team that was the world record keeper in 4 × 100 m relay race was eliminated in the preliminaries, because the third and fourth runner had dropped the baton in the baton transition period (Takagi et al., 1982).
4. Analyses
The present case study based on the Athletics World Championships 4 × 100 m relay race final. The result of this final is very close to the result of 29th 4 × 100 relay race world record in 2008. The following data of the table is from “China Sport Science and Technology” (Arjmandi et al., 2010).
The world top relay race runners’ v-t curves (Shen, 1997) are between figure 1 and figure 2. They use very short time in baton transition so that the initial speed vT is almost near to vw the explanation is shown in tables 1-3. The data in tables 1-3 is from Tokyo World Championships men’s 4 × 100 m relay race final in 1991. In table 1, there are cumulative time of 100 m, 200 m, 300 m, 400 m, the rank and the average speed (vA) of 4 team members; In table 2, there are times of each 100 m and the average speed vw of hundred meter; In table 3, there are the former three runners’ time that spend in take-over zones and the average speed vT in the baton transition period; In table 4, there are data comparisons of various speeds.
As Table 1 shows us, for the calculation of average times tA of each 100 m in 4 × 100 m relay race, the 400 m’s total time t4 is divided by 4. For instance, American team’s average time of each 100 m is tA = 37.50/4 = 9.38 s, and the slowest team’s average time is 9.88 s that is very near to Jamaican athlete Usain Bolt’s 100 m world record 9.69 s at the Beijing Olympic Games in 2008. Thus, each relay race team member’s average rate is better than their own hundred meter rate, and they accomplish 1 + 1 + 1 + 1 > 4 (Kennedy et al., 1990). Then, we can divide 400 m by t4 and get the 4 runners’ average speed vA, for instance, American team’s average speed is vA = 400/37.50 = 10.67 (m/s). Other teams’ average speeds are 10.56, 10.50, 10.41, 10.38, 10.34, 10.12 m/s, respectively.
In Table 2, for calculating the last three runners’ each hundred meter’s average time tA , the last three runners’ hundred meter time is added together and then divided by three, for instance, the American relay race team’s the last three runners’ each hundred meter’s average time is tA = (8.91 + 9.22 + 9.07)/3 = 9.07 s. Then, we can divide 100 m by the average time and get the last three runners’ average speed. Since the last three runners do not have starting in the relay race, the average speed can be regarded as their hundred meter rate Vw. The American team’s last three runners’ average speed is Vw = 100 m/9.07s = 11.03 m/s, and the other teams’ last three runners’ average speed are 10.94, 10.87, 10.71, 10.71, 10.66, 10, respectively.
In table 3, there are the former three runners’ time that spend in take-over zones (each take-over zone shall be 20 m long, and the time that spend in take-over zones are from the transmitter enters the take-over zone
Table 1. Cumulative time of 100 m, 200 m, 300 m, 400 m, the rank, and vA.
Table 3. The former three runners’ duration in take-over zones and the average speed vt in the baton transition period.
|
Country |
1st take-over zone |
2nd take-over zone |
3rd take-over zone |
Total time in the former 3 take-over zone |
Average time △t in each take-over zone |
Average speed VT in baton transition period |
|
America |
1.81 |
1.82 |
2.02 |
5.65 |
1.88 |
10.62 |
|
French |
1.88 |
1.79 |
1.82 |
5.49 |
1.83 |
10.93 |
|
British |
1.91 |
1.83 |
1.85 |
5.59 |
1.86 |
10.73 |
Table 4. Data comparison of various speeds and the ratio of baton transition period speed to speed that is en route (vT/vw).
|
Country |
0 - 100 m |
100 - 200 m |
200 - 300 m |
300 - 400 m |
The last 3 runners’ each 100 m’s average time tA |
The last 3 runners’ each 100 m’s average speed vw m/s |
|||
|
America |
10.30(1) |
8.91(1) |
9.22(2) |
9.07(1) |
9.07(1) |
11.03 |
|
||
|
French |
10.46(3) |
9.04(2) |
9.12(1) |
9.25(3) |
9.14(2) |
10.94 |
|
||
|
British |
10.50(4) |
9.20(4) |
9.25(3) |
9.14(2) |
9.20(3) |
10.87 |
|
||
|
Niger |
10.41(2) |
9.16(3) |
9.46(5) |
9.40(5) |
9.34(4) |
10.71 |
|
||
|
Italy |
10.51(5) |
9.21(5) |
9.31(4) |
9.49(6) |
9.34(4) |
10.71 |
|
||
|
Soviet Union |
10.53(6) |
9.24(6) |
9.53(6) |
9.38(4) |
9.38(6) |
10.66 |
|
||
|
Canada |
10.53(6) |
9.59(7) |
9.67(7) |
9.72(7) |
9.66(7) |
10.35 |
|
||
to the receiver cross out the take-over zone) and the average speed vT in the baton transition period. As seen in table 3 the runners’ duration in the take-over zone are less than 2 s. The average speed in the baton transition period VT = △x/△t. In this way, we can calculate the vT of the teams of America, French, and British, they are 10.62, 10.93, 10.73 m/s, respectively.
Various speeds are listed in table 4 which clearly shows the relationship between speeds in each period. It shows us quantitative data about speeds so that we can easily compare and analyze the different speeds (Ahmad et al., 2013).
Firstly, as shown in table 4, the teams’ each 100 m’s average speed are 10.67, 10.56, and 10.50 m/s, respectively, which are higher than Bolt’s 100 m world record 9.03 m/s (vA = 100 m/9.69s) It proves that we cannot get highest speed in single member’s effort.
Secondly, through calculating the last three runners’ 100 m averages speed in the 4 × 100 m relay race, we can get the exact data of speed vw subtract from the highest speed. And table 4 explains the relationship between vT, vA and vw, that is vT < vA < vw, thus, we can clearly see that vw is the fastest (Arjmandi et al., 2010).
Thirdly, the ratios of baton transition period speed to fastest speed are shown in table 4. The average speed of baton transition period are 10.62, 10.93, and 10.73 (m/s), respectively. The ratio of baton transition period speed to speed that is en route are 96.28%, 99.91%, and 98.71%, respectively (He, 2011).
5. Conclusion
1) The baton transition period is the most important factor in the success of 4 × 100 m relay race.
2) In the moment of baton transition period, the speeds of transmitter and receiver must be the fastest, and their relative speed is very tiny. In other words, when the transmitter and receiver have no relative speed the state of baton transition is the most ideal, as we can see in figure 1.
3) No deceleration during the baton transition period may effectively improve the results of the game, but it demands higher baton transition skills.
Acknowledgements
This research was supported by the Humanities and Social Science Research Youth Foundation of the Ministry of Education in China (Grant No.12YJC880005) and “the Fundamental Research Funds for the Central Universities (Grant No.SWU1309230)” and “the Fundamental Research Funds for the Central Universities (Grant No.SWU1309231)”. Chongqing Higher Education Reform Project (No.120388) and the Humanities and Social Science Research Youth Foundation of the Ministry of Education in China (Grant No.13YJC890014). Corresponding author Chang Jin-dong is a lecturer in the College of Physical Education at Southwest University; e-mail: changlai@swu.edu.cn. Here the authors would like to express heartfelt thanks.
References
- Ahmad, A., Latif, K., Javaidl, N., Khan, Z. A., & Qasim, U. (2013). Density Controlled Divide-and-Rule Scheme for Energy Efficient Routing in Wireless Sensor Networks. In 26th Annual IEEE Canadian Conference on Electrical and Computer Engineering (CCECE) (pp. 1-4), Regina, 5-8 May 2013.
- Arellano, R., Brown, P., Cappaert, J., & Nelson, R. C. (1994). Analysis of 50-, 100-, and 200-m Freestyle Swimmers at the 1992 Olympic Games. Journal of Applied Biomechanics, 10, 189-199.
- Arjmandi, B., Rahnama, N., & Bambaeichi, E. (2010). A Comparison of Bone Mineral Density Values in Professional Female Handball and Futsal Players and Non-Athletes. Medicine and Science in Sports and Exercise, 42, 702-702.
- Baeg, S. B., & Cho, T. H. (2005). Transmission Relay Method for Balanced Energy Depletion in Wireless Sensor Networks Using Fuzzy Logic. In Fuzzy Systems and Knowledge Discovery (pp. 998-1007). Berlin, Heidelberg: Springer. http://dx.doi.org/10.1007/11540007_127
- Barbosa, T. M., Fernandes, R. J., Morouco, P., & Vilas-Boas, J. P. (2008). Predicting the Intra-Cyclic Variation of the Velocity of the Centre of Mass from Segmental Velocities in Butterfly Stroke: A Pilot Study. Journal of Sports Science & Medicine, 7, 201.
- Berthelot, G., Thibault, V., Tafflet, M., Escolano, S., El Helou, N., Jouven, X., Toussaint, J. F., et al. (2008). The Citius End: World Records Progression Announces the Completion of a Brief Ultra-Physiological Quest. PLoS One, 3, e1552. http://dx.doi.org/10.1371/journal.pone.0001552
- Chollet, D., Pelayo, P., Tourny, C., & Sidney, M. (1996). Comparative Analysis of 100 m and 200 m Events in the Four Strokes in Top Level Swimmers. Journal of Human Movement Studies, 31, 25-38.
- Chollet, D., Pelayo, P., Delaplace, C., Tourny, C., & Sidney, M. (1997). Stroking Characteristic Variations in the 100-m Freestyle for Male Swimmers of Differing Skill. Perceptual and Motor Skills, 85, 167-177. http://dx.doi.org/10.2466/pms.1997.85.1.167
- Chollet, D., Tourny-Chollet, C., & Gleizes, F. (1999). Evolution of Co-Ordination in Flat Breaststroke in Relation to Velocity. Biomechanics and Medicine in Swimming, VIII, 29-32.
- Du, X., & Lin, F. (2005). Improving Routing in Sensor Networks with Heterogeneous Sensor Nodes. IEEE 61st Vehicular Technology Conference, 4, 2528-2532.
- He, X. H. (2011). Research the Feasibility to Involve the 4 × 100 m Hurdles Relay into Olympic Game as a Formal Competition Event. Journal of the Qiannan Normal College for Nationalities, 3, 20.
- Kennedy, P.W., Brown, P., Chengalur, S. N., & Nelson, R. C. (1990). Analysis of Male and Female Olympic Swimmers in the 180-Meter Events. International Journal of Sport Biomechanics, 6, 187-197.
- Messerli, D. F. M. (1952). Women’s Participation to the Modern Olympic Game. Report to the International Olympic Committee, Lausanne: International Olympic Committee.
- Salo, A., & Bezodis, I. (2004). Athletics: Which Starting Style Is Faster in Sprint Running Standing or Crouch Start? Sports Biomechanics, 3, 43-54. http://dx.doi.org/10.1080/14763140408522829
- Shen, Q. (1997). Up for Cardiff, Swamp Show Cardiff qing, Treatment ji (Japan), Analyze Excellent Sprinter 4 × 100 m Relay Time. China Sport Science and Technology, 9, 28-32.
- Takagi, T., Yamakoshi, Y., Yamaura, M., Kondow, R., & Matsushima, T. (1982). Development of a New Type Fault Locator Using the One-Terminal Voltage and Current Data. IEEE Transactions on Power Apparatus and Systems, 8, 2892- 2898. http://dx.doi.org/10.1109/TPAS.1982.317615
- Tourny, C., Chollet, D., Micallef, J. P., & Macabies, J. (1992). Comparative Analysis of Studies of Speed Variations within a Breaststroke Cycle. Biomechanics and Medicine in Swimming, VI, 161-166.
- Wang, Y., Qiu, Y., & Cheng, Q. L. (2009). Optimal Starting Time for Each Member in Wheelchair Race 4 × 100 m Relays. Journal of Wuhan Institute of Physical Education, 6, 16.
- Zhang, S. H. (1999). Editor College Physics (First Copies of the Mechanics). Beijing: Tsinghua University Press, 4-27.
- Zhang, S. H. (2000). Editor College Physics (2nd ed.). Beijing: Tsinghua University Press, 7-36.
NOTES

*Corresponding author.


