Vol.3, No.5, 414-418 (2011) Natural Science
http://dx.doi.org/10.4236/ns.2011.35056
Effect of EXAFS on the dosimetric related parameters
Chitralekha Alur, Sharanabasappa Patil, Manjunath Apparao, Basavaraj Kerur*
Department of Physics, Gulbarga University, Gulbarga, India; *Corresponding Author:
Received 4 March 2011; revised 22 March 2011; accepted 27 March 2011.
ABSTRACT
Mass attenuation coefficients have been meas-
ured for rare earth compounds at photon ener-
gies 8.041, 8.907 and 13.347 keV. The measured
values are compared with theoretical calcula-
tions. The agreement between experiment and
theory is more than the experimental error when
the incident photon energy is around the edge
of the element present in the compound and
agreeing with the theoretical values elsewhere.
The difference in agreement between the theo-
retical values and experimental values is attrib-
uted to the EXAFS effects on the mass attenua-
tion coefficient values.
Keywords: Mass Attenuation Coefficient;
Near Absorption Edge; Cross Section;
Resonance Raman Scattering; EXAFS
1. INTRODUCTION
In medical physics, it is important to measure the
amount of radiation delivered by the ionizing radiation,
in composite substances. For photon interactions in
composite substances a single number cannot represent
the atomic number uniquely across the entire energy
region. This number in composite substances is called
the effective atomic number and it varies with energy
and is denoted here by Zeff. On the other hand, the con-
cept of z-dependence of photon attenuation has led to
many applications in radiation studies. For example pre-
cise knowledge of effective atomic numbers is very im-
portant in medical radiation dosimetry and medical im-
aging, where the cross-sectional anatomy is generated by
computer-assisted tomography (CAT) scans [1]. It is a
common practice to verify the validity of calculation
algorithms by comparing the generated doses with the
measured doses in tissue equivalent phantom substances.
Similarly, tissue-equivalent phantoms are specifically
designed to study the image quality and performance of
the CAT scanners. In both instances, a precise knowl-
edge of the effective atomic number and electron density
of the composite substances is necessary in the low en-
ergy region and have proved to be a convenient parame-
ter for interpretation of x-ray attenuation by a complex
medium like a biological tissue and particularly for the
calculation of dose in radiography and radiation do-
simetry etc.
The x-ray mass attenuation coefficient,

 , for any
material are usually estimated from Bragg’s additivity
law or more commonly called mixture rule. Thus


for any chemical compound/material is given by

ii

  ,
where


i

 is the mass attenuation coefficient of the
ith element and

i is the fraction by weight of the ith ele-
ment. For a compound/material with chemical formula
(Z1)a1, (Z2)a2, ···, (Zn)an the weight factor for the ith ele-
ment is given by

 


iii ii
aA aA  ,
where Ai is the atomic weight of the ith element. Hence
an attempt has been in this regard to determine the


of x-rays for the dosimetric materials (sulphides of Mg,
Ca, Mn Fe and Zn elements) and then determined the Zeff
of these materials by LSF method from

ln

 Vs lnZ
graph. These values are compared with the theoretical
values. The so determined value of Zeff has been used in
the expression
eAeffi
NNZ nA


to calculate the electron density.
In the present measurement the validity of Bragg’s
law is studied for rare earths compounds of L edges. The
selection of the compounds was dictated by the incident
photon energy and the by the limitations of our experi-
mental facilities such that thin foils from the compounds.
The former factor determined the region of the atomic
numbers, wherein the absorption edges energy are close
to the incident photon energy, and the latter determined
the selection of particular compounds in this region. We
have used 241Am (VEC) radioactive x-ray source with
weighted average energy of Kα of 8.041 and 13.347 keV
energy respectively and the compounds of rare earths

C. Alur et al. / Natural Science 3 (2011) 414-418 415
having the elements in the region 57 ≤ Z ≤ 70.
2. EXPERIMENTAL ARRANGEMENT
The good-geometry experimental arrangement used
for the determination of the mass attenuation coefficient
is similar to the one described in detail by us earlier [2];
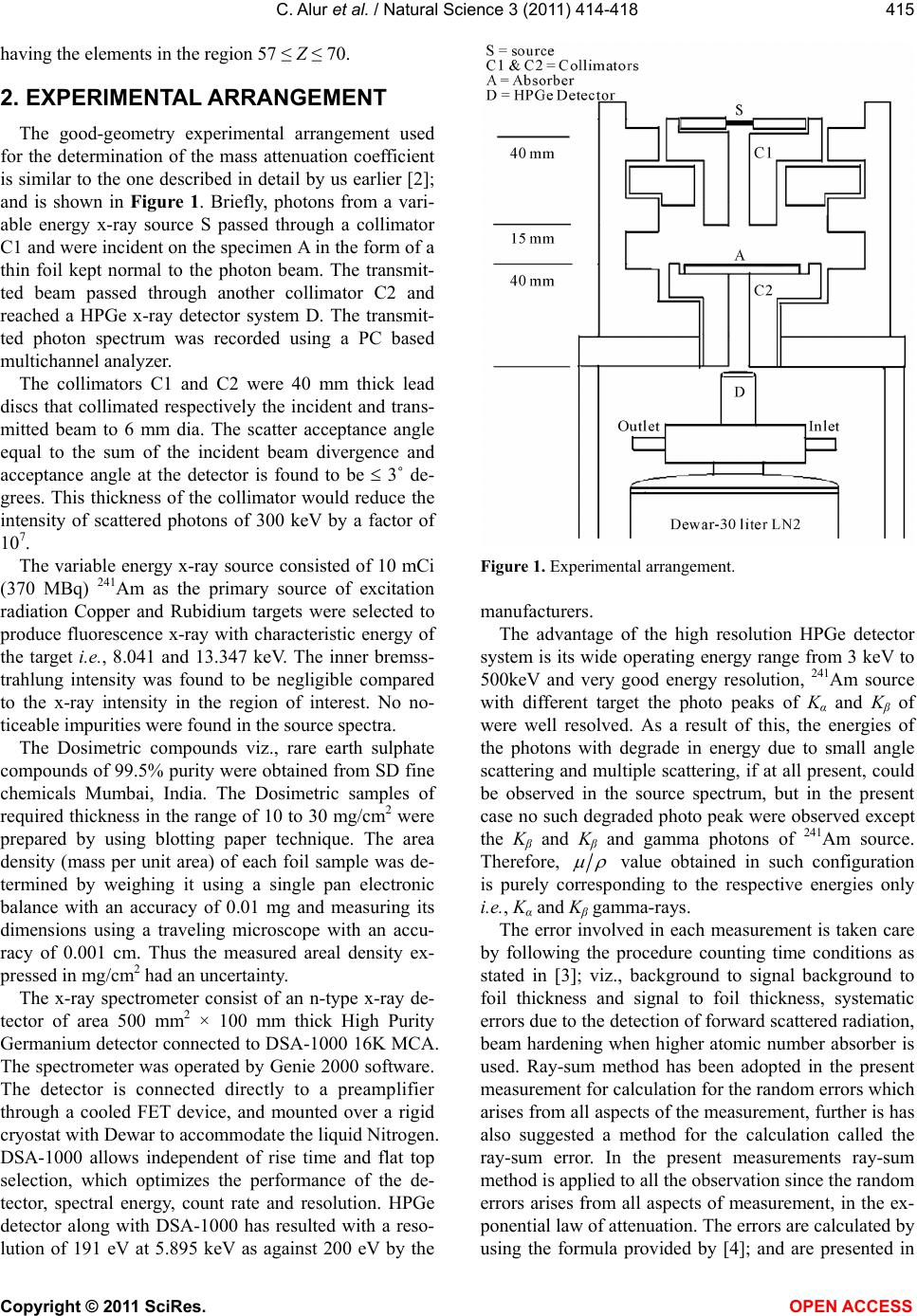
and is shown in Figure 1. Briefly, photons from a vari-
able energy x-ray source S passed through a collimator
C1 and were incident on the specimen A in the form of a
thin foil kept normal to the photon beam. The transmit-
ted beam passed through another collimator C2 and
reached a HPGe x-ray detector system D. The transmit-
ted photon spectrum was recorded using a PC based
multichannel analyzer.
The collimators C1 and C2 were 40 mm thick lead
discs that collimated respectively the incident and trans-
mitted beam to 6 mm dia. The scatter acceptance angle
equal to the sum of the incident beam divergence and
acceptance angle at the detector is found to be  3˚ de-
grees. This thickness of the collimator would reduce the
intensity of scattered photons of 300 keV by a factor of
107.
The variable energy x-ray source consisted of 10 mCi
(370 MBq) 241Am as the primary source of excitation
radiation Copper and Rubidium targets were selected to
produce fluorescence x-ray with characteristic energy of
the target i.e., 8.041 and 13.347 keV. The inner bremss-
trahlung intensity was found to be negligible compared
to the x-ray intensity in the region of interest. No no-
ticeable impurities were found in the source spectra.
The Dosimetric compounds viz., rare earth sulphate
compounds of 99.5% purity were obtained from SD fine
chemicals Mumbai, India. The Dosimetric samples of
required thickness in the range of 10 to 30 mg/cm2 were
prepared by using blotting paper technique. The area
density (mass per unit area) of each foil sample was de-
termined by weighing it using a single pan electronic
balance with an accuracy of 0.01 mg and measuring its
dimensions using a traveling microscope with an accu-
racy of 0.001 cm. Thus the measured areal density ex-
pressed in mg/cm2 had an uncertainty.
The x-ray spectrometer consist of an n-type x-ray de-
tector of area 500 mm2 × 100 mm thick High Purity
Germanium detector connected to DSA-1000 16K MCA.
The spectrometer was operated by Genie 2000 software.
The detector is connected directly to a preamplifier
through a cooled FET device, and mounted over a rigid
cryostat with Dewar to accommodate the liquid Nitrogen.
DSA-1000 allows independent of rise time and flat top
selection, which optimizes the performance of the de-
tector, spectral energy, count rate and resolution. HPGe
detector along with DSA-1000 has resulted with a reso-
lution of 191 eV at 5.895 keV as against 200 eV by the
Figure 1. Experimental arrangement.
manufacturers.
The advantage of the high resolution HPGe detector
system is its wide operating energy range from 3 keV to
500keV and very good energy resolution, 241Am source
with different target the photo peaks of Kα and Kβ of
were well resolved. As a result of this, the energies of
the photons with degrade in energy due to small angle
scattering and multiple scattering, if at all present, could
be observed in the source spectrum, but in the present
case no such degraded photo peak were observed except
the Kβ and Kβ and gamma photons of 241Am source.
Therefore,

 value obtained in such configuration
is purely corresponding to the respective energies only
i.e., Kα and Kβ gamma-rays.
The error involved in each measurement is taken care
by following the procedure counting time conditions as
stated in [3]; viz., background to signal background to
foil thickness and signal to foil thickness, systematic
errors due to the detection of forward scattered radiation,
beam hardening when higher atomic number absorber is
used. Ray-sum method has been adopted in the present
measurement for calculation for the random errors which
arises from all aspects of the measurement, further is has
also suggested a method for the calculation called the
ray-sum error. In the present measurements ray-sum
method is applied to all the observation since the random
errors arises from all aspects of measurement, in the ex-
ponential law of attenuation. The errors are calculated by
using the formula provided by [4]; and are presented in

C. Alur et al. / Natural Science 3 (2011) 414-418 416
the tables.
No dead time corrections are found for photon inten-
sity measurements considered area under photopeak.
However, in the present case we have selected the live
time of the MCA for sources. With these conditions, the
transmitted intensity of x-rays for various combinations
of specimen thickness is recorded and corrected for
background intensity [5,6]. A plot of logarithm of trans-
mission as a function of specimen thickness yielded a
straight line for the entire transmission region, verifying
the validity of the Beer-Lambert’s law. This is confirmed
for different materials also.
3. RESULTS AND DISCUSSION
The plots of the logarithm of transmitted intensity
versus specimen thickness were linear for all the samples
and the

 was obtained from the plots by linear
regression over the 50% - 2% transmission range [7].
The

 obtained for the all dosimetric compounds at
three different photon energies are presented in Table 1.
The theoretical estimated errors are between 1% and
2% taken from the WinXCom. The error involved in
over all experimental values is about 2% to 3% for the
dosimetric samples.
In Table 1 the second column represent L shell Bind-
ing energies EL of the element present in the compound,
third column represents difference, (Ex − EL) between the
incident photon energy Ex (viz., 8.041, 8.907 and 13.347
keV) and L shell binding energy EL. The fourth column
represents experimental

 value, fifth column repre-
sents theoretical

 value (WinXom), and the per-
centage difference (PD) between experiment and theory
is given in the last column of the table. The positive and
negative sign indicates that the interested element L shell
binding energy is above or below the incident photon
energy, and the binding energies are taken from Kor-
tright et al. [8].
Further from Table 1, there is a good agreement be-
tween theoretical and experimental value for all the
compounds at 13.347 keV, it is confirmed fact that when
the incident photon energy is far away from the absorp-
tion edge, there is good agreement between experimental
value with theoretical value within 4%. On the other
hand, the same mixture rule can’t be applicable for
Neodymium and Europium sulphate with Ex-EL ranging
from 0.915 to 0.429. Here, the experimental value devi-
ates from the theoretical value by −7.7% and −16.2%
and next, in case of Europium and Terbium sulphate at
8.907 keV with Ex-EL in range from 1.295 and 0.655 keV
for L edge shows a deviation in the mass attenuation
Table 1. Experimental and theoretical mass attenuation coefficient.
Mass attenuation coefficient (cm2/kg)
Name of the Sample EL binding energy in keVE = Ex − EL (keV)
Expt. value WinXcom
P.D in %
Ex = 8.041 keV
Nd2(SO4)3·8H2O 7.126 0.915
165.3  1.7 179.2 −7.7
Eu2(SO4)3·8H2O 7.612 0.429 150.5  1.5 179.6 −16.2
Tb2(SO4)3·8H2O 8.252 −0.211 152.8  1.6 147.8 +3.3
Yb2(SO4)3·8H2O 9.978 −1.937 80.32  0.9 79.62 +0.8
Ex = 8.907 keV
Nd2(SO4)3·H2O 7.126 1.781 140.2  1.6 137.2 +2.2
Eu2(SO4)3·H2O 7.612 1.295 168.4  1.8 157.8 +6.7
Tb2(SO4)3·H2O 8.252 0.655 193.4  2.0 171.6 +12.7
Yb2(SO4)3·H2O 9.978 −1.071 59.76  0.8 60.97 −1.6
Ex = 13.347 keV
Nd2(SO4)3·H2O 7.126 6.221 47.21  0.5 46.89 +0.8
Eu2(SO4)3·H2O 7.612 5.735 55.43  0.6 54.62 +1.5
Tb2(SO4)3·H2O 8.252 5.095 59.01  0.7 59.84 −1.3
Yb2(SO4)3·H2O 9.978 3.369 75.23  0.6 74.81 +0.5
PD = Percent difference = [(Experimental mean μ/ρ − Computed μ/ρ)/Computed μ/ρ] × 100

C. Alur et al. / Natural Science 3 (2011) 414-418 417
Figure 2. Experimental and Theoretical estimated values of mass attenuation coefficient of rare earth sulphates.
coefficient between the experimental and theoretical by
6.7%, and 12.7% respectively. Thus mixture rule cannot
be applicable for the rare earths compounds where L
edge energy is close to the incident photon energy. This
deviation is also shown in Figure 2 by plotting mass
attenuation coefficient (Experimental and Theoretical)
Vs rare earths compound. The experimental and theo-
retical mass attenuation coefficient value for Neodym-
ium and Europium sulphates at 13.347 shows a good
agreement within the 2% and other hand the same com-
pound at 8.041 and 8.907 keV shows a deviation with
theoretical value. This results purely the mixture rule
can’t be applicable in both the cases otherwise it can be
applicable provided that the edge effects/chemical ef-
fects are taken into account.
In the Table 2 effective atomic number are estimated
using the least squares formula by ploting the mass at-
tenuation coefficient versus the elements for each ener-
gies. From the said graph the estimated value of effec-
tive atomic number of the dosimetric materials found to
vary from 10% to 18% for all the suphides. The theo-
retical values of the Zeff are also calculated using the for-
mula given by Jackson and Hawkes [1] and these values
are discussed in the light of the dosimetry point of view
and as discussed in the introduction. The experimental
Table 2. Determined and estimated values of effective atomic
number and electron density of the rare earths compounds.
Effective Atomic Number (Zeff)
Name of the
Sample Expt Jackson 1981
Electron Density
Ne (1024 elec g–1)
8.041 keV
Nd2(SO4)3·8H2O48.14 41.41 1.649
Eu2(SO4)3·8H2O46.58 43.79 1.562
Tb2(SO4)3·8H2O46.83 45.63 1.542
Yb2(SO4)3·8H2O37.37 49.91 1.185
8.907 keV
Nd2(SO4)3·8H2O45.44 41.41 1.557
Eu2(SO4)3·8H2O48.45 43.79 1.625
Tb2(SO4)3·8H2O50.87 45.63 1.674
Yb2(SO4)3·8H2O37.69 49.91 1.196
13.347 keV
Nd2(SO4)3·8H2O31.02 41.41 1.063
Eu2(SO4)3·8H2O32.82 43.79 1.101
Tb2(SO4)3·8H2O33.55 45.63 1.104
Yb2(SO4)3·8H2O36.53 49.91 1.159

C. Alur et al. / Natural Science 3 (2011) 414-418 418
and theoretical Zeff values are agreeing within 5% except
the edge region. It is important to mention that the theo-
retical/calculated values have not considered the edge
effects and since the effective atomic numbers are un-
der/over estimated when any element falls below the
absorption edge.
In the present work, there is a good indication that
even in the low photon energy region say that up to 15 keV
the effective atomic number can be determined with
greater accuracy but one should take into account of
edge effects.The electron density of the dosimetric mate-
rials is calculated using the experimental Zeff values and
found to vary 0.478 to 0.676 (1024 electrons g–1). In con-
clusion, the determined Zeff value is agreeing with the
theoretical values within 5% at this energy range and
one should also take care of effects if high atomic num-
ber dosimeters are involved.
4. ACKNOWLEDGEMENTS
One of the authors BRK is expressed sincere thanks since this work
is financially support by UGC New Delhi.
REFERENCES
[1] Jackson, D.F and Hawkes, J.D. (1981) X-ray attenuation
coefficients of elements and mixtures. Physics Reports,
70, 169. doi:10.1016/0370-1573(81)90014-4
[2] Nagabhushan, N.M., Kerur, B.R., Lagare, M.T., Nathu-
ram, R., Abani, M.C., Thontadarya, S.R. and Hanumaiah,
B. (2004) Technique for measurement of photon intensity
for the determination of  in the low photon energy re-
gion. Journal of X-Ray Science and Technology, 12,
161-168.
[3] Rose, E.M. and Shapiro, M.M. (1948) Statistical error in
absorption experiments. Physical Review A, 74 , 1853-
1864. doi:10.1103/PhysRev.74.1853
[4] Pearson, J.J. and Osborne, R.R. (1960) Practicle nucle-
onics; a course of experiments in nuclear physics. E &
F.N. Spon, London.
[5] Sharnabasappa, K.B.R., Anilkumar, S. and Hanumaiahm,
B. (2010) Determination of X-ray mass attenuation coef-
ficients using HPGe detector. Applied Radiation and
Isotopes, 68, 76-83.
doi:10.1016/j.apradiso.2009.08.004
[6] Kerur, B.R., Thontadarya, S.R. and Hanumaiah, B. (1993)
Measurement of x-ray mass attenuation coefficients us-
ing mixed radiation emitters. Indian Journal of Physics,
67, 163.
[7] Gerward, L., Guilbert, N., Jensen, K.B. and Levring, H.
(2001) X-ray absorption in matter Reengineering XCOM.
Radiation Physics and Chemistry, 60, 23-24.
doi:10.1016/S0969-806X(00)00324-8
[8] Kortright, J.B. (1986) Characteristic X-ray energies,
X-ray data booklet. In: Vaughan, D. Ed., Lawrence
Berkeley Laboratory, University of California, Berkeley.