 Applied Mathematics, 2011, 2, 521-526 doi:10.4236/am.2011.25068 Published Online May 2011 (http://www.SciRP.org/journal/am) Copyright © 2011 SciRes. AM Surface Wave Propagation in a Generalized Thermoelastic Material with Voids Baljeet Singh1, Raj Pal2 1Department of Mathematics, Post Graduate Government College, Chandigarh, India 2Department of Mathematics, Government Post Graduate College, Hisar, India E-mail: bsinghgc11@gmail.com Received February 21, 2011; revised March 6, 2011; accepted March 10, 2011 Abstract In the present paper, the propagation of surface wave in a generalized thermoelastic solid with voids is con- sidered. The governing equations are solved to obtain the general solution in x-z plane. The appropriate boundary conditions at an interface between two dissimilar half-spaces are satisfied by appropriate particular solutions to obtain the frequency equation of the surface wave in the medium. Some special cases are also discussed. Keywords: Thermoelasticity, Surface Waves, Boundary Conditions, Voids 1. Introduction Theory of linear elastic materials with voids is an impor- tant generalization of the classical theory of elasticity. The theory is used for investigating various types of geo- logical and biological materials for which classical theo- ry of elasticity is not adequate. The theory of linear elas- tic materials with voids deals the materials with a distri- bution of small pores or voids, where the volume of void is included among the kinematics variables. The theory reduces to the classical theory in the limiting case of vo- lume of void tending to zero. Non-linear theory of elastic materials with voids was developed by Nunziato and Cowin [1]. Cowin and Nunziato [2] developed a theory of linear elastic materials with voids to study mathe- matically the mechanical behavior of porous solids. Puri and Cowin [3] studied the behavior of plane waves in a linear elastic material with voids. Iesan [4] developed the linear theory of thermoelastic materials with voids. Dhaliwal and Wang [5] formulated the heat-flux de- pendent thermoelasticity theory for an elastic material with voids. This theory includes the heat-flux among the constitutive variables and assumes an evolution equation for the heat-flux. Ciarletta and Scalia [6] developed a nonlinear theory of non-simple thermoelastic materials with voids. Ciarletta and Scarpetta [7] studied some re- sults on thermoelasticity for dielectric materials with vo- ids. Marin [8-9] studied uniqueness and domain of influ- ence results in thermoelastic bodies with voids. Chirita and Scalia [10] studied the spatial and temporal behavior in linear thermoelasticity of materials with voids. A theo- ry of thermoelastic materials with voids and without en- ergy dissipation is developed by Cicco and Diaco [11]. Ciarletta et al. [12] presented a model for acoustic wave propagation in a porous material which also allows for propagation of a thermal displacement wave. Singh [13] studied the wave propagation in a homogeneous, iso- tropic generalized thermoelastic half space with voids in context of Lord and Shulman theory. Ciarletta et al. [14] studied the linear theory of micropolar thermoelasticity for materials with voids. Recently, Aoudai [15] derived the equations of the linear theory of thermoelastic diffu- sion in porous media based on the concept of volume fraction. Lord Rayleigh [16] investigated the surface wave along the plane surface of an elastic solid. Chandrasekharaiah [17] discussed the effect of voids on Rayleigh waves in an elastic solid with voids and on Rayleigh-lamb waves in homogeneous elastic plate with voids. Many research- ers have studied the surface waves in various theories of thermoelasticity. For example, Chadwick and Windle [18], Agarwal [19], Sharma and Singh [20], Mayer [21], Semerak [22], Chandrasekharaiah [23], Sharma et al. [24], Sharma and Kaur [25] and many others. The present paper is motivated by the linear theory of thermoelasticity with voids developed by Iesan [4]. In Section 2, the governing equations are generalized with the help of Lord and Shulman [26] theory. In Section 3,  B. SINGH ET AL. 522 these equations are solved for general solutions. In Sec- tions 4 and 5, the particular solutions are obtained and applied at required boundary conditions to obtain the fre- quency equation of surface waves in thermoelastic mate- rial with voids. In Section 6, some limiting cases of the problem are discussed. In last section, some concluding remarks are given. 2. Governing Equations Following, Iesan [4] and Lord and Shulman [26], the constitutive equations and field equations in terms of the displacement, volume fraction and temperature, for ho- mogeneous isotropic generalized thermo-elastic material with voids in the absence of the body forces, heat sources and extrinsic equilibrated body forces are 2δ ijij ijkk ee b (1) 0ii qqK ,i (2) ,i hi (3) kk eam (4) kk be m (5) 0ii Tq , (6) ,,,,ijjjij iii uubu (7) 00,0, 00 , kk kk ii cTu mT K u (8) ,,iik k bu m (9) where , are Lame’s constants. 00 is the temperature of the medium in its natural state as- sumed to be such that ,TTT 01, T is the absolute temperatu ij T re, are the components of the stress tensor, ,, 1, 2 ijijj ii euuu are the components of the displa- cement vector, η is the entropy per unit mass, Κ is the coefficient of thermal conductivity, 0 is the thermal relaxation time. ,,b are void material parameters, m is thermo-void coefficient, tt 32 , δ is the coefficient of linear thermal expansion, ij is Kroneck- er deltai q are the components of heat flux vector, i h are the components of equilibrated stress tensor, , is change in volume fraction field, g is the intrinsic equili- brated body force and a is thermal constant. The Equations (7) to (9) are written in x-z plane as 2 22 3 1 2 2 1 2 2 , u uu xz 22 2 33 1 22 2 3 2 2uu u zx zx u b zz t (11) 2 2 3 1 00 22 22 , E u u cT mT txtzt Kxz t (12) 22 3 1 22 2 2, u u b z xz mt (13) where 0 1t . Now the displacement components 1 and 3 are written in terms of potential function u u and as 13 ,uu zzx (14) Using Equation (14) into Equations (10) to (13), we have 22 2 22 2 , zt (15) 22 2 22 2b2 zt , (16) 22 *2 1 22 tt t xz , (17) 22 222 22 222 , bm zxz (18) t where, *00 1 ,, EE Tm K cc T c . Here Equation (15) is uncoupled, whereas Equations (16), (17) and (18) are coupled in , and . 3 1 2 z u b xx t . Solutions o solve the Equations (16) to (18), we consider (10) T ,,,,e, ik xct zzz (19) Substituting (19) in Equations (16)-(18), we obtain Copyright © 2011 SciRes. AM 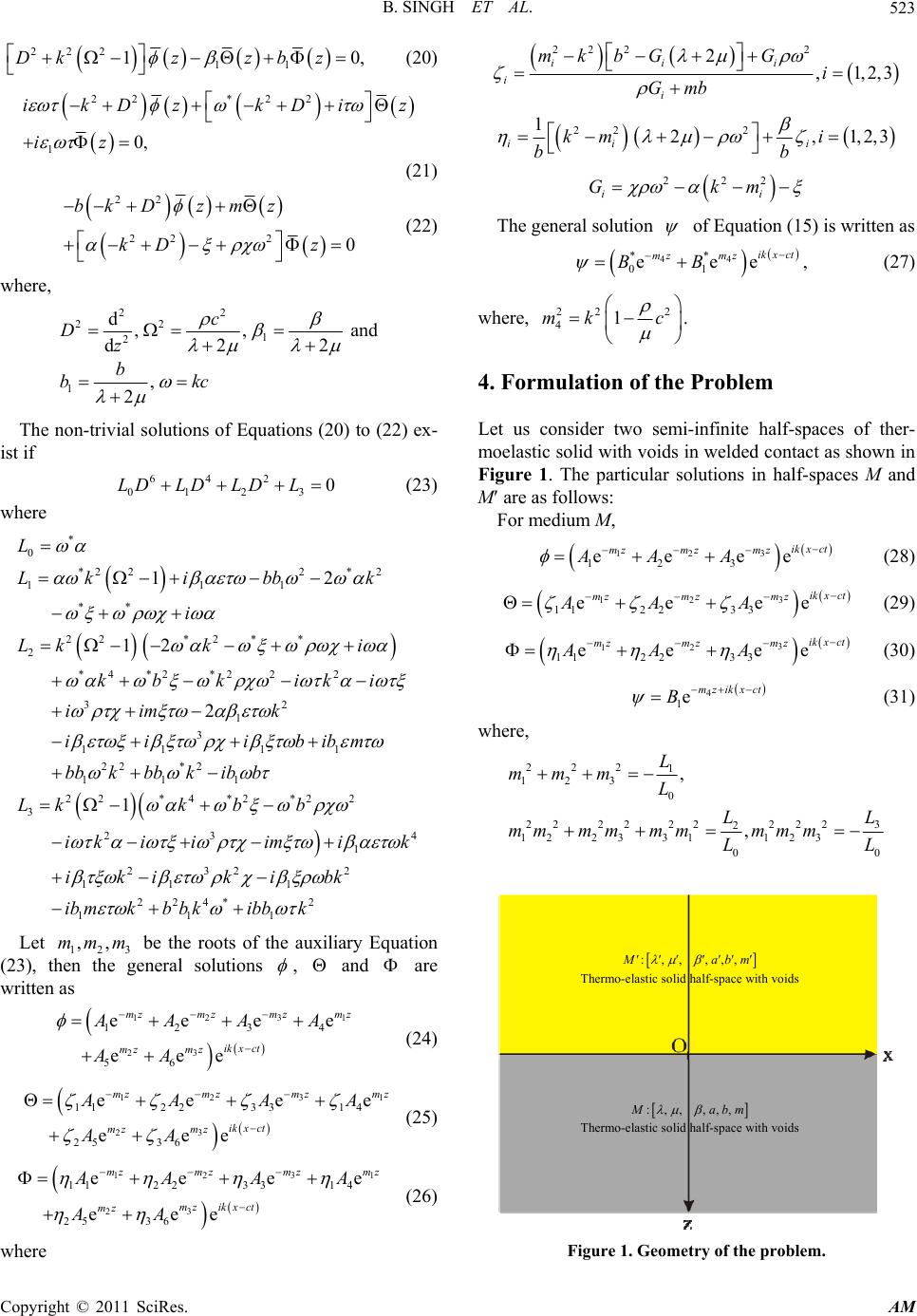 B. SINGH ET AL.523 222 11 10,Dkzzb z (2 0) 22 *22 10, ikDz kDi iz (2 z 1) 22 2220 bkDzm z kD z (22) where, 22 22 1 2 1 d,, 22 d , 2 c Dz b bkc and The non-trivial solutions of Equations (20) to (22) ex- is (23) where t if 642 0123 0LD LD LD L * *222* 2 111 ** 22*2 ** 2 *4 *2*222 32 1 3 11 11 22 *2 111 3 12 12 2 Lki bbk i Lk ki kb kiki iim k ii ibibm bbkbbkib b Lk 0 L 22*4*2*22 23 1 22 2 11 1 224* 2 111 1kb b ik iiimik ikiki bk ib mkbb kibbk 4 Let be the roots of the auxiliary Equation (2 123 ,,mm m hen the g3), teneral solutions , and are written as 3 12 3 2 1234 56 eeee eee mz mzm zmz ik xct mz mz AAA A AA 1 (24) 1 e (25) 1 e (26) where 3 12 3 2 112 23 314 25 36 eee eee mz mzm zmz ik xct mz mz AA AA AA 3 12 3 2 112 23 31 4 25 36 eee eee mz mzmzmz ik xct mz mz AA AA AA 222 2 2,1,2,3 ii i i i mkbGG i Gmb 22 2 12, ii i km i bb 1,2,3 222 ii Gkm The general solution of Equation (15) is written as 44 ** 01 eee ik xct mz mz BB , (27) where, 22 2 41.mkc 4. Formulation of the Problem Let us consider two semi-infinite half-spaces of ther- ic solid with voids welded contact as shown in igure 1. The particular solutions in half-spaces M and moelast in F M are as follows: For medium M, 3 12 12 3 eeee ik xct mz mzm z AA A (28) 3 12 112 23 3 eeee ik xct mz mzm z AA A (29) 3 2 223 3 eee ik xct mz m z A A 1 11 e mz A (30) where, 4 1emz ikx ct B (31) 2221 123 0 2222 222223 123 L m m 2 12 23 31 00 , , L mm mL L mmm mmmm LL :,,,,, ab m Thermo-elastic solid half-space with voids :,,,,, abm Thermo-elastic solid half-space with voids Figure 1. Geometry of the problem. Copyright © 2011 SciRes. AM  B. SINGH ET AL. 524 Similarly, for medium M (32) (33) (34) (35) Here, the symbols with primes in the following sec- tions correspond to medium M. 5. Boundary Conditions The boundary conditions at the interface z = 0 are e continuity placement coperature and volume frac- tional field, i.e. 3 12 12 3 eeee ik xct mz mzm z AA A 3 12 11223 3 eeee ik xct mz mzm z AAA 3 12 112 23 3 eeee ik xct mz mzm z AAA 4 1emz ikx ct B th of force stress components, dis mponents, heat flux, tem 12 3311 ,, ,, ,,, zzzz zxzx zzz z uuuu . where, (36) 33 11 ,, zx z x uu xzx z 0 12 0 2 , , 1, 1 zx b e uu ikc K Kikc The particular solutions (29) to (35) satisf boundary conditions if 2, 2 zzzz kk zzzz kk ee b ee 2, zx zx zx e y the above 11 21 a 12 13 14 1516 17 18 22 23 24 2526 27 28 3132 3334 3536 37 38 4142 4344 4546 47 48 5152 5354 5556 57 58 6162 6364 6566 67 68 7172 7374 7576 77 78 818283 84 8586 87 88 0 aaaaaaaa aaaaaaa aaa aaaaa aaaaaaaa aaa aaaaa aaaaaaaa aaaaaaaa aaaaaaaa (37) where 22 1111 1 2akm b 22 12 22 2 2 ak m b 2 33 3 14 4 22 1511 1 22 1622 2 22 1733 3 184 211 222233 22 244 25 , 2, 2, 2, 2, 2, 2, 2, 2, , m b aikm akm b akm b akmb aikmai km aikmaikm akma 126 2 22 3 284 311 13222333334 351113621237313 38 411 14222433344 45112462224733 2, 2 2, . ,,,0, ,,, 0. ,,,0, ,, ikma ikm ikma km amam ama am amam a amamama am amam 2 48 511 52253354 55156257358 61626364 4 65666768 4 711 72273374 751 762773 78 811 82 , 0. ,,,, ,,,. ,,, , ,,,. ,,,0, ,,,0. , a amamama ik amamamaik aikaikaikam aikaikaikam aa aa aa a a aa 283 384 851 86287388 ,,0, ,,,0. aa aa aa 2 13 2ak 27 a , The Equation (37) gives the frequency equation for surface wave in a generalized thermo-elastic medium with voids. 6. Limiting Cases 1) If we neglect the void parameters, then the Equation (37) reduces to 111213 14 1516 2122 2324 2526 3132 3334 35 36 4142 4344 4546 5152 5354 55 56 6162 6364 6566 aaaaaa aaaaaa aaaaaa aaaaaa aaaaaa aaaaaa (3) w 8 here, 22 11 ak m 1 1 22 22 134 2 141 1 2, 2,2 , m aikm m 12 2 2 ak ak , Copyright © 2011 SciRes. AM  B. SINGH ET AL.525 , , The Equation (38) gives the frequency equation for surface waves in a generalized thermo-elastic medium. 2) If we neglect thermal parameters, then the Equation (37) reduces to 22 222 234 22 241 252 264 311 1322233 3411 13512236 411 4 2,2 2,2 , ,,0, ,,0, , akma ikm aimkaimka km amama amama ama 2243 44145246 515253 4, 545556 4, 611 622 63 641 65266 ,, ,,. ,, ,, ,,0, ,,0. ma ik amamaik aikaikam ikaikam aa a aaa 15 22 164 22 21 1 2, 2 ,,aimkaimkakm a 1112 1314 15 16 2122 2324 25 26 3132 3334 35 36 4142 4344 45 46 5152 5354 55 56 6162 6364 65 66 0 bbbbbb bbbbbb bbbbbb bbbbbb bbbbbb bbbbbb (39) where , , . The Equation (39) gives the frequency equation for surface wave in an elastic medium with voids. 3) If we neglect void and thermal parameters, then the Equation (37) reduces to 22 111 1 22 1233 134 22 223 234 22 241 253 264 2, 2,2 , 2, 2, 2, bk mb bk mbbikm kbimkbkm bimkbimkbkm b 311 1323333 3421 13523336 411 42343 44145346 5253 4, 545556 4 611 623 63 641 653 66 ,,0, ,,0. ,, , ,,. ,, ,, ,,0, ,,0. mbm b bmbmb bmbmbik bmbmbik bikbikbm bikbikbm bb b bbb 14 1 1 22 2, 2, 2, bk mb bkmbb ikm 15 33164 22 21 1 2bi m 51 11 12 13 14 21 22 23 24 31 32 33 34 41 42 43 44 0 cccc cccc cccc cccc (40) where 22 111 124 22 131 144 22 211 224 22 231 244 311 32 33134 4142 4 43444 2, 2 2,2 2,, 2, . ,, , ,, ,. ck mcikm ck mcikm cimkckm cimkckm cmc ik cmcik cikcm cikcm , , The Equation (40) gives the frequency equation for surface wave in an elastic medium 4) If we remove the upper half-space, then the Equa- tion (37) reduces to 11 121314 21 222324 31 323334 41 424344 0 dddd dddd dddd dddd (41) where 22 1111 1 22 12222 22 1333 3 144 21122 22 233 244 311 13222333334 411 14222433344 2, 2, 2, 22,2 2,. ,,, ,,, dkm b dkm b dkm b di kmdi kmdi km dikmd km dmdmdmd dmdmdmd . 2, 0. 0 The Equation (41) gives the frequency equation of a Ra The frequency equation of surface waves in generalized thermoelastic material with voids is ob quency equation of Rayleigh surface wav limiting case. The theoretical results indicate that the speed of surface wave depends on various material pa- yleigh surface wave in a half-space of a generalized thermo-elastic material with void. 7. Conclusions tained. The fre- e is obtained as Copyright © 2011 SciRes. AM  B. SINGH ET AL. Copyright © 2011 SciRes. AM 526 s. Present analytical solutions can be used to numerically the speed of surface wave for a particular material modeled as thermoelastic material with voids. 8. References [1] J. W. Nunziato and S. C. Cowin, “A Nonlinear Theor Elastic Materials with Voids,” Archive for Rational chanics and Analysis, Vol. 72, No. 2, 1979, pp. 175-201. [2] S. C. Cowin and J. W. Nunziato, “Linear Elastic Materi- als with Voids,” Journal of Elasticity, Vol. 13, No. 2 1983, 125-147. doi:10.1007/BF00041230 [3] oelastic Materials echanica, Vol. 60, No. 1-2, 1986, pp. 67- r- nal of Applied Mathematics and Mechanics, Vol. 75, No. 7-714. ] M. Marin, “A Uniqueness Result for Body with Voids in and Temporal in Elastic Materials ation in a Generalized Thermoe- s with Voids,” European Zeitschrift für Angewandte Mathe- gs of the London ol. 25, No. aries,” ty of America, Vol. 280, Surface tion of Surface of Thermal Relaxation on h, “Thermoelastic Rayleigh Waves d 9 99-309. rameterfind [14] M. Ciarletta, M. Svanadze and L. Buonanno, “Plane Waves and Vibrations in the Theory of Micropolar Thermoelasticity for Material y M of e- matik und Physik, Vol. 61, No. 2, 2010, pp. 357-379. , Mat P. Puri and S. C. Cowin, “Plane Waves in Linear Elastic Materials with Voids,” Journal of Elasticity, Vol. 15, No. 2, 1985, pp. 167-183. doi:10.1007/BF00041991 ] D. Iesan, “A Theory of Therm[4 with 2, 1987, pp. 205-211. [18] P. Chadwick and D. W. Windle, “Propagation of Ray- leigh Waves along Isothermal and Insulated Bound Proceedings of the Royal Socie Voids,” Acta M 89. doi:10.1007/BF01302942 [5] R. S. Dhaliwal and J. Wang, “A Heat-Flux Dependent Theory of Thermoelasticity with Voids,” Acta Mechanica, Vol. 110, No. 1-4, 1993, pp. 33-39. [6] M. Ciarletta and A. Scalia, “On the Nonlinear Theory of Nonsimple Thermoelastic Materials with Voids,” Journal of Applied Mathematics and Mechanics, Vol. 73, No. 2, 1993, pp. 67-75. [7] M. Ciarletta and E. Scarpetta, “Some Results on Ther- Moelasticity for Dielectric Materials with Voids,” Jou Wave 9, 1995, pp. 70 [8 Linear Thermoelasticity,” Rendiconti di Matematica, Vol. 17, No. 1, 1997, pp. 103-113. [9] M. Marin, “On the Domain of Influence in Thermoelas- ticity of Bodies with Voids,” Archiv der Mathematik, Vol. 33, No. 4, 1997, pp. 301-308. [10] S. Chirita and A. Scalia, “On the Spatial Rayl Behavior in Linear Thermoelasticity of Materials with Voids,” Journal of Thermal Stresses, Vol. 24, No. 5, 2001, pp. 433-455. [11] S. D. Cicco and M. Diaco, “A Theory of Thermoelastic Materials with Voids without Energy Dissipation,” Jour- nal of Thermal Stresses, Vol. 25, No. 5, 2002, pp. 493- 503. doi:10.1080/01495730252890203 [12] M. Ciarletta, B. Straughan and V. Zampoli, “Thermo- poroacoustic Acceleration Waves with Voids without Energy Dissipation,” International Journal of Engineering Science, Vol. 45, No. 9, 2007, pp. 736-743. doi:10.1016/j.ijengsci.2007.05.001 [13] B. Singh, “Wave Propag lastic Material with Voids,” Applied Mathematics and Computation, Vol. 189, No. 1, 2007, pp. 698-709. doi:10.1016/j.amc.2006.11.123 Journal of Mechanics-A-Solids, Vol. 28, No. 4, 2009, pp. 897-903. doi:10.1016/j.euromechsol.2009.03.008 [15] M. Aoudai, “A Theory of Thermoelastic Diffusion Ma- terial with Voids,” doi:10.1007/s00033-009-0016-0 [16] L. Rayleigh, “On Waves Propagating along the Plane Surface of an Elastic Solid,” Proceedin hematical Society, Vol. 17, No. 1, 1885, pp. 4-11. doi:10.1112/plms/s1-17.1.4 [17] D. S. Chandrasekharaiah, “Effects of Surface Stresses and Voids on Rayleigh Waves in an Elastic Solid,” In- ternational Journal of Engineering Science, V No. 1380, 1964, pp. 47-71. doi:10.1098/rspa.1964.0130 [19] V. K. Agarwal, “On Surface Waves in Generalized Thermoelasticity,” Journal of Elasticity, Vol. 8, No. 2, 1978, pp. 171-177. doi:10.1007/BF00052480 [20] J. N. Sharma and H. Singh, “Thermoelastic s in a Transversely Isotropic Half-Space with Thermal Relaxation,” Indian Journal of Pure and Applied Mathematics, Vol. 16, No. 10, 1985, pp. 1202-1219. [21] A. P. Mayer, “Thermoelastic Attenua Acoustic Waves,” International Journal of Engineering Science, Vol. 28, No. 10, 1990, pp. 1073-1082. doi:10.1016/0020-7225(90)90135-6 [22] F. V. Semerak, “The Effect eigh Surface Waves in a Thermoelastic Medium,” Journal of Mathematical Sciences, Vol. 88, No. 3, 1997, pp. 396-399. [23] D. S. Chandrasekharaia without Energy Dissipation,” Mechanics Research Com- munication, Vol. 24, No. 1, 1997, pp. 93-102. doi:10.1016/S0093-6413(96)00083-3 [24] J. N. Sharma, D. Singh and R. Kumar, “Generalize Thermoelastic Waves in Homogeneous Isotropic Plates,” Journal of the Acoustical Society of America, Vol. 108, No. 2, 2000, pp. 848-851. doi:10.1121/1.42961 [25] J. N. Sharma and D. Kaur, “Rayleigh Waves in Rotating Thermoelastic Solids with Voids” International Journal of Applied Mathematics and Mechanics, Vol. 6, No. 3, 2010, pp. 43-61. [26] H. W. Lord and Y. Shulman, “A Generalized Dynamical Theory of Thermoelasticity,” Journal of the Mechanics and Physics of Solids, Vol. 15, No. 5, 1967, pp. 2 doi:10.1016/0022-5096(67)90024-5
|