Materials Sciences and Applications, 2011, 2, 411-415
doi:10.4236/msa.2011.25053 Published Online May 2011 (http://www.SciRP.org/journal/msa)
411
Structural Properties of Synthetic Na-Hectorite
Exchanged with Heavy Metals
Karmous Mohamed Salah
Département de physique, Faculté des Sciences de Sfax Route de la Soukra km 4, Sfax, Tunisie.
Received March 1st, 2011; revised March 16th, 2011; accepted March 30th, 2011.
ABSTRACT
The main objective of this study is to determine the structural characteristics of synthetic Na-Hectorite (H-Na) ex-
changed with heavy metals: Ni2+, Pb2+, Zn2+, Cd2+, Co2+ and Mg2+ using quantitative analysis based on the comparison
between the theoretical and experimental XRD patterns. The different complexes are not homogenous. The hectorite
saturated by the lead and cadmium present a segregation distribution of the layers, where as the others complexes pre-
sent a random distribution.
Keywords: Hectorite, XRD, Heavy Metals, Simulation
1. Introduction
Heavy metal pollution occurs in many industrial wastewa-
ter such as those produced by metal-plating, finishing fa-
cilities, dyeing operations, mining and metallurgical engi-
neering, electroplating, nuclear power plants, aerospace
industries, battery manufacturing processes and glass pro-
duction etc. The presence of heavy metals in the aquatic
ecosystem has been of increasing concern because of their
toxic properties and other adverse effects on natural waters
quality, such as Ni, Cu, Zn, Cr, Cd and Pb.
The most important distinctive feature of clay minerals
is their ability to balance with geochemical conditions.
Clay mineral stability in changing environment is the
function of their origin, structure, elementary cell charge
and dispersion [1-3]. Heavy metal cations can be immo-
bilized on silicates by two mechanisms: ion-exchange
and chemisorption [4]. Ion-exchange involves a substitu-
tion of ions present in silicate crystalline lattice by metal
ions from the solution. Ion exchange properties of smec-
tites are connected with the presence of non-compensated
negative charges [5]. Clay minerals (smectites) in soil
play the role of a natural barrier.
In one hand, Pb (II) can be removed from aqueous/
acidic solutions by using bentonite and natural sepiolite
as an adsorbent [6,7]. The removal of Cu (II) from
aqueous solution by using kaolinite, montmorillonite and
their modified adsorbents [8]. In other hand, Ni(II) and
Cu(II) were trapped in smectite structure using
ion-exchange mechanism [2,5].
Hectorite is one of the triocathedral subgroup of the
smectite. The Mg (II) dominates the octahedral sites in
both these minerals and a partial substitution of Li(I) for
Mg(II) occurs in hectorite. Isomorphic substitution within
the tetrahedral and/or octahedral sheets causes a negative
charge on the layers, which is balanced by hydrated ex-
changeable cations in the interlayer space (mainly Ca2+,
Mg2+ and Na+). The hydration states varies and depends
on many factor related to the composition of the layers
and the nature of the interlayer cation [2,3].
The work described in this paper was designed to
study the possibility of trapping heavy metals into hec-
torite structure using XRD simulation.
2. Materials and Methods
2.1. Synthesis
The synthetic hectorite sample was prepared by hydro-
thermal treatment of hydrolyzed gels prepared by co-
precipitation of Na, Mg, Al, and Si hydroxides at pH =
14, according to a slightly modified version of the gelling
method of Hamilton and Henderson [9]. The source of
Na was sodium carbonate, the sources of Al and Mg
were titrated solutions of their nitrates. The source of Si
was (C2H5O)4Si (TEOS). This resulting gel is slowly
dried up to 200˚C. It is then calcined at 600˚C by further
temperature increase. It is then introduced in Morey type
externally heated pressure vessels in which the samples

Structural Properties of Synthetic Na-Hectorite Exchanged with Heavy Metals 412
are insulated from the vessel wall by a silver coating. The
hydrothermal reactor was then heated at 400˚C under a
1000 bar water pressure. Samples were recovered after
four weeks. The started synthetic material has a structural
formulae: [Na0.4]inter[Mg2.6Li0.4]oct[Si4]tetO10(OH)2, x H2O
(x is the number of water molecule per cation). Hectorite
exchanged with Cd2+, Pb2+, Ni2+, Zn2+, Mg2+ and Co2+
were prepared by conventional ions exchanges reactions
using respectively aqueous solutions of 0.1M of CoCl2,
CdCl2, PbCl2, MgCl2, ZnCl2 and NiCl2. Removal of ex-
cess chloride was performed by washing in distilled wa-
ter until a negative AgNO3 test was obtained; the solids
were deposed on glass slide to obtain an oriented aggre-
gate; the samples are referred as H-Cd and H-Co, H-Ni,
H-Zn, H-Mg and H-Pb.
2.2. X-Ray Measurement and Simulation
Principle
XRD patterns were recorded using a Brüker D8-advance
using Cu-Kα radiation (1.5406 Å). Data were recorded in
the range 5˚ - 50˚2θ with a step of 0.02˚2θ and 0.05 s per
step. The mineralogical and structural characteristics
were determined by comparing the experimental X-ray
patterns with the theoretical patterns calculated from
structural models [10,11] and permits determination of
the number and the position of the intercalated water
molecules. The XRD patterns were calculated using the
z-coordinates, where the origin of the atomic coordinates
was taken at the basal oxygen atoms [2]. The diffracted
intensity for a unit-cell along the 00 rod of the reciprocal
space is given by the following expression [12]:

 


00
1n
2
SpurRe2M
p
n
LMnM




 
 




I
WI Q 
(1)
with
2
1cos2
sin 2
p
L




 [12], where Ψ is the orientation
factor of the particles and where Re is the real part of the
final matrix, Spur, the sum of the diagonal terms of the
real matrix; M, the number of layers per stack; n, an in-
teger varying between 1 and M − 1; [

], the structure
factor matrix; [I], the unit matrix; [W], the diagonal ma-
trix of the proportions of the different kinds of layers,
and [Q] the matrix representing the interference phe-
nomena between adjacent layers. For a system made up
of two types of layers (A and B) and a nearest neighbour
interaction, [Q] takes the form:
 
 
exp2 πexp2π
det exp2πexp2π
AAA ABA
BAB BBB
Pi sdPisd
PisdPi sd

 Q (2)
where s is the modulus of the scattering vector;
2sin
s


, dA and dB are the d-spacing of layer A and
layer B, respectively, and PAB is the conditional probabil-
ity of passing from a layer A to layer B. The relationship
between the different kinds of layer proportions and
probabilities are given by:
WA + WB = 1, PAA + PAB = 1, PBA + PBB = 1
and WAPAB = WBPAB.
The relationships between these probabilities and the
abundances WA and WB of the different types of layers
are given by Drits and Tchoubar [11]: 1) the segregation
tendency is given by: WA < PAA and WB < PBB, 2) The
total demixion is obtained for PAA = PBB= 1, 3) The regu-
lar tendency is obtained if: WA < PBA < 1 and WB < PAB <
1 and finally the limit between the last distribution la-
belled random distribution when WA = PBA = PAA and WB
= PAB = PBB; with ΣWA = 1, ΣPAB = 1.
The overall fit quality was assessed using the un-
weighted Rp parameter [13]:
 

2
2
22
2
obs icalc i
p
obs i
II
R
I








 (3)
where Iobs and Icalc represent respectively measured and
calculated intensities, at position 2θi, the subscript I run-
ning over all points in the refined angular range. This
parameter is mainly influenced by the most intense dif-
fraction maxima, such as the 001 reflection, which con-
tains essential information on the proportions of the dif-
ferent layer types and on their layer thickness.
3. Results and Discussion
3.1. Qualitative Description of Experimental
Patterns
Figure 1 shows the evolution of the d(001) values mea-
sured on the experimental XRD patterns. The different
values of the samples are listed also in the Table 1 with
the full width at half maximum intensity (FWHM) of the
001 reflection. The qualitative survey of diffractions pat-
terns shows that in most of these diffractograms a dis-
symmetry with regard to the first order and it is very re-
markable for the two complex H-Ni and H-Pb (Figures
1(d), 1(e)), for all the complexes the basal distances are
all inferior to 15Ǻ except the H-Zn complex (Figure 1(f))
where the d(001) is equal to 15.58 Ǻ. For superior orders,
it is clear the presence of the 002-003-004-005 reflec-
tions (H-Zn), the 002 reflection decreases for the H-Cd,
H-Co and H-Mg (Figures 1(a) to (c)) and this reflection
disappears for the H-Ni and H-Pb (Figures 1(d), 1(e)),

Structural Properties of Synthetic Na-Hectorite Exchanged with Heavy Metals 413
Figure 1. XRD patterns of the Hectorite saturated by: (a) Cd, (b) Co, (c) Mg, (d) Ni, (e) Pb and (f) Zn.
Table 1. The different basal distance of the hectorite com-
plexes and their respective FWHM.
Complexes d001(Ǻ) FWHM
H-Cd 14.91 0.416
H-Co 14.92 0.278
H-Pb 14.74 0.690
H-Ni 14.89 0.590
H-Mg 14.66 0.276
H-Zn 15.58 0.221
the 003-004 and 005 reflections decreases for all the
complexes H-Cd, H-Co (Figures 1(a), 1(b)) and these
orders had a weak intensity for the H-Mg, H-Ni and
H-Pb (Figures 1(c)-(e)). We can concluded that all the
complexes presents a bilayer water in the interlayer space;
now we will try to determine the number and the position
of the water molecules surrounding the different cations
and shows if our complexes are homogenous or not.
Looking at the FHWM values (Table 1), we remark that
the biggest values are attributed to the H-Pb and H-Ni
complexes and for the others these values still so similar.
3.2. Quantitative Description of Some Complexes
The hydration state of smectites has been described using
four layer types of different layer thickness and corre-
sponding to the most common hydration states reported
for smectites in no saturated conditions: dehydrated lay-
ers (0 W, layer thickness ~9.6 - 10.1 Å), monohydrated
layers (1 W, layer thickness ~12.3 - 12.7 Å), and bi-hy-
drated layers (2 W, layer thickness ~15.1 - 15.8 Å) and
trihydrated (3 W, layer thickness ~18 - 19 Å) layers, the
latter being less common [14,15].
H-Mg: The first remark to mention is that the H-Mg is
not totally homogenous, we try to determine the different
phases which exists in the complexes and this using the
quantitative study of the XRD pattern. The best agree-
ment (Figure 2) between the theoretical and experimen-
tal pattern is obtained with an abundance of: WA = 0.912,
WA = 0.0880 and the respective probabilities are: PAA=
0.912, PAB = 0.0880, PBA = 0.912, PBB = 0.0880, the ma-
jor phase (phase A) is a bilayer one characterised by a
basal distance of 15.2 Ǻ, the second phase (phase B) is so
minor and characterised by a basal distance of 18.44 Ǻ.
This agreement is obtained with a Rp factor equal to
3.5%. This result converge to the results found by Ski-
pper [16] and Greathouse [17] using Monte Carlo simu-
lation concluded that the Mg2+ cations are systematically
octahedrally coordinated in 2W smectites and located in
the mid-plane of the interlayer.
H-Cd and H-Co: The H-Cd complex is characterised
by a basal distance situated at 14.91 Ǻ. The best agree-
ment between theoretical and experimental pattern is
obtained using with an abundance of: WA = 0.78, WB =
0.22 and the respective probabilities are: PAA = 0.9, PAB =
0.1, PBA = 0.35, PBB =0.65 (Figure 3). With A is a bi
layer hydrated state (d001 = 15.2 Ǻ) and B is a one hy-
drated layer (d001 = 12.4 Ǻ) The Rp factor is equal to
6.2%.
H-Pb: The d001 basal distance appears at 14.91 Ǻ. The
complex is not homogenous; the abundance of each
phase are WA = 0.6, WB = 0.4 where A is a bilayer hy-
drated state (d001 = 15.2 Ǻ), and B is a one hydrated layer
(d001 = 12.4 Ǻ), the respective probabilities are PAA = 0.8,
PAB = 0.2, PBA = 0.3, PBB = 0.7. The best agreement be-
tween calculated and experimental XRD patterns is re-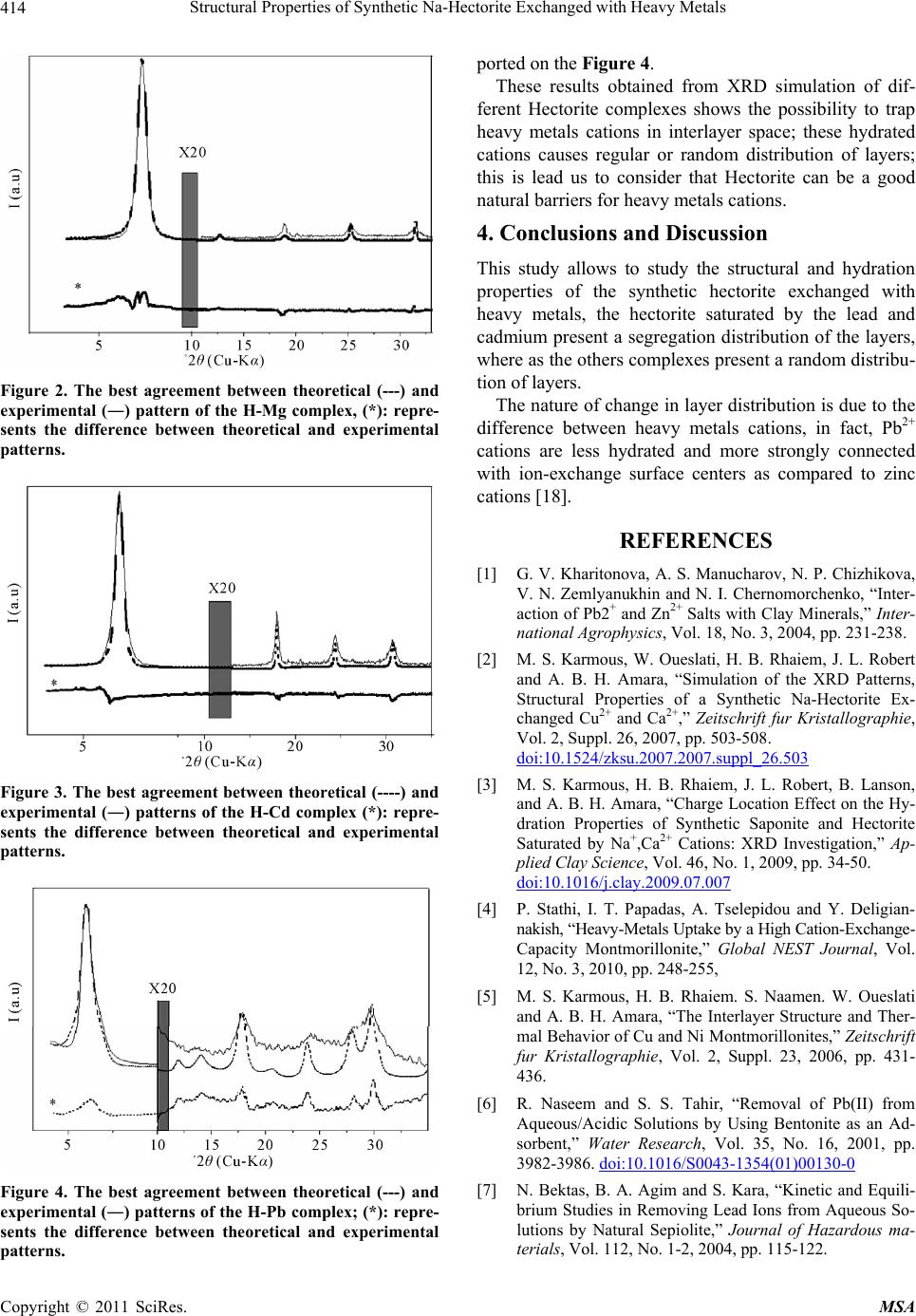

Structural Properties of Synthetic Na-Hectorite Exchanged with Heavy Metals 414
Figure 2. The best agreement between theoretical (---) and
experimental (―) pattern of the H-Mg complex, (*): repre-
sents the difference between theoretical and experimental
patterns.
Figure 3. The best agreement between theoretical (----) and
experimental (―) patterns of the H-Cd complex (*): repre-
sents the difference between theoretical and experimental
patterns.
Figure 4. The best agreement between theoretical (---) and
experimental (―) patterns of the H-Pb complex; (*): repre-
sents the difference between theoretical and experimental
patterns.
ported on the Figure 4.
These results obtained from XRD simulation of dif-
ferent Hectorite complexes shows the possibility to trap
heavy metals cations in interlayer space; these hydrated
cations causes regular or random distribution of layers;
this is lead us to consider that Hectorite can be a good
natural barriers for heavy metals cations.
4. Conclusions and Discussion
This study allows to study the structural and hydration
properties of the synthetic hectorite exchanged with
heavy metals, the hectorite saturated by the lead and
cadmium present a segregation distribution of the layers,
where as the others complexes present a random distribu-
tion of layers.
The nature of change in layer distribution is due to the
difference between heavy metals cations, in fact, Pb2+
cations are less hydrated and more strongly connected
with ion-exchange surface centers as compared to zinc
cations [18].
REFERENCES
[1] G. V. Kharitonova, A. S. Manucharov, N. P. Chizhikova,
V. N. Zemlyanukhin and N. I. Chernomorchenko, “Inter-
action of Pb2+ and Zn2+ Salts with Clay Minerals,” Inter-
national Agrophysics, Vol. 18, No. 3, 2004, pp. 231-238.
[2] M. S. Karmous, W. Oueslati, H. B. Rhaiem, J. L. Robert
and A. B. H. Amara, “Simulation of the XRD Patterns,
Structural Properties of a Synthetic Na-Hectorite Ex-
changed Cu2+ and Ca2+,” Zeitschrift fur Kristallographie,
Vol. 2, Suppl. 26, 2007, pp. 503-508.
doi:10.1524/zksu.2007.2007.suppl_26.503
[3] M. S. Karmous, H. B. Rhaiem, J. L. Robert, B. Lanson,
and A. B. H. Amara, “Charge Location Effect on the Hy-
dration Properties of Synthetic Saponite and Hectorite
Saturated by Na+,Ca2+ Cations: XRD Investigation,” Ap-
plied Clay Science, Vol. 46, No. 1, 2009, pp. 34-50.
doi:10.1016/j.clay.2009.07.007
[4] P. Stathi, I. T. Papadas, A. Tselepidou and Y. Deligian-
nakish, “Heavy-Metals Uptake by a High Cation-Exchange-
Capacity Montmorillonite,” Global NEST Journal, Vol.
12, No. 3, 2010, pp. 248-255,
[5] M. S. Karmous, H. B. Rhaiem. S. Naamen. W. Oueslati
and A. B. H. Amara, “The Interlayer Structure and Ther-
mal Behavior of Cu and Ni Montmorillonites,” Zeitschrift
fur Kristallographie, Vol. 2, Suppl. 23, 2006, pp. 431-
436.
[6] R. Naseem and S. S. Tahir, “Removal of Pb(II) from
Aqueous/Acidic Solutions by Using Bentonite as an Ad-
sorbent,” Water Research, Vol. 35, No. 16, 2001, pp.
3982-3986. doi:10.1016/S0043-1354(01)00130-0
[7] N. Bektas, B. A. Agim and S. Kara, “Kinetic and Equili-
brium Studies in Removing Lead Ions from Aqueous So-
lutions by Natural Sepiolite,” Journal of Hazardous ma-
terials, Vol. 112, No. 1-2, 2004, pp. 115-122.

Structural Properties of Synthetic Na-Hectorite Exchanged with Heavy Metals 415
doi:10.1016/j.jhazmat.2004.04.015
[8] G. Krishna and S. S. S. Gupta, “Kaolinite, Montmorillo-
nite and Their Modified Derivatives as Adsorbents for
Removal of Cu (II) from Aqueous Solution,” Separation
and purification Technology, Vol. 50, No. 3, 2006, pp.
388-397. doi:10.1016/j.seppur.2005.12.014
[9] D. L. Hamilton, and C. M. B. Henderson, “The Prepara-
tion of Silicate Compositions by a Gelling Method,”
Mineralogy Magazine, Vol. 36, No. 1, 1968, pp. 832-838.
doi:10.1180/minmag.1968.036.282.11
[10] C. H. Pons, C. de la Calle and M. de Vidales, “Quantifi-
cation Curves for XRD Analysis of Mixed-Layer 14A/
10A Clay Minerals,” Clays and Clay Minerals, Vol. 43,
No. 1, 1995, pp. 246-254.
doi:10.1346/CCMN.1995.0430212
[11] V. A. Drits and C. Tchoubar, “X-Ray Diffraction by Dis-
ordered Lamellar Structures: Theory and Application to
Microdevided Silicates and Carbons,” Springer Verlag,
New York, 1990, pp. 165-198.
[12] A. B. H. Amara, J. B. Brahim, A. Plançon and H. B.
Rhaiem, “Étude par Diffraction X des Modes d’Empi-
lement de la Nacrite Hydratée et Deshydratée,” Journal of
Applied Crystallography, Vol. 31, No. 5, 1998, pp. 654-
662.
[13] S. A. Howard and K. D. Preston, “Profile Fitting of Pow-
der Diffraction Patterns,” In: D. L. Bish and J. E. Post,
Eds., Modern Powder Diffraction, Reviews in Mineralogy
20, Mineralogical Society of America, Wahington D.C.,
1989, pp. 217-275.
[14] E. Ferrage, B. Lanson, N. Malikova, A. Plançon, B. A.
Sakharov and V. A. Drits, “News Insights in the Distribu-
tion of Interlayer H2O Molecules in Bi-Hydrated Smec-
tites from X-Ray Diffraction Profile Modeling of 00l Re-
flections,” Chemistry of Materials, Vol. 17, No. 13, 2005,
pp. 3499-3512. doi:10.1021/cm047995v
[15] E. Ferrage, B. Lanson, B. A. Sakharov and V. A. Drits,
“Investigation of Smectites Hydration Properties by
Modeling Experimental X-Ray Diffraction Patterns. Part I.
Montmorillonite Hydration Properties,” American Miner-
alogist, Vol. 90, No. 8, 2005, pp. 1358-1374.
doi:10.2138/am.2005.1776
[16] N. T. Skipper, K. Refson and J. D. C. McConnell,
“Computer Simulation of Interlayer Water in 2:1 Clays,”
Journal of Chemical Physics, Vol. 4, No. 11, 1991, pp.
7434-7445. doi:1 0.1063/ 1.46 017 5
[17] J. A. Greathouse, K. Refson and G. Sposito, “Molecular
Dynamics Simulation of Water Mobility in Magnesium
Smectite Hydrates,” Journal of American Chemical Soci-
ety, Vol. 122, No. 46, 2000, pp. 11459-11464.
doi:10.1021/ja0018769
[18] U. Förstner and G. T. W. Wittmann, “Metal Pollution in
the Aquatic Environment,” Springer, New York, 1983.