Applied Mathematics, 2011, 2, 379-382
doi:10.4236/am.2011.23045 Published Online March 2011 (http://www.SciRP.org/journal/am)
On Hilbert’s Integral Inequality and Its Applications
Waad T. Sulaiman
Department of Computer Engineering, College of Engineering, University of Mosul, Mosul, Iraq.
Received December 3, 2010; revised January 29, 2011; accepted Febru ar y 2, 2011
Abstract
The main result of this paper is presented as follows Let ,,, 0,fghk h is homogeneous and symmetric of
degree

and
 
,1 0.Fxykx ky Then
 
  


  
422
12 12
000 0
22
12 12
00
,
,
fxgydxdyCxfx dxCkxCxxfx dx
hxy
CxgxdxCkx Cxxgxdx


 




  



 


  


 


 

 

 

where
 



00
,,
1, 1,
a
akxtt
t
CdtCx dt
ht ht



provided the integrals on the RHS do exists. Some other special cases are also deduced.
Keywords: Hilbert's Integral Inequality, Holder's Inequality, Weight Function
1. Introduction
If
 
00
11
,0, 1,
0,0,
pq
fg pq
fxdx gxdx




then
 
  
00
1/ 1/
00
sin
p
q
pq
fxgy
dxdy
xy
f
xdxg xdx
p










(1)
where the constant factor

sin p  is the best possi-
ble. Many mathematicians presented generalizations or
new kinds of (1). Hardy inequality is very important in
analysis theory and applications, it has been absorbing
much interest of analysis see ([1,2]) .Very recently P. X.
Ying and G. Mingzhe (see [3]) proved the following new
kind
Theorem 1.1. Let


f
xbe a real function. If

2
0
0,fxdx



 then
 
 
2
00
22
2
00
pp
fxfy
dxdy
xy
fxdx xfxdx

















(2)
where

11
1
1
x
.
x
x
 


2. Lemma
The following lemma is needed for our aim.
Lemma 2.1. Let


,hxy be symmetric. Then




   

00 00
,
,,
fxfy fxfy
F
x ydxdydxdy
hxy hxy
 


(3)
where




1
F
x,yk xky
Proof.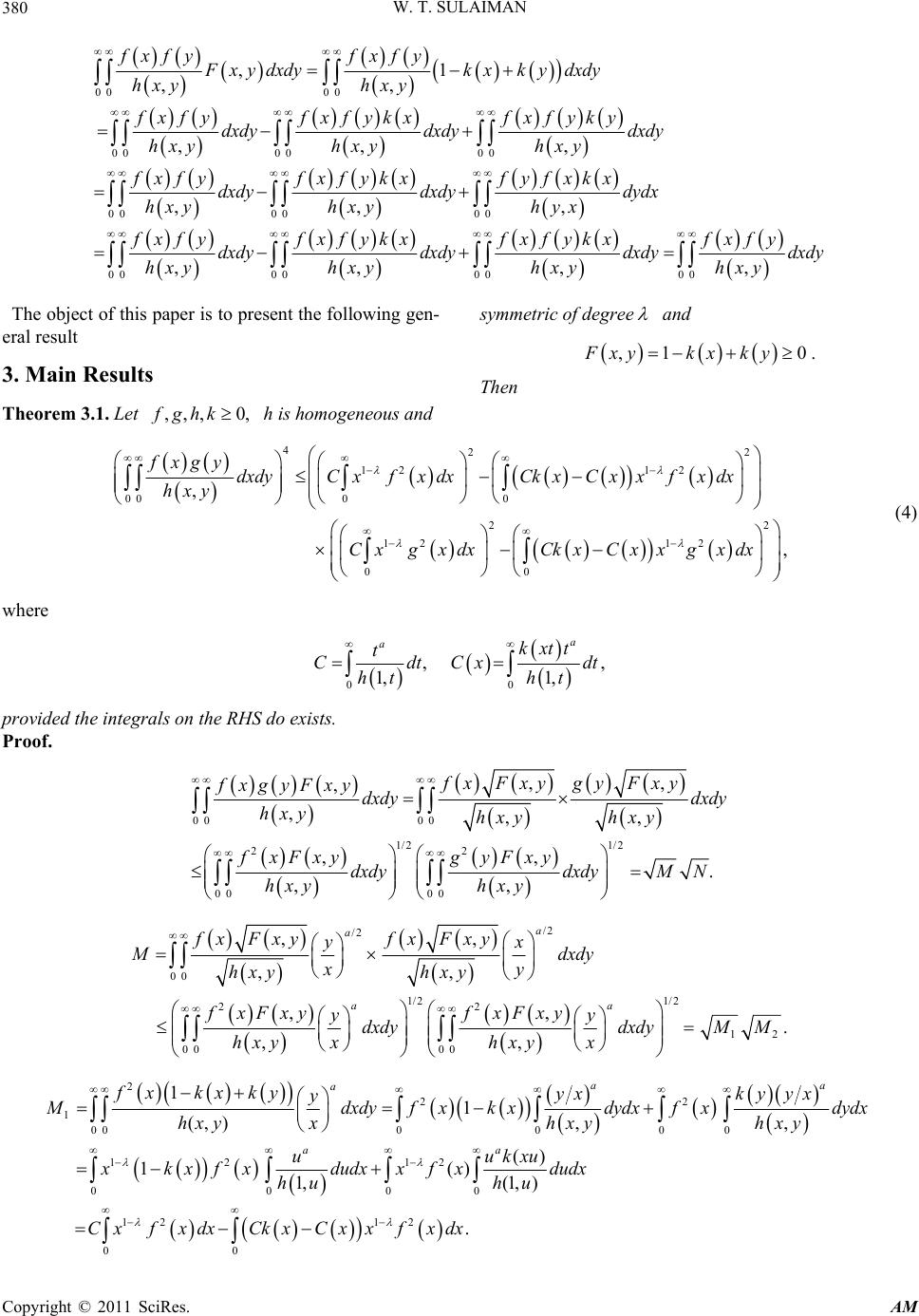

W. T. SULAIMAN 380
 
  


  

 

 

 

 

 

 

 

 

00 00
00 0000
00 0000
00
,1
,,
,, ,
,, ,
,,
fxfyfxfy
Fx ydxdykxkydxdy
hxy hxy
fxfyfxfykx fxfyky
dxdy dxdydxdy
hxy hxyhxy
fxfy fxfykxfyfxkx
dxdy dxdydydx
hxy hxyhyx
fxfyfxfykx
dxdy
hxy hxy
 
 
 


 
 

 
 
 

 

 

00 0000
,,
fxfykx fxfy
dxdydxdy dxdy
hxy hxy
 

 
The object of this paper is to present the following gen-
eral result
3. Main Results
Theorem 3.1. Let ,,, 0,fghk h is homogeneous and
symmetric of degree

and


,1 0Fxykxky

 .
Then
 
 


  
422
12 12
000 0
22
12 12
00
,
,
fxgydxdyCxfxdxCkxCxxfxdx
hxy
CxgxdxCkx Cxxgxdx


 





 



 


 










 

(4)
where
 



00
,,
1, 1,
a
akxtt
t
CdtCx dt
ht ht



provided the integrals on the RHS do exists.
Proof.
 





 

 

0000
1/21/2
22
00 00
,,
,
,,,
,,
.
,,
fx Fxygy Fxy
fxgyFxy
dxdy dxdy
hxy hxy hxy
fxFxy gyFxy
dxdydxdyMN
hxy hxy
 
 






 
 




 

 

/2
/2
00
1/21/2
22
12
00 00
,,
,,
,,
.
,,
a
a
aa
fx Fxyfx Fxy
yx
Mdxdy
xy
hxy hxy
fxFxy fxFxy
yy
dxdydxdyM M
hxy xhxy x

 



 
 

 
 
 

 


 
 
  

  


   
   
2
22
100000 0
12 12
0000
12 12
00
11
(, ),,
()
1()
1,(1, )
.
aa
a
aa
fx kxkyyxky yx
y
M
dxdyf xkxdydxfxdydx
hxyxhxyhxy
uukxu
xkxfxdudxxfxdudx
hu hu
CxfxdxCkxCxxfxdx


 




 



 

 



W. T. SULAIMAN 381
Similarly,
   
12 12
200
.
M
CxfxdxCkxCx xfxdx





Therefore

  

2
212
0
2
12
0
,
MCxfxdx
CkxCxxfx dx













and

 


2
212
0
2
12
0
.
NCxgxdx
CkxCxxgx dx













Applying lemma 2.1 to have
 

 

   
 


44
00 00
22
12 12
00
22
12 12
00
,
,,
.
fxgy fxgyFxy
dxdy dxdy
hxy hxy
CxfxdxCkxCx xfxdx
CxgxdxCkx Cxxgxdx


 




 

 
 
 

 


 

 









 


Remark. It may be mentioned that theorem 1.1 follows
from corollary 4.1 by putting
 
,,12,1,()11hxyx y akxx  
as follows
12
2
00
2
11
/
udu
Cdu
uu

 



.


2
1/2
2
00
() 11
kxu
kxuu
C xdudu
uu






22
0
,
1 11
du
x
xu u



 

and
 
11
11
Ck xC xx
x


 



Theorem 3.2. Let 0,01,0.fg

 Then
 


 
222
212 12
22
00 00
11 ,. fxfygxgydxdyBxf xdxxf xgxdx
xy



 

 
 
 


 



  (5)
Proof.
 

 

 

 


 


1
142
42
/2 /2
00 00
1/2
1/21
22 12
2
00 00
111 1
11
.
fxfy gxgyfx gxfygy
yx
dxdy dxdy
xy
xyxy xy
fx gxfygy
yx
dxdydxdyG H
xy
xy xy








 


 
 






 




 


  


 



 
 
 

  



 

11
1
22
22 12
22
0000 0
()
11,1
1
yxx t
GfxgxdydxfxgxdtdxB xfxgxdx
xy t





  
 
 
 
 

 
 

Similarly

W. T. SULAIMAN 382

  
12
22 0
,1
H
Byfygydy




,
and hence
 
  
222
212 12
00 00
1()1,.
22
fxfygxgydxdyGHBxfx dxxfxgxdx
xy



 

 
 

 







 
 
4. Applications
Corollary 4.1. Let ,, 0,fhk h is homogeneous and
symmetric of degree

.
Then
 
  


222
12 12
000 0
,
fxfy
dxdyCxfxdxCkxCxxfxdx
hxy

 



 



 


 


  (6)
where
   
00
,,
1, 1,
a
akxtt
t
CdtCx dt
ht ht



provided the integrals on the RHS do exists.
Proof. The proo f follows from theo rem 3.1 by putting
g
f

Corollary 4.2. Let ,, 0,fhk h is homogeneous and
symmetric of degree

.
Then
 
  
 
222
12 12
0000
()
fxfydxdyCxfxdxCkxCxxfx dx
xy




 

 


 



 (7)
where
    00
,,
11
a
akxtt
t
CdtCxdt
tt





provided the integrals on the RHS do exists.
Proof. The proof follows from corollar y 4 .1 by pu tting
 
,.hxyx y


Corollary 4.3. Let ,, 0,fhk h is homogeneous and
symmetric of degree

.
Then
  


222
12 12
0000
fxfydxdyCxfx dxCkxCxxfxdx
xy





  


  

 


 (8)
where
 
00
,,
11
a
akxtt
t
CdtCxdt
tt





provided the integrals on the RHS do exists.
Proof. The proof follows from corollary 4.1 by putting

,.hxy xy



5. References
[1] G. H. Hardy, J. E. Littlewood and G. Polya, “Inequali-
ties,” Cambridge University Press, Cambridge, 1952.
[2] J. E. Mitronovic, J. E. Pecaric and A. M. Fink, “Inequali-
ties Involving Functions and Their Integrals and Deriva-
tives,” Boston Kluwer Academic Publishers, 1991.
[3] P. X. Ying and G. Mingzhe, “On Hilbert’s Integral In-
equality and Its Applications,” Journal of Applied Ma-
thematics, Preprint.