Applied Mathematics, 2011, 2, 329-342
doi:10.4236/am.2011.23039 Published Online March 2011 (http://www.SciRP.org/journal/am)
Vibration and Buckling Approximation of an Axially
Loaded Cylindrical Shell with a Three Lobed Cross
Section Having Varying Thickness
Mousa Khalifa Ahmed
Department of Mathematics, Faculty of Science at Qena, South Valley University, Qena City, Egypt
Received November 2, 2010; revised January 17, 2011; accepted January 20 , 20 1 1
Abstract
On the basis of the thin-shell theory and on the use of the transfer matrix approach, this paper presents the vi-
brational response and buckling analysis of three-lobed cross-section cylindrical shells, with circumferen-
tially varying thickness, subjected to uniform axial membrane loads. A Fourier approach is used to separate
the variables, and the governing equations of the shell are formulated in terms of eight first-order differential
equations in the circumferential coordinate, and by using the transfer matrix of the shell, these equations are
written in a matrix differential equation. The transfer matrix is derived from the non-linear differential equa-
tions of the cylindrical shells with variable thickness by introducing the trigonometric series in the longitu-
dinal direction and applying a numerical integration in the circumferential direction. The natural frequencies
and critical loads beside the mode shapes are calculated numerically in terms of the transfer matrix elements
for the symmetrical and antisymmetrical vibration modes. The influences of the thickness variation of cross-
section and radius variation at lobed corners of the shell on the natural frequencies, mode shapes and critical
loads are examined.
Keywords: Free Vibration, Buckling, Vibration of Continuous System, Noncircular Cylindrical Shell,
Transfer Matrix Approach, Variable Thickness, Axial Loads
1. Introduction
In recent years, structural engineers have been gradually
concerned with the analysis of vibration and stability
problems of circular cylindrical shells which have non-
circular profiles because of the greatest important in many
engineering applications such as in the design of mach-
ines and structures. The vibration and buckling modes of
thin elastic shells essentially depend on some determin-
ing functions such as the radius of the curvature of the
neutral surface, the shell thick ness, the shape of the shell
edges, etc. In simple cases when these functions are con-
stant, the vibration and buckling modes occupy the entire
shell surface. If the determining functions vary from point
to point of the neutral surface then localization of the vi-
bration and buckling modes lies near the weakest lines
on the shell surface, and this kind from problems is too
difficult because the radius of its curvature varies with
the circumferential coordinate, closed-form or analytic
solutions cannot be obtained, in general, for this class of
shells, numerical or approximate techniques are necessa-
ry for their analysis. The vibration and stability of circu-
lar cylindrical shells have been studied by many resear-
chers since the basic equations for these were established
by Flügge [1] and Donnell [2]. Becker and Gerard [3],
Gerard [4] Cheng and Ho [5], Jones [6], Stavsky and
Friedland [7] and Lei and Cheng [8] studied the elastic
stability of orthotropic or composite shells under axial
loading. Greenberg and Stavsky [9-12] studied the vibra-
tions and buckling of laminated orthotropic shells under
compression. Rosen and Singer [13] have carried out a
theoretical and experimental investigation of the vibra-
tions of axially loaded stiffened cylindrical shells. Ya-
mada et al. [14,15] studied the vibration and stability of
circular cylindrical shells subjected to axial load and also
the free vibration of noncircular cylindrical shells with
variable circumferential profiles. In recent years, Suzuki
and Leissa [16] beside Kumar and Singh [17] studied the
approximate vibrational analysis of noncircular cylin-
drical shells having circumferentially varying thickness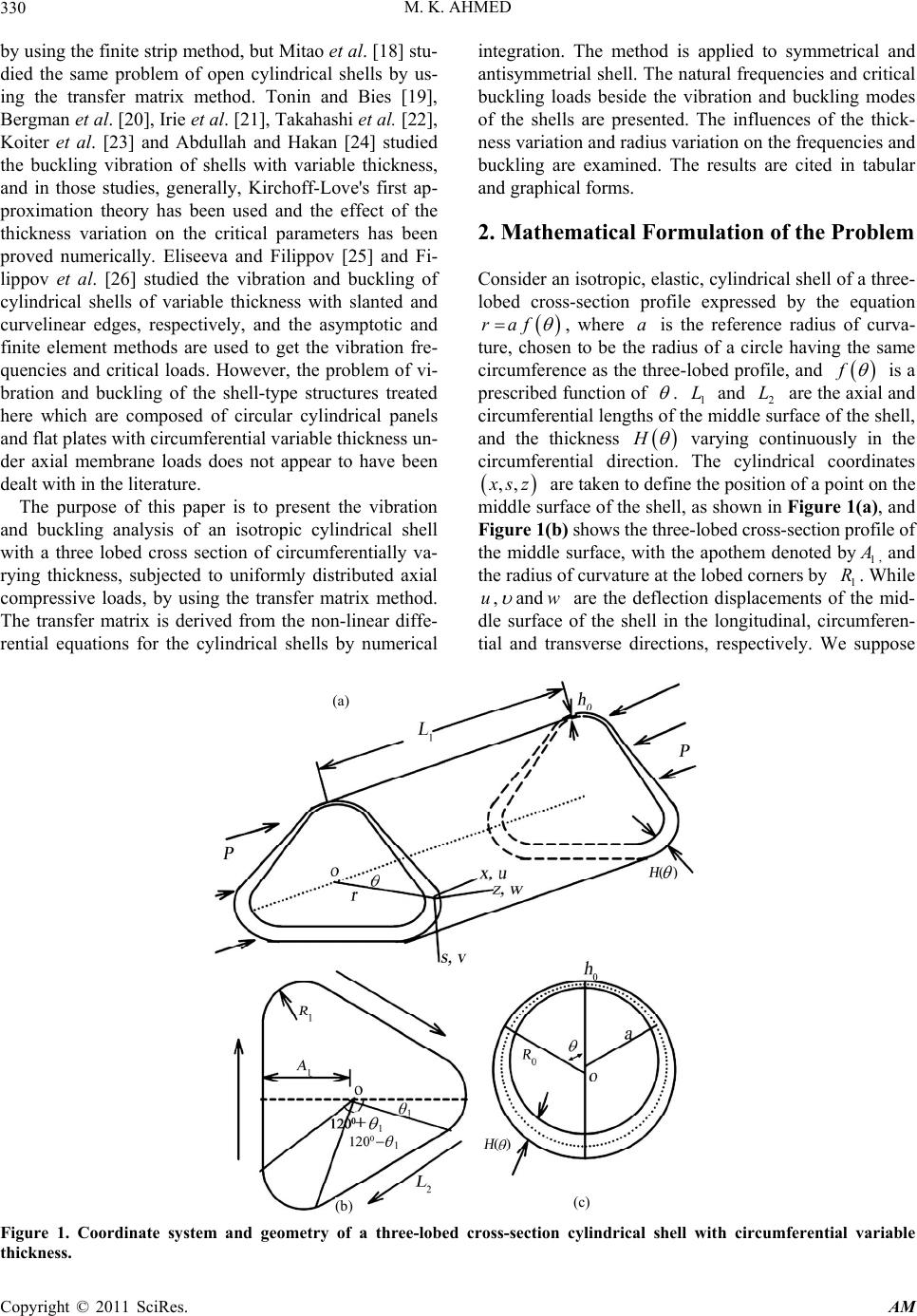

M. K. AHMED 330
by using the finite strip method, but Mitao et al. [18] stu-
died the same problem of open cylindrical shells by us-
ing the transfer matrix method. Tonin and Bies [19],
Bergman et al. [20], Irie et al. [21], Takahashi et al. [22],
Koiter et al. [23] and Abdullah and Hakan [24] studied
the buckling vibration of shells with variable thickness,
and in those studies, generally, Kirchoff-Love's first ap-
proximation theory has been used and the effect of the
thickness variation on the critical parameters has been
proved numerically. Eliseeva and Filippov [25] and Fi-
lippov et al. [26] studied the vibration and buckling of
cylindrical shells of variable thickness with slanted and
curvelinear edges, respectively, and the asymptotic and
finite element methods are used to get the vibration fre-
quencies and critical loads. However, the problem of vi-
bration and buckling of the shell-type structures treated
here which are composed of circular cylindrical panels
and flat plates with circumferential variable thickness un-
der axial membrane loads does not appear to have been
dealt with in the literature.
The purpose of this paper is to present the vibration
and buckling analysis of an isotropic cylindrical shell
with a three lobed cross section of circumferentially va-
rying thickness, subjected to uniformly distributed axial
compressive loads, by using the transfer matrix method.
The transfer matrix is derived from the non-linear diffe-
rential equations for the cylindrical shells by numerical
integration. The method is applied to symmetrical and
antisymmetrial shell. Th e natural frequencies and critical
buckling loads beside the vibration and buckling modes
of the shells are presented. The influences of the thick-
ness variation and radius variation on the frequencies and
buckling are examined. The results are cited in tabular
and graphical forms.
2. Mathematical Formulation of the Problem
Consider an isotropic, elastic, cylindrical shell of a three-
lobed cross-section profile expressed by the equation


raf

, where a is the reference radius of curva-
ture, chosen to be the radius of a circle having the same
circumference as the three-lobed profile, and


f

is a
prescribed function of

. 1 L and 2 L are the axial and
circumferential lengths of the middle su rface of the shell,
and the thickness


H

varying continuously in the
circumferential direction. The cylindrical coordinates


,,
x
sz are taken to define the position of a point on the
middle surface of the shell, as shown in Figure 1(a), and
Figure 1(b) shows the three-lobed cross-section profile of
the middle surface, with the apothem denoted by1
A
, and
the radius of curvature at the lobed corners by 1 R. While
,u

and w are the deflection displacements of the mid-
dle surface of the shell in the longitudinal, circumferen-
tial and transverse directions, respectively. We suppose
Figure 1. Coordinate system and geometry of a three-lobed cross-section cylindrical shell with circumferential variable
thickness.
(a)
(b) (c)

M. K. AHMED 331
that the shell thickness
H
at any point along the cir-
cumference is small and depends on the coordinate

and takes the following form:
 
0
H h

  (1)
where 0 h is a small parameter, chosen to be the average
thickness of the shell over the length 2 L. For the cylin-
drical shell which cross-section is obtained by the cuta-
way the circle of the radius 0 r from the circle of the
radius 0 R(see Figure 1(c)) functio n


 have the form:
 
11cos

    where

is the amplitude of thi-
ckness variation, 0 dh

, and d is the distance be-
tween the circles centers. In general case


00hH
 
is the minimum value of


 while


mHh

  is
the maximu m value of



 , and in case of 0d

the
shell has constant thickness 0 h. The dependence of the
shell thickness ratio 0m hh


on

has the form
12



.
For studying the free harmonic vibrations and buck-
ling of the shell under consideration, the equilibrium
equations of forces for the cylindrical shell, subjected to
an axial compressive load P, based on the Goldenveiz-
er-Novozhilov theory are taken from [27,28] as follows:
 

22
2
0, 0,
0, 0,
0, 0,0,
xsxxs ss
xs sxsxx
xssss ssxxssxsx
NN PuHuNNQRPH
QQNRPwHwMM Q
MMQ SQMNNMR
 




   
   
  
(2)
where Nx, Ns and Qx, Qs are the normal and transverse
shearing forces in the x and s directions, respectively, Nsx
and Nxs are the in-plane shearing forces, Mx, Ms and Mxs,
Msx are the bending moment and the twisting moment,
respectively, Ss is the equivalent (Kelvin-Kirchoff) shea-
ring force, P is the axial force per unit length, constant
along the circumference,

is the mass density, R is the
radius of curvature of the middle surface,

is the an-
gular frequency of vibration, 'x , and
s


 .
The relations between strains and deflections for the
cylindrical shells used here are taken from [29] as fol-
lows:

,,, 0,
0, ,,
xsxsxz x
szsx xs ssx sxsx
uwRuw
wRkkwRR,kk R
 
 



 


  (3)
where
x

and
s

are the normal strains of the middle
surface of the shell, ,
x
sxz

 and
s
z

are the shear
strains, and the quantities ,,
x
ssx
kkk and
x
s
k represen-
ting the change of curvature and the twist of the middle
surface,
x

is the bending slope, and
s

is the angu-
lar rotation.
The components of force and moment resultants in
terms of Equation (3) are given as:





  
,,12
,,1
xxs ssxxsxs
xxsss xsxsx
NDNDN D,
MKkkMKkkM kk
 

 
 (4)
From Equations (2)-(4 ), with eliminating the variables
,,,, ,
x
sxxs xxs
QQN NMM and
s
x
M
wh ich are not dif-
fer-rentiated with respect to
s
, the system of the partial
differential equations fo r the state variables ,,, ,
s
uw


,,
s
ss
M
SN and
s
x
N of the shell are obtained as fol-
lows:

 


2
22 2
22
2( (1))6,,,
,21,
1, ,
1.
sx sss
ss xssss
sssss sx
sx s
uD NHRNDwRuwr
MKNRDRuMSK
SNRM KwPwHwNPSRNH
NDuPu NHu
 
 

 



 

 
 
 
 
 
 
 
 
(5)
The quantities D and K, respectively, are the ex-
tensional and flexural rigidities expressed in terms of the
Young’s modulus E, Poisson’s ratio

and the wall
thickness


H

as the form:

2
1DEH

 and


32
12 1KEH

, and under the variable thickness
case, using Equation (1), those are written as follows:

M. K. AHMED 332
 
 
2
00
1DEh D



  (6)



 
323 3
00
1KEh K

   (7)
where 0 D and 0
K
are the reference extensional and
flexural rigidities of the shell, chosen to be the averages
on the middle surface of the shell over the length 2 L.
For the free vibration of a simply supported shell, the
solution of the system of Equation (5) is sought as fol-
lows:
     

 
 

  

 

  

 

 

     
111
1
1
1
,cos,,,,,sin,, sin,
,,,,,,,,,,sin,
,,,, ,,,cos,
,,,,sin,
,,, c
ss
xsss xsss
xssxxxssxx
xs xs
xssxxs sx
mmm
u xsUsxxswxsVsWsxxssx
LLL
m
NxsNxsQxsSxsNsNsQsSsx
L
m
N xsN xsQxsNsNsQsx
L
m
MxsMxsMsMsx
L
M xsM x,sM sM s











1
os ,1,2,
mxm
L


(8)
where m is the axial half wave number and the quanti-
ties
 
,UsV s are the state variables and undeter-
mined functions of
s
.
3. Matrix Form of the Basic Equations
The differential equations as shown previously are mod-
ified to a suitable form and solved numerically. Hence,
by substituting Equation (8) into Equation (5) and take
relations (6) and (7) into account, the system of vibration
equations of the shell can be written in non-linear ordi-
nary differential equations referred to the variable s only
are obtained, in the following matrix form:
12 1418
21 2327
32 34
41 43 45 47
54 56
63 65 67
7276 78
81 87
00000
0 0000
000000
0000
000 0 00
00 00 0
0000 0
000000
s s
s s
s
s
sx
VV V
UU
VVV
VV
VV
WW
VVVV
d
aVV
ds MM
VVV
S
VVV
N
VV
N

 
 
 
 
 
 

 
 
 
 
 
 










s
s
sx
.
S
N
N















(9)
By using the state vector of fundamental unknowns

Z
s, system (9) can be written as follows:
    
d
aZsVsZs
ds
  
 
 (10)


,, ,,,,,,
T
sssssx
ZsUVWM S N N  
 





0
2
0
,,,, ,
,1,
s
sss
UVWk UVW
kM M  








3
1
,,1,, ,
sssxsssxm
SNN SNN.
L

 

For the noncircular cylindrical shell which cross-sec-
tion profile is obtained by function (


raf

), the
hypotenuse


ds of a right triangle whose sides are
infinitesimal distances along the surface coordinates of
the shell takes the form
 
22 2
ds drrd

 , then we
have
 

2
2df ds
f
d
ad








(11)
By using Equation (11), the system of vibration Equa-
tions (10) takes the form:
    
dZVZ
d

 

 
 
 (12)

M. K. AHMED 333
Where
 


2
2,
df
fd


 




and the coefficients matrix


V




are give n as:

12
Vml

 ,


22
14 6Vmlh

 ,
 


32
18 61Vmlh

  ,

21
Vml  ,
23 1Vc ,



32
27 12Vmlh

 , 32 1Vc

,


34
Vml   , 41 Vc



2
43
Vml   , 3
45 1Vh

, 46 12Vhc


,

22
54 21Vhml

 
56 1V

,

 
43
232
63 1212Vml hmlPml

   ,


65
Vml  ,

67
Vm/lc  ,
 
3
2
72 12VPml hml
  ,
76 1Vc , 78
Vml

,



 
3
22 2
81 11212VhmlPmlhml
   ,

87
Vml

 
in terms of the following dimensionless shell parameters:
frequency parameter222
00
haD  , load factor

20
PaK P, 1 lLa and 0 hha.
As the function


f

formulates the profile of a shell
with a three lobed cross section, and expressed as in [15]
 





2
22
111 111
0 0
11 11
2
022 000
111 1111
00
11 1
2cos4 sin,for0
cosec30, ,for120
() ,
2cos1204sin120,for 120120
sec, ,for120180
acc ac
ac
f
acc ac
ac
 




 
 
 
 

 
 
 
 
 
 
 
 



1
111111 1111
,, ,tan343aAacRacacac 

 
The state vector

Z

of fundamental unknowns can
be expressed as in [30]
    
0ZYZ   
 (13)
by using the transfer matrix

Y




of the shell, and the
substitution of the expression into Equation (10) yields
 
 
,
0
dd YVY
YI.
    
 
 

(14)
The governing system (14) is too complicated to ob-
tain any closed form solution, and this problem is highly
favorable for solving by numerical methods. Hence, the
matrix

Y



is obtained by using numerical integra-
tion, by use of the Runge-kutta integration method of
forth order, with the starting value



0YI
 (unit
matrix) which is given by taking 0

 in Equation (13),
and its solution depends only on the geometric and mar-
tial properties of the shell, and the same solution can be
used for appropriate boundary conditions imposed at the
shell circumference.
For a plane passing through the central axis in a shell
with structural symmetry, symmetrical and antisymme-
trical profiles can be obtained, and consequently, only
one-half of the shell circumference is considered with the
boundary conditions at the ends taken to be the symme-
tric or antisymmetric conditions. Therefore, the boundary
conditions for symmetrical and antisymmetrical vibra-
tions are:
0, 0,
0,0,respectively
sssx
ss
VSN
UWN M
  
 



 (15)
4. Natural Frequencies and Modes
A shell with (




1f 

) represents a circular
cylindrical shell of constant thickness. The substitution
of Equation (14) into Equation (12) results the frequency
equations



21 23 25 27
41 43 45 47
61 63 65 67
81 83 85 870
0
s
s
YYYYU
YYYYW
YYYYM
YYYYN



















for symmetrical vibration, (16)

M. K. AHMED 334
 
12 14 16 18
32 34 36 38
52 45 56 58
72 74 76 780
0
s
s
sx
YYYY V
YYYY
YYYY S
YYYY N




















for antisymmetrical vibration. (17)
The matrix [V] depends on the frequency parameter

and the load factor Pin addition to

,

Y



is also
a function of these parameters. Equations (16) and (17)
give a set of linear homogenous equations with unknown
coefficients


0
T
ss
U,W,M ,N
  and


0
T
ss sx
V,,S,N 
 
,
respectively, at 0

. For the existence of a nontrivial
solution of these coefficients, the determinant of the co-
efficient matrix should be vanished. The standard proce-
dures cannot be employed for obtaining the eigenvalues
of the frequency equations. The nontrivial solution is
found by searching the values

which make the deter-
minant zero by using Lagrange interpolation procedure.
The eigenvalues of

which make the determinant zero
give the natural frequencies of the shell. The mode sha-
pes at any point of the cross-section of the shell, over the
length 2 L, are determined by calculating the eigenvec-
tors corresponding to the eigenvalues by using Gaussian
elimination procedure. For free vibration problems with-
out prestress the natural frequencies result when 0P

,
and for vibration problems with prestress the natural
frequencies result for non-zero values of P. For buck-
ling problems, the buckling load results when 0


in
Equations (16) and (17), and the lowest values of the
buckling loads give the critical loads of the shell.
5. Numerical Results and Discussion
A computer program based on the analysis described
herein has been developed to study the symmetrical and
antisymmetrical vibrations of the considered shell, and
the vibration frequencies and the critical buckling loads
of the shell with circumferential variable thickness, are
calculated numerically. Results for uniform thickness of
circular cylindrical shells (1

and 1

) are obtained.
The correctness of applied method is cited in [31] with
other literature. Our study is divided into two parts:
5.1. Vibrations
The study of vibration s is determined by finding the nat-
ural frequencies

and corresponding mode shapes at
0P

. The numerical results presented herein pertain to
the minimum frequencies and the associated mode shapes
of the shell.
The effect of variation in thickness on the minimum
frequencies of vibration, Tables 1 and 2 give the funda-
Table 1. The fundamental frequencies λ for symmetric modes of a three-lobed cross-section cylindrical shell with variable
thickness, for which (=1,= 0.3,= 4,=0.02mνlh ).


1 2 3 4 5
0.0 0.019568 0.028312 0.035171 0.041386 0.047378
0.2 0.019736 0.027475 0.034116 0.040315 0.046313
0.4 0.029985 0.035825 0.040712 0.045741 0.051032
0.6 0.039787 0.050925 0.056946 0.061702 0.066208
0.8 0.053618 0.068977 0.079662 0.086458 0.091184
1.0 0.074135 0.087694 0.100293 0.110095 0.115710
Table 2. The fundamental frequenc ies λ for antisymmetric modes of a three-lobed cross-section cylindrical shell with vari-
able thickness, for which (=1,=0.3,= 4,=0.02mνlh ).


1 2 3 4 5
0.0 0.019568 0.026504 0.033007 0.039530 0.046143
0.2 0.019736 0.026235 0.032464 0.038710 0.045019
0.4 0.029985 0.036643 0.042989 0.049309 0.055683
0.6 0.045266 0.054520 0.062245 0.069595 0.076761
0.8 0.070167 0.082516 0.091719 0.100270 0.108014
1.0 0.074135 0.087694 0.100293 0.110095 0.115710

M. K. AHMED 335
mental frequencies for symmetric and antisymmetric vi-
brations of a three-lobed cross-section cylindrical shell,
with the same circumferential length, versus the radius
ratio

for different values of

. The results presen-
ted in these tables show that the increase of the thickness
ratio resulted in an increase in the fundamental frequen-
cies for each value of the radius ratio. These results con-
firm the fact that the effect of increasing the shell flexur-
al rigidity becomes larger than that of increasing the shell
mass when the thickness ratio in creases. Also, the incr-
ease of the radius ratio for the chosen values of the thick-
ness ratio results in an increase in the frequencies

and they become larger at 1


(circular cylinder). In
case of a constant thickness (1

), the symmetric and
antisymmetric type vibrations have the same values ver-
sus the radius ratio, except for the symmetric and antisy-
mmetric ones with respect to three planes passing through
a corner and an axial mid-line of the opposite wall give
unidentical values. For a circular cylindrical shell (1


),
the symmetric and antisymmetric type vibrations give the
same values versus the thickness ratio. In Tables 3 and 4,
the first five frequencies

of symmetric and antisym-
metric type vibrations are presented for different values
of radius ratios (05,07,10...


) and thickness ratios
(1,2,5


). The numbers in the parentheses are the axi-
al half wave numbers of the mode in the
x
-direction.
An ordering change of the mode in the
x
-direction can
be seen for certain values of

and

.
In Table 5, the comparison of the fundamental fre-
quencies

for symmetric and antisymmetric vibrations
of a shell with radius ratio (05.

) is cited for different
axial half wave numbers and thickness ratio. With an in-
crease of axial half wave number aspect the thickness
ratio, the fundamental frequencies increase. It is shown by
this table that the values of fundamental frequencies

for symmetric and antisymmetric modes are very close to
each other for the large mode number, m.
Figures (2-4) show the first five circumferential mode
shapes of a three-lobed cross-section cylindrical shell of
variable thickness for symmetric and antisymmetric vi-
bration modes corresponding to the frequencies

listed
in Tables 3 and 4. The thick lines show the composi tion
of the circumferential and transverse deflections, and the
numbers in the parentheses are the axial half wave num-
Table 3. The first five frequencies λ for symmetric modes of the shell, with (=0.3,=4,=0.02νlh ).
Modes


First Second Third Forth Fifth
1 0.036513(1) 0.037069(1) 0.046870(2) 0.052419(2) 0.065142(3)
[15]0.03653 0.03716 0.04691 0.05248 -
0.5 2 0.043201(1) 0.056216(1) 0.061026(2) 0.082699(3) 0.086711(2)
5 0.057253(1) 0.092093(2) 0.100173(1) 0.125091(3) 0.162623(2)
0.7 2 0.059096(1) 0.075956(1) 0.086524(2) 0.116652(3) 0.121253(2)
5 0.077638(1) 0.116732(1) 0.125442(2) 0.170122(3) 0.200812(1)
1.0 2 0.087641(1) 0.114102(1) 0.130261(1) 0.177653(2) 0.201403(1)
5 0.114922(1) 0.133672(1) 0.210313(2) 0.218261(1) 0.246320(1)
Table 4. The first five frequencies λ for antisymmetric modes of the shell, with (=0.3,=4,=0.02νlh ).
Modes


First Second Third Forth Fifth
1 0.037069(1) 0.052419(2) 0.064586(1) 0.068488(3)0.089686(1)
[15]0.03716 0.05240 0.06470 - 0.8982
0.5 2 0.044870(1) 0.066191(2) 0.080338(1) 0.086261(3)0.124450(1)
5 0.065098(1) 0.099283(2) 0.121120(1) 0.131662(3)0.194172(1)
0.7 2 0.066803(1) 0.092671(2) 0.093671(1) 0.117432(3)0.139351(1)
5 0.091152(1) 0.131372(1) 0.136280(2) 0.172388(3)0.201140(1)
1.0 2 0.087659(1) 0.114122(1) 0.130263(1) 0.177656(2)0.201405(1)
5 0.115203(1) 0.133701(1) 0.210395(2) 0.218264(1)0.246371(1)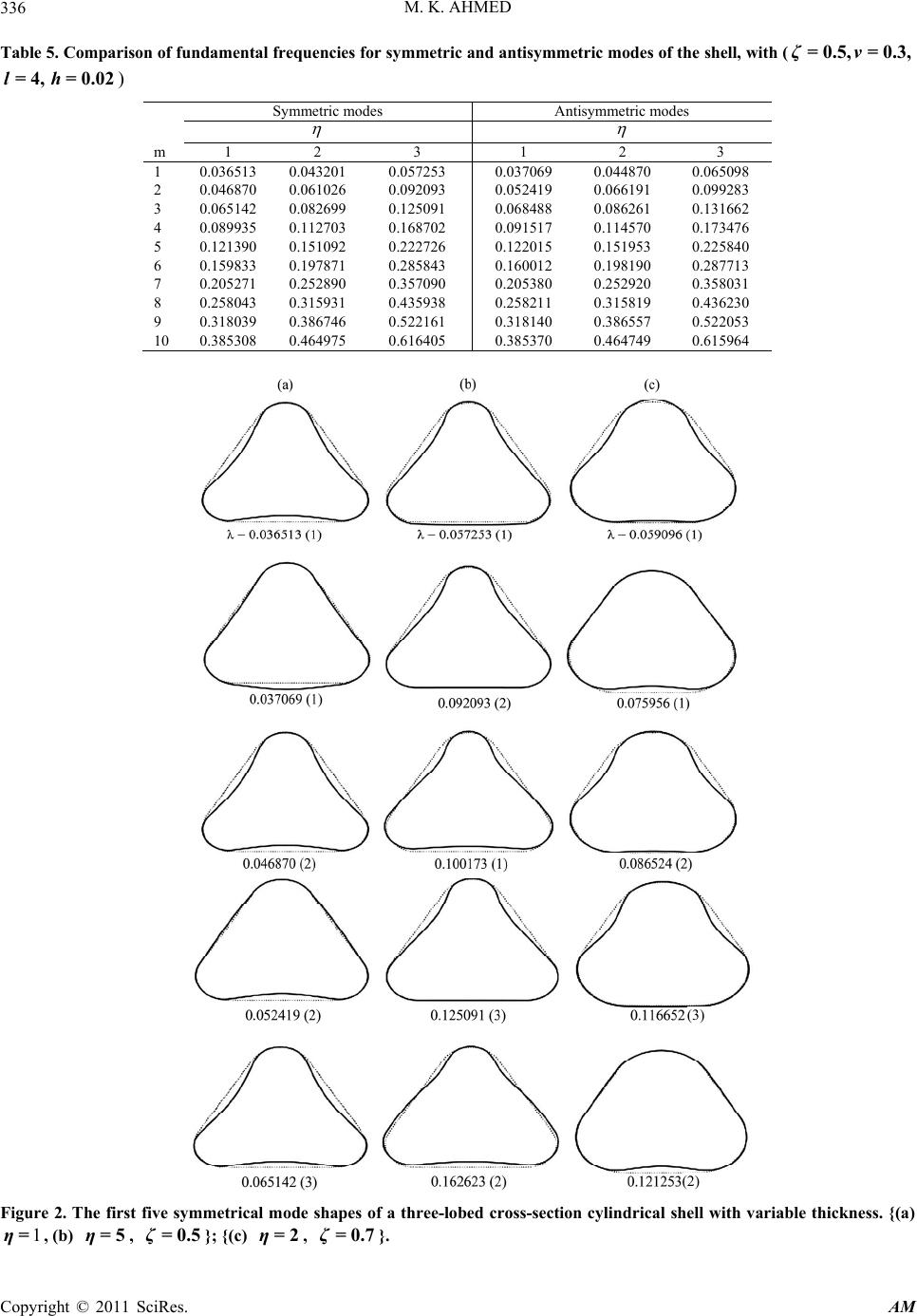

M. K. AHMED 336
Table 5. Comparison of f undamental fr equencie s for symmetric and anti symmetric modes of the shell, with (0.5,=0.3,ζ=ν
= 4,= 0.02lh )
Symmetric modes Antisymmetric modes


m 1 2 3 1 2 3
1 0.036513 0.043201 0.057253 0.037069 0.044870 0.065098
2 0.046870 0.061026 0.092093 0.052419 0.066191 0.099283
3 0.065142 0.082699 0.125091 0.068488 0.086261 0.131662
4 0.089935 0.112703 0.168702 0.091517 0.114570 0.173476
5 0.121390 0.151092 0.222726 0.122015 0.151953 0.225840
6 0.159833 0.197871 0.285843 0.160012 0.198190 0.287713
7 0.205271 0.252890 0.357090 0.205380 0.252920 0.358031
8 0.258043 0.315931 0.435938 0.258211 0.315819 0.436230
9 0.318039 0.386746 0.522161 0.318140 0.386557 0.522053
10 0.385308 0.464975 0.616405 0.385370 0.464749 0.615964
Figure 2. The first five symmetrical mode shapes of a three-lobed cross-section cylindrical shell with variable thickness. {(a)
1η=, (b) 5η=, 0.5ζ=}; {(c) 2η=, 0.7ζ=}.
(3)
(2)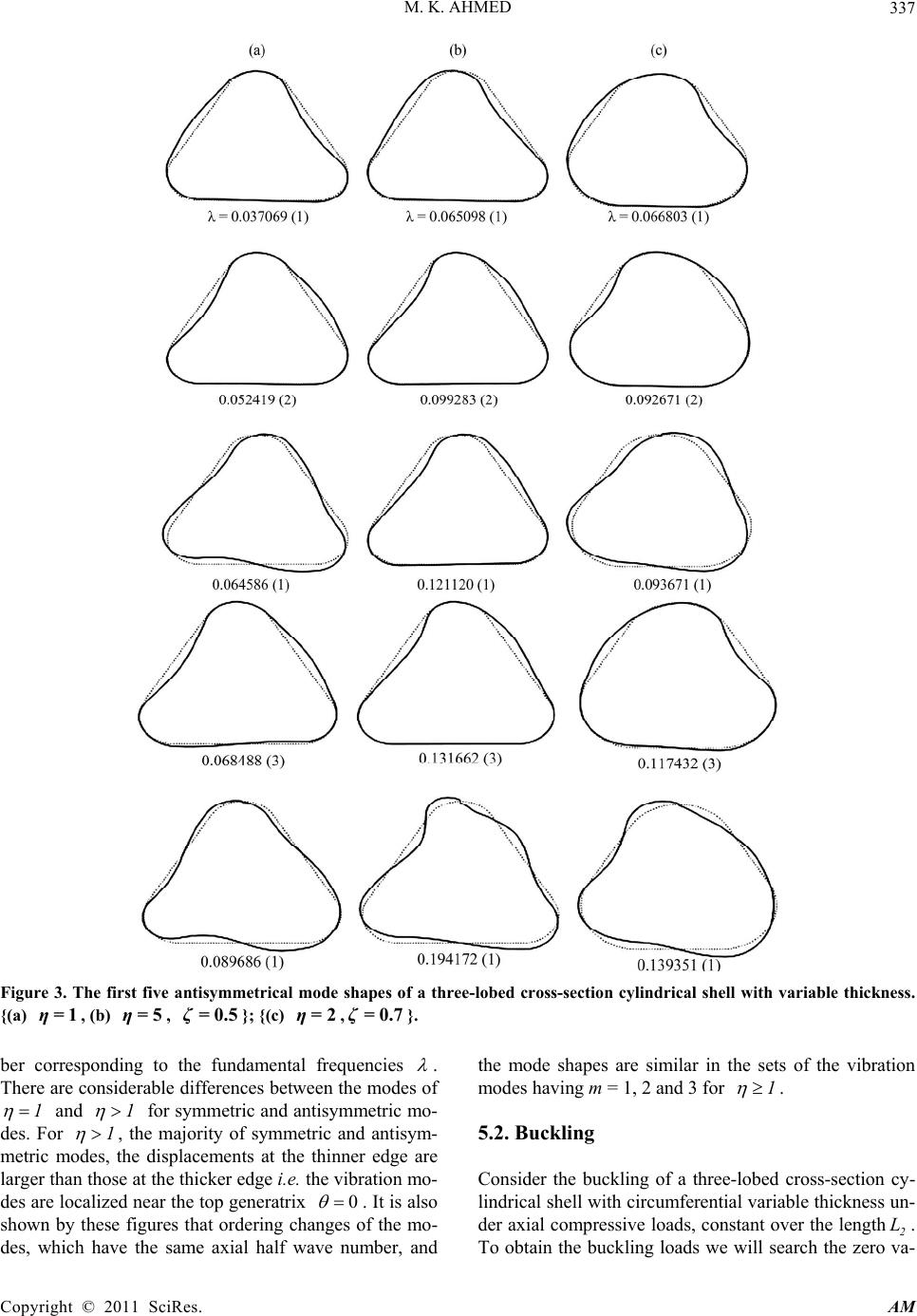

M. K. AHMED 337
Figure 3. The first five antisymmetrical mode shapes of a three-lobed cross-section cylindrical shell with variable thickness.
{(a) 1η=, (b) 5η=, 0.5ζ=}; {(c) 2η=,0.7ζ=}.
ber corresponding to the fundamental frequencies

.
There are considerable differences between the modes of
1

 and 1

 for symmetric and antisymmetric mo-
des. For 1

, the majority of symmetric and antisym-
metric modes, the displacements at the thinner edge are
larger than those at the thicker edge i.e. the vibration mo-
des are localized near the top generatrix 0

. It is also
shown by these figures that ordering changes of the mo-
des, which have the same axial half wave number, and
the mode shapes are similar in the sets of the vibration
modes having m = 1, 2 and 3 for 1

.
5.2. Buckling
Consider the buckling of a three-lobed cross-section cy-
lindrical shell with circumferential variable thickness un-
der axial compressive loads, constant over the length2 L.
To obtain the buckling loads we will search the zero va-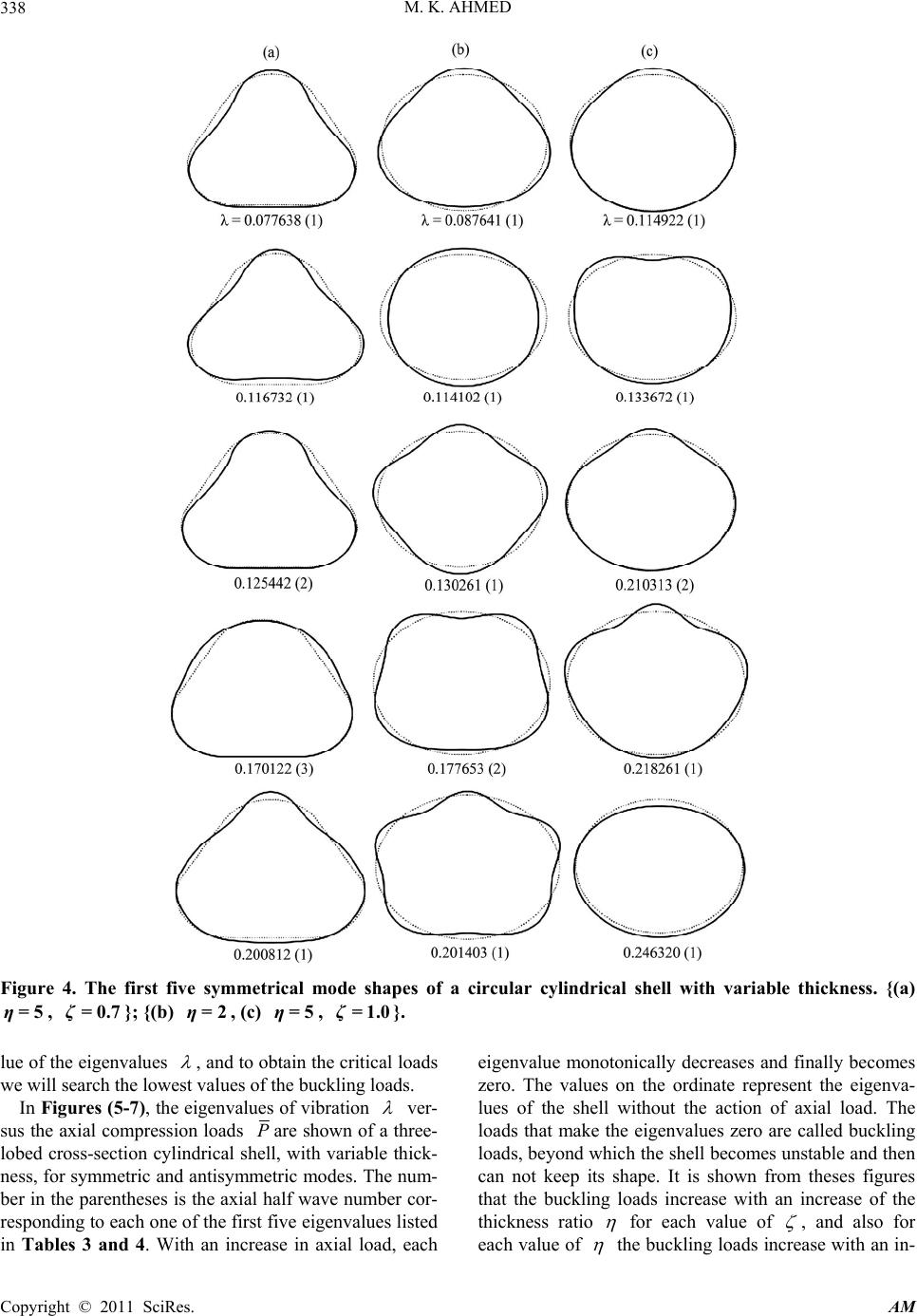

M. K. AHMED 338
Figure 4. The first five symmetrical mode shapes of a circular cylindrical shell with variable thickness. {(a)
5η=, 0.7ζ=}; {(b) 2η=, (c) 5η=, 1.0ζ=}.
lue of the eigenvalues

, and to obtain the critical loads
we will search the lowest values of the buckling loads.
In Figures (5-7 ), the eigenvalues of vibration

ver-
sus the axial compression loads Pare shown of a three-
lobed cross-section cylindrical shell, with variable thick-
ness, for symmetric and antisy mmetr ic mo de s. Th e nu m-
ber in the parentheses is the axial half wave number cor-
responding to each one of the first five eigenvalues listed
in Tables 3 and 4. With an increase in axial load, each
eigenvalue monotonically decreases and finally becomes
zero. The values on the ordinate represent the eigenva-
lues of the shell without the action of axial load. The
loads that make the eigenvalues zero are called buckling
loads, beyond which the shell beco mes unstable and then
can not keep its shape. It is shown from theses figures
that the buckling loads increase with an increase of the
thickness ratio

for each value of

, and also for
each value of

the buckling loads increase with an in-

M. K. AHMED 339
crease of

, and for 1

 they become larger. The
critical loads for symmetric and antisymmetric vibrations
are occurred with 3m.
Figure 7 shows the eigenvalues of vibration

ver-
sus the axial compression loads for a circular cylindrical
shell with circumferential variable thickness. In Figure
7(a), the plotted eigencurve gives the critical buckling
load of a circular cylindrical shell of a constant thickness,
(266 579P.), and good agreement result with [14], and
it is occurred with 1m. While the critical buckling
loads of a circular cylindrical shell having variable thick-
ness are occurred with 2m.
Figure 8 shows the buckling loads versus the axial
half wave number of a shell with radius ratio (05.


)
for symmetric and antisymmetric modes. It is shown from
this figure, symmetric case gives lower buckling loads
than antisymmetric one, and the buckling loads are very
close to each other for the large mode number m. Also,
with an increase of axial half wave number, the buckling
loads increase after once decreasing. For each value of

, the critical buckling loads of the shell are occurred
with 3m

for the symmetric and antisymmetric mod-
es.
6. Conclusions
An approximate analysis for studying the free vibration
and buckling of a three-lobed cross-section cylindrical
shell having circumferential varying thickness under
axial membrane loads is presented. The numerical results
presented herein pertain to the fundamental frequencies
and buckling loads as well as the associated mode shapes
Figure 5. Eigenvalues of vibration versus axial load of a three-lobed cross-section cylindrical shell with variable
thickness, symmetric case. (= 0.3,= 0.02νh).

M. K. AHMED 340
Figure 6. Eigenvalues of vibration versus axial load of a three-lobed cross-section cylindrical shell with variable
thickness, antisymmetric case. (=0.3, =0.02νh).
Figure 7. Eigenvalues of vibration versus axial compression load of a circular cylindrical shell with circumferen-
tial vari abl e thickness. (=0.3, =0.02νh).

M. K. AHMED 341
Figure 8. Buckling loads versus axial half wave number of a
three-lobed cross-section cylindrical shell with variable thi-
ckness. (=0.3,= 0.02νh).
by using the transfer matrix method. The method is
based on thin shell theory and applied to a shell of sym-
metric and antisymmetric vibration modes, and the anal-
ysis is formulated to overcome the mathematical difficul-
ties associated with mode coupling caused by variable
shell wall curvature and thickness. The first five funda-
mental frequencies and mode shapes as well as critical
buckling loads have been presented, and the effects of
the thickness ratio of the cross-section on the natural fre-
quencies, mode shapes, and buckling loads were exa-
mined. For the thickness ratio 1

, the vibration mod-
es are distributed regularly over the shell surface, but for
1

 the modes are localized near the weakest genera-
trix 0

, (thinner edge), in most cases of the vibration
modes. However, the critical buckling loads increase
with either increasing radius ratio or increasing thickness
ratio and become larger for a circular cylindrical shell.
For the cylindrical shell of (1


and 1

), the criti-
cal loads are occurred with 2m

, but for the shell un-
der consideration of (1

 and 1

) they occurred
with 3m for the symmetric and antisymmetric vibra-
tion modes.
7. Acknowledgments
The author is grateful to anonymous reviewers for their
good efforts and valuable comments which helped impr-
ove the quality of this paper.
8. References
[1] W. Flügge, “Die Stabilität der Kreiszylinderschale,” In-
genieur Archiv, Vol. 3, No. 5, 1932, pp. 463-506.
[2] L. H. Donnell, “Stability of Thin-Walled Tubes under
Torsion,” NACA Report, No. NACA TR-479, 1933.
[3] H. Becker and G. Gerard, “Elastic Stability of Orthotrop-
ic Shells,” Journal of Aerospace Science, Vol. 29, No. 5,
1962, pp. 505-512.
[4] G. Gerard, “Compressive Stability of Orthotropic Cy-
linders,” Journal of Aerospace Science, Vol. 29, 1962,
pp. 1171-1179.
[5] S. Cheng and B. Ho, “Stability of Heterogeneous Aeolo-
tropic Cylindrical Shells under Combined Loading,”
AIAA Journal, Vol. 1, No. 40, 1963, pp. 892-898.
[6] R. M. Jones, “Buckling of Circular Cylindrical Shells
with Multiple Orthotropic Layers and Eccentric Stiffen-
ers,” AIAA Journal, Vol. 6, No. 12, 1968, pp. 2301-2305.
doi:10.2514/3.4986
[7] Y. Stavsky and S. Friedl, “Stability of Heterogeneous
Orthotropic Cylindrical Shells in Axial Compression,”
Israel Journal of Technology, Vol. 7, 1969, pp. 111-119.
[8] M. M. Lei and S. Cheng, “Buckling of Composite and
Homogeneous Isotropic Cylindrical Shells under Axial
and Radial Loading,” Journal of Applied Mechanics, Vol.
36, No. 4, 1969, pp. 791-798.
[9] J. B. Greenberg and Y. Stavsky, “Buckling and Vibration
of Orthotropic Composite Cylindrical Shells,” Acta Me-
chanica, Vol. 36, No. 12, 1980, pp. 15-29.
doi:10.1007/BF01178233
[10] J. B. Greenberg and Y. Stavsky, “Vibrations of Axially
Compressed Laminated Orthotropic Cylindrical Shells,
Including Transverse Shear Deformation,” Acta Mecha-
nica, Vol. 37, No. 1-2, 1980, pp. 13-28.
doi:10.1007/BF01441240
[11] J. B. Greenberg and Y. Stavsky, “Stability and Vibrations
of Compressed Aeolotropic Composite Cylindrical
Shells,” Journal of Applied Mechanics, Vol. 49, No. 4,
1982, pp. 843-848. doi:10.1115/1.3162625
[12] J. B. Greenberg and Y. Stavsky, “Vibrations and Buck-
ling of Composite Orthotropic Cylindrical Shells with
Nonuniform Axial Loads,” Composites Part B: Engi-
neering, Vol. 29, No. 6, 1998, pp. 695-702.
doi:10.1016/S1359-8368(98)00029-8
[13] A. Rosen and J. Singer, “Vibrations of Axially Loaded
Stiffened Cylindrical Shells,” Journal of Sound and Vi-
bration, Vol. 34, No. 3, 1974, pp. 357-378.
doi:10.1016/S0022-460X(74)80317-2
[14] G. Yamada, T. Irie and M. Tsushima, “Vibration and
Stability of Orthotropic Circular Cylindrical Shells Sub-
jected to Axial Load,” Journal of Acoustical Society of
America, Vol. 75, No. 3, 1984, pp. 842-848.
doi:10.1121/1.390594
[15] G. Yamada, T. Irie and Y. Tagawa, “Free Vibration of
Non-Circular Cylindrical Shells with Variable Circumfe-
rential Profile,” Journal of Sound and Vibration, Vol. 95,
No. 1, 1984, pp. 117-126.
doi:10.1016/0022-460X(84)90264-5
[16] K. Suzuki and A. W. Leissa, “Free Vibrations of Noncir-
cular Cylindrical Shells Having Circumferentially Vary-
ing Thickness,” Journal of Applied Mechanics, Vol. 52,

M. K. AHMED 342
No. 1, 1985, pp. 149-154. doi:10.1115/1.3168986
[17] V. Kumar and A. V. Singh, “Approximate Vibrational
Analysis of Noncircular Cylinders Having Varying Thi-
ckness,” AIAA Journal, Vol. 30, No. 7, 1991, pp. 1929-
1931. doi:10.2514/3.11161
[18] O. Mitao, T. Hideki and S. Tsunemi, “Vibration Analysis
of Curved Panels with Variable Thickness,” Engineering
Computations, Vol. 13, No. 2, 1996, pp. 226-239.
doi:10.1108/02644409610114549
[19] R. F. Tonin and D. A. Bies, “Free Vibration of Circular
Cylinders of Variable Thickness,” Journal of Sound and
Vibration, Vol. 62, No. 2, 1979, pp. 165-180.
doi:10.1016/0022-460X(79)90019-1
[20] R. M. Bergman, S. A. Sidorin and P. E. Tovstik, “Con-
struction of Solutions of the Equations for Free Vibration
of a Cylindrical Shell of Variable Thickness along the
Directrix,” Mechanics of Solids, Vol. 14, No. 4, 1979, pp.
127-134.
[21] T. Irie, G. Yamada and Y. Kaneko, “Free Vibration of a
Conical Shell with Variable Thickness,” Journal of Sound
and Vibration, Vol. 82, No. 1, 1982, pp. 83-94.
doi:10.1016/0022-460X(82)90544-2
[22] S. Takahashi, K. Suzuki and T. Kosawada, “Vibrations of
Conical Shells with Varying Thickness,” Japan Society of
Mechanical Engineering, Vol. 28, No. 235, 1985, pp.
117-123.
[23] W. I. Koiter, I. Elishakoff, Y. W. Li and J. H. Starness,
“Buckling of an Axially Compressed Cylindrical Shell of
Variable Thickness,” International Journal of Solids and
Structures, Vol. 31, No. 6, 1994, pp. 797-805.
doi:10.1016/0020-7683(94)90078-7
[24] H. Abdullah and H. Erdem, “The Stability of Non-Ho-
mogenous Elastic Cylindrical Thin Shells with Variable
Thickness under a Dynamic External Pressure,” Turkish
Journal of Engineering and Environmental Sciences, In
Turkish, Vol. 26, No. 2, 2002, pp. 155-164.
[25] S. L. Eliseeva and S. B. Filippov, “Buckling and Vibra-
tions of Cylindrical Shell of Variable Thickness with
Slanted Edge,” Vestnik Sankt-Peterskogo Universiteta, In
Russian, No. 3, 2003, pp. 84-91.
[26] S. B. Filippov, D. N. Ivanov and N. V. Naumova, “Free
Vibrations and Buckling of a Thin Cylindrical Shell of
Variable Thickness with Curelinear Edge,” Technische
Mechanik, Vol. 25, No. 1, 2005, pp. 1-8.
[27] A. L. Goldenveizer, “Theory of Thin Shells,” Pergamon
Press, New York, 1961.
[28] V. V. Novozhilov, “The Theory of Thin Elastic Shells,” P.
Noordhoff Ltd., Groningen, 1964.
[29] R. Uhrig, “Elastostatik und Elastokinetik in Matrizen-
schreibweise,” Springer-Verlag, Berlin, 1973.
[30] A. Tesar and L. Fillo, “Transfer Matrix Method,” Kluwer
Academic, Dordrecht, 1988.
[31] M. Khalifa, “A Study of Free Vibration of a Circumfe-
rentially Non-Uniform Cylindrical Shell with a Four
Lobed Cross Section,” In Press, Journal of Vibration and
Control, 2010.