Mathematical Reasoning of Treatment Principle Based on “Yin Yang Wu Xing” Theory in Traditional Chinese Medicine

doi:10.4236/cm.2011.21002

Paper Menu >>

Journal Menu >>

Chinese Medicine, 2011, 2, 6-15

doi:10.4236/cm.2011.21002 Published Online March 2011 (http://www.SciRP.org/journal/cm)

Mathematical Reasoning of Treatment Principle

Based on “Yin Ya ng Wu Xing” Theory in Traditional

Chinese Medicine

Yingshan Zhang

School of Finance and Statistics, East China Normal University, Shanghai, China

E-mail: ysh_zhang@163.com

Received December 21, 201 0; revised January 20, 2011; accepted January 28, 2011

Abstract

By using mathematical reasoning, this paper demonstrates the treatment principle: “Virtual disease is to fill

his mother but real disease is to rush down his son” and “Strong inhibition of the same time, support the

weak” based on “Yin Yang Wu Xing” Theory in Traditional Chinese Medicine (TCM). We defined two kinds

of opposite relations and one kind of equivalence relation, introduced the concept of steady multilateral sys-

tems with two non-compatibility relations, and discussed its energy properties. Later based on the treatment

of TCM and treated the healthy body as a steady multilateral system, it has been proved that the treatment

principle is true. The kernel of this paper is the existence and reasoning of the non-compatibility relations in

steady multilater al systems, and it accords with the oriental thinking model.

Keywords: Traditional Chinese Medicine, “Yin Yang W u Xing” Theory, Steady Multilateral Systems,

Opposite Relations, Side Effects, Medical and Drug Resistance Problem

1. Main Differences between Traditional

Chinese Medicine and Western

Medicine

Western medicine treats disease from Microscopic point

of view, always destroys the original human being’s

balance, and has none beneficial to human’s immunity.

Western medicine can produce pollution to human’s

body, having strong s ide ef fects. E xcessi vely usi ng med-

icine can easily paralysis the human’s immunity, which

AIDS is a unique product of Western medicine. Using

medicine too little can easily produce the medical and

drug resistance problem.

Traditional Chinese Medicine (TCM) studies the

world from the macroscopic point of vie w, a nd its tar get

is in order to maintain the original balance of human

being and in order to enhance the immunity. TCM be-

lieves that each medicine has one-third of drug. She ne v-

er encourages patients to use medicine in long term. Tra-

ditional Chinese Medicine has over 5,000-year history. It

almos t has none side effects or medical and drug resis-

tance problem [19].

After long period of practicing, Chinese ancient med-

ical scientists use “Yin Yang Wu Xing” Theory exten-

sively in the tra ditional treat ment to expl ain t he ori gin o f

life, human body, pathological changes, clinical diagno-

sis and prevent i on. It has become an important part of t he

Traditional Chinese Medicine. “Yin Yang Wu Xing”

Theory has a strong influence to the formation and de-

velopment of Chinese medicine theory. But, many Chi-

nese and foreign scholars still have some questions on

the reasoning of Tra ditional Chinese Medic ine.

Zhang’s theory, multilateral matrix theory [1] and

multilateral system theory [13,14,19], have given a new

and strong mathematical reasoning method from macro

(Global) analysis to micro (Local) analysis. He and his

colleagues have made some mathematical models and

methods of reasoning [2-17], whic h make the mathemat-

ical reasoning of T CM possible [13] based o n “Yin Yang

Wu Xing” Theory [18]. T his paper will use stead y multi-

lateral systems to demonstrate the treatment principle of

TCM : “Real disease is to rush down his son but virtual

disease is to fill his mother ” and “Str o ng i nhi b ition of the

same time, support the weak”.

The article proceeds as follows. Section 2 contains ba-

sic concepts and main theorems of steady multilateral

systems while the treatment principle of Traditional

Chinese Medicine is demonstrated in Section 3 and 4.

Y. S. ZHANG

Conclusions are drawn in S ect ion 5.

2. Basic Concept of Steady Multilateral

Systems

In the real world, we are enlightened from some concepts

and phenomena such as “biosphere”, “food chain”,

“ecological balance” etc. With research and practice, by

using the theory of multilatera l matrices[1] and analyzing

the conditions of s ymmetr y [1-2] and orthogonality [3-5,

12,17] what a stable system must satisfy, in particular,

with analyzing the basic conditions what a stable work-

ing procedure of good product quality must satisfy [11,

17], we are inspired and find some rules and methods,

then present the logic model of analyzing stability of

complex systems [7-10]—steady multilateral systems

[13,14,19]. There are a number of essential reasoning

met hods based on the stable logic analysis model, such

as “transition reasoning”, “atavism reasoning”, “genetic

reasoning” etc.

2.1. Equivalence Relations

Let V be a nonempt y se t a nd x, y, z be their elements. We

call it an equivalence relation, denoted ~, if the follo wing

3 conditions are all true:

1) Reflexive: x ~ x for all x;

2) Symmetric: if x ~ y, then y ~ x;

3) Conveyabl e (Transitivity): if x ~ y, y ~ z, then x ~ z.

If there are some x, y, z such that at least one of the

conditions abo ve is true, the relation is called a compati-

bility relation. Any one of compatibility relations can be

expa nded into an equivalence relation [19].

Western Science only considers the reasoning under

one Axio m sys te m such t hat o nl y rese arc hes on compati-

bility rela tion reaso ning. However, there are many Axio m

syste ms in Nat ure. T radit ional Chinese Science (TCS, or

Oriental Science) mainly researches the reasoning among

many Axio m s yste ms in Nature. Of course, she also con-

siders the reasoning under one Axiom system but she

only expands the reasoning as the equivalence relation

reasoning [19].

2.2. Two Kinds of Opposite Relations

Equivalence relations, even compatibility relations, can-

not portray the structure of the complex systems clearly.

For example, assume that A and B are good friends and

they ha ve clo se relat ions. S o are B and C. However, you

canno t get t he conclusion that A and C are good friends.

We denote A → B as that A and B have close relations.

Then the example above can be d enoted as: A → B, B →

C do not imply A → C, i.e., the relation → is a non-

conveyable (or non-transitivity) relation, of course, a

non-equivalence relation.

In the following, we consider two non-compatibility

relations.

Let V be a nonempty set and x, y, z be none equal.

There are two kinds of opposite relations, called neigh-

boring relations → and alternate relations ⇒, having the

property:

1) If x → y, y → z, then x ⇒ z;

⇔ if x → y, x ⇒ z, then y → z;

⇔ if x ⇒ z, y → z, then x → y.

2) If x ⇒ y, y ⇒ z, then z → x;

⇔ if z → x, x ⇒ y, then y ⇒ z;

⇔ if y ⇒ z, z→ x, then x ⇒ y.

Two kinds of opposite relations cannot be exist sepa-

rately.

Such reasoning can be expressed as follows:

z y

⇓

←



y z

⇓

⇒



The first triangle reasoning is known as a jumping-

transition reasoning, while the second triangle reasoning

is known as an atavism reasoning. Both neighboring re-

lations and alternate relations are not compatibility rela-

tions, of course, none equivalence relations, called

non-compatibility relations.

2.3. Genetic Reasoning

Let V be a nonempty set and x, y, z be not equal one

another. If equivalence relations exist, neighboring rela-

tions, a nd alterna te r elation s i n V at the same time, then a

genetic reasoning i s defined as follo ws:

1) if x ~ y, y → z, then x → z;

2) if x ~ y, y ⇒ z, then x ⇒ z;

3) if x → y, y ~ z, then x → z;

4) if x ⇒ y, y ~ z, the n x ⇒ z.

2.4. Multilateral Systems

For a nonempty set V, if there exists at least an non-

compatibilit y relatio n, then i t is called a multilateral sys-

tem about complexit y [13,14,19], or eq uival ent ly, a l ogi c

analysis model of complex systems [7-10].

Assu me that t he r e e xist e q ui vale nc e r e la t ions, ne i g hbo r-

ing relations, and alternate relations in system V, which

satisfy genetic reasoning. If for every x, y ∈ V, at least

there is one of the three relations between x and y, and

there are not x → y and x ⇒ y at the same time, then V is

called a lo gic analysis model o f complex syste ms, which

is equivalent to the logic architecture of reasoning model

of “Yin Yang” Theory in Ancient China. Obviously, V is

Y. S. ZHANG

a multilateral system with two non-compatibility rela-

tions. In this paper, we only consider this multilateral

s ys t em.

Theorem 2.1 For a multilateral system V with two

non-compatibility relations, ∀ x, y ∈ V, only one of the

following fiv e re lations is existent and correct:

x ~ y, x → y, x ← y, x ⇒ y, x ⇐ y.

Theorem 2.2 For a multilateral system V with two

non-compatibility relations, ∀ x, y, z ∈ V, the following

reasoning holds:

1) if x → z , y → z, then x ~ y;

2) if x ⇒ z, y ⇒ z, then x ~ y;

3) if x → y , x→ z, then y ~ z;

4) if x ⇒ y, x ⇒ z, then y ~ z.

2.5. Steady Multilateral Systems

The multilateral system V is known as a steady multila-

teral system (or, a stable multilateral system) with two

non-compatibility relations if there exists at least the

chain x1,



, xn ∈ V, which satisfy any one of the two

conditions below:

x1→ x2 →



→ xn → x1;

x1 ⇒ x2 ⇒



⇒ xn ⇒ x1.

Theorem 2.3 For a steady multilateral system V with

two non-compatibility relations, there exists five-length

chain, and the length of the chain is inte ger times of 5.

Theorem 2.4 For a steady multilateral system V with

two non-compatibility relations, there exists a partition

of V as follows:

V = V1 + V2 + V3 + V4 + V5;

{ }

,1, 5.

Vy Vyxi= ∈∀=

which x1, x2, x3, x4, x5 is a chain. The notation that V = V1

+ V2 + V3 + V4 + V5 means that V = V1 ∪ V2 ∪ V3 ∪ V4

∪ V5, Vi ∩ Vj = ∅,

∀

i ≠ j.

Theorem 2.5 The decomposition above for the steady

multilateral system with two non-compatibility relations,

there exist relatio ns below Figure 1.

Theorem 2.6 For each element x in a steady multila-

teral system V with two non-compatibility relations,

there exist five equ ivalen ce classes below:

{ }

,Xy Vyx= ∈

{ }

Xy Vxy=∈→

{ }

Xy Vxy=∈⇒

{ }

Ky Vyx=∈⇒

{ }

Sy Vyx=∈→

which the five equivalence classes have relations below

Figure 2.

3. Relationship Analysis of Steady

Multilateral Systems

3.1. Energy of a Multilateral System

Energy concept is an important concept in Physics. Now,

we intr oduc e this concep t to the multilate ral syste ms and

use these concepts to deal with the multilateral system

disea ses.

In mathematics, a multilateral system is said to have

energy (or dyn a m i c ) if there is a none negative function

φ( *) which makes every subsystem meaningful of the

multilateral system.

For two subsystems Vi and Vj of multilatera l system V,

denote Vi → Vj (or Vi ⇒ Vj, or Vi ~ Vj) me ans xi → xj, xi

∈

Vi, xj

∈

Vj (or xi ⇒ xj, xi

∈

Vi, xj

∈

Vj or xi ~ xj, xi

∈

Vi,

∈

Vj).

For sub s ys te ms Vi, Vj and Vi ∪ Vj where Vi ∩ Vj = ∅, i

≠ j, let φ(Vi) = |Vi |, φ(Vj) = |Vj | and φ(Vi ∪ Vj) = |Vi ∪ Vj|,

where φ(Vi ∪ Vj) is the total energy of both Vi and Vj.

For an equivalence relation Vi ~ Vj, if |Vi ∪ Vj | = |Vi | +

|Vj | (the normal state of the energy of Vi ~ Vj), then the

equivalence relation Vi ~ Vj is called that Vi likes Vj

whic h mea ns that Vi is similar to Vj. In this case, the Vi is

also called the brother of Vj while the Vj is also called the

brother of Vi. In the causal model, the Vi is called the

similar family member of Vj while the Vj is also called

the similar family me mber of Vi. There are not any causal

relation considered between Vi and Vj.

For a neig hbo ring r elat ion Vi → Vj, if |Vi ∪ Vj| > |Vi| +

|Vj| (the normal state of the energy of Vi → Vj), then the

neighboring relation Vi → Vj is called that Vi bears (or

loves) Vj [or that Vj is born (or loved) by Vi]whic h mea ns

that Vi is be nefic ial on Vj each other. In this case, the Vi is

called the mother of Vj while the Vj is calle d the son o f Vi.

In the causal model, the Vi is called the beneficial cause

of Vj while the Vj is called the beneficial effect of Vi.

For an alternate relation Vi ⇒ Vj, if |Vi ∪ Vj| < |Vi| +

|Vj| (the normal state of the energy of Vi ⇒ Vj), then the

alternate relation Vi ⇒ Vj is called as that Vi kills Vj (or

that Vj is killed by Vi) which means that Vi is harmful o n

Vj each othe r. In this case, the Vi is called the bane of Vj

while the Vj is called the prisoner of Vi. In the causal

model, the Vi is called the harmful cause of Vj while the

Vj is called the harmful effect of Vi.

In the future, unless stated otherwise, any equivalence

relation is the liking relation, any neighboring relation is

the bear ing relation (or the loving relation), and any al-

ternate relatio n is the killing re la tion.

Suppose V is a steady multilateral system having

energ y, t he n during no rmal opera tion, its ener gy function

for any subsystem of the multilateral system has an av-

erage (or expected value in Statistics), the state is called

Y. S. ZHANG

no rmal whe n the ener g y funct io n is ne arl y to the a vera ge.

Normal state is the better state.

A subsystem of a multilateral syste m is called not run-

ning properly (or disease, abnormal), if the energy devia-

tion from the average of the subsystems is too large, the

high [real disease] or the low [virtual disea se ].

In a subsystem of a multilateral system being not run-

ning properly, if the energy of this sub-system is in-

creased or decreased by using external forces and re-

turned to its average (or its expected value), this method

is called intervention (or mak i n g a medical treatment) to

the mult ila te ral system.

The purp ose of interventio n is to make the multilateral

system return to normal state. The method of interven-

tion is to increase or to decrease the energy of a subsys-

tem.

What kind of treatment should follow the principle to

treat it? Western medicine emphasizes direct treatment,

but the indirect treatment of oriental medicine (or Tradi-

tional Chinese Medicine) is required. In mathematics,

which is more reaso nable?

Based on this idea, many issues are worth further dis-

cussion. For example, if an intervention treatment has

been done to a multilateral system, what situation will

happen?

3.2. Intervention Rule of a Multilateral System

For a steady multilateral system V with two

non-compatibility relations, suppose that there is an ex-

ternal force (or an intervenin g force ) on the sub system X

of V which makes the energy φ(X) of X changed by the

increment ∆φ(X), then the energies φ(XS), φ(XK), φ(KX),

φ(SX) of other subsystems XS, XK, KX, SX (defined in

Theorem 2.6) of V will be changed by the increments

∆φ(XS), ∆φ(XK), ∆φ(KX) and ∆φ(SX), respectively.

It is said that a multilateral system has the capability

of intervention reaction if the multilateral system has

capability to response the intervention force.

If a sub syst em X of multilater al system V is inter vene d,

then the energies ∆φ(XS) and ∆φ(SX) of t he subsystems XS

and SX which have neighboring relations to X will chan ge

in the same direction of the force outside on X.We call

them beneficiaries. But the energies ∆φ(XK) and ∆φ(KX)

of the subsystems XK and KX which have alternate rela-

tions to X will change in the opposite direction of the

force outside on X. We call them victims.

Further more, i n general, there is an essential principle

of interventio n: a ny one of energies ∆φ(XS) and ∆φ(SX) of

beneficial subsystems XS and SX of X changes in t he same

direction of the force outside on X, and any one of ener-

gies ∆φ(XK) and ∆φ(KX) of harmful subsystems XK and

KX of X changes in the opposite direction of the force

outside on X. The changed size of the energy ∆φ(XS) (or

∆φ(SX)) is equal to that of ∆φ(XK) (or ∆φ(KX)), but the

direction opposite.

Intervention Rule: In the case of virtual disease, the

treatment method of intervention is to increase the ener-

gy. If the treatment has been done on X, the energy in-

crement (or, increase degree) |∆φ(XS)| of the son XS of X

is greater than the energy increment (or, increase degree)

|∆φ(SX)| o f the mother SX of X, i.e., the b est benefit is t he

son XS of X. But the energy decrease degree |∆φ(XK)| of

the prisoner XK of X is greater than the energy decrease

degree |∆φ(KX)| of the bane KX of X, i.e., the worst victim

is the prisoner XK of X.

In the case of real disease, the treatment method of in-

tervention is to decrease the energy. If the treatment has

been done on X, the ener g y decrease degree |∆φ(SX)| of

the mother SX of X is greater than the energy decrease

degree |∆φ(XS)| of the son XS of X, i.e., the best benefit is

the mother SX of X. But the energy increment (or, in-

crease degree) |∆φ(KX)| of the bane KX of X is greater

than the energy increment (or, increase degree) |∆φ(XK)|

of the prisoner XK of X, i.e., the worst victim is the bane

KX of X.

In mathematics, the changing la ws are as follows.

1) If ∆φ(X) = ∆ > 0, then ∆φ(XS) = ρ1∆, ∆φ(XK) = –ρ1∆,

∆φ(KX) = －ρ2∆, ∆φ(SX) = ρ2∆;

2) If ∆φ(X) = –∆ < 0, then ∆φ(XS) = –ρ2∆, ∆φ(XK) =

ρ2∆, ∆φ(KX) = ρ1∆, ∆φ(SX) = –ρ1∆;

where 1 ≥ ρ1 ≥ ρ2 ≥ 0. Both ρ1 and ρ2 are called interven-

tion reaction coefficients, which are used to represent t he

capability of i nter ve ntion reactio n. The larger ρ1, the better

the capabilit y of inter vention reactio n. T he state ρ1 = 1 is

the best state but the state ρ1 = 0 is the worst state.

Medical and drug resistance problem is that such a

question, beginning more appropriate medical treatment,

but is no longer valid after a period. It is because the ca-

pabilit y of interve ntion reaction is bad, i.e., the inter ven-

tion reaction coefficients ρ1 and ρ2 are too small. In the

state ρ1 = 1, any medical and drug resistance problem is

non-existence but in the state ρ1 = 0, medical and drug

resistance p roblem is always existence. At this point, t he

paper advocates the principle of treatment to avoid med-

ical and drug resistance problems.

This intervention rule is similar to force and reaction

in Physic s.

3.3. Self-Protection Rule of a Multilateral System

If there is an intervening force on the subsystem X of a

steady multilatera l system V whic h makes the energy φ(X)

changed by increment ∆φ(X) s uch t hat the e ner gies φ(XS),

φ(XK), φ(KX), φ(SX) of other subsystems XS, XK, KX, SX

(defined in Theorem 2.6) of V will be changed by the

Y. S. ZHANG

increments ∆φ(XS), ∆φ(XK), ∆φ(KX), ∆φ(SX), respectively,

then can the multilateral system V have capability to

protect the worst victim to restore?

It is said that the steady multilateral system has the

capability of self-protection i f the multi latera l sys te m has

capability to protect the worst victim to restore. The ca-

pability of self-protection of the steady multilateral sys-

tem is said to be better if the multilateral system has ca-

pability to protect the all victi ms to restore.

In general, there is an essential principle of

self-protection: any harmful subsystem of X should be

protected by using the same intervention force but any

beneficial subsystem of X s hould not.

Self-protec tion Rule: in the case of virtual disease, the

treatment method of intervention is to increase the ener-

gy. If the treatment has been done on X, the wo rs t v ictim

is the prisoner XK of X. Thus, the treatment of

self-protection is to restore the prisoner XK of X and the

restoring method of self-protection is to increase the

energy φ(XK) of the prisoner XK of X by using the inter-

vention force on X acc ording to t he intervention rule.

In the case of real disease, the treatment method of in-

tervention is to decrease the energy. If the treatment has

been done on X, the worst victim is the bane KX of X.

Thus, the treatment of self-protection is to restore the

bane KX of X and the restoring method of self-protection

is to decrease the energy φ(KX) of the bane KX of X by

using the same intervention force on X according to the

inter venti on r ule.

In mathematics, the following self-protection laws

hold.

1) If ∆ φ(X) = ∆ > 0, then the energy o f subsys tem XK

will decrease the increment (–ρ1∆), which is the worst

victim. So the capability of self-protection increases the

energy of subsystem XK by increment (ρ1∆) in order to

restore the worst victim XK by using the same interven-

tion force on X according to the intervention rule.

2) If ∆φ(X) = –∆ < 0, then the energy ∆φ(KX) of sub-

system KX will increase the increment (∆φ(KX) = ρ1∆),

which is the worst victim. So the capability of

self-protection decreases the energ y of sub system KX, by

the same size to ∆φ(KX) but the direction opposite, i.e.,

by increment (∆φ(XK)1 = –ρ1∆), in order to restore the

worst victim KX by u sin g the sa me inte rve ntion forc e on

X according to the intervention rule.

The self-protection rule can be explained as: the gen-

eral principle of self-protection subsystem is the most

affected is protected firstly; the protection method is in

the same way to the i ntervention force.

Theorem 3.1 Suppose that a steady multilateral sys-

tem V which has energy and capability of self-protection

is with interve ntion reaction coefficients ρ1 and ρ2. If the

capability of self-protection can make the subsystem XK

to be restore d, then the following statements are true.

1) In the case of virtual disea se, the treatment method

is to increase the energy. If an intervention force on the

subsy st em X of steady multilateral system V is imple-

mented such that its energy φ(X) has been changed by

increment ∆φ(X) = ∆ > 0, then all five sub systems will be

changed finally by the increments as follows:

( )( )( )()

( )( )( )

( )

( )( )( )()

( )()( )

( )

( )( )( )

( )

1 21

1 0,

S SS

K KK

X XX

K KK

S SS

ϕϕ ϕρρ

ϕϕϕρ ρρ

ϕϕ ϕρρ

∆=∆+∆= −∆>

∆=∆+∆= +∆>

∆=∆+∆=−+∆=

∆=∆+∆=− −∆

∆=∆+∆=− ∆

∀∆=∆ >

2) In the case of real disease, the treatment method is

to decrease the energy. If an intervention force on the

subsystem X of steady multilateral system V is imple-

mented such that its energy φ(X)' has been changed by

increment ∆φ(X)' =

－

∆ < 0, then all five subsystems

will be changed finally by the increments as follows:

( )( )( )()

( )( )( )

( )

( )( )( )

( )

( )()( ) ()

( )( )( )()

( )

1 21

1 0,

S SS

K KK

X XX

K KK

S SS

ϕϕ ϕρρ

ϕϕϕρ ρρ

′ ′′

∆=∆+∆=− −∆<

′ ′′

∆=∆+∆=− −∆

′ ′′

∆=∆+∆=− ∆

′ ′′

∆=∆+∆=− ∆=

′ ′′

∆=∆+∆=− +∆<

′

∀∆=−∆ <

where the ∆φ(*)1 ’s and ∆φ(*)1' ’s are the increments

under the capability o f self-protection.

Corollary 3.1 Suppose that a steady multilateral sys-

tem V which has energy and capability of self-protection

is with intervention reaction coefficients ρ1 and ρ2. Then

the capability of self-protection can make both subsys-

tems XK and KX to be restored at the same time, i.e., the

capability of self-protection is better, if and only if ρ2 =

Side effects of medical problems were the questio n: i n

the medical process, destroyed the normal balance of a

normal system which is not falling-ill system or inter-

vening syst em. B y T he or e m 3.1 and Corollary 3.1, it can

be seen that a necessary and sufficient condition is ρ2 =

if the capabilit y of self-prote ction of t he steady mul-

tilateral system is better, i.e., the multilateral system has

capability to protect all victims to restore. At this point,

the paper advocates the principle to avoid any side ef-

fects of treatment.

Y. S. ZHANG

3.4. Mathematical Reasoning of Treatment

Principle by Using the Neighboring

Relations of Steady Multilateral Systems

Tr ea tment p r inci p le by usi n g t he ne ig hb o ri n g re la tions of

steady multilateral systems is “Virtual disease is to fill

his mother but real disease is to rush down his son”. In

order to show the ra tionality o f the treat ment principle , it

is needed to prove the following theorems.

Theorem 3.2 Suppose that a steady multilateral sys-

tem V which has energy and capability of self-protection

is with intervention reaction coefficients ρ1 and ρ2 satis-

fying

ρρ

. Then the following statements a re true.

In the case of virtual disea se, if an intervention force

on the subsystem X of steady multilateral system V is

implemented such that its energy φ(X) increases the in-

crement ∆φ(X) = ∆ > 0, then the subsystems SX, XK and

KX can be restored at the same time, but the subsystems

X and XS will increase their energies finally by the in-

crements

( )()( )

( )

12 1

X XX

ϕρρ ϕρϕ

∆=− ∆=−∆

=−∆

and

( )

()( )

( )

112 11

X XX

ϕρρρ ϕρρϕ

ρρ

∆=+ ∆ =+∆

=+∆

respectively.

On the other hand, in the case of real disease, if an

intervention force on the subsystem X of steady multila-

teral system V is implemented such that its energy φ(X)′

decreases, i.e., by the increment ∆φ(X)′ = –∆ < 0, the

subsystems XS, XK and KX can also be restored at the

same time, and the subsystems X and SX will decrease

their energies finally, i.e., by the increments

( )()( )

( )

12 1

X XX

ϕρρ ϕρϕ

′ ′′

∆=− ∆=−∆

=−−∆

and

()()( )

( )

112 11

S XX

ϕρρρ ϕρρϕ

ρρ

′ ′′

∆=+∆=+∆

=−+ ∆

respectively.

Theorem 3.3 For a steady multilateral system V

which has energy and capability of self-protection, as-

sume intervention reaction coefficients are ρ1 and ρ2

which satisfy

ρρ

and ρ1 ≥ 0.5897545123. Then the

following statements are true .

1) If an intervention force on the subsystem X of

steady multilateral system V i s implemented such that its

energy φ(X) has been changed by increment ∆φ(X) = ∆ >

0, then the fin al increme nt (

ρρ

)∆ of the energy φ(XS)

of the subsystem XS changed is greater than the final

increment (

−

)∆ of the energy φ(X) of the subsystem

X changed based on the capability of self-protection.

2) If an intervention force on the subsystem X of steady

multilateral sys tem V is imple mented such that its energy

φ(X) has been changed by increment ∆φ (X) = –∆ < 0,

then the final increment –(

ρρ

)∆ of the energy φ(SX)

of the subsystem SX changed is less than the final incre-

ment –(

−

)∆ of the energy φ(X) of the subsystem X

changed based on the capability of self-protection.

Corollary 3.2 For a steady multilateral system V

which has energy and capability of self-protection, in-

tervention reaction coefficients are ρ1 and ρ2 which sa-

tisfy

ρρ

and ρ1 < 0.5897545123. Then the follow-

ing statements are true.

1) In the case of virtual disease, if an intervention

force on the subsystem X of steady multilateral system V

is implemented such that its energy φ(X) has been

changed by increment ∆φ(X) = ∆ > 0, then the final in-

crement (

ρρ

)∆ of the energy φ(XS) of the subsystem

XS changed is less than the final increment (

−

)∆ of

the energy φ(X) of the subsystem X changed based on the

capability of self-protection.

2) In the case of real disease, if an intervention force

on the subsystem X of steady multilateral system V is

implemented such that its energy φ(X) has been changed

by increment ∆φ(X) = –∆ < 0, then the final increment

–(

ρρ

)∆ of the energy φ(SX) of the subsystem SX

changed is greater than the final increment – (1 –

)∆

of the energy φ(X) of the subsystem X changed based on

the cap ab ility of self-protection.

3.5. Mathematical Reasoning of Treatment

Principle by Using the Alternate Relations

of Steady Multilateral Systems

Treatment principle by using the alternate relations of

steady multilateral systems is “Strong inhibition of the

same time, support the weak”. In order to show the ra-

tionality of t he tre at ment principle, it needed to prove the

following theo rem.

Theorem 3.4 Suppose that a steady multilateral sys-

tem V which has energy and capability of self-protection

is with intervention reaction coefficients ρ1 and ρ2 satis-

fying

ρρ

. Assume there are two subsystems X and

XK of V with an alternate relatio n such that X encounters

virtual disease, and at the same time, XK befalls real

disease. Then the following statements are true.

If an intervention force on the subsystem X of steady

multilateral sys tem V is imple mente d such th at its energy

φ(X) has been changed by increment ∆φ(X) = ∆ > 0 , and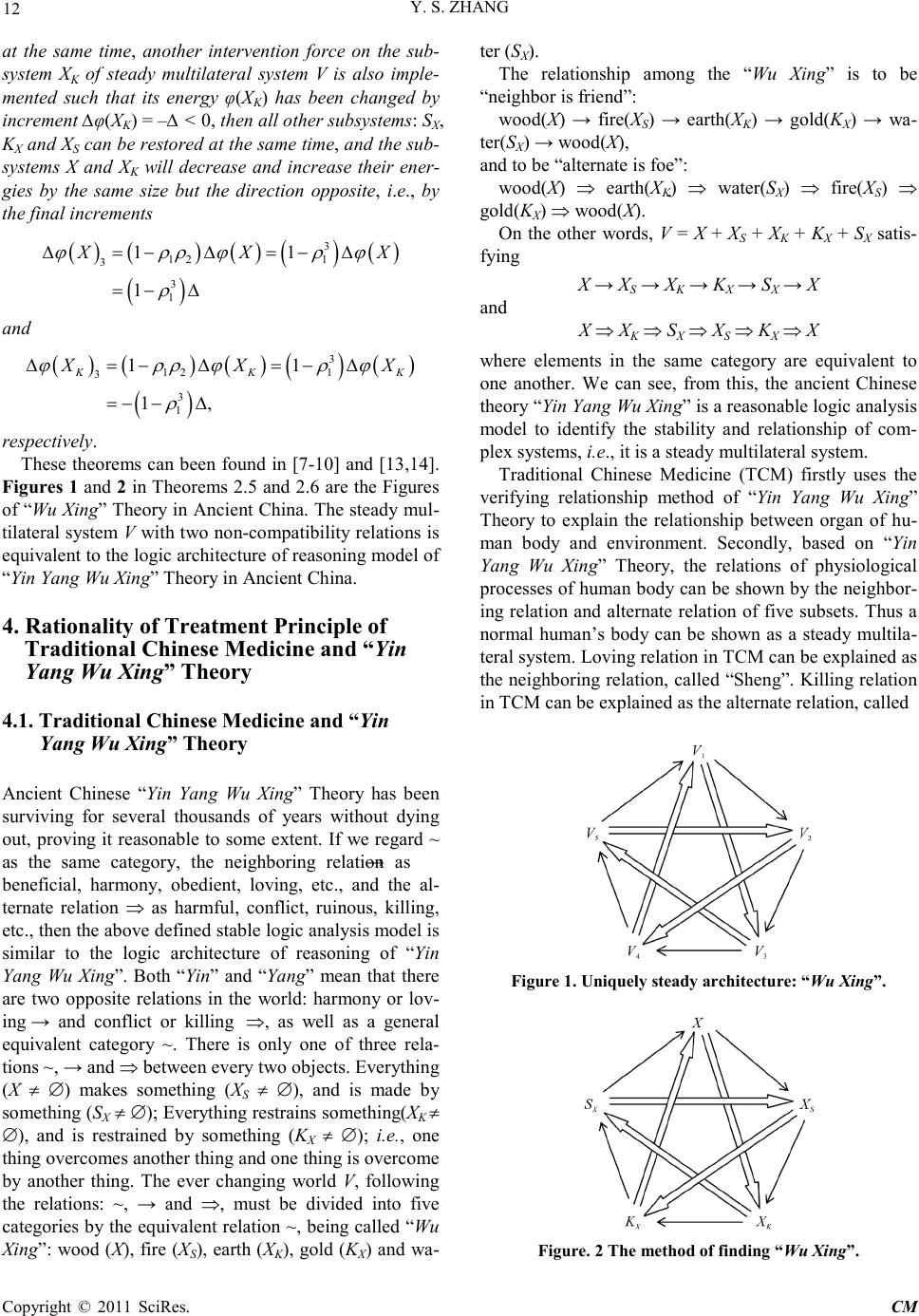

Y. S. ZHANG

at the same time, another intervention force on the sub-

system XK of steady multilateral system V is also imple-

mented such that its energy φ(XK) has been changed by

increment ∆φ(XK) = –∆ < 0, then all other subsystems: SX,

KX and XS can be restored at the same time, and the sub-

systems X and XK will decrease and increase their ener-

gies by the same size but the direction opposite, i.e., by

the final increments

( )()( )

( )

12 1

X XX

ϕρρϕρϕ

∆=− ∆=−∆

=−∆

and

( )()( )

( )

12 1

K KK

X XX

ϕρρ ϕρϕ

∆=− ∆=−∆

=−−∆

respectively.

These theorems can been found in [7-10] and [13,14].

Figures 1 and 2 in T heorems 2.5 and 2.6 are the Fi gure s

of “Wu Xing” The ory in Anci ent China . The steady mul-

tilateral system V with t wo no n-compa tibility relatio ns is

equivale nt to the lo gic archi te cture o f reasoni ng model of

“Yin Yang Wu Xing” Theor y in Anci ent C hi na.

4. Rationality of Treatment Principle of

Traditional Chinese Medicine and “Yin

Yang Wu Xing” Theory

4.1. Traditi ona l Chines e Med icin e and “Yin

Yang Wu Xing” Theory

Ancient Chinese “Yin Yang Wu Xing” Theory has been

surviving for several thousands of years without dying

out, proving it reasonable to some extent. If we regard ~

as the same category, the neighboring relation → as

beneficial, harmony, obedient, loving, etc., and the al-

ternate relation ⇒ as harmful, conflict, ruinous, killing,

etc., then the above defined stable logic analysis model is

similar to the logic architecture of reasoning of “Yin

Yang Wu Xing”. Both “Yin” and “Yang” mean that there

are two opposite relations in the world: harmony or lov-

ing → and conflict or killing ⇒, as well as a general

equivalent category ~. There is only one of three rela-

tions ~, → and ⇒ between every two objects. Everything

(X ≠ ∅) mak e s something (XS ≠ ∅), and is made by

somethi ng (SX ≠ ∅); Eve rythi ng rest rai ns s omet hi ng(XK ≠

∅), and is restrained by something (KX ≠ ∅); i.e., one

thing overcomes another thing and o ne thing is ove rcome

by another thing. The ever changing world V, following

the relations: ~, → and ⇒, must be divided into five

categories by the equivalent relation ~, being called “Wu

Xing”: wood (X), fire (XS), earth (XK), go ld (KX) and wa-

ter (SX).

The relationship among the “Wu Xing” is to be

“neighbor is friend”:

wood(X) → fire(XS) → earth(XK) → gold(KX) → wa-

ter(SX) → wood(X),

and to be “alternate is foe”:

wood(X) ⇒ earth(XK) ⇒ water(SX) ⇒ fire(XS) ⇒

gold(KX) ⇒ wood(X).

On the other words, V = X + XS + XK + KX + SX satis-

fying

X → XS → XK → KX → SX → X

and X ⇒ XK ⇒ SX ⇒ XS ⇒ KX ⇒ X

where elements in the same category are equivalent to

one another. We can see, from this, the ancient Chinese

th eo ry “ Yin Yang Wu Xing” is a reasonable logic analysis

model to identify the stability and relationship of com-

plex systems, i.e., it is a stea dy multilate r a l system.

Traditional Chinese Medicine (TCM) firstly uses the

verifying relationship method of “Yin Yang Wu Xing”

Theory to explain the relationship between organ of hu-

man body and environment. Secondly, based on “Yin

Yang Wu Xing” Theory, the relations of physiological

pro cesses of human b ody ca n be shown b y the ne ighb or-

ing relation and alternate relation of five subsets. Thus a

normal human’s body can be shown as a steady multila-

teral system. Loving relation in TCM can be explained as

the neighboring re lation, called “Sheng”. Killing relation

in T CM can be explained as the alternate relation, called

Figure 1. Uniquely steady archi tecture: “Wu Xing”.

Figure. 2 The method of finding “Wu Xing”.

Y. S. ZHANG

“Ke”. Co nstraints and conversion between five subsets

are equivalent to the two kinds of triangle reasoning. So

a normal human’s body can be classified into five equi-

valence classes. It has been shown in Theorems 2.1-2.6

that the classification of five subsets is quite possible

based on the mathematical logic. To make sure the cha-

racteristics of the five subsets is reasonable or not, it

needs more research work. It has been also shown in

Theorems 3.2-3.4 that the logical basis of TCM is a

steady mu lt ilater a l system.

The gas [“Chi”, or “Qi”, energy of life] of TCM

means the energy in a steady multilateral syste m.

There are two kinds of diseases in TCM: real disease

and virtual disease. They generally mean the subsystem

is abnormal (or disease), its energy [“Chi”, or “Qi”,

energy of l ife] is too high or too low.

The treatment method of TCM is to “Xie Qi” which

means to rush down the energy if a real disease is treated,

or to “Bu Qi” which means to fill the energy if a virtual

disease is treated. Like intervening the subsystem, de-

crease when the energy is too high, increase when the

energy is too low.

Both the capability of intervention reaction and the

capability of self-protection of the multilateral system are

equivalent to the Immunization of TCM. This capability

is really exist. Its target is to protect other organs while

treating one organ.

4.2. Treatment Principle if Only One Organ of

the Human Body System Falls Ill

If we always intervene the abnormal organ of the human

body system directly, the intervention method always

destroy the balance of the human body systems because

it is ha vi ng strong side effects to the mother or the son of

the or gan which is non-diseas e syst em b y usin g T heor em

3.2. The intervening directly method also decreases the

capability of interventio n reaction ρ1, because the method

which doesn’t use the capability of intervention reaction

make s the ρ1 near to 0. T he state ρ1 = 0 is the worst state

of the human body system, namely AIDS. On the way,

the resistance problem will occur since any medicine or

treatment ha s little effect for small ρ1.

Howe v er, b y Co r o ll a r y 3.2, it will e ven be better if we

intervene subsystem X itself directly while ρ1<

0.5897545123, i.e. ρ1 + ρ12 < 0.9375648971. It can be

explained that if a multilateral system which has a poor

capability of intervention reaction, then it is better to

intervene the subsystem X itself directly than indirectly.

However, similar to above, the intervening directly me-

thod always destroys the balance of multilateral systems

such that there is at least one side effect occurred.

Moreover, the intervening directly method is also harm-

ful to the capability of intervention reaction and might

causes the medical and drug resistance problem. There-

fore , the inte rve nti on met hod directly can be used in case

ρ1 < 0.5897545123 but should be used as little as possi-

ble.

If we always intervene in the abnormal organ of the

human body system indirectly, the intervention method

can be to maintain the balance of the human body system

because it has not any side effects to all other organs

which are not both the disease organ and the intervened

organ by using Theorem 3.2. The intervening indirectly

method also increase the capability of intervention reac-

tion because the method of using the intervention reac-

tion makes the ρ1 near to 1. The state ρ1 = 1 is the best

state of the human body system. On the way, it almost

has none medical and drug resistance problem since any

medicine is possible good for some large ρ1.

Overa ll, t he huma n bod y syst em satisfies the interven-

tion rule and the self-protection rule. It is said healthy

while intervention reaction coefficient ρ1 satisfies ρ1 >

0.5897545123. In logic and practice, it's reasonable ρ1 +

ρ2 near to 1. In case: ρ1 + ρ2 = 1, all the energy for inter-

vening human body organ can transmit to other human

body organs which have neighboring relations or alter-

nate relations with the intervening human body organ.

The healthy condition ρ1 > 0.5897545123 can be satis-

fied when ρ2 = ρ12 for a healthy human body since ρ1 + ρ2

= 1 implies ρ1 = (√5-1)/2 ≈ 0.618 > 0.5897545123. If this

assumptions is set up , then th e treat ment principle: “Real

disea se is to rush down his son and virtual disease is to

fill his mother” based on “Yin Yang Wu Xing” Theory of

TCM , is quite reasonable.

On the other hand, in TCM, real disease and virtual

disease have their reasons. Th e bear organ XS causes real

disea se of X, while the born organ SX causes virtual dis-

ease of X. Altho ugh the rea s on cannot be proved easily in

mathematics or experime nts, the treatment method under

the assumption is quite equal to the treatment method in

the intervention indirectly. It has also proved that the

treatment pr inciple is true from the other side.

4.3. Treatment Principle if Two Organs with the

Loving Relation of the Human Body System

Encounter Sick

Supp ose t hat t he t wo orga ns X and XS of the human body

system are abnormal (or disease). In the human body of

relations between non-compatible with the constraints,

only two situations may occur:

1) X encounters virtual disease, and at the same time,

XS befalls virtual disease, i.e., the energy of X is too low

and the e ne r gy o f XS is also to o low. It is because X bears

XS. The disease causal is X.

Y. S. ZHANG

2) X encounters real disease, and at the same time, XS

befalls real disease, i.e., the energy of X is too high and

the energy of XS is al so to o high. It is because X bears XS.

The disease causal is XS.

If intervention reaction coefficients satisfy

ρρ

it can be sho wn by The ore m 3. 2 that if one wants to trea t

the abnormal organs X and XS, then the following state-

ments are true.

1) For virtual disea se of both X and XS, t he one sho uld

intervene organ X directly by increasing its energy. It

means, “Virtual disease is to fill his mother” because the

disease causal is X.

2) For real disease of both X and XS, the one should

intervene organ XS directly by decreasing its energy. It

means, “Real disease is to rush down his son” because

the disease causal is XS.

The intervention method can be to maintain the bal-

ance of the human body because only the energies of two

disease organs are changed by using Theorem 3.2, such

that there is no side effect for all other organs. And the

interventi on method c an al so be to enhance the capability

of intervention reaction because the method of using

intervention reaction makes the ρ1 greater and near to 1.

The state ρ1 = 1 is the best state of the human body sys-

tem. On the way, it almost has none medical and drug

resistance problem since any medicine is possible good

for some large ρ1.

4.4. Treatment Principle if Two Organs with the

Killing Relation of the Human Body System

Encounter Sick

Suppose that the organs X and XK of a human body sys-

tem ar e abnormal (or disease). In the human body system

of relations bet we e n no n-co m patib le with the constraints,

only a situation may occur: X encounters virtual disease,

and at the same time, XK befalls real disease, i.e., the

energy of X is too low and the energy of XK is too high. It

is because it is nor mal when X kills XK but it i s abnormal

when X doesn’t kill XK.

If intervention reaction coefficients satisfy

ρρ

it can be sho wn by The ore m 3.4 that if one wants to tr eat

the abnormal organs X and XK, the one should intervene

organ X di re c t ly by increasing its e nergy, a nd at the sa me

time , intervene organ XK directly by decreasing its ener-

gy. It means that “Strong inhibition of the same time,

support the weak”.

The intervention method can be to maintain the bal-

ance of human body system because only two energies of

both disease organs are changed by using Theorem 3.4,

such that there is no side effect for all other organs. And

the i nterve ntio n metho d can a lso b e to enha nce t he capa-

bility of intervention reaction because the method of us-

ing interve ntion reac tion makes the ρ1 greater and near to

1 such t hat X can kill XK. The state ρ1 = 1 i s t he b est sta te

of the steady multilateral system. On the way, it almost

has none medical and drug resistance problem since any

medicine is possible good for some large ρ1.

5. Conclusions

This work shows how to treat the diseases of a human

body system and three methods are presented. If only

one organ falls ill, mainly the treatment method should

be to intervene it indirectly for case: the capability coef-

ficient ρ1 ≥ 0.5897545123 of intervention reaction, ac-

cording to the treatment principle of “Real disease is to

rush down his son but virtual disease is to fill his moth-

er” . The int er ve nti o n method directly can be used in case

ρ1 < 0.5897545123, but should be used as little as possi-

ble.

If two organs with the loving relation encounter sick,

the treatment method should be intervene them directly

also according to the treatment principle of “Real disease

is to rush down his son but virtual disease is to fill his

mother”.

If two organs with the killing relation encounter sick,

the treatment method should intervene in them directly

accord ing to the treatme nt principle of “Strong inhibition

of the same time, support the weak”.

Other properties such as balanced, orderly nature, and

so on, will b e discusse d in t he next articles.

6. Acknowledgements

This article has been repeatedly invited as reports, such

as People’s University of China in medical meetings,

Shanxi University, Xuchang College, and so on. The

work was supported by Specialized Research Fund for

the Doctoral Program of Higher Education of Ministry of

Education of China (Grant No.44k55050).

7. Referen ces

[1] Y. S. Zhang, “Multilateral Matrix Theory,” Chinese Sta-

tistics Press, 1993. http://www.mlmatrix.com

[2] Y. S. Zhang, S. Q. Pang, Z. M. Jiao and W. Z. Zhao,

“Group Partition and Systems of Orthogonal Idempo-

tents,” Linear Algebraand and Its A pp lications, Vol. 278,

1998, pp . 249-262.

[3] Y. S. Zhang, Y. Q. Lu and S. Q. Pang, “Orthogonal Ar-

rays Obtained by Orthogonal Decomposition of Projec-

tion Matrices,” Statistica Sinica, Vol. 9, 1999, pp. 595-

604.

[4] Y. S. Zhang, S. Q. Pang and Y. P. Wang, “Orthogonal

Arrays Obtained by Generalized Hadamard Product,”

Y. S. ZHANG

Discrete Math, Vol. 238, 2001, pp. 151-170.

doi:10.1016/S0012-365X(00)00421-0

[5] Y. S. Zhang, L. Duan, Y. Q. Lu and Z. G. Zheng, “Con-

struction of Generalized Had amard Matrices,” Journal of

Statistical Planning and Inference, Vol. 104, 2002, pp.

239-258. doi:10.1016/S0378-3758(01)00249-X

[6] Y. S. Zhang and S. S. Mao, “The Origin and Develop-

ment Philosophy Theory of Statistic,” Statistical Re-

search, Vol. 12, 2004, pp. 52-59.

[7] Y. S. Zhang, S. S. Mao, C. Z. Zhan and Z. G. Zheng,

“Stable Structure of the Logic Model with Two Causal

Effects,” Journal of Applied Probability & Statistics, Vol.

21, No. 4, 20 05, pp. 366-374.

[8] N. Q. Feng, Y. H. Qiu, F. Wang, Y. S. Zhang and S. Q.

Yin, “A Logic Analysis Model about Complex System’s

Stability: Enlightenment from Nature,” Lecture Notes in

Compute r Sc ience, Vol. 364 4, 20 05, pp. 828-838.

[9] N. Q. F eng, Y. H . Qiu, Y . S. Zhang, F. Wang an d Y. He,

“A Intelligent Inference Model about Complex System’s

Stability: Inspiration from Nature, ” International Journal

of Intelligent Technology, Vol. 1, 200 5, pp . 1-6.

[10] N. Q. Feng, Y. H. Qiu, Y. S. Zhang, C. Z. Zhan and Z. G.

Zheng, “A Logic Analysis Model of Stability of Complex

System Based on Ecology,” Computer Science, Vol. 33,

No. 7, 2006, pp. 213-216.

[11] Y. S. Zhang, “Data Analysis and Construction of Ortho-

gonal Arrays,” East China Normal University, 2006.

[12] Y. S. Zhang, “Orthogonal Arrays Obtained by Repeat-

ing-Colu mn Differen ce Matrices, ” Discrete Mat hematics,

Vol. 307, 2007, pp. 246-261.

doi:10.1016/j.disc.2006.06.029

[13] C. Y. Pan, X . P . Chen, Y. S. Zhang and S. S. Mao , “ Lo g-

ical Model of Five-Element Theory in Traditional Chi-

nese Medicine,” Journal of Chinese Modern Traditional

Chinese Medicine, Vol. 4, No. 3, 2008, pp. 193-196.

[14] X. P. Chen, W. J. Zhu, C . Y. P an and Y. S. Zhang, “Mul-

tilateral System,” Journal of Systems Science, Vol. 17,

No. 1, 2009, pp. 55-57.

[15] C. Luo and Y. S. Zhang, “Framework Definition and

Partition Theorems Dealing with Complex Systems: One

of the Series of New Thinking,” Journal of Shanghai In-

stitute of Technology (Natural Science), Vol. 10, No. 2,

2010, pp . 109-114.

[16] C. Luo and Y. S. Zhang, “Framework and Orthogonal

Arrays: The New Thinking of Dealing with Complex

Systems Series Two,” Journal of Shanghai Institute of

Technology (Natural Science), Vol. 10, No. 3, 2010, pp.

159-163.

[17] X. D. Wang, Y. C. Tang, X. P. Chen and Y. S. Zhang,

“Design of Experiment in Global Sensitivity Analysis

Based on ANOVA High-Dimen sio nal Mod el Rep resen ta-

tion,” Communication in Statistics: Simulation and

Computation, Vol. 39, 2010, pp. 1183-1195.

doi:10.1080/03610918.2010.484122

[18] Research Center for Chinese and Foreign Celebrities and

Developing Center of Chinese Culture Resources, “Chi-

nese Philosophy Encyclopedia,” Shanghai People Press,

1994.

[19] Y. S. Zhang, “Theory of Multilateral Systems,” 2007.

http://www.mlmatrix.com