J. Biomedical Science and Engineering, 2013, 6, 71-83 JBiSE
http://dx.doi.org/10.4236/jbise.2013.612A009 Published Online December 2013 (http://www.scirp.org/journal/jbise/)
Effect of screw position on bone tissue differentiation
within a fixed femoral fracture
Saghar Nasr1,2, Stephen Hunt1,3, Neil A. Duncan1,3,4*
1McCaig Institute for Bone and Joint Health, Calgary, Canada
2Department of Mechanical & Manufacturing Engineering, Schulich School of Engineering, University of Calgary, Calgary, Canada
3Department of Orthopedic Surgery, University of Calgary, Calgary, Canada
4Department of Civil Engineering, Schulich School of Engineering, University of Calgary, Calgary, Canada
Received 30 October 2013; revised 28 November 2013; accepted 17 December 2013
Copyright © 2013 Saghar Nasr et al. This is an open access article distributed under the Creative Commons Attribution License,
which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
ABSTRACT
Plate and screw constructs are routinely used in the
treatment of long bone fractures. Despite consider-
able advancements in technology and techniques,
there can still be complications in the healing of long
bone fractures. Non-unions, delayed unions, and
hardware failures are common complications ob-
served in clinical practice following open reduction
and internal fixation of fractures [1]. Potential causes
of these adverse clinical effects may be disruptive to
the periosteal and endosteal blood supply, stress
shielding effects, and inadequate mechanical stability.
The goal of the present study was to explore the effect
of screw position on the fracture healing and forma-
tion of new bone tissue with mechanoregulatory algo-
rithms in a computational model. An idealized poroe-
lastic 3D finite element (FE) model of a femur with a
5 mm fracture gap, including a plate-screw construct
was developed. Nineteen different plate-screw com-
binations, created by varying the number and posi-
tion of screws within the plate, were created to iden-
tify a construct with the most favourable attributes
for fracture healing. The first phase of the study
evaluated constructs through mechanical stress
analyses to identify those constructs with high load-
support capability. The second phase of the study
evaluated healing and bone formation with a biphasic
mechanoregulatory algorithm to simulate tissue dif-
ferentiation for fixation within selected constructs.
The results of our analysis demonstrated a 4-screw
symmetrical construct with the largest distance be-
tween screws to provide the most favourable balance
of stability and optimized conditions to pro mote frac-
ture healing.
Keywords: Femoral Fracture; Internal Fixation; Screw
Number; Screw Position; Tissue Differentiation; Finite
Element Analysis
1. INTRODUCTION
The treatment of long bone fractures is a significant
health problem, particularly for femoral fractures [2-5].
Although bone has outstanding mechanical properties
and a remarkable ability to heal, in some cases healing is
impaired or delayed [6,7]. Internal fixators have been
used as the most common treatment technique for open
and closed fractures [3,8-10]. Despite the advent of in-
tramedullary fixation, there are still clinical indications
for plating of long bone fractures (e.g., pulmonary com-
plications, damage control orthopaedics, revision proce-
dures, absence of X-ray, obliterated canal, small canal
and periarticular fractures). The main goals of any or-
thopaedic fixation technique are to maintain anatomic
alignment, provide stability of the fracture to facilitate
healing, and increase load bearing to facilitate rehabilita-
tion [11]. Internal fixation is commonly a fracture span-
ning plate with a combination of screws through the plate.
The two main drawbacks of internal fixations are: 1)
disruption of blood supply, and 2) shielding the under-
lying bone and fracture gap from mechanical stresses. To
overcome vascularisation issues and surgical exposure,
orthopaedic hardware is installed with utmost care to
protect soft tissues, and minimize soft tissue stripping in
order to lessen devascularisation of bone [3,10,11]. One
technique to minimize vascular damage to bone includes
reducing the number of screws used to anchor the plate
to bone [12]. Reducing the number of screws may not
significantly affect the structural stiffness of the fracture,
but may increase the strain at the fracture site [12]. Fur-
thermore, the mismatch between bone and plate stiffness
*Corresponding author.

S. Nasr et al. / J. Biomedical Science and Engineering 6 (2013) 71-83 72
can cause stress shielding which may lead to complica-
tions of bone resorption and focal osteoporosis [13-16].
A comprehensive and algorithmic approach to selecting
screw type and configuration may minimize the delete-
rious effects of screw installation, provide mechanical
and biological insight on the contributions of screw type
and position, and provide an evidence-based justification
for surgical technique. It is our hope that this will pro-
vide the basis for further advancement of open reduction
and internal fixation techniques for fracture care.
Empirical and computational data support that me-
chanical stimulation of bone is an important factor in
bone healing [17-20]. A number of different mech-
anoregulatory algorithms have been developed and are
generally classified into 1) single-solid phase and 2) bi-
phasic algorithms. These algorithms are regulated by one
or two mechanical stimuli, which may include inter-
fragmentary gap movement, octahedral shear strain, hy-
drostatic stress, fluid velocity and fluid shear stress
[17,18,21-24]. Isaksson et al. (2006) compared tissue dif-
ferentiation patterns in a finite element model (FE) of an
ovine tibia implementing different proposed algorithms
[17]. The computational predictions were then validated
against experimental observations in vivo. The biphasic
algorithm proposed by Lacroix and Prendergast (2002)
was found to most closely model experimental observa-
tions [17]. The biphasic algorithm has been widely used
to investigate skeletal tissue repair [17,23, 25-32], and
thus we selected this biphasic algorithm to predict tissue
differentiation in our analyses.
Prediction of the bone mechanobiology conditions at
the fracture site has been previously investigated to op-
timize fixation constructs [33-35]. Kim et al. (2012) used
a single-solid phase mechanoregulatory model regulated
by deviatoric tissue strain to predict tissue differentiation
in a 3D idealized fracture tibia using plates with different
material properties. The carbon/epoxy composite plate
was found to highly promote tissue differentiation [35].
Son and Chang (2013) used the same single-solid phase
mechanoregulatory algorithm as Kim et al. (2012) to
predict the healing process in idealized 3D tibia models
with different oblique fractures [34]. It was observed that
the plate material (stiffness) that should be used was
highly dependent on the angle of the fracture. The car-
bon/epoxy composite plate had the highest healing rate
when the fracture angle was lower than 25˚, whereas for
higher angles, the glass/polypropylene composite plate
led to more bone formation [34].
Dubov et al. (2011) explored optimal cable-screw po-
sitioning in femoral fracture models using an eight
screw-hole plate and six screws (using 2 screws in the
proximal femur). The stability and biomechanical per-
formance of different cable-screw constructs were com-
putationally predicted, and then validated against ex-
perimental results [10]. The femur was modelled as an
elastic material and quasi-static loads were applied to
one end while the other end was fixed. The construct
experiencing the least von Mises stress values at the
screws, plate and bone was selected as the optimal struc-
ture. However, to the best of our knowledge, no studies
have been performed to predict tissue differentiation
within the fracture for different bone-screw assemblies.
With the advancement of minimally invasive surgical
techniques, optimal screw configuration may influence
surgical technique and equipment design. The prevalence
of orthopaedic joint replacements has resulted in a sig-
nificant increase in the incidence of periprosthetic frac-
tures. The limited space and bone available for fixation
with plate and screw constructs present the orthopaedic
surgeon with considerable operative challenges. Better
understanding of the optimal screw number and position
has the potential to advance fracture care in these situa-
tions [36,37].
Improper screw spacing and number may result in
non-ideal mechanical conditions and consequently, dis-
rupted healing and cell apoptosis [38-40]. Hence, predic-
tion of the mechanical environmental and tissue differen-
tiation may help us to obtain a better understanding of
the optimal screw number and position. This knowledge
can improve internal fixation techniques to better prevent
non-unions and accelerate the healing process. Therefore,
the objective of this study was to evaluate the effect of
internal fixation configuration on the mechanical stabil-
ity and the healing progression for a transverse femoral
fracture. We varied the screw positions within a standard
orthopaedic plate installed across a 5 mm fracture gap
[10] and identified a construct that provided the most
favourable mechanical and mechanobiological condi-
tions for healing. This systematic computational analysis
can contribute to an evidence-based justification of op-
timal plate-screw combination and can provide important
mechanical performance guidelines for surgical tech-
nique and product selection.
2. METHODS
An idealised 3D finite element model of a human femur
with a 5 mm fracture gap [10] was developed (ABAQUS
v6.11, Simulia, USA) to represent a transverse fracture in
the femoral shaft (Figure 1). The femur was modelled as
an outer cortical layer and an inner trabecular core with
diameters of 32 mm and 16 mm, respectively [10]. The
fracture gap was assumed to be initially filled with a
scaffold consisting of stem-cell-seeded granulation tissue.
To model the internal fixation, a 3.5 mm Low Contact-
Dynamic Compression Plate (LC-DCP, Synthes, Paoli,
PA, USA) containing eight screw-holes with uniform
spacing was created (Figure 1). LC-DCPs are mainly
used for fractures in long bones and have minimal direct

S. Nasr et al. / J. Biomedical Science and Engineering 6 (2013) 71-83 73
Lateral
Axial load
Fixed
Medial
5 mm
106 mm
3.5 mm
3.5 mm
13 mm
3.3 mm
6 mm
Figure 1. Finite element representation of the bone, plate and screws assembly.
contact with underlying bone to preserve blood supply
[14,41]. To accommodate the irregular surface of bone,
the mismatch between the curvature of the bone and the
plate, and interposed fascia and soft tissue between the
plate and bone (commonly observed in surgical expo-
sures that minimize soft tissue insult in the zone of in-
jury), we modelled our plate elevated 1 mm from the
surface of the bone [42].
The distance between the hole centers was 13 mm
(VS3041.08, Synthes, USA). Size-matched locking
screws (VS301.038, Synthes, USA) with a diameter of
3.5 mm were modelled [3,11]. The plate and locking
screws had lengths of 106 mm and 38 mm, respectively
(Figure 1). Both the screws and plate holes were fully
threaded, and thus their contacting pairs (i.e. bone-screw
and plate-screw) had negligible relative motion with re-
spect to each other. Therefore, a tie constraint was de-
fined between all contacting pairs (including bone-scaf-
fold) to make the translational and rotational motions
equal for each contacting surface. An axial compressive
load, which is the predominant load in long bones
[43,44], was considered in our analyses. The distal end of
the femur was completely constrained while a cyclic load
was applied to the proximal end. According to the ex-
perimental study of Aranzulla et al. (1998), the weight
bearing load to a fractured bone was reported to be ~50%
body weight (BW) at 6 weeks post fracture [45]. Patients
generally limit their weight bearing on their affected ex-
tremity due to pain, the use of walking aids, and clinical
direction from their surgeon to minimize the chances of
premature hardware failure. Therefore, an average axial
compression load of 350 N (~50% BW) was used in our
simulations to be representative of a typical orthopaedic
post-operative protocol [46,47]. The plate-screw con-
struct shifted the neutral axis of the bone toward the plate.
Therefore, the axial compressive load also applied a
marginal bending moment to the assembly (bone and in-
ternal fixation).
The bone and scaffold were defined as isotropic and
poroelastic materials, whereas the plate and screws were
modelled as isotropic and linear elastic materials. Based
on the experimental/numerical study of Papini et al.
(2007), the Young’s modulus (E) and Poisson’s ratio (ν),
respectively, were defined as 16.7 GPa and 0.3 for corti-
cal; and 0.155 GPa and 0.3 for trabecular bone, respect-
tively (Table 1). The permeability and void ratio were
defined as 10 - 5 mm4/Ns [48] and 0.041 [49] for cortical
bone and 0.37 mm4/Ns and 4.0 [26] for the trabecular
bone, respectively (Ta b l e 1 ). The plate and screws were
considered as 316 L medical-grade stainless steel (Syn-
thes, Paoli, Pennsylvania, USA) with a Young’s modulus
and a Poisson’s ratio of 193 GPa and 0.3, respectively
(Table 1).
Cortical and trabecular bone were meshed using 4-
node linear tetrahedron pore pressure elements (C3D4P).
The linear tetrahedral elements were selected over the
quadratic elements to decrease the computational costs.
The scaffold was meshed using 10-node modified quad-
ratic tetrahedron pore pressure elements (C3D10MP).
The plate and screw structures were meshed using
10-node quadratic tetrahedron elements (C3D10). Three
meshes with increasing density (62545, 71763 and 95175
elements) were created to assess mesh convergence. The
average octahedral shear strain, fluid velocity and
maximum displacement of the scaffold were considered
for convergence analysis. The increase in the element
number, from 62545 to 95175, resulted in ~0.6% differ-
ence for the average octahedral shear strain and maxi-
mum displacement, and ~0.03% for the average fluid
velocity. Hence, 62545 elements were considered ade-
quate for an accurate estimation of the mechanical be-
haviour within the model.
Tissue differentiation within the scaffold was modelled
using a biphasic algorithm based on octahedral shear

S. Nasr et al. / J. Biomedical Science and Engineering 6 (2013) 71-83 74
Table 1. Mechanical properties of the tissue and internal fixation materials [26,48,49].
Femur Scaffold Internal fixation
Cortical Trabecular Granulation Fibrous tissue Cartilage Bone Plate/Screw
Young’s modulus [GPa] 16.7 0.155 0.0002 0.002 0.01 1 - 6 193
Poisson’s ratio 0.3 0.3 0.167 0.167 0.167 0.3 0.3
Permeability [mm4/Ns] 10−5 0.37 0.01 0.01 0.005 0.1 - 0.37 -
Void ratio 0.041 4.0 4.0 4.0 4.0 4.0 -
strain () and interstitial fluid velocity (
oct
ε
f
v) [24,25]:
00370003
oct
f
εv
S.. . (1)
High values for mechanical stimulus (S) promote the
differentiation of cells into fibrous tissues (3 < S < 6),
intermediate values stimulate cartilage differentiation (1
< S < 3), and low levels lead to formation of immature
(0.267 < S < 1), and mature bony tissue (0.011 < S <
0.267).
For each element, differentiation and the formation of
bone tissue was simulated by a gradual change of mate-
rial properties over time. The initial cellular tissue gradu-
ally differentiated into fibrous tissue, immature and ma-
ture cartilage, and immature and mature bone depend-
ing on the local mechanical environment. A user defined
FORTRAN subroutine, USDFLD, was developed to up-
date the material properties based on the average of
computed mechanical stimulus (S) in the previous 10
steps (weeks) [34,35]. In each step, the mechanical stim-
uli were calculated at the point of maximum loading, and
a cyclic compressive load of 350 N was applied to the
proximal end of femur. One loading step was considered
as a surrogate for one week of healing [34,35], and the
applied load represented the average load that was ap-
plied to the bone during one week of healing [34,35].
To investigate the effect of screw spacing on the me-
chanical stability of the fracture and bone healing proc-
ess, nineteen distinct constructs were created (Figure 2).
The models were composed of (a) eight screws: C1
(Figure 2); (b) seven screws: C2, C3; (c) six screws: C4,
C5, C6, C2a, C2b, C3a and C3b; (d) five screws: C4a,
C5a and C6a; (e) four screws: C4b, C5b and C6b; and (f)
two screws: C7, C8 and C9 (Figure 2). Considering all
models illustrated in Figure 2: (1) in each column, the
number and location of screws in the proximal femur are
held fixed, whereas variations are applied to the screws
in the distal femur; (2) in the first two rows, the number
and location of the screws in the distal femur are held
fixed, whereas variations are applied to the screws in the
proximal femur; and (3) the configurations of third and
fourth rows are symmetric about the center line passing
through the fracture zone. Note that each column in Fig-
ure 2 is called “a family of constructs” in the rest of the
paper.
Figure 2. Constructs representing different screw combina-
tions. The cortical bone, trabecular bone and scaffold are
shown in dark grey, green and orange, respectively. The
screws are numbered starting from the proximal end as
shown in construct C1. The arrows show the sequential
change in each family of constructs.
A number of experimental/numerical studies [50-53]
have shown that bone fails by brittle fracture. Schileo et
al. (2008) computationally predicted the location of ca-
daver femur fractures using the maximum principal
stress, maximum von Mises stress and maximum strain
criteria in comparison to experimental observations (i.e.,
the load-displacement curves and high-speed movies)
[50]. Comparing the computational predictions to the
experimental observations, they observed that the strain
criterion better predicted the risk of bone fracture and its
location [50]. In the present study, the maximum princi-
pal strain and von Mises criteria were both used to dis-
tinguish the models with lower risk of fracture and
higher load-support capabilities [10,50,54]. Ultimate
compressive and tensile strains of 0.0104 and 0.0073,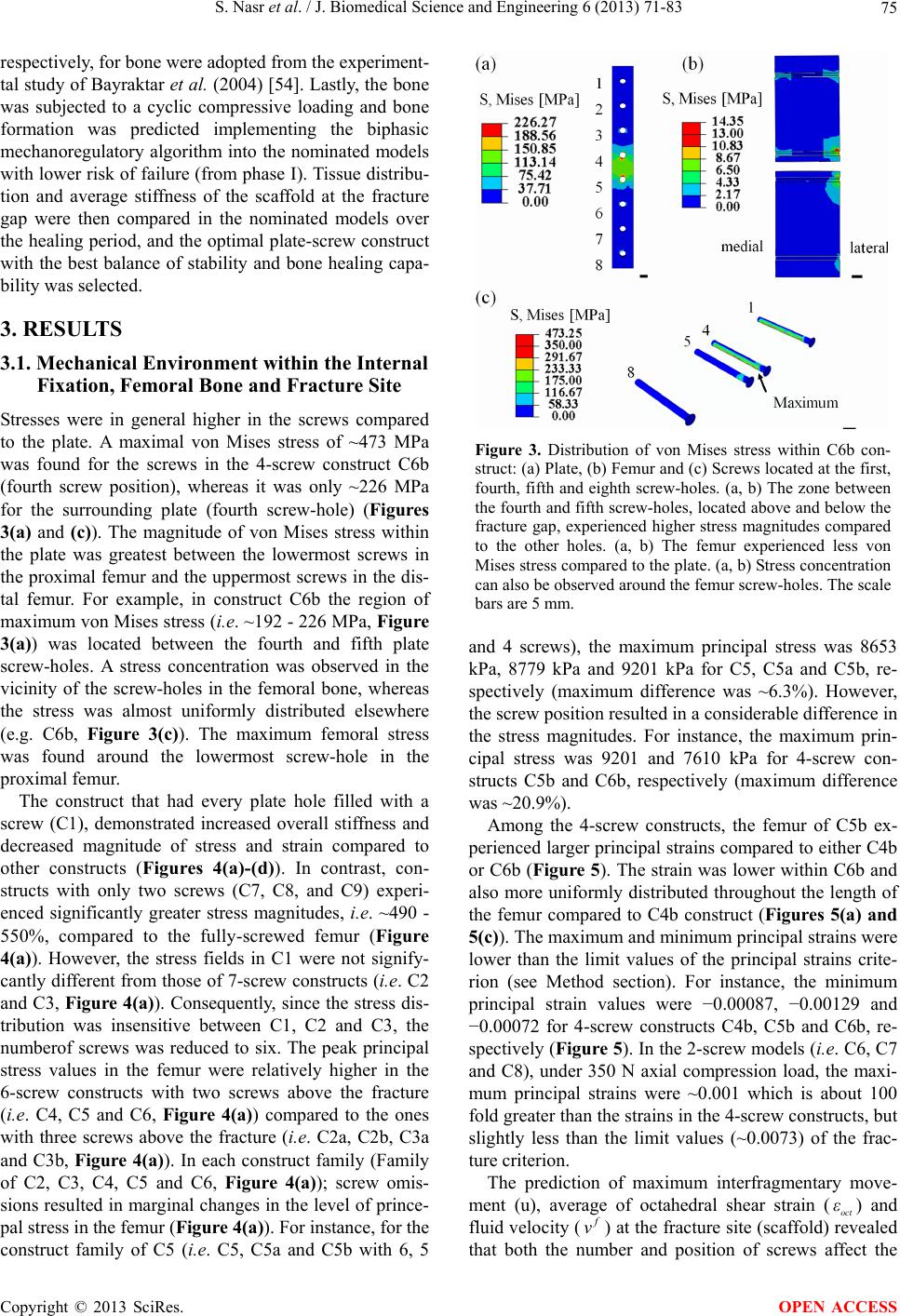

S. Nasr et al. / J. Biomedical Science and Engineering 6 (2013) 71-83 75
respectively, for bone were adopted from the experiment-
tal study of Bayraktar et al. (2004) [54]. Lastly, the bone
was subjected to a cyclic compressive loading and bone
formation was predicted implementing the biphasic
mechanoregulatory algorithm into the nominated models
with lower risk of failure (from phase I). Tissue distribu-
tion and average stiffness of the scaffold at the fracture
gap were then compared in the nominated models over
the healing period, and the optimal plate-screw construct
with the best balance of stability and bone healing capa-
bility was selected.
3. RESULTS
3.1. Mechanical Environment within the Internal
Fixation, Femoral Bone and Fracture Site
Stresses were in general higher in the screws compared
to the plate. A maximal von Mises stress of ~473 MPa
was found for the screws in the 4-screw construct C6b
(fourth screw position), whereas it was only ~226 MPa
for the surrounding plate (fourth screw-hole) (Figures
3(a) and (c)). The magnitude of von Mises stress within
the plate was greatest between the lowermost screws in
the proximal femur and the uppermost screws in the dis-
tal femur. For example, in construct C6b the region of
maximum von Mises stress (i.e. ~192 - 226 MPa, Figure
3(a)) was located between the fourth and fifth plate
screw-holes. A stress concentration was observed in the
vicinity of the screw-holes in the femoral bone, whereas
the stress was almost uniformly distributed elsewhere
(e.g. C6b, Figure 3(c)). The maximum femoral stress
was found around the lowermost screw-hole in the
proximal femur.
The construct that had every plate hole filled with a
screw (C1), demonstrated increased overall stiffness and
decreased magnitude of stress and strain compared to
other constructs (Figures 4(a)-(d)). In contrast, con-
structs with only two screws (C7, C8, and C9) experi-
enced significantly greater stress magnitudes, i.e. ~490 -
550%, compared to the fully-screwed femur (Figure
4(a)). However, the stress fields in C1 were not signify-
cantly different from those of 7-screw constructs (i.e. C2
and C3, Figure 4(a)). Consequently, since the stress dis-
tribution was insensitive between C1, C2 and C3, the
numberof screws was reduced to six. The peak principal
stress values in the femur were relatively higher in the
6-screw constructs with two screws above the fracture
(i.e. C4, C5 and C6, Figure 4(a)) compared to the ones
with three screws above the fracture (i.e. C2a, C2b, C3a
and C3b, Figure 4(a)). In each construct family (Family
of C2, C3, C4, C5 and C6, Figure 4(a)); screw omis-
sions resulted in marginal changes in the level of prince-
pal stress in the femur (Figure 4(a)). For instance, for the
construct family of C5 (i.e. C5, C5a and C5b with 6, 5
Figure 3. Distribution of von Mises stress within C6b con-
struct: (a) Plate, (b) Femur and (c) Screws located at the first,
fourth, fifth and eighth screw-holes. (a, b) The zone between
the fourth and fifth screw-holes, located above and below the
fracture gap, experienced higher stress magnitudes compared
to the other holes. (a, b) The femur experienced less von
Mises stress compared to the plate. (a, b) Stress concentration
can also be observed around the femur screw-holes. The scale
bars are 5 mm.
and 4 screws), the maximum principal stress was 8653
kPa, 8779 kPa and 9201 kPa for C5, C5a and C5b, re-
spectively (maximum difference was ~6.3%). However,
the screw position resulted in a considerable difference in
the stress magnitudes. For instance, the maximum prin-
cipal stress was 9201 and 7610 kPa for 4-screw con-
structs C5b and C6b, respectively (maximum difference
was ~20.9%).
Among the 4-screw constructs, the femur of C5b ex-
perienced larger principal strains compared to either C4b
or C6b (Figure 5). The strain was lower within C6b and
also more uniformly distributed throughout the length of
the femur compared to C4b construct (Figures 5(a) and
5(c)). The maximum and minimum principal strains were
lower than the limit values of the principal strains crite-
rion (see Method section). For instance, the minimum
principal strain values were −0.00087, −0.00129 and
−0.00072 for 4-screw constructs C4b, C5b and C6b, re-
spectively (Figure 5). In the 2-screw models (i.e. C6, C7
and C8), under 350 N axial compression load, the maxi-
mum principal strains were ~0.001 which is about 100
fold greater than the strains in the 4-screw constructs, but
slightly less than the limit values (~0.0073) of the frac-
ture criterion.
The prediction of maximum interfragmentary move-
ment (u), average of octahedral shear strain (oct ) and
fluid velocity (
ε
f
v) at the fracture site (scaffold) revealed
hat both the number and position of screws affect the t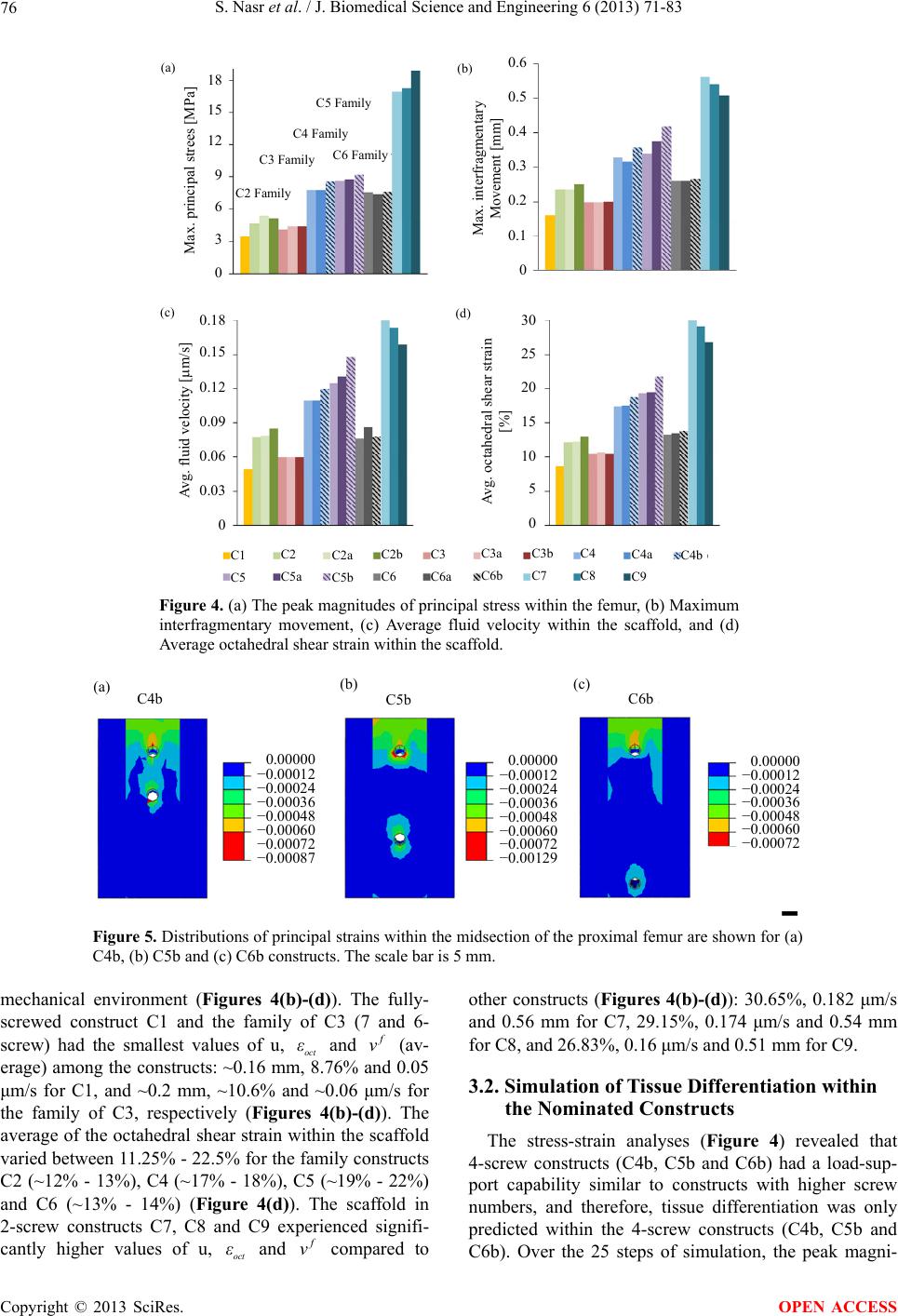

S. Nasr et al. / J. Biomedical Science and Engineering 6 (2013) 71-83 76
0.6
0.5
0.4
0.3
0.2
0.1
0
Max. principal strees [MPa]
C2 Family
C3 Family
C4 Family
C5 Family
C6 Family
(a) (b)
(c) (d)
Max. interfragmentary
Movement [mm]
0.18
0.15
0.12
0.09
0.06
0.03
0
Avg. fluid velocity [m/s]
Avg. octahedral shear strain
[%]
18
15
12
9
6
3
0
30
25
20
15
10
5
0
C1
C5
C2
C5a
C2a
C5b
C2b
C6
C3
C6a
C3a
C6b
C3b
C7
C4
C8
C4a
C9
C4b
Figure 4. (a) The peak magnitudes of principal stress within the femur, (b) Maximum
interfragmentary movement, (c) Average fluid velocity within the scaffold, and (d)
Average octahedral shear strain within the scaffold.
C4b C5b C6b
0.00000
−0.00012
−0.00024
−0.00036
−0.00048
−0.00060
−0.00072
−0.00087
0.00000
−0.00012
−0.00024
−0.00036
−0.00048
−0.00060
−0.00072
−0.00129
0.00000
−0.00012
−0.00024
−0.00036
−0.00048
−0.00060
−0.00072
(c)(b)
(a)
Figure 5. Distributions of principal strains within the midsection of the proximal femur are shown for (a)
C4b, (b) C5b and (c) C6b constructs. The scale bar is 5 mm.
mechanical environment (Figures 4(b)-(d)). The fully-
screwed construct C1 and the family of C3 (7 and 6-
screw) had the smallest values of u, oct and ε
f
v (av-
erage) among the constructs: ~0.16 mm, 8.76% and 0.05
μm/s for C1, and ~0.2 mm, ~10.6% and ~0.06 μm/s for
the family of C3, respectively (Figures 4(b)-(d)). The
average of the octahedral shear strain within the scaffold
varied between 11.25% - 22.5% for the family constructs
C2 (~12% - 13%), C4 (~17% - 18%), C5 (~19% - 22%)
and C6 (~13% - 14%) (Figure 4(d)). The scaffold in
2-screw constructs C7, C8 and C9 experienced signifi-
cantly higher values of u, and
oct
ε
f
v compared to
other constructs (Figures 4(b)-(d)): 30.65%, 0.182 μm/s
and 0.56 mm for C7, 29.15%, 0.174 μm/s and 0.54 mm
for C8, and 26.83%, 0.16 μm/s and 0.51 mm for C9.
3.2. Simulation of Tissue Differentiation within
the Nominated Constructs
The stress-strain analyses (Figure 4) revealed that
4-screw constructs (C4b, C5b and C6b) had a load-sup-
port capability similar to constructs with higher screw
numbers, and therefore, tissue differentiation was only
predicted within the 4-screw constructs (C4b, C5b and
C6b). Over the 25 steps of simulation, the peak magni-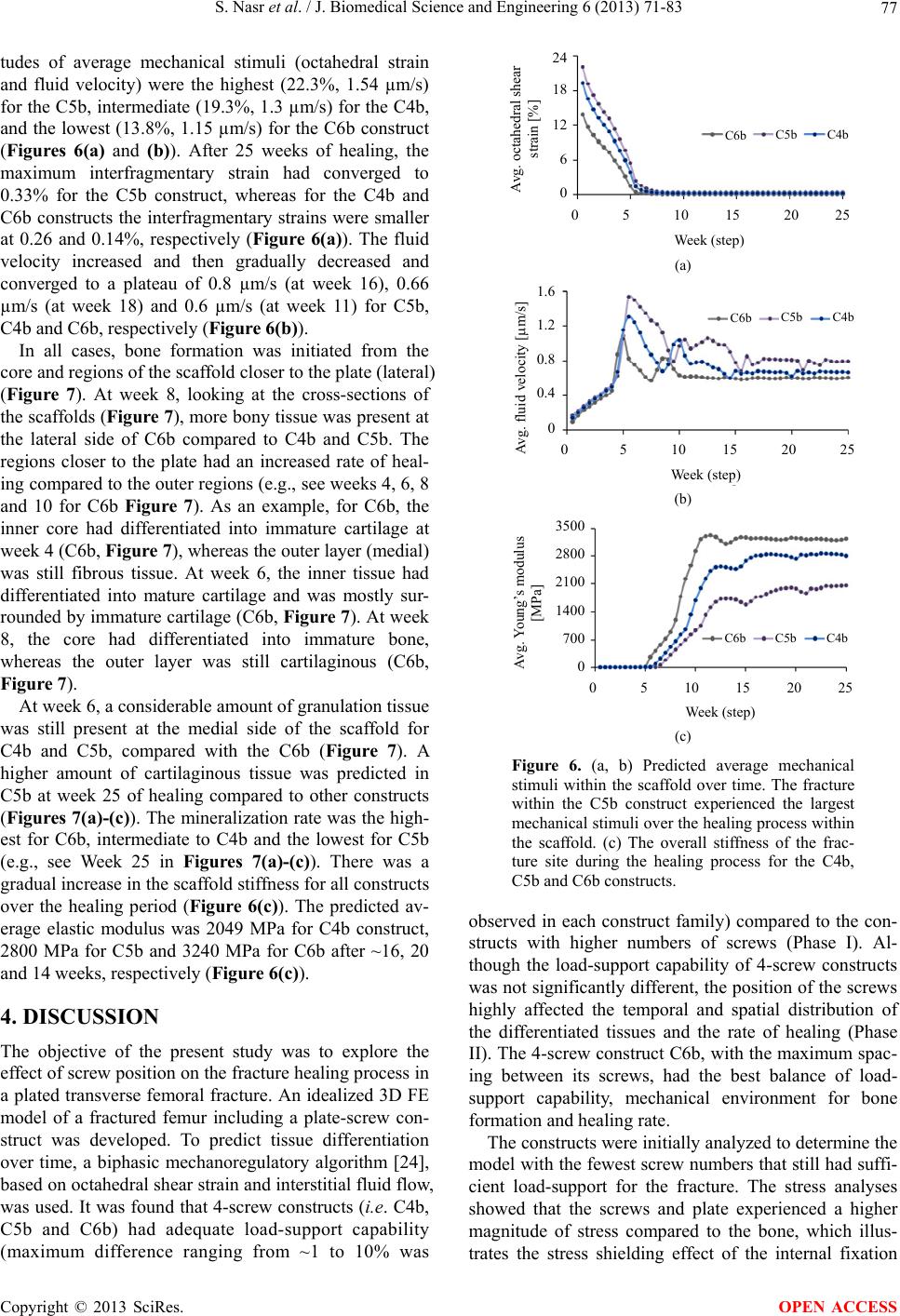

S. Nasr et al. / J. Biomedical Science and Engineering 6 (2013) 71-83 77
tudes of average mechanical stimuli (octahedral strain
and fluid velocity) were the highest (22.3%, 1.54 µm/s)
for the C5b, intermediate (19.3%, 1.3 µm/s) for the C4b,
and the lowest (13.8%, 1.15 µm/s) for the C6b construct
(Figures 6(a) and (b)). After 25 weeks of healing, the
maximum interfragmentary strain had converged to
0.33% for the C5b construct, whereas for the C4b and
C6b constructs the interfragmentary strains were smaller
at 0.26 and 0.14%, respectively (Figure 6(a)). The fluid
velocity increased and then gradually decreased and
converged to a plateau of 0.8 µm/s (at week 16), 0.66
µm/s (at week 18) and 0.6 µm/s (at week 11) for C5b,
C4b and C6b, respectively (Figure 6(b)).
In all cases, bone formation was initiated from the
core and regions of the scaffold closer to the plate (lateral)
(Figure 7). At week 8, looking at the cross-sections of
the scaffolds (Figure 7), more bony tissue was present at
the lateral side of C6b compared to C4b and C5b. The
regions closer to the plate had an increased rate of heal-
ing compared to the outer regions (e.g., see weeks 4, 6, 8
and 10 for C6b Figure 7). As an example, for C6b, the
inner core had differentiated into immature cartilage at
week 4 (C6b, Figure 7), whereas the outer layer (medial)
was still fibrous tissue. At week 6, the inner tissue had
differentiated into mature cartilage and was mostly sur-
rounded by immature cartilage (C6b, Figure 7). At week
8, the core had differentiated into immature bone,
whereas the outer layer was still cartilaginous (C6b,
Figure 7).
At week 6, a considerable amount of granulation tissue
was still present at the medial side of the scaffold for
C4b and C5b, compared with the C6b (Figure 7). A
higher amount of cartilaginous tissue was predicted in
C5b at week 25 of healing compared to other constructs
(Figures 7(a)-(c)). The mineralization rate was the high-
est for C6b, intermediate to C4b and the lowest for C5b
(e.g., see Week 25 in Figures 7(a)-(c)). There was a
gradual increase in the scaffold stiffness for all constructs
over the healing period (Figure 6(c)). The predicted av-
erage elastic modulus was 2049 MPa for C4b construct,
2800 MPa for C5b and 3240 MPa for C6b after ~16, 20
and 14 weeks, respectively (Figure 6(c ) ).
4. DISCUSSION
The objective of the present study was to explore the
effect of screw position on the fracture healing process in
a plated transverse femoral fracture. An idealized 3D FE
model of a fractured femur including a plate-screw con-
struct was developed. To predict tissue differentiation
over time, a biphasic mechanoregulatory algorithm [24],
based on octahedral shear strain and interstitial fluid flow,
was used. It was found that 4-screw constructs (i.e. C4b,
C5b and C6b) had adequate load-support capability
(maximum difference ranging from ~1 to 10% was
24
18
12
6
0
0 5 10 15 20 25
Week (step)
Avg. octahedral shear
strain [%]
C6b C5
b
C4
b
(a)
C6b C5
b
C4
b
0 5 10 15 20 25
Week (step)
Avg. fluid velocity [m/s]
1.6
1.2
0.8
0.4
0
(b)
C6b C5
b
C4
b
0 5 10 15 20 25
Week (step)
3500
2800
2100
1400
700
0
Avg. Young’s modulus
[MPa]
(c)
Figure 6. (a, b) Predicted average mechanical
stimuli within the scaffold over time. The fracture
within the C5b construct experienced the largest
mechanical stimuli over the healing process within
the scaffold. (c) The overall stiffness of the frac-
ture site during the healing process for the C4b,
C5b and C6b constructs.
observed in each construct family) compared to the con-
structs with higher numbers of screws (Phase I). Al-
though the load-support capability of 4-screw constructs
was not significantly different, the position of the screws
highly affected the temporal and spatial distribution of
the differentiated tissues and the rate of healing (Phase
II). The 4-screw construct C6b, with the maximum spac-
ing between its screws, had the best balance of load-
support capability, mechanical environment for bone
formation and healing rate.
The constructs were initially analyzed to determine the
model with the fewest screw numbers that still had suffi-
cient load-support for the fracture. The stress analyses
showed that the screws and plate experienced a higher
magnitude of stress compared to the bone, which illus-
trates the stress shielding effect of the internal fixation

S. Nasr et al. / J. Biomedical Science and Engineering 6 (2013) 71-83 78
Week 4 Week 6 Week 8 Week 10 Week 14 Week 25
lateral
medial
C4b
C5b
C6b
granulation tissue fibrous tissue immature cartilagemature cartilageimmature bone mature bone
Figure 7. The predicted tissue formation pattern within the fracture for C4b, C5b and C6b fixation cases.
In all constructs, tissue differentiation is accelerated at the lateral side of the scaffold closest to the fixa-
tion compared to the medial side. The formation of bony tissue was initiated from the core and lateral
side of the scaffold (see week 8). Scale bar is 5 mm.
and the fact that fixation carried more load. Furthermore,
the screws experienced a higher magnitude of stress
compared to the plate which supports the clinical obser-
vation that screw breakages are more common than plate
failures [55]. This was likely because the screws were in
direct contact with the bone and transferred the load di-
rectly from the bone to the plate. This is also in agree-
ment with the experimental/numerical study of Dubov et
al. (2011) which found screw stresses were ~30% greater
than the plate in the optimal fixation construct. Further-
more, in both studies, the maximum stress in the plate
was observed around the screw-holes in the vicinity of
the gap, and the highest stress within the femur was
found around the bone screw-holes [10]. The maximum
displacement and strain within the scaffold occurred in
the elements farthest from the bone plate (medial). This
is consistent with the linear FE study of Kim et al. (2010)
that also indicated a gradual increase in the strain values
within the scaffold toward the opposite side of the bone
plate [35].
The fixation of a fracture with a fully-screwed plate
(C1) significantly decreased the stress within the scaffold.
However, a fully-screwed bone might be subjected to
stress shielding. The interfragmentary motion is limited,
but this may lead to less bone formation, higher bone
resorption and consequently fixation loosening [13,56,
57]. Furthermore, the direct contact between the screws
and bone might lead to disruption of the blood supply
[58].
No significant difference was observed between the 8
and 7-screw constructs (C1, C2 and C3, Figure 4(a)).
The stress distribution was also insensitive to the posi-
tion of screws in the 8 and 7-screw constructs (C2 and
C3, Figure 4(a)). The magnitude of maximum principal
strain within bone was less than the limit values defined
by the strain criterion [50,54]. Therefore, it was con-
cluded that the number of screws could be reduced.
Four-screw constructs (e.g. C4b and C6b) were found to
have almost the same load-support capability compared
to 5 and 6-screw constructs (e.g. construct family of C4
and C6). The maximum difference between the predicted
maximum von Mises stress within the 6-screw constructs

S. Nasr et al. / J. Biomedical Science and Engineering 6 (2013) 71-83 79
(C4 and C6, with 25% screw omission) and 4-screw con-
structs (C4b and C6b, with 50% screw omission) were
~10 and ~1.3%, respectively. In particular, the construct
C6b better distributed the strains throughout the femur.
These predictions were in general agreement with the
experiments of Field et al. (1999) on cadaveric bones, in
which the omission of 25% - 50% of the total screws did
not affect the structural rigidity [12]. Furthermore, in the
study of Dubov et al. (2011) [10], the construct with the
largest distance between the screws above the fracture
site (with a similar pattern to construct C6b in the present
study) had the best load-support mechanism and showed
minimum stress values in the bone. This was also in
agreement with our results in which the bone in the
4-screw construct C6b experienced the least stress com-
pared to 4-screw constructs C4b and C5b.
In each family of construct, the removal of 25% to
50% of the total number of screws (removal of two to
four screws) did not change considerably the stress dis-
tribution within the femur. However, using fewer screws
resulted in higher strain magnitudes within the fracture
gap which may help the tissue differentiation process.
These findings are in agreement with the studies of Kor-
vick et al. (1988) and Field et al. (1999) that showed
omission of 25% - 50% of screws did not deleteriously
affect the structural rigidity of the bone-plate assembly
[12,59]. However, in certain assemblies, with all screws
situated at both ends of the plate (similar to C4b in the
present study) or with screws located at the ends of the
plate as well as either side of the fracture gap (similar to
C6b in the present study), then higher interfragmentary
movement was observed. In these assemblies, the screw
distribution led to an increase of strain within the fracture
gap which could stimulate tissue differentiation [12,59].
According to the theory of Prendergast et al. (1997), the
octahedral shear strain and fluid velocity to promote fi-
brous tissue differentiation and callus formation must
satisfy this condition: 3
0037 0003
oct
f
εv
..
6
[18,24,60].
The average octahedral shear strain and fluid velocity
within the scaffold of the 4-screw constructs (C4b, C5b
and C6b) were in the proposed range by Lacroix and
Prendergast (Figures 4(c) and (d)). In contrast, the scaf-
fold of the 2-screw constructs (C7, C8 and C9) had much
higher strain magnitudes (26% - 30%, Figures 4(c) and
(d)), which were above the threshold values and thus the
mechanical conditions were not conducive to bone for-
mation. High strain values may cause cell apoptosis and
delay the healing process [38-40]. Furthermore, the
4-screw constructs, will also have less contact surface
compared to the constructs with higher number of screws
(i.e. 5 to 8-screw constructs) and consequently there
would be less risk of blood supply disruption. The pre-
dicted magnitudes of strain and fluid velocity for these
4-scew structures were appropriate for tissue differentia-
tion. Therefore, not only did 4-screw constructs (C4b,
C5b and C6b) with a 50% screw omission have sufficient
structural stability, they also provided favourable me-
chanical environment for bone formation.
Mechanical stimuli and tissue differentiation were pre-
dicted in the 4-screw constructs (C4b, C5b and C6b)
over 25 weeks of healing. The predicted sequence of
tissue regeneration in the femoral fracture model oc-
curred in the same pattern observed in vivo [61,62]; bone
formation was successfully simulated over time with the
sequential prediction of fibrous, cartilage and bony tissue
(endochondral ossification). At the initial stages of tissue
formation, the stiffness of the scaffold was very low
(~0.2 - 10 MPa), and the stress at the fracture site was
relatively low. As an example, in the 4-screw construct
C6b at week 4, the scaffold with the average stiffness of
~4.1 MPa (Figure 6c) had an average von Mises stress
value as ~0.13 MPa. As the scaffold stiffened over time,
the stress carried by the bone was gradually transmitted
to the scaffold, which resulted in an increase in the scaf-
fold stress which decreased the stress shielding effect.
For instance, in the 4-screw construct C6b, at week 8 the
average of von Mises stress was ~0.45 MPa within the
fracture site (average Young’s modulus: ~1189 MPa),
whereas this value was only ~1.15 MPa at week 16 (av-
erage Young’s modulus: ~3250 MPa). In the final stages
of healing, the variations of stress as well as the stiffness
were negligible. For instance, the stiffness of the scaffold
at week 16 (3250 MPa) was not much different than the
stiffness of the scaffold at week 20 (3253 MPa), and
consequently the von Mises stress carried by the fracture
site remained unchanged (~1.15 MPa) between weeks 16
and 20 of healing. The linear FE study of Fouad (2010)
predicted von Mises stresses at the fracture site at differ-
ent healing stages. Consistent with our study, the value of
von Mises stress within the fracture site at 50% healing
was much greater than its value at initial stages of heal-
ing and the stress shielding effect decreased over time.
Furthermore, in agreement with our study, the variation
of von Mises stress and stiffness at the fracture site were
predicted to remain constant at final stages of healing
[13].
The magnitudes of local mechanical stimuli regulated
the healing pathways within different regions of the scaf-
fold. The differentiation of the bony tissue initiated from
the regions that experienced less mechanical stimuli (e.g.
the core and lateral side of the scaffold, Figure 7). Due
to the plate-screw construct and model asymmetry, the
geometric center of the bone shifted toward the plate.
Therefore, the axial compressive load resulted in a mar-
ginal moment to the bone which was greater at the outer
layer of the scaffold (medial). Hence, the outer layer of
the scaffold was exposed to a higher range of mechanical

S. Nasr et al. / J. Biomedical Science and Engineering 6 (2013) 71-83 80
stimuli and the time for the bone to heal was longer in
that region. For instance, at week 4, the octahedral shear
strain and fluid velocity were 5.3% and 0.4 µm/s, respec-
tively, for an outer layer sample element, whereas these
values were 2.1% and 0.1 µm/s at the core. These predic-
tions are in agreement with the X-ray observations of
Fan et al. (2008) in which at 4 weeks after operation a
stiffer callus was observed in the regions closer to the
plate compared to the regions at the opposite side [63].
Furthermore, the histological slides from Uhthoff et al.
(1983), in which the structural remodelling of 27 frac-
tured femora was investigated, revealed that the osteons
seemed more prominent under the stainless steel plates
[64].
The general trend of tissue differentiation within the
fracture was similar in the present study compared to the
FE studies of Son et al. (2013) and Kim et al. (2012) that
used an internal fixation for an idealized 3D long bone
fracture. In the above-mentioned models, bone was sub-
jected to a cyclic axial compression load at one end,
while the other end was fixed. Similar to our predictions,
bone healing was accelerated in the elements located
closer to the plate and was delayed in the elements far-
ther from the plate. Moreover, in agreement with the
present study, the core of the scaffold was surrounded by
softer tissues over the healing period. However, the spa-
tial and temporal distributions were not identical, and
overall, the healing was faster in their simulations com-
pared to ours. The first difference that might have caused
these variations was the use of different loading regimes.
In our analysis, the applied load was 50% of the body
weight, whereas in their studies it ranged between 10% -
300% of the body weight. Secondly, in the current study,
poroelastic material properties and a biphasic mech-
anoregulatory algorithm were implemented into our FE
model, which means that the effect of fluid velocity was
taken into account and tissue differentiation was reduced
where fluid velocity was too high. On the other hand,
linear elastic material properties and a single-solid phase
algorithm, based solely on the strain, were used in the
studies of Son et al. (2013) and Kim et al. (2012) to pre-
dict tissue differentiation. Hence, the effect of fluid ve-
locity was neglected, which might have resulted in the
faster healing rate [17].
The mechanical stimuli and consequently the healing
progression were affected by the position of the screws
(Figure 7). Among the 4-screw constructs, C6b had the
highest healing rate, C4b intermediate and C5b the
slowest (Figure 7). The delayed healing in 4-screw con-
struct C5b, with screws placed in the first, third, sixth
and eighth screw holes, might result from lower stability,
higher interfragmentary movement of the fracture gap,
and higher mechanical stimuli within the scaffold over
the healing period (Figures 6(a) and (b)). The predicted
temporal stiffness of the scaffold again suggests that the
gap in C6b, with the highest Young’s modulus, was
bridged more rapidly compared to C4b and C5b (Figure
6(c)). The stiffness of the scaffold in C6b converged to a
plateau after 14 weeks of healing (3240 MPa for C6b);
however, for C4b and C5b it took 2 and 6 weeks longer
(2049 MPa for C4b and 2800 MPa for C5b), respectively.
The scaffold stiffness in our simulations (2049 MPa for
C4b, 2800 MPa for C5b and 3240 MPa for C6b) and the
stiffness predicted by Kim et al. (2012) using a stainless
steel plate were in a similar range (~1750 MPa). The
difference in the values may result from the fact that
lower magnitudes of load were used in our simulations,
which might lead to greater bone formation and a stiffer
scaffold [20]. In other words, the higher stiffness in our
models might be due to lower load magnitude (50% BW)
and consequently lower mechanical stimuli compared to
Kim et al. where the bone was subjected to a load of
100% BW.
There were limitations associated with our computa-
tional study. The 3D idealized model used did not fully
represent the exact geometry and loading on the femur.
For instance, due to the natural curvature of the femur,
the axial compression load applied to the cortical shaft
induces combined compression and bending strains.
Therefore, to ensure more accurate distributions of the
tissue strain and stress, a 3D CT based FE model could
be reconstructed in future studies. Moreover, only axial
compressive load was applied to the model, whereas the
simulation would be more realistic, if the forces and
moments from the surrounding muscles were considered
(e.g., better mimic a walking condition). The standard
clinical treatment protocol would be none or partial
weight bearing for 6 - 12 weeks followed by full weight
bearing. However, only a simple cyclic load was used
since there is no clear consensus among surgeons, and
weight bearing is often evaluated by the radiographic
appearance of fracture. A simplified loading protocol
allowed us to highlight the pure mechanical differences
between constructs without the confounding effects of
progressive weight bearing. In future research, we will
focus on the experimental validation of the computa-
tional studies using gene expression patterns and high-
resolution μCT images of mineralization patterns.
5. CONCLUSION
This computational study has shown that the healing
progression was greatly affected by the stability of the
bone and the position of the screws. It was found that the
symmetrical omission of 50% of the screws had almost
the same load-bearing capability as the constructs with
higher screw number. The 4-screw symmetrical construct
C6b, with the largest distance among the screws, of-

S. Nasr et al. / J. Biomedical Science and Engineering 6 (2013) 71-83 81
fered the best bone-healing potential with sufficient sta-
bility at the fracture site. Using fewer screws provided
the advantage of less damage to blood supply with an
appropriate mechanical environment for tissue differen-
tiation. Furthermore, the temporal increase of stress
within the C6b scaffold (stiffness) may decrease the
stress shielding effect and prevent focal bone loss and
osteoporosis. It is our expectation that clinical applica-
tion of the principles identified in this study may lead to
less invasive surgical techniques that can maximize me-
chanical performance in the setting of minimal soft tissue
injury.
6. ACKNOWLEDGEMENTS
Funding for this study was provided by the Natural Sciences and Engi-
neering Research Council of Canada (NSERC).
REFERENCES
[1] Rockwood, C.A., Green, D.P. and Bucholz, R.W. (2010)
Rockwood and Green’s fractures in adults. Wolters Klu-
wer Health/Lippincott, Williams & Wilkins, Philadelphia.
[2] Howard, A. and Giannoudis, P.V. (2012) Proximal fe-
moral fractures: Issues and challenges. Injury, 43, 1975-
1977. http://dx.doi.org/10.1016/j.injury.2012.09.013
[3] Sabharwal, S., Kishan, S. and Behrens, F. (2005) Princi-
ples of external fixation of the femur. American Journal
of Orthopedics (Belle Mead NJ), 34, 218-223.
[4] Papini, M., Zdero, R., Schemitsch, E.H. and Zalzal, P.
(2007) The biomechanics of human femurs in axial and
torsional loading: Comparison of finite element analysis,
human cadaveric femurs, and synthetic femurs. Journal
of Biomechanical Engineering, 129, 12-19.
http://dx.doi.org/10.1115/1.2401178
[5] Heineman, D.J., Poolman, R.W., Nork, S.E., Ponsen, K.J.
and Bhandari, M. (2010) Plate fixation or intramedullary
fixation of humeral shaft fractures. Acta Orthopaedica,
81, 216-223.
http://dx.doi.org/10.3109/17453671003635884
[6] Einhorn, T.A. (1998) One of nature’s best kept secrets.
Journal of Bone and Mineral Research, 13, 10-12.
http://dx.doi.org/10.1359/jbmr.1998.13.1.10
[7] Egermann, M., Goldhahn, J. and Schneider, E. (2005)
Animal models for fracture treatment in osteoporosis,
Osteoporosis International, 16, S129-S138.
http://dx.doi.org/10.1007/s00198-005-1859-7
[8] Souna, B.S., Ganda, S., Amadou, S. and Abdoulaye, A.
(2008) The treatment of tibia open fractures by Hoffmann
external fixation in Niamey. About 50 cases. Mali Médi-
cal, 23, 11-15.
[9] Putnam, M.D. and Walsh, T.M.t. (1993) External fixation
for open fractures of the upper extremity. Hand Clinics, 9,
613-623.
[10] Dubov, A., Kim, S.Y., Shah, S., Schemitsch, E.H., Zdero,
R. and Bougherara, H. (2011) The biomechanics of plate
repair of periprosthetic femur fractures near the tip of a
total hip implant: The effect of cable-screw position. Pro-
ceedings of the Institution of Mechanical Engineers, Part
H, 225, 857-865.
http://dx.doi.org/10.1177/0954411911410642
[11] Taljanovic, M.S., Jones, M.D., Ruth, J.T., Benjamin, J.B.,
Sheppard, J.E. and Hunter, T.B. (2003) Fracture fixation.
Radiographics, 23, 1569-1590.
http://dx.doi.org/10.1148/rg.236035159
[12] Field, J.R., Tornkvist, H., Hearn, T.C., Sumner-Smith, G.
and Woodside, T.D. (1999) The influence of screw omis-
sion on construction stiffness and bone surface strain in
the application of bone plates to cadaveric bone. Injury,
30, 591-598.
http://dx.doi.org/10.1016/S0020-1383(99)00158-8
[13] Fouad, H. (2010) Effects of the bone-plate material and
the presence of a gap between the fractured bone and
plate on the predicted stresses at the fractured bone. Me-
dical Engineering & Physics, 32, 783-789.
http://dx.doi.org/10.1016/j.medengphy.2010.05.003
[14] Ramakrishna, K., Sridhar, I., Sivashanker, S., Khong, K.S.
and Ghista, D.N. (2004) Design of fracture fixation plate
for necessary and sufficient bone stress shielding. JSME
International Journal Series C Mechanical Systems, Ma-
chine Elements and Manufacturing, 47, 1086-1094.
http://dx.doi.org/10.1299/jsmec.47.1086
[15] Ganesh, V.K., Ramakrishna, K. and Ghista, D.N. (2005)
Biomechanics of bone-fracture fixation by stiffness-
graded plates in comparison with stainless-steel plates.
BioMedical Engineering OnLine, 4, 46.
http://dx.doi.org/10.1186/1475-925X-4-46
[16] Carter, D.R., Vasu, R., Spengler, D.M. and Dueland, R.T.
(1981) Stress fields in the unplated and plated canine fe-
mur calculated from in vivo strain measurements. Journal
of Biomechanics, 14, 63-70.
http://dx.doi.org/10.1016/0021-9290(81)90081-6
[17] Isaksson, H., Wilson, W., van Donkelaar, C.C., Huiskes,
R. and Ito, K. (2006) Comparison of biophysical stimuli
for mechano-regulation of tissue differentiation during
fracture healing. Journal of Biomechanics, 39, 1507-1516.
http://dx.doi.org/10.1016/j.jbiomech.2005.01.037
[18] Prendergast, P.J., Huiskes, R. and Soballe, K. (1997) ESB
Research Award 1996. Biophysical stimuli on cells dur-
ing tissue differentiation at implant interfaces. Journal of
Biomechanics, 30, 539-548.
http://dx.doi.org/10.1016/S0021-9290(96)00140-6
[19] Zhang, P. and Yokota, H. (2011) Knee loading stimulates
healing of mouse bone wounds in a femur neck. Bone, 49,
867-872.
[20] Gardner, M.J., van der Meulen, M.C., Demetrakopoulos,
D., Wright, T.M., Myers, E.R. and Bostrom, M.P. (2006)
In vivo cyclic axial compression affects bone healing in
the mouse tibia. Journal of Orthopaedic Research, 24,
1679-1686. http://dx.doi.org/10.1002/jor.20230
[21] Carter, D.R., Blenman, P.R. and Beaupre, G.S. (1988)
Correlations between mechanical stress history and tissue
differentiation in initial fracture healing. Journal of Or-
thopaedic Research, 6, 736-748.
http://dx.doi.org/10.1002/jor.1100060517
[22] Gardner, T.N., Stoll, T., Marks, L., Mishra, S. and Kno-

S. Nasr et al. / J. Biomedical Science and Engineering 6 (2013) 71-83 82
the Tate, M. (2000) The influence of mechanical stimulus
on the pattern of tissue differentiation in a long bone
fracture—An FEM study. Journal of Biomechanics, 33,
415-425.
[23] Sandino, C. and Lacroix, D. (2011) A dynamical study of
the mechanical stimuli and tissue differentiation within a
CaP scaffold based on micro-CT finite element models.
Biomechanics and Modeling in Mechanobiology, 10,
565-576. http://dx.doi.org/10.1007/s10237-010-0256-0
[24] Lacroix, D. and Prendergast, P.J. (2002) A mechano-
regulation model for tissue differentiation during fracture
healing: Analysis of gap size and loading. Journal of Bio-
mechanics, 35, 1163-1171.
http://dx.doi.org/10.1016/S0021-9290(02)00086-6
[25] Isaksson, H., van Donkelaar, C.C., Huiskes, R. and Ito, K.
(2006) Corroboration of mechanoregulatory algorithms
for tissue differentiation during fracture healing: Com-
parison with in vivo results. Journal of Orthopaedic Re-
search, 24, 898-907. http://dx.doi.org/10.1002/jor.20118
[26] Isaksson, H., van Donkelaar, C.C., Huiskes, R. and Ito, K.
(2008) A mechano-regulatory bone-healing model incur-
porating cell-phenotype specific activity. Journal of The-
oretical Biology, 252, 230-246.
[27] Checa, S. and Prendergast, P.J. (2009) A mechanobiolo-
gical model for tissue differentiation that includes angio-
genesis: A lattice-based modeling approach. Annals of
Biomedical Engineering, 37, 129-145.
http://dx.doi.org/10.1007/s10439-008-9594-9
[28] Checa, S. and Prendergast, P.J. (2010) Effect of cell seed-
ing and mechanical loading on vascularization and tissue
formation inside a scaffold: A mechano-biological model
using a lattice approach to simulate cell activity. Journal
of Biomechanics, 43, 961-968.
[29] McMahon, L.A., O’Brien, F.J. and Prendergast, P.J.
(2008) Biomechanics and mechanobiology in osteochon-
dral tissues. Regenerative Medicine, 3, 743-759.
http://dx.doi.org/10.2217/17460751.3.5.743
[30] Nagel, T. and Kelly, D.J. (2010) Mechano-regulation of
mesenchymal stem cell differentiation and collagen or-
ganisation during skeletal tissue repair. Biomechanics and
Modeling in Mechanobiology, 9, 359-372.
http://dx.doi.org/10.1007/s10237-009-0182-1
[31] Perez, M.A. and Prendergast, P.J. (2007) Random-walk
models of cell dispersal included in mechanobiological
simulations of tissue differentiation. Journal of Biome-
chanics, 40, 2244-2253.
[32] Prendergast, P.J., Checa, S. and Lacroix, D. (2010) Com-
putational models of tissue differentiation. Springer
Netherlands, Dordrecht, 353-372.
[33] Mehboob, H., Son, D.S. and Chang, S.H. (2013) Finite
element analysis of tissue differentiation process of a
tibia with various fracture configurations when a com-
posite intramedullary rod was applied. Composites Sci-
ence and Technology, 80, 55-65.
http://dx.doi.org/10.1016/j.compscitech.2013.02.020
[34] Son, D.S. and Chang, S.H. (2013) The simulation of bone
healing process of fractured tibia applied with composite
bone plates according to the diaphyseal oblique angle and
plate modulus. Composites Part B: Engineering, 45,
1325-1335.
http://dx.doi.org/10.1016/j.compositesb.2012.07.037
[35] Kim, H.J., Chang, S.H. and Jung, H.J. (2012) The simu-
lation of tissue differentiation at a fracture gap using a
mechano-regulation theory dealing with deviatoric strains
in the presence of a composite bone plate. Composites
Part B: Engineering, 43, 978-987.
http://dx.doi.org/10.1016/j.compositesb.2011.09.011
[36] Egol, K.A., Kubiak, E.N., Fulkerson, E., Kummer, F.J.
and Koval, K.J. (2004) Biomechanics of locked plates
and screws. Journal of Orthopaedic Trauma, 18, 488-493.
http://dx.doi.org/10.1097/00005131-200409000-00003
[37] Kumar, A., Gupta, H., Yadav, C.S., Khan, S.A. and Ras-
togi, S. (2013) Role of locking plates in treatment of dif-
ficult ununited fractures: A clinical study. Chinese Jour-
nal of Traumatology, 16, 22-26.
[38] Kwong, F.N. and Harris, M.B. (2008) Recent develop-
ments in the biology of fracture repair. The Journal of the
American Academy of Orthopaedic Surgeons, 16, 619-
625.
[39] Geris, L., Vandamme, K., Naert, I., Vander Sloten, J., Duyck,
J. and Van Oosterwyck, H. (2009) Numerical simulation
of bone regeneration in a bone chamber. Journal of Den-
tal Research, 88, 158-163.
[40] Einhorn, T.A. (2005) The science of fracture healing.
Journal of Orthopaedic Trauma, 19, S4-S6, 158-163.
[41] Miclau, T. and Martin, R.E. (1997) The evolution of
modern plate osteosynthesis. Injury, 28, A3-A6.
http://dx.doi.org/10.1016/S0020-1383(97)90109-1
[42] Karnezis, I.A., Miles, A.W., Cunningham, J.L. and Lear-
month, I.D. (1998) “Biological” internal fixation of long
bone fractures: A biomechanical study of a “noncontact”
plate system. Injury, 29, 689-695.
http://dx.doi.org/10.1016/S0020-1383(98)00168-5
[43] Edwards, W.B., Gillette, J.C., Thomas, J.M. and Derrick,
T.R. (2008) Internal femoral forces and moments during
running: Implications for stress fracture development. Cli-
nical Biomechanics, 23, 1269-1278.
http://dx.doi.org/10.1016/j.clinbiomech.2008.06.011
[44] Taylor, M.E., Tanner, K.E., Freeman, M.A. and Yettram,
A.L. (1996) Stress and strain distribution within the intact
femur: Compression or bending? Medical Engineering &
Physics, 18, 122-131.
http://dx.doi.org/10.1016/1350-4533(95)00031-3
[45] Aranzulla, P.J., Muckle, D.S. and Cunningham, J.L. (1998)
A portable monitoring system for measuring weight-bear-
ing during tibial fracture healing. Medical Engineering &
Physics, 20, 543-548.
http://dx.doi.org/10.1016/S1350-4533(98)00061-7
[46] Riemer, B.L., Foglesong, M.E. and Miranda, M.A. (1994)
Femoral plating. The Orthopedic Clinics of North Ameri-
ca, 25, 625-633.
[47] Paterno, M.V. and Archdeacon, M.T. (2009) Is there a
standard rehabilitation protocol after femoral intramedul-
lary nailing? Journal of Orthopaedic Trauma, 23, S39-
S46. http://dx.doi.org/10.1097/BOT.0b013e31819f27c2
[48] Johnson, M.W., Chakkalakal, D.A., Harper, R.A., Katz,
J.L. and Rouhana, S.W. (1982) Fluid flow in bone in vi-

S. Nasr et al. / J. Biomedical Science and Engineering 6 (2013) 71-83 83
tro. Journal of Biomechanics, 15, 881-885.
http://dx.doi.org/10.1016/0021-9290(82)90054-9
[49] Schaffler, M.B. and Burr, D.B. (1988) Stiffness of com-
pact bone: Effects of porosity and density. Journal of
Biomechanics, 21, 13-16.
http://dx.doi.org/10.1016/0021-9290(88)90186-8
[50] Schileo, E., Taddei, F., Cristofolini, L. and Viceconti, M.
(2008) Subject-specific finite element models implement-
ing a maximum principal strain criterion are able to esti-
mate failure risk and fracture location on human femurs
tested in Vitro. Journal of Biomechanics, 41, 356-367.
http://dx.doi.org/10.1016/j.jbiomech.2007.09.009
[51] Currey, J.D. (2012) The structure and mechanics of bone.
Journal of Materials Science, 47, 41-54.
http://dx.doi.org/10.1007/s10853-011-5914-9
[52] Jepsen, K.J. and Andarawis-Puri, N. (2012) The amount
of periosteal apposition required to maintain bone strength
during aging depends on adult bone morphology and tis-
sue-modulus degradation rate. Journal of Bone and Min-
eral Research, 27, 1916-1926.
http://dx.doi.org/10.1002/jbmr.1643
[53] Boskey, A.L. and Coleman, R. (2010) Aging and bone.
Journal of Dental Research, 89, 1333-1348.
http://dx.doi.org/10.1177/0022034510377791
[54] Bayraktar, H.H., Morgan, E.F., Niebur, G.L., Morris,
G.E., Wong, E.K. and Keaveny, T.M. (2004) Comparison
of the elastic and yield properties of human femoral tra-
becular and cortical bone tissue. Journal of Biomechanics,
37, 27-35.
http://dx.doi.org/10.1016/S0021-9290(03)00257-4
[55] Stoffel, K., Dieter, U., Stachowiak, G., Gachter, A. and
Kuster, M.S. (2003) Biomechanical testing of the LCP-
how can stability in locked internal fixators be controlled?
Injury, 34, 11-19.
http://dx.doi.org/10.1016/j.injury.2003.09.021
[56] Uhthoff, H.K., Poitras, P. and Backman, D.S. (2006)
Internal plate fixation of fractures: Short history and re-
cent developments. Journal of Orthopaedic Science, 11,
118-126. http://dx.doi.org/10.1007/s00776-005-0984-7
[57] Huiskes, R., Weinans, H., Grootenboer, H.J., Dalstra, M.,
Fudala, B. and Slooff, T.J. (1987) Adaptive bone-remo-
deling theory applied to prosthetic-design analysis. Jour-
nal of Biomechanics, 20, 1135-1150.
http://dx.doi.org/10.1016/0021-9290(87)90030-3
[58] Noor, S., Pridham, C., Fawcett, T., Barclay, M., Feng, Y.
T., Hassan, O. and Pallister, I. (2013) Finite element ana-
lysis modelling of proximal femoral fractures, including
post-fixation periprosthetic fractures. Injury, 44, 791-795.
http://dx.doi.org/10.1016/j.injury.2012.10.023
[59] Korvick, D.L., Monville, J.D., Pijanowski, G.J. and Phil-
lips, J.W. (1988) The effects of screw removal on bone
strain in an idealized plated bone model. Veterinary Sur-
gery, 17, 111-116.
http://dx.doi.org/10.1111/j.1532-950X.1988.tb00288.x
[60] Lacroix, D. (2000) Simulation of tissue differentiation
during fracture healing. Ph.D. Dessertation, University of
Dublin, Dublin.
[61] Bailon-Plaza, A. and van der Meulen, M.C. (2001) A
mathematical framework to study the effects of growth
factor influences on fracture healing. Journal of Theore-
tical Biology, 212, 191-209.
http://dx.doi.org/10.1006/jtbi.2001.2372
[62] Gerstenfeld, L.C., Cullinane, D.M., Barnes, G.L., Graves,
D.T. and Einhorn, T.A. (2003) Fracture healing as a
post-natal developmental process: Molecular, spatial, and
temporal aspects of its regulation. Journal of Cellular
Biochemistry, 88, 873-884.
http://dx.doi.org/10.1002/jcb.10435
[63] Fan, Y., Xiu, K., Duan, H. and Zhang, M. (2008) Bio-
mechanical and histological evaluation of the application
of biodegradable poly-L-lactic cushion to the plate inter-
nal fixation for bone fracture healing. Clinical Biome-
chanics, 23, S7-S16.
http://dx.doi.org/10.1016/j.clinbiomech.2008.01.005
[64] Uhthoff, H.K. and Finnegan, M. (1983) The effects of me-
tal plates on post-traumatic remodelling and bone mass.
The Journal of Bone and Joint Surgery, 65, 66-71.