 Open Journal of Nephrology, 2013, 3, 194-204 Published Online December 2013 (http://www.scirp.org/journal/ojneph) http://dx.doi.org/10.4236/ojneph.2013.34034 Open Access OJNeph Ratio of Capacitance/BMI Reflects Deficit in Nutritional Concentration While CH2 Reflects Total Nutritional Deficit in CAPD Patients and General Population *# Keng-Hee Koh1,2†, Hin-Seng Wong1,3 1Department of Nephrology, Kuala Lumpur Hospital, Kuala Lumpur, Malaysia 2Department of Medicine, Miri Hospital, Miri, Malaysia 3Department of Nephrology, Selayang Hospital, Selayang, Malaysia Email: †kohkenghee@yahoo.com, †kohkh@moh.gov.my Received September 20, 2013; revised October 15, 2013; accepted November 12, 2013 Copyright © 2013 Keng-Hee Koh, Hin-Seng Wong. This is an open access article distributed under the Creative Commons Attribu- tion License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited. ABSTRACT Traditionally phase angle was the best predictor in BIA for nutrition and survival in dialysis population. We recently showed that normalized bioimpedance indices are a better risk discriminator for dialysis patients and the general popu- lation. We hereby aimed to explore discriminating factors behind them. Methods: We assessed the body capacitive index (BCI = Capacitance × Height2/Weight); body resistive index (BRI = Resistance × Weight/Height2); and also, CH2 (= Capacitance × Height2) which represents total body capacitive volume in physics. We initially performed BIA for 206 female, 116 male healthy volunteers, followed by, prospective study for 128 CAPD patients (47 diabetes mellitus (DM), 81 non-DM; 59 male, 69 female) for >2 years. Results: Moderately good negative correlation of albumin and BCI (r = −0.533, p < 0.001) with linear regression (BCI = 8.780 − 0.184 × [albumin], R2 = 0.339, p < 0.001) was shown in CAPD patients. BCI and CH2 were much higher in CAPD patients in comparison to healthy volunteers (3.4 ± 0.1 vs 2.0 ± 0.0 nFm2/kg, p < 0.001 and 203 ± 8 vs 125 ± 1 nFm2, p < 0.001, respectively). In age and gender adjusted logistic regression model of 128 CAPD patients versus 322 healthy volunteers, the best risk discriminator was BCI (X2 = 165.6), followed by CH2 (X2 = 140), phase angle (X2 = 59.3) and BRI (X2 = 52.2). Thirty five (27.3%) patients died during the study period (Fatal cause: infection (54%), cardiovascular (26%)). In Cox regression, CH2 (2 4 = 32.4) was the best predictor for all-cause mortality, followed by BCI (2 4 = 27.7) and phase angle (2 4 = 19.3). Conclusion: The phase angle was a compound parameter of the body capacity index (BCI) and body resistive index (BRI). BCI has a moder- ately good negative correlation with albumin and this supports its role in reflecting the severity of malnutrition in CAPD patients. CH2 represents total nutrition deficit and thus the major risk indicator for the survival of CAPD pa- tients. Keywords: Bioimpedance Analysis; Continuous Ambulatory Peritoneal Dialysis; Comparison with General Population; Nutrition; Survival 1. Introduction Bioimpedance analysis (BIA) has been recognized as a powerful tool to assess nutrition and hydration status in patients on CAPD [1-4] with standardized methodology [5,6] and various resistive indexes have been described [7-9]. We have recently shown that normalized capaci tive indices (e.g., Capacitance/Body Mass Index and Ca- pacity × Height2) carried more predictive value than non-normalized parameter like the phase angle [10]. Here we aim to further explore the physics rationale of such parameters by analyzing our cohort of CAPD patients and the general population. Gender differences in non normalized bioimpedance parameters (e.g., phase angle and resistive index) have been reported [11,12]. However we have demonstrated that these gender differences disappeared when normal- *Conflict of interest statement: None declared. #This paper has not been published previously in whole or part, except in abstract format; Grant support: none. †Corresponding author.  K.-H. KOH, H.-S. WONG 195 ized bioimpendance parameters (e.g., body capacitive index) were used [10]. The higher body resistive index (BRI) in female normal subjects is probably due to the higher percentage of fat which resulted in the lower pha- se angle [10]. This current study serves to further verify the roles of varying normalized bioimpedance parameters with vari- ous analytical models in line with current thought of translating physiological science into clinical practice [13], as well as exploring the underlying risk discrimi- nating factor. 2. Methods 2.1. Study Design and Population We initially surveyed the bioimpedance profile of the general population by recruiting 322 healthy volunteers as representation of normal population in the neighbour- hood of our hospital. We then prospectively studied all stable CAPD patients (a total of 128 patients) in Kuala Lumpur Hospital. These patients were performing 4 ex- changes a day with 3 exchanges during daytime and a long dwell at night. The study subjects were followed up for 2.2 to 2.3 years. 2.2. Bioelectric Impedance Analysis (BIA) BIA was performed at time of enrolment, with Bioelec- trical Impedance Analyzer, Maltron Bioscan 916 v3, with single frequency 50 kHz with alternating sinusoidal cur- rent, 0.7 mA on all CAPD patients with emptied perito- neal cavity in tetrapolar placement on the hand and foot. After the patient drained off the dialysate and was in a supine position for at least 10 minutes, the standard tetra- polar electrodes were placed on the dorsum of the wrist and anterior aspect of the ankle on the left side of the bo- dy [1]. Bioimpedance measurements obtained at the time of enrollment were used in the predictive model for this study. The clinical value of BIA indexes has been postulated in previous study [7,8]. We derived body resistive index (BRI), body capacitive index (BCI), CH2 or H2/XC with the formulae from A pp en dix A. Fat free mass, fat mass and fat percentage were de- rived with equation of Fat Mass = Weight − Fat Free Mass and fat percentage = Fat Mass / Weight × 100%, in which calculation of fat free mass were as described in past literature by Kotler et al. [14]. 2.3. Statistical Methods The statistical data were analyzed using Microsoft excel and SPSS (Statistical package for Social Science. SPSS Inc. 233 South Wacker Drive, 11th Floor, Chicago, Illi- nois 60606-6307). Kolmogorov Smirnov test was ini- tially used to determine whether the data is in statistical normal distribution. Logarithm transformation would be performed to achieve statistical normal distribution in data with non-normal distrubution. Parametric test would be performed in data with normal distribution. In pa- rameter that could not achieve normal distribution even with logarithm transformation with low number of ana- lyzed subjects, non-parametric test would be utilized. Logistic regression was used to identify the best risk discriminator among BIA parameters between Malaysian normal population and CAPD cohort in Table 1. All means were presented with ±standard error of mean (± SEM). Demographic features, blood investiga- tions, peritoneum equilibrium test, anthropometry and BIA parameters of CAPD patients were studied to iden- tify the risk factors for mortality in Table 2. Univariate analysis was performed with parametric test (e.g., student t-test, ANOVA) for survival comparison in data with statistical normal distribution, whereas non-parametric test (e.g., Mann Whitney U test) would be used in others. Factors that significantly affect the predictor and survival were analyzed with ANCOVA test. In study of correlation relationship between data, Spearman correlation was used as non-parametric test. Cox regression was performed to analyse the significance of the relevant clinical parameters in survival (Table 3). This was a prospective observational study of clinical practice, applying BIA and other investigations in CAPD patients. The protocol was in consistence with the princi- ples of the Declaration of Helsinki as amended in Tokyo (1975), Venice (1983), and Hong Kong (1989) [15]. 3. Results 3.1. Healthy Volunteers We enrolled 322 healthy volunteers, i.e., 206 female and 116 male healthy volunteers. They consist of 178 Malays, 89 Chineses, 54 Indians and 2 of other ethnicity. Their age ranged from 16 to 68 years old with the median of 38 years. 3.2. BIA Parameters of CAPD Patients and Correlation with Serum Albumin We subsequently enrolled 128 CAPD patients with eth- nicity composed of 64 Malays, 50 Chinese and 14 Indi- ans. Aetiology of renal failure included diabetes mellitus (43 patients); chronic glomerulonephritis (27 patients); hypertension (10 patients); obstructive uropathy (6 pa- tients); adult polycystic kidney disease (1 patient); con- current diabetes nephropathy and obstructive uropathy (4 patients); while 37 patients have unknown cause of renal failure. For the CAPD patients, albumin level has the best correlation with BCI (r = −0.533, p < 0.001), followed by phase angle (r = 0.520, p < 0.001), capacitance (r = −0.457, p < 0.001), XC/H (r = 0.426, p < 0.001) and CH2 (−0.386, r < 0.001). Open Access OJNeph 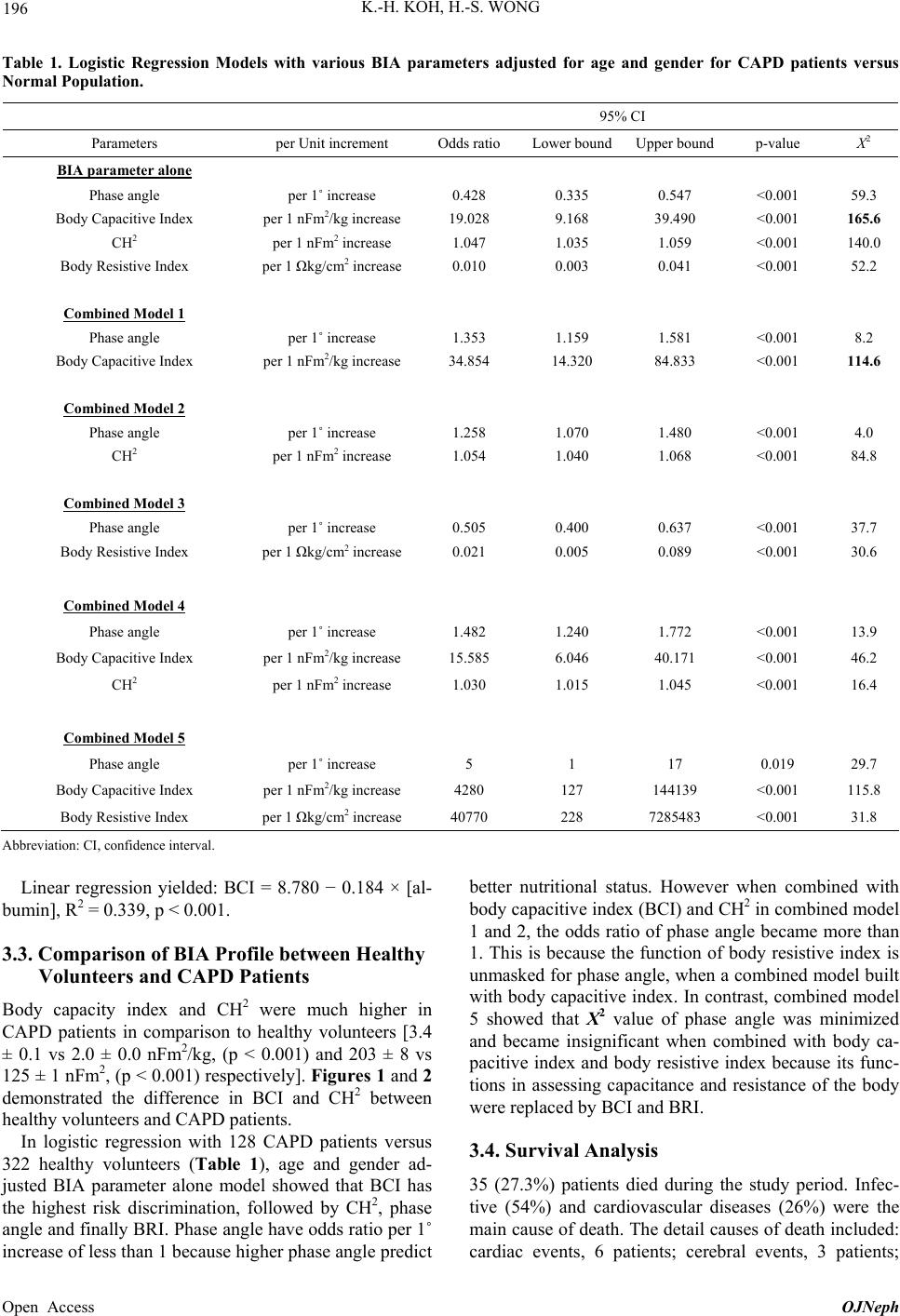 K.-H. KOH, H.-S. WONG Open Access OJNeph 196 Table 1. Logistic Regression Models with various BIA parameters adjusted for age and gender for CAPD patients versus Normal Population. 95% CI Parameters per Unit increment Odds ratio Lower boundUpper bound p-value X2 BIA parameter alone Phase angle per 1˚ increase 0.428 0.335 0.547 <0.001 59.3 Body Capacitive Index per 1 nFm2/kg increase 19.028 9.168 39.490 <0.001 165.6 CH2 per 1 nFm2 increase 1.047 1.035 1.059 <0.001 140.0 Body Resistive Index per 1 Ωkg/cm2 increase 0.010 0.003 0.041 <0.001 52.2 Combined Model 1 Phase angle per 1˚ increase 1.353 1.159 1.581 <0.001 8.2 Body Capacitive Index per 1 nFm2/kg increase 34.854 14.320 84.833 <0.001 114.6 Combined Model 2 Phase angle per 1˚ increase 1.258 1.070 1.480 <0.001 4.0 CH2 per 1 nFm2 increase 1.054 1.040 1.068 <0.001 84.8 Combined Model 3 Phase angle per 1˚ increase 0.505 0.400 0.637 <0.001 37.7 Body Resistive Index per 1 Ωkg/cm2 increase 0.021 0.005 0.089 <0.001 30.6 Combined Model 4 Phase angle per 1˚ increase 1.482 1.240 1.772 <0.001 13.9 Body Capacitive Index per 1 nFm2/kg increase 15.585 6.046 40.171 <0.001 46.2 CH2 per 1 nFm2 increase 1.030 1.015 1.045 <0.001 16.4 Combined Model 5 Phase angle per 1˚ increase 5 1 17 0.019 29.7 Body Capacitive Index per 1 nFm2/kg increase 4280 127 144139 <0.001 115.8 Body Resistive Index per 1 Ωkg/cm2 increase 40770 228 7285483 <0.001 31.8 Abbreviation: CI, confidence interval. Linear regression yielded: BCI = 8.780 − 0.184 × [al- bumin], R2 = 0.339, p < 0.001. 3.3. Comparison of BIA Profile between Healthy Volunteers and CAPD Patients Body capacity index and CH2 were much higher in CAPD patients in comparison to healthy volunteers [3.4 ± 0.1 vs 2.0 ± 0.0 nFm2/kg, (p < 0.001) and 203 ± 8 vs 125 ± 1 nFm2, (p < 0.001) respectively]. Figures 1 and 2 demonstrated the difference in BCI and CH2 between healthy volunteers and CAPD patients. In logistic regression with 128 CAPD patients versus 322 healthy volunteers (Table 1), age and gender ad- justed BIA parameter alone model showed that BCI has the highest risk discrimination, followed by CH2, phase angle and finally BRI. Phase angle have odds ratio per 1˚ increase of less than 1 because higher phase angle predict better nutritional status. However when combined with body capacitive index (BCI) and CH2 in combined model 1 and 2, the odds ratio of phase angle became more than 1. This is because the function of body resistive index is unmasked for phase angle, when a combined model built with body capacitive index. In contrast, combined model 5 showed that X2 value of phase angle was minimized and became insignificant when combined with body ca- pacitive index and body resistive index because its func- tions in assessing capacitance and resistance of the body were replaced by BCI and BRI. 3.4. Survival Analysis 35 (27.3%) patients died during the study period. Infec- tive (54%) and cardiovascular diseases (26%) were the main cause of death. The detail causes of death included: cardiac events, 6 patients; cerebral events, 3 patients;  K.-H. KOH, H.-S. WONG 197 Table 2. Univariate analysis Comparison of clinical parameters during enrolment for survival. Mean p-value p-value Survived Died Non-adjusted Adjusted# Age year 48.7 ± 1.5 55.4 ± 2.3 0.018 0.141 Duration of dialysis year 1.9 ± 1.1 2.2 ± 1.1 0.467 BMI kg/m2 24.3 ± 0.5 24.7 ± 0.7 0.716 Albumin g/L 30.1 ± 0.5 27.7 ± 0.9 0.016 0.028## TSF cm 1.86 ± 0.10 1.82 ± 0.19 0.852 MAC cm 28.2 ± 0.5 29.1 ± 1.0 0.442 MAMC cm 22.4 ± 0.4 23.4 ± 0.6 0.190 AMA cm2 40.7 ± 1.3 44.1 ± 2.2 0.201 Body Resistive Index Ω kg/cm2 1.325 ± 0.032 1.294 ± 0.034 0.506 Body Capacitive Index* nFm2/kg 2.855 3.985 <0.001 0.004 CH2* nFm2 168.70 244.73 <0.001 0.001 Phase angle** ˚ 4.86 3.58 <0.001 Fat Percentage** % 30.2 25.9 0.506 Mean are expressed with ±standard error of mean. Student t-test was used for comparison except in data with **. *Geometric means were shown and compared. **Medians were shown and Mann Whitney U test were performed. Anthropometry was measured using triceps skin fold thickness (TSF), mid arm circumfer- ence (MAC), mid arm muscle circumference (MAMC), and arm muscle area (AMA) and calculated using formulae of MAMC = MAC − л.TSF; and AMA = (MAC − л.TSF)2 / 4л; #Univariate adjusted analysis with age, DM status and albumin via ANCOVA were performed for factor with normal distribution, if the unadjusted analysis by t-test demonstrated significant differences. ##Albumin analysis was adjusted with DM status and age. Table 3. Cox Regression Survival Hazard Ratio Model with various BIA parameters for CAPD patients. 95% CI 95% CI Parameters per Unit increase Hazard ratio Lower boundUpper boundp-valueHazard ratioLower bound Upper boundp-value Model with phase angle Model with Capacitive Index Age per 1 year increase 1.022 0.996 1.050 0.0971.030 1.002 1.059 0.036 Diabetes Status DM:nonDM 1.402 0.680 2.891 0.3591.597 0.769 3.315 0.209 Albumin per 1 g/L increase 0.968 0.909 1.032 0.3210.954 0.894 1.019 0.160 Phase angle per 1˚ increase 0.454 0.310 0.664 <0.001 Capacitive Index per 1 nFm2/kg increase 1.389 1.139 1.695 0.001 Overall model* 2 4 X = 19.3 0.001 2 4 = 27.7 <0.001 Model with XC/H Model with CH2 Age per 1 year increase 1.024 0.997 1.053 0.0871.028 1.000 1.058 0.053 Diabetes Status DM:nonDM 1.187 0.561 2.511 0.6541.275 0.592 2.743 0.535 Albumin per 1 g/L increase 0.961 0.901 1.026 0.2360.942 0.891 0.995 0.034 XC/H per 1 Ω/cm increase 0.000 0.000 0.012 <0.001 CH2 per 1 nFm2 increase 1.006 1.003 1.009 <0.001 Overall model* 2 4 X = 17.5 0.002 2 4 = 32.4 <0.001 Abbreviation: CI, confidence interval; *Overall model tested were with Omnibus test of Model Coefficients. Note: CH2 per 1 nFm2 increase is replaceable by CH2 per 100 nFm2 increase with hazard ratio of 1.753 (CI: 1.302 - 2.368, p < 0.001), or H2/XC in the unit of cm2/Ω with hazard ratio of 1.002 (CI: 1.001 - 1.003, p < 0.001). peritonitis, 7 patients; other infection, 12 patients; ma- lignancy, 1 patient; other causes of death, 2 patients; un- known cause of death, 4 patients. Twenty out of 47 pa- tients with diabetes died (43%) in comparison to 15 out of 81 non diabetic patients (19%) (p = 0.003). There was no gender predisposition for survival in this cohort with 52 out of 69 female patients (75%) survived and 41 out of 59 male patients (69%) survived (p = 0.458). Adjusted univariate survival analyses were performed with age, diabetes mellitus status and albumin as in Ta- ble 2, for those parameters which had significant differ- ence between the survival and fatal patients. We have Open Access OJNeph 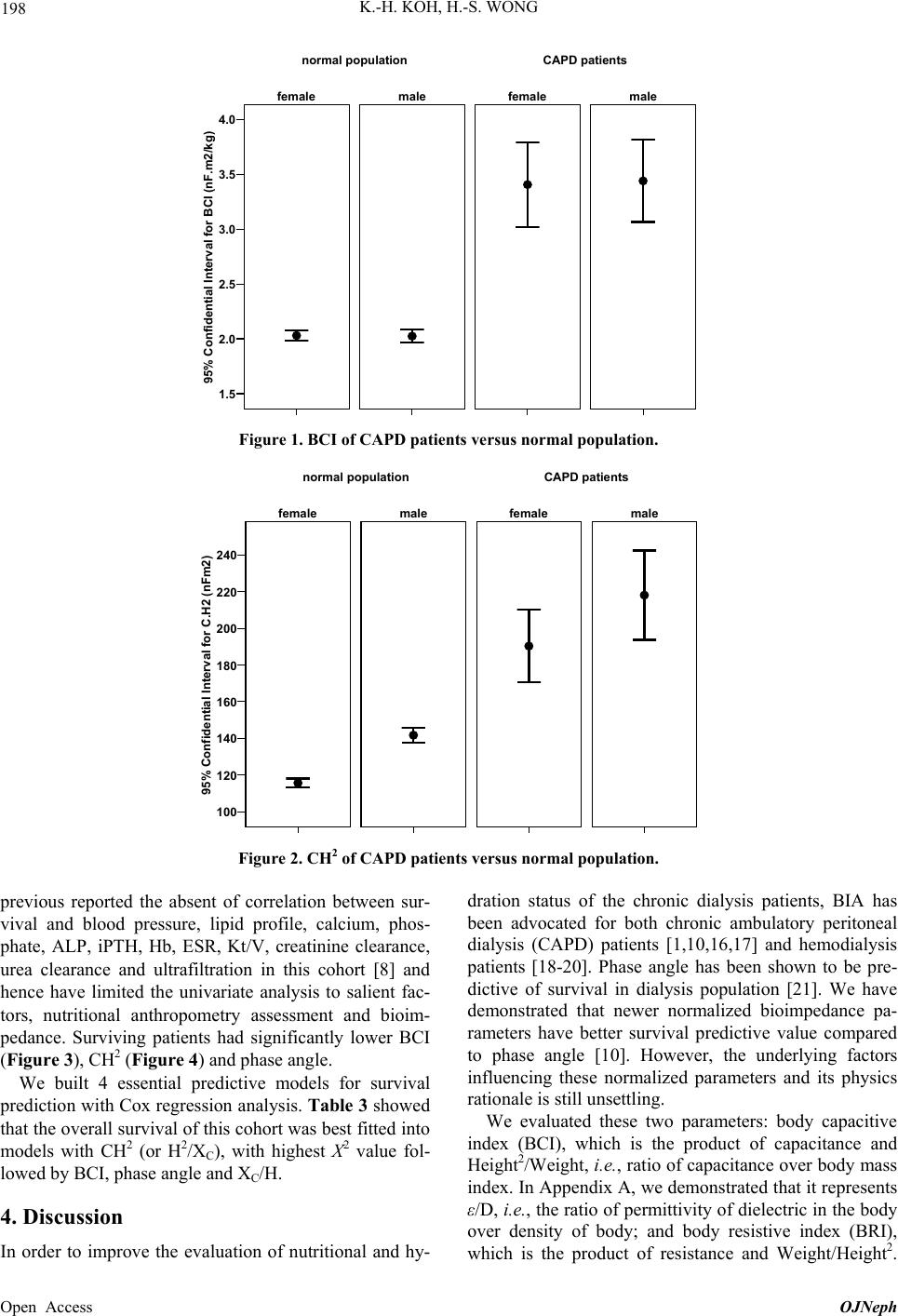 K.-H. KOH, H.-S. WONG 198 95% Confidential Interval for BCI (nF.m2/kg) 4.0 3.5 3.0 2.5 2.0 1.5 malefemale malefemale CAPD patientsnormal population Figure 1. BCI of CAPD patients versus normal population. 95% Confidential Interval for C.H2 (nFm2) 240 220 200 180 160 140 120 100 malefemale malefemale CAPD patientsnormal population Figure 2. CH2 of CAPD patients versus normal population. previous reported the absent of correlation between sur- vival and blood pressure, lipid profile, calcium, phos- phate, ALP, iPTH, Hb, ESR, Kt/V, creatinine clearance, urea clearance and ultrafiltration in this cohort [8] and hence have limited the univariate analysis to salient fac- tors, nutritional anthropometry assessment and bioim- pedance. Surviving patients had significantly lower BCI (Figure 3), CH2 (Figure 4) and phase angle. We built 4 essential predictive models for survival prediction with Cox regression analysis. Table 3 showed that the overall survival of this cohort was best fitted into models with CH2 (or H2/XC), with highest X2 value fol- lowed by BCI, phase angle and XC/H. 4. Discussion In order to improve the evaluation of nutritional and hy- dration status of the chronic dialysis patients, BIA has been advocated for both chronic ambulatory peritoneal dialysis (CAPD) patients [1,10,16,17] and hemodialysis patients [18-20]. Phase angle has been shown to be pre- dictive of survival in dialysis population [21]. We have demonstrated that newer normalized bioimpedance pa- rameters have better survival predictive value compared to phase angle [10]. However, the underlying factors influencing these normalized parameters and its physics rationale is still unsettling. We evaluated these two parameters: body capacitive index (BCI), which is the product of capacitance and Height2/Weight, i.e., ratio of capacitance over body mass index. In Appendix A, we demonstrated that it represents ε/D, i.e., the ratio of permittivity of dielectric in the body over density of body; and body resistive index (BRI), which is the product of resistance and Weight/Height2. Open Access OJNeph  K.-H. KOH, H.-S. WONG 199 95% Confidentia l Interval for BCI ( nFm 2/ kg) 5.5 5.0 4.5 4.0 3.5 3.0 2.5 FatalSurvived FatalSurvived MaleFemale Figure 3. BCI at enrolment in Survived versus Fatal CAPD patients with 2 years follow-up. 95% Confidential Interval for C.H2 (nFm2) 350 300 250 200 150 FatalSurvived FatalSurvived MaleFemale Figure 4. CH2 at enrolment in Survived versus Fatal CAPD patients with 2 years follow-up. The phase angle is actually the arctangent of 1/ωCIRI. We also showed that CH2 represents εV, the total body capacitive volume in physics. BCI reflects nutritional deficit concentration, i.e., both nutritional and hydration status while CH2 reflects total nutritional deficit. (Note that H2/XC = ωCH2− to compare the result with previous study of general population in Germany [7]) BCI correlated with albumin, better than all other pa- rameters. Linear regression of BCI and albumin sug- gested that the presence of albumin and other unmeas- ured substance reduce the capacitive indexes. Previous literature has discussed regarding the survival predictive value of albumin [17] and the potential relation of hypo- albuminaemia with overhydration [16]. In current study, although BCI has higher predictive value than albumin alone in predictive model, we are still not able to differ- entiate the causal-result relationship of fluid and nutrition with BCI. Nonetheless, higher BCI in CAPD patients reflected their higher nutritional deficit concentration while higher CH2 reflected their higher total nutritional deficit. Logis- tic regression of various BIA parameters showed that body capacitive index is the main risk discriminator be- tween CAPD patients and general population. This showed the marked change in the deficit in nutrition con- centration with disease occurrence. The combined model of bioimpedance parameters revealed the interesting un- derlying interplay between BCI, BRI and phase angle. The hidden BRI property of phase angle unmasked and its risk discriminating property disappeared, when model involved phase angle and BCI. Meanwhile, the hidden BCI property of phase angle unmasked when it is in Open Access OJNeph  K.-H. KOH, H.-S. WONG 200 combined model with BRI. CH2 is the second risk dis- criminator as total nutritional deficit would be increased when end stage renal failure set in. Both CH2 and BCI were the key survival predictors for CAPD patients as demonstrated by Cox regression mod- els (Table 3). Total nutrition deficit (with the marker of CH2) and nutritional deficit concentration (BCI) of a CAPD patient predicts his/her survival. Therefore, we proposed their assessment in line with noble clinical opinion of systematic nutritional assessment with bioim- pedance for end stage renal failure patients [13,22-24]. Our previous study demonstrated that both BCI and CH2 were better risk indicators than phase angle because of the gender effect on the latter which actually makes it less discriminatory [10]. In addition, because BRI was non-significantly higher in survived than diseased pa- tients, they reduced the phase angle of the survived pa- tients mathematically and thus limited the survival pre- dictability of the phase angle. In short, we showed the better correlation of albumin and BCI in comparison to other bioimpedance parame- ters. And we also demonstrated the better risk discrimi- natory effect of BCI in comparison to phase angle with logistic regression which is not presented in our last pa- per [10]. Besides, we reported the use of single frequency BIA in determining the nutritional and hydration status of the patient as well as survival prediction [7,25-27]. We sug- gest further exploration in regard to resistive and capaci- tive indices [25,26] and extension of research into hae- modialysis [28], HIV [29] and other diseases and healthy control populations. Certainly further study is needed to reaffirm the clinical role of BCI and CH2. These are in line with current opinion on BIA research [22,30]. Unlike other sophisticated BIA derived parameters, BCI, CH2 and BRI are practical factors that easily meas- ured and derived from height, weight, capacitance and resistance of the patient, in routine clinical assessment. Nevertheless, just like other BIA parameters, e.g., phase angle, the baseline normal reference of BCI and CH2 are needed for one to confidently conclude the extent of nu- tritional deficit. Gaining normal reference for various ethnic and disease population is one of the main hurdles in bioimpedance clinical use and we have shared this concern to the fellow researchers [31]. Recently, Zhu F et al. has significant break through with innovative advance using segment-specific resistiv- ity to derive accurate water distribution information [32,33]. At the same time, phase angle of various body compartment was explored by Nescolarde L [34]. We hereby hope to propose that future research possibly should also look into segment-specific capacitive permit- tivity. One might be able to postulate higher significance in nutritional assessment and risk discriminating effect with trunk capacitive index. 5. Conclusions In summary, BCI represents nutritional deficit concen- tration with good negative correlation with albumin and was the main risk discriminator for CAPD patients ver- sus general population. On the other hand, CH2 repre- sents total nutrition deficit and thus the major risk indi- cator for the survival of CAPD patients. And, the tradi- tionally measured phase angle was a compound parame- ter for BCI and BRI. Further research for BCI, BRI and CH2 in other healthy community and other disease groups are needed before we could draw a firm conclusion to their specific role in physiology and clinical management. 6. Acknowledgements We thank all clinical staffs of Kuala Lumpur Hospital in managing this study cohort and accomplishing the study. We also thank Director General of Health, Malaysian Ministry of Health in approving the publication of this research paper. REFERENCES [1] L. T. Cheng, W. Tang and T. Wang, “Strong Association between Volume Status and Nutritional Status in Perito- neal Dialysis Patients,” American Journal of Kidney Dis- eases, Vol. 45, No. 5, 2005, pp. 891-902. http://dx.doi.org/10.1053/j.ajkd.2005.01.037 [2] A. Picolli, “Bioelectric Impedance Vector Distribution in Peritoneal Dialysis Patients with Different Hydration Status,” Kidney International, Vol. 65, No. 3, 2004, pp. 1050-1063. http://dx.doi.org/10.1111/j.1523-1755.2004.00467.x [3] F. Zhu, G. Wystrychowski, T. Kitzler, S. Thijssen, P. Kotanko and N. W. Levin. “Application of Bioimpedance Techniques to Peritoneal Dialysis,” Contributions to Ne- phrology, Vol. 150, 2006, pp. 119-128. http://dx.doi.org/10.1159/000093511 [4] G. A. Young, J. D. Kopple and B. Lindholm, “Nutritional Assessment of Continuous Ambulatory Peritoneal Dialy- sis Patients: An international Study,” American Journal of Kidney Diseases, Vol. 17, No. 4, 1991, pp. 462-471. [5] U. G. Kyle, I. Bosaeus, A. D. De Lorenzo, P. Deurenberg, M. Elia, J. M. Gómez, B. L. Heitmann, L. Kent-Smith, J. C. Melchior, M. Pirlich, H. Scharfetter, A. M. Schols and C. Pichard, “Composition of the ESPEN Working Group. Bioelectrical Impedance Analysis, Part I: Review of Prin- ciples and Methods,” Clinical Nutrition, Vol. 23, No. 5, 2004, pp. 1226-1243. http://dx.doi.org/10.1016/j.clnu.2004.06.004 [6] U. G. Kyle, I. Bosaeus, A. D. De Lorenzo, P. Deurenberg, M. Elia, J. M. Gómez, B. L. Heitmann, L. Kent-Smith, J.-C. Melchior, M. Pirlich, H. Scharfetter, A. M. W. J. Schols and C. Pichard, “Bioelectrical Impedance Analy- Open Access OJNeph  K.-H. KOH, H.-S. WONG 201 sis—Part II: Utilization in Clinical Practice,” Clinical Nutrition, Vol. 23, No. 6, 2004, pp. 1430-1453. http://dx.doi.org/10.1016/j.clnu.2004.09.012 [7] A. Bosy-Westphal, S. Danielzik, R. P. Dörhöfer, A. Pic- coli and M. J. Muller, “Patterns of Bioelectrical Imped- ance Vector Distribution by Body Mass Index and Age: Implications for Body-Composition Analysis,” The Ame- rican Journal of Clinical Nutrition, Vol. 82, No. 1, 2005, pp. 60-68. [8] R. F. Kushner, D. A. Schoeller, C. R. Fjeld and L. Dan- ford, “Is the Impedance Index (ht2/R) Significant in Pre- dicting Total Body Water?” The American Journal of Clinical Nutrition, Vol. 56, No. 5, 1992, pp. 835-839. [9] H. C. Lukaski, P. E. Johnson, W. W. Bolonchuk, G. I. Lykken, “Assessment of Fat-Free Mass Using Bioelectri- cal Impedance Measurements of the Human Body,” The American Journal of Clinical Nutrition, Vol. 41, No. 4, 1985, pp. 810-817. [10] K. H. Koh, H. S. Wong, K. W. Go and Z. Morad, “Nor- malized Bioimpedance Indices Are Better Predictors of Outcome in Peritoneal Dialysis Patients,” Peritoneal Di- alysis International, Vol. 31, No. 5, 2011, pp. 574-582. http://dx.doi.org/10.3747/pdi.2009.00140 [11] M. Dittmar, “Reliability and Variability of Bioimpedance Measures in Normal Adults: Effects of Age, Gender, and Body Mass,” American Journal of Physical Anthropology, Vol. 122, No. 4, 2003, pp. 361-370. http://dx.doi.org/10.1002/ajpa.10301 [12] M. Arroyo, A. M. Rocandio, L. Ansotegui, H. Herrera, I. Salces and E. Rebato, “Comparison of Predicted Body Fat Percentage from Anthropometric Methods and from Impedance in University Students,” British Journal of Nutrition, Vol. 92, No. 5, 2004, pp. 827-832. http://dx.doi.org/10.1079/BJN20041273 [13] P. A. Fein, G. Gundumalla, A. Jorden, B. Matza, J. Chat- topadhyay and M. M. Avram, “Usefulness of Bioelectri- cal Impedance Analysis in Monitoring Nutrition Status and Survival of Peritoneal Dialysis Patients,” Advances in Peritoneal Dialysis, Vol. 18, 2002, pp. 195-199. [14] D. P. Kotler, S. Burastero, J. Wang and R. N. Pierson Jr., “Prediction of Body Cell Mass, Fat-Free Mass, and Total Body Water with Bioelectrical Impedance Analysis: Ef- fects of Race, Sex, and Disease,” American Journal of Physical Anthropology, Vol. 64, Suppl. 3, 1996, pp. S489- S497. [15] “Declaration of Helsinki,” Bulletin of the Pan American Health Organization, Vol. 24, 1990, pp. 606-609. [16] C. H. Jones, L. Wells, J. Stoves, F. Farquhar and G. Woodrow, “Can a Reduction in Extracellular Fluid Vol- ume Result in Increased Serum Albumin in Peritoneal Dialysis Patients?” American Journal of Kidney Diseases, Vol. 39, No.4, 2002, pp. 872-875. http://dx.doi.org/10.1053/ajkd.2002.32010 [17] D. G. Struijk, R. T. Krediet, G. C. Koomen, E. W. Boeschoten and L. Arisz, “The Effect of Serum Albumin at the Start of Continuous Ambulatory Peritoneal Dialysis Treatment on Patient Survival,” Peritoneal Dialysis In- ternational, Vol. 14, No. 2, 1994, pp. 121-126. [18] N. W. Levin, F. Zhu, E. Seibert, C. Ronco and M. K. Kuhlmann, “Use of Segmental Multifrequency Bioimpe- dance Spectroscopy in Hemodialysis,” Contributions to Nephrology, 2005, Vol. 149, 2005, pp. 162-167. http://dx.doi.org/10.1159/000085482 [19] P. L. Cox-Reijven, J. P. Kooman, P. B. Soeters, F. M. Van der Sande and K. M. Leunissen, “Role of Bioim- pedance Spectroscopy in Assessment of Body Water Compartments in Hemodialysis Patients,” American Jour- nal of Kidney Diseases, Vol. 38, No. 4, 2001, pp. 832-838. http://dx.doi.org/10.1053/ajkd.2001.27703 [20] A. E. Jabara and R. L. Mehta, “Determination of Fluid Shifts during Chronic Hemodialysis Using Bioimpedance Spectroscopy and an In-Line Hematocrit Monitor,” ASAIO Journal, Vol. 41, No. 3, 1997, pp. M682-M687. http://dx.doi.org/10.1097/00002480-199507000-00098 [21] R. Mushnick, P. A. Fein, N. Mittman, N. Goel, J. Chattopadhyay and M. M. Avram, “Relationship of Bio- electrical Impedance Parameters to Nutrition and Survival in Peritoneal Dialysis Patients,” Kidney International, Vol. 64, Suppl. 87, 2003, pp. S53-S56. http://dx.doi.org/10.1046/j.1523-1755.64.s87.22.x [22] M. C. Barbosa-Silva, A. J. Barros, J. Wang, S. B. Heyms- field and R. N. Pierson Jr., “Bioelectrical Impedance Analysis: Population Reference Values for Phase Angle by Age and Sex,” The American Journal of Clinical Nu- trition, Vol. 82, No. 1, 2005, pp. 49-52. [23] K. Kalantar-Zadeh, T. A. Ikizler, G. Block, M. M. Avram and J. D. Kopple, “Malnutrition-Inflammation Complex Syndrome in Dialysis Patients: Causes and Conse- quences,” American Journal of Kidney Diseases, Vol. 42, No. 5, 2003, pp. 864-881. http://dx.doi.org/10.1016/j.ajkd.2003.07.016 [24] G. M. Chertow, J. M. Lazarus, N. L. Lew, L. Ma and E. G. Lowrie, “Bioimpedance Norms for the Hemodialysis Population,” Kidney International, Vol. 52, No. 6, 1997, pp. 1617-1621. http://dx.doi.org/10.1038/ki.1997.493 [25] M. K. Kuhlmann, F. Zhu, E. Seibert and N. W. Levin, “Bioimpedance, Dry Weight and Blood Pressure Control: New Methods and Consequences,” Current Opinion in Nephrology & Hypertension, Vol. 14, No. 6, 2005, pp. 543-549. http://dx.doi.org/10.1097/01.mnh.0000185983.48319.00 [26] A. Piccoli, G. Pastori, M. Guizzo, M. Rebeschini, A. Naso and C. Cascone, “Equivalence of Information from Single versus Multiple Frequency Bioimpedance Vector Analysis in Hemodialysis,” Kidney International, Vol. 67, No. 1, 2005, pp. 301-313. http://dx.doi.org/10.1111/j.1523-1755.2005.00083.x [27] R. N. Baumgartner, W. C. Chumlea and A. F. Roche, “Bioelectric Impedance Phase Angle and Body Com- position,” The American Journal of Clinical Nutrition, Vol. 48, No. 1, 1998, pp. 16-23. [28] G. M. Chertow, D. O. Jacobs, J. M. Lazarus, N. L. Lew and E. G. Lowrie, “Phase Angle Predicts Survival in Hemodialysis Patients,” Journal of Renal Nutrition, Vol. 7, No. 4, 1997, pp. 204-207. http://dx.doi.org/10.1016/S1051-2276(97)90020-0 [29] M. Ott, H. Fischer, H. Polat, E. B. Helm, M. Frenz, W. F. Caspary and B. Lembcke, “Bioelectrical Impedance Open Access OJNeph  K.-H. KOH, H.-S. WONG Open Access OJNeph 202 Analysis as Predictor of Survival in Patients with Human Immunodeficiency Virus Infection,” Journal of Acquired Immune Deficiency Syndromes and Human Retrovirology, Vol. 9, No. 1, 1995, pp. 20-25. http://dx.doi.org/10.1097/00042560-199505010-00003 [30] P. Kotanko, N. W. Levin and F. Zhu, “Current State of Bioimpedance Technologies in Dialysis,” Nephrology Dialysis Transplantation, Vol. 23, No.3, 2008, pp. 808- 812. http://dx.doi.org/10.1093/ndt/gfm889 [31] K. H. Koh, H. S. Wong, K. W. Go and Z. Morad, “The Implication of Bioimpedance Analysis in Peritoneal Di- alysis patients,” Asian Chapter Newsletter, International Society of Peritoneal Dialysis, Vol. 9, No. 1, 2011, pp. 2- 3. [32] F. Zhu, M. K. Kuhlmann, G. A. Kaysen, S. Sarkar, C. Kaitwatcharachai, R. Khilnani, L. Stevens, E. F. Leonard, J. Wang, S. Heymsfield and N. W. Levin, “Segment- Specific Resistivity Improves body Fluid Volume Esti- mates from Bioimpedance Spectroscopy in Hemodialysis Patients,” Journal of Applied Physiology, Vol. 100, No. 2, 2006, pp. 717-724. http://dx.doi.org/10.1152/japplphysiol.00669.2005 [33] F. Zhu, P. Kotanko, G. J. Handelman, J. G. Raimann, L. Liu, M. Carter, M. K. Kuhlmann, E. Seibert, E. F. Leo- nard and N. W. Levin, “Estimation of Normal Hydration in Dialysis Patients Using Whole Body and Calf Bioim- pedance Analysis,” Physiological Measurement, Vol. 32, No. 7, 2011, pp. 887-902. http://dx.doi.org/10.1088/0967-3334/32/7/S12 [34] L. Nescolarde, J. Rosell-Ferrer and T. Doñate, “Relation- ship between Segmental and Whole-Body Phase Angle in Peritoneal Dialysis Patients,” Physiological Measurement, Vol. 29, No. 9, 2008, pp. N49-N57. http://dx.doi.org/10.1088/0967-3334/29/9/N01  K.-H. KOH, H.-S. WONG 203 Appendix A For a given electrical conductor undergoing direct cur- rent, resistance is proportionately correlated with length and inversely proportionately correlated with area. It is generally hard to convert the cylinder model to human body. However, we could deduce their relationship with length (height) and weight with below approximation (7) with modification : LkH 2 2 2 Weig ht Weig ht Let LL RAV DV kH D R BRIk D 2 Weight or R BRIBRIR BMI H (1) whereby R represents resistance; ρ, apparent resistivity of the conductor; A, Area; L, Length, converting to H, height in subsequent equations; D, density of the body; W, weight; BMI, body mass index; k, converting factor of body; And, Body resistive index (BRI) reflects the product of resistivity of the body and density of body. In same simulation model, during the alternative cur- rent, we assume that the cell membrane and other protein substance can act as a capacitor due to its dielectric property, where the A is area of cell membrane, L is the thickness of cell membrane plus body compartments, and ε is the permittivity of cell membrane. The reactance was calculated from wrist to ankle bioimpedance measure- ment which reflects all parallel connected cells in the body. Therefore, the reactance of the capacitor is reflected by the below equations: 1 C XC (2) whereby 2 whereby C, capacitance; f, frequency of applied alterna- tive current; XC, reactance. For a given capacitor consisting of 2 metal plates, the capacitance could be measured by: CL whereby C represents capacitance; ε, permittivity of the dielectric between the two plates; A, Area; L, Distance between these plates. Therefore, we could derive body capacitive index (BCI), as well as C·H2 for human in BIA with the below equation: 22 22 Weigh tAV CLkHkD H (3) Let 2 BCI kD 2 or Weight CH C BCIBCI BMI (4) Besides, from Equation (3), 2 2 V CH k (5) whereby V represents volume of body; D, density of body; H, height; k, converting factor of body. Thus, BCI represents the ratio of capacitive permittiv- ity over body density, while CH2 represents the total body capacitive volume in physics. It is worth pointing out that the renowned terms of height2/reactance (5) could be derived with: 2 2 C HCH X (6) Thus, the clinical implication of height2/reactance (H2/XC) is equivalent to CH2. However, Height2/resistance is actually mathemati- cally representing V/ρ: 22 kH RV 2 2 V Rk (7) whereby R represents resistance; H, height; V, body volume; ρ, resistivity of body. Phase angle (α) is defined mathematically as arc tan- gen of reactance over resistance, being measured in de- gree. Reactance 1 Resistance C X ArcTan ArcTan RC R (8) From Equation (1), 2 weight BRI H R (9) From Equation (4), 2 Weight BCI CH (10) Putting the above Equations (9) and (10) into Equation (8), we get: Open Access OJNeph  K.-H. KOH, H.-S. WONG 204 11 C X RCRBCIBRI 1 ArcTan BCI BRI (11) Take note that resistance for measurement of BRI in our current study is taken from direct current. Because of mathematically low phase angle range for all subjects and thus cosine is closed to 1, the body resistance in al- ternative current was thus not differed much from resis- tance in direct current. Putting 2 BCI kD and into Equa- 2 BRIk D tion (11) 2 2 11 ArcTan ArcTan kD kD (12) Inserting these into Equation (8) with info from Equa- tion (9) and (10), we get CRBCI BRI (13) Therefore, CR, the product of capacitance and resis- tance is representing ερ, i.e., the product of resistivity of body and permittivity of body dielectric. Open Access OJNeph
|