Engineering, 2013, 5, 396-399
http://dx.doi.org/10.4236/eng.2013.510B080 Published Online October 2013 (http://www.scirp.org/journal/eng)
The Wall Shear Stress of a Pulsatile Blood Flow in a
Patient S pecific Stenotic Rig ht Coronary Artery
Biyue Liu
Department of Mathematics Monmouth University West Long Branch, NJ, USA
Received 2013
ABSTRACT
A computer simulation of the blood flow in a patient specific atherosclerotic right coronary artery is carried out to study
the blood flow pattern and the wall shear stress (WSS) distribution in the artery. Both temporal and special distr ibution
patterns of the WSS of the non-Newtonian blood flow are presented and the regions on the lumen surface where the
WSS is constantly lower than 1 N/m2 are identified.
Keywords: Wall Shear Stress; Stenotic Right Coronary Artery; Non-Newtonian
1. Introduction
Atherosclerosis is the chief cause of death in the United
States. It is a disease which involves complex interac-
tions of many factors. It is widely believed that the wall
shear stress (WSS) is one of the important local biome-
chanical factors responsible for the initiation and the pro-
gression of atherosclerosis [1-7]. A detailed hemodyna-
mic evaluation of the spatial and temporal WSS distrib u-
tions may give additional insight to understanding the
progression of the disease and may have useful clinical
value.
In the past few decades, numerous clinical researches
and computer simulations have been performed to study
the flow phenomena in human atherosclerotic arteries
and to investigate the correlation between the WSS and
the intima-media thickness [1-10]. Caro et al. [1] sug-
gested that the distribution of fatty streaking in human
aorta might be coincident with the regions in which the
shear rate at the arterial wall is locally reduced. Friedman
et al. [2] and Nerem et al. [6] performed experiments that
showed an accelerated occurrence of atherosclerosis in
human subjects with a coronary geometry. Zarins et al.
[7] and Ku et al. [4] estimated shear stress with laser-
Doppler anemometry and found that intimal thickening
bears an inverse relationship to both maximum shear
stress and minimum shear stress. Gibson et al. [3] inves-
tigated the relationship between the vessel wall shear
stress and the r ate of atherosclerosis progression by means
of quantitative angiography. They found a significant
correlation between the low shear stress and an increased
rate of atherosclerosis progression. Johnston et al. [10]
compared models of Newtonian and Non-Newtonian flow
in healthy right coronary arteries with no sign of athero-
ma. Although the blood flow in stenotic arteries has been
extensively studied, there is much to be done both in un-
derstanding the disease process itself and also in under-
standing the role of hemodynamics and the associated
WSS as an influence factor on the initiation and the pro-
gression of athe ros c l erosis .
The objectives of the current work are to simulate the
blood flow in a stenotic human right coronary artery and
to investigate the spatial and temporal WSS distribution
patterns during a cardiac cycle.
2. Mathematical Model
This study assumes that the fluid is Laminar, Non-New-
tonian, viscous and incompressible and the artery wall is
rigid. These assumptions have been shown to be ade-
quate for the pulsatile blo od flow simulation in th e artery
models under physiological flow conditions by many
investigators. The computational domain is a patient spe-
cific atherosclerotic right coronary artery shown in Fig-
ure 1(a), re-constructed based on the in vivo intravascu-
lar ultrasound (IVUS) image of a patient [9]. The lumen
cross section area fro m the inlet to the outlet is plotted in
Figure 1(b), where the horizontal axis is the normalized
axial length with inlet z = 0 and the outlet z = 1. The
minimum lumen cross section area at the neck of the
stenosis is 0.042 cm2. The maximum area of the cross
section proximal to the stenosis is 0.130 cm2. The r educ-
tion of the lumen area at the neck of stenosis is approx-
imately 68%. It is a severely stenotic right coronary ar-
tery segment.
The time dependent three dimensional Navier Stokes

B. Y. LIU ET AL.
397
(a)
(b)
Figure 1. (a) Geometry of the computational domain; (b)
Lumen cross section area from the inlet to the outlet. Neck
of the stenosis at z = 0.6.
equations are used as the governing equations. The inlet
boundary is imposed with a fully developed flow with a
physiological human right coronary waveform (Figure
2), scaled to yield a time averaged flow velocity of 0.166
m/s at the centre-line. A no-slip condition is applied to
the velocities at the wall boundary, treated to be inelastic
and impermeable. The outlet boundary is treated as an
open boundary with the zero normal stress:
((() ))
T
p η −+∇ +∇=I uu0n
(1)
where n = (n1, n2, n3) is the outward normal unit vector at
the outlet boundary. The initial cond itions for the veloci-
ty and the pressure are obtained by solving the system of
steady state Navier Stokes equations. The blood is treated
as a non-Newtonian fluid obeying the Carreau model with
the viscosity-shear rate relation:
1
22
0
()[1()]
n
η ηηηλγ
−
∞∞
=+−+

(2)
where η0 = 0.056 Pa∙s is the zero shear rate viscosity,
η
∞
= 0.00345 Pa ·s is the infinite sh ear rate viscos ity, λ =
3.313s is a parameter, and n = 0.3568 is a dimensionless
parameter [7,10]. The blood density
ρ is assumed to be
constant at 1050 kg/m3.
3. Observations and Discussion
The Navier Stokes equations are solved numerically us-
ing the finite element method with piecewise quadratic
functions for velocity and piecewise linear functions for
pressure over a tetrahedral mesh. Four consecutive car-
diac cycles are simulated to ensure that the flow is truly
periodic and the computations are repeated over different
meshes to ensure that the numerical solutions are mesh
independent. The numerical computations are performed
using COMSOL 4.2.
Figure 3 presents the contour plots of the WSS (N/m2)
Figure 2. Pulsatile coronary velocity waveform at the inlet.
Figure 3. Spatial distribution of the WSS (N/m2) along the
artery wall when (a) t/ tp = 0.1, (b) t/tp = 0.35, (c) t/tp = 0.85,
respectively.
(a) during the deceleration in systole (t/tp = 0.1), (b) at
the peak in systole (t/tp = 0.35), and (c) at the maximum
flow rate (t/tp = 0.85), respectively. Here the inlet is on
the top, and the outlet is on the bottom. Figure 3 shows
the spatial distribution pattern of the WSS on the lumen
surface. The negative WSS means that the flow moves
backwards. The overall negative WSS along the artery
wall showing in Figure 3(a) is due to the factor that it is
during the reverse flow in the systole (see the first doted
point on the velocity waveform in Figure 2). The nega-
tive WSS showing in Figure 3(c) indicates that flow re-
circulation appeared in these regions with a negative
WSS. F ig ures 3(b) and (c) show a basically similar pat-
tern of the spatial distribution of the WSS along the ar-
tery at the peaks of the systole and the diastole. The ma-
ximum WSS appears at the neck of the stenosis and the
WSS is overall high in stenotic region. There is another
region with a relatively high WSS downstream where the
artery is slightly narrowed. The minimum WSS o ccurs at
the inner wall in the post stenotic region and in the region
proximal to the stenosis where the artery expands. The
plot of the contour of the WSS at any other time during
the forw ard flow has the same pattern as that showing in
Figure 3(b).
-0.01
0.04
0.09
0.14
00.2 0.4 0.6 0.81
Lumen Area (cm2)
Normalized Axial Length
-0.05
0
0.05
0.1
0.15
0.2
0.25
00.2 0.4 0.6 0.81
Velocity (m/s)
t/t
p

B. Y. LIU ET AL.
398
To quantitatively examine the v ariation of the WSS on
the lumen surface, the WSS is averaged on the wall
boundary of each lumen cross section and the plots at
some representing times are included in Figure 4 (t/tp =
0.1, 0.25, 0.35 and 0.85, respectively, which are doted
times in Figure 2). The horizontal axis is the normalized
axial length. The inlet is at z = 0 and the outlet is at z = 1.
The neck of the stenosis is at z = 0.6. Figure 4 confirms
the above observation on the special distribution of the
WSS from Figure 3. The maximum average WSS occurs
at the neck of the stenosis.
Figure 5 plots the WSS in an entire cardiac cycle at
various points on the ar tery wall: (a) at the proximal side
of the stenosis and at the neck of the stenosis, (b) in the
post stenosis and the downstream. It demonstrates the va-
riation of the local WSS during an entire cardiac cycle at
the four locations of most interest: two around the neck
of stenosis and two in the post-stenosis region. From
Figure 5 we can see that other than the area on the inner
wall in the post-stenosis region, the local WSS at any
point basically experiences the same fluctuation as that
of the flow waveform at the inlet: a dip with negative
WSS value caused by the reverse flow in systole follow-
ed by two small peaks and a large elevation in diastole.
However, the purple curve (labeled PostS) in Figure 5(b)
shows that the WSS is constantly low in a whole cardiac
cycle on the inner wall in the post stenotic region, with-
out a dominantly high peak when t/tp = 0.85. These be-
haviors are consistent with the observation made by the
author previously to the simulation based on a simplified
geometry with idealized circular cross sections [7].
Many studies [1-5,7,9] have attempted to correlate the
WSS with the intimal thickness, suggesting that overall it
is in low shear regions that intimal thickness will be
greater. Low local velocity, resulting low WSS values,
increases residence time and interaction between the
blood lipoproteins and vessel endothelium. Long resi-
dence time with endothe lium results in an increased lipo-
protein intake, which might cause the thickening of arte-
rial wall. Clinical studies suggest that intimal thickening
Figure 4. WSS averaged on each lumen cross section when
t/tp = 0.1, t/tp = 0.25, t/tp = 0.35, t/tp = 0.85.
Figure 5. Temporal variation of the WSS at various points
on the inner wall (a) at the proximal side of the stenosis and
at the neck of the stenosis, (b) in the post stenosis and the
downstream of the stenosis.
likely occurs when the average WSS is below 1 N/m2 (=
10 dynes/cm2), which presents an inverse hyperplasia
with respect to the shear stress [5]. Therefore, it is of
special interest to know the location and the size of the
region on the lumen surface where the WSS is lower than
1 N/m2 (including the negative WSS resulted from the
reverse flow or flow recir culati on ) an d the duration of the
low WSS area in a cardiac cycle.
At each point on the lumen surface, we calculated the
total length of the time when the location experiences a
low WSS < N/m2. Figure 6 presents the contour plot of
the duration of the WSS under N/m2 in a cardiac cycle.
Figures 6(a)-(c) are the views of the artery from the side,
the inner wall and the outer wall, respectively. A point
with a value of 1.0 on the lumen surface indicates that
the WSS at this location is lower than 1 N/m2 anytime
during the entire cardiac cycle. The accumulated duration
time of the WSS lower than 1 N/m2 in a cycle has the
minimum value of 0.17 in the region of stenosis neck.
From the length of the interval where the grey curve (la-
beled Neck) is below the horizontal axis line setting at
1.0 level in Figure 5(a), we can tell that this duration of
the low WSS occurs approximately during the systole
between t/tp = 0.03 and t/tp = 0.2 in a cardiac cycle. It is
also apparent that the duration value is 1.0 in the area on
the inner wa ll in the post -stenosis region and the area on
the inner wall in the region proximal to the stenosis
where the lumen is expanded. This indicates that these

B. Y. LIU ET AL.
399
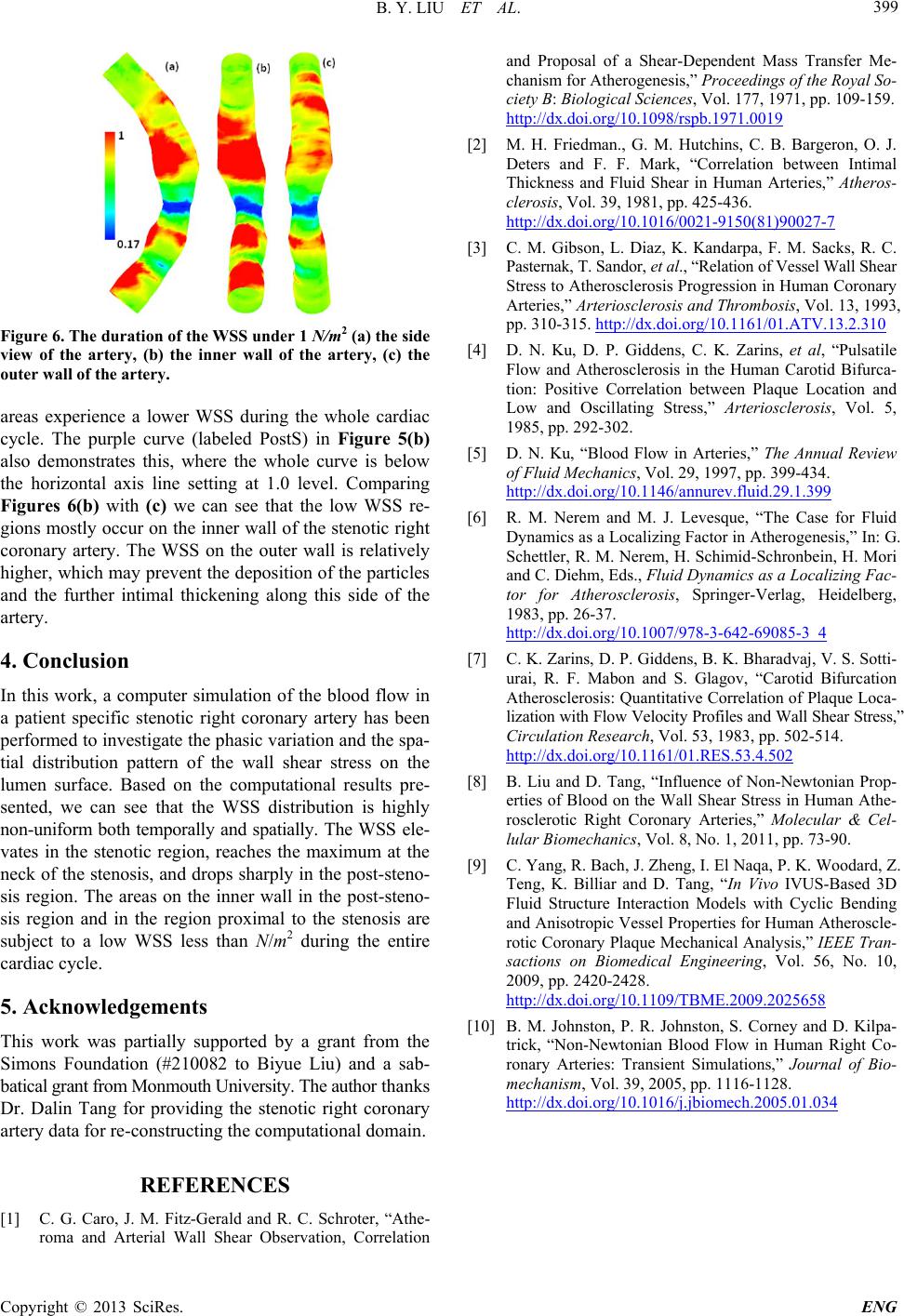
Figure 6. The duration of the WSS under 1 N/m2 (a) the side
view of the artery, (b) the inner wall of the artery, (c) the
outer wall of the artery.
areas experience a lower WSS during the whole cardiac
cycle. The purple curve (labeled PostS) in Figure 5(b)
also demonstrates this, where the whole curve is below
the horizontal axis line setting at 1.0 level. Comparing
Figures 6(b) with (c) we can see that the low WSS re-
gions mostly occur on the inner wall of the stenotic right
coronary artery. The WSS on the outer wall is relatively
higher, which may prevent the deposition of the particles
and the further intimal thickening along this side of the
artery.
4. Conclusion
In this work, a computer simulation of the blood flow in
a patient specific stenotic right coronary artery has been
performed to investigate the phasic variation and the spa-
tial distribution pattern of the wall shear stress on the
lumen surface. Based on the computational results pre-
sented, we can see that the WSS distribution is highly
non-uniform both temporally and spatially. The WSS ele-
vates in the stenotic region, reaches the maximum at the
neck of the stenosis, and drops sharply in the post-steno-
sis region. The areas on the inner wall in the post-steno-
sis region and in the region proximal to the stenosis are
subject to a low WSS less than N/m2 during the entire
cardiac cycle.
5. Acknowledgements
This work was partially supported by a grant from the
Simons Foundation (#210082 to Biyue Liu) and a sab-
batic al gra nt f rom Monm o uth Uni ve rsi ty. The author thanks
Dr. Dalin Tang for providing the stenotic right coronary
artery data for re-constructing the computational domain.
REFERENCES
[1] C. G. Caro, J. M. Fitz-Gerald and R. C. Schroter, “Athe-
roma and Arterial Wall Shear Observation, Correlation
and Proposal of a Shear-Dependent Mass Transfer Me-
chanism for Atherogenesis,” Proceedings of the Royal So-
ciety B: Biological Sciences, Vol. 177, 1971, pp. 109-159.
http://dx.doi.org/10.1098/rspb.1971.0019
[2] M. H. Friedman., G. M. Hutchins, C. B. Bargeron, O. J.
Deters and F. F. Mark, “Correlation between Intimal
Thickness and Fluid Shear in Human Arteries,” Atheros-
clerosis, Vol. 39, 1981, pp. 425-436.
http://dx.doi.org/10.1016/0021-9150(81)90027-7
[3] C. M. Gibson, L. Diaz, K. Kandarpa, F. M. Sacks, R. C.
Pasternak, T. Sandor, et al. , “Rel a t ion of Ve ssel Wall Shear
Stress to Atherosclerosis Progression in Human Coronary
Arteries,” Arteriosc le rosis and Thrombos is, Vol. 13, 1993,
pp. 310-315. http://dx.doi.org/10.1161/01.ATV.13.2.310
[4] D. N. Ku, D. P. Giddens, C. K. Zarins, et al, “Pulsatile
Flow and Atherosclerosis in the Human Carotid Bifurca-
tion: Positive Correlation between Plaque Location and
Low and Oscillating Stress,” Arteriosclerosis, Vol. 5,
1985, pp. 292-302.
[5] D. N. Ku, “Blood Flow in Arteries,” The Annual Review
of Fluid Mechanics, Vol. 29, 1997, pp. 399-434.
http://dx.doi.org/10.1146/annurev.fluid.29.1.399
[6] R. M. Nerem and M. J. Levesque, “The Case for Fluid
Dynamics as a Localizing Factor in Atherogenesis,” In: G.
Schettler, R. M. Nerem, H. Schimid-Schronbein, H. Mori
and C. Diehm, Eds., Fluid Dynamics as a Localizing Fac-
tor for Atherosclerosis, Springer-Verlag, Heidelberg,
1983, pp. 26-37.
http://dx.doi.org/10.1007/978-3-642-69085-3_4
[7] C. K. Zarins, D. P. Giddens, B. K. Bharadvaj, V. S. Sotti-
urai, R. F. Mabon and S. Glagov, “Carotid Bifurcation
Atherosclerosis: Quantitative Correlation of Plaque Loca-
lization with Flow Velocity Profiles and Wall Shear Stress,”
Circulation Research, Vol. 53, 1983, pp. 502-514.
http://dx.doi.org/10.1161/01.RES.53.4.502
[8] B. Liu and D. Tang, “Influence of Non-Newtonian Prop-
erties of Blood on the Wall Shear Stress in Human Athe -
rosclerotic Right Coronary Arteries,” Molecular & Cel-
lular Biomechanics, Vol. 8, No. 1, 2011, pp. 73-90.
[9] C. Yang, R. Bach, J. Zheng, I. El Naqa, P. K. Woodard, Z.
Teng, K. Billiar and D. Tang, “In Vivo IVUS-Based 3D
Fluid Structure Interaction Models with Cyclic Bending
and Anisotropic Vessel Properties for Human Atheroscle-
rotic Coronary Plaque Mechanical Analysis,” IEEE Tran-
sactions on Biomedical Engineering, Vol. 56, No. 10,
2009, pp. 2420-2428.
http://dx.doi.org/10.1109/TBME.2009.2025658
[10] B. M. Johnston, P. R. Johnston, S. Corney and D. Kilpa-
trick, “Non-Newtonian Blood Flow in Human Right Co-
ronary Arteries: Transient Simulations,” Journal of Bio-
mechanism, Vol. 39, 2005, pp. 1116-1128.
http://dx.doi.org/10.1016/j.jbiomech.2005.01.034