Engineering, 2013, 5, 381-385
http://dx.doi.org/10.4236/eng.2013.510B077 Published Online October 2013 (http://www.scirp.org/journal/eng)
DNA Circuit System and Charge Transfer Mechanism
Kunming Xu
College of Ocean and Earth Sciences, Xiamen University, Xiamen, China
Received 2013
ABSTRACT
Based on the accurate identification of chemical structures as electric elements, we described charge transport mechan-
ism in a DNA molecule by a stepwise LC oscillatory circuitry, in which every base pair is a capacitor, every phosphate
bridge is an inductor, and every deoxyribose is a charge router. The circuit model agrees with established experimental
evidence that has supported super-exchange and hopping mechanisms so far. This alternative charge transport mechan-
ism through both strands of DNA matches the fidelity and reliability of its chemical structure.
Keywords: Conductivity; Capacitor; Inductance; Oscillatory
1. Introduction
The electrical conductivity of a DNA molecule is of pa-
ramount important in biological function. It has been
established that very fast charge transport can take place
over a short distance (~37 Å) and a slower transport may
propagate over a long distance (~200 Å) [1-6]. The re-
sults have led to two hypotheses of charge transport me-
chanism in DNA, one indicating super-exchange, or tun-
nel, through the sugar phosphate bridge between the
bound charge donor and acceptor for the short distance,
and the other suggesting charge hopping between dis-
crete bases through the DNA π-stack over long distance.
Although both mechanisms are widely regarded, they are
incompatible and ill-defined, giving the impression of
loose or uncertain charge transfer along nucleotides. As
genetic substance, DNA must possess well-defined elec-
trical behavior. Here we show a DNA double helix as a
stepwise LC oscillatory circuitry, in line with the expe-
rimental evidence. Electrical harmonic oscillations have
significant biological implications.
2. DNA Circuit System
The circuit model regards each base pair as an electric
capacitor because the base pair is composed of two hete-
rocyclic amines placed at a semi-conductive distance,
capable of storing opposite charges. The hydrogen bonds
between the base pair deliver electric pulse but prohibit
direct current between them, which is a typical characte-
ristic of electric capacitors. In the double helix, the phos-
phate bridges are twisted physically like both strands of a
rope under torsion. The wound phosphate bridge can be
treated as an electric inductor, capable of storing energy
as well. The rigid structures of the Watson-Crick parities
and of the pentose sugars help in forcing the torsion onto
the phosphate bridges mechanically. Charge transport
through the strands, like electric current through a coiled
wire, produces electric inductance. Hence DNA structure
is a circuitry composed of multiple oscillatory LC cir-
cuits (Figure 1).
The deoxyribose is a key electric element in the circuit,
which serves as a switch at the juncture (Figure 2). It has
Figure 1. DNA double strands (a) along with stepwise oscillatory circuits (b) and (c), where the dashed arrows indicate elec-
tric current directions and the deoxyriboses are represented by electric switches.

K. M. XU
382
Figure 2. Electric currents directe d by chiral carbons through
a nucleoside. The dotted arrows represent electric current
directions.
been stated that a chiral carbon center may transfer elec-
trons towards a selective pathway due to its asymmetric
polarizations [7]. Three chiral carbons in a deoxyribose
direct electric charges in a selective course: C1’ and C4’
are levorotatory, and C3’ is dextrorotatory [8]. Depend-
ing on whether the nucleotide base is positively charged
or negatively charged, the switch connects C1’ to C5’ or
connects C1’ to C3’ respectively. Direct charge flow
between C3’ and C4’ is choked by both chiral carbons.
The anti-parallel alignment of the pentose sugars on both
strands determines that electric current forms a stepwise
closed loop between every two adjacent base pairs.
Suppose in the closed circuit (Figure 1(b)), charges
stored in capacitor C1 transport through the phosphate
inductors L1 and L2 to reach capacitor C2 under the
routing of the deoxyriboses. The anti-parallel alignment
of the pentose sugars on both strands determines that
positive charges transfer along th e strand in the direction
of 3’ → 5’ while negative charges transfer along the
strand in the direction of 5’ → 3’. Electric current forms
a closed circuit so that by Kirchhoff’s loop rule, we have
voltage drop relationship of
12
112 2
12
QQ dI dI
IR LIRL
Cdt Cdt
=+++ +
, (1)
where I is electric current around the circuit unit, Q1 and
Q2 are charges stored in base pair capacitances C1 and C2
respectively, and R1 and R2 are representative resistors
along the path. Let
12
RR R+=
, (2)
12
LL L+=
, (3)
12
111
CC C
+=
, (4)
12 0
QQ Q+=
, (5)
then upon differentiation on both sides, Equation (1) be-
comes
2
2
dd 0
d d
I II
LR
tC
t+ +=
. (6)
This second-order differential equation is a damped
harmonic oscillator. Depending on the relative values of
L, R and C, the system may be over-damped, critically
damped, un der-damped, or simple harmonic. When
24/R LC<
, the circuit property falls into the two latter
categories with a current function of
2
0
cos( )
Rt
L
I Iet ω
−
=
, (7)
where
0
I
is the initial curren t and
ω
is angular veloc-
ity of the oscillation with a value of
2
2
1
4
R
LC L
ω = −
. (8)
The circuit system transfers electric charges from a
base pair to another in stepwise oscillatory processes, i.e.,
from capacitor C1 to C2 (Figure 7.1b) and then from ca-
pacitor C2 to C3 (Figure 1(c)) along the double helix.
Simple as it is, this model is in line with experimental
evidence [1-6] so far established concerning DNA con-
ductivity and reconciles both super-exchange and mul-
ti-step hopping mechanisms. The LC circuit is robust and
classical in physics, yet revolutionary in chemistry and
biology. This interdisciplinary analysis produces a result
of general interest in biological physics and may have
potential influence in molecular electronics.
3. Charge Transfer Mechanism
It has been reported that the rate of electron transfer
within a short distance decreases exponentially with in-
creasing distance [2-5]. Such a phenomenon corresponds
to the under-damped condition of the circuit system due
to the relatively low (
24/R LC<
) resistance response of
DNA backbones to the artificial introduction of voltage
drop between base pairs in the experiments. However,
when R value is considerable, the exponential signal of
electric current prevails over the sinusoidal cycle in Equ-
ation (7) and vanishes within a few oscillatory cycles.
Since the distance between the b ase pairs of B-DNA is a
fixed value of 3.4 Å and each stepwise oscillatory cycle
takes a certain interval of time for that distance, we may
replace the time variable in Equation (7) with a distance
variable
r∆
. In considering that electric current is a
measure of electron transfer rate k, Equ a tion (7) is equiv-
alent to the Marcus correlation [9]
r
ke
β −∆
∝
(9)
where
β
values between 0.1 and 1.4 Å−1 have been
estimated for the double helix [2-5]. The dramatic dif-
ference can be ascribed to the uncertainty of resistance R
that is sensitive to various experimental conditions. The
presence of considerable R value along the circuit is be-
cause the phosphate bridges are under persistent high
voltage drop induced artificially in experiments so that
inductances partially manifest as resistances in the cir-
cuit.

K. M. XU
383
Based on frequency value of 10−10 s−1 measured by
Fukui et al. [2], we simulate the under-damped oscilla-
tion with parameters of C = 0.02 pF, L = 0.01 μH, and R
= 100 Ω in Equation (8). Assuming three radical cations
are initially generated to trigger charge migration from a
base pair to anot her t hrough the ste pwis e oscill atory cycles,
the curre nt funct i on of Equation (7) is calculated to be
0.005
5.4cos(0.071 )
t
Ie t
−
=
, (10)
where electric current is in the unit of nA and time in ps
(Figu re 3) . Suppos e at t = 0, capacitor C1 carries charges
in the polarity as shown in Figure 1(b), the deoxyribose
switches (S1 to S4) will route the charges in the dotted
arrow direction. After one oscillatory cycle at t = 90 ps,
the charges reach capacitor C2 so that switches S3 and S4
change their connections. The charges at capacitor C2
will then be transferred to capacitor C3 in the next step
(Figu re 1(c)). The amplitude of the electric current along
the double helix decreases exponentially with each oscil-
latory cycle in the under-damped situation (Figure 3).
Because each oscillatory cycle takes 90ps for charges to
migrate 3.4 Å along DNA strands, the β value in Equa-
tion (9) is 0.13 Å−1 in this case.
In v ivo, we believe that natural electron transport from
a base pair to another should incur trivial electric resis-
tance. Even if there is certain electric resistance along the
strands, the thermal energy produced by resistors would
immediately be absorbed by both energy storage com-
ponents of the base pair capacitor and the phosphate in-
ductor. Hence electric resistance can be neglected. Let C
= 0.02 pF, L = 0.05 μH, and R = 5 Ω, the circuit declines
into a series of LC oscillators that transfer charges step
by step along the strands harmonically at a slower pace.
The frequency of the harmonic oscillation is slower than
that of under-damped oscillation as can be predicted
from
ω
formula under the relatively high value of in-
ductance and trivial resistance. It takes about 200 ps for
electrons to transfer from a base pair to the next. But the
amplitude of the electric curren t remains almost the same
in each oscillatory cycle. A comparison of under-damped
oscillation and simple harmonic oscillation can be found
in Figure 3.
In the stepwise LC circuits, electric current is defined
as positive when a base pair capacitor is being charged
and negative when discharged in the next cycle down the
chain. The stepwise oscillations agree with the evidence
that has supported hopping mechanism through the base
π-stack [1-6], but electric current through the sugar phos-
phate bridges is more reliable than the haphazard migra-
tion by hopping across the base rungs. Charges stored in
capacitor C1 transport through both strands to capacitor
C2, and will continue to move towards C3 in the similar
sinusoidal manner but at a lagging phase of
π
in the
cycle. During the processes, positive holes move in the
Figure 3. Model predictions for charge transfer of DNA
circuit by fast stepwise under-damped oscillations in vitro
(black curve) in contrast to slower stepwise simple harmon-
ic oscillations in vivo (gray curve) where each cycle spans
3.4 Å in distance. Charges undergoi ng under-damped osc il-
lations have a hasty speed but cannot reach a long distance.
direction of 3’ → 5’ on one strand while electrons flow
in the direction of 5’ → 3’ on the other strand in good
synchronization. The mechanisms for charge transport
through long and short distances are the same. It takes
more cycle s of osci ll ations f or char ges t o transport through
longer sequences of base pairs. Without considering the
effect of sequence dependence, traveling time is propor-
tional to distance.
From Equation (8), we kno w that
ω
is codetermined
by inductance L and capacitance C when R is negligible.
Assuming constant inductance for all nucleotides, the
harmonic frequency would be determined by two neigh-
boring capacitance values in the closed circuit, such as C1
and C2, which are specific to the base pairs. And this is
perhaps the most subtle part of the story for it indicates
that charge transfer rate is sequence dependent [3-5,10].
Because nucleotide bases have ionization potentials in
the order of G < A < C < T and electron affinities in the
order of C < T < G < A (disregarding negative sign) [11],
it takes the least amount of energy to charge G:C base
pair in the polarity of +G:C− and requires the highest
amount of energy to charge +T:A− capacitor. This means
that the capacitances of the base pairs are in the order of
+G:C− > +A:T− > +C:G− > +T:A− polarities. Thus there
are four distinct capacitance values depending on the
base pair and polarity.
Since
ω
is determined by two neighboring capacit-
ances in series, it may take eight possible values. It is
predicted that charge transport along the strands will pro-
duce various frequencies reflecting the identity of the
bases. In other words, the pattern of charge transport is
precisely controlled by the gene sequence. The +G:C−
capacitor has a higher capacitance than other base pairs
and carries higher amount of charges in experiments whe-
reas +T:A− has a lower capacitance than other base pairs

K. M. XU
384
and is the limiting step or bottleneck in charge transfer
along the DNA strands [6,9]. However, at long (+T:A−)n
sequences the size of the bottleneck remains the same so
that the sequence distance dependence vanishes [9]. Be-
cause +G:C− has a relatively high capacitance, it is easy
to trap charges, so it is likely to become the end point of
a charge transport [6,9]. In this regard, the circuit model
prediction agrees with experimental resu lts completely.
Oscillatory current through the double helix is likely to
have physiological significance. For example, if a base
pair is mismatched at a certain position, then the oscilla-
tory rhythms would be broken. Proofreading enzyme that
scans the DNA sequence continually might easily locate
the trouble point by the abnormal electric signal. Fur-
thermore, bio molecules are inherentl y unstable. Only con-
stant flow of energy prevents them from being disorga-
nized. It stands to reason that the incessant charge vibra-
tions in the genetic substance are vital for living organ-
isms to maintain the integrity of the gene sequence.
From organic chemistry perspective, the base pair is
capable of storing considerable amount of charges in ei-
ther polarity by at least two conceivable mechanisms.
First, both pyrimidine and purine are heterocyclic rings
composed of carbon and nitrogen atoms. On the one hand,
nitrogen is more electronegative than carbon for attract-
ing higher electron density in covalent bonds with carbon.
On the other hand, nitrogen atom has lone pair electrons
to share with carbon under electron deficiency. The com-
bination imparts great flexibility to the nucleotide bases
for either holding or releasing electrons. Second, the he-
terocyclic rings are aromatic that possess diamagnetic
ring current. In the circuit, aromatic ring current may re-
duce the charge saturation of the nitrogenous base through
electromagnetic effect, and as a result increase the elec-
tric capacitance of the base pair. Both properties enable a
base pair to be a good bipolar capacitor. The recognition
of a base pair as a valid capacitor provides a sharp insight
into molecular electronics.
A base pair is an electric capacitor. Alternate current
passes through th e hydrogen bonds. There are oscillating
waves between each pair of nitrogenous bases within
DNA nucleotides. Hydrogen bonding is a dynamic elec-
tronic action that delivers messages between the two
bases, helping to balance the strain in a DNA double he-
lix. Hydrogen bonds serve as the gateways to deliver
messages. In this way, we describe the hydrogen bonds
as a portal between the pair of bases. This dynamic in-
terpretation is contrary to traditional static model of hy-
drogen bonds. Since the e xpressi on of any forces r equires
message exchanges, it is the wave information conveyed
between pairs of bases that makes hydrogen bonds strong
enough to hold the double helix of DNA together. With-
out the wave message, the atoms on both sides of hydro-
gen bonds would be detached and the organization of
DNA woul d start to u nravel.
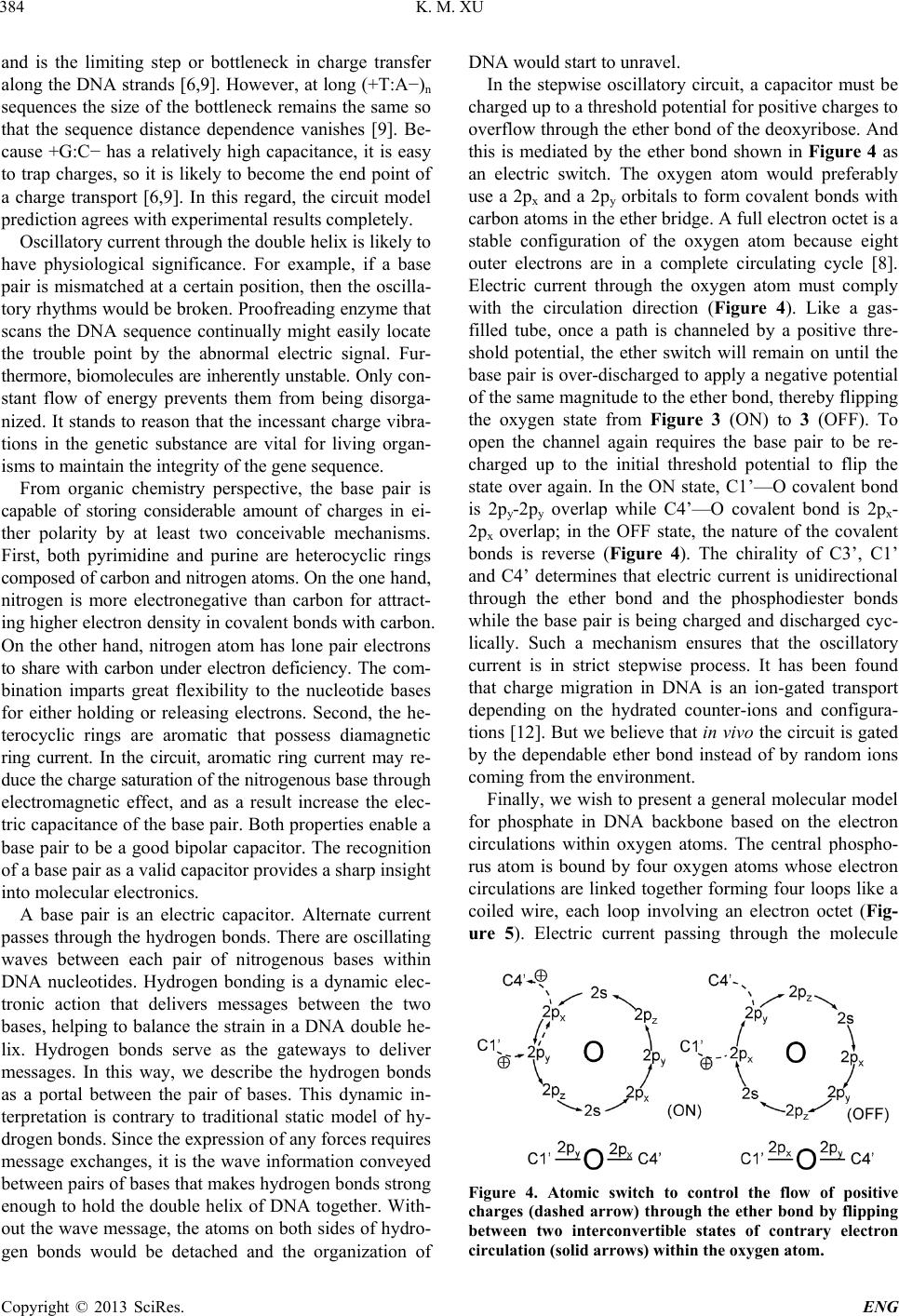
In the stepwise oscillatory circuit, a capacitor must be
charged up to a threshold potential for positive charges to
overflow throug h the ether bond of the deoxyr ibose. And
this is mediated by the ether bond shown in Figure 4 as
an electric switch. The oxygen atom would preferably
use a 2px and a 2py orbitals to form covalent bonds with
carbon atoms in the ether bridge. A full electron octet is a
stable configuration of the oxygen atom because eight
outer electrons are in a complete circulating cycle [8].
Electric current through the oxygen atom must comply
with the circulation direction (Figure 4). Like a gas-
filled tube, once a path is channeled by a positive thre-
shold potential, the ether switch will remain on until the
base pair is over-discharged to apply a negative potential
of the same magnitude to the ether bond, thereby flipping
the oxygen state from Figure 3 (ON) to 3 (OFF). To
open the channel again requires the base pair to be re-
charged up to the initial threshold potential to flip the
state over again. In the ON state, C1’—O covalent bond
is 2py-2py overlap while C4’—O covalent bond is 2px-
2px overlap; in the OFF state, the nature of the covalent
bonds is reverse (Figure 4). The chirality of C3’, C1’
and C4’ determines that electric current is unidirectional
through the ether bond and the phosphodiester bonds
while the base pair is being charged and discharged cyc-
lically. Such a mechanism ensures that the oscillatory
current is in strict stepwise process. It has been found
that charge migration in DNA is an ion-gated transport
depending on the hydrated counter-ions and configura-
tions [12]. But we believe that in vivo the circuit is gated
by the dependable ether bond instead of by random ions
coming from the environment.
Finally, we wish to present a general molecular model
for phosphate in DNA backbone based on the electron
circulations within oxygen atoms. The central phospho-
rus atom is bound by four oxygen atoms whose electron
circulations are linked together forming four loops like a
coiled wire, each loop involving an electron octet (Fig-
ure 5). Electric current passing through the molecule
Figure 4. Atomic switch to control the flow of positive
charges (dashed arrow) through the ether bond by flipping
between two interconvertible states of contrary electron
circulation (solid arrows) within the oxygen atom.

K. M. XU
385
Figure 5. Schematic diagram of electron flow via the conca-
tenated electron circulations within a phosphate bridge for
electric induction corresponding to its chemical structure.
inevitably incurs considerable induction due to the con-
voluted electron flow, which explains why phosphate is
an inductor and perhaps why ATP can serve as a physio-
logical energy source when broken into ADP + Pi even
though the energy of the phosphoanhydride bond is not
so large. The convolution collapse is the key factor in re-
leasing a large amount of inductive energy. In DNA the
electrical tension of phosphate chains must equilibrate
with the mechanical torsion of the strands.
REFERENCES
[1] D. B. Hall, Holmlin, R. E., Barton and J. K. Oxidative,
“DNA Damage through Long-Range Electron Transfer,”
Nature, Vol. 382, 1996, pp. 731-735.
http://dx.doi.org/10.1038/382731a0
[2] K. Fukui, K. Tanaka, M. Fujitsuka, A. Watanabe and O.
Ito, “Distance Dependence of Electron Transfer in Acri-
dine-Intercalated DNA,” Journal of Photochemistry and
Photobiology B: Biology, Vol. 50, 1999, pp. 18-27.
http://dx.doi.org/10.1016/S1011-1344(99)00063-9
[3] C. Wan, T. Fiebig, O. Schiemann, J. K. Barton and A. H.
Zewail, “Femtosecond Direct Observation of Charge Trans-
fer between Bases in DNA,” Proceedings of the National
Academy of Sciences of the USA, Vol. 97, 20 00, p p. 14052-
14055.
http://dx.doi.org/10.1073/pnas.250483297
[4] B. Giese, J. Amaudrut, A.-K. Köhler, M. Spormann and S.
Wessely, “Direct Observation of Hole Transfer through
DNA by Hopping between Adenine Bases and by Tunne-
ling,” Nature, Vol. 412, 2001, pp. 318-320.
http://dx.doi.org/10.1038/35085542
[5] M. E. Nunez, D. B. Hall and J. K. Barton, “Long-Range
Oxidative Damage to DNA: Effects of Distance and Se-
quence,” Chemical Biology, Vol . 6, 1999, pp. 85-97.
http://dx.doi.org/10.1016/S1074-5521(99)80005-2
[6] M. Bixon and J. Jortner, “Long-Range and Very Long-
Range Charge Transport in DNA,” Chemical Physics,
Vol. 281, 2002, pp. 393-408.
http://dx.doi.org/10.1016/S0301-0104(02)00495-0
[7] A. S. Garay, “On the Role of Molecular Chirality in Bio-
logical Electronic Transport and Luminescence,” Life Sci-
ences, Vol. 10, 1971, pp. 1393-1398.
http://dx.doi.org/10.1016/0024-3205(71)90348-1
[8] K. Xu, “The Law of Nature: Spherical Quantity in Dyna -
mic Calculus,” Nova Scientific Publishers, Inc., New
York, 2011, pp. 1-261.
[9] B. Giese, “Electron Transfer in DNA,” Current Opinion
in Chemical Biology, Vol. 6, No. 5, 2002, pp. 612-618.
http://dx.doi.org/10.1016/S1367-5931(02)00364-2
[10] C. Nogues, S. R. Cohen, S. Daube, N. Apter and R. Naa-
man, “Sequence Dependence of Charge Transport Prop-
erties of DNA,” Journal of Physical Chemistry B, Vol.
110, 2006, pp. 8910-8913.
http://dx.doi.org/10.1021/jp060870o
[11] S. D. Wetmore, R. J. Boyd and L. A. Eriksson, “Electron
Affinities and Ionization Potentials of Nucleotide Bases,”
Chemical Physics Letters, Vol. 322, 2000, pp. 129-135.
http://dx.doi.org/10.1016/S0009-2614(00)00391-2
[12] R. N. Barnett, C. L. Cleveland, A. Joy, U. Landman and
G. B. Schuster, “Charge Migration in DNA: Ion-Gated
Transport,” Science, Vol. 294, 2001, pp. 567-574.
http://dx.doi.org/10.1126/science.1062864