Applied Mathematics, 2013, 4, 1637-1646
Published Online December 2013 (http://www.scirp.org/journal/am)
http://dx.doi.org/10.4236/am.2013.412223
Open Access AM
Solutions of Impulsive Diffusion and
Von-Foerster-Makendrick Models Using the B-Transform*
Benjamin Oyediran Oyelami, Samson Olatunji Ale
National Mathematical Centre, Abuja, Nigeria
Received October 2, 2013; revised November 2, 2013; accepted November 9, 2013
Copyright © 2013 Benjamin Oyediran Oyelami, Samson Olatunji Ale. This is an open access article distributed under the Creative
Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original
work is properly cited.
ABSTRACT
In this paper we explore the possibility of using the scientific computing method to obtain the inverse B-Transform of
Oyelami and Ale [1]. Using some suitable conditions and the symbolic programming method in Maple 15 we obtained
the asymptotic expansion for the inverse B-transform then used the residue theorem to obtain solutions of Impulsive
Diffusion and Von-Foerster-Makendrick models. The results obtained suggest that drugs that are needed for prophylac-
tic or chemotherapeutic purposing the concentration must not be allowed to oscillate about the steady state. Drugs that
are to be used for immunization should not oscillate at steady state in order to have long residue effect in the blood.
From Von-Foerster-Makendrick model, we obtained the conditions for population of the specie to attain super satura-
tion level through the “dying effect” phenomenon ([2-4]). We used this phenomenon to establish that the environment
cannot accommodate the population of the specie anymore which mean that a catastrophic stage t* is reached that only
the fittest can survive beyond this regime (i.e. t > t*) and that there would be sharp competition for food, shelter and
waste disposal etc.
Keywords: B-Transform; Impulsive Diffusion; Von-Foerster-Makendrick Models; Residue Theorem; Maple Symbolic
Programme and Asymptotic Expansion
1. Introduction
Impulsive differential equations (IDEs) describe proc-
esses which quickly change their states for short moment
of times when compare to the total evolution time for the
systems. The impulses may happen at fixed or non-fixed
moments and the behavior of state variables describing
the processes may show some “jumps”, “shocks” attri-
butes etc. This kind of impulsive behavior makes the
IDEs not easily accessible to most existing concepts and
theories in differential equations, ecology, biomathe-
matics, engineering and control systems ([2-9]). More-
over, impulsive moments not only depend on some im-
pulsive sets but also on the dynamics of the systems, this
special feature gives rich perspective for investigation for
many real life processes ([2,3,8,10]).
B-transform is an operational calculus method de-
veloped by Oyelami and Ale almost a decade ago for
finding closed solution forms for fixed moment im-
pulsive systems ([1]). Most known existing transform
methods are not known to be applicable to the (IDEs).
It’s interesting to say that the evolution times describing
(IDEs) are intermittently interrupted by small perturb-
ations (impulses) at certain fixed or non-fixed moments.
This peculiarity makes the solutions of IDEs to exhibit
some strange behaviors. Therefore any transform method
for finding solution of IDEs must take into consideration
the impulsive effect of the systems. The B-transform was
developed to take into consideration the impulsive effect
of the system to provide a method for finding solutions
for the fixed moments IDEs ([1,11-13]).
B-transform has been successfully applied to solve a
variety of problems in Biomedicine, Physics, engineering,
control theory etc (see [1,7,12]). One major problem that
one encounters just like most known transform methods
([1]) is how to obtain in the inverse transform for some
particular problems ([14]). In this paper we explore the
possibility of introducing scientific computing method to
obtain the inverse B-Transform. We would make use of
*Dedicated to: Professor V. Lakshimikantham who has contributed ex-
tensively to the development of Differential Equations, Impulsive Dif-
ferential Equations and Nonlinear Analysis.

B. O. OYELAMI, S. O. ALE
1638
some suitable conditions and the symbolic programming
method in Maple 15 to obtain the asymptotic expansion
of the inverse B-transform, then followed by the use of
residue theorem to obtain solutions to Impulsive Diffu-
sion and Von-Foerster-Makendrick models.
We note that, the Impulsive Diffusion model is very
useful for modelling the diffusion of drug across the cells
and tissues in the body and the attendant osmo-regulation
in the apparent volume and also modelling of environ-
mental degradation problems ([5,15]). More recently,
stochastic diffusion models are used for analyzing be-
havior of financial markets. The entropy trap threatens
the existence of man as a result of continuous emission
of carbon dioxide into the air and water. The earth is
getting more saturated carbon dioxide than the oxygen
that plants absorb and release. The sea water is fast
becoming acidic because of dissolution of carbon dioxide
in the water and hence the marine lives are also threat-
ened. The diffusion model considered here or its modi-
fications have potential applications for studying dif-
fusion of carbon dioxide into the atmosphere and the sea
and the long time chaotic effect can be studied from
impulsive perspective.
The theory of taxism, loosely speaking is the tendency
of an organism to move forward or be away from some
stimuli. There are many taxes, positive and negative
which influence presumably direct organisms in favor-
able physical environments ([15]). Most notable or
perhaps widely studied taxes are the chemo taxi that is
response for organisms to be sensitive to chemical chan-
ges. If the response is measured in term of concen-
tration of such chemicals, the impulsive diffusion model
will give a clue to study chemo taxis of the expected
organisms. More research works are expected to be on
taxes in cellular ecology, that is, molecular and cellular
biology. The study of bacterial and viral chemotaxes
from impulsive point of view will offer researchers the
opportunity that has integrated approach to understand
the interaction of genetics, physiology and ecology.
We must emphasize that under certain conditions like
rapid changes i.e. advent of war, earthquake, displace-
ment of persons etc., the population tends to be impulsive
in nature (see [2,8,16-18]). For this reason, we consider
impulsive analog of the Von-Foerster-Makendrick model
of an age-dependent population in given ecosystem. The
model is typical impulsive partial differential equations
and has potential applications in modelling the popula-
tion of species stratified into age groups and epidemiol-
ogy of infectious diseases like malaria and HIV/AIDs.
2. B-Transform
Consider the impulsive differential equations


 
,,, 0,1,2,
k
k
x ftx t k
t
xIxt



(IDE)
where
:
:
nn
n
f
RR R
IR R


f and I are assumed to satisfy the continuity and Lipchitz
conditions for the existence and uniqueness of solution of
(IDE) (see [3,4]).
The B-transform of the function

x
t with impulses
at fixed moments


,1,2,
k
tk during the evolution-
ary process is [1,11]




1
1
nc

x
tq
xx
Bq
(1)
where


C
x
q and


I
x
q are the components of
the B-transform and are defined as
and
c
LI L
 
1
0
d,, 0,1,2,
en
tq
c ck
qxt xtttk
xt
L


 
 (2)
And
   
1
1 1en
k
ok
q
tk
t
tt
qxt Ixt
xL




(3)
where
0,1,2,,nn


; n

is the order of the transform. For
sake of simplicity, we will choose . The advantage
of taking
1n
1n


lies in the derivation of the inverse
transform.
The inverse transform for components of


C
x
q and


I
x
q was obtained (see [1], Theorem 1) as follows:
 
1d
e
2π
vi sq
cc
vi
txq
xi


q

k
x
(4)
 

1,
ok
k
t
tt
tqI
x
t


t (5)
The B-transform is valid in the union of the sets





1
0
:ed ,
,1,2,,1,2,
n
k
tq n
k
A
xtRxtt m
tRtkn



 

 


And








0
2
:e
1, 2,
n
k
k
tq n
kk
ttt
BIxtRI xtm
n



 



,
And such that
Open Access AM

B. O. OYELAMI, S. O. ALE
Open Access AM
1639
 




Diammax, k ABxt Ixt

ing effect”, “loss of autonomy” and “dying effect” are
hampering the rate of its development ([2,4,9,15]).
Let us return to the problem, if we slot


,Ctx into
Equations (6) and (7), we get
where |.| is the n-dimensional Euclidean norm, m1 and m2
are constants that are assumed to exit and finite.


d,,0,1,2,
d
i
iik
Ct kCt t tk
t

  (10) 3. Impulsive Diffusion Problems
Consider an example of application of B-transform to
impulsive partial differential equations. Impulsive partial
differential equations have so many interesting applica-
tions. We will consider a diffusion problem of a porous
media of substrate whose concentration is given by
at time t and a distance of x from the source.
This type of problem is very useful in drug administra-
tion. The underlying mathematical model can be stated as
follows:

,Cxt

 


2
2
d,
d
,0,1,2,
i
ii iiii
k
Cx Cxkrxth ut
x
t k
t



 
(11)



ki k
Ct t ICt


(12)
012
0;
,constants,1,2,
lim
k
ki
k
tttt
t i 



 


  
  
2
2
,,
,
,
ii
iii k
Ctx Ctx
kCt xD
tx
krx thuttt


 

 
(6) Subject to given boundary conditions applying the
B-transform to Equations (10)-(12) and solving the equa-
tions we get
 
,,,0,1
ik ik
Ct tx ICtx k  

  
12
01
1
,
ok
i
ii
i
ttti kk
q
Cq k C
qkq
tqICt







 
 


(13)
,2, (7)
Subject to the boundary conditions

at 0,,0
i
C
x tx
x



(8)

1
deq
k
i
t
t
ik c
t,q q 








(14)

,
at ,0
i
Ctx
x L
x




k



(9)
And solving Equation (11) we get
where

   

sin cos
,d
O
ii i
x
ii i
x
CxAx x
Gstrxsh s s




 (15)

,
i
Ctx
and
i
k

is the concentration of the substance that
diffuses across the apparent volume into gastrointestinal
tracts at time t and the thickness of the tract being x units.
i
are some rate constants, Di the coefficients of
diffusion.
 


,cos
iii
Gst t s  . (16)


i
rxt h accounts for the biological gestation
function lagged by a constant Ci. Now, we determine the inverse of and
the constants

,1,2
i
Cqi
i
A
and . Thus
i
B

i
ut is the relevant biological control function that is
responsible for osmo-regulation of the medium.

  
2
ee
,,1,
ii
ok
kt itk
io
i
ik k
ttt
Ct C
k
ttICti 2,








(17)
Using a transformation of variable, a kind of separa-
tion of variable per say, we can express in the
form where and

,
i
Ctx
  
,CtxCtCxCt


Cx
are purely functions of t and x respectively. This separa-
tion works for this type of problem, for other forms of
impulsive partial differential equations, it may not work.
This kind of features makes the theory of impulsive dif-
ferential equation to be expanding at a rapid rate of late,
even though the discovery of new phenomena like “beat-
The values for constants i
A
and ,
i
B1, 2i

are
found to be




cos 0
iiii
i
i
tr ut
A
 


 (18)


 





32
cos 0coscos
sin
iii iiiiiii
i
ii
tr utL L uL
BL
 

 
 (19)

B. O. OYELAMI, S. O. ALE
1640
Therefore given the require solution to the
model, that is,

,Ctx

   
,,
ee
ii
ok
i ktt k
ttt otk
i
Ctx C ttICt
k









k








 
   

2
sin cos,
d
,
ee
cos
,d
o
i
ok
o
iii ii
x
iii
i ktt k
ttt ok i
i
iii
ii i
x
A
x x Gst
rxth utt
C tt
k
Cx
Gstrxth utt
 

















(20)
where
22 1
,tan i
iiii
i
A
C AB B 

 


The problem exhibits oscillatory behavior at

14 π2
2
i
i
i
k
x




If
 


0,1, 2,
iii
rxt hut i   
At steady state, when ;
t
 
0
lim ,
tCtxC x  

where

 

 
 



(, cos
lim
d.
ok
o
sikkiii
tttt
x
ii iii
x
Cx ttICt Cx
+ Gstrxsh +uss



 
 



(21)
If the system does not oscillate about the points xi as
enumerated above and if it oscillates.

0
s
Cx
From the above derivation, drugs that are needed for
prophylactic or chemotherapeutic purposes the concen-
tration must not be allowed to oscillate about the equilib-
rium at the steady state. Drugs that are to be used for
immunization should not oscillate about the equilibrium
at steady state in order to have long residue effect in the
blood.
4. Impulsive Population Model
We consider impulsive analog of the Von-Foerster-Mak-
endrick model of an age-dependent population in given
ecosystem. The equation is given by
 
12 ,,, ,k
NN
txNFNtxt t
xt
 


 
 (22)


,0Nx x

t
(23)
 
0
0,,, dNt xtNxt 

 (24)




,
k
Nxt t INxt ,
k
(25)
012
lim
0,
kk
tt t t t
k.




where ,
:,NDR R



CR 




0,R

 ,


t,Nx is the population of a single species in given
ecological set-up at time t and x age group. If




,
k
INxt 
if
0 substrate (biomass) is taken away and




,
k
INxt 0

biomass is added to the environment
([3]).
We must emphasize that under certain conditions like
rapid changes i.e. advent of war, earthquake, displace-
ment of persons etc., and the population tends to be im-
pulsive in nature [18].
++
: D R
RR
  
:
F
RR



 Is the growth rate, F is a non-linear function and
,1,2
ii


. are some relevant rate constants. Applying
B-transform to model we arrive at
 



 
 
  
 
0
1
0
0
0
2
12
,, ,d
e
,, ,
e
,,d
e
,d ,
e
1
,,d
e
,,
k
ok
tq
cc
qt
Ik
ttt
tq
c
tq
tq
c
c
cc
LN xtNxqN xtt
LNxtNxq INxt
LNxt NxttNxq
xx x
Nxtt Nxq
xx
LNxtNxtt xq
N
xx q
NxqNxqx Gxq
xq









 



 

















,
,.
,
(26)
  
 

0
11
00
11
00
,,,,
e
0,d,,d d.
,,dd.
e
tq
tq
c
tq
GxqtxNFNtxt
Nq txtNxttt
e
xtNxttt












1
d

Substituting the value of and then solving
Equation (26) for

,
c
Nxq


,
c
Nxq for fixed q, we get
Open Access AM

B. O. OYELAMI, S. O. ALE 1641
   
 
22
111
11
00
d, 1
,
d
0,e,,d d.
c
c
tq
c
Nxq aa
Nxqx Gxq
xaqa a
Nq xtNxttt


 


1
,
(27)
Solving Equation (27) we get
 
 
2
1 0
2
1 0
1
,0,exp
1
,0,exp
cc
cc
NxqNq s
q
NxqNq s
q
















d
d



,.
(28)
Therefore
 
1
,,
c
NxqNxq Nxq
It follows that
 
  

1
1
,,
e
2π
,,
ok
i
tq
cc
i
kk
ttt
Nxt Nxqq
i
Nxq ttINxt










d
,
,.
(29)
Let C be contour for which the integration is carried
out in the complex plain.
Therefore

1
,,
c
Nxt Nxt Nxt (30)
 
 


0
2
1
222
111
2
1
1d
,0,exp
2π
d
exp ,
1
expdde,
k
k
x
c
Ca
xx
Ca a
xtq
k
attt
as
Nxt Nqtqq
iaq
aaa
stqsGx q
aqa a
asq INxt
aq










 





 



 


 


d 



(31)
We note that the system has no solution passing
through if
 
,tNt

 

122
1
,,0,1,
,
ik
k
IN ttk
CN tttC 

 
2,
where are possitive constans such that
i
C

2
2
22
,,
k
C
C
tt Ntt




 0.
This model wills exhibits the ‘dying effects’, which
mean that the solution is not continuous across some
natural boundary containing the solution ([2-4]). To this
particular model, this means that the population of the
specie attains super saturation level such that after
time the population starts showing some strange
behavior that the population cannot be quantified.
t
tt


The “dying effect” as tt

 suggests that the envi-
ronment cannot accommodate the population of the spe-
cie any more. By Darwin’s theory a catastrophic stage is
reached that only the fittest can survive beyond this re-
gime (i.e. tt

); there expected to be sharp competition
for food, shelter and waste disposal etc. It is interesting
to note that dying effect does not exist if 20



,,
.
We consider special cases of Equations (22)-(25).
Case I
When if μ = const and


F
NxtNtx.
Then
   

2
1
2
0,
,
1
c
c
Nqx s
Nxq x
x








0
d
x
x s 

(32)

2
1
:exp
x
xq






. Thus using Equations (28), (30)
& (32), we find


,Nxt for this particular case. If
2
20,0,0 and xx



 
Then
  
0
,
x
s 
22
1
,0d
cc
NqxN qs
xx











By asymptotic expansion using Maple 15 Symbolic
Programming at dos command prompt:
> asympt (exp (theta/q), q);
23 4
23 46
12624 120
qqqqq
 
  
5
5
1
O
q



> subs (theta = alpha [2]/alpha [1]*x, %);
22 3355
22 22
22 33556
111 1
1
126 120
xx xx
O
qqqqq
 
 

 



> %*exp (t*q);
22 3355
22 22
22 33556
111 1
1
1e
2 6120
tq
xx xx
O
qqqqq
 
 


 






And the asymptotic expansion of NI(tk,q) being

2
5
62
0
35 4
345
1
1e
2
,6e
11
1
6 120 24
tq
tq k
tq
Ik k
k
tq tq tq
kk
k
et I
Ntq IOtI
q tq
et Iet I
etI
qq q





 

 






 



where the summation over is taken over




,,0
kk
.
I
INt ttt


Therefore
Open Access AM

B. O. OYELAMI, S. O. ALE
Open Access AM
1642

 

 

22 3355
22 22
22 3355
111 1
6
2
0
0
,
11
2π26 120
0,e d
1
e0,d 2π
e
1dd ,
4πk
C
tq
c tp
C
C
tq x
kk
tt
C
Nxt
xx xx
iq qq q
Nq q
NqqO
iq
s
sqttINt
it
 
 






















,
N

,
F
NtNKN K
K
 is the saturation (maxi-
mum) population the environment can support. For this
case, we found that
  


  
22 2
2
2
1
,1 ,
0,d ,
cc
x
c
x
x
Nxq xxNxq
K
Nqx xss










It follows that
Case II: For μ = const and






 

2232
2
1
2
,
4
11 10,d
2
c
t
co
Nxq
x
x
x xx xNq xss
K
xx
K


  




 



 (33)






 

2232
2
1
2
,
4
11 10,d
2
c
t
co
Nxq
x
x
x xx xNq xss
K
xx
K


 




 



 (34)
And is any real number.
0
If we slot Equations (33) and (34) into Equations (29)
and (30) the solution to the model can be found for cases
II and I respectively.
qIf 1

 and K is very large than

1
,2
22
c
B
Nqx y
A




If we let Hence


2
1
,
1exp2expd0
2π
as
c
C
Ntx
x
Ktqq
xq
x








 
 
2
2
2
2
2
,1, 0,
8
d and .
c
x
x,
A
BxCNq
K
A
Dxssy
B




 
 

x


We can approximate


,
c
Nxq
 in Taylors series us-
ing the Maple 15 software as Since is analytic in C and by the Cauchy
integration theorem it is zero.
exp d
C
tqq



23
456
11 1
,11
2281
57
128 256
c
B
Nqxyyy
A
yyOy







 

The asymptotical expansion for by Dos
command prompt in Maple 15 we have:

,
c
Ntx
6
(35)
> N[c](x,q):=1/2*pi/i*int(%%*N[c](0,q),q)+alpha2/2*
int(exp(t*q),q)*int(phi(s), s=0…x);

  
22 334455
22 222
2223344
111 11
2
26
0
,1
16 π2624 120
0, e
11
0,ddedd
4π
4π
cC
tq x
Ctq
cC
C
xx xxx
K
Nxq q
xiq qqq
Nq
KK
NqqOq qss
xixit
q
 


















55


where the integration is take over a contour C containing
the pole of order . By the use of Cachy-
Goursat theorem, the integral can be reduced to only an
integration around a small circle about the pole 0q6k0q

of

B. O. OYELAMI, S. O. ALE 1643
order . The value for 6ked
tq
k
Cq
q
 can be obtained
by the use of residue theorem as





1
1
0
0
ed
1de
2πRee2π
1!d
2π
1!
tq
k
C
ktq
tq
k
q
q
k
q
q
is i
kx
t
ik








(37)
Substitute the above value for gives
the asymptotic approximation for and
where
0,1, 2,, 6k

,
C
Ntx
  
,,
IC
NtxN txNtx

,





 

1
0,0,,5
6
66
1
,
!
,,
k
kk
Ik
ttk
k
Ntxt t
k
INtxtOtINt xt

 


 (38)
By residue theorem which is unbounded as
if and excepttx
 

 
0
0
,exp
where 1 and 0 are constants.
kk k
k
k;
I
Nt xttt
xt
k






The Maple was used to find involving some
special functions see the appendix and the use of the
residue theorem can then be applied to complete the so-
lution.

,
c
Ntq
5. Conclusions
From the two models considered, the B-transform has
been demonstrated to offer simple way for solving fixed
moment impulsive system (see [19]) for more applica-
tions). More works need to be done on applications of
B-transform especially taxism problems by studying the
response of living organisms to stimulus. Moreover, in
molecular chemistry, the use of impulsive diffusion mod-
el can be explored to investigate the movement of che-
mical substances in and out cells and tissues of organ-
isms.
Furthermore, the Von-Foerster-Makendrick models
can be further exploited to study epidemiology of infec-
tious diseases like malaria and HIV/AIDS. In view of
these, it is recommended that more work should be done
on the applications of B-transform couple with the use
symbolic programming approach to solve problems.
6. Acknowledgements
The authors are grateful to the National Mathematical
Centre, Abuja, Nigeria and the Kaduna State University
Kaduna, Nigeria for their supports.
REFERENCES
[1] B. O. Oyelami and S. O. Ale, “B-transform Method and
its Applications, in obtaining Solutions of some Impul-
sive Models,” International Journal of Mathematical Edu-
cation in Science and Technology, Vol. 31, No. 4, 2000,
pp. 525-538. http://dx.doi.org/10.1080/002073900412633
[2] S. O. Ale and B. O. Oyelami, “Impulsive System and
Applications,” International Journal of Mathematical
Education in Science and Technology, Vol. 31, No. 4,
2000, pp. 539-544.
http://dx.doi.org/10.1080/002073900412642
[3] D. D. Bainov, V. Lakshikantham and P. S. Simeonov,
“Theory of Impulsive Differential Equations,” World
Scientific Publication, Singapore, 1989.
[4] P. S. Simeonov and D. D. Bainov, “Theory of Impulsive
Differential Equations: Periodic Solutions and Applica-
tions,” Essex, Longman, 1993.
[5] E. Beltrami, “Mathematics for Dynamic Modeling,”
Academy Press, London, 1987.
[6] B. O. Oyelami, “On Military Model for Impulsive Rein-
forcement Functions using Exclusion and Marginalization
Techniques,” Nonlinear Analysis, Vol. 35, No. 8, 1999,
pp. 947-958.
http://dx.doi.org/10.1016/S0362-546X(98)00114-X
[7] B. O. Oyelami, S. O. Ale, P. Onumanyi and J. A. Ogidi,
“B-Transform Method and Application to Sickle Cell
Aneamia,” 2008, pp. 202-220.
http://sirius-c.ncat.edu/asn/ajp/allissue/ajp-ISOTPAND/in
dex.html
[8] B. O. Oyelami and S. O. Ale, “On Existence of Solution,
Oscillation and Non-Oscillation Properties of Delay
Equations Containing ‘Maximum’,” Acta Applicandae
Mathematicae Journal, Vol. 109, No. 3, 2010, pp. 683-
701. http://dx.doi.org/10.1007/s10440-008-9340-1
[9] P. S. Simeonov and D. D. Bainov, “Impulsive Differential
Equation: Asymptotic Properties of the Solutions,” World
Scientific Publication, Singapore, 1989.
[10] S. G. Pandit and H. Deo Sudashiv, “Differential Systems
Involving Impulsive. Lecture Notes in Maths,” Springer-
Verlag, Berlin-Heidelberg-New York, 1982.
[11] B. O. Oyelami and S. O. Ale, “B-Transform and Its Ap-
plications to a Fish-Hyacinth Model,” International Jour-
nal of Mathematical Education in Science and Technol-
ogy, Vol. 33, No. 4, 2002, pp. 565-573.
http://dx.doi.org/10.1080/00207390210131353
[12] B. O. Oyelami, S. O. Ale, P. Onumanyi and J. A. Ogidi,
“Impulsive HIV-1 Model in the Presence of Antiretroviral
Drugs Using B-Transform Method,” Proceedings of Af-
rican Mathematical Union, Vol. 1, No. 1, 2003, pp. 62-
76.
[13] B. O. Oyelami, S. O. Ale and P. Onumanyi, “Impulsive
HIV Model Using B-Transform,” The Proceedings of Na-
tional Mathematical Centre on Conference on Computa-
tional Mathematics, Vol. 2, No. 1, 2005, pp. 50-64.
http://nmcabuja.org/nmc_proceedings.html
Open Access AM

B. O. OYELAMI, S. O. ALE
Open Access AM
1644
[14] B. Davies, “Brian Davies Integral Transforms and Their
Applications,” Springer Publisher, Berlin-Heidelberg-
New York, 2002.
[15] M. B. V. Robert, “Biology; a Functional Approval,” Nel-
son Butler and Tanner Ltd., Rome and London, 1971.
[16] V. Lakshimikantham and Z. Dric, “Positivity and Bound-
edness of Solutions of Impulsive Reaction-Diffusion
Equations,” Journal of Computational and Applied Ma-
thematics, Vol. 88, No. 1, 1988, pp. 175-184.
http://dx.doi.org/10.1016/S0377-0427(97)00210-0
[17] L. H. Erbe, H. I. Freeman, X. Z. Lin and H. J. Wu, “Com-
parison Principles for Impulsive Parabolic Species Grow-
th,” The Journal of the Australian Mathematical Society,
Series B, Vol. 32, No. 4, 1991, pp. 382-400.
[18] S. O. Ale and B. O. Oyelami, “On Chemotherapy of Im-
pulsive Models Involving Malignant Cancer Cells,” Aba-
cus, Journal of Mathematical Association of Nigeria, Vol.
24, No. 2, 1996, pp. 1-10.
[19] B. O. Oyelami and S. O. Ale, “Impulsive Differential
Equations and Applications to Some Models: Theory and
Applications. A Monograph,” Lambert Academic Pub-
lisher, Saarbrücken, 2012.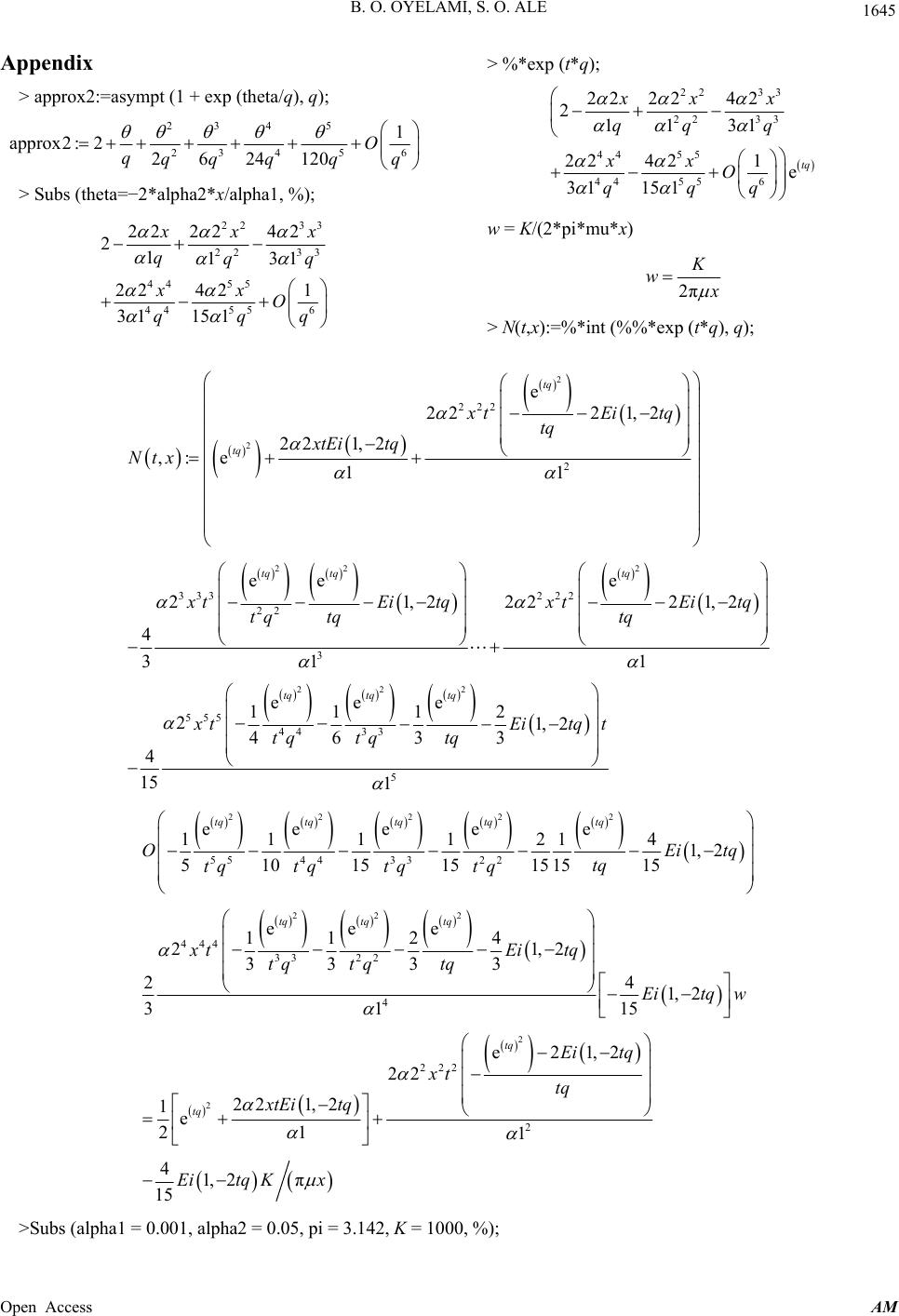

B. O. OYELAMI, S. O. ALE 1645
Appendix
> approx2:=asympt (1 + exp (theta/q), q);
23 45
23 456
1
approx2:22624 120 O
qqqqqq
 

 

> Subs (theta=−2*alpha2*x/alpha1, %);
22 33
22 33
44 55
4455 6
222242
21131
22 421
31 151
x
xx
qqq
xx
O
qq
 



 

 


> %*exp (t*q);

22 33
22 33
44 55
4455 6
22 2242
21131
22421 e
31 151
tq
xx x
qqq
xx
O
qqq
 



 




 





w = K/(2*pi*mu*x)
2π
K
w
x


q
> N(t,x):=%*int (%%*exp (t*q), q);



















2
2
22 2
2
222
2
333 222
22
3
555
44
e
222 1,2
22 1,2
,: e11
ee e
21,222
4
31
1
ee
11
246
4
15
tq
tq
tq tqtq
tq tq
xtEitq
tq
xtEi tq
Ntx
21,2
x
tEitqxtEi
tq tq
tq
xt tq


















 
















tq








22
33
5
e
12
1, 2
33
1
tq
Eitq t
tq tq































22222
22 2
2
55 44 33 22
444
33 22
4
2
eeee e
11 1 1 21 4
1, 2
5101515151515
eee
11 24
21,2
33 33
24
1, 2
31 1
22
22 1,2
1e
21
tq tq tq tqtq
tq tq tq
tq
OE
tq tq tqtq tq
xtEitq
tq
tq tq
Eitq w
xtEi tq







   








5
itq









 






2
22
2
e21,2
1
41,2π
15
tq Ei tq
xt tq
Eitq Kx










>Subs (alpha1 = 0.001, alpha2 = 0.05, pi = 3.142, K = 1000, %);
Open Access AM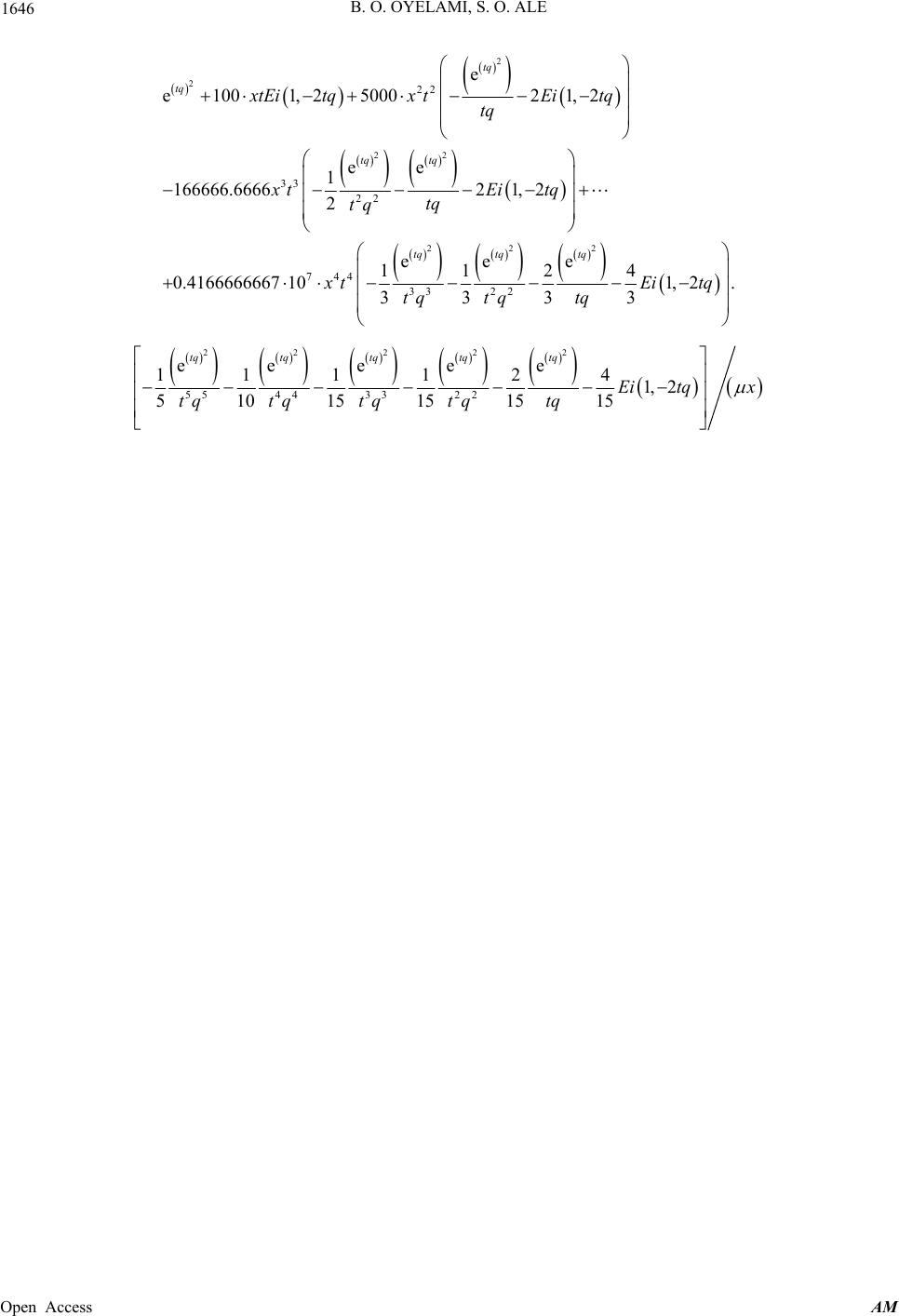

B. O. OYELAMI, S. O. ALE
1646


















2
2
22
22 2
22
33
22
744
33 22
e
e1001, 2500021, 2
ee
1
166666.666621, 2
2
eee
11 24
0.4166666667 101,2.
33 33
tq
tq
tq tq
tq tqtq
xtEi tqxtEi tq
tq
xtEi tq
tq
tq
x
tE
tq
tq tq


 
















itq













22222
55 44 3322
eeeee
111124
1, 2
51015151515
tq tq tq tq tq
Ei tqx
tq tq tqtq tq







Open Access AM