Modern Mechanical Engineering, 2013, 3, 191-201
Published Online November 2013 (http://www.scirp.org/journal/mme)
http://dx.doi.org/10.4236/mme.2013.34026
Open Access MME
Analytical Solutions of Dynamic Crack Models of Bridging
Fiber Pull-Out in Unidirectional Composite Materials
Yuntao Wang1, Yunhong Cheng2, Nianchun Lü3, Jin Cheng4
1College of Mechanical Engineering, Liaoning Technical University, Fuxin, China
2Department of Civil Engineering, Northeastern University, Shenyang, China
3School of Material Science and Engineering, Shenyang Ligong University, Shenyang, China
4Department of Astronautics and Mechanics, Harbin Institute of Technology, Harbin, China
Received September 22, 2013; revised October 29, 2013; accepted November 15, 2013
Copyright © 2013 Yuntao Wang et al. This is an open access article distributed under the Creative Commons Attribution License,
which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
ABSTRACT
An elastic analysis of an internal central crack with bridging fibers parallel to the free surface in an infinite orthotropic
anisotropic elastic plane was analyzed, and the crack extension should occur in the format of self-similarity. When the
fiber strength is over its maximum tensile stress, the fiber breaks. By means of complex variable functions, the problem
considered can be easily translated into Reimann-Hilbert mixed boundary value problem. Utilizing the built dynamic
model of bridging fiber pull-out in unidirectional composite materials, analytical solutions of the displacements, stresses
and stress intensity factors under the action of increasing loads Pt5/x5, Px5/t4 are obtained, respectively. After those ana-
lytical solutions were used by superposition theorem, the solutions to arbitrary complex problems were acquired.
Keywords: Composite Materials; Bridging Fibers; Analytical Solutions; Crack; Variable Loads
1. Introduction
It is well known that the matrix cracking as well as frac-
ture process of the bridging fibers is one of the signifi-
cant mechanisms of the cracking expansion in fiberrein-
forced composite materials, such as unidirectional fiber-
reinforced brittle matrix composites [1,2], and threedi-
mensional fiber-reinforced composites with an ortho-
gonal fiber structure [3]. Literature [4] proposed an ap-
proach for the assessment of the distribution of the trac-
tion force for a crack with bridging fibers in an infinite,
orthotropic elastic plane under a uniform remove tension
stress. Most researchers, such as Woo, Lee and Tsai [5-7]
etc, almost investigated static problems of composite ma-
terials; moreover, they obtained only numerical solutions.
Literature [8] set up a model of bridging fiber pull-out,
but it also acquired the numerical solutions under the sta-
tic conditions. It is indispensable to consider the mecha-
nical analysis of matrix cracking with bridging fibers, so
as to evaluate the distribution of the axis traction force in
each fiber. However, the fractures of composite materials
often arise in dynamic conditions, so accordingly it is ex-
tremely important to research their fracture dynamics
problems. In an orthotrpic medium, elastodynamics crack
problems were studied and closed solutions were also
gained, but bridging fiber pull-out problems weren’t
dealt with in literatures [9,10]. Bridging fiber pull-out is
very complicated and cockamamie in dynamic fracture
process of composite materials, so a lot of difficulty must
be overcome in studying dynamic crack expansion prob-
lems on bridging fiber pull-out of composite materials.
When composite materials occur in a crack, bridging
fiber pull-out often exists ahead of the crack tips, and this
is a frequent phenomenon.
Because the fiber failure is governed by maximum ten-
sile stress, which appears at the crack plane, the fiber
breaks and hence the crack propagation should occur in a
self-similar fashion. The fiber breaks along a transverse
line and therefore present a notch [8,11-12]. When a
crack runs at higher velocity, bridging fiber pull-out still
exists in the dynamic case of composite materials, which
are more important than those in the statics.
The problem under consideration is that of a crack,
moving in one plane, presumed to nucleate from an in-
finitesimally small micro-crack with maximum velocity
from the start. This modality of symmetrical crack, run-
ning with constant velocity in both the positive and
negative directions of the x-axis, has been researched by
V

Y. T. WANG ET AL. 192
Broberg [13] and Craggs [14]. Both considered motions
in materials postulated to be homogeneous and isotropic,
as regards stress-strain relationships and fracturing char-
acters. If the fiber failure is governed by maximum ten-
sile stress, which appears at the crack plane, the fiber
breaks and hence the crack expansion should occur in the
modality of self-similarity [13,14]. The fiber breaks
along a transverse line and therefore presents a notch [7,
11-12]. When a crack runs at higher speed, bridging fi-
bers still exist in the dynamic situation of composite ma-
terials, which are more significant than those in the stat-
ics. Since bridging fibers can result in stabilizing effect
of crack extension problem along the original notch
plane, the dynamic fracture influence on bridging fibers
of composite materials will be shown in detail; at the
same time, stresses and displacements as well as stress
intensity factors are deduced properly.
In this paper, the dynamic expansion problem on an
internal central crack with bridging fibers of composite
materials is analyzed by the ways of Keldysh-Sedov
mixed boundary value problem, and analytical solutions
for unidirectional reinforced material with fibers parallel
to the free surface are shown. First, the solution of a sole
dislocation in an elastically half-plane is derived from the
uses of complex variable analysis. The crack is then dis-
played in terms of a consecutive distribution of disloca-
tion. This solution which has relation to a bridging fiber
force induces a system of self-similar functions with dis-
location density as unknown quantities. Then self-similar
functions are resolved analytically by means of Keldysh-
Sedov’s method.
2. A Dynamic Model of Bridging Fiber
Pull-out of Composite Materials
When fiber-reinforced composite materials occur a crack,
phenomenon of bridging fiber pull-out will often occur
ahead of the crack tips and off x-axis on occasion, and
analyzing crack problems in this situation is more diffi-
cult. Composite materials are often referred to as ortho-
tropic aniostropic body in virtue of the direction of their
fibers, while bridging fibers play an important role in
their strength, consequently queries on bridging fiber
pull-out are one of the most complex advancing tasks in
mechanics of composite materials. When a crack moves
with high speed, bridging fiber pull-out phenomenon of
composite materials is still likely to exist. Because the
problems of bridging fibers are more complicated and
cockamamie, there is a lot of difficulty in mathematical
calculations. In order to resolve dynamic fracture queries
of bridging fiber pull-out of unidirectional composite
materials, it is indispensable to establish a suitable sym-
metrical dynamic model of bridging fiber pull-out, hence
fracture dynamics problems of bridging fiber pull-out of
composite materials are effectively solved.
2.1. Characterization of Dynamic Fracture
Problems Concerning Bridging Fiber
Pull-Out
The problem of an internal central crack with bridging
fiber pull-out of composite materials is analyzed under
the dynamic conditions by means of Reimann-Hilbert
mixed boundary value, and that analytical solutions for
unidirectionally reinforced composite materials with bri-
dging fibers parallel to the free surface are presented.
This solution in conjunction with a bridging fiber force
gives rise to a system of self-similar functions with dis-
location density as unknown units. The self-similar func-
tions are solved analytically using Reimann-Hilbert me-
thod. In order to settle efficaciously fracture problems on
bridging fibers of composite materials, it is inevitable to
establish dynamic models of bridging fibers. Since
bridging fibers can conduce a stabilizing effect on crack
extension problems along the original notch plane, the
dynamic fracture effect of bridging fiber pull-out in com-
posite materials will be shown, at the same time, stresses
and displacements as well as stress intensity factors are
deduced appropriately. In order to resolve efficiently
fracture problems of bridging fiber pull-out of composite
materials, proper dynamic models of bridging fiber pull-
out must be built. Only this approach, can a dynamic
crack expansion problem of bridging fiber pull-out of
composite materials obtain content solutions.
2.2. Base of A Dynamic model of Bridging Fibers
The crack is postulated to nucleate an infinitesimally
small micro-crack situated along the x-axis in the form of
self-similarity with the high speed extension, and to run
symmetrically in the positive and negative x directions
with the constant crack tip velocity V in the matrix.
Bridging fiber pull-out of composite materials discussed
is modeled as a two-dimensional region, having a sole
row of parallel, same, equally spaced fibers, separated by
matrix [15,16]. Initial breaks originate from an arbitrary
number broken fibers leading to the fiber breaks along a
transverse line and therefore present a notch. Further-
more, an arbitrary number of self-similar (off-axis) fiber
breaks, i.e. fiber pull-out, with symmetry at the origin of
coordinates and along a transverse line are also consid-
ered. A schematic of a dynamic model of bridging fiber
pull-out configuration is described in Figure 1. Since the
configuration in Figure 1 is symmetry both in geometry
and loading, respectively, with respective to y-axis, only
the right half-plane of the zone needs to be considered
for analyses. The fibers and the matrix are taken to be
linearly elastic. It is further presumed that the fibers have
a much higher elastic modulus in the axial direction than
the matrix and therefore the fibers are usually taken as
supporting all of the axial loadings in composite materi-
Open Access MME

Y. T. WANG ET AL. 193
y
x
t

Vt
t


Vt
Figure 1. Schematic of a dynamic model of bridging fiber
pull-out configuration.
als. Load is transferred between adjacent fibers through
the matrix by a straightforward shear mechanism [15,16].
The shear stresses are independent of transverse displa-
cements and the equilibrium equation in the fiber direc-
tion reduces to an equation in the longitudinal displace-
ments alone, as is a typical of shear-lag theory [11,12].
The solution approaches and modeling procedure put
forward by [11] will be utilized, which discussed static
problems, the fiber fractures in turns occur along two
sole planes, i.e. the fiber fracture was self-similar fiber
(off-axis) break. By virtue of the point, break lie is same
in geometry off x-axis, i.e. break is symmetry about the
origin of the coordinate. In short, the fiber fracture was
self-similar fiber (off-axis) break and presented a notch.
Bridging fiber pull-out occur ahead of the crack tips and
off x-axis. Bridging fibers do not break in the area of the
crack tips along the crack plane, but the others fracture
off the further of the crack tips, i.e. at the central section
of crack or notch. When the crack runs, fibers continu-
ously break with the constant velocity α according to my
assumption. As shown in Figure 1, at y = 0, the realm of
crack or notch in matrix is
x
Vt

; and the fibers broke
at the extent of
x
t

; while the zone of bridging fi-
bers is txVt
 , respectively [15,16]. At y ≠ 0, the
bridging pull-out positions locate at |x| > Vt [8].
Evidently, the dynamic model of crack extension pro-
blem with bridging fiber pull-out in Figure 1 is clarified
by that in Figure 2. This is a model of symmetrical crack
propagation, running with constant velocity V in both the
positive and negative directions of x-axis, at the same
time, bridging fibers fracture with constant velocity α.
The area of bridging fibers has the symmetrical state with
respect to y-axis [15,16]. Each bridging fiber is replaced
by a pair of vertical traction forces which act at the points
with the same x-coordinate on the upper and lower crack
surfaces, but in an opposite direction. Each bridging fiber
is postulated to be balanced with the fracture load of a
fiber from the matrix. The present model has the symme-
tries of geometrical and mechanical conditions with re-
spect to the x- and y-axes on account of the symmetrical
y
x
t

Vt
t


Vt

Figure 2. Dynamic model of crack-face bridging fiber zone.
crack expansion. At y = 0, traction forces act in the zone
of txVt 

, which represent fibrous tensile stresses
which don’t act in the rest of the crack [15,16]. Bridging
fibers of composite materials are usually arranged tightly,
separated by matrix, therefore bridging fiber traction
forces are presumed to be distributed consecutively. At y
= 0, txVt 

, bridging fiber pull-out has symmetry
with respective to the origin of the coordinate; the dis-
placements of the crack face are not the same, but trac-
tion forces of bridging fibers are identical [15-17]. In
short, traction forces of bridging fibers are homogenous
in this section, whose magnitude is P assumed. On the
other hand, when the crack extends with high speed, the
magnitude of crack will increase with time t; the longer
the crack spreads, the more fibers break. The above
analyses are postulated that fibers in matrix are distrib-
uted uniformly, and each fiber has the identical strength,
moreover, the fracture fibers and matrix simultaneity
occur in the same segment of the crack expansion plane
[7,15-17]. It is distinct that traction forces are larger near
the points of ± αt, and they are smaller close to the points
of ± Vt. When the crack runs at high speed, its dimension
has relation to the parameters x and t, and the surfaces of
the crack subjected to loads must also be related to x and
t. When the fracture occurs, both the fiber and the matrix
are in the same plane of crack expansion [15-17]. Of
course, this is an assumed model which maybe not coin-
cident with that in practicality. We can reasonably inter-
pret this to mean that, outside the crack or notch, condi-
tions are steady-state because the crack had no effect it,
after all we can only expect the crack to affect apprecia-
bly the part of the body which lies in a certain proximity
to it.
3. Universal Expressions of Electrodynamics
Equations for Orthotropic Anisotropy
In order to solve efficaciously fracture dynamics queries
of bridging fibers of composite materials, solutions will
be attained under the action of point forces for mode I
motive crack. In terms of the theorem of generalized
functions, the problems dealt with unlike boundary con-
Open Access MME

Y. T. WANG ET AL. 194
ditions will be facilely translated into Reimann-Hilbert
mixed boundary value problem by means of self-similar
functions, then correlative solutions will be obtained.
Postulate at y = 0 that there are any number of loaded
sections and displacement sections along the x-axis, and
the ends of these segments are running with unlike con-
stant velocity. At t = 0, the half-plane is at rest. In these
sections the loads and displacements are discretionary
linear compages of the following functions [15-22]:
 
11
dd
ks
ks
dd
ks
f
xft
xt
 (1)
Where (2)
Here k, k1 and s, s
1 are discretionary integer positive
nu
ccessive function of two variables x
an

00
0
ii
f






mbers [15-22].
A discretional su
d t may be shown as a linear superposition of Equation
(1), therefore resolving loads or displacements with the
form of Equation (2) will possess significance in princ-
ple. Introduce the linear differential operator as well as
inverse:
mn
mn
L
x
t


, inverse:
mn
mn
L
x
t




 (3)
Here +m +n,
 m  n and 0 represent the (m + n) th or-
de
namics equation
of
r derivative, the (m + n) th order integral and func-
tion’s self. It is facile to prove that there exist constants
m and n, when L is put into Equations (1), (2), homoge-
neous functions of x and t of zeroth dimension (homoge-
neous) are gained. The coefficients m, n will be called
the indices of self-similarity [15-22].
Using relative expressions of elastody
motion for an orthotropic anisotropic body [15-22]:
For the case when function Lv is homogeneous [5,6]:
00
,
y
y
vLvL

  (4)
For the case when function Lσy is homogeneous:
00
,yy vLvtL t   (5)
The relative self-similar functions are as [15-23]:
  
00
Re,1 RevW tF
y

  (6)
Where: v0 and 0
y

23]
in Equations (4)-(6) ar
tio
e the nota-
n in [15-17,20-, and they are relevant variables τ
and t which directly work out displacements and stresses
by the course of respective calculations in Equation (7).
 
WDDF
1


 

(7)
Where: τx/t, F(τ), W(τ) are self-sim
Th
D1(τ)/D(τ) in the neighborhood of the subsonic speeds is
ic body. Assume at the ini-
tia
mat of the Solution of
Symmetrical Dynamic Extension Query
At o
apt the Cartesian co-
= ilar functions.
e values of D1(τ)/D(τ) can be ascertained from Appen-
dix 1 of literatures [15-17,20-22], indicated here are only:
purely imaginary for the considered values. Thus, elas-
todynamics problems for an orthotropic anisotropic body
studied can be changed into seeking the sole unknown
function problems of F(τ) and W(τ) for which must meet
the boundary-value conditions. In the universal situation
this is Riemann-Hilbert problem in the theory of complex
functions (in the simplest cases we have Keldysh-Sedov
or Dirchlet problem), this kind of problem is facilely set-
tled by the usual approaches, for example, in the books
by Muskhelishvili [24,25].
Fracture dynamics problems will be investigated for an
infinite orthotropic anisotrop
l moment t = 0 a crack occurs at the origin of coordi-
nates and begins spreading at constant velocity V (for the
subsonic velocities) along the positive direction of x-axis;
and at t < 0, the half-plane was at rest. The surfaces of
the crack are subjected to the unlike types of loads under
the plane strain states.
4. Fundamental For
Concerning Mode I Crack
the initial moment t = 0, a micro-crack is supposed t
pear in an orthotropic anisotropy. Le
ordinate axes align with the axes of elastic symmetry of
the body. The problem considered is restricted to motion
in the x-y-plane. The crack is moving symmetrically with
constant velocity V along the positive and negative direc-
tions of x-axis respectively. The problems will be chang-
ed into the following boundary condition queries:





1
,0,,,
y
,0 , 0,
x
tfxtxVt 
vx tx Vt


 (8)
Introducing the variable τ = x/t. By m
correlative expressions and
eans of the above
 
tx xt   in the the-
or y of generalized functions [26-28], the boundary con-
ditions can be transformed as:



2
Re, ,
Re 0,
F
fV 
WV 

 

 (9)
In the light of the relationship of F
Equation (7) and the previous conditions, the format of
so
(τ) and W(τ) in
le unbeknown function W′(τ) can be confirmed:


3,Wf








 (10)
The problems can reduce to Keldysh-Sedov problem:



Re 0,;
Im 0,
V
V
 
 



 (11)
Considering symmetry and the
plane corresponding to the origin of coordinates of the
ph
infinite point of the
ysical plane as well as singularities of the stress at the
Open Access MME

Y. T. WANG ET AL. 195
crack tip [29,30], the solution in the above problems can
be readily deducted by literatures [15-16,20] as:

,TV V

 

(12)
Using Equations (6) or (7), we will e
stress, the displacement and the stress int
un
s with
e mate-
spread at constant
ve
asily obtain the
ensity factor
der the conditions of mode I crack extension problems.
5. The Solutions of Practical Problems
In order to solve symmetrical dynamics problem
bridging fiber pull-out of unidirectional composit
rials, solutions will be found under the conditions of dif-
ferent loads for mode I expanding crack. According to
the theorem of generalized functions, the unlike bound-
ary condition problems investigated will be translated
into Keldysh-Sedov mixed boundary value problem by
the approaches of self-similar functions, and the corre-
sponding solutions will be acquired.
1) Suppose at the initial moment t = 0 a crack occurs
at the coordinate origin and begins to
locity V in both directions along the x-axis. The edges
of the crack are subjected to normal point force Pt5/x5,
moving at a constant velocity β along the positive direc-
tion of x-axis, where β < V; at t < 0 the half-plane was at
rest. The boundary conditions will be as follows:
 

55
,0,,
y
,0 , 0,
x
tPtxxtxVt  
vx tx Vt (13)
In this case the displacement will distinctly b
geneous functions, in which L = 1. Using τ = x/t and the
th
e homo-
eory of generalized functions [26-28] and Equations (4)
and (6), the first of Equation (13) can be as:




55
5
Re FPtxtxt 

 
PV      (14)
In the light of Equation (7), boundary c
will be further rewritten:
onditions (14)
 
 

5
1
Re D W




 ,
Re 0,
P V
D
WV





 




(15)
Deducting from the above-mentioned formulae, the
unique solution of W′(τ) must have the modality:
 
5
W






(16)
ξ(τ) has no singularity in the domain
D1(τ)/D(τ) is purely imaginary for the s
co
of |τ| < V, while
ubsonic speeds,
nsequently ξ(τ) must be purely real in this segment.
Thus, question (15) can conduce the following problems:


Re 0, ;V   
(17)
Im 0, V
  
According to symmetry and the con
nite point of the plane correspondin
ordinates
ditions of the infi-
g to the origin of co-
of the physical plane as well as singularities of
the stress of the crack tip [29,30], the sole solution of the
Keldysh-Sedov problem (17) under the given conditions
must have the following modality:


12
22
AV  

 (18)
where A is an unknown constant.
Inserting Equation (18) into (16), (7), one can gain:
 

12
5
WA V 
2 2




 (19)








1
52
AD D
F
V2









Then putting Equation (20) into (14),
A can be determined from that
(20)
at τ→β, constant
 


22
1
ImAPVD D   

(21)
Putting Equation (20) into (6) and (4), at the surface y
= 0, the stress σy, the displacement v and the s
sity factor
tress inten-
K1(t) are acquired, respectively:

 
5222
1
Im ,
y
DA
x
Vt
DxVt







 (22)
 


111 2
1
Im DV
A
Kt tD
VV 


 



V

Replacing Equation (19) into (4), (6), after
with respective to τ one can obtain the displace
(23)
integrating
ment v:

432234
11 11
Re xt
A
v

54 22
11 1
d
V

 


 





 

 


(24)
Utilizing correlative integral formulas [31] to yield:
22
22
dl
n
V
V
11VV





 (2
 5)
22
2
222
1dV
V
V







 (26)
22 22
V
22 3
322
11
dln
22
VV
VV
V







 


(27)
422
23 4
22
2
d33
VV
VV
V
22





 



(28)
Open Access MME

Y. T. WANG ET AL. 196
22
5
522
22 22
24 42
13
dln
8
13
48
VV
V
V
VV
VV











(29)



22
2222
22
1d
1ln
V
VVV
V
V

 
2





 




(30)
The crack extends along the x-axis, therefore W(τ) can
be worked out in the operation of the definite integral, we
take constant C = 0. Then putting Equations (25)-(30)
into (24), the displacement v is given as follows:
 

22 2
4424
11 3
ln
28
AVtVtx
vVx
VV






22 2222
42 2
23
22 22232
22 2
ln
12 3
233 48
,
VtxVVtx
A
Vtx
V
Att tt
x VxVxVx
Vt xxVt
x






  








(31)
By means of the solution of Equation (31), the bridg-
ing fiber fracture speed α can be readily attained as:


22
4424
11 3
ln
28
AVV
VVV








22
222
42 2
22 22232
22
ln
11 123
233 48
,
v
VVV
A
V
V
A
VVV
Vxt
  



  


  









(32)
Each fibre has same strength [15-17,20-22] accord-
ing to assumption, hence the bridging fiber fracture
strength must be equal. Where Δ can be ascertaine
an
in the format of explicit function.
will
be
d by
axial tensile test of bridging fibers of composite
materials with V and ß regarded as known constants,
respectively.
In terms of this approach, the bridging fiber fracture
speed α can be only gained numerical solution, because it
can not be shown
2) With all conditions remaining the same as those in
the above sample, the applied loads become variable
loads Px5/t4. The boundary conditions of the problem
as follows:



54
,0, ,
,0,
y
0,
x
tPxtxtxVt
vxt

x Vt

 
(33)

In this case the stresses are apparently homo
functions, in which L = 1, making use of the t
generalized functions [26-28] and Equations (5) and (6),
th
geneous
heory of
e first of the boundary conditions (33) can be rewritten
in the following modality:





55
5
Re 4
4
F
Px ttxt
PV



 
 


Owing to the derivative of Dirac’s funct
zero at x ≠ βt, the above expression will be d
In terms of Equation (7), boundary conditions (33) will
be
(34)
ion equaling
educted.
further rewritten:
 
 
5
1
Re4 ,
D
WP V
D






Re 0,WV


 


 


(35)
Known from the above, the sole solution of W′(τ) is:



 
5
W

 


 (36)
D1(τ)/D(τ) is purely imaginary for the s
ξ(τ) must be purely real in this area. T
w
ξ(τ) has no singularity in the domain of |τ| < V, while
ubsonic speeds, so
hus, question (36)
ill be the following boundary value problems:



Re 0,;
Im 0,
V
V
 
 



 (37)
In the light of the symmetrical con
larities of the stress as well as the
plane corresponding to the coordinate origin of the phy-
si
ditions and singu-
infinite point of the
cal plane, the sole solution of Keldysh-Sedov problem
(37) takes the format:


32
22
AV  

 (38)
where A is an unknown constant.
Putting Equation (38) into (37), (7), one can attain:
 

32
52
WA V 
2






(39)








5
1
32
22
ADD
F
V





Putting Equation (40) into (35), we have the result:
(40)
Open Access MME

Y. T. WANG ET AL. 197




5
32
22
1
5
4P 

Re
Re ,
DA
DV
iV

 














(41)
At τ→β, constant A can be determined from that:

 
32
22
1
4
Im
PV
ADD




 

(42)
nge
of elastic wave can be shown by the circular area of ra-
dius c1t and c2t. Here c1 and c2 are the
gitudinal and transverse waves (c1 > c2) o
respect
In an orthotropic isotropic body, the disturbance ra
velocities of lon-
f elastic body,
ively. In an orthotropic anisotropic body, the dis-
turbance range of elastic wave is not the circular area and
can not exceed threshold value

12
d11
CC

 of elas-
tic body, where C11 is an elastic constant of materials. At
d
x
Ct, with



1
Im 0DD   

, thus displace-
ments and stresses are zero with the initiate cases; and
lastic wave ca
Then iEquation (40) into (
this shows that disturbance of en not ex-
ceed Cdt.
nserting 6) and (5), at the
surface y = 0, the stress σy and dynamic stress intensity
factor K1(t) are gained, respectively:
 


4
1
32
22
Re d ,
y
Im
xt DD
A
xVt
V



  

 (43)
  


 


52 DV
AV 
1
ImKt tVDV






(44)
The limit of Equation (44) belongs to the form 0·∞,
which should be only changed into the type of ∞/∞, then
its result can be worked out by the approach
tal theorem [31,32]. Dynamic stress intensity facto1
slowly a
of L’Hospi-
r K (t)
ugments from zero and even reaches or exceeds
fracture toughness of this material, because unique vari-
able t lies in the numerator, and the rest are also referred
to as real constants.
Equation (39) can rewrite as follows
 


5
32
22
A
W
V



5
43
2234

 

 



4
132
22
22
22
22
d
3arcsin
22
A
W
V
AAVAV
VV
V










(46)
 

3
22 32
22
2
dd
A
WW
V

22
22
AV
AV
V

 





(47)

   

 

2
2
33 32
22
2
22
dd
arcsin
A
WW
V
AV
V


 



 







(48)


3
3
432 22
22
d
AA
W
V
V







 (49)


4
4
532 222
22
d
AA
W
VV
V







 (50)
Denominator in Equation (45) contains this term


32
22
V  , calculation will not be preformed in
the light of integral formulae, therefore integral format
must be translated into integral which can be fu
For the sake of convenience, we assume:
lfilled.
22
XV



By variable replacement: 1

 , th
be rewritten as follows:
2
is term X can
222 2
11
2XV V



  (51)
[31] is:
Known from it, the following relationship in literature
22
1
aV


, 12b


, 1c ,
22
11
44DacbV.
Integrating the sixth term of Equation (45) in terms of
relevant formulae in literature [31], we will gain W(τ):
6
 
55
1
632 32
d d A
W
 
 

1
5
11
2
5
11 1
11
11
dd 1
22
ln 2
A
XX
b A
b b
A
C
aa


 


32
22
A
V
 



(45)
Integrating Equation (45), one will attain W(τ). But it
has six items, separate denotation is more convenient,
Integral formulas are used in literature [31], now assume:
1

32
112
aX
XX
Db


1
1
aDX DX
Xa b










 











(52)
Known from Equation (45):








123456
WWWWWWW

   .
Open Access MME

Y. T. WANG ET AL. 198
The crack runs along x-axis, hence W(τ) comprising
Equations (46)-(50) and (52) can be performed in the
definite integral operation, one takes constant C = 0.
Known from Equation (45):
 


123456
WWWWWWW

 
The crack runs along the x-axis, so W(τ) co

.
Equations (46)-(50) and (53) can be performed i
definite integral operation, one takes constant C = 0.
Making use of relative integral formulas [31] to yield:
mprising
n the
22
1darcsin V
V





 (53)
22
V22
22
dln
VV
VV





 (54)


22 22
2darcsin
VV
V





 
 (55)
Pug Equationtttin (46) ino (6), (5), the divisional dis-
placement v1 will be obtained as:
2
22
122
12
Re
2
xt
Ax V
vV 

2
V
V
2
3arcsin d
V





 (56)




Now putting Equations (54), (26) into (56), there re-
sults the divisional displacement v1:


 


2
222
1
3arcsin ,
22
AxAV tx
vVtx x
tV
Vt
t
(57)
Inserting Equations (47) into (6), (5), by m
Eq
eans of
uations (55), (26), the displacement v2 are obtained:
22 2
22arcsinvA Vtxx




,
xxVt
Vt



(58)
Replacing Equations (48) into (6), (5), by applica-
tion of Equation (25), there results the sub-di
ment v3:
splace-
2
3arcsin ,vAt xVt
Vt
  (59)
Substituting Equation (49) into (6
x
), (5), by means of
Equation (26) there results the sub-displacement v4 as:
22
3
42
Re
3
22 2
2,
x
t
AVtxxVt
V



)
V
vAx V





  


(60
Then putting Equation (50) into (6), (5), by means of
Equation (25), there results the sub-displacement v5:
4222
53ln ,
Ax VtVtx
vx
x V
 
 Vt (61)
Now substituting Equation (52) into Eq
there results the divisional displacement v
uations (6), (5),
6 as:
2 5
11
62
1
2 1
Re xt Db b
Ax
vaDX
 

111
11
2 1ln2
Xa bb
aa D

d
X


  











(62)
Integral of the second term of Equation (62) without
comprising coefficient can be written as:
11
2
1ln d
Xa b
11
11
11
1
1
2
11
ln d
ln 2
d 11d1 1
aa
Xa b
aa
XX
11
11
2
1
aa
Xa b




 

(63)






 
 





  


Using integral formulas in Literature [31], one gains:
1
11
1
11
d1ln 2
Xa b
1
X
aa



 
 (64)

where: 22
1
aV


, 12b


,
.
1c ,
22
44cb
11
DaV
Putting Equations (64), (25) into (63), the following
representation is given as:
11
2
11
11
11
22
1lnd
2
11 1
ln 2
1ln
Xa b
aa
Xa b
aa
VV

V


 






 
 




(65)
Inserting Equations (65), (26) into (62), the divisional
displacement v7 will be obtained as follows:

54222
22 2
623
n
A t
v xx 
1
11
11
l
2
AxVtVx
Vt
Va V
xVt
xt
aa



 
22 2
5
32 ln Vt xt ab
Ax
t





 


(66)
The displacement v is the sum of divisional displace-
ment: 123456 vv vvvvv


ons (57)-(61) and (66), th
. The addition of
Equatie displacement is
acquired as:
v
Open Access MME

Y. T. WANG ET AL. 199
222
5
11
32
11
2
2
3
22 2
1
ln 2
3arcsin
2
2,
2
Vtxt ab
Ax
vt xt
aa
Vt x
AxtVt
x
A
Vtxx Vt
at








 
 






 


(67)
where: 22
1
aV

, 12b

 , 1c ,
22
11
44Dacb V.
Using the similar ways as that for finding Equation
(33), put |x| = αt into (67) while regarding V, β and t as
known constants, respectively. Bridging fiber fracture
speed α can be only gained numerical solution, because it
can also not be shown in the form of explicit function.
6. Law of Dynamic Stress Intensity Facto
In
e law of dynamic stress inte
s (23), (44) to plot K1(t) as a function of
time t, and their numerical solutions are facilely gained.
The following constants are as [8,17,21-22,33-36]:
C11 = 19.24 GPa; C12 = 1.25 GPa; C11 = 17.83 GPa; P
= 200 N; C66 = 1.00 GPa; V = 300 m/s; β = 200 m/s; ρ =
4.9 × 1000 N/m3.
Known from Equation (23), dynamic stress int
factor K1(t) reduces tardily and has obvious singularity
on account of unique variable t in the denominator, and
the rest units are regarded as real constants. Such a tend
n Figure This variable current is similar to
e result of Literatu,17,21,33-38]. It is known
fr
.
7,
r
the light of practical situations of concrete problems,
mutativ nsity factor should be
interpreted better. The corresponding parameters are put
into Equation
ensity
is shown i3.
th,22res [8
om Equation (44) that dynamic stress intensity factor
K1(t) increases slowly from zero and even reaches or
overruns fracture toughness of this material, because sole
variable t lies in its numerator, while the rest quantities
are also referred to as real constants. This result must
conduce the structural instability, as shown in Figure 4
This trend is similar to the result in references [8,1
21,22,33-36,38-40], consequently it is also right.
The relative numerical values between dynamic stress
intensity factor K1(t) and time t are expressed in Tab l e s 1,
2 in terms of curves in Figures 3, 4, respectively.
7. Conclusions
By means of correlative expression:
 
,, ,
n
f
xyttfxt yt, where n is an integral number,
the problem considered can be facilely changed into ho-
mogeneous function of x and t of zeroth dimension, i.e.
self-similar functions. All suffice the relationship of this
function, and thus the analytical solutions can be gained
by Equations (4)-(7) with homogeneous function of
0 2 46 810 1214 16 18 20
0
0.2
0.4
0.6
0.8
1
1.2
1.4 x 10-11
t/ms
Stress intensity fa ctor K
1
(t )/(N/m
3/2
)
Figure 3. Dynamic stress intensity factor K1(t) versus time t.
0 2 46 810 12 14 16 1820
0
0.5
1
1.5
2
2.5
3
3.5 x 10
14
factor K
1
(t)/(N/m
3/2
)
t/ms
S t res s i nt ensi t y
Figure 4. Dynamic stress intensity factor K1(t) versus time t.
Table 1. Relative numerical values between K1(t) versus t.
t/ms 4 8 12 16 20
K1(t) 2.9552 2.0896 1.7062 1.4776 1.3216
Table 2. Relative numerical values between K1(t) versus t.
t/ms 4 8 12 16 20
K1(t) 1.5511 2.1936 2.6866 3.1023 3.4684
variable τ. This measure can use not only in electrody-
namics [15-20,22,33-34], but also in electrostatics [24,30]
and even in other situations, referring to literatures [30,
41].
Analytical solutions of the symmetrical dynamic crack
extension model for bridging pull-out of unidirectional
composite materials were found by way of the theoretical
usage of a complex variable function. The method de-
veloped in this paper based on the approaches of the self-
similar functions makes it conceivable to obtain the con-
Open Access MME

Y. T. WANG ET AL. 200
crete solution of this model and bridging fiber fracture
speed α. According to the concrete boundary conditions,
self-similar function W(τ) can be easily deducted by
means of corresponding to variable τ. Consequently,
analytical solutions of stresses, displacements and dy-
namic stress intensity factors will be readily worked out.
This is referred to as the analogous class of dynamic
problem of the elasticity theory. However, the present
solution occurs to be the simplest and intuitive of all al-
ternative approaches appearing by so far. Indeed, we
have succeeded in a mixed Keldysh-Sedov boundary va-
lue problem on a half-plane. The query is of adequate
actual interest, since all the members of structures in
which fractures may move are of finite dimensions
are frequently in the modality of long strips. The ways
solution are based exclusively on techniques of analyt
l
m
thent ohe comtativerk neo rlve
such a crack expansion query.
. Acknowledgements (Heading 5)
Pro
neecentref hydraic andpmeor min of
Liaong profT).
posites,” Acta Metallurgical, Vol. 33, No. 11, 1985
and
of
i-
cal-function theory and are simple and compendious. By
making some observations regarding the soution of the
ixed boundary value problem we have rather decreased
amouf tpu woeded teso
8
ject is supported by the open foundation of the engi-
ring oul equint fing
inovince China (No. CMH-201207
REFERENCES
[1] D. B. Marshall, B .N. Cox and A .G. Evens, “The Me-
chanics of Matrix Cracking in Brittle-Matrix Fiber Com-
, pp.
2013-2021.
http://dx.doi.org/10.1016/0001-6160(85)90124-5
[2] B. Budiansky, J. W. Hutchinson and A. G. Evens, “Ma-
trix Fracture in Fiber-Reinforced Ceramics,” Journal of
the Mechanics and Physics of Solids. Vol. 34, No. 2, 1986,
pp. 167-189.
http://dx.doi.org/10.1016/0022-5096(86)90035-9
[3] M. Ji and H. Ishikawa, “Analysis of an Internal Central
Crack with Bridging Fibers in a Finite Orthotropic Plate,”
International Journal of Engineering Science, Vol. 35,
No. 4, 1997, pp. 549-560.
http://dx.doi.org/10.1016/S0020-7225(96)00099-7
[4] D. B. Marshall and B. N. Cox. “Tensile Fracture of Brittle
Matrix Composites: Influence of Fiber Strength,” Acta
Metallurgical, Vol. 35, No. 11, 1987, pp. 2607-2619.
http://dx.doi.org/10.1016/0001-6160(87)90260-4
[5] Z.-M. Wang, “Mechanics and Structural Mechanics of
Composite Materials,” Publisher of Machinery Industry,
Beijing, 1991.
[6] G.-L. Shen. “Mechanics of Composite Materials,” Tsing-
hua University Press, Beijing, 1996.
[7] C. W. Woo and Y. H. Wang, “Analysis of an Internal
Crack in a Fine Anisotropic Plate,” International Journal
of Fracture, Vol. 62, No. 2, 1993, pp. 203-208.
[8] J. C. Lee, “Analysis of Fiber Bridged Crack near a Free
Surface in Ceramic Matrix Composites,” Engineering
Fracture Mechanics, Vol. 37, No. 2, 1990, pp. 209-219.
http://dx.doi.org/10.1016/0013-7944(90)90344-G
[9] W. T. Tsai and I. R. Dharani, “Non Self-Similar Fiber
Fracture in Unidirectional Composites,” Engineering
p. 43-49.
80-C
Fracture Mechanics, Vol. 44, No. 1, 1993, p
http://dx.doi.org/10.1016/0013-7944(93)900
1994,
pp. 31-35.
[11] K. Liao and K.th Model for
[10] W. N. Liu, “Stress Ahead of the Tip of a Finite-Width
Center-Crack in Fiber-Reinforced Composite Specimens:
Subjected to Non-Linearly Distributed Bridging Stresses,”
International Journal of Fracture, Vol. 70, No. 1,
Reifsnider, “A Tensile Streng
Unidirectional Fiber-Reinforced Brittle Matrix Compos-
ite,” International Journal of Fracture, Vol. 106, No. 1,
2000, pp. 95-115.
http://dx.doi.org/10.1023/A:1007645817753
[12] V. Tamuzs, S. Tarasovs and U. Vilks, “Progressive De-
lamination and Fibre Bridging Modeling in Double Can-
tilever Beam Composite Specimens,” Engineering Frac-
ture Mechanics, Vol. 68, No. 5, 2001, pp. 513-525.
http://dx.doi.org/10.1016/S0013-7944(00)00131-4
[13] A. Piva and E. Viola, “Crack Propagation in an Ortho-
tropic Media”, Engineering Fracture Mechanics, Vol. 29,
No. 5, 1988, pp. 535-547.
http://dx.doi.org/10.1016/0013-7944(88)90179-8
[14] J. De and B. Patra, “Elastod
Orthotrpic Medium through Complex Variable Appr
ynimic Crack Problems in An
oach,”
k,”
Engineering Fracture Mechanics, Vol. 41, No. 5, 1998,
pp. 895-909.
[15] K. B. Broberg, “The Propagation of a Brittle Crac
Arkve Fysik, Vol. 18, No. 2, 1960, pp. 159-192.
[16] Y. W. Craggs, “The Growth of a Disk-Shaped Crack,”
International Journal of Engineering Science, Vol. 4, No.
2, 1966, pp. 113-124.
http://dx.doi.org/10.1016/0020-7225(66)90019-X
[17] J. G. Goree and R. S. Gross, “Analysis of a Unidirectional
Composite Containing Broken Fibers and Matrix Dam-
age,” Engineering Fracture Mechanics, Vol. 33, No. 3,
1979, pp. 555-578.
[18] G. P. Cherepanov and E. F. Afanasov, “Some Dynamic
Problems of the Theory of Elasticity—A Review,” Inter-
national Journal of Engineering Science, Vol. 12, No. 8,
1974, pp. 665-690.
http://dx.doi.org/10.1016/0020-7225(74)90043-3
[19] G. P. Charepanov, “Mechanics of Brittle Fracture,” Nau-
ka, Moscow, 1973.
[20] C. Atkinson, “The Propagation of a Brittle Crack in Anis-
tropic Material,” International Journal of Engineering
Science, Vol. 3, No. 1, 1965, pp. 77-91.
http://dx.doi.org/10.1016/0020-7225(65)90021-2
[21] N.-C. Lü, X.-G. Li, Y.-H. Cheng and J. Cheng, “Fracture
Dynamics Problem on Mode I Semi-Infinite Crack,” Ar-
chive of Applied Mechanics, Vol. 81, No. 9, 2011, pp.
1181-1193. http://dx.doi.org/10.1007/s00419-010-0476-x
[22] N. C. Lü, Y. H. Cheng. X. G. Li and J. Cheng, “Dynamic
Propagation Problem of Mode I Semi-Infinite Crack
Open Access MME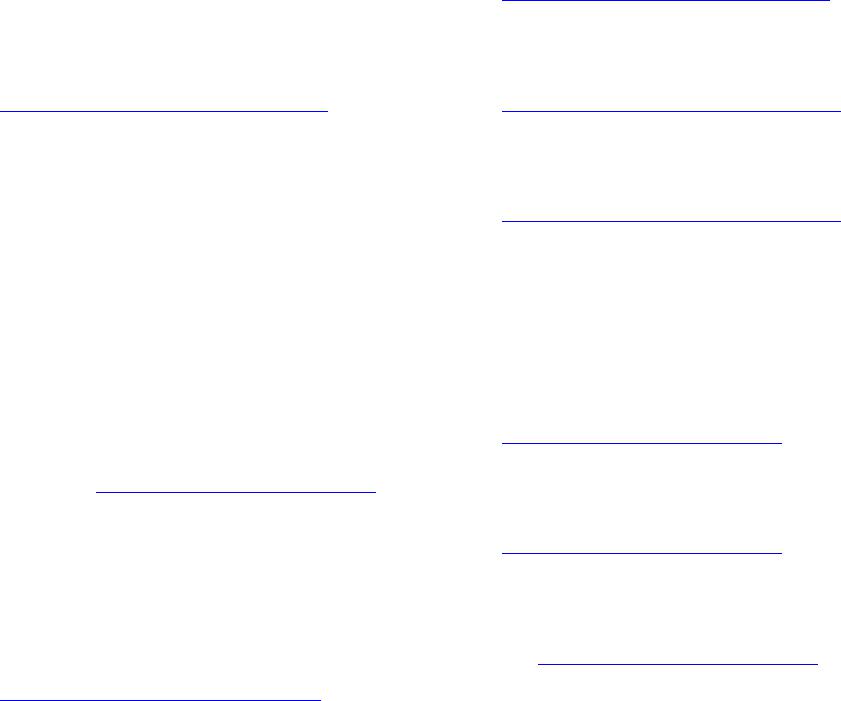

Y. T. WANG ET AL.
Open Access MME
201
racture of
a
Subjected to Superimpose Loads,” Fatigue & F
Engineering Materials & Structures. Vol. 33, No. 3, 2010,
pp. 141-148.
[23] N. C. Lü, Y. H. Cheng. X. G. Li and J. Cheng, “An
Asymmetrical Dynamic Model for Bridging Fiber Pull-
Out of Unidirectional Composite Materials,” Meccnica,
Vol. 47, No. 5, 2012, pp. 1247-1260.
http://dx.doi.org/10.1007/s11012-011-9509-y
[24] N. I. Muskhlishvili, “Singul
Moscow, 1968.
ar Integral Equations,” N
, “Boundary-Value Problems,” Fitzmatigiz,
orwood
auka,
[25] N. I. Muskhlishvili, “Some Fundamental Problems in the
Mathematical Theory of Elasticity,” Nauka, Moscow,
1966.
[26] F. D. Gakhov
Moscow, 1963.
[27] R. F. Hoskins, “Generalized Functions,” Ellis H,
Chichester, 1979.
[28] X. S. Wang, “Singular Functions and Their Applications
in Mechanics,” Scientific Press, Beijing, 1993.
[29] G. C. Sih, “Mechanics of Fracture 4. Elastodynamics
Crack Problems,” Noordhoff, Leyden, 1977.
[30] R. P. Kanwal and D. L. Sharma, “Singularity Methods for
Eastostatics,” Journal of Elasticity, Vol. 6, No. 4, 1976,
pp. 405-418. http://dx.doi.org/10.1007/BF00040900
[31] Editorial Group of Mathematics Handbook, “Mathemati-
cal Handbook,” Advanced Education Press, Beijing, 2002.
[32] Teaching Office of
“Advanced Mathematics,” Advanced Education P
Mathematics of Tongji University
Crack Growth in Anisotropic Mate-
.
ress,
Beijing, 1994.
[33] K. C. Wu, “Dynamic
rial,” International Journal of Fracture, Vol. 106, No. 1,
2000, pp. 1-12.
http://dx.doi.org/10.1023/A:1007621500585
[34] X.-G. Li, Y.-H. Cheng, N.-C. Lv, G.-D. Hao and J. Cheng
cal Science and Technology, Vol. 25,
,
“A Dynamic Asymmetrical Crack Model of Bridging Fi-
ber Pull-Out in Unidirectional Composite Materials,”
Journal of Mechani
No. 9, 2011, pp. 2297-2309.
http://dx.doi.org/10.1007/s12206-011-0526-5
[35] N. C. Lv, Y. H. Cheng. H. L. Si and J. Cheng, “Dynamics
of Asymmetrical Crack Propagation in Composite Mate-
rials,” Theoretical and Applied Fracture Mechanics, Vol.
47, No. 3, 2007, pp. 260-273.
http://dx.doi.org/10.1016/j.tafmec.2007.01.004
[36] N. C. Lü, Y. H. Cheng and J. Cheng, “Mode I Crack Tips
Propagating at Different Speeds under Differential Sur-
face Tractions,” Theoretical and Applied Fracture Me-
chanics, Vol. 46, No. 3, 2006, pp. 262-275.
http://dx.doi.org/10.1016/j.tafmec.2006.09.004
[37] A. S. Kobayashi, “Dynamic Fracture Analysis by Dy-
n Society of Mechanical Engineers,
963460
namic Finite Element Method. Generation and Prediction
Analyses,” In: Nonlinear and Dynarnic Fracture Me-
chanics, America
New York, 1979, pp. 19-36.
[38] K. Ravi-Chandar and W. G. Knauss, “An Experimental
Investigation into Dynamic Fracture: Part 1, Crack Initia-
tion and Arrest,” International Journal of Fracture, Vol.
25, No. 41, 1984, pp. 247-262.
http://dx.doi.org/10.1007/BF00
erimental
52313
[39] K. Ravi-Chandar and W. G. Knauss, “An Exp
Investigation into Dynamic Fracture: Part 2, Microstruc-
tural Aspects,” International Journal of Fracture, Vol. 26,
No. 11, 1984, pp. 65-80.
http://dx.doi.org/10.1007/BF011
erimental [40] K. Ravi-Chandar and W. G. Knauss, “An Exp
Investigation into Dynamic Fracture: Part 3, on Steady-
State Crack Propagation and Crack Branching,” Interna-
tional Journal of Fracture, Vol. 26, No. 2, 1984, pp. 141-
152. http://dx.doi.org/10.1007/BF01157550
[41] L. A. Galin, “Contact Problems in Elasticity Theory,”
GITTL, Moscow, 1953.