J
ournal o
f
A
pp
Published Onli
n
http://dx.doi.or
g
Open Access
ABSTRA
C
In the analys
i
internal force
s
loss of precis
i
p
resent accur
a
variational pr
i
b
est stress fi
e
with drilling
D
two displace
m
Keywords: F
i
1. Introdu
c
For traditio
n
from the prin
c
functions are
displacement
s
b
e derived b
y
of formulatio
n
curate displa
c
p
rocedures o
f
most situatio
n
tant than the
high-rise bui
l
are depende
d
stresses. For
t
the accuracy
p
lacement re
s
overcoming
t
formulations
Taylor [2].
On the oth
e
veloped from
Washisu, suc
h
Pian and Su
m
ment-
b
ased e
l
internal force
first. Without
p
lied Mathemat
i
n
e November 2
0
g
/10.4236/jamp
.
Hy
D
i
2
Department
o
C
T
i
s of high-rise
s
of the shear
w
i
on induced b
y
a
cy enough fo
r
i
nciple is use
d
e
ld, three diff
e
D
OF. Numeri
c
m
en
t
-
b
ased pla
n
i
nite Element;
c
tion
n
al displacem
c
iple of mini
m
usually assu
m
s
, and then the
y
stress-strain
n
, these kind
s
c
ement result
s
f
these formul
n
s, the stress
r
displacemen
t
l
dings. The r
e
d
on the int
e
t
he sake of d
i
of stresses i
s
s
ults. Many
e
t
his shortage,
developed b
y
e
r hand, the h
y
the modifie
d
h
as the hybrid
m
ihara [4]. It i
l
ements. To
f
field or stres
the differenti
a
i
cs and Ph
y
sics
,
0
13 (http://ww
w
.2013.16004
brid P
o
i
splace
m
Xiaoming
C
1
China S
t
o
f Engineering
M
E
building, tra
d
w
alls by stres
s
y
using differe
n
r
design. In th
i
d
for improvin
g
e
rent forms ar
c
al results sho
w
n
e elements c
a
Displacemen
t
m
en
t
-
b
ased el
e
m
um potential
m
ed as a poly
n
internal force
s
relationship.
B
s
of elements
s
, otherwise t
h
ations are als
o
r
esults are mu
c
t
s such as t
h
e
inforcements
e
rnal forces
i
i
fferential wit
h
s
usually low
e
e
fforts have
b
such as the
y
MacNeal [
y
brid/mixed
e
d
variational
p
method prop
o
s different fr
o
f
ormulate thes
s field shoul
d
a
l process whi
c
,
2013, 1, 15-1
9
w
.scirp.org/jour
n
o
st-Pro
c
m
ent-B
a
C
hen
1
, Song
C
t
ate Constructio
n
M
echanics, Sch
o
E
mail: chenxia
o
Recei
v
d
itional displa
c
s
integration.
L
n
tial method t
o
i
s paper, the h
y
g
the stress p
r
e assumed fo
r
w
that by usi
n
a
n be improve
d
t
-Based Plane
e
ments deriv
e
e
nergy, the tr
i
n
omial of no
d
s
or stresses c
a
B
y this proce
can present a
h
e constructi
o
o
easier. But
i
c
h more imp
o
h
e designing
o
of shear wa
l
i
ntegrated fro
m
h
displaceme
n
e
r than the d
i
b
een made
fo
assumed stra
i
1], Piltner a
n
e
lements are d
p
rinciple of H
u
o
sed b
y
Pian [
3
om
the displac
e elements, t
h
d
be assumed
c
h is needed
fo
9
n
al
/
jamp )
c
essing
a
sed P
l
C
en
2
, Jiany
u
n
Technical Ce
n
o
ol of Aerospa
c
o
h
v
e
d
August 201
3
c
emen
t
-
b
ased
L
imited by th
e
o
derive strai
n
y
brid pos
t
-
p
ro
c
r
ecision of tw
o
r
the displace
m
n
g the propose
d
d
.
Element; Hyb
e
d
i
al
d
al
a
n
ss
c-
o
n
in
or
-
o
f
l
ls
m
n
t,
i
s-
fo
r
in
n
d
e-
u
-
3
],
e-
h
e
at
fo
r
the di
s
they c
a
sent o
b
tion pr
o
tion of
dable,
f
usuall
y
eleme
n
By
c
displac
b
rid p
o
metho
d
place
m
interna
l
Equati
o
functio
n
mined
p
b
e sol
v
calcula
t
ing th
e
paper,
t
ments.
2. H
yb
For th
e
tional
c
Proce
d
l
ane El
e
u
n Sun
1
, Yu
n
n
ter, Beijing, C
h
c
e, Tsinghua U
n
h
ua.org.cn
3
plane elemen
t
e
singular pro
b
n
s, the displac
e
c
essing proce
d
o
quadrilateral
m
en
t
-
b
ased pl
d
method, the
rid Pos
t
-Proc
e
s
placemen
t
-
b
a
s
a
n exhibit bet
t
b
vious disadv
a
o
cedures are
m
matrix invers
i
f
urthermore, t
h
y
are not as
a
n
ts.
c
ombining the
emen
t
-
b
ased
e
o
st procedure
d
, the nodal
d
m
en
t
-
b
ased pla
t
l
force field
w
o
n is assumed
n
al, by the
p
p
arameters of
v
ed out, and fi
n
t
ed easily. It i
e
internal forc
e
t
his method i
s
b
rid Post-
P
e
hybrid plate
c
an be written
d
ure fo
r
e
ments
n
gui Li
1
h
ina
n
iversity, Beijin
g
t
s are often u
s
b
lem produce
d
e
men
t
-
b
ased e
l
d
ure based on
plane eleme
n
ane elements
accuracy of s
t
e
ssing Proced
u
s
ed elements
t
er stress resu
l
a
ntages. For
e
m
uch more co
m
i
on and conde
n
h
e accuracy
o
a
ccurate as t
h
merits of bot
h
e
lements, a n
e
was propose
d
d
isplacements
t
e elements at
w
hich should
s
to substitute
i
p
rinciple of st
the assu
m
ed
i
n
ally the new
s seemed ver
y
e
accuracy of
s
extended to
i
P
rocessin
g
P
elements, the
as follows [5,
6
r
g
, China
s
ed to get the
d
by wall hole
s
l
ements cann
o
the Hellinge
r
-
n
ts. In order to
AQ4 
and
t
ress solutions
u
re
to derive st
r
l
ts. But they
a
e
xample, the
c
m
plex, and the
n
se are usuall
y
o
f displaceme
n
h
e displacem
e
h
hybrid ele
m
e
w method na
d
by Cen [5]
are derived f
r
first, after thi
s
atisfy the eq
u
i
nto the hybri
d
ationary, the
i
nternal force
f
internal forc
e
y
effective for
plate elemen
t
i
mprove the
pl
P
rocedure
discrete ener
g
6
]:
JAMP
in-plane
s
and the
o
t always
Reissner
find the
AQ4 
of these
r
ains, so
a
lso pre-
c
onstruc-
calcula-
y
unavoi-
n
t results
e
n
t
-based
m
ents and
med hy-
. In this
r
om dis-
i
s, a new
u
ilibrium
d
energy
undete
r
-
f
ield can
e
s can be
improv-
t
. In this
l
ane ele-
g
y func-

X. M. CHEN ET AL.
Open Access JAMP
16
1
1
1{}[ ]{}
2
1{}[ ]{}
2
{}{} {}{}
()
e
e
eee
eT
Rb
A
T
s
A
TT
z
AAA
nxnxyny
s
dA
dA
dAdAf wdA
Tw MMds






 


 

MD M
TD T
MκTγ
(1)
where Wexp is the work of external forces:
exp ()
eznxnxyny
As
WfwdATwMMds  
  (2)
Assume the new internal force field as follows:
,,
,,
{}[ ]{}
{}[ ]{}
MM
xxxxyy
TM
yxyxyy
TMM
TMM


 
 
 
 

 
 
MPα
TPα (3)
where

11 121112
21 222122
21 222122
11 121112
1211 12
{}[ ]
100000000
[] 000010000
000 0000 01
00000
[] 00 000
0
0
{}
T
xyxy
M
T
T
M
MMM
jjj j
jjj j
jjj j
jjjj








 














M
P
P
α
(4)
and 11
j, 12 j, 21 j, 22 j are components of the inver-
sion matrix of Jacobian .
After substituting Equation (3) into Equation (1), the
new form of energy functional can be written as:
1
1
exp
[][][]
1{} {}
2[][][]
{} ([][]
[][]) {}
e
e
e
T
MbM
A eT
R
MM
T
TsT
A
TT
MMb
A
Te
Ts
dA
dA
dA W




 







PDP
αα
PD P
αPB
PB q
(5)
By the principle of stationary:
0
{}
e
R
M
 
α (6)
the parameters of the assumed internal force field can be
written as:
1
{} [][]{}
e
MMMMq

αKKq (7)
where
1
1
[] ([][][]
[][][])
[]([] [][][])
e
e
T
MMMb M
A
T
TsT
TT
MqM bT s
A
dA
dA







KPDP
PD P
KPBPB
(8)
Substitute Equation (7) into Equation (3), the new in-
ternal force can be obtained.
Compared with plate elements, it is much easier to ex-
tend this method to plane elements. The according hybrid
discrete energy functional of plane elements can be writ-
ten as [6]:
1
1
{}{}{}[] {}V
2
e
eT T
RVDd



 




 σuσDσ (9)
Similar to Equation (4), a new form of stress field of
displacement-based plane element can be assumed as:
{} []{}



σPα (10)
Substitute Equation (10) into Equation (9), the para-
meters i

in []

P can be obtained by using the prin-
ciple of stationary. The according matrix named []


K
and []
q 
K for plane elements are as follows:
1
[] [][][]
[] [][]
e
e
T
A
T
qb
A
tdA
tdA
 






KPDP
KPB
(11)
With different matrix of []

P, the stress field will be
different too. This new method will be used to try to im-
prove the stress accuracy of plane element named
AQ4

and AQ4

 [7].
3. Introduction of AQ4θ and AQ4θλ
AQ4

and AQ4

 are two plane elements with drill-
ing DOF formulated using quadrilateral area coordinate
methods presented by Long et al. [8,9]. They have the
merits of high accuracy and robust against mesh distor-
tions. After having been programmed into the software
for the analysis of high-rise buildings, the results show
that better accuracy is still needed for the stress of shear
walls with holes.
The definition of DOF of these two elements is:



1234
T
T eTT T





qqqqq (12)
where


T
iiii
uv

q


1, 2,3, 4i (13)
and i

is the additional rigid rotation at element node.
The displacements of element are as follows:



 
0

uu u
(14)
where


0
u is a polynomial about i
u and i v,



u is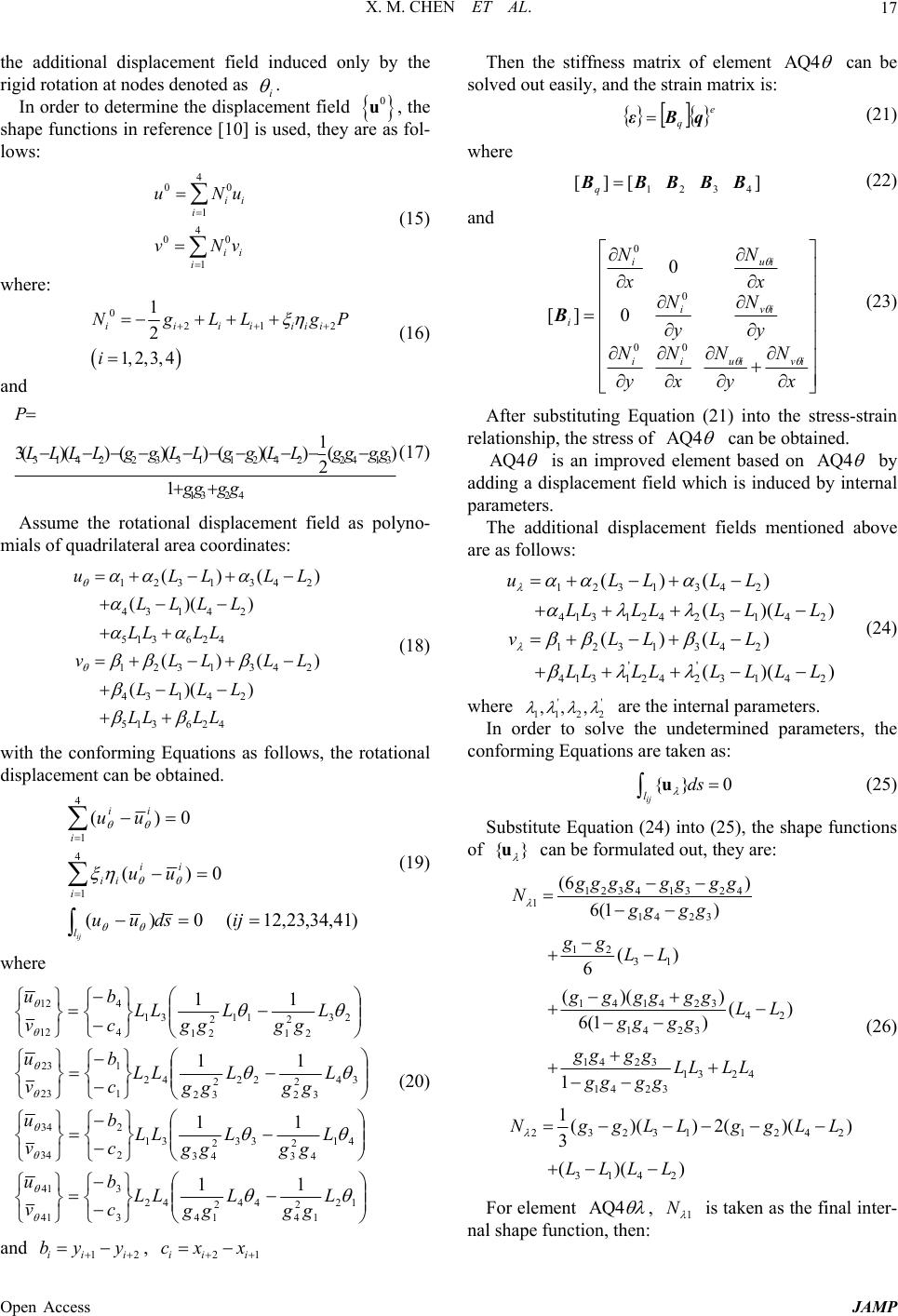

X. M. CHEN ET AL.
Open Access JAMP
17
the additional displacement field induced only by the
rigid rotation at nodes denoted as i

.
In order to determine the displacement field


0
u, the
shape functions in reference [10] is used, they are as fol-
lows:
4
00
1
4
00
1
ii
i
ii
i
uNu
vNv






(15)
where:

0
212
1
2
1, 2,3, 4
iiiiiii
NgLLgP
i


 

(16)
and
314 2233112422413
132 4
1
3( )()()()()()()
2
1
P
LLLLg gLLggLLgg gg
gg gg

 

(17)
Assume the rotational displacement field as polyno-
mials of quadrilateral area coordinates:
1231 342
431 42
513624
1231 342
431 42
513624
()( )
()()
()( )
()()
uLLLL
LLLL
LL LL
vLLLL
LLLL
LL LL


 


 



 


 

(18)
with the conforming Equations as follows, the rotational
displacement can be obtained.
)41,34,23,12(0)(
0)(
0)(
4
1
4
1








ijsduu
uu
uu
ij
l
i
ii
ii
i
ii




(19)
where




























































































12
1
2
4
44
2
14
42
3
3
41
41
41
4
2
3
33
2
43
31
2
2
34
34
34
3
2
2
22
2
32
42
1
1
23
23
23
2
2
1
11
2
21
31
4
4
12
12
11
11
11
11












L
gg
L
gg
LL
c
b
v
u
L
gg
L
gg
LL
c
b
v
u
L
gg
L
gg
LL
c
b
v
u
L
gg
L
gg
LL
c
b
v
u
(20)
and 12ii i by y

, 21ii i cx x


Then the stiffness matrix of element AQ4

can be
solved out easily, and the strain matrix is:



 
e
qqBε (21)
where
][][4321 BBBBB 
q (22)
and



































x
N
y
N
x
N
y
N
y
N
y
N
x
N
x
N
iviuii
ivi
iui
i



00
0
0
0
0
][B (23)
After substituting Equation (21) into the stress-strain
relationship, the stress of AQ4

can be obtained.
AQ4

is an improved element based on AQ4

by
adding a displacement field which is induced by internal
parameters.
The additional displacement fields mentioned above
are as follows:
1231 342
41312 423142
1231 342
''
41312 423142
()( )
()( )
()( )
()( )
uLLLL
L
LLLLLLL
vLLLL
L
LLL LLLL


 

 



  

(24)
where ''
11 2 2
,,,

 are the internal parameters.
In order to solve the undetermined parameters, the
conforming Equations are taken as:
{} 0
ij
lds


u (25)
Substitute Equation (24) into (25), the shape functions
of {}

u can be formulated out, they are:
12341324
1
14 23
12
31
141423
42
14 23
14 23
13 24
14 23
232311242
314 2
(6 )
6(1 )
()
6
()( )
()
6(1 )
1
1()()2()()
3
()( )
gg ggggg g
Ngg gg
gg
LL
gggg ggLL
gggg
ggggLL LL
gggg
NggLLggLL
LLLL













 
 
(26)
For element AQ 4

 , 1 N

is taken as the final inter-
nal shape function, then:

X. M. CHEN ET AL.
Open Access JAMP
18



λNu

}{ (27)
where



'
11
1
1
[]
0
0
T
N
N



 



λ
N (28)
Through the condense calculation, stiffness matrix of
AQ4

 can be written as:
   
q
T
qqq
e

kkkkk 1  (29)
where
  


 
   tdA
tdA
tdA
q
T
q
T
q
T
qqq
BDBk
BDBk
BDBk








(30)
and



B is the strain matrix of additional displacement
field. The according stress fields are as follows:
 

 λBελ 

(31)
Combined Equation (21) with (31), the stress field of
AQ4

 can be obtained.
4. Hybrid Post-Processing of AQ4θ and
AQ4θλ
In the formulating of stress in elements AQ4

and
AQ4

 , the strain matrix q




B and



B are the
differential results of displacements to coordinates. The
differential calculation lowered the accurate order of
stress. To avoid the differential, Hybrid Post-processing
procedures can be used to improve the stress accuracy.
Based on this theory, three forms of stress fields are pre-
sented for these two displacement-based elements.
4.1. Stress Field I
The first form of stress field is assumed as Equation (32).
It is the stress field of a hybrid element developed by
Pian and Wu [6]:

22
131
22
13
113 35
1
1
1
aa
bb
ab ab















σ (32)
where
444
12 3
11 1
444
123
111
11 1
44 4
111
444
ii iii ii
ii i
ii iii ii
iii
axa xax
byb yby

 


 
 
 
 
 

(33)
4.2. Stress Field II
In order to raise the complete order of stress field, the
second form is assumed as a polynomial of analytical
trial function method presented by Fu and Long [11]:

1
22
9
001020 206
01020 2060
100002233
yx xy
xy xy
xyxy



 












 
σ(34)
4.3. Stress Field III
Try to make the three stress components to be indepen-
dent, the third form of stress field is assumed as follows:

1
12
1
1
1
xyxy
xyxy
xyxy



 











 
σ(35)
5. Numerical Examples
5.1. Strict Patch Test
The constant strain/stress patch test using irregular mesh
is shown in Figure 1. Let Young’s modulus E = 1000,
Poisson’s ratio  = 0.25, and thickness of the patch t = 1.
After modified by three different forms of Hybrid Post-
processing, these two elements can produce exact solu-
tions without any problem.
5.2. Cook’s Skew Beam
This example was proposed by Cook et al. [12]. As
shown in Figure 2, a skew cantilever beam subjected to
distributed shear load along its free edge. The results of

max at point A and

min at point B are listed in Tables 1
and 2.
6. Conclusion
Using the traditional method to calculate stress of dis-
placement-based elements, the accuracy will descend for
the reason of differential when strains are derived from
the displacement fields. For improving the stress accura-
cy, hybrid/mix elements are very effective. However, the
formulations of the hybrid elements are more compli-
cated than those displacement-based elements. Based on

X. M. CHEN ET AL.
Open Access JAMP
19
y
4
2
2
2
2.5
1.5
4
E
= 1500, μ = 0.25
7 3
5 6
1000
Figure 1. Patch test.
48
P=1
E
=1500, μ=1 /3
44
44 A
x
y
B
Figure 2. Cook’s skew beam.
Table 1. Stress at point A and B of Cook’s Beam of AQ4θ.
Method
σAmax σBmin
2 × 2 4 × 4 8 × 8 2 × 2 4 × 48 × 8
Field I 0.1791 0.2261 0.2338 −0.1700 −0.1929−0.2002
Field II 0.1951 0.2298 0.2348 −0.1942 −0.1933−0.2010
Field III 0.1914 0.2240 0.2319 −0.1769 −0.1938−0.2009
Source Val. 0.1917 0.2241 0.2377 −0.1877 −0.1939−0.2060
Ref. Val. 0.2362 −0.2023
Table 2. Stress at point A and B of Cook’s Beam of AQ4θλ.
Method
σAmax σBmin
2 × 2 4 × 4 8 × 8 2 × 2 4 × 48 × 8
Field I 0.1913 0.2271 0.2342 −0.1748 −0.1919 −0.2009
Field II 0.2145 0.2358 0.2364 −0.2084 −0.2032 −0.2027
Field III 0.2147 0.2358 0.2364 −0.2092 −0.2033 −0.2027
Source Val. 0.2498 0.2338 0.2358 −0.1729 −0.1896 −0.2018
Ref. Val. 0.2362 −0.2023
the Hellinger-Reissner variational principle, hybrid post-
process procedure can take advantage of the merits of
these two kinds of elements to establish the relationship
between the displacement and the stress or internal force
fields. In this paper, based on this theory, three forms of
stress fields are used to improve the stress of plane ele-
ments with drilling DOF. Through the numerical results,
for element AQ 4 θ, only the second form of stress field
is effective, but for AQ 4θ

, except for the first form of
stress, the other two forms can present better results than
the source elements. It is proved that the method of hy-
brid post-process procedure is workable.
REFERENCES
[1] R. H. MacNeal, “Derivation of Element Stiffness Matric-
es by Assumed Strain Distributions,” Nuclear Engineer-
ing and Design, Vol. 70, No. 1, 1982, pp. 3-12.
ttp://dx.doi.org/10.1016/0029-5493(82)90262-X
[2] R. Piltner and R. L. Taylor, “A Systematic Constructions
of B-Bar Functions for Linear and Nonlinear Mixed-En-
hanced Finite Elements for Plane Elasticity Problems,”
International Journal for Numerical Methods in Engi-
neering, Vol. 44, No. 5, 1997, pp. 615-639.
http://dx.doi.org/10.1002/(SICI)1097-0207(19990220)44:
5<615::AID-NME518>3.0.CO;2-U
[3] T. H. H. Pian, “Derivation of Element Stiffness Matrices
by Assumed Stress Distributions,” AIAA Journal, Vol. 2,
No. 7, 1964, pp. 1333-1336.
http://dx.doi.org/10.2514/3.2546
[4] T. H. H. Pian and K. Sumihara, “Rational Approach for
Assumed Stress Finite Elements,” International Journal
for Numerical Methods in Engineering, Vol. 20, No. 9,
1984, pp. 1685-1695.
http://dx.doi.org/10.1002/nme.1620200911
[5] S. Cen, Y. Q. Long, et al., “Application of the Quadrila-
teral Area Co-Ordinate Method: A New Element for
Mindlin-Reissner Plate,” International Journal for Nume-
rical Methods in Engineering, Vol. 66, No. 1, 2006, pp.
1-45. http://dx.doi.org/10.1002/nme.1533
[6] T. H. H. Pian and C. C. Wu, “Hybrid and Incompatible
Finite Element Methods,” Chapman & Hall/CRC, Boca
Raton, 2006.
[7] X. M. Chen, Y. Q. Long and Y. Xu, “Construction of
Quadrilateral Membrane Elements with Drilling DOF Us-
ing Area Coordinate Method,” Engineering Mechanics,
Vol. 20, No. 6, 2003, pp. 6-11.
[8] Y. Q. Long, J. X. Li, Z. F. Long and S. Cen, “Area Coor-
dinates Used in Quadrilateral Elements,” Communica-
tions in Numerical Methods in Engineering, Vol. 15, No.
8, 1999, pp. 533-545.
http://dx.doi.org/10.1002/(SICI)1099-0887(199908)15:8<
533::AID-CNM265>3.0.CO;2-D
[9] Z. F. Long, J. X. Li, S. Cen and Y. Q. Long, “Some Basic
Formulae for Area Coordinates Used in Quadrilateral
Elements,” Communications in Numerical Methods in
Engineering, Vol. 15, No. 12, 1999, pp. 841-852.
http://dx.doi.org/10.1002/(SICI)1099-0887(199912)15:12
<841::AID-CNM290>3.0.CO;2-A

X. M. CHEN ET AL.
Open Access JAMP
20
[10] Z. F.Long, X. M. Chen and Y. Q. Long, “Second-Order
Quadrilateral Plane Element Using Area Coordinates,”
Engineering Mechanics, Vol. 18, No. 4, 2001, pp. 95-101.
[11] X. G. Fu and Y. Q. Long, “Generalized Conforming Qua-
drilateral Plane Elements Based on Analytical Trial Func-
tions,” Engineering Mechanics, Vol. 19, No. 4, 2002, pp.
12-16.
[12] R. D. Cook, D. S. Malkus and M. E. Plesha, “Concepts
and Applications of Finite Element Analysis,” 3rd Edition,
John Wiley & Sons, Inc., New York, 1989.