Engineering
Vol.09 No.05(2017), Article ID:76642,15 pages
10.4236/eng.2017.95024
Numerical Investigation of Turbulent Flow through Rectangular and Biconvex Shaped Trash Racks
Hans O. Åkerstedt*, Sebastian Eller, T. Staffan Lundström
Division of Fluid and Experimental Mechanics, Luleå University of Technology, Luleå, Sweden

Copyright © 2017 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: March 22, 2017; Accepted: May 24, 2017; Published: May 27, 2017
ABSTRACT
Turbulent flow through a trash rack of bars of rectangular and biconvex shapes is considered. A trash rack is composed of an array of bars fitted into a hydro-electric power station to prevent debris and fish to enter the waterway towards the turbine. The work is directed towards modeling a large number of bars for which the flow turn out to have a periodic structure. It is here shown that this case can be simplified with the flow past a single bar together with periodic boundary conditions. Using this approach the head loss is derived for different angles of attack α and blockages P for two shapes of the rack, a rectangular bar and an aerodynamically shaped biconvex bar. It is found that overall loss of the biconvex bars is in general about 15% of the loss for the rectangular case for small angles of attack. For large angle of attack this difference diminishes. Of interest for the biconvex bars is also a local minimum in the head loss for angles approximately greater than 20˚ and for a blockage P around 0.35. This combination of parameters gives a low loss together with an efficient barrier for debris and fishes.
Keywords:
Trash Racks, Head Loss, Turbulence, CFD, Hydropower, Fish Migration

1. Introduction
In all kinds of energy production engineering there is a desire to minimize the energy losses at the same time as to fulfill certain environmental demands. For instance in hydro-electrical engineering there is a desire to minimize the energy losses (Marjavaara and Lundström 2006 [1] , Marjavaara et al. 2007 [2] , Andersson et al. 2013 [3] and Andersson et al. 2014 [4] ) at the same time as there are demands on safe fauna passage and low maintenance costs (Laine et al. 1998 [5] , Laine et al. 1998 [6] , Lundström et al. 2010 [7] , Lundström et al. 2015 [8] , Green et al. 2011 [9] and Andersson et al. 2012 [10] ). To avoid damaging of the water turbines from large floating trash in the waterways, the turbine intakes are protected by grids of racks, so called trash racks as exemplified in Figure 1 being a sketch of one of the turbine intakes in Bruksfors a Power-station in Rickleån, Västerbotten, Sweden own by Skellefteå Kraft. This trash rack is placed in a horizontal plane but the rack can be at any angle to the ground (Calles et al. 2013 [11] ).
The racks may also serve as a barrier for larger fishes like Kelt hinder them to migrate via the turbines where they may be hit by the blades or subjected to high pressure variations which in both cases may lead to a severe injury and death (Schilt 2007 [12] , Whitney et al. 1997 [13] ). Naturally the racks implies a loss of energy and it is therefore important to optimize the form of the racks with respect to energy losses (Bengoechea et al. 2014 [14] ) and at the same time prevent larger fish to enter into the waterways towards the turbine. Different shapes and sizes of the bars of the rack and bar spacing are variables that influence the loss.
There are several experimental studies published with different shape and size of the racks many of which are reviewed in Tsikata et al. (2007 [15] , 2009a [16] , 2009b [17] ). There are, however, only a few numerical studies of the flow in trash racks. This includes Herman et al. (1998) [18] and Meusburger et al. (2001) [19] who used direct numerical simulation (DNS) and k-ε models to analyze the flow through an array of rectangular bars. Ghamry et al. (2012) [20] applied several turbulence models to analyze the flow through an array of 3, 7 - 14 rectangular bars. They show that the turbulent models applied, k-ε, k-ω and the Reynolds stress models, all predict almost the same results. In the experimental and computational studies mentioned above the number of bars in a rack is rather low while in practice the number of bars is quite large. The only numerical study done with a large number of bars is Raynal et al. [21] who considered 51 rectangular bars. Their model was 2D and several different two-equation turbulence models were tested. When compared with experiments their models typically underestimated the head loss with about 10%. They explain this difference that some 3D flow effects cannot be modeled by 2D computations and that the spacer lines which block a fraction of the water depth were included in their model. In
Figure 1. The fish way at Bruksfors in Rickleån with the indicated position of the trashrack. The size of the trash rack is 3 m ´ 6 m. The blue surface represents the free water surface.
the present paper we will address the effect of increasing the number of bars in the model geometry, a model which is slightly different than models considered previous, and show that for a very large number of bars it is possible to simplify the problem of just one single bar with periodic flow conditions. The paper is organized as follows. In Section 2 the geometry and the problem is formulated. In Section 3 we validate the turbulence model by comparison with experimental data by Idelchik, 2008 [22] .
In Section 4 a convergence study is considered in which we increase the number of bars until a well-defined limit of the overall head loss emerges. We compare this limit with the result of using one single bar and periodic flow conditions and suggest that the latter simplified flow configuration can be used for calculations of the head loss for a large number of bars. In Section 5 we apply this simplified geometry to calculate the head loss for different angles of attack and different blockage ratios. In Section 6 we provide similar results of head loss for the more streamlined biconvex shaped bars.
2. Problem Formulation
We consider fluid flow in the geometry shown in Figure 2 and Figure 3. Fluid enters from the negative x-axis with a uniform velocity  and at an angle of attack α and/or incident angle β. In this region (x < 0) a slip boundary condition is applied since the flow is assumed to enter from a larger channel, see Figure 1, where the trash rack is submerged into the bottom of the larger channel. Please notice that it is more common that the trash rack is vertical and that part of it above the water surface. At
and at an angle of attack α and/or incident angle β. In this region (x < 0) a slip boundary condition is applied since the flow is assumed to enter from a larger channel, see Figure 1, where the trash rack is submerged into the bottom of the larger channel. Please notice that it is more common that the trash rack is vertical and that part of it above the water surface. At  the flow is assumed confined and no slip
the flow is assumed confined and no slip
Figure 2. A 3D view of the trash rack geometry and the different inlet flow conditions.
Figure 3. Trash rack geometry in the xy-plane.
boundary conditions are applied. The distance upstream and downstream of the computational domain is chosen such that the results of the computations are not affected by their positions.
The separation between the bars is denoted a, the width of the bars is denoted h and the length of the bars in the y-direction is denoted l. The length of the bars in the z-direction is . The overall direction of the flow towards the trash rack can be described in terms of two angles, an angle of attack α and an incident angle β see Figure 2. In this study case
. The overall direction of the flow towards the trash rack can be described in terms of two angles, an angle of attack α and an incident angle β see Figure 2. In this study case . Since the lengths of the bars in the z-direction are considered very large
. Since the lengths of the bars in the z-direction are considered very large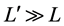 , the fluid flow is approximately two-dimensional, see Figure 3. The second case with oblique incidence angle β is three-dimensional and is not considered in the present paper.
, the fluid flow is approximately two-dimensional, see Figure 3. The second case with oblique incidence angle β is three-dimensional and is not considered in the present paper.
It is convenient to introduce relevant dimensionless parameters. Hence let
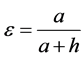 (1)
(1)
be a dimensionless spacing,
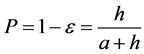 (2)
(2)
a blocking ratio,
 (3)
(3)
the Reynolds number and
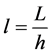 (4)
(4)
a dimensionless length. Also let the number corresponding to the head loss being called loss coefficient H defined as
 (5)
(5)
where  is the pressure far upstream and
is the pressure far upstream and 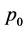 the pressure far downstream of the bars. In this definition, note the normalization with the flux, which is proportional to
the pressure far downstream of the bars. In this definition, note the normalization with the flux, which is proportional to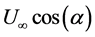 . Dimensional analysis then gives the relation
. Dimensional analysis then gives the relation
 (6)
(6)
Notice that the head loss may also be expressed in meters according to
 .
.
The fluid flow is assumed incompressible and the turbulence is modeled using the k-ω based shear stress transport model (SST) by Menter (1994) implemented in the commercial software Comsol Multiphysics 5.1. The turbulence model belong to the class of Reynolds-averaged Navier-Stokes equations of the form




The SST model has proven valid in a number of cases (Menter [23] ) but in future studies simulations may also be carried out with the more advanced Reynolds stress models or LES (Pope [24] ). This, however, requires even better control of the mesh. There are several alternatives to model the turbulence flow besides; SST-model is not perfect. The meshing can be done in two ways by using the Comsol software, giving an unstructured mesh as well as designing it manually to obtain a structured mesh. For all the results presented the mesh is refined until the value of the loss coefficient becomes independent of the size of the mesh verifying the numerics. In Comsol we use the auto generated mesh feature predefined for fluid dynamic applications for which there are nine different mesh levels going from extremely coarse to extremely fine. In all the results we have started from a coarser mesh and then refined the mesh until the result becomes independent of the mesh. In some cases we have also utilized a user build structured mesh which in general gives faster convergence but in some cases no convergence at all. Therefore the predefined mesh alternative is the one chosen for all the cases considered.
3. Validation of the Turbulence Model with Experimental Data
Let’s first consider a validation of the turbulence models with some experimentally measured head loss data. In the literature the most comprehensive experimental data are from Idelchik [22] in which head loss are given for six rectangular bars and for different angle of attacks and different blockage ratios. In the Figure 4 the normalized numerically calculated head loss is plotted as a function of blockage P and different angle of attack α. The numerical results are compared with the experimental data provided by Idelchik [22] , which are valid for Reynolds number greater than 105. Here the Reynolds number chosen as 

For small angle of attack and small blockage the agreement is good but increasing the blockage the error increases. As for Rayal it is reasonable that this underestimate has to do with 3D effects. For large angle of attack greater than 30 degrees the numerical results get worse with a rather strong deviation from experimental results with an error of 30% for the largest blockage
Considering the case with an angle of attack of 40 degrees there is a somewhat unexpected result for small blockage ratio between 



A conclusion out of this validation is that the SST turbulence model gives good agreement for small angle of attack but for larger angles of attack the agreement is poor.
Whether this has to do with the turbulence model or 3D effects can only be answered by considering more advanced turbulence models such as Reynolds stress models or LES and including 3D effects.
Figure 4. New figure head loss as a function of blockage P and angle of attack α.

Figure 5. New figures flow pattern for angle of attack 


4. Convergence Study of the Flow past an Array of Rectangular Bars
In reality the number of bars in a trash-rack is large. To explore how the head loss vary with the number of bars a study is carried out starting with just a few bars and then the number of bars is increased until the head loss reaches a definite convergent value. For this convergence study the following parameters are used, 




The results indicate that there is a convergence towards a limit for N > 30. An inspection of the corresponding flow field for N = 30 shows that the flow field around each bar seem to have a periodic structure within the inner part of the rack away from the channel walls, see Figure 6.
This is also verified when the flow field is magnified, see Figure 7. The conclusion is that for a large number of bars, the influence on the losses from the channel wall region becomes relatively small. It is then possible to simplify the calculation of the total head loss by considering only one bar and using periodic boundary conditions, see Figure 8. Also since the streamlines in the middle position between two bars are straight up to
By using periodic boundary conditions the computation of the loss coefficient is simplified saving a considerably amount of computational time. The derivation of the loss coefficient for the periodic case becomes 
Figure 6. The flow field for N = 30 showing the periodic structure of the flow.
Figure 7. Magnification of the detailed flow around the bars in Figure 6 with velocity magnitude and streamlines.
Figure 8. Geometric set-up using the simplification of periodic boundary conditions.
Figure 9. Head loss as a function of the number of bars N. The dashed line corresponds to the head loss for one single bar with periodic flow conditions.
5. Head Loss for Rectangular Bars Using Periodic Flow Boundary Conditions
Consider now the case of one bar and periodic boundary conditions, thus simulating a rack with a large number of bars, N > 30. The geometrical set-up is presented in Figure 8 where the inlet and outlet are placed sufficiently far upstream and downstream ensuring a uniform pressure. The loss coefficient as a function of P and 





Next, focus is on Re and for trash racks the typical velocity is around 1 m/s. The following Re are therefore considered 5000, 10,000, 50,000 and 100,000, which for bar thickness 


Expressing the head loss in meters (
Next the influence of 

The conclusions for a trash rack with rectangular bars are that the dimensionless head loss depend strongly on the angle of attack and the blockage, while there is only a weak dependence on Re and the length ratio l. Expressing the losses in meters discloses that Re strongly influence the losses.
6. The Flow past an Array of Biconvex Airfoils
It is interesting to consider the effect of using other shapes of the racks than the
Figure 10. Loss coefficients as a function of the blockage and the angle of attack for periodic boundary conditions.
Figure 11. Loss coefficients as a function of the angle of attack and Reynolds number. Blockage P = 0.4.
Figure 12. Loss coefficients in meters as a function the angle of attack and Reynolds number. P = 0.4.
rectangular bar form, such as more streamlined shapes, since these are expected to give lower values of the head loss. As an example consider a specific biconvex airfoil shape used in supersonic flight, for which the shape is defined by the expression

where s is a parameter with values from 0.0 to 1.0. In the handbook by Idelchik [22] experimental results, in which several types of shapes are considered indicate that this shape overall gives the smallest values of the head loss. The problem is then defined by Figure 14 below.
Figure 13. Loss coefficients as a function of the blockage P and dimensionless length of the bars l. Angle of attack 20˚.
Figure 14. Geometry of flow past an array of biconvex airfoils.
As for the rectangular bars there is a periodicity of the flow as exemplified in Figure 15. The head loss can therefore be derived based on one airfoil together with periodic boundary conditions.
The loss coefficient results while varying the flow ratio and angle of attack are presented in Figure 16. Comparing the loss coefficient for rectangular shape, Figure 10, and the more streamlined airfoil shape in Figure 16, for small angle of attack the latter is considerably smaller, about 15% of the rectangular shape. For large angle of attack this difference is however diminished. Surprisingly for larger angles of attack to the airfoil shape there is a local minimum in the head loss. This result is also found in experiments for the biconvex shaped rack (Idelchik [22] ). This minimum may be of interest in hydraulic-engineering in optimizing loss together with a rather large blockage, leading to a more efficient barrier for large floating trash and fish.
As an example for an angle of attack equal to 30 degrees there is a head loss equal to 

Figure 15. Flow pattern past an array of biconvex airfoils.

Figure 16. Loss coefficient for different blockage and angle of attack 0 - 30 degrees (a) and 30 - 60 degrees (b).
corresponding value for rectangular bars which is 

7. Discussion and Conclusions
The energy losses for so called trash racks are investigated. Trash racks are used in to prevent large debris and fish to enter the water way leading into hydro-turbines. A trash rack can be composed of an array of bars of the different shapes such as rectangular and more aerodynamically shaped bars. The flow direction of water into the rack can be described by the magnitude of the velocity 

Using dimensional analysis a dimensional head loss H is defined according to Equation (9). The head loss is then a function of the dimensionless variables, the angle of attack α the length ratio


The present study is by no means complete since only the two-dimensional flow characterized by the flow in the x-y plane with an angle of attack α is considered. The case with the angle 
Acknowledgements
The authors wish to thank Björn Bragée at Comsol Multiphysics with valuable support using the Comsol Multiphysics software. The work was sponsored by Tillväxtverket, the Swedish Agency of Energy and Stand Up for Energy.
Cite this paper
Åkerstedt, H.O., Eller, S. and Lundström, T.S. (2017) Numerical Investigation of Turbulent Flow through Rectangular and Biconvex Shaped Trash Racks. Engineering, 9, 412-426. https://doi.org/10.4236/eng.2017.95024
References
- 1. Marjavaara, B.D. and Lundström, T.S. (2006) Redesign of a Sharp Heel Draft Tube by a Validated CFD-Optimization International Journal for Numerical Methods in Fluids, 50, 911-924.
https://doi.org/10.1002/fld.1085 - 2. Marjavaara, B.D., Lundström, T.S., Goel, T., Mack, Y. and Shyy, W. (2007) Hydraulic Diffuser Shape Optimisation by Multiple Surrogate Model Approximations of Pareto Fronts. Journal of Fluids Engineering, 129, 1228-1240.
https://doi.org/10.1115/1.2754324 - 3. Andersson, A.G., Andreasson, P. and Lundström, T.S. (2013) CFD-Modelling and Validation of Free Surface Flow during Spilling of a Reservoir in a Down-Scale Model. Engineering Applications of Computational Fluid Mechanics, 7, 159-167.
https://doi.org/10.1080/19942060.2013.11015461 - 4. Andersson, A.G., Hellström, J.G.I., Andreasson, P. and Lundström, T.S. (2014) Effect of Spatial Resolution of a Rough Surface on a Numerically Computed Flow Field with Application to Hydraulic Engineering. Engineering Applications of Computational Fluid Mechanics, 8, 373-381.
https://doi.org/10.1080/19942060.2014.11015522 - 5. Laine, A., Ylinäaräa, T., Heikkiläa, J. and Hooli, J. (1998) Behaviour of Upstream Migrating Whitefish, Coregonus lavaretus in the Kukkolankoski Rapids, Northern Finland. In: Jungwirth, M., Schmutz, S. and Weiss, S., Eds., Fish Migration and Fish Bypasses, Fishing News Books, Oxford, 33-44.
- 6. Laine, A., Kamula, R. and Hooli, J. (1998) Fish and Lamprey Passage in a Combined Denil and Vertical Slot Fishway. Fisheries Management and Ecology, 5, 31-44.
https://doi.org/10.1046/j.1365-2400.1998.00077.x - 7. Lundström, T.S., Hellström, J.G.I. and Lindmark, E.M, (2010) Flow Design of Guiding Device for Downstream Fish Migration. River Research and Applications, 26, 166-182.
- 8. Lundström, T.S., Brynjell-Rahkola, M., Ljung, A.-L., Hellström, J.G.I. and Green, T.M. (2015) Evaluation of Guiding Device for Downstream Fish Migration with In-Field Particle Tracking Velocimetry and CFD. Journal of Applied Fluid Mechanics, 8, 579-589.
https://doi.org/10.18869/acadpub.jafm.67.222.21391 - 9. Green, T., Lindmark, E.M, Lundström, T.S. and Gustavsson, L.H. (2011) Flow Characterization of an Attraction Channel as Entrance to Fishways. River Research and Application, 27, 1290–1297.
https://doi.org/10.1002/rra.1426 - 10. Andersson, A., Lindberg, D.-E., Lindmark, E.M., Leonardsson, K., Andreasson, P., Lundqvist, H. and Lundstrom, T.S. (2012) A Study of the Location of the Entrance of a Fishway in a Regulated River with CFD and ADCP. Modelling and Simulation in Engineering, 2012, Article ID: 327929.
https://doi.org/10.1155/2012/327929 - 11. Calles, O., Karlsson, S., Vezza, P., Comoglio, C. and Tielman, J. (2013) Success of a Low-Sloping Rack for Improving Downstream Passage of Silver Eels at a Hydroelectric Plant. Freshwater Biology, 58, 2168-2179.
https://doi.org/10.1111/fwb.12199 - 12. Schilt, C.R. (2007) Developing Fish Passage and Protection at Hydropower Dams. Applied Animal Behaviour Science, 104, 295-325.
https://doi.org/10.1016/j.applanim.2006.09.004 - 13. Whitney, R.R., Calvin, L.D., Erho, M.W. and Coutant, C.C., (1997) Downstream Passage for Salmon at Hydroelectric Projects in the Columbia River Basin: Development, Installation, and Evaluation. Northwest Power Planning Council, Portland, Document No: 97-15.
- 14. Bengoechea, R.A., Larraonaa, G.S., Ramosa, J.C. and Rivas, A. (1997) Influence of Geometrical Parameters in the Downstream Flow of a Screen under Fan-Induced Swirl Condition. Engineering Applications of Computational Fluid Mechanics, 8, 623-638.
https://doi.org/10.1080/19942060.2014.11083312 - 15. Tsitaka, J.M., Tachie, M.F., Katopodis, C., Teklemariam, E., Ghamry, H., Sydor, K. and Shumilak, B. (2007) A Particle Image Velocimetry Study Turbulent Through Model Trash Rack. Proceedings of the 18th Hydrotechnical Conference, Winnipeg, 22-24 August 2007, 1-10.
- 16. Tsitaka, J.M., Katopodis, C. and Tachie, M.F. (2009a) Experimental Study of Turbulent Flow Near Model Trash Racks. Journal of Hydraulic Research, 47, 275-280.
https://doi.org/10.3826/jhr.2009.3381 - 17. Tsitaka, J.M., Tachie, M.F. and Katopodis, C. (2009b) Particle Image Velocimetry Study of Flow near Trash Rack Models. Journal of Hydraulic Engineering, 135, 671-684.
https://doi.org/10.1061/(ASCE)HY.1943-7900.0000070 - 18. Herman, F., Billeter, P. and Hollenstein, R. (1998) Investigations on the Flow through a Trash Rack under Different Inflow Conditions. Hydroinformatics, Balkema, Rotterdam, 121-128.
- 19. Mueusberger, H., Volkart, P. and Minor, H.-E. (2001) A New Improved Formula for Calculating Trashrack Losses. Proceedings of the 29th IAHR Congress, 4, 804-809.
- 20. Ghamry, H. and Katopodis, C. (2012) Numerical Investigation of Turbulent Flow through Bar Racks in Closed Conduits. Proceedings of the 9th International Symposium on Ecohydraulics, Vienna, 17-21 September 2012.
- 21. Raynal, S., Chatellier, L., David, L., Courret, D. and Larinier, M. (2013) Numerical Simulations of Fish-Friendly Angled Trashracks at Model and Real Scale. 35th IAHR World Congress, Chengdu, 8-13 September 2013.
- 22. Idelchik, I.E. (2008) Handbook of Hydraulic Resistance. Begell House, Danbury.
- 23. Menter, F.R. (1994) Two-Equation Eddy-Viscosity Turbulence Models for Engineering Applications. AIAA Journal, 32, 269-289.
https://doi.org/10.2514/3.12149 - 24. Pope, S.B. (2009) Turbulent Flows. Cambridge University Press, Cambridge.

















