Journal of Modern Physics
Vol.07 No.14(2016), Article ID:71312,12 pages
10.4236/jmp.2016.714167
Linear and Nonlinear Electron Beam Heating of Magnetized, Motional, and Dusty Plasma
Sherif Mohamed Khalil1,2, Badriah Mesfer AL Alotaibi1
1Physics Department, Faculty of Science, Princess Nora Bint Abdurrahman University, Riyadh, KSA
2Plasma Physics & Nuclear Fusion Department, N.R.C., Atomic Energy Authority, Cairo, Egypt
Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: September 1, 2016; Accepted: October 15, 2016; Published: October 19, 2016
ABSTRACT
Recent theoretical work on electron beam heating of magneto-active motional Plasma is presented. Power transfer from beam (plasma heating) and generated electric fields for different physical situations of linear and nonlinear beam-plasma interaction, are studied. Based on previous works [1] [2] , we shall study the effects of dusts and plasma motion (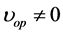 ) on plasma heating. Besides, the case of an inhomogeneity of beam velocity (
) on plasma heating. Besides, the case of an inhomogeneity of beam velocity (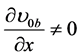 ) is also considered. Taking into consideration nonlinear process, dust, plasma motion, and beam velocity inhomogeneity, are found to play a crucial role via power absorbed by the beam and the generated electric field in the system.
) is also considered. Taking into consideration nonlinear process, dust, plasma motion, and beam velocity inhomogeneity, are found to play a crucial role via power absorbed by the beam and the generated electric field in the system.
Keywords:
Beam-Plasma Interaction, Motional Plasma, Dusty Plasmas, Plasma Heating, Electric Field Generation

1. Introduction
Electron beam-plasma interaction presents a great interest for many applications in areas like development of new methods in amplification and generation of electromagnetic waves, acceleration of charged particles in plasma (Plasma Accelerator). Besides, the electron beam-plasma system showed great importance via plasma generation, design of microwave tubes waveguides, explanation of natural phenomena that occur in space and solar plasmas, material studies, compact torus formation, generation of x-ray and microwave, and others.
The recent development in the electron beam technology has shown the capability of generating powerful electron energy sources, making the electron beam very useful to controlled thermonuclear fusion research or in general, to plasma heating. Besides, the electron beam provides a free energy source for a rich variety of nonlinear process to evolve in a plasma. Accordingly, It is not surprising to find up till now a long list of studies and research on beam-plasma interaction and applications, which was also reviewed by many authors, (e.g., see [1] - [8] and references therein).
Multiple harmonic generation by laser/beam-plasma interaction has been widely investigated [9] - [13] . The generation of harmonics through a nonlinear mechanism driven by bunching at the fundamental has sparked interest as a path toward enhancing and extending the usefulness of an x-ray free-electron laser (FEL) facility. Besides, the sensitivity of nonlinear harmonic generation to electron beam quality is found to play a crucial role via FEL [14] [15] .
Currently, high-order harmonic generation (HHG) is considered as one of the more efficient technique for producing coherent short-wavelength radiation in a broad spectral range [16] . Also, the nonlinear cold plasma-bunched beam interaction represents an interesting application for plasma wakefield accelerator [17] .
From the point of view of beam-plasma interaction, the impact of dust on plasma is an important explosed field of research. It is increasingly being studied these days due to their applications in a wide range of fields. The presence of relatively highly charged and massive dust grains in a plasma can modify or influence the collective phenomena of the plasma. The possible dust modes may explain the extremely low-frequency fluctuations, new channels for the parametric coupling of other waves, generation of wakefields, etc., in dusty plasma. The impurities coming off from the walls of fusion device can create a dusty plasma at the edge of the discharge. These particles can enhance power loss due to radiation and dilution of fuel as well as can cool down the hot ion by charge-exchange process [18] - [23] .
The subject of this paper is to study and investigate the electron beam heating of magneto-active motional dusty plasma under discuss different effects or parameters.
Section 2 explores the interaction of electron beam with a homogeneous magnetized non-motional plasma and plasma heating. Section 3 studies linear, non-motional (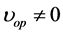 ) and clean plasma in static magnetic field, while Section 4 is devoted to study the effects of the dust on the nonlinear beam interaction with non-motional plasma. Dusty plasma is characterized as a low-temperature ionized gas whose constituents are electrons, ions, and micron-sized dust particulates. The latter is usually negatively charged due to the attachment of the background plasma electrons on the surface of dust grains via collisions. The physical processes in dusty plasmas are interesting because of their importance for a number of applications in space plasmas and the earth s environment, as well as in the laboratory, and in several technologies. The dust grains have a strong effect on energy absorbed in the plasma. In Section 5, we study and investigates the effects of motional plasma (
) and clean plasma in static magnetic field, while Section 4 is devoted to study the effects of the dust on the nonlinear beam interaction with non-motional plasma. Dusty plasma is characterized as a low-temperature ionized gas whose constituents are electrons, ions, and micron-sized dust particulates. The latter is usually negatively charged due to the attachment of the background plasma electrons on the surface of dust grains via collisions. The physical processes in dusty plasmas are interesting because of their importance for a number of applications in space plasmas and the earth s environment, as well as in the laboratory, and in several technologies. The dust grains have a strong effect on energy absorbed in the plasma. In Section 5, we study and investigates the effects of motional plasma (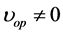 ) on the heating processes due to beam-plasma interaction. Finally, in Section 6, we investigate the effects due to the inhomogeneity of beam velocity on both power absorption and generated electric field.
) on the heating processes due to beam-plasma interaction. Finally, in Section 6, we investigate the effects due to the inhomogeneity of beam velocity on both power absorption and generated electric field.
The electron beam is considered to be injected into plasma under the effect of static magnetic field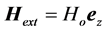 . We use the well known expression for the amount of energy absorbed by the plasma per unit time S as:
. We use the well known expression for the amount of energy absorbed by the plasma per unit time S as:
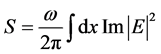 (1)
(1)
where E is the electric field generated in the system.
2. Linear, Non-Motional and Clean Plasma in Static Magnetic Field
The equations of motion, and the continuity equation for electron beam, which travels along the magnetic field are:
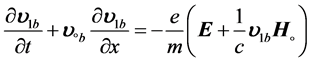 (2)
(2)
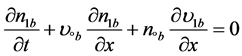 (3)
(3)
while the equation of motion, and the continuity equation for cold inhomogeneous plasma electrons in the static magnetic field are given by:
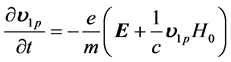 (4)
(4)
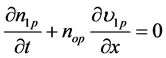 (5)
(5)
where, 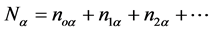 ,
, 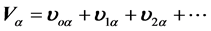 and
and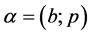 .
.
For non motional plasma .
. 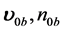 and
and  are the unperturbed velocity and density of the beam and plasma respectively.
are the unperturbed velocity and density of the beam and plasma respectively.
Solving the above system of equations we can derive the following expressions for the perturbed densities:
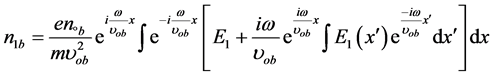 (6)
(6)
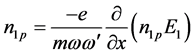 (7)
(7)
where, 

Using relations (6) and (7) in Poisson’s equation

we obtain the following wave equation for the electric field:

where,
Introducing the electric field form:


Then we can write the final solution of (9) in the simple form:

Set (11) into (1), the energy absorbed absorbed by the beam reads:

Relation (12) is investigated for different cases of magnetic field: zero, weak and strong magnetic fields.
3. Linear Dusty Plasma Heating
For dusty plasma, we use the system of Equations (2)-(5) with


Solving the above system of equations we can derive the following expressions for the dust perturbed density:

where, 
Using relations (7) and (8), (15) in Poisson’s equation:

we obtain the following wave equation for the perturbed electric field:

where, 

The energy absorbed in this case reads:


In linear regime, if 

It is clear that, existence of dust leads to less energy absorption by the plasma.
The generated electric field under the effect of dusty and clean plasma is shown in Figure 3.
4. Nonlinear Dusty Plasma Heating
In nonlinear regime, the equation of motion, and the continuity equation for electron beam, which travels along the magnetic field are:


The equation of motion, and the continuity equation for homogeneous plasma electrons in a static magnetic field perpendicular to the plasma are given by:


The dusty nonlinear equations of motion, and continuity are:


Solving the above system of equations we can derive the following expressions for the nonlinear perturbed densities:



where, 
Using relations (25)-(27), and considering the nonlinear form of Poisson’s Equation (16), we obtain the following wave equation for the nonlinear generated electric field:

where, 

Accordingly, we obtain the energy absorption from the beam as:


From (18) and (29) we conclude that:

Ratio (30) shows that, for nonlinear interaction the existence of dust leads to less energy absorption by the plasma as in the linear regime.
Figure 4 shows the generated electric field under the effect of dusty and clean nonlinear plasma. In this regime amplification of electric field in dusty plasma is clear compared to clean plasma.
Generally speaking, nonlinearity plays a crucial role in the amplification of the electric field generated due to beam-plasma interaction, for different situations, compared to linear case.
5. Motional Plasma
For motional plasma, for the electron beam, we use the above system of Equations (2), (3 and (6). On the other hand, the equation of motion, and the continuity equation for homogeneous plasma electrons in a static magnetic field perpendicular to the plasma are given by:



Accordingly, we obtain the following expressions for the perturbed plasma density

Using relations (6) and (34) in Poisson’s Equation (8), we obtain the following wave equation for the electric field in motional plasma

where,


In this case the energy absorbed is:

From this relation and non-motional relation (12) we conclude that:

Ratio (37) shows that, the existence of plasma motion leads to less energy absorption from the beam, as indicated in Figure 6.
Figure 7 shows the reduction of the generated electric field under the effect of motional plasma compared to non-motional plasma.
6. Inhomogeneity of Beam Velocity Effects
Let us consider the case of an electron beam moves with inhomogeneous velocity, i.e.,

motion (2) and continuity. Accordingly, we obtain the perturbed beam velocity and density as:


where, 


Using mathematical tools used in previous sections, we obtain, 

Figure 8 shows that the sharp increase in the generated electric field due to

7. Conclusions and Final Remarks
The Electron Beam Heating of Magneto-Active Motional Dusty Plasma is investigated.
Generally speaking, nonlinear process, dust, plasma motion, and beam velocity inhomogeneity, are found to play a crucial role via power absorbed by the beam and the generated electric field in the system.
It is shown that strong magnetic field generates an intense electric field compared to zero, and weak magnetic fields (Figure 1). In turn, an applied external static magnetic field leads to enhanced power absorption from the electron beam, and accordingly to plasma heating in beam-plasma system.
The energy absorbed from the beam in clean (S) and dusty (Sd) plasma (Figure 2), shows that dust causes a loss in the power energy absorbed in the plasma.
Considering nonlinear interaction, ratio (30) shows that dust leads to less energy absorption by the plasma as in the linear regime.
Figure 3 and Figure 4, shows the generated electric field under the effect of dusty and non-dusty plasmas in linear and nonlinear stages. In this regime amplification of electric field in dusty plasma is less compared to clean plasma. However, in clean plasms, the nonlinear effects associated with the generation of second harmonics, plays an important role in the process of energy transfer from the beam to the plasma as
Figure 1. Generated electric field under the effect of zero, weak and strong magnetic fields.
Figure 2. Energy absorbed from the beam in clean (S) and dusty (Sd) plasmas.
Figure 3. Generated electric field under the effect of dusty and clean linear plasma.
Figure 4. Generated electric field under the effect of dusty and clean nonlinear plasma.
compared with linear stage. This is due to the fact that the electric field intensity at double harmonics is stronger than that of the basic frequency (Figure 5 shows electric field amplification in nonlinear regime).
As application, the generation of second harmonics through a nonlinear mechanism driven by bunching at the fundamental has sparked interest as a path toward enhancing and extending the usefulness of an x-ray free-electron laser (FEL) facility [14] . Currently, high-order harmonic generation (HHG) is considered as one of the more efficient technique for producing coherent short-wavelength radiation in a broad spectral range [16] [24] [25] .
In the motional plasma, the interaction between the electron beam and plasma will occur and induce electrons to exchange energy with plasma waves. The self-magnetic field of the motional electrons is a key parameter of this interaction.
Ratio (37) shows that, the existence of plasma motion leads to less energy absorption of the beam, as indicated in Figure 6. The reduction of the generated electric field under the effect of motional plasma compared to non-motional plasma, is shown in Figure 7.
An interesting result is shown in Figure 8, the sharp increase in the generated electric field due to beam velocity inhomogeneity

Figure 5. Electric field amplification in the nonlinear regime.
Figure 6. Energy absorbed from the beam in non-motional (S) and motional (Smot.) plasmas.
Figure 7. Generated electric field under the effect of motional and nonmotional plasma.
Figure 8. Generated electric field under the effect of inhomogeneous and homogeneous beam velocity.
beam, and positively affects the plasma heating, and may create a type of plasma acceleration.
In due course, we are going to investigate the effects of an inhomogeneous relativistic electron beam (REB) interaction with dusty, motional inhomogeneous plasma placed in an oscillating magnetic field.
Cite this paper
Khalil, Sh.M. and AL Alotaibi, B.M. (2016) Linear and Nonlinear Electron Beam Heating of Magnetized, Motional, and Dusty Plasma. Journal of Mo- dern Physics, 7, 1889-1900. http://dx.doi.org/10.4236/jmp.2016.714167
References
- 1. Khalil, Sh.M. and Al-Enazi, M.M. (2009) Advanced Studies in Theoretical Physics, 3, 369.
http://www.m-hikari.com/astp/astp2009/astp9-12-2009/index.html - 2. Khalil, Sh.M. and Al-Enazi, M.M. (2010) Journal of Modern Physics, 2, 79.
http://dx.doi.org/10.4236/jmp.2011.22013 - 3. Karlicky, M. (2009) The Astrophysical Journal, 690, 189.
http://iopscience.iop.org/article/10.1088/0004-637X/690/1/189/meta - 4. Kartashov, N., Kuzelev, M.V. and Rukhadze, A.A. (2009) Plasma Physics Reports, 35, 169.
http://dx.doi.org/10.1134/S1063780X09020093 - 5. El-Sharif, R.N. and El-Shorbagy, Kh.H. (2013) Physics of Wave Phenomena, 21, 222.
http://dx.doi.org/10.3103/S1541308X13030084 - 6. Llor Aisa, E., et al. (2015) Physics of Plasmas, 22, Article ID: 102704.
http://dx.doi.org/10.1063/1.4933119 - 7. Johzaki, T., et al. (2015) Nuclear Fusion, 55, Article ID: 053022.
http://dx.doi.org/10.1088/0029-5515/55/5/053022 - 8. Lobaev, M.A., et al. (2016) Radiophysics and Quantum Electronics, 58, 816.
http://dx.doi.org/10.1007/s11141-016-9656-z - 9. Voronov, S.L., et al. (2001) Physics Review Letter, 87, Article ID: 133902.
http://dx.doi.org/10.1103/PhysRevLett.87.133902 - 10. Dahiya, D., et al. (2007) Physics of Plasmas, 14, Article ID: 123104.
http://dx.doi.org/10.1063/1.2817088 - 11. Gibbon, P. (1997) IEEE Journal of Quantum Electronics, 33, 1915.
http://dx.doi.org/10.1109/3.641306 - 12. Jarque, E.C. and Plaja, L. (1998) Physics Review, E, 58, 7864.
http://dx.doi.org/10.1103/PhysRevE.58.7864 - 13. Lichters, R., et al. (1996) Physics of Plasmas, 3, 3425.
http://dx.doi.org/10.1063/1.871619 - 14. Biedron, S.G., et al. (2002) Nuclear Instruments and Methods in Physics Research A, 483, 1.
http://dx.doi.org/10.1016/S0168-9002(02)00292-9 - 15. Biedron, S.G., et al. (2002) Physical Review—Special Topics—Accelerators and Beams, 5, Article ID: 030701.
http://dx.doi.org/10.1103/PhysRevSTAB.5.030701 - 16. Corkum, P.B. and Krausz, F. (2007) Nature Physics, 3, 381.
http://dx.doi.org/10.1038/nphys620 - 17. Bilikmen, S. and Nazih, M.R. (1993) Physica Scripta, 47, 204.
http://iopscience.iop.org/article/10.1088/0031-8949/47/2/013 - 18. Gupta, M.R., Sarkar, S., Khan, M. and Ghosh, S. (2003) Pramana Journal of Phys., Indian, 61, 1197.
http://link.springer.com/article/10.1007/BF02704416 - 19. Vranjes, J. and Tanaka, M.Y. (2002) Physical Review E, 66, Article ID: 037401.
http://dx.doi.org/10.1103/PhysRevE.66.037401 - 20. Rao, N.N. (1998) Physica Scripta, T75, 1506.
http://iopscience.iop.org/article/10.1238/Physica.Topical.075a0017
9/pdf - 21. Ricci, P., et al. (2001) Physics of Plasmas, 8, 769.
https://www.deepdyve.com/lp/american-institute-of-physics/plasma-kinetics-in-dusty-plasmas-IMFSzIh2Rv - 22. Johzahi, T., et al. (2003) Fusion Science and Technology, 43, 428.
http://www.ans.org/store/article-288/ - 23. Korenev, S. (2001) 19th IEEE Particle Accelerator Conference, Chicago, IL, 18-22 June 2001, 2332.
http://dx.doi.org/10.1109/pac.2001.987369 - 24. ozmus, W., et al. (2005) 32nd EPS Conference on Plasma Physics, Tarragona, 27 June-1 July 2005, 29C, O-1.006
http://citeseerx.ist.psu.edu/viewdoc/summary?doi=10.1.1.493.4476 - 25. Dromey, B., et al. (2006) Nature Physics, 2, 456.
http://dx.doi.org/10.1038/nphys338














