Journal of Modern Physics
Vol. 4 No. 8A (2013) , Article ID: 36070 , 13 pages DOI:10.4236/jmp.2013.48A004
A Cyclic Cosmological Model Based on the f(ρ) Modified Theory of Gravity
University of California, Berkeley, USA1
Email: yaoming_shi@yahoo.com
Copyright © 2013 Yaoming Shi. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Received June 6, 2013; revised July 1, 2013; accepted August 3, 2013
Keywords: Modified Gravity; Dark Energy; Cosmology; Nonsingular; Cosmological Constant
ABSTRACT
We consider FLRW cosmological models for perfect fluid (with ρ as the energy density) in the frame work of the f(ρ) modified theory of gravity [V. N. Tunyak, Russ. Phys. J. 21, 1221 (1978); J. R. Ray, L. L. Smalley, Phys. Rev. D. 26, 2615 (1982)]. This theory, with total Lagrangian R-f(ρ), can be considered as a cousin of the F(R) theory of gravity with total Lagrangian F(R)-ρ. We can pick proper function forms f(ρ) to achieve, as the F(R) theory does, the following 4 specific goals, 1) producing a non-singular cosmological model (Ricci scalar and Ricci tensor curvature are bounded); 2) explaining the cosmic early inflation and late acceleration in a unified fashion; 3) passing the solar system tests; 4) unifying the dark matter with dark energy. In addition we also achieve goal number 5) unify the regular matter/energy with dark matter/energy in a seamless fashion. The mathematics is simplified because in the f(ρ) theory the leading terms in Einstein’s equations are linear in second order derivative of metric wrt coordinates but in the F(R) theory the leading terms are linear in fourth order derivative of metric wrt coordinates.
1. Introduction
The rapid development of observational cosmology started from 1990s shows that the universe has undergone two phases of cosmic acceleration. The first one is called cosmic early inflation [1-5] that occurred prior to the radiation domination (see [6-8] for reviews). The second accelerating phase started after the matter domination. The unknown component (dark energy) gave rise to this late time cosmic acceleration [9-28] (see [29-34] for reviews).
Various theories are developed in an effort to explain the cosmic early inflation and late accelerated expansion. The 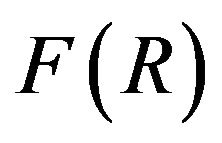 modified theories of gravity (see [35-37] for reviews) have recently [38,39] become one of the leading popular candidates in 1) producing a non-singular cosmology model
modified theories of gravity (see [35-37] for reviews) have recently [38,39] become one of the leading popular candidates in 1) producing a non-singular cosmology model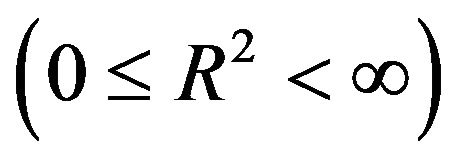 ,
,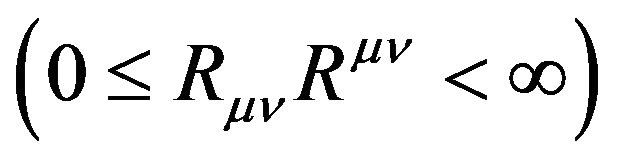 ; 2) unifying the cosmic early inflation and late accelerated expansion in a continuous fashion; 3) passing the solar tests; 4) unifying the dark matter with dark energy.
; 2) unifying the cosmic early inflation and late accelerated expansion in a continuous fashion; 3) passing the solar tests; 4) unifying the dark matter with dark energy.
In this paper we consider cosmological models based on the (less well known) 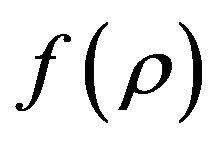 modified theories of gravity for perfect fluid [40,41]. We show that, like the
modified theories of gravity for perfect fluid [40,41]. We show that, like the
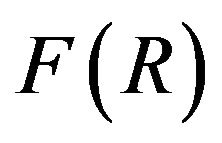 theories, the
theories, the 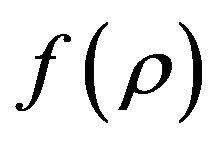 theory can also accomplish the same 4 goals. In addition we show that with
theory can also accomplish the same 4 goals. In addition we show that with 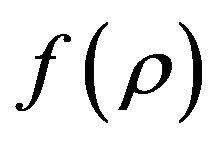 theory we can accomplish goal number 5) unifying the regular matter/energy with dark matter/energy in a seamless fashion. One added benefit is that the mathematics is simplified in
theory we can accomplish goal number 5) unifying the regular matter/energy with dark matter/energy in a seamless fashion. One added benefit is that the mathematics is simplified in 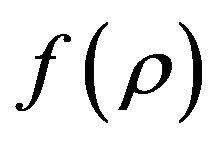 theory. This is because the leading terms in Einstein’s equations are linear in
theory. This is because the leading terms in Einstein’s equations are linear in 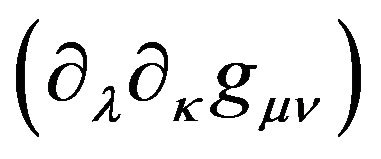 in the
in the 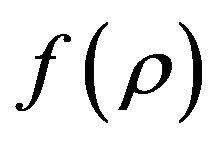 theory but are linear in
theory but are linear in 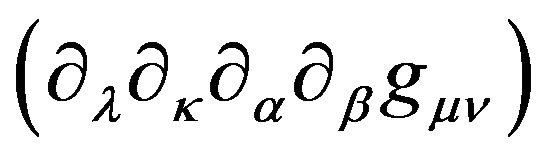 in the
in the 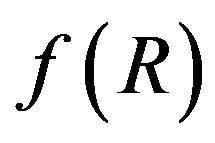 theories.
theories.
In Section 2, we briefly review the 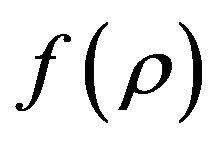 theory. In Section 3, we consider FLRW cosmology and discuss the resulting Friedmann equations in two flavors: one kind is in terms of
theory. In Section 3, we consider FLRW cosmology and discuss the resulting Friedmann equations in two flavors: one kind is in terms of 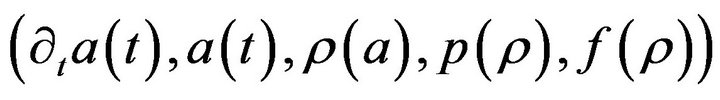 and the other kind is in terms of
and the other kind is in terms of
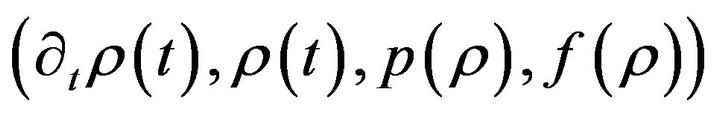 . In Section 4, we consider a cyclic cosmological model and go through the checklist to see if we can accomplish 5 goals mentioned in the abstract. In Section 5, we compare
. In Section 4, we consider a cyclic cosmological model and go through the checklist to see if we can accomplish 5 goals mentioned in the abstract. In Section 5, we compare 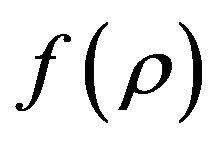 theory with
theory with 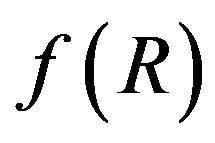 theories, Chaplygin gas, NED etc. Section 6 is the conclusion.
theories, Chaplygin gas, NED etc. Section 6 is the conclusion.
2. The 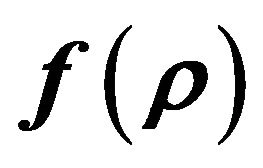 Theory of Gravity
Theory of Gravity
In 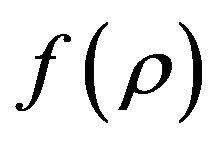 theory of gravity for isentropic perfect fluid [40,41], the Einstein equations and the energy-momentum density tensor, derived from a Hilbert-Einstein like action,
theory of gravity for isentropic perfect fluid [40,41], the Einstein equations and the energy-momentum density tensor, derived from a Hilbert-Einstein like action, 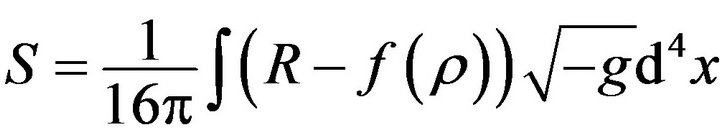 , are given by (with units
, are given by (with units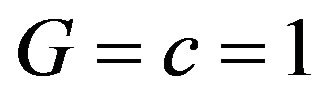 ):
):
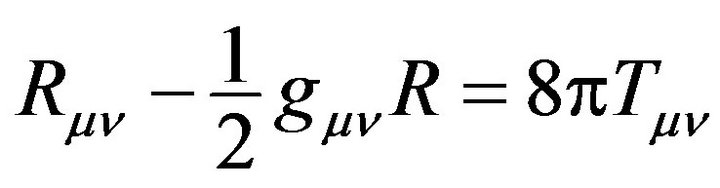 (2.1)
(2.1)
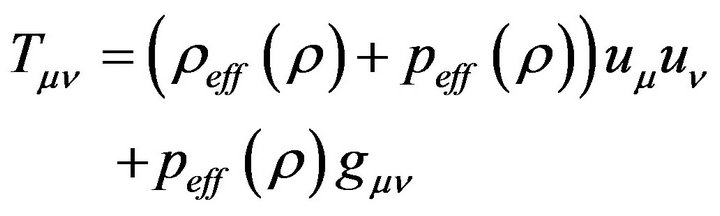 (2.2)
(2.2)
where the effective energy density and the effective pressure are given by:
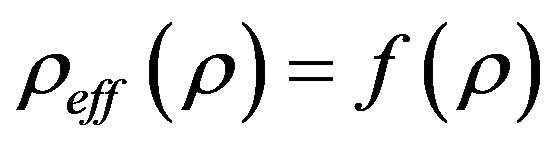 (2.3)
(2.3)
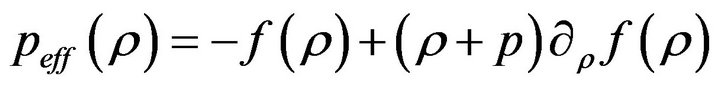 (2.4)
(2.4)
In (2.1)-(2.4)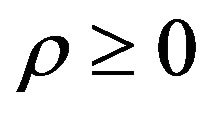 ,
, 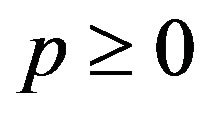 are the energy density and the pressure of the perfect fluid. Throughout this paper, they are always nonnegative. The effective equation of state parameter,
are the energy density and the pressure of the perfect fluid. Throughout this paper, they are always nonnegative. The effective equation of state parameter, 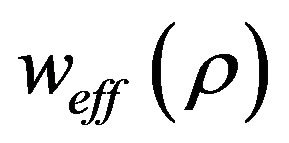 , is then given by:
, is then given by:
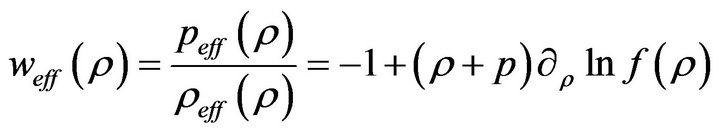 (2.5)
(2.5)
When 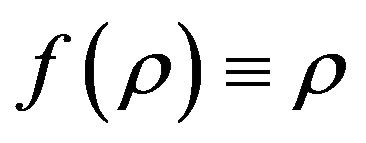 we have
we have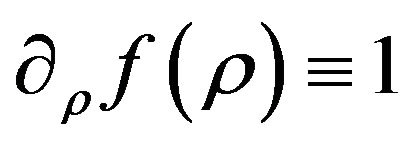 ,
, 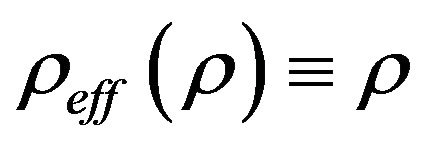 ,
, 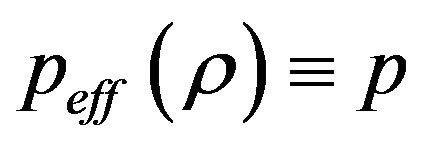 ,
, 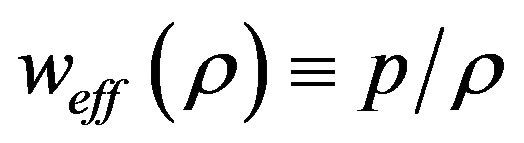 and (2.2) reduces to the standard expression for perfect fluid. The terms
and (2.2) reduces to the standard expression for perfect fluid. The terms 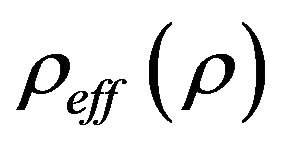 and
and 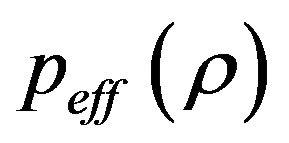 may be considered as the pressure and energy density for an effective perfect fluid.
may be considered as the pressure and energy density for an effective perfect fluid.
We would like to emphasize that the gravitational Lagrangian, 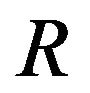 , is the same as in the Einstein’s general relativity. Only the material Lagrangian is generalized from
, is the same as in the Einstein’s general relativity. Only the material Lagrangian is generalized from 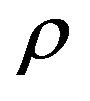 to
to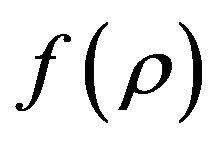 . We would also like to emphasize that the function
. We would also like to emphasize that the function 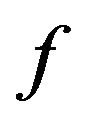 in
in 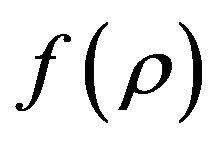 theory is an arbitrary function, just like function
theory is an arbitrary function, just like function  in
in 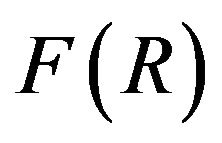 theory.
theory.
Since there is no detailed derivation of the energymomentum tensor (2.2)-(2.4) in [40], and the formulas for energy-momentum tensor in [41] are different from (2.2)-(2.4), we follow the standard text book procedure and provide a detailed derivation of the energy-momentum tensor (2.2)-(2.4) in Appendix A.
One benefit of using the concept of effective perfect fluid is that all the exact solutions in the literature for perfect fluid with nonzero and independent pressure are solutions of (2.1) and (2.2). For example, if  and
and  are not related by an equation of state and if
are not related by an equation of state and if
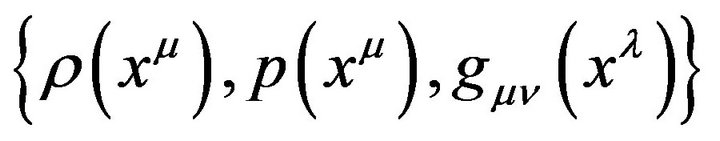 solves the traditional Einstein’s equation for perfect fluid, then
solves the traditional Einstein’s equation for perfect fluid, then
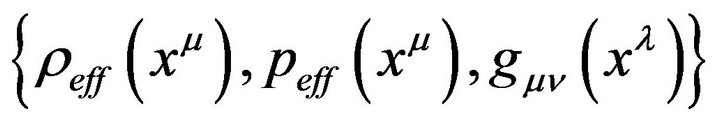 is a solution as well. For given
is a solution as well. For given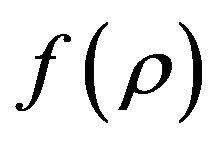 , we can then obtain
, we can then obtain

 .
.
We remark that the conservation of the enegy-momentum becomes:
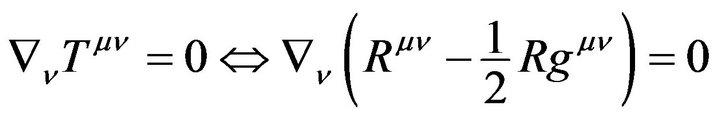 (2.6)
(2.6)
The conventional formula for the conservation of the enegy-momentum becomes the low-energy approximation of (2.6):
 (2.7)
(2.7)
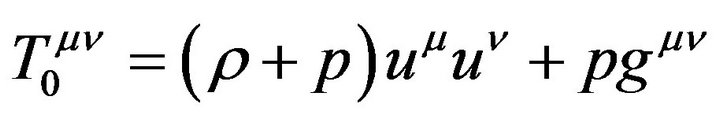 (2.8)
(2.8)
One consequence of using this effective perfect fluid concept is that the following energy conditions may or may not be satisfied in general.
WEC: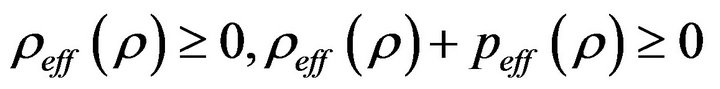 ; (2.9)
; (2.9)
NEC: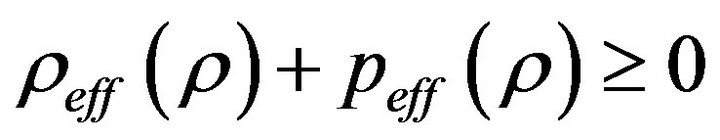 (2.10)
(2.10)
SEC: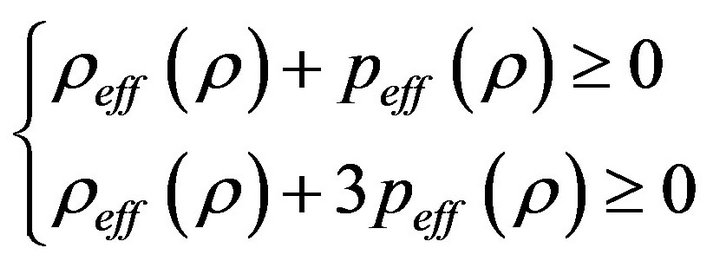 ; (2.11)
; (2.11)
DEC: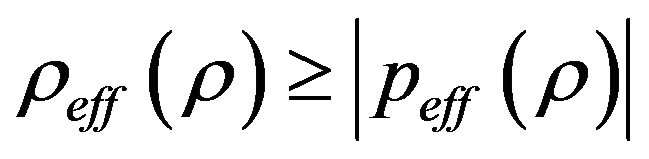 . (2.12)
. (2.12)
We remark that energy-momentum conservation in (2.6) is a different concept than various energy conditions in (2.9)-(2.12). (2.6) is an equality but (2.9)-(2.12) are inequalities. (2.5) involves covariant-derivative but (2.9)-(2.12) do not.
The criteria for the selection of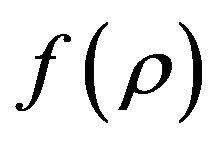 , in our opinion, is
, in our opinion, is  when
when 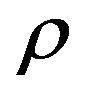 is small. This condition is necessary for passing the solar system tests. The cosmological constant
is small. This condition is necessary for passing the solar system tests. The cosmological constant  could be included in
could be included in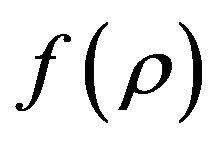 .
.
In Table 1, we present blackbody radiation inspired choices of function 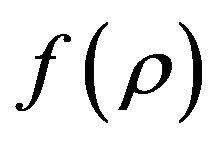 that, as we show later, can be used to solve the intrinsic singularity problems in GR when
that, as we show later, can be used to solve the intrinsic singularity problems in GR when 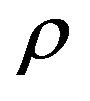 goes to infinity and to make the
goes to infinity and to make the 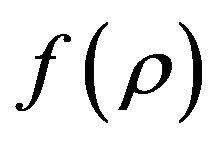 theory non-singular.
theory non-singular.
In Table 1, the form 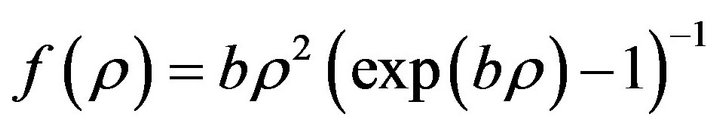
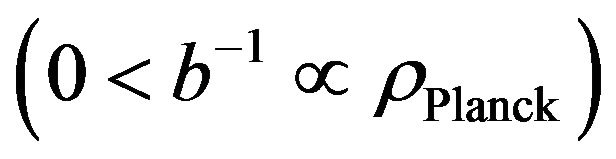 of Table 1 is inspired by the radiation energy density distribution formula,
of Table 1 is inspired by the radiation energy density distribution formula,
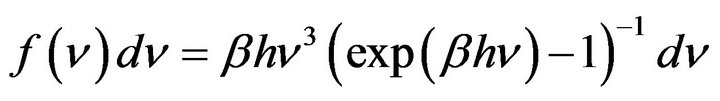 , that Planck [42,43]
, that Planck [42,43]
derived in 1901 to solve the ultraviolet  divergence problem of blackbody radiation. The form
divergence problem of blackbody radiation. The form 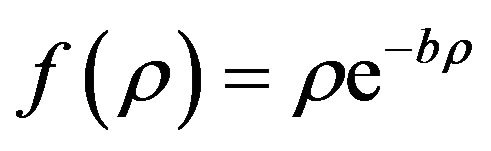 in Table 1 is similar to Wien’s distribution formula,
in Table 1 is similar to Wien’s distribution formula,
 . In this sense, the form of
. In this sense, the form of 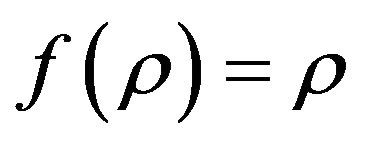 in Einstein’s original general relativity theory, resembles the Rayleigh-Jeans distribution formula,
in Einstein’s original general relativity theory, resembles the Rayleigh-Jeans distribution formula,
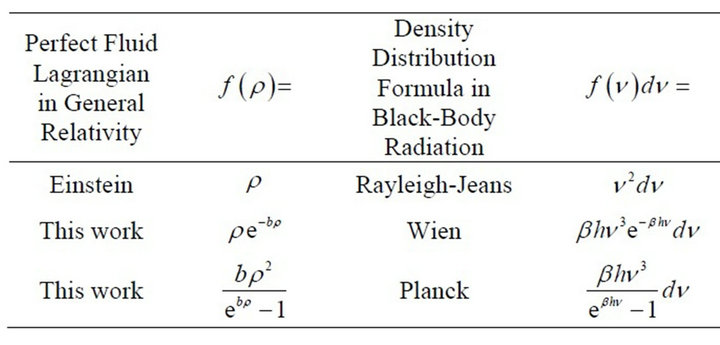
Table 1. Comparison of the perfect fluid Lagrangian f(ρ) in General Relativity with the density distribution formula f(v) in black-body radiation (for simplicity, unimportant constants are removed in various black-body radiation formulas).
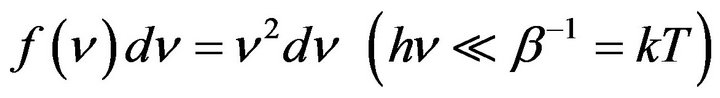 .
.
We are amazed, as we show later, that Planck’s magic black-body radiation formula would show its charm again, after over 100 years, in shedding some light on solving the UV divergence problem (intrinsic singularity inside of black hole or at naked singularity, etc.) in Einstein’s general relativity theory of gravity as well. Later on we find out that the exponential in
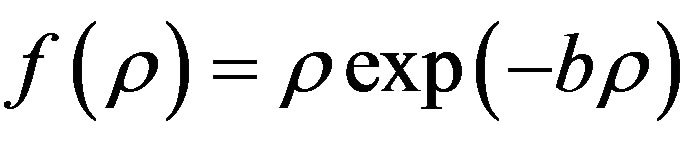 or
or 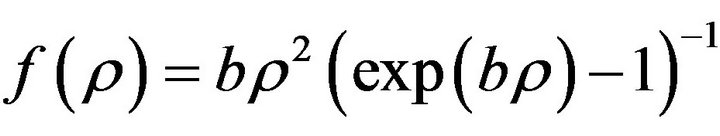 are not convenient for subsequent mathematical manipulation and rational functions like
are not convenient for subsequent mathematical manipulation and rational functions like 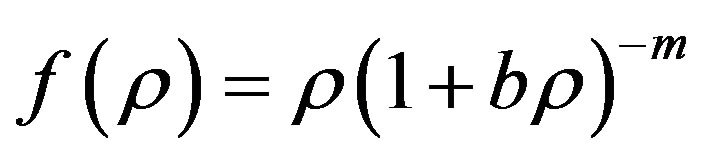 (
(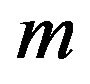 is a positive integer) can do similar job in making
is a positive integer) can do similar job in making 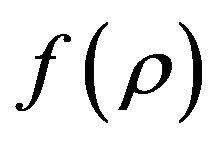 nonsingular.
nonsingular.
From (2.1)-(2.4) we obtain:
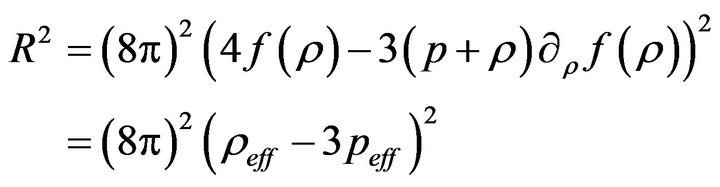 (2.13)
(2.13)
 (2.14)
(2.14)
So for given  as long as we pick function
as long as we pick function 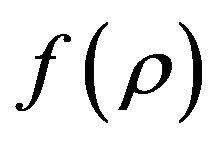 such that:
such that:
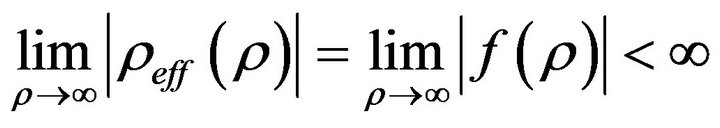 (2.15)
(2.15)
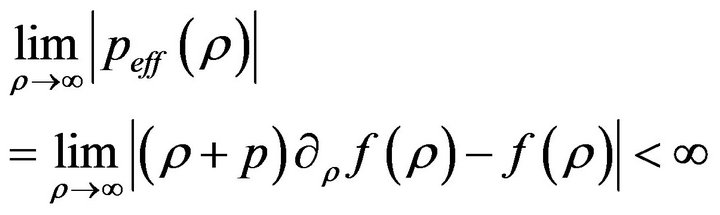 (2.16)
(2.16)
We can deduce from (2.13)-(2.14) that
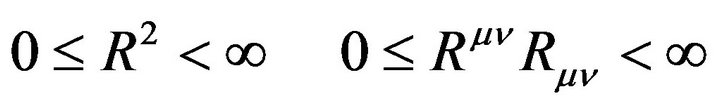 (2.17)
(2.17)
The black-body radiation inspired formulas
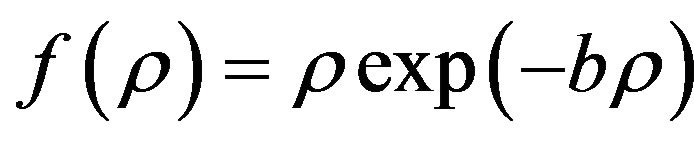 or
or  are clearly satisfying the conditions (2.13)-(2.14) and can be used to make Ricci scalar curvature and the Ricci curvature tensor nonsingular. If we assume
are clearly satisfying the conditions (2.13)-(2.14) and can be used to make Ricci scalar curvature and the Ricci curvature tensor nonsingular. If we assume
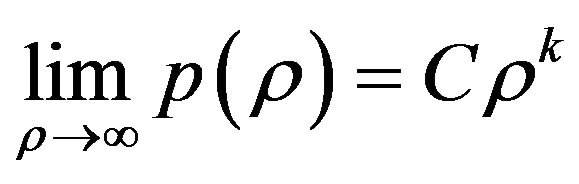 , then we may select
, then we may select
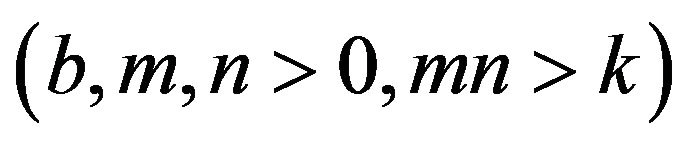
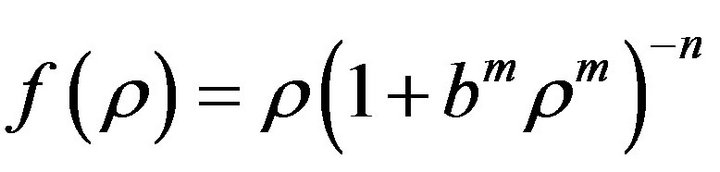 to make R2
to make R2
and 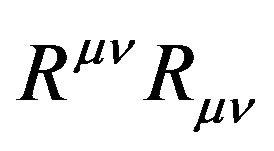 nonsingular as well. Equations (2.15) and (2.16) serve as one of the sufficient conditions for making the
nonsingular as well. Equations (2.15) and (2.16) serve as one of the sufficient conditions for making the 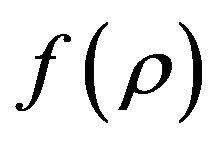 theory of gravity nonsingular.
theory of gravity nonsingular.
3. The Friedmann Equations
In Friedmann-Lemaitre-Robertson-Walker (FLRW) cosmology, the comoving (infalling) spherical symmetric line element is given by [44]:
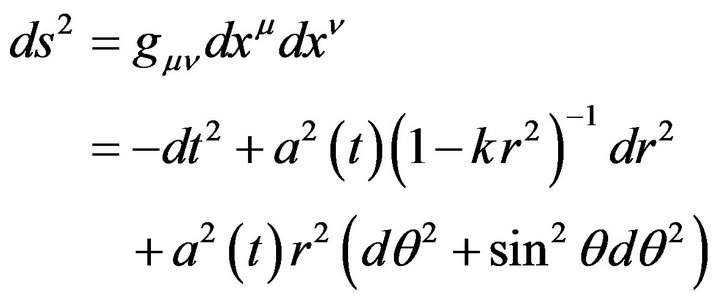 (3.1)
(3.1)
where 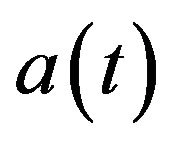 is the scale factor and
is the scale factor and 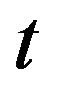 is the cosmological time. The energy density
is the cosmological time. The energy density 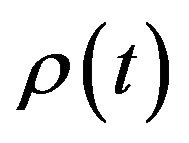 and pressure
and pressure  are assumed to be functions of
are assumed to be functions of  as well. The Einstein’s equations (2.1) then reduce to the Friedmann equations [40]:
as well. The Einstein’s equations (2.1) then reduce to the Friedmann equations [40]:
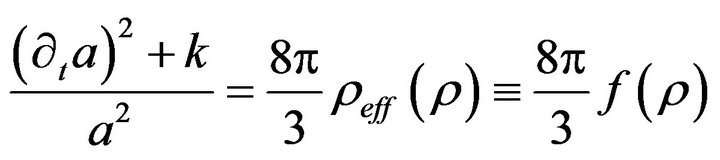 (3.2)
(3.2)
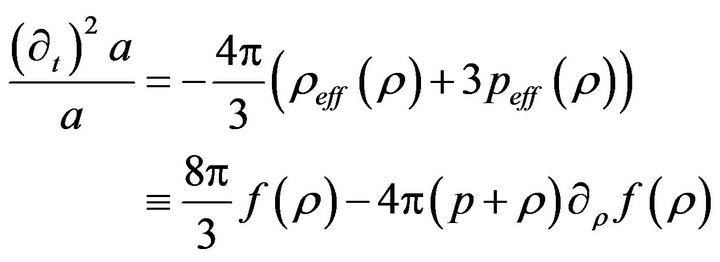 (3.3)
(3.3)
From the conservation of the enegy-momentum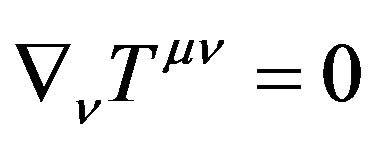 , one nonzero condition remains [40]:
, one nonzero condition remains [40]:
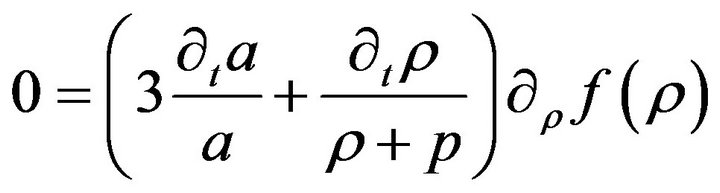 (3.4)
(3.4)
Assuming that 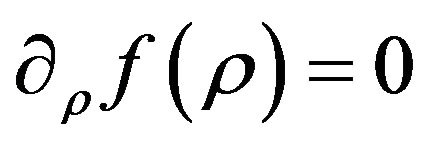 only occurs at isolated points, (3.4) then reduces to
only occurs at isolated points, (3.4) then reduces to
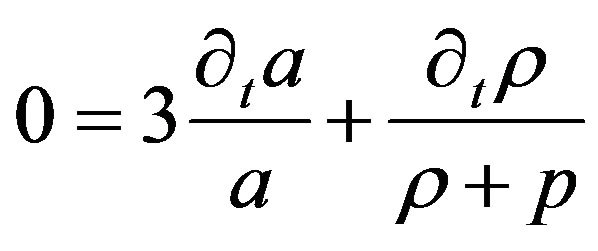 . (3.5)
. (3.5)
Equations (3.3) and (3.5) are not independent; we can pick one and derive the other. Given 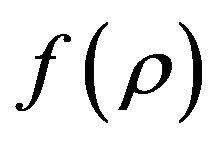 and
and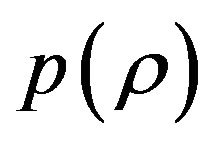 , Equations (3.2) and (3.5) can be used to solve the time evolution for the scale factor
, Equations (3.2) and (3.5) can be used to solve the time evolution for the scale factor  and energy density
and energy density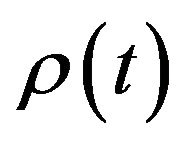 . Equation (2.5) for
. Equation (2.5) for 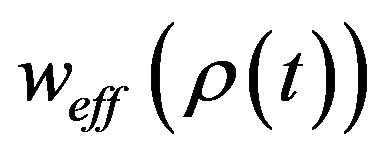 will then tell us what effective equation of state looks like at that
will then tell us what effective equation of state looks like at that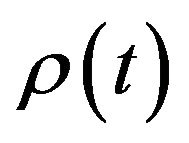 .
.
We copied Table 2 from [45] to demonstrate what effective equation of state 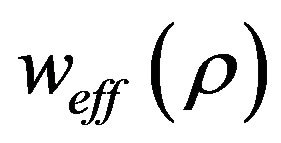 may look like at various stages of
may look like at various stages of  (the last entry is from [46]). In Figure 1, we will show that the effective equation of state
(the last entry is from [46]). In Figure 1, we will show that the effective equation of state 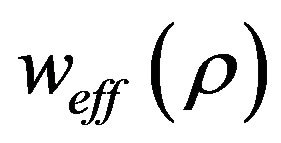 for a new cyclic universe model does vary from less than −1 to larger than +1 in a continuous fashion.
for a new cyclic universe model does vary from less than −1 to larger than +1 in a continuous fashion.
Using (3.5) we obtain

Table 2. Effective equation of state weff(ρ) at various values of ρ.

Figure 1. The plot of effective equation of state weff(ρ) vs. ρ for the M-shaped f(ρ) of (4.1) Numerical constants are l = m = n = 1, p = wρ, w = 0, α = 10, β = 6, γ = 1, λ = 10−3. For convenience, we also indicate the points A, B, C, D, and E that are shown in Figure 1 and defined in the main text above. The two dashed lines are for weff(ρ) = −1, the phantom cross; and weff(ρ) = +1, the cross over to ultrastiff perfect fluid (see the last entry of Table 2).
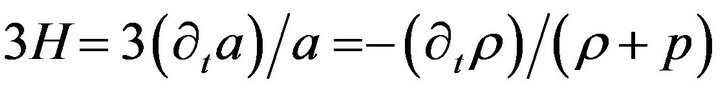 (
(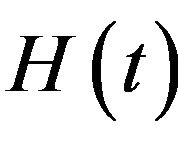 is Hubble function) and
is Hubble function) and 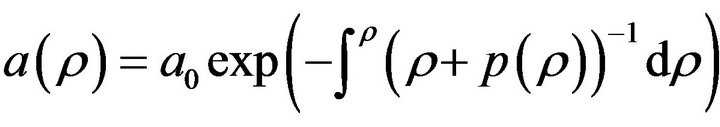 where
where 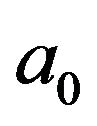
is a constant of integration. Substituting these results into (3.2), we can rewrite the Friedmann Equation (3.2) as:
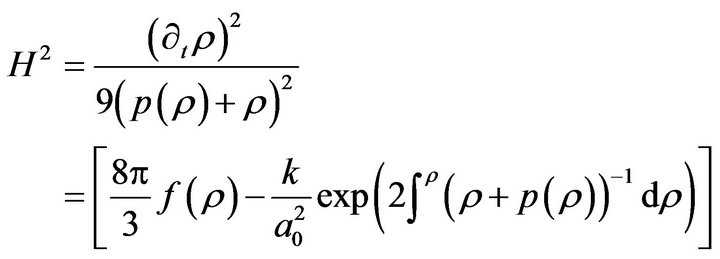 (3.6)
(3.6)
For spatially flat  model, (3.6) reduces to:
model, (3.6) reduces to:
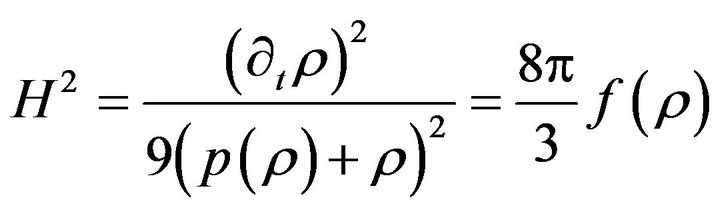 (3.7)
(3.7)
This is probably as far as we can go without specifying 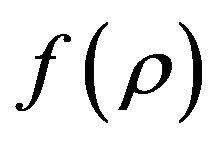 and
and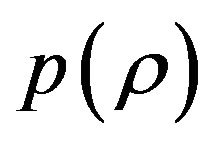 . We would prefer to work with Friedman equations in the format of (3.6) and (3.7) instead of the Friedman equations in the format of (3.2) and (3.5). This is because, as we show in the next section, that we do not need to specify the Equation of State
. We would prefer to work with Friedman equations in the format of (3.6) and (3.7) instead of the Friedman equations in the format of (3.2) and (3.5). This is because, as we show in the next section, that we do not need to specify the Equation of State 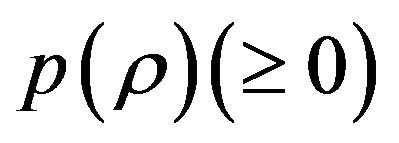 to obtain major features of cosmology models when
to obtain major features of cosmology models when 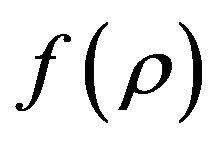 is given.
is given.
We could bundle (3.2) and (3.5) together and write the Friedmann equations in the following different formats as [40]:
 (3.8)
(3.8)
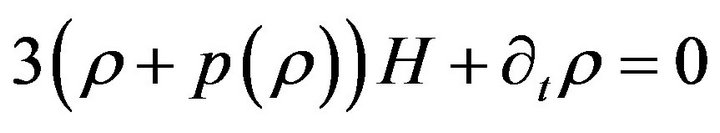 (3.9)
(3.9)
We would consider the Friedmann equations in terms of 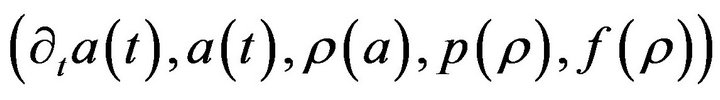 as in (3.8) and (3.9) or in terms of
as in (3.8) and (3.9) or in terms of 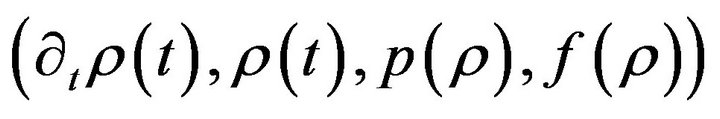 as in (3.6)(3.7) as one of the major results of the
as in (3.6)(3.7) as one of the major results of the 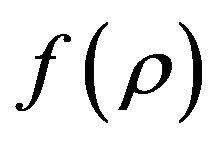 theory of gravity.
theory of gravity.
This set of Friedmann equations are clearly different from but also closely related to various (modified/generalized) Friedmann equations in the literature [47-53]. For example [47] considered a dark fluid with Equation of State like,
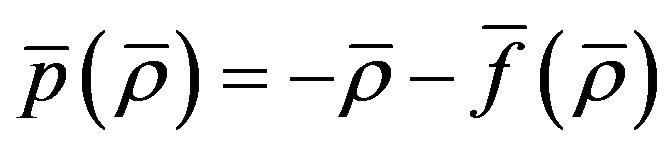 (3.10)
(3.10)
In notation of the current work, the Einstein’s equations are given by
 (3.11)
(3.11)
The Friedmann equations in this case become,
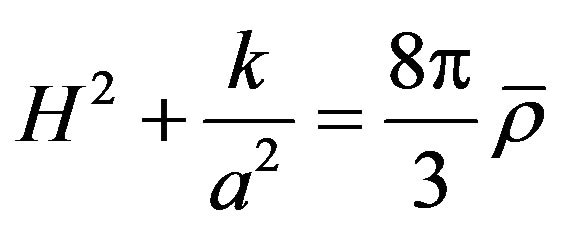 (3.12)
(3.12)
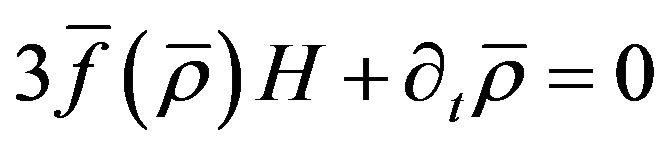 (3.13)
(3.13)
We can clearly see that (3.11) and (3.12) is similar to but different from (3.8) and (3.9).
1) If we identify 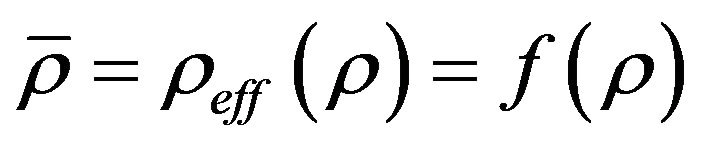 and identify
and identify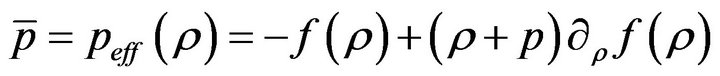 , then (3.12) is identical to (3.8), but (3.13) is different from (3.9). The function
, then (3.12) is identical to (3.8), but (3.13) is different from (3.9). The function 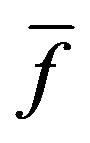 and
and 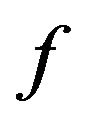 are related via,
are related via,
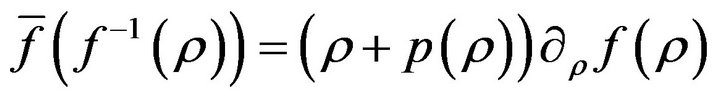 .
.
2) If we identify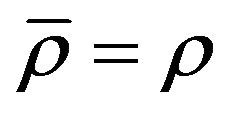 , then (3.13) is identical to (3.9), but (3.12) is different from (3.8).
, then (3.13) is identical to (3.9), but (3.12) is different from (3.8).
Other examples are considered in [48] where the Friedman equations and Equation of State go like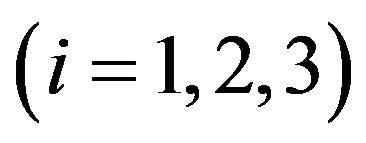 ,
,
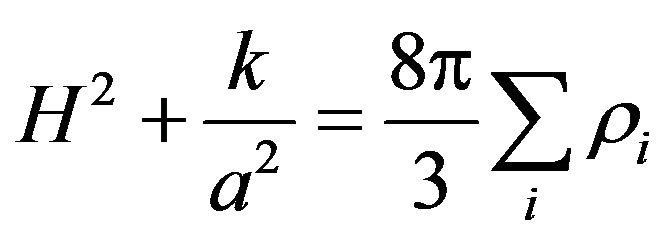 (3.14)
(3.14)
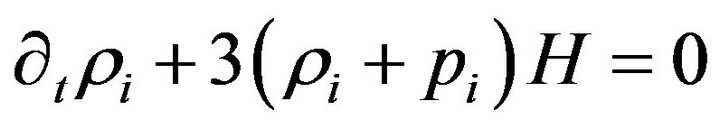 (3.15)
(3.15)
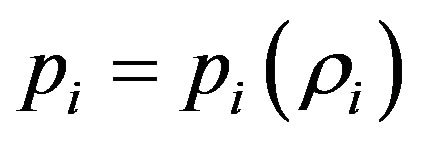 (3.16)
(3.16)
We can also show that (3.14)-(3.16) is similar to but different from (3.8) and (3.9).
1) If we identify 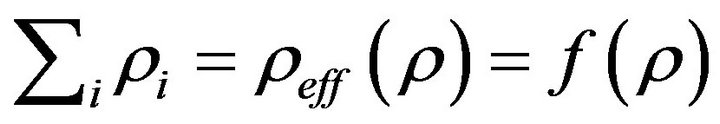 and identify
and identify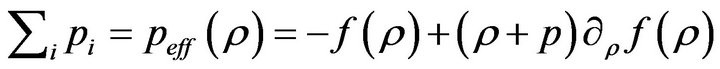 , then (3.14) is identical to (3.8), but (3.15) is different from (3.9).
, then (3.14) is identical to (3.8), but (3.15) is different from (3.9).
2) If we identify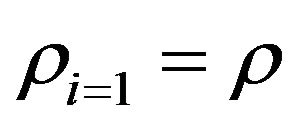 , then (3.15) with
, then (3.15) with 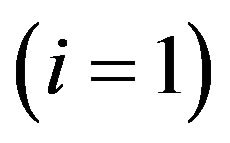 is identical to (3.9), but (3.14) is different from (3.8).
is identical to (3.9), but (3.14) is different from (3.8).
4. A Cyclic Cosmological Model
We now consider a concrete and spatially flat 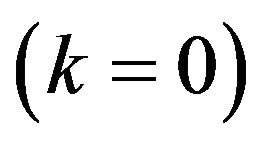 cosmological model. We will show that our model does not rely on the signature of the 3D space curvature
cosmological model. We will show that our model does not rely on the signature of the 3D space curvature 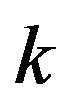 to make universe close, open, or flat anymore. The function
to make universe close, open, or flat anymore. The function 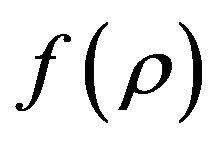 is assumed to be a 7-parameter M-shaped:
is assumed to be a 7-parameter M-shaped:
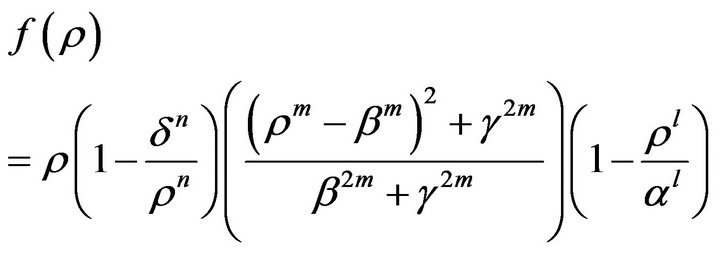 (4.1)
(4.1)
where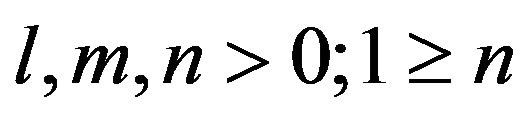 ;
;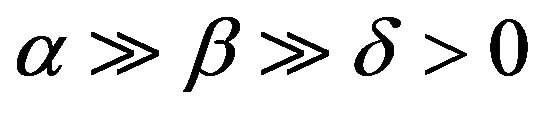 ;
; .
.
Function 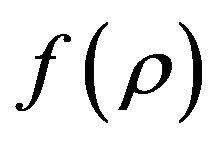 has two positive roots
has two positive roots 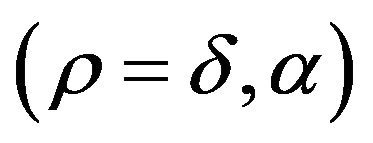
and two complex-conjugated roots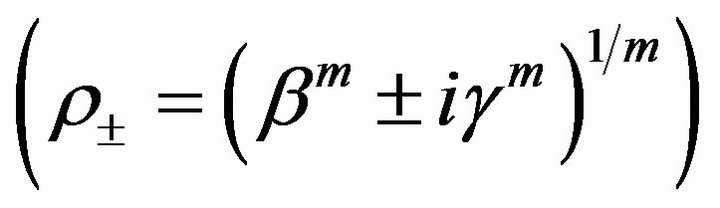 .
.
And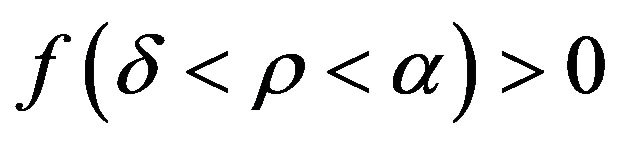 . The constant
. The constant 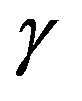 is selected such that
is selected such that 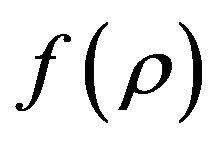 has a local minimum near
has a local minimum near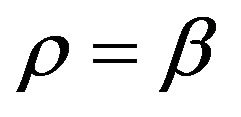 . Thus
. Thus 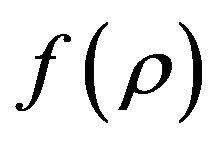 is M-shaped.
is M-shaped.
This form of 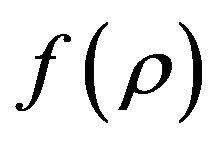 is the combination of 3 forms that often appeared in the literature; namely the RS brane world [54-57] induced form (a)
is the combination of 3 forms that often appeared in the literature; namely the RS brane world [54-57] induced form (a)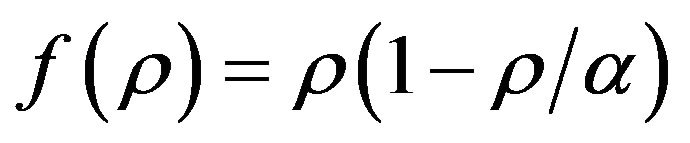 , the Cardassian expansion [58] form (b)
, the Cardassian expansion [58] form (b)

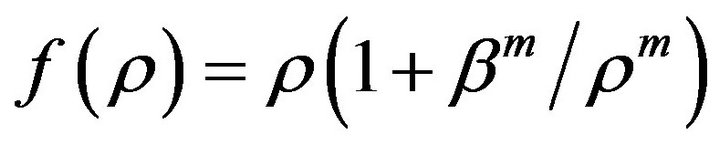 , and cosmology constant form (c)
, and cosmology constant form (c)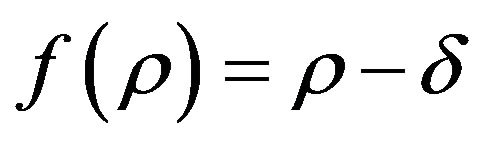 . The form (a) is used for getting rid of intrinsic singularity and producing bouncing solution; form (b) and (c) for explaining cosmic late accelerated expansion. We would like to mention that form
. The form (a) is used for getting rid of intrinsic singularity and producing bouncing solution; form (b) and (c) for explaining cosmic late accelerated expansion. We would like to mention that form 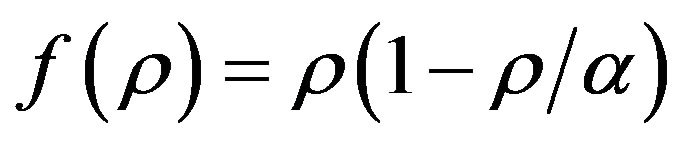 was already used in the original paper of
was already used in the original paper of 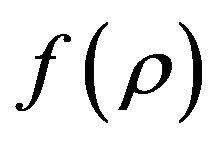 theory of gravity [40] for getting rid of the intrinsic singularity at
theory of gravity [40] for getting rid of the intrinsic singularity at 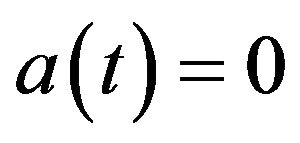 and providing a bouncing solution in the Oppenheimer-Snyder dust collapsing model [59,60].
and providing a bouncing solution in the Oppenheimer-Snyder dust collapsing model [59,60].
We substitute (4.1) into (3.7) and also rewrite the result in a form mimic 1D Newtonian dynamics:
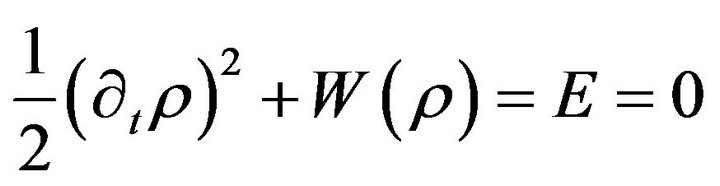 (4.2)
(4.2)
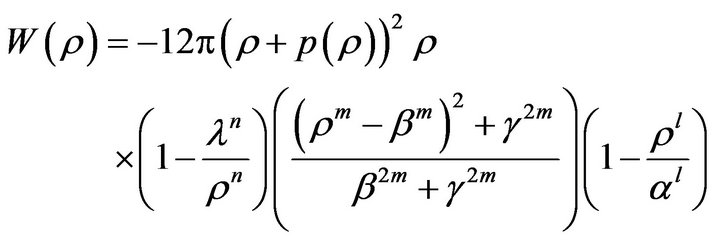 (4.3)
(4.3)
Equation (4.2) represents a particle moving in a 1D potential well 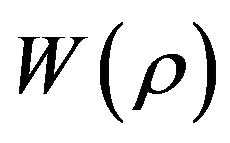 in classic mechanics.
in classic mechanics.
We remark that the Firedmann equations expressed in terms of  like those in (4.2) and (4.3) are better suited for further discussion and treatment in
like those in (4.2) and (4.3) are better suited for further discussion and treatment in 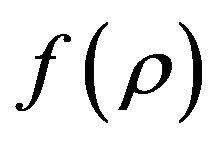 theory than those traditional ones expressed in terms of
theory than those traditional ones expressed in terms of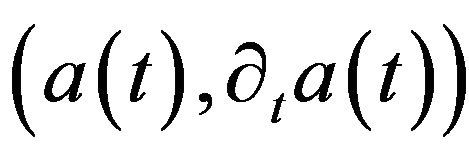 . This is because we do not need to specify function
. This is because we do not need to specify function 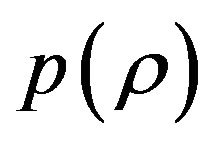 yet in (4.2) and (4.3).
yet in (4.2) and (4.3).
As shown in the Figure 2 below that potential well 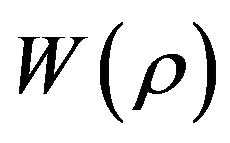 is W-shaped (inverse of M-shape because of the negative sign in front of it). Inherited from
is W-shaped (inverse of M-shape because of the negative sign in front of it). Inherited from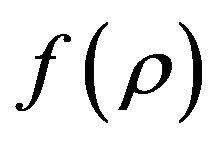 ,
,  also has two positive roots
also has two positive roots 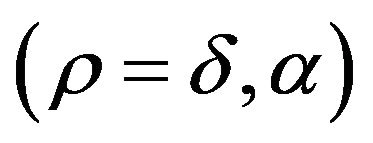 and two complex-conjugated roots
and two complex-conjugated roots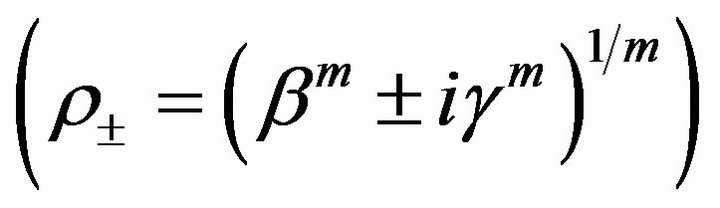 .
.
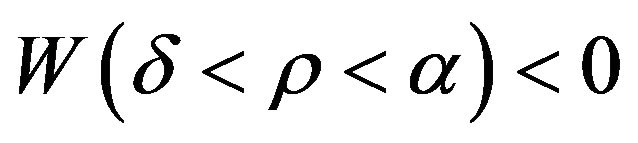 . This is the reason we would name it
. This is the reason we would name it 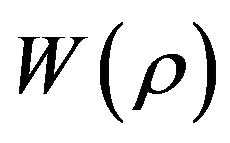 instead of
instead of 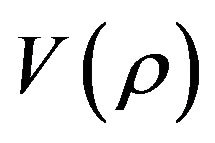 or
or  in this paper.
in this paper.
Without specifying 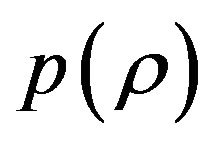 and solving the Fridmann Equation (4.2), we can already illustrate the main features of this cosmological model. The big-bang starts at (Point A)
and solving the Fridmann Equation (4.2), we can already illustrate the main features of this cosmological model. The big-bang starts at (Point A) 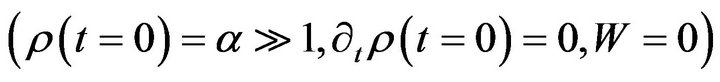 as shown Figure 2. The top of the middle hump (Point B) is near
as shown Figure 2. The top of the middle hump (Point B) is near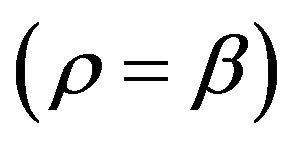 , the early cosmic inflation happened roughly from
, the early cosmic inflation happened roughly from 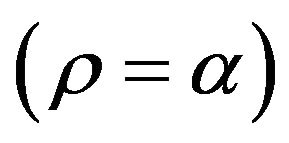 (Point A) to
(Point A) to 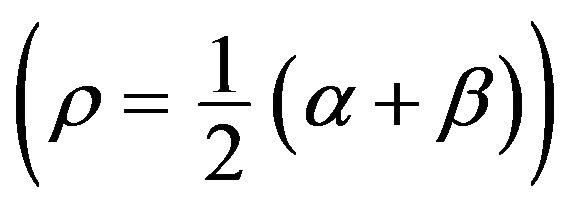 (Point B). The universe expansion described by the original FLWR model is roughly between
(Point B). The universe expansion described by the original FLWR model is roughly between 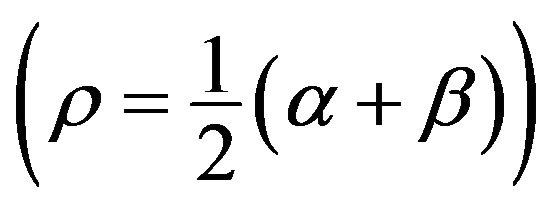 (Point B) to
(Point B) to 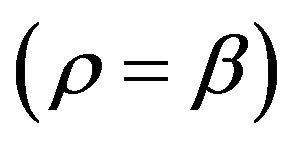 (Point C). We are currently undergoing an accelerated expansion probably in the range from
(Point C). We are currently undergoing an accelerated expansion probably in the range from 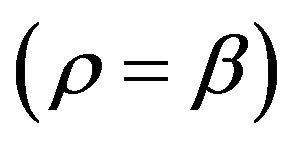 (Point C) to
(Point C) to  (Point D). The universe will stop expansion at
(Point D). The universe will stop expansion at 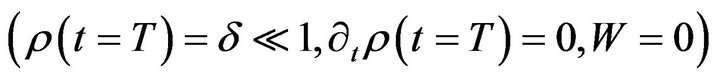 (Point E) and turn itself around and start the big crunch and go all the way back to end the big crunch at
(Point E) and turn itself around and start the big crunch and go all the way back to end the big crunch at
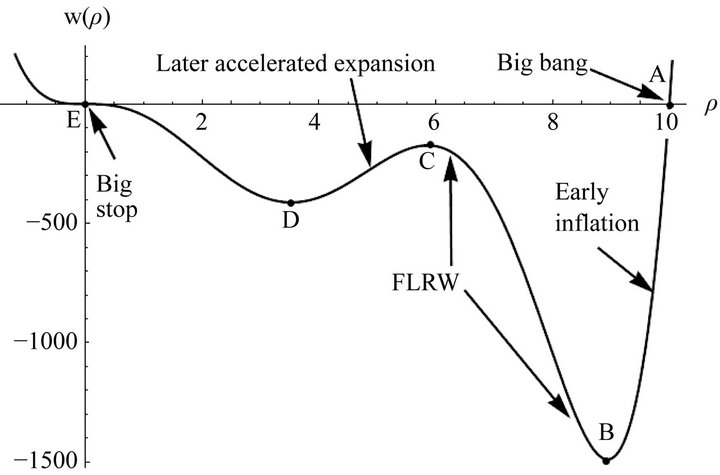
Figure 2. History of cosmology for a cyclic universe model: From big band to cosmic early inflation to cosmic late accelerated expansion to big stop. Numerical constants are l = m = n = 1, p = wρ, w = 0, α = 10, β = 6, γ = 1, λ = 10−3. The points A, B, C, D, and E are defined in the main text below.
 (Point A). It then starts a new big bang—big crunch process. So this cosmological model is a cyclic universe model. This model also covers the eras (like bouncing at both ends which are) beyond the early cosmic inflation and late cosmic accelerated expansion.
(Point A). It then starts a new big bang—big crunch process. So this cosmological model is a cyclic universe model. This model also covers the eras (like bouncing at both ends which are) beyond the early cosmic inflation and late cosmic accelerated expansion.
Our cyclic universe model with W-shaped potential well do incorporate the cosmic early inflation era as well as the cosmic late accelerated expansion, thanks to two downhill slopes (Point A to Point B and Point C to Point D in Figure 2). This cyclic universe model is thus different from those in [61-63] where there is only one downhill slope because their potential 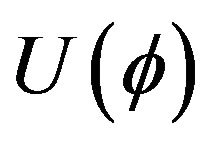 is a Ushaped.
is a Ushaped.
We now define 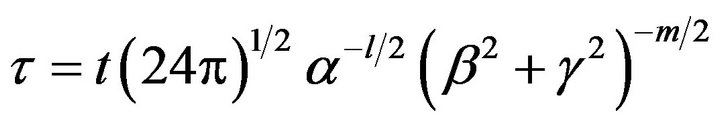 and also consider
and also consider 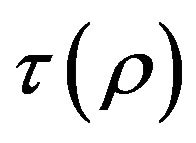 as a function of
as a function of 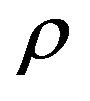 instead of
instead of 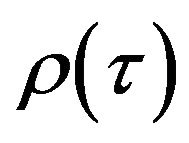 as a function of
as a function of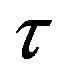 . The solution to (4.2) and (4.3) then becomes,
. The solution to (4.2) and (4.3) then becomes,
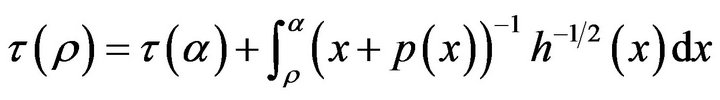 (4.4)
(4.4)
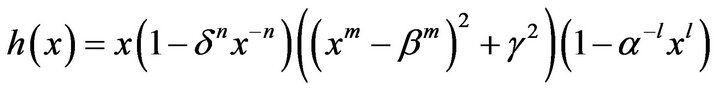 (4.5)
(4.5)
The time, 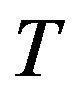 , it takes to go from big-band at Point A to big-stop at Point E is given by:
, it takes to go from big-band at Point A to big-stop at Point E is given by:
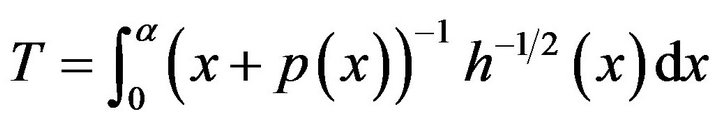 (4.6)
(4.6)
We now assume 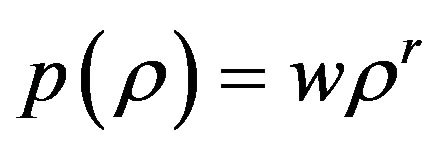
 . We emphasize that if
. We emphasize that if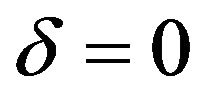 , then the dominant part of integrand in (4.6) near
, then the dominant part of integrand in (4.6) near 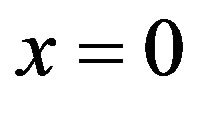 becomes
becomes  so (4.6) diverges. Thus we need a positive but tiny
so (4.6) diverges. Thus we need a positive but tiny  (no matter how tiny it is, e.g.,
(no matter how tiny it is, e.g.,  in metric unit) to obtain a finite
in metric unit) to obtain a finite 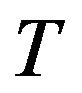 and thus a cyclic universe model. When
and thus a cyclic universe model. When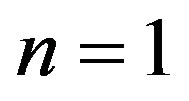 , we can relate
, we can relate  to the cosmology constant
to the cosmology constant  via
via  (we recovered the unit of gravitational constant
(we recovered the unit of gravitational constant 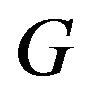 and speed of light
and speed of light  here). Hence we need a negative but tiny cosmology constant
here). Hence we need a negative but tiny cosmology constant  to make
to make 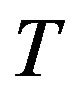 finite and the cosmology model cyclic.
finite and the cosmology model cyclic.
In Appendix B, we show that when , (4.4) and (4.5) can be expressed as a linear combination of Elliptical integrals of first kind, third kind, as well as an elementary function
, (4.4) and (4.5) can be expressed as a linear combination of Elliptical integrals of first kind, third kind, as well as an elementary function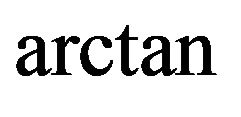 . The optimal values for 7-parameters
. The optimal values for 7-parameters 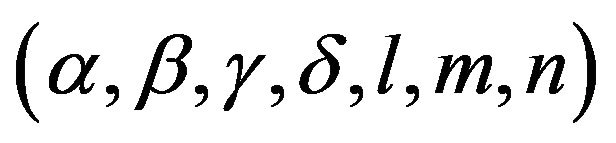 of the M-shaped
of the M-shaped 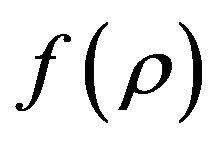 should be determined by the fitting of this model with observational data. This is beyond our current capability.
should be determined by the fitting of this model with observational data. This is beyond our current capability.
We now go through our check list to see if we have achieved 5 goals mentioned in the abstract using the cyclic universe model with 7-parameter M-shaped 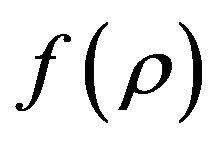 of (4.1).
of (4.1).
Goal number (1): producing a non-singular cosmological model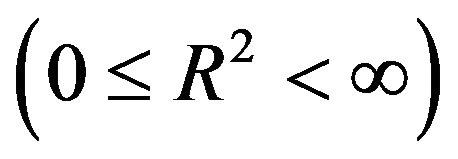 ,
, . From (4.2) and (4.3), because
. From (4.2) and (4.3), because  we get
we get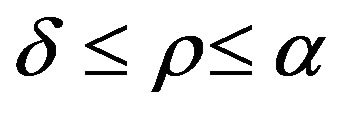 .
.
Thus 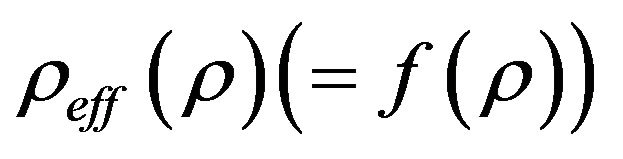 is bounded from above and below in this range. And WEC is also satisfied. To show
is bounded from above and below in this range. And WEC is also satisfied. To show 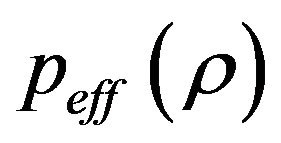 is bounded; it is suffice to show that
is bounded; it is suffice to show that 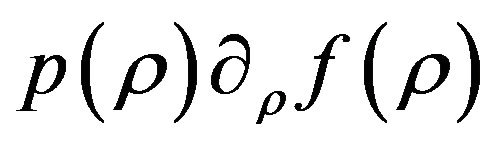 is bounded. Substituting p = wρr
is bounded. Substituting p = wρr  and (4.1) into
and (4.1) into , It is straight forward to show that
, It is straight forward to show that  is bounded from above and below in the range
is bounded from above and below in the range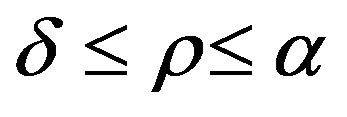 . Thus via (2.15) and (2.16) we have achieved goal number (1).
. Thus via (2.15) and (2.16) we have achieved goal number (1).
Goal number (2): explaining the cosmic early inflation and late acceleration in a unified fashion. See Figure 2 and the description right after.
Goal number (3): passing the solar system tests. As long as 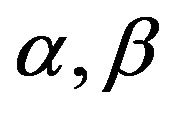 large enough and
large enough and 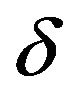 is tiny enough, we have
is tiny enough, we have 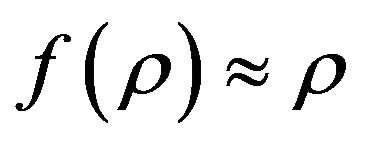 and we can pass the solar system tests.
and we can pass the solar system tests.
Goal number (4): unifying the dark matter with dark energy. See Figures 1 and 2 and the description in between.
Goal number (5): unifying the regular matter/energy with dark matter/energy in a seamless fashion. Unlike other dark energy (+ regular matter) theory, there is only one material (one energy density 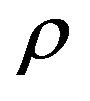 and one pressure
and one pressure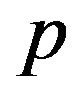 ) in our
) in our  theory based cyclic universe model. This single material plays both the role of regular matter/energy when needed in the FLRW era and the role of dark matter/energy when needed in other eras in the cyclic universe model. See Figures 1 and 2 and the description in between for details. It is in this sense that we meant we achieve goal number (5). Maybe regular matter/energy (like perfect fluid) and dark matter/energy are just two aspects of the same material. In other words, we have shown the bright side of the dark matter/energy or the dark side of the regular matter/energy (perfect fluid).
theory based cyclic universe model. This single material plays both the role of regular matter/energy when needed in the FLRW era and the role of dark matter/energy when needed in other eras in the cyclic universe model. See Figures 1 and 2 and the description in between for details. It is in this sense that we meant we achieve goal number (5). Maybe regular matter/energy (like perfect fluid) and dark matter/energy are just two aspects of the same material. In other words, we have shown the bright side of the dark matter/energy or the dark side of the regular matter/energy (perfect fluid).
The concept of cyclic universe model itself is not new. For example, any cosmology model in general and cyclic cosmology model in particular, could be reconstructed in 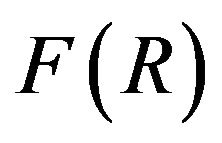 theories of gravity. The corresponding technique is described in [64,65]. Realistic
theories of gravity. The corresponding technique is described in [64,65]. Realistic 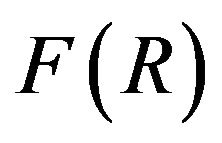 gravity cosmology model has recently been proposed in [66]. This model can also achieve goals (1) through (4) mentioned above.
gravity cosmology model has recently been proposed in [66]. This model can also achieve goals (1) through (4) mentioned above.
One unique thing about the 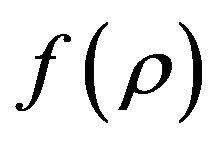 theory based cyclic model of (4.2) and (4.3) is that, the mathematics is very much simplified. Without specifying
theory based cyclic model of (4.2) and (4.3) is that, the mathematics is very much simplified. Without specifying 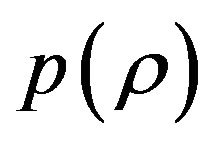 and solving the Fridmann equations (4.2) and (4.3), we can already illustrate the main features of this cosmological model.
and solving the Fridmann equations (4.2) and (4.3), we can already illustrate the main features of this cosmological model.
5. The Relation between f(ρ) Theory and F(R) Theories, Chaplygin Gas, NED, etc.
5.1. The Relation between f(ρ) Theory and F(R) Theories
In 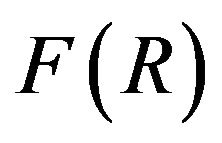 theory [67], the Einstein’s equations can be cast into a form similar to (2.1) and (2.2):
theory [67], the Einstein’s equations can be cast into a form similar to (2.1) and (2.2):
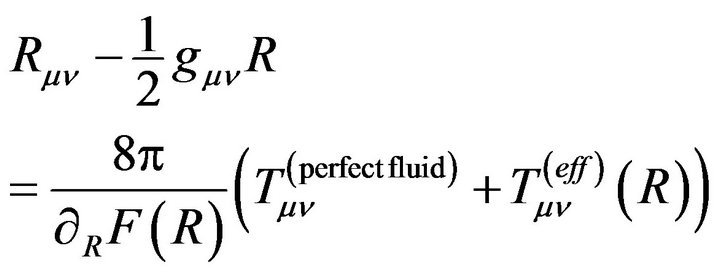 (5.1)
(5.1)
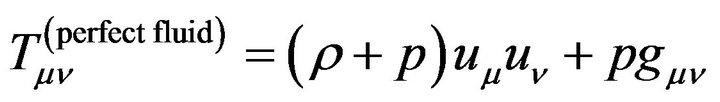 (5.2)
(5.2)
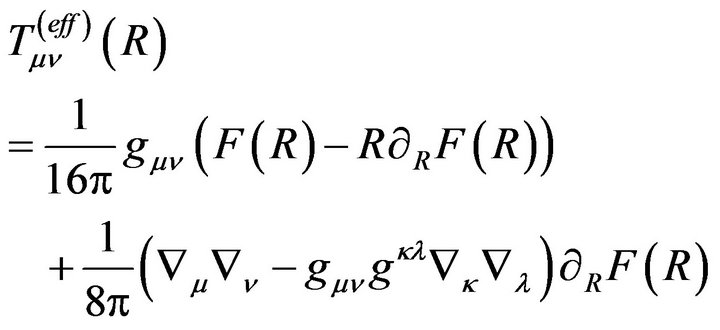 (5.3)
(5.3)
If we equate (2.1) to (5.1), then we obtain a formal relation between the energy-momentum tensor of  theory in (2.2) and that of
theory in (2.2) and that of  theories in (5.2):
theories in (5.2):
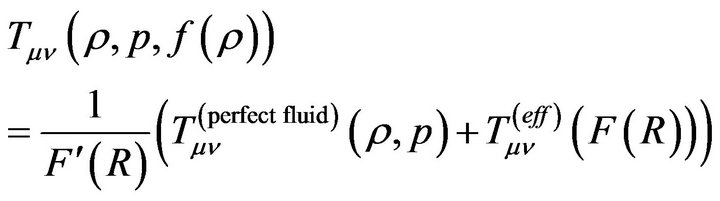 (5.4)
(5.4)
It remains to be seen if anything significant can be deduced from (5.4). We would look at the relation between  theory and
theory and 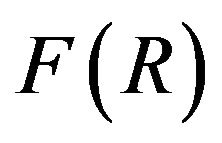 theories (and Palatini
theories (and Palatini 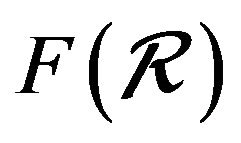 theory) from a different angle. We can start by looking at their modified actions against the original Hilbert-Einstein action. Let us first compare the corresponding Lagrangians with the corresponding trace equations (with units
theory) from a different angle. We can start by looking at their modified actions against the original Hilbert-Einstein action. Let us first compare the corresponding Lagrangians with the corresponding trace equations (with units 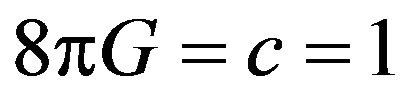 in this section) for dust (
in this section) for dust (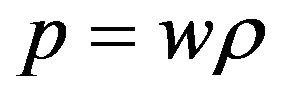 ,
,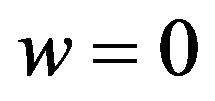 ) in Table 3 below.
) in Table 3 below.
We start with (5.5b), the trace of the original Einstein’s equation for dust,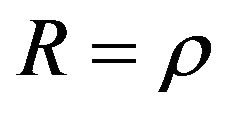 . We observe that as long as
. We observe that as long as 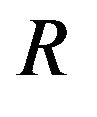 and
and 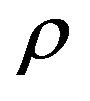 go to infinity at the same speed, the trace equation
go to infinity at the same speed, the trace equation 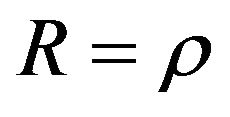 can still be satisfied. We think that this is the cause of the intrinsic singularity.
can still be satisfied. We think that this is the cause of the intrinsic singularity.
1) One way to break up this running away (to infinity) situation is to replace 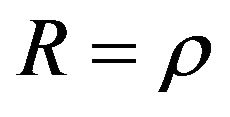 with
with
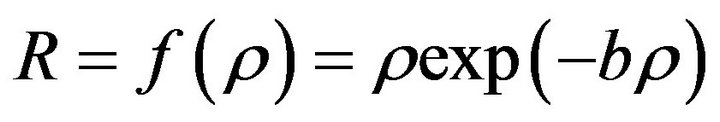 with
with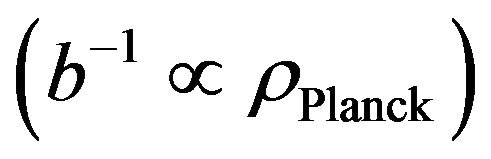 . From this equation, we can immediately obtain
. From this equation, we can immediately obtain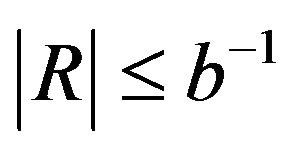 . Consequently ρ is, via
. Consequently ρ is, via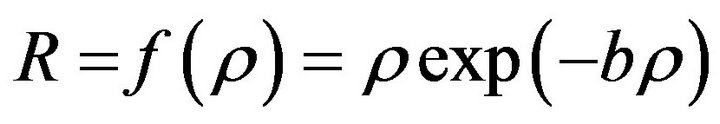 , also bounded from above and below. If we replace
, also bounded from above and below. If we replace 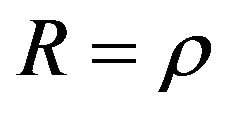 with
with
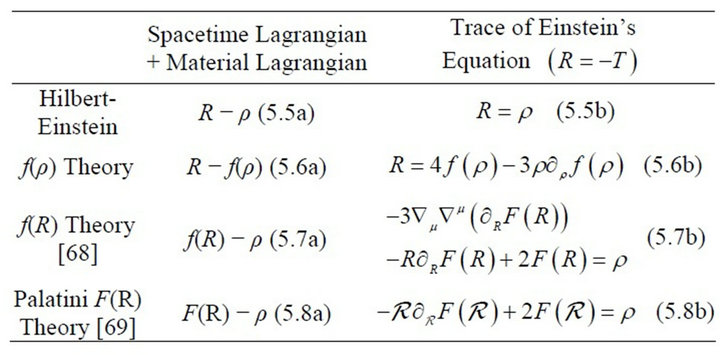
Table 3. Comparison of the Lagrangians and trace equations for f(ρ) theory, F(R) theories, and Palatini 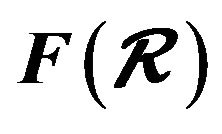 theory.
theory.
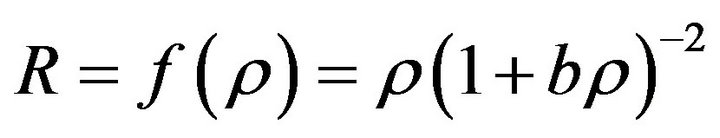 , we can obtain
, we can obtain 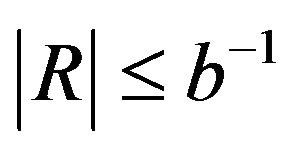 as well. Of course, we can not just replace
as well. Of course, we can not just replace  with
with 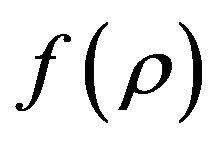 in the trace of Einstein’s equations. We have to do it at the Lagrangian level. Thus we are led to replace Lagrangian of (5.5a) in Einstein’s theory,
in the trace of Einstein’s equations. We have to do it at the Lagrangian level. Thus we are led to replace Lagrangian of (5.5a) in Einstein’s theory,  , with that of (5.6a) in
, with that of (5.6a) in 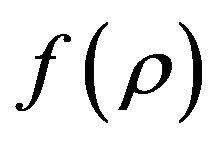 theory,
theory,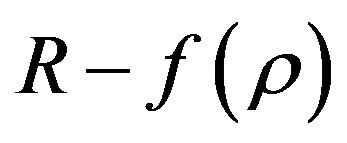 . The resulting trace equation is (5.6b),
. The resulting trace equation is (5.6b), . Assuming
. Assuming
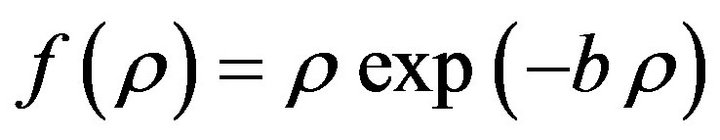 or
or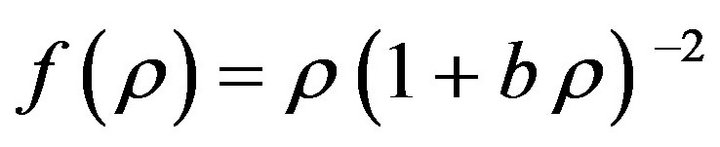 , it is straight forward to show from (5.6b) that
, it is straight forward to show from (5.6b) that 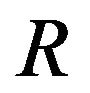 is bounded from above and below and so is
is bounded from above and below and so is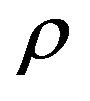 .
.
2) Another way to break up this running away (to infinity) situation is to replace Lagrangian of (5.5a) in Einstein’s theory,  , with that of (5.8a) in Palatini
, with that of (5.8a) in Palatini 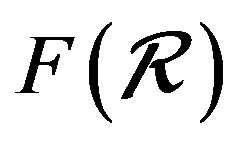 theory,
theory,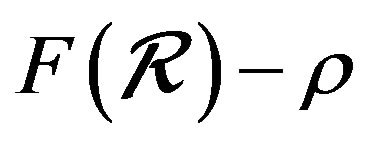 . The resulting trace equation is (5.8b),
. The resulting trace equation is (5.8b), . Assuming
. Assuming 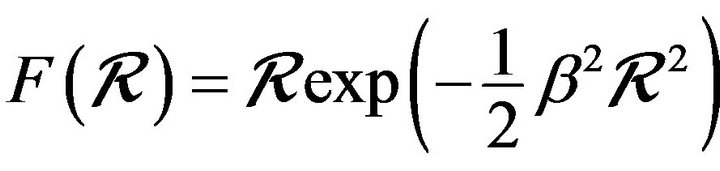 with
with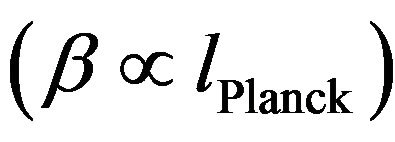 , it is straight forward to show from (5.8b) that
, it is straight forward to show from (5.8b) that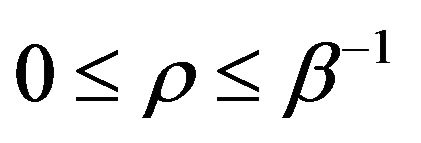 , i.e., bounded from above and below. Consequently
, i.e., bounded from above and below. Consequently  is, via
is, via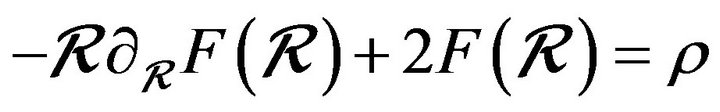 , also bounded from above and below. If we select
, also bounded from above and below. If we select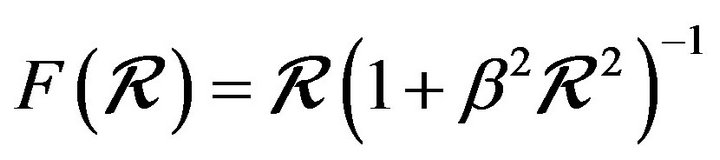 , we can show that
, we can show that 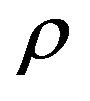 and
and  are bounded from above and below as well.
are bounded from above and below as well.
Because of the higher order derivative term in (5.7b), a similar analysis is probably doable but less straight forward.
5.2. The Relation between f(ρ) Theory and Nonlinear Electro Dynamics (NED)
We are intrigued by the nonsingular exact black hole solutions with Nonlinear Electro Dynamics (NED) [70- 72]. As a matter of factor, the inspiration to both the original authors of [40,70] can be traced back to the famous Born-Infeld theory [73].
The Lagrangian 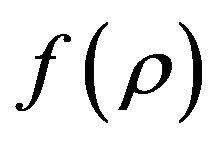 and the NED Lagrangian
and the NED Lagrangian 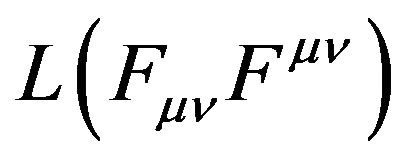 can be used as material Lagrangian in the Hilbert-Einstein like action
can be used as material Lagrangian in the Hilbert-Einstein like action 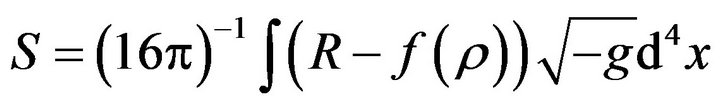 and
and 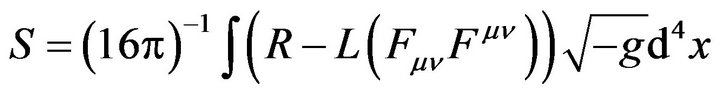 . One major difference between these two theories is that the energymomentum tensor generated from
. One major difference between these two theories is that the energymomentum tensor generated from  is traceless while as the energy-momentum tensor generated from
is traceless while as the energy-momentum tensor generated from 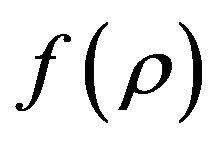 is not.
is not.
Recall that in Born-Infeld theory the action is given by  with
with 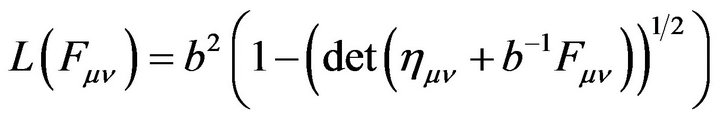 . The square root function is used to break up the running away to infinity situation (at the center of a charged particle). Thus we would guess that
. The square root function is used to break up the running away to infinity situation (at the center of a charged particle). Thus we would guess that 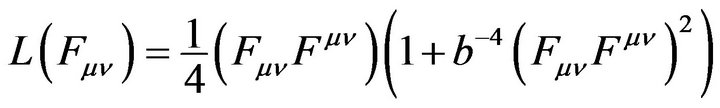 might do a similar job in breaking up the running away situation.
might do a similar job in breaking up the running away situation.
5.3. The Relation between f(ρ) Theory and Chaplygin Gas
The Chaplygin gas is an exotic perfect fluid (a kind of dark energy) with equation of state [74-76]:
 (5.9)
(5.9)
It is used to explain the cosmic late accelerated expansion. Notice that the equation of state behaves like:
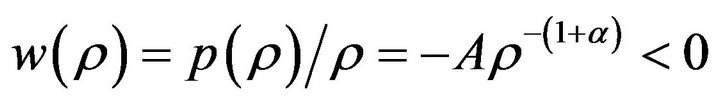 (5.10)
(5.10)
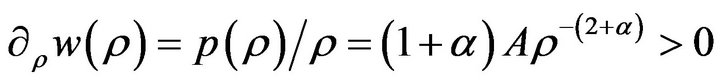 (5.10)
(5.10)
From Figure 1, we deduce that  near
near 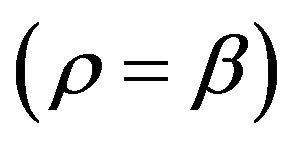 (Point C) behaves like a Chaplygin gas.
(Point C) behaves like a Chaplygin gas.
The problem with Chaplygin gas of (5.9) is that it diverges at  in the range
in the range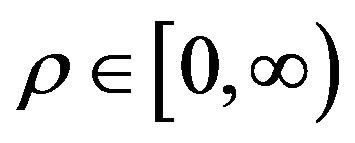 . The
. The 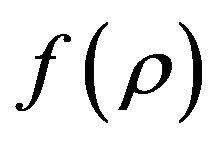 based cyclic universe model of (4.1) does not have this kind of divergence problem.
based cyclic universe model of (4.1) does not have this kind of divergence problem.
6. Conclusions
We considered FLRW cosmological model for perfect fluid (with  as the energy density) in the frame work of the
as the energy density) in the frame work of the  modified theory of gravity. With an M-shaped function
modified theory of gravity. With an M-shaped function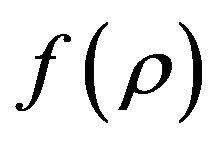 , we achieved, like in
, we achieved, like in 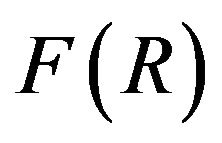 modified theory of gravity, the following 4 specific goals, 1) producing a non-singular cosmological model
modified theory of gravity, the following 4 specific goals, 1) producing a non-singular cosmological model 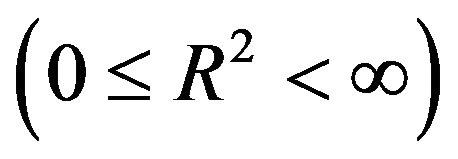 ,
,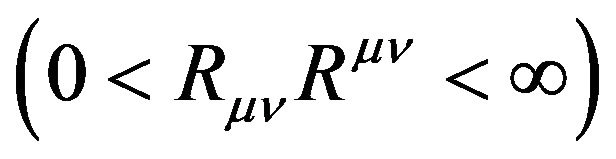 ; 2) explaining the cosmic early inflation and late acceleration in a unified fashion; 3) passing the solar system tests; 4) unifying the dark matter with dark energy.
; 2) explaining the cosmic early inflation and late acceleration in a unified fashion; 3) passing the solar system tests; 4) unifying the dark matter with dark energy.
In addition, we also achieve goal number 5) unifying the regular matter/energy with dark matter/energy in a seamless fashion in 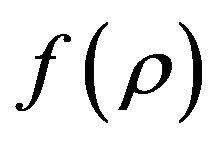 theory and goal number 6) simplifying the Einstein’s equations (in comparison to
theory and goal number 6) simplifying the Einstein’s equations (in comparison to 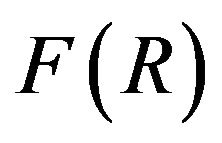 theory).
theory).
We would like to emphasize that in our 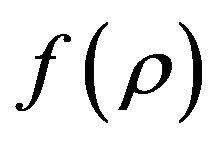 theory based cyclic universe model, the single material (energy density
theory based cyclic universe model, the single material (energy density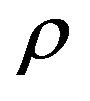 ) plays both the role of regular matter/energy when needed in the FLRW era and the role of dark matter/energy when needed in other eras in the cyclic universe. Thus we conjecture that the regular matter/energy (like perfect fluid) and dark matter/energy might just be two aspects of the same material. In other words, we have probably shown the bright side of the dark matter/ energy or the dark side of the regular matter/energy (perfect fluid).
) plays both the role of regular matter/energy when needed in the FLRW era and the role of dark matter/energy when needed in other eras in the cyclic universe. Thus we conjecture that the regular matter/energy (like perfect fluid) and dark matter/energy might just be two aspects of the same material. In other words, we have probably shown the bright side of the dark matter/ energy or the dark side of the regular matter/energy (perfect fluid).
The apparent unification of the regular matter/energy with dark matter/energy in a seamless fashion and simplification of Einstein’s equations (Friedman’s equations) are probably the two interesting benefits of 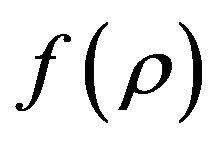 theory.
theory.
7. Acknowledgements
During the development of this work, we have greatly benefited from the stimulating discussions with Dr. Jie Qing, Dr. J. E. Hearst, Dr. W. M. McClain, Dr. R. A. Harris, Dr. R. P. Lin, Dr. Lei Xu, Dr. Zixiang Zhou, Dr. Ying-Qiu Gu, Dr. Ru-keng Su, Dr. Bin Wang, Dr. Jinglan Sun, and Dr. W. Liu.
We would also appreciate the feedback from Dr. S. D. Odintsov, Dr. S. Nojiri, Dr. E. Elizalde, Dr. T. P. Sotiriou, Dr. V. Faraoni, and Dr. K. Lake.
REFERENCES
- A. A. Starobinsky, Physics Letters B, Vol. 91, 1980, pp. 99-102. doi:10.1016/0370-2693(80)90670-X
- D. Kazanas, The Astrophysical Journal, Vol. 241, 1980, pp. L59-L63. doi:10.1086/183361
- A. H. Guth, Physical Review D, Vol. 23, 1981, pp. 347- 356. doi:10.1103/PhysRevD.23.347
- K. Sato, Monthly Notices of the Royal Astronomical Society, Vol. 195, 1981, pp. 467-479.
- G. F. Smoot, C. L. Bennett, A. Kogut, E. L. Wright, J. Aymon, N. W. Boggess, E. S. Cheng, G. de Amici, S. Gulkis, M. G. Hauser, G. Hinshaw, P. D. Jackson, M. Janssen, E. Kaita, T. Kelsall, P. Keegstra, C. Lineweaver, K. Loewenstein, P. Lubin, J. Mather, S. S. Meyer, S. H. Moseley, T. Murdock, L. Rokke, R. F. Silverberg, L. Tenorio, R. Weiss and D. T. Wilkinson, The Astrophysical Journal, Vol. 396, 1992, pp. L1-L5. doi:10.1086/186504
- D. H. Lyth and A. Riotto, Physics Reports, Vol. 314, 1999, pp. 1-146. doi:10.1016/S0370-1573(98)00128-8.
- A. R. Liddle and D. H. Lyth, “Cosmological Inflation and Large-Scale Structure,” Cambridge University Press, Cambridge, New York, 2000. doi:10.1017/CBO9781139175180
- B. A. Bassett, S. Tsujikawa and D. Wands, Reviews of Modern Physics, Vol. 78, 2006, pp. 537-589. doi:10.1103/RevModPhys.78.537
- D. Huterer and M. S. Turner, Physical Review D, Vol. 60, 1999, Article ID: 081301. doi:10.1103/PhysRevD.60.081301
- S. Perlmutter, G. Aldering, G. Goldhaber, R. A. Knop, P. Nugent, P. G. Castro, S. Deustua, S. Fabbro, A. Goobar, D. E. Groom, I. M. Hook, A. G. Kim, M. Y. Kim, J. C. Lee, N. J. Nunes, R. Pain, C. R. Pennypacker, R. Quimby, C. Lidman, R. S. Ellis, M. Irwin, R. G. McMahon, P. Ruiz-Lapuente, N. Walton, B. Schaefer, B. J. Boyle, A. V. Filippenko, T. Matheson, A. S. Fruchter, N. Panagia, H. J. M. Newberg and W. J. Couch, The Astrophysical Journal, Vol. 517, 1999, pp. 565-586. doi:10.1086/307221
- A. G. Riess, A. V. Filippenko, P. Challis, A. Clocchiatti, A. Diercks, P. M. Garnavich, R. L. Gilliland, C. J. Hogan, S. Jha, R. P. Kirshner, B. Leibundgut, M. M. Phillips, D. Reiss, B. P. Schmidt, R. A. Schommer, R. C. Smith, J. Spyromilio, C. Stubbs, N. B. Suntzeff and J. Tonry, The Astrophysical Journal, Vol. 116, 1998, pp. 1009-1038. doi:10.1086/300499
- A. G. Riess, R. P. Kirshner, B. P. Schmidt, S. Jha, P. Challis, P. M. Garnavich, A. A. Esin, C. Carpenter, R. Grashius, R. E. Schild, P. L. Berlind, J. P. Huchra, C. F. Prosser, E. E. Falco, P. J. Benson, C. Bricẽno, W. R. Brown, N. Caldwell, I. P. Dell’Antonio, A. V. Filippenko, A. A. Goodman, N. A. Grogin, T. Groner, J. P. Hughes, P. J. Green, R. A. Jansen, J. T. Kleyna, J. X. Luu, L. M. Macri, B. A. McLeod, K. K. McLeod, B. R. McNamara, B. McLean, A. A. E. Milone, J. J. Mohr, D. Moraru, C. Peng, J. Peters, A. H. Prestwich, K. Z. Stanek, A. Szentgyorgyi and P. Zhao, The Astrophysical Journal, Vol. 117, 1999, pp. 707-724. doi:10.1086/300738
- M. Tegmark, et al. (SDSS Collaboration), Physical Review D, Vol. 69, 2004, Article ID: 103501. doi:10.1103/PhysRevD.69.103501
- M. Tegmark, et al. (SDSS Collaboration), Physical Review D, Vol. 74, 2006, Article ID: 123507. doi:10.1103/PhysRevD.74.123507
- D. J. Eisenstein, et al. (SDSS Collaboration), The Astrophysical Journal, Vol. 633, 2005, pp. 560-574. doi:10.1086/466512
- W. J. Percival, S. Cole, D. J. Eisenstein, R. C. Nichol, J. A. Peacock, A. C. Pope and A. S. Szalay, Monthly Notices of the Royal Astronomical Society, Vol. 381, 2007, pp. 1053-1066. doi:10.1111/j.1365-2966.2007.12268.x
- D. N. Spergel, et al. (WMAP Collaboration), The Astrophysical Journal Supplement Series, Vol. 148, 2003, pp. 175-194. doi:10.1086/377226
- D. N. Spergel, et al. (WMAP Collaboration), The Astrophysical Journal Supplement Series, Vol. 170, 2007, pp. 377-408. doi:10.1086/513700
- E. Komatsu, et al. (WMAP Collaboration), The Astrophysical Journal Supplement Series, Vol. 180, 2009, pp. 330-376. doi:10.1088/0067-0049/180/2/330
- V. Sahni, Classical and Quantum Gravity, Vol. 19, 2002, pp. 3435-3448.
- S. E. Deustua, et al., “Cosmological Parameters, Dark Energy and Large Scale Structure,” APS/DPF/DPB Summer Study on the Future of Particle Physics (Snowmass 2001) ed N Graf, eConf C010630 P342, arXiv:astro-ph/0207293v1.
- M. S. Turner and D. Huterer, Journal of the Physical Society of Japan, Vol. 76, 2007, Article ID: 111015. doi:10.1143/JPSJ.76.111015
- T. Padmanabhan, Current Science, Vol. 88, 2005, p. 1057.
- M. Ishak, Foundations of Physics, Vol. 37, 2007, pp. 1470- 1498. doi:10.1007/s10701-007-9175-z
- M. Szydlowski, JCAP, Vol. 0709, 2007, p. 007.
- E. V. Linder, Journal of Physics A, Vol. 40, 2007, p. 6697. doi:10.1088/1751-8113/40/25/S14
- C. Clarkson, M. Cortes and B. Bassett, JCAP, Vol. 0708, 2007, p. 011.
- Y.-Q. Gu, “Dynamic Behavior and Topological Structure of the Universe with Dark Energy,” arXiv:0709.2414v5 [gr-qc].
- V. Sahni and A. A. Starobinsky, International Journal of Modern Physics D, Vol. 9, 2000, pp. 373-443. doi:10.1142/S0218271800000542
- S. M. Carroll, Living Reviews in Relativity, Vol. 4, 2001, 1-56. http://relativity.livingreviews.org/Articles/lrr-2001-1
- T. Padmanabhan, Physics Reports, Vol. 380, 2003, pp. 235-320. doi:10.1016/S0370-1573(03)00120-0
- P. J. E. Peebles and B. Ratra, Reviews of Modern Physics, Vol. 75, 2003, pp. 559-606. doi:10.1103/RevModPhys.75.559
- E. J. Copeland, M. Sami and S. Tsujikawa, International Journal of Modern Physics D, Vol. 15, 2006, pp. 1753- 1935. doi:10.1142/S021827180600942X
- L. Amendola and S. Tsujikawa, “Dark Energy: Theory and Observations,” Cambridge University Press, Cambridge, New York, 2010. doi:10.1017/CBO9780511750823
- T. P. Sotiriou and V. Faraoni, Reviews of Modern Physics, Vol. 82, 2010, pp. 451-497. doi:10.1103/RevModPhys.82.451
- A. De Felice and S. Shinji Tsujikawa, Living Reviews in Relativity, Vol. 13, 2010, p. 3.
- S. Nojiri and S. D. Odintsov, Physics Reports, Vol. 505, 2011, pp. 59-144.
- S. Nojiri and S. D. Odintsov, Academic Journal, Vol. 1241, 2010, p. 1094.
- S. Capozziello, M. De Laurentis, S. Nojiri and S. D. Odintsov, Physical Review D, Vol. 79, 2009, Article ID: 124007. doi:10.1103/PhysRevD.79.124007
- V. N. Tunyak, Russian Physics Journal, Vol. 21, 1978, pp. 1221-1223.
- J. R. Ray and L. L. Smalley, Physical Review D, Vol. 26, 1982, pp. 2615-2618. doi:10.1103/PhysRevD.26.2615
- M. Planck, Annalen der Physik, Vol. 4, 1901, pp. 553-563. doi:10.1002/andp.19013090310
- L. D. Landau and E. M. Lifshitz, “Statistical Mechanics,” John Wiley, New York, 1972.
- S. Weinberg, “Gravitation and Cosmology: Principles and Applications of the General Theory of Relativity,” John Wiley, New York, 1972.
- R. J. Nemiroff and B. Patla, American Journal of Physics, Vol. 76, 2008, pp. 265-276. doi:10.1119/1.2830536
- J. M. Heinzle and P. Sandin, Communications in Mathematical Physics, Vol. 313, 2012, pp. 385-403.
- S. Nojiri and S. D. Odintsov, International Journal of Geometric Methods in Modern Physics, Vol. 4, 2007, pp. 115-146.
- S. Capozziello, V. F. Cardone, E. Elizalde, S. Nojiri and S. D. Odintsov, Physical Review D, Vol. 73, 2006, Article ID: 043512. doi:10.1103/PhysRevD.73.043512
- S. Nojiri and S. D. Odintsov, Physical Review D, Vol. 72, 2005, Article ID: 023003. doi:10.1103/PhysRevD.72.023003
- K. Lake, Physical Review D, Vol. 74, 2006, Article ID: 123505. doi:10.1103/PhysRevD.74.123505
- M. Szydlowski, A. Kurek and A. Krawiec, Physics Letters B, Vol. 642, 2006, pp. 171-178. doi:10.1016/j.physletb.2006.09.052
- Y. Q. Gu, International Journal of Modern Physics A, Vol. 22, 2007, pp. 4667-4678. doi:10.1142/S0217751X07037925
- Y. Q. Gu, “Accelerating Expansion of the Universe with Nonlinear Spinors,” 2006.
- L. Randall and R. Sundrum, Physical Review Letters, Vol. 83, 1999, pp. 3370-3373. doi:10.1103/PhysRevLett.83.3370
- L. Randall and R. Sundrum, Physical Review Letters, Vol. 83, 1999, pp. 4690-4693. doi:10.1103/PhysRevLett.83.4690
- Y. V. Shtanov, Reports on Progress in Physics, Vol. 67, 2004, pp. 2183-2232.
- Y. Shtanov and V. Sahni, Physics Letters B, Vol. 557, 2003, pp. 1-6. doi:10.1016/S0370-2693(03)00179-5
- K. Freese and M. Lewis, Physics Letters B, Vol. 540, 2002, pp. 1-8. doi:10.1016/S0370-2693(02)02122-6
- J. R. Oppenheimer and H. Snyder, Physical Review, Vol. 56, 1939, pp. 455-459. doi:10.1103/PhysRev.56.455
- [61] S. Weinberg, “Gravitation and Cosmology: Principles and Applications of the General Theory of Relativity,” John Wiley, New York, 1972.
- [62] P. J. Steinhardt and N. Turok, Science, Vol. 296, 2002, pp. 1436-1439. doi:10.1126/science.1070462
- [63] P. J. Steinhardt and N. Turok, New Astronomy Reviews, Vol. 49, 2005, pp. 43-57. doi:10.1016/j.newar.2005.01.003
- [64] J. Khoury, P. J. Steinhardt and N. Turok, Physical Review Letters, Vol. 92, 2004, Article ID: 031302. doi:10.1103/PhysRevLett.92.031302
- [65] S. Nojiri and S. D. Odintsov, Physical Review D, Vol. 74, 2006, Article ID: 086005. doi:10.1103/PhysRevD.74.086005
- [66] S. Nojiri and S. D. Odintsov, Journal of Physics: Conference Series, Vol. 66, 2007, Article ID: 012005.
- [67] E. Elizalde, S. Nojiri, S. D. Odintsov, L. Sebastiani and S. Zerbini, Physical Review D, Vol. 83, 2011, Article ID: 086006.
- [68] T. P. Sotiriou and V. Faraoni, Reviews of Modern Physics, Vol. 82, 2010, pp. 451-497. Eq.(10)
- [69] T. P. Sotiriou and V. Faraoni, Reviews of Modern Physics, Vol. 82, 2010, pp. 451-497. Eq.(8)
- [70] T. P. Sotiriou and V. Faraoni, Reviews of Modern Physics, Vol. 82, 2010, pp. 451-497. Eq.(20)
- [71] E. Ayon-Beato and A. Garcia, Physics Letters B, Vol. 464, 1999, pp. 25-29. doi:10.1016/S0370-2693(99)01038-2
- [72] K. A. Bronnikov, Physical Review D, Vol. 63, 2001, Article ID: 044005. doi:10.1103/PhysRevD.63.044005
- [73] J. Matyjasek, Physical Review D, Vol. 70, 2004, Article ID: 047504. doi:10.1103/PhysRevD.70.047504
- [74] M. Born and L. Infeld, Proceedings of the Royal Society of London. Series A, Vol. 144, 1934, pp. 425-451. doi:10.1098/rspa.1934.0059
- [75] M. C. Bento, O. Bertolami and A. A. Sen, Physical Review D, Vol. 66, 2002, Article ID: 043507. doi:10.1103/PhysRevD.66.043507
- [76] A. Y. Kamenshchik, U. Moschella and V. Pasquier, Physics Letters B, Vol. 511, 2001, pp. 265-268. doi:10.1016/S0370-2693(01)00571-8
- [77] M. Chevallier and D. Polarski, International Journal of Modern Physics D, Vol. 10, 2001, pp. 213-224. doi:10.1142/S0218271801000822
- [78] S. W. Hawking and G. F. R. Ellis, “Gravitation and Cosmology: Principles and Applications of the General Theory of Relativity,” John Wiley, New York, 1972.
- [79] Z. X. Wang and D. R. Guo, “Special Functions,” Science Press, Beijing, 1979; (English Translation, World Scientific, Singapore, 1988).
8. Appendices
8.1. Apendex A. Derivation of the Energy-Momentum Tensor from Lagrangian 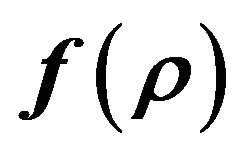
We now follow the standard text book procedure (cf. Hawking and Ellis [77]) to derive the energy-momentum density tensor using the material Lagrangian 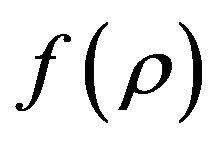 for the ideal fluid.
for the ideal fluid.
We denote  the mass density,
the mass density, 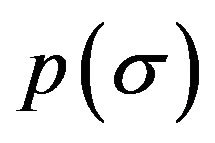 the pressure,
the pressure, 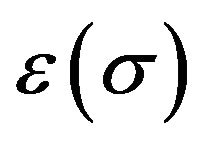 the internal energy density, and
the internal energy density, and 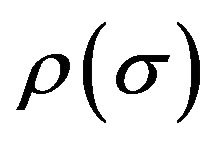 the (total) energy density of the ideal fluid. We remark that in [77] symbol
the (total) energy density of the ideal fluid. We remark that in [77] symbol 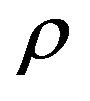 represents mass density (so it is equivalent to our
represents mass density (so it is equivalent to our  here) and symbol
here) and symbol 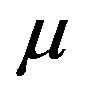 represents (total) energy density (so it is equivalent to our
represents (total) energy density (so it is equivalent to our 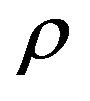 here).
here).
The energy-momentum tensor is defined as:
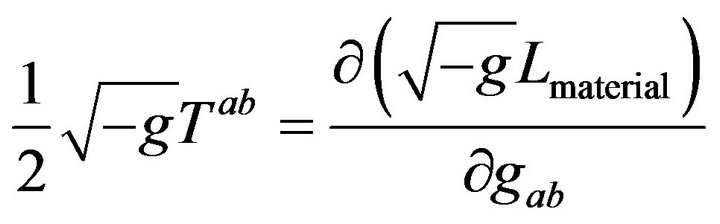 (A.1)
(A.1)
In [77], the material Lagrangian is chosen as:
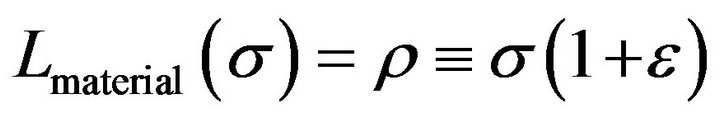 . (A.2)
. (A.2)
Here we choose:
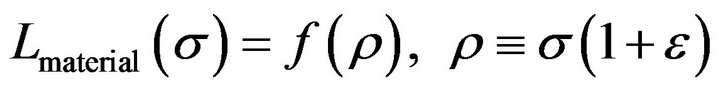 (A.3)
(A.3)
Substitution of (A.3) into (A.1), we have:
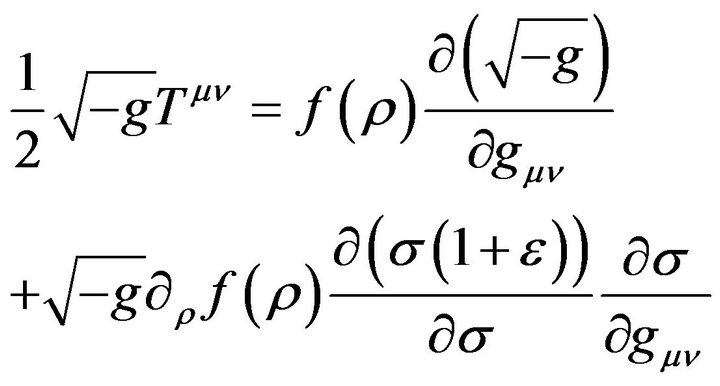 (A.4)
(A.4)
The derivation may be simplified by noting that the conservation of the current 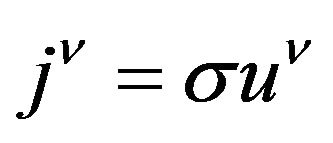 may be expressed as:
may be expressed as:
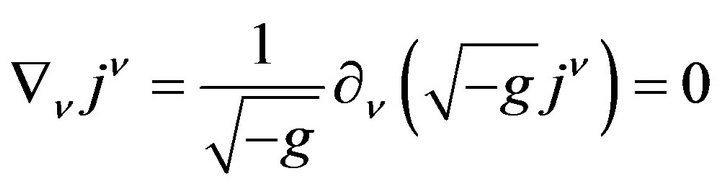 (A.5)
(A.5)
Given the flow lines, the conservation equations determine 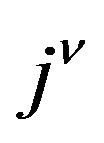 uniquely at each point on a flow line in terms of its initial value at some given points on the same flow line. Therefore
uniquely at each point on a flow line in terms of its initial value at some given points on the same flow line. Therefore 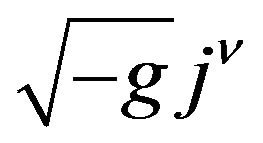 is unchanged when the metric is varied. Since
is unchanged when the metric is varied. Since
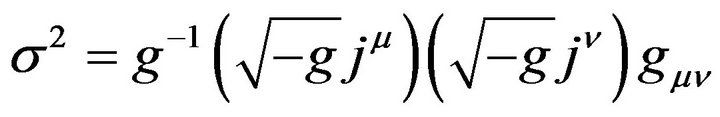 (A.6)
(A.6)
So
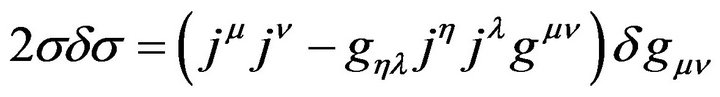 (A.7)
(A.7)
Substitution of 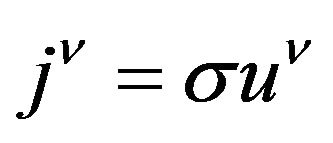 into (A.7) and realizing that
into (A.7) and realizing that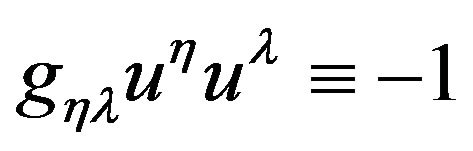 , we obtain:
, we obtain:
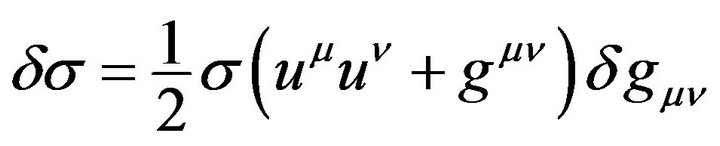 (A.8)
(A.8)
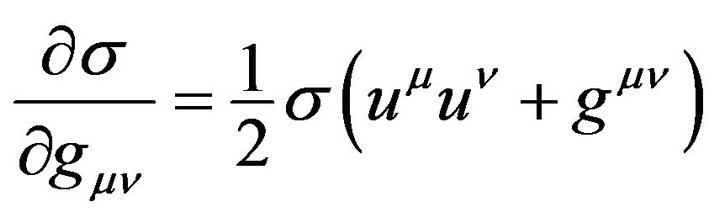 (A.9)
(A.9)
We also have:
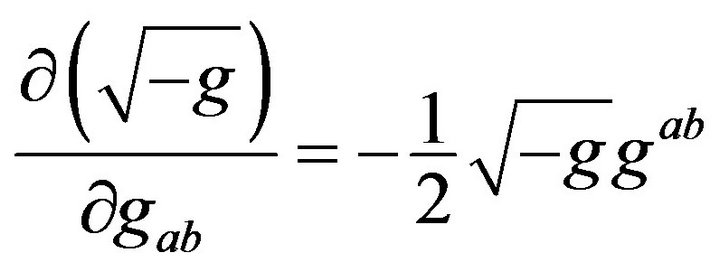 (A.10)
(A.10)
We recall that the combined First law and Second law of thermodynamics in the rest frame is given by:
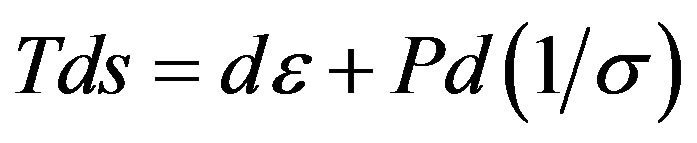 (A.11)
(A.11)
In (A.11), 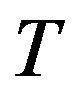 is temperature and
is temperature and 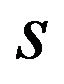 is the entropy. For the isentropic perfect fluid
is the entropy. For the isentropic perfect fluid 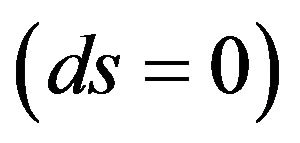 we thus obtain:
we thus obtain:
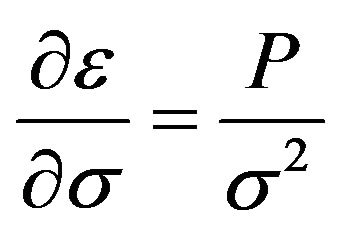 (A.12)
(A.12)
Substitution of (A.9), (A.10), and (A.12) into (A.4), we finally obtain:
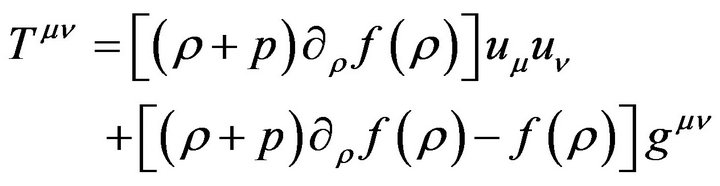 (A.13)
(A.13)
and
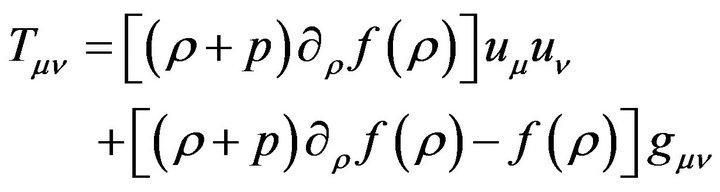 (A.14)
(A.14)
We shall call a perfect fluid isentropic if the pressure 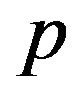 is independent of entropy s and is a function of (total) energy density only,
is independent of entropy s and is a function of (total) energy density only,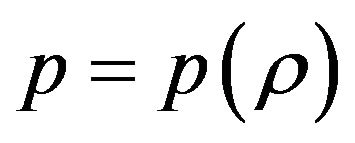 . In this case one can introduce a conserved mass density
. In this case one can introduce a conserved mass density  and an internal energy
and an internal energy 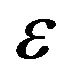 and derive the energy-momentum tensor from a Lagragian
and derive the energy-momentum tensor from a Lagragian 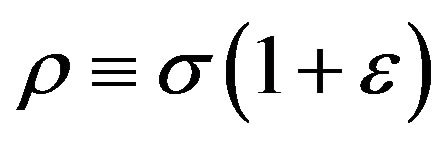 as in [77] or a Lagragian
as in [77] or a Lagragian 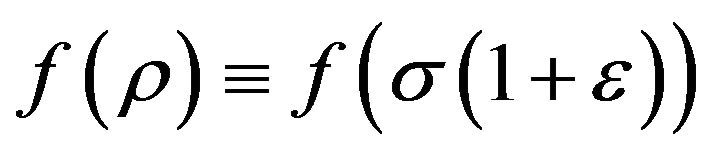 as here.
as here.
Equation (A.14) is equivalent to (2.2)-(2.4) of the main text. So we accomplish the first task in this appendix.
The second task we want to accomplish is to convert the energy-momentum tensor in [41] into the same form as in (A.13). We remark that in [41] symbol 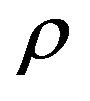 represents mass density (so it is equivalent to our
represents mass density (so it is equivalent to our  here).
here).
In our notation the energy-momentum tensor (in the absence of torsion) is given by (cf. (3.4h) of [41]):
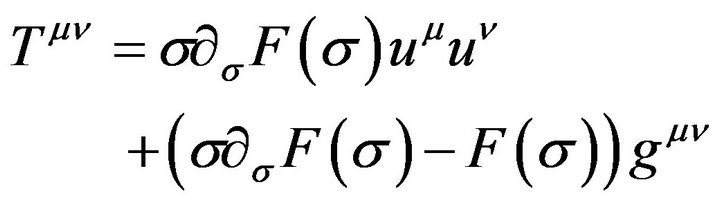 (A.15)
(A.15)
Notice that (A.15) looks different from (A.13) in the following ways: 1) the differentiation is wrt to mass density  [in (A.13), the differentiation is wrt to (total) energy density
[in (A.13), the differentiation is wrt to (total) energy density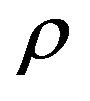 ]; 2) the pressure p does not appear explicitly [in (A.13), the pressure p does appear explicitly]. We remark that (A.15) is less suitable for practical calculation because pressure p does not appear explicitly.
]; 2) the pressure p does not appear explicitly [in (A.13), the pressure p does appear explicitly]. We remark that (A.15) is less suitable for practical calculation because pressure p does not appear explicitly.
In [41], 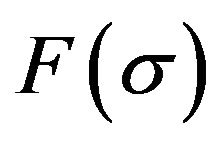 is eventually chosen as
is eventually chosen as 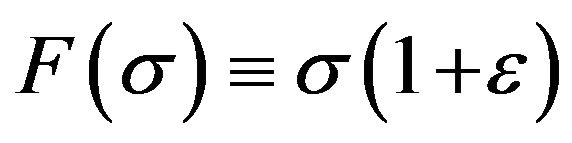 . In this appendix, we identifying
. In this appendix, we identifying 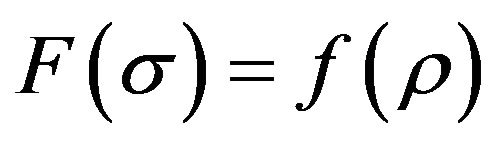 (remembering
(remembering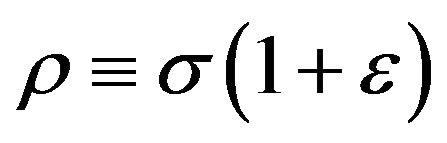 ). Using chain rule
). Using chain rule , and the pressure definition (A.12), we obtain:
, and the pressure definition (A.12), we obtain:
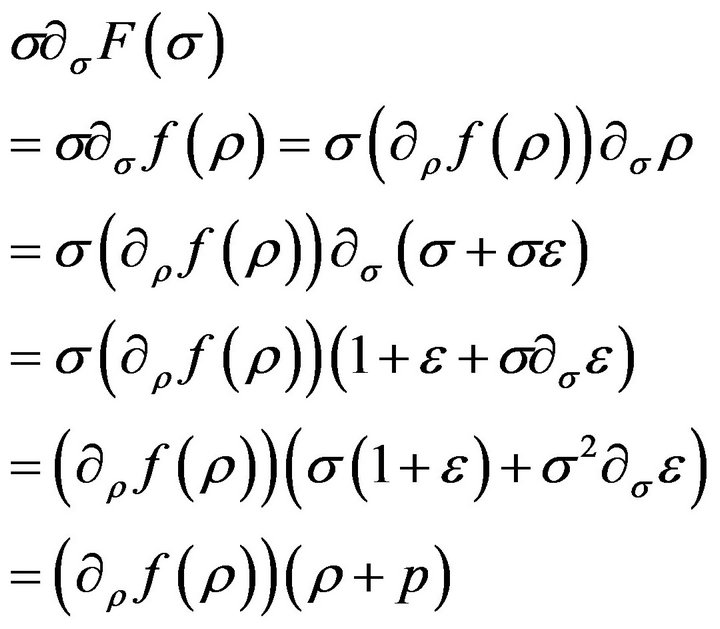 (A.16)
(A.16)
Substitution of (A.16) into (A.15), we find out that the result is identical to (A.13).
8.2. Appendex B. Conversion of an Elliptical Integral into Legendre Standard Form
The elliptical integral we want to convert, (4.4) and (4.5) with 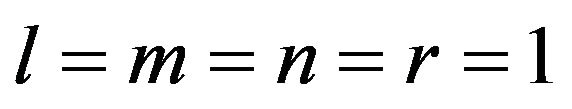 and
and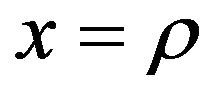 ,
, 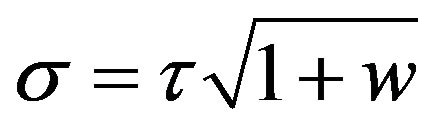 can be rewritten as:
can be rewritten as:
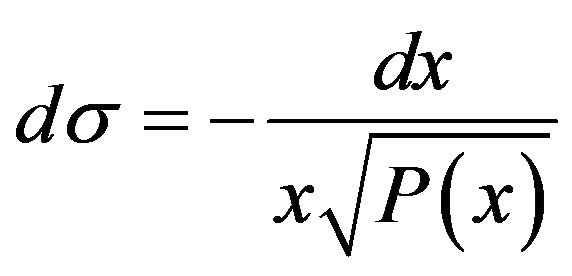 (B.1)
(B.1)
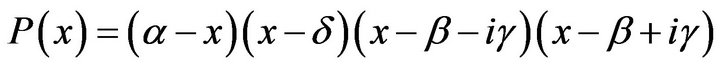 (B.2)
(B.2)
Following the standard procedures [78], we can convert (B.1) to:
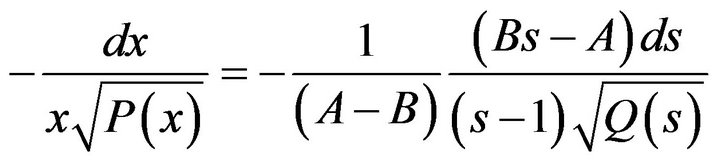 (B.3)
(B.3)
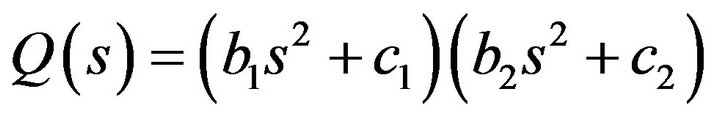 (B.4)
(B.4)
where
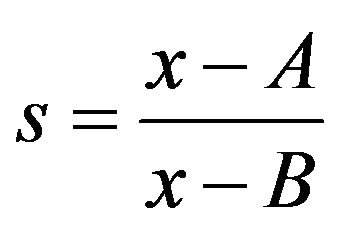 (B.5)
(B.5)
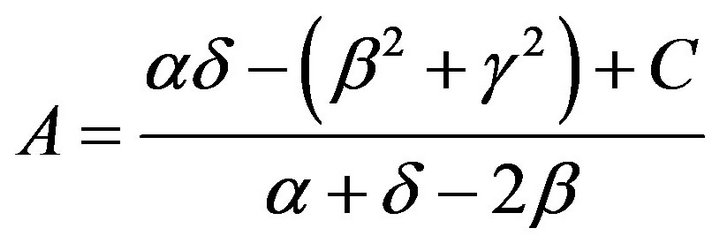 (B.6)
(B.6)
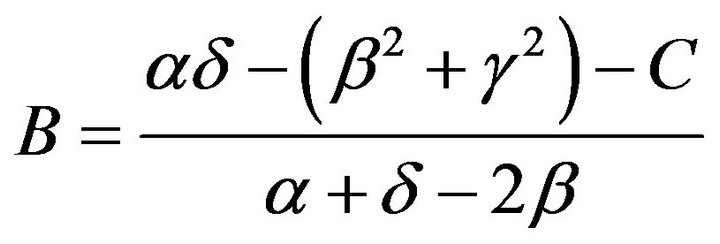 (B.7)
(B.7)
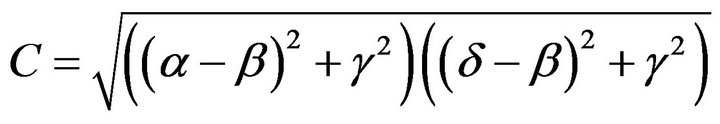 (B.8)
(B.8)
 (B.9)
(B.9)
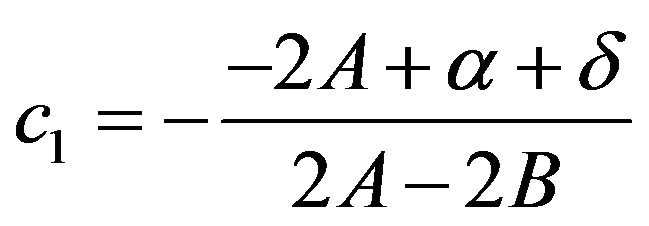 (B.10)
(B.10)
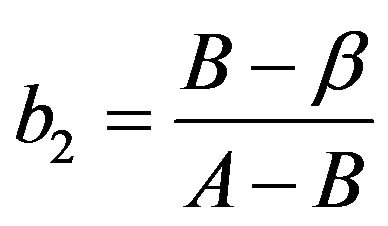 (B.11)
(B.11)
 (B.12)
(B.12)
We next multiply the numerator and denominator of RHS of (B.3) by 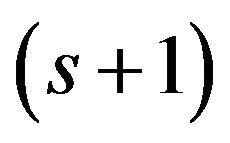 and obtain:
and obtain:
 (B.13)
(B.13)
For our case we have 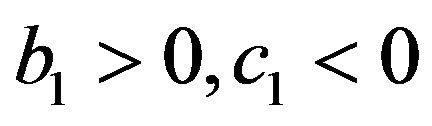
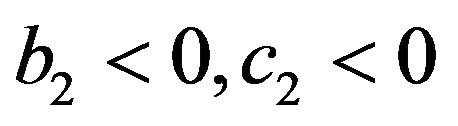 . So we can rewrite
. So we can rewrite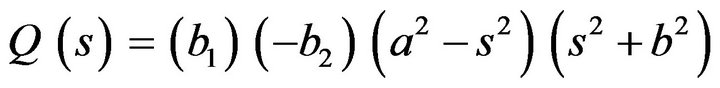 where
where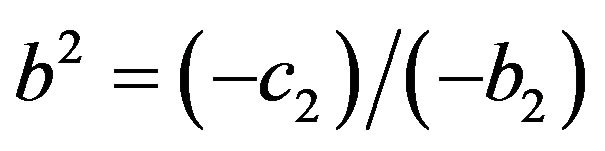 ,
, . This belongs to case (4) [73]:
. This belongs to case (4) [73]: . We now define
. We now define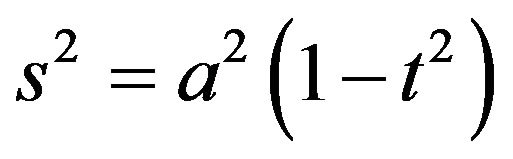 ,
,
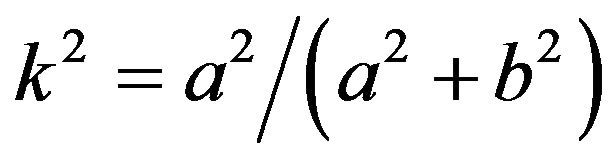 . The first term on the RHS of (B.13) becomes the elliptical integral of the first kind:
. The first term on the RHS of (B.13) becomes the elliptical integral of the first kind:
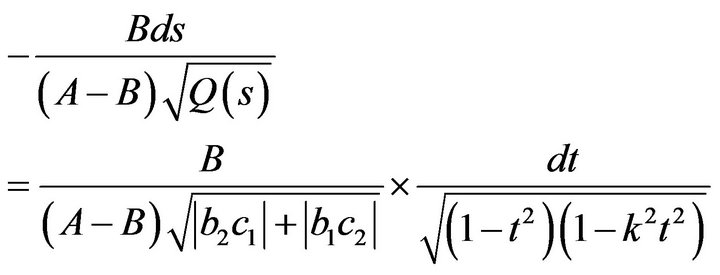 (B.14)
(B.14)
The second term on the RHS of (B.13) becomes the elliptical integral of the third kind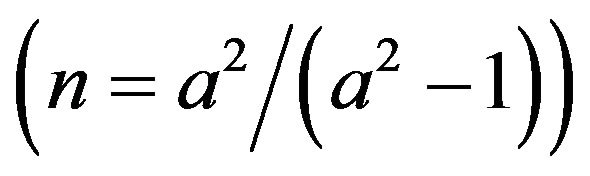 :
:
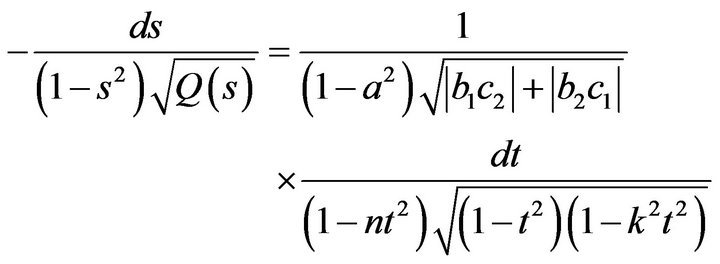 (B.15)
(B.15)
The third term on the RHS of (B.13) becomes the one of the elementary functions,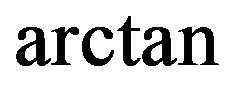 :
:
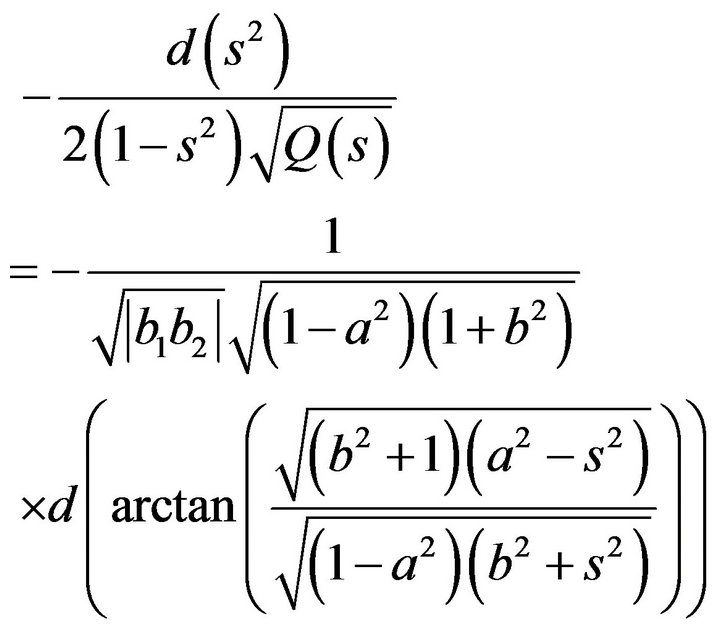 (B.16)
(B.16)
If , (B.3) will not apply. In this case we have
, (B.3) will not apply. In this case we have ,
,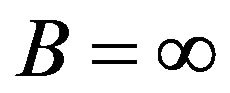 . We can define
. We can define
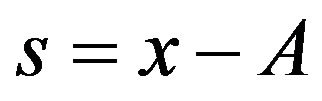 and obtain
and obtain  and (B.2) becomes
and (B.2) becomes
 , (B.17)
, (B.17)
Thus we converted (B.2) into (B.3) and (B.4). The rest of treatment is similar to (B.13) through (B.16).
NOTES
1Major ideas of this paper were conceived when Y. Shi was a postdoc researcher at University of California at Berkeley.

