Received 15 February 2015; accepted 5 March 2015; published 10 March 2015

ABSTRACT

We establish the conditions for the compute of the stability restriction and local accuracy on the time step and we prove the consistency and local truncation error by using 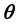-scheme and 3-level scheme for Heat Equation with smooth initial conditions and for some parameter.

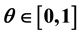Keywords:

Global Truncation, Local Accuracy, Stability Restriction

1. Introduction

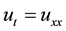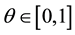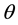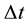In this paper we have considered the heat equation with. Using -scheme and 3-level scheme in space we compute the order of local accuracy in space and time and stability restriction as a function of on the time step. Much attention has been paid to the development, analysis and implementation of accurate methods for the numerical solution of this problem in the literature. Many problems are modeled by smooth initial conditions and Dirichlet boundary conditions. A number of procedures have been suggested (see, for instance [1] - [3] ). We can say that three classes of solution techniques have emerged for solution of PDE: the finite difference techniques, the finite element methods and the spectral techniques (see [4] and [5] ). The last one has the advantage of high accuracy attained by the resulting discretization for a given number of nodes [6] - [8] .

We consider Scheme (1) for the 1D heat equation for some parameter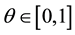. We compute the order of local accuracy in space and time as a function of 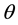and its the stability restriction. Until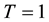, we compute the solution with some fixed 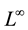error with the smallest amount of CPU time, and finally we can see this findings producing the relevant convergence and efficiency plot. For the 3-level scheme we consider (11) for the 1D heat equation and we compute the local truncation error. For different values of 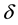and 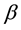we find the stability criterion of the scheme and its accuracy.

2. 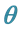-Scheme

Let

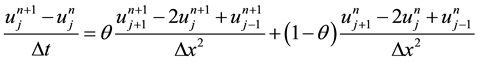(1)

be the -scheme applied to the one-dimensional heat equation

(2)

Now for the order of local accuracy in space and time as a function of we write the local truncation error. In time we have

where represents the exact solution of the heat equation. Now we perform Taylor expansion of at.

We can write the LHS of (1) as

(3)

(4)

here represents the derivative with respect to time, of. On the RHS, we have a centered difference approximating second derivative of

(5)

As we are solving the heat equation, the previous expression is

(6)

Now, at time we have

(7)

(8)

therefore, applying Taylor expansion with respect to we can write

So (8) becomes

(9)

Here RHS of (1) becomes

After the elimination of some terms we have

Now simplifying we obtain

Cancelling and moving all terms to the right side, we get

(10)

Scheme (10) is first order in time, second order in space. If for example, it becomes second order, this is due to cancellation of the.

Stability Restriction as a Function of

Here we will apply Von Neumann stability. Let and. Then Equation (1) can be written as

Now dividing by we have

Therefore by using and we can rewrite the expression as

By using the identity we have

We can say this scheme is stable only for. Now, let. The inequality is

thus

Now multiplying by the denominator we have

The expression in the absolute value becomes

Therefore by the Von Neumann stability condition, the scheme is stable if.

In this case we can say the following about the best combination for and. In order to have both local accuracy and stability, the optimal value of is and therefore this scheme represents the Crank-Ni- cholson scheme. Here and appear in the form.

In Figure 1 the convergence plot equation (varying the radio r) is

with matrix A described in the heat equation. We can say the scheme is unconditionally stable. We can see in Figure 1 that we have a linear convergence with respect to r.

3. Three-Level Scheme

We start by computing the stability restriction one has to impose on. We apply Von Neumannstability analysis to the scheme.

Let

(11)

where

(12)

and

(13)

By using (12) and (13) we can rewrite (11) as

(14)

or as

The local truncation error for this scheme is as follow.

where represents the exact solution of the heat equation. Therefore we have

(15)

Now expanding operator on the left side, we can isolate the forward difference in time at, then

however,

Expanded this expression becomes

Finally we have

4. Stability Criterion for the Three-Level Scheme and Its Accuracy When and

By using Equation (14) we have

Now applying Von Neumann stability again, the aim is to use and , therefore

Multiplying both sides by and write we obtained

Using the cosine identity that we have

We have a quadratic equation in, where, therefore

After some cancellations, we can write

Here, if all we need

Acknowledgements

We would like to thank the referee for his valuable suggestions that improved the presentation of this paper.

References