Journal of Biophysical Chemistry
Vol.5 No.3(2014), Article
ID:47924,7
pages
DOI:10.4236/jbpc.2014.53013
Acid Dissociation Constants and Related Thermodynamic Functions of Protonated 2,2-Bis(Hydroxymethyl)-2,2’,2”- Nitrilotriethanol (BIS-TRIS) from (278.15 to 328.15) K
Rabindra N. Roy, Lakshmi N. Roy, Katherine E. Hundley, John J. Dinga, Mathew R. Medcalf, Lucas S. Tebbe, Ryan R. Parmar, Jaime A. Veliz
Hoffman Department of Chemistry, Drury University, Springfield, USA
Email: rroy@drury.edu
Copyright © 2014 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 14 May 2014; revised 12 June 2014; accepted 11 July 2014
ABSTRACT
Thermodynamic dissociation constants pKa of 2,2-bis(hydroxymethyl)-2,2’,2”-nitrilotriethanol have been determined at 12 temperatures from (278.15 to 328.15) K including the body temperature 310.15 K by the electromotive-force measurements (emf) of hydrogen-silver chloride cells without liquid junction of the type: Pt(s), H2(g), 101.325 kPa|BIS-TRIS (m) + BIS-TRIS·HCl (m)| AgCl(s), Ag(s), where m denotes molality. The pKa values for the dissociation process of BISTRIS·H++ H2O = H3O+ + BIS-TRIS given as a function of T in Kelvin (K) by the equation pKa = 921.66 (K/T) + 14.0007 − 1.86197 ln(T/K). At 298.15 and 310.15 K, the values of pKa for BIS-TRIS were found to be 6.4828 ± 0.0005 and 6.2906 ± 0.0006 respectively. Thus buffer solutions composed of BIS-TRIS and its hydrochloride would be useful as secondary pH buffer standards and for control of acidity in the pH range 6 to 8. At 298.15 K the thermodynamic functions ∆G˚, ∆H˚, ∆S˚ and ∆Cp˚ for the dissociation process of BIS-TRIS·H+ are ∆G˚ = 37,005 J·mol−1, ∆H˚ = 28,273 J·mol−1, ∆S˚ = 29.3 J·K−1·mol−1 and ∆Cp˚ = 36 J·K−1·mol−1. These results are compared with the dissociation of protonated bases structurally related to BIS-TRIS·H+.
Keywords:Dissociation Constant, pH, Buffer, emf, Thermodynamic Function, Ionic Strength

1. Introduction
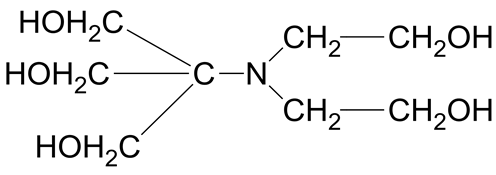
Many biochemical reactions that normally occur in living systems are highly sensitive to pH. For example, some enzyme catalysts involved in important biochemical reactions become very significant and effective with a narrow range of pH [1] . The phosphate buffer has been widely used as a biological pH standard, but it is not an ideal pH standard in the pH range 6 to 8 for physiological application [2] -[5] . The buffer substances recommended by Good et al. [6] have proved useful for clinical pH measurements in the range 7 to 9. These compounds, mostly protonated amines or N-substituted amino acids, are compatible with many media including isotonic saline solution of physiological importance. BIS-TRIS is a free base. The nitrogen atom is protonated to form BIS-TRIS∙H+ which dissociated to BIS-TRIS + H+. The dissociation constant is abbreviated pKa. The biological buffers listed in the text have pK1 and pK2 values. However, only pK2 values are mentioned because these values lie in the physiological pH range, whereas the pK1 values do not. In the present study BIS-TRIS has only one pK1 designated as pKa, whose value does lie in the physiological pH range of interest. In previous work from the authors laboratory and by other investigators, the pKa values and associated thermodynamic functions of N-substituted glycine including N-tris(hydroxymethyl)methylglycine (TRICINE) [7] and N,N-bis(2-hydroxyethyl)glycine [8] have been reported. Other substances that have been studied include tris(hydroxymethyl)- aminomnethane (TRIS or THAM) [9] , and 2-ammonium-2-methyl-1,3-propanediol (AMP) [10] . The pKa values of N, N-bis(2-hydroxyethyl) known as BIS-TRIS and a derivative of (TRIS) have been determined from (273.15 to 323.15) K by Paabo and Bates [11] . At 298.15 K, its pKa value is 6.483. Thus it has a great potential to be a useful buffer standard for pH control in the pH region of 6 to 8 close to that of blood serum. In order to provide very accurate, reliable and reproducible pH data in isotonic saline solutions we have now undertaken to study in detail the acid-base and thermodynamic properties of protonated (BIS-TRIS) at 12 temperatures from (278.15 to 328.15) K at 5˚ intervals. The free base (BIS-TRIS) has the following structure.
The precise electromotive force (emf) measurements were made using hydrogen and silver-silver chloride electrodes without liquid junction of the type:
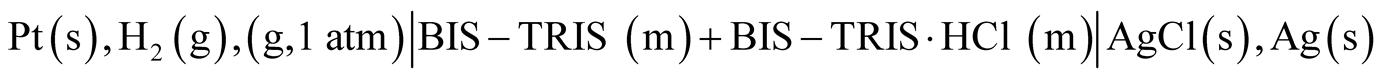 (A)
(A)
For all 16 buffer solutions, the molalities of (BIS-TRIS) and (BIS-TRIS·HCl) were of equal molality (m). It is to be noted that the solubility of the silver chloride in the presence of [BIS-TRIS] base to form silver complex ion is negligible [12] , hence no molality correction is necessary for the chloride ion.
2. Experimental
The commercial sample of (BIS-TRIS) obtained from Research Organics (Cleveland, Ohio) was used without further purification. It was 99% pure. Preliminary measurements gave emf data within ±0.04 mV for the purified and unpurified sample. Thus the unpurified material was used as received and was always dried and stored in a desiccator. Hydrochloric acid was prepared from the constant boiling twice distilled 6 M acid. The middle fraction of the distillate was used. The concentration of the hydrochloric acid solution was determined gravimetrically by weighing as AgCl from the precipitation with AgNO3. All sixteen different buffer concentrations were prepared by adding a standard solution of hydrochloric acid to a solution of (BIS-TRIS) base in such amount as to produce equal molalities of the base and its hydrochloride. The molality range was 0.009 to 0.08 mol·kg−1. The buffer ratio of (BIS-TRIS) and [BIS-TRIS·HCl] was 1 and the pH of the buffer solution was very close to neutral. Thus hydrolysis correction of the basic species to m was unnecessary. Vacuum corrections were applied to all these masses.
3. Methods and Results
In previous communications [7] [13] [14] the preparation of the hydrogen and silver-silver chloride electrode (thermal electrolytic type), cell design, preparation of cell solutions followed usual procedure of the author’s laboratory. The cell voltage (emf) of the cell (A) for 16 solutions from (278.15 to 328.15) K corrected to 1 atm hydrogen partial pressure, is given in Table1 At 298.15 K, agreement of the emf readings among the initial, middle and sometimes final (at the end of the temperature series) on the average were 0.04 mV. The thermodynamic dissociation constant of pKa for cell (A) is expressed as:
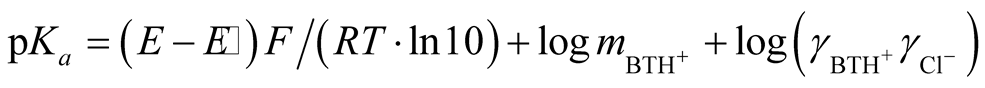 (1)
(1)
Table 1. Electromotive force (emf) of Cell A (in volts): Pt(s); H2(g), 1 atm|BIS-TRIS (m)+ BIS-TRIS∙HCl(m)|AgCl(s), Ag(s).
aUnits of m, mol·kg−1.
The “apparent” thermodynamic constant 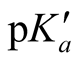 is rearranged from Equation (2).
is rearranged from Equation (2).
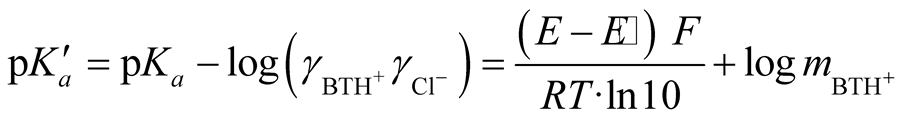 (2)
(2)
where BTH+ stands for BIS-TRIS·H+, m is the molality of BIS-TRIS·H+, pKa is the thermodynamic dissociation constant, γ is the activity coefficient of the corresponding species, E is the corrected emf to 1 atm, E˚ is the standard electrode potential of silver-silver chloride, R, T, F have their usual physical significances. Since hydrolysis of BIS-TRIS·H+ is negligible at the almost neutral pH of these buffer solutions, so only the stoichiometric molality m appears in Equation (1).
The term 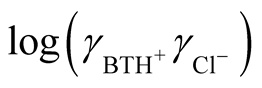 is expected to vary linearly with I (ionic strength) because of the charge type.
is expected to vary linearly with I (ionic strength) because of the charge type.
Hence, the quantity 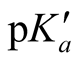 is defined by:
is defined by:
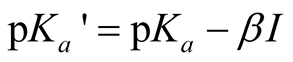 (3)
(3)
and the ionic strength is given by
 (4)
(4)
The values of pKa (the intercept at I = 0), β (the slope parameter) were found by the least-squares methods and are entered in Table 2 together with the standard deviation in the temperature range (278.15 to 328.15) K which were fitted to the following equation
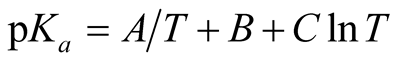 (5)
(5)
by the method proposed by Ives and Moseley [15] . The final Equation (6) takes the form:
 (6)
(6)
with a residual standard deviation of 0.0004. Quantities for the dissociation of BIS-TRIS·H+ were computed by applying the usual thermodynamic equations from the constants of Equation (6). The values of ∆G˚, ∆H˚, ∆S˚ and ∆Cp˚ along with standard deviations were calculated by the method of Please [16] , and are summarized in Table3 At 298.15 K, the estimated uncertainties of these values are; ∆G˚, 3 J∙mol−1; ∆H˚, 22 J∙mol−1; ∆S˚, 0.07 J∙K−1∙mol−1; and ∆Cp˚, 2 J∙K−1∙mol−1.
Table 2. Dissociation constant pKa of BIS-TRIS from (278.15 to 328.15) K.
aStandard deviation of pKa. bSlope parameter.
4. Discussion
There are some reliable thermodynamic data with which to compare the present work. The pKa and standard thermodynamic functions of BIS-TRIS·H+ are compared in Table 4 with the corresponding data for other compounds such as (CH3)3CN+H3 [17] and CH3(CH2OH)2CN+H3 [10] [18] which are structurally related to BIS-TRIS·H+ (this work). For the interpretation of the data given in Table 4, Timimi and Everett [18] , in their publication indicated that for isoelectric dissociation process (positively charged acid) hydroxymethyl or hydroxyethyl substitution to primary, secondary, or tertiary amine compounds usually lowers the values of pKa, ∆G˚ and ∆H˚. This trend is consistent in the present work. For example at 298.15 K, the value of pKa for 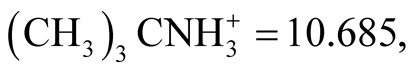 ∆H˚ = 60,070 J∙mol−1 compared to pKa = 6.483, ∆H˚ = 28,273 J∙mol−1 for BISTRIS·H+. This enhancement of the acidic strength is through the inductive effect of the oxygen atoms. The dissociation of BIS-TIS·H+ is an isoelectric process where no new charge is created. It appears that somewhat bulky hydrophilic substituent groups partially shield the basic nitrogen. Hence the interactions of the substituent groups with hydrogen ions become a problem. This is also consistent with N substitution. It is evident from Table 4 that the values of ∆S˚ and ∆Cp˚ for
∆H˚ = 60,070 J∙mol−1 compared to pKa = 6.483, ∆H˚ = 28,273 J∙mol−1 for BISTRIS·H+. This enhancement of the acidic strength is through the inductive effect of the oxygen atoms. The dissociation of BIS-TIS·H+ is an isoelectric process where no new charge is created. It appears that somewhat bulky hydrophilic substituent groups partially shield the basic nitrogen. Hence the interactions of the substituent groups with hydrogen ions become a problem. This is also consistent with N substitution. It is evident from Table 4 that the values of ∆S˚ and ∆Cp˚ for 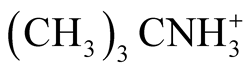 [17] are small (−3.1 J∙K−1∙mol−1 and ∆Cp˚ = 15 J∙K−1∙mol−1), but when substitution of three hydroxymethylgroups in -(CH3)3 occur, as in the case of (CH2OH)3CN+H3 [19] ∆S˚ = 4.8 J∙K−1∙mol−1 and ∆Cp˚ = −78 J∙K−1∙mol−1 which is substantial. This data suggests a decreased ordering in terms of the destabilization of the solvent structure through hydroxyl groups. This contrary variation of negative value of ∆S˚ and positive value of ∆Cp˚ is difficult to explain quantitatively. Usually electrostatic interactions with charged species cause an orientation of polar solvent molecules in the proximity of the ion. This leads to lower both ∆S˚ and ∆Cp˚ of the system. However, the present thought is that other hydrophobic interactions between polar water structure and -(CH3)3 groups play an important role for a decrease in ∆S˚ and an increase in
[17] are small (−3.1 J∙K−1∙mol−1 and ∆Cp˚ = 15 J∙K−1∙mol−1), but when substitution of three hydroxymethylgroups in -(CH3)3 occur, as in the case of (CH2OH)3CN+H3 [19] ∆S˚ = 4.8 J∙K−1∙mol−1 and ∆Cp˚ = −78 J∙K−1∙mol−1 which is substantial. This data suggests a decreased ordering in terms of the destabilization of the solvent structure through hydroxyl groups. This contrary variation of negative value of ∆S˚ and positive value of ∆Cp˚ is difficult to explain quantitatively. Usually electrostatic interactions with charged species cause an orientation of polar solvent molecules in the proximity of the ion. This leads to lower both ∆S˚ and ∆Cp˚ of the system. However, the present thought is that other hydrophobic interactions between polar water structure and -(CH3)3 groups play an important role for a decrease in ∆S˚ and an increase in
Table 3 . Thermodynamic functions for the dissociation of protonated BIS-TIS from (278.15 to 328.15) K.
Units: ∆G˚, ∆H˚, J∙mol−1; ∆S˚, ∆Cp˚, J∙K−1∙mol−1.
Table 4. Comparison of the thermodynamic functions for the dissociation of protonated BISTRIS and related protonated bases at 298.15 K.
Units: ∆H˚, J∙mol−1; ∆S˚, ∆Cp˚, J∙K−1∙mol−1.
∆Cp˚ [20] . As can be seen from Table 4, comparisons of the thermodynamic properties of (CH2CH2OH)3NH+ [21] ,  [10] , and BIS-TRIS [this work] reveal the increase in acidic strength due to the inductive effect in the presence of more oxygen atoms.
[10] , and BIS-TRIS [this work] reveal the increase in acidic strength due to the inductive effect in the presence of more oxygen atoms.
5. Conclusion
The emf data are stable, reliable, and very accurate with an uncertainty of ±0.04 mV. The pKa value of BISTRIS·H+ is 6.483 at 298.15 K. Hence this is of great importance in the preparation of a buffer solution composed of BIS-TRIS and BIS-TRIS·HCl for the control of acidity in the physiological pH range 6 - 8. These pH results will be published in a separate communication. The detailed procedure will be followed as reported in recent publications for (TAPSO) [22] and (ACES) [23] .
Acknowledgements
The authors are grateful for the funding from the National Institutes of Health (NIH-AREA) under the grant 2R15GM66866-3. The content of this paper is the sole responsibility of the authors and does not necessarily represent the official views of the NIH of the National Institutes of the General Medical Science. Rabindra N. Roy is indebted to the Hoffman Research Fund.
References
- Bates, R.G., Roy, R.N. and Robinson, R.A. (1973) Buffer Standards of Tris(Hydroxymethyl)Methylglycine (“Tricine”) for the Physiological Range pH 7.2 to 8.5. Analytical Chemistry, 45, 1663-1666. http://dx.doi.org/10.1021/ac60331a022
- Wu, Y.C., Berezansky, P.A., Feng, D. and Koch, W.F. (1993) Second Dissociation Constant of 3-(N-Morpholino)-2- Hydroxypropanesulfonic Acid and pH of Its Buffer Solutions. Analytical Chemistry, 65, 1084-1087. http://dx.doi.org/10.1021/ac00056a023
- Durst, R.A. and Staples, B.R. (1972) Itrs/Tris HCl: A Standard Buffer for Use in the Physiological pH Range. Clinical Chemistry, 18, 206-208.
- Roy, R.N., Roy, L.N., Le Noue, S.R., Denton, C.E., Simon, A.N., Richards, S.J., et al. (2006) Thermodynamic Constants of N-[Tris(Hydroxymethyl)Methyl-3-Amino]Propanesulfonic Acid (TAPS) from the Temperatures 278.15 K to 328.15 K. Journal of Chemical Thermodynamics, 38, 413-417. http://dx.doi.org/10.1016/j.jct.2005.06.009
- Bates, R.G., Vega, C.A. and White, D.R. (1978) Standards for pH Measurements in Isotonic Saline Media of Ionic Strength I = 0.16. Analytical Chemistry, 50, 1295-1300. http://dx.doi.org/10.1021/ac50031a026
- Bates, N.E., Winget, G.D., Winter, W., et al. (1966) Hydrogen Ion Buffers for Biological Research. Biochemistry, 5, 467-477. http://dx.doi.org/10.1021/bi00866a011
- Roy, R.N., Robinson, R.A. and Bates, R.G. (1973) Thermodynamics of the Two Dissociation Steps of N-Tris(Hydroxymethyl)Methylglycine (“Tricine”) in Water from 5 to 50°. Journal of the American Chemical Society, 95, 8231-8235. http://dx.doi.org/10.1021/ja00806a004
- Roy, L.N., Roy, R.N., Denton, C.E., LeNoue, S.R., Roy, C.N., et al. (2006) Second Dissociation Constant of Bis- [(2-Hydroxyethyl)Amino]Acetic Acid (BICINE) and pH of Its Buffer Solutions from 5 to 55°C. Journal of Solution Chemistry, 35, 605-624. http://dx.doi.org/10.1007/s10953-005-9009-6
- Hetzer, H.B. and Bates, R.G. (1961) Dissociation Constant of the Protonated Acid Form of 2-Amino-2-(Hydroxymethyl)-1,3-Propanediol [Tris-Hydroxymethyl)-Aminomethane] and Related Thermodynamic Quantities from 0 to 50°. Journal of Physical Chemistry, 65, 667-671. http://dx.doi.org/10.1021/j100822a017
- Bates, R.G. and Hetzer, H.B. (1962) Dissociation Constant 2-Ammonium-2-Methyl-1,3-Propanediol in Water from 0 to 50 and Related Thermodynamic Quantities. Journal of Physical Chemistry, 66, 308-311. http://dx.doi.org/10.1021/j100808a027
- Paabo, M. and Bates, R.G. (1970) Dissociation Constant of Protonated 2,2-Bis(Hydroxymwthyl)-2,2’,2”-Nitrilotriethanol (Bis-Tris) and Related Thermodynamic Functions from 0 to 50°. Journal of Physical Chemistry, 74, 702-705. http://dx.doi.org/10.1021/j100699a003
- Bates, R.G. and Pinching, G.D. (1949) Acidic Dissociation Constant of Ammonium Ion at 0 to 50°C and the Base Strength of Ammonia. Journal of Research of the National Bureau of Standards, 42, 419-430. http://dx.doi.org/10.6028/jres.042.037
- Roy, R.N., Moore, C.P., Carlsten, J.A., Good, W.S., Harris, P., Rook, J.M., Roy, L.N. and Kuhler, K.M. (1997) Second Dissociation Constants of Two Substituted Aminoethansulfonic Acids (MES) and (TES) in Water from 5 to 55°C. Journal of Solution Chemistry, 26, 1209-1216. http://dx.doi.org/10.1023/A:1022937324983
- Bates, R.G. (1973) Determination of pH. Wiley, New York, Chapter 10.
- Ives, J.G. and Moseley, P.G.N. (1975) Derivation of Thermodynamic Functions of Ionization from Acidic Dissociation Constants. Journal Chemical Society Faraday Transactions I, 72, 1132-1143. http://dx.doi.org/10.1039/f19767201132
- Please, N.W. (1954) Estimation of the Variances of the Data Used in the Calculation of Dissociation Constants. Biochemistry Journal, 56, 196-201.
- Hetzer, H.B., Robinson, R.A. and Bates, R.G. (1962) Dissociation Constant of t-Butylammonium Ion and Related Thermodynamic Quantities from 5 to 35°C. Journal of Physical Chemistry, 66, 2696-2698. http://dx.doi.org/10.1021/j100818a080
- Timimi, B.A. and Everett, D.H. (1968) The Thermodynamics of the Acid Dissociation of Some Amino-Alcohols in Water. Journal Chemical Society B, 1380-1386. http://dx.doi.org/10.1039/j29680001380
- Datta, S.P., Grzybowski, A.K. and Weston, B.A. (1963) The Acid Dissociation Constant of the Protonated Form of Tri(Hydroxymethyl)Methylamine. Journal Chemical Society, 792-796. http://dx.doi.org/10.1039/jr9630000792
- Cox, M.C., Everett, D.H., Landsmen, D.A. and Munn, R.J. (1968) The Thermodynamics of the Acid Dissociation of Some Alkylammonium Ions in Water. Journal Chemical Society B, 1373-1379. http://dx.doi.org/10.1039/j29680001373
- Bates, R.G. and Allen, G.F. (1960) Acid Dissociation Constant and Related Thermodynamic Quantities for Triethanolammonium Ion in Water from 0 to 50°C. Journal of Research of the National Bureau of Standards, 64A, 343-346. http://dx.doi.org/10.6028/jres.064A.033
- Roy, L.N., Roy, R.N., Bodendorfer, B., Downs, Z., et al. (2011) Buffer Standards for the Physiological pH of the Zwitterionic Buffer 3-[N-Tris(Hydroxymethyl)Methylamino]-2-Hydroxypropanesulfonic Acid (TAPSO) from (278.15 to 328.15) K. Journal of Biophysical Chemistry, 2, 414-421. http://dx.doi.org/10.4236/jbpc.2011.24048
- Roy, L.N., Roy, R.N., Downs, Z.M., Bodendorfer, B.M., Veliz, J.A., Stegner, J.M., Henson, I.B. and Wollen, J.T. (2011) Buffer Standards for Physiological pH of the Buffer N-(2-Acetamido)-2-Aminoethanesulfonic Acid from (278.15 - 328.15) K. Open Journal of Physical Chemistry, 1, 118-123. http://dx.doi.org/10.4236/ojpc.2011.13016


