Received 20 May 2016; accepted 14 August 2016; published 17 August 2016

ABSTRACT

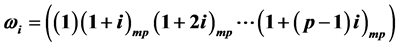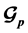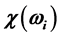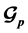Representation theory is concerned with the ways of explaining or visualizing a group as a group of matrices. In this paper, we extend the permutation pattern of to a two-line notation. We consider the representations of this G₁ non-deranged permutation group (p ≥ 5 and p a prime). Also we reveal some interesting properties and results of the character of where.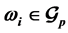

Keywords:

Representation, Non-Deranged, Permutation Group, -Permutation Group, 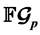-Module, Character

1. Introduction

The beauty of Group as a topic is the various properties that can arise from its studies. Its interesting nature has encouraged various studies in this field over the years. For instance, for every n a positive integer, the set of all permutations of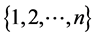, under the product operation of composition is a group. This group is known as a symmetric group (Permutation group) of degree n. According to [1] , the study of the symmetric group by Georg Frobenius in 1903, opened the door to the various works that was further developed by many mathematicians, including Percy MacMahon, W. V. D. Hodge, G. de B. Robinson, Gian-Carlo Rota, Alain Lascoux, Marcel-Paul Schtzenberger and Richard P.

Conscious efforts by different researchers over the years led to the discovery of other form of permutation patterns, groups and their subsequent representations; [2] shows how functions acting on a finite set can be con- veniently expressed using matrices, whereby the composition of functions corresponds to multiplication of the matrices. Essentially, they considered the induced action on the vector space with the elements of the set acting as a basis. This action extends to tensor powers of the vector space and can be extended also to symmetric powers, antisymmetric powers, etc., that yielded representations of the multiplicative semi-group of functions and representations of permutation groups.

To be precise, [3] described a representation as a homomorphism from G into a group of invertible matrices. [4] described a representation as an (linear) action of a group or Lie algebra on a vector space. (Say, for every there is an associated operator, which acts on the vector space V.) In fact, 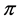is a representation of G acting on the space V. Most of the informations contained in the representation of a group can be distilled into one simple statistic, the trace of the corresponding matrices; [5] .

Over the years, deranged permutation, a permutation with no fix points has been studied with various results established. One of the many works in this field is the group theoretical interpretation of Bara’at Model by [6] to establish a deranged permutation pattern. The theoretic and topological properties have also been studied and established by [7] . More recently is the use of Catalan numbers by [8] to develop the scheme for prime numbers and which generate the cycles of permutation patterns using to determine the arrangements.

This permutation pattern was further worked upon by [9] to establish a permutation group. This was achieved by embedding an identity element 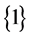in the collection of. Furthermore on the discovery of the special permutation group, several other works have been done to show some interesting results and properties of. Some of these works include [10] a paper that studied the Algebraic properties of the (132)―avoiding class of this permutation pattern and its applications to graph. The comparison of the group permutation pattern and generalized permutation patterns using Wilf-equivalence has also been studied by [11] .

Besides, as established by [12] , that not every transitive group contains a derangement. Hence we will in this paper, take a lead from the representations of symmetry groups as shown by [13] [14] and [15] to show the representations of Γ₁ non-deranged permutation group; this will be achieved by extending the work of [8] , to a two-line notation; we will also introduce another identity element for this Γ₁ non-deranged permutation group while we study some other results as it relates to representations of groups.

2. Notation

In an attempt to simplify this paper, basic concepts and notation as related to the work are defined below.

Definition 2.1:

Γ₁-non deranged permutation group is a special permutation group with a fixed element on the first column from the left.

Definition 2.2:

A permutation of a set X is a bijective function. It is a quantity or function that carries n indices or variables (where each can run from). For instance, Let be a non-empty ordered set such that. Let be a subgroup of symmetry group, such that every is generated by arbitrary set for any prime using the following

(1)

Lemma 2.3:

The order of the group p, a prime is ().

Proof. We recall that Langrange’s theorem says that order of the group is divisible by the order of the subgroup. If then

where q is a positive integer. We claim that is a factor of hence is the order of □

Example 2.3.1:

For p = 5 Equation (1) will generate a Γ₁ permutation group

and written in cycle form,

Definition 2.4:

A representation of G over is a homomorphism from G to, for some n. The degree of the is the integer n. Thus if is a function from G to, then is a representation if and only if for all: Since a representation is a homomorphism, it follows that for every representation, we have

1)

2)

for all, where denotes the identity matrix.

Definition 2.5:

Let G be a subgroup of, so that G is a group of permutations of. Let V be a n-dimensional vector space over, with bases for each i with and each permutation define for all i, and all is called the permutation module for G over F. We call the natural basis of V.

Definition 2.6:

Two-line notation is a notation used to describe a permutation on a (usually finite) set. For a finite set sup- pose S is a finite set and is a permutation. The two-line notation for is a description of in two aligned rows. The top row lists the elements of S, and the bottom row lists, under each element of S, its image under.

If, the two-line notation for is:

Definition 2.7:

Consider a finite set S and an ordering of the elements of S, with the elements (in order), given as. For a permutation of S, the one-line notation for is the string. The one-line notation for a permutation is a compressed form for the two-line notation where the first line is omitted because it is implicitly understood.

3. Representation of G_p

In considering -non deranged permutation group, and its representation. Let be a group and let be or and let denotes the group of invertible matrices with entries in.

A representation of over is a homomorphism from to for some p. The degree of is the prime p. Thus if is a function from to, then is representation if and only if for all. Since a representation is a homomorphism, it follows that for every representation, we have and for all where denotes the identity matrix

3.1. G_p as FG_p-Module

We need to introduce the concept of an module, and show that there is a close connection between the module and the representation of this -non deranged permutation group over.

Let be a group and let be. suppose that is representation of write, the vector space of all row vectors with for all and, the matrix product of the row vector V with the matrix is a row vector in V. (since the product of a matrix with an matrix is again matrix).

3.2. Proposition

Let V be a vector space over and let be a group then V is an -module if a multiplication (,) is defined satisfying the following conditions for all and

1)

2)

3)

4)

5)

Proof:

1) Let such that. Then

which implies that and. Therefore

2) Let and and are given as and, then

3) Let and

4) Let then

5) Let and and given that then

□

3.3. Corollary

Let be a subgroup of (; p a prime) so that is a -permutation group of. Let be a representation define as (where), for all and V a p-dimensional vector space over, with bases for each. Then is a permutation module over F with natural bases of V.

Example

Let and let B denote the basis v₁, v₂, v₃, v₄, and v₅ of V. If then

And if then

We have

and

3.4. Character of a Representation

Suppose that is a representation of a finite group G. With each matrix, we associate the complex number given by adding all the diagonal entries of the matrix, and call this number. The function is called the character of the representation.

Suppose that V is an CG-module with basis. Then the character of V is the function define by

.

Naturally enough, we define the character of a representation to be the character of the corresponding CG-module namely

.

Similarly, suppose that V is an -module with basis β. Then the character of V is the function define by

Naturally enough, we can also define the character of our representation to be the character of the corresponding -module namely

3.5. Theorem

Let be a -non deranged permutation group, (p = prime and p ≥ 5), the character of is never zero.

Proof:

To prove that, then it’s sufficient enough if we can show at least one diagonal ele-

ment of since

Suppose that and recall that is defined as

Therefore the character of every is at least 1. □

3.6. Corollary

Every (where) has a trivial character.

3.7. Theorem

Let be a permutation group, (p = prime and p ≥ 5) and, then the character of is

Proof:

From Corollary 3.6 above the first part of the proof is obvious, for the second part.

Let be a subgroup of, so that is a group of permutations of. Let V be a p-dimen- sional vector space over, with basis from 2.1 it implies that

then for all p ≥ 5, the representation will be

Applying the definition 2.2 and corollary 3.5, then taking the summation of the diagonal elements will give p as the character. □

4. Conclusion

This paper has extended the one line permutation pattern of Abor and Ibrahim (2010) to a two-line notation and hence is a -non deranged permutation group with a natural identity. The representation of as a finite permutation group was done and the character of was computed to be 1 if otherwise p.

Cite this paper

Ibrahim A. Aminu,Ejima Ojonugwa,Kazeem O. Aremu, (2016) On the Representations of Γ1-Nonderanged Permutation Group g_p. Advances in Pure Mathematics,06,608-614. doi: 10.4236/apm.2016.69049

References